Numerical Study on Auxiliary Propulsion Performance of Foldable Three-Element Wingsail Utilizing Wind Energy
Abstract
1. Introduction
2. Model and Methods
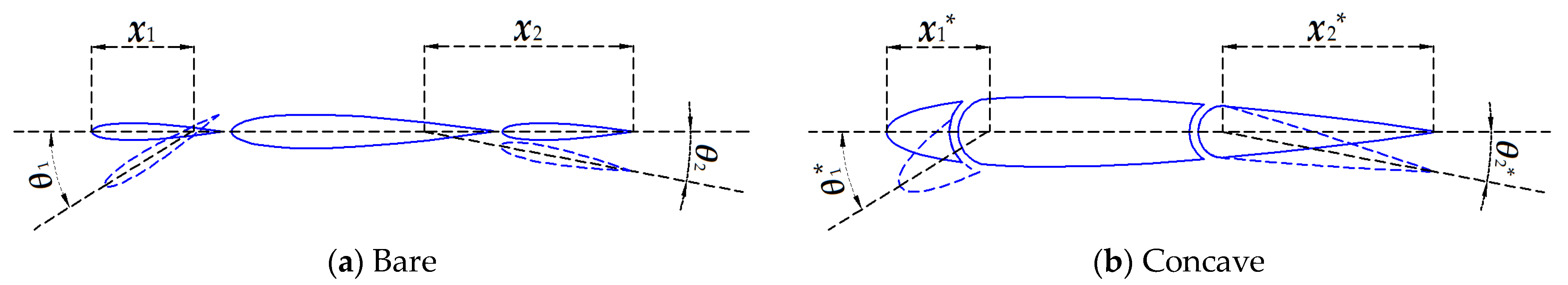
2.1. Geometry
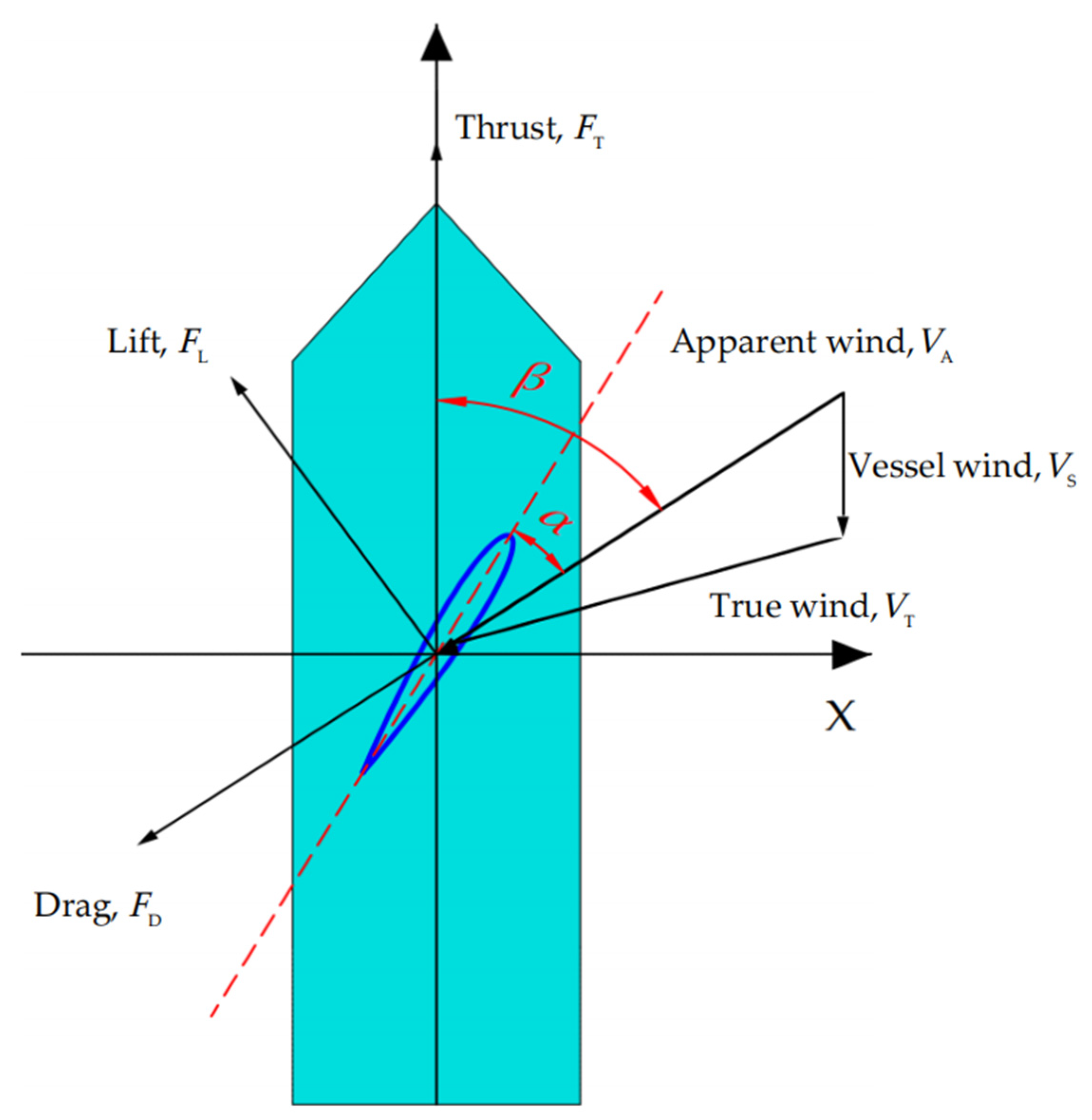
2.2. Force Analysis of Wingsail
2.3. Numerical Setup
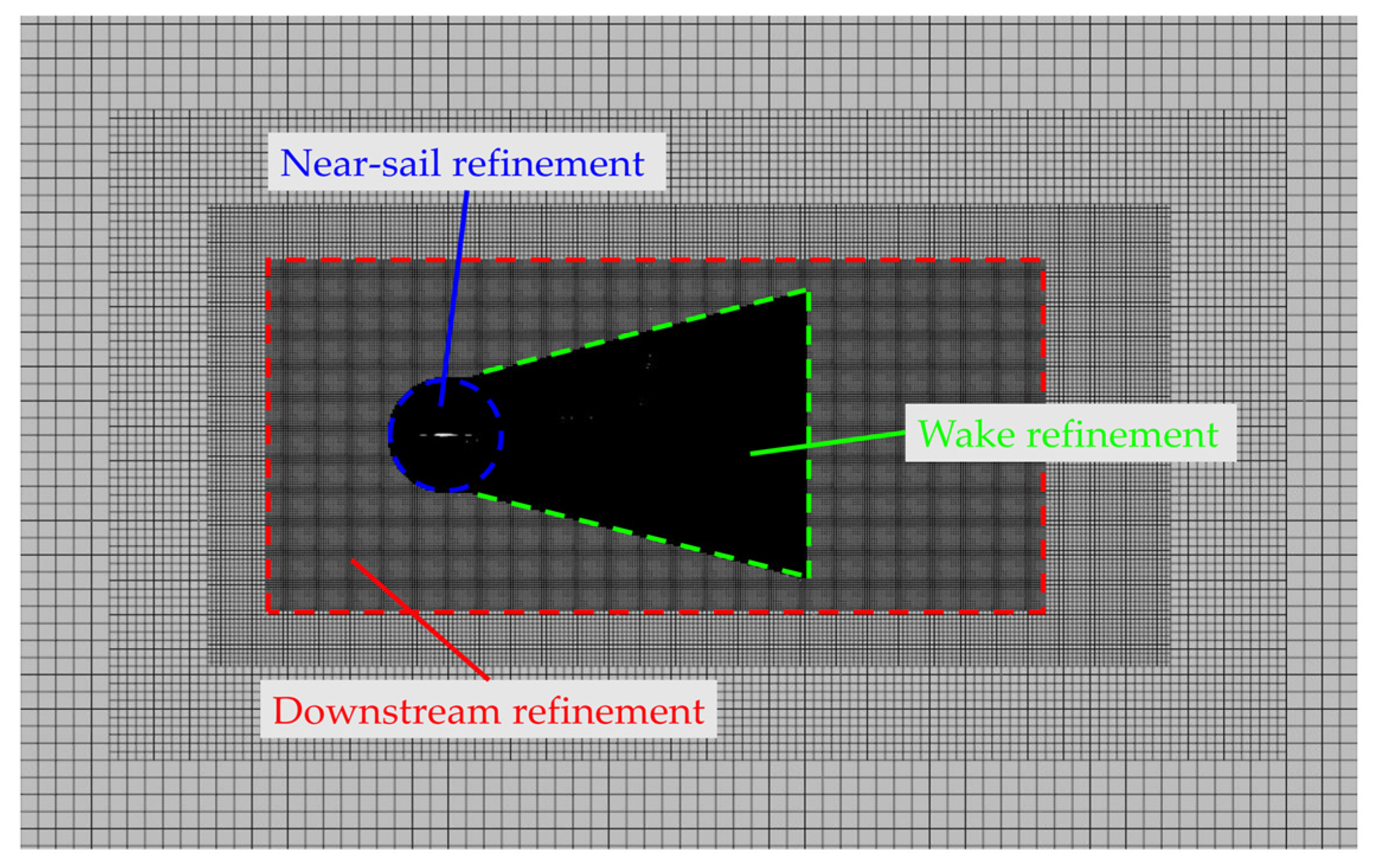
2.3.1. Computational Domain
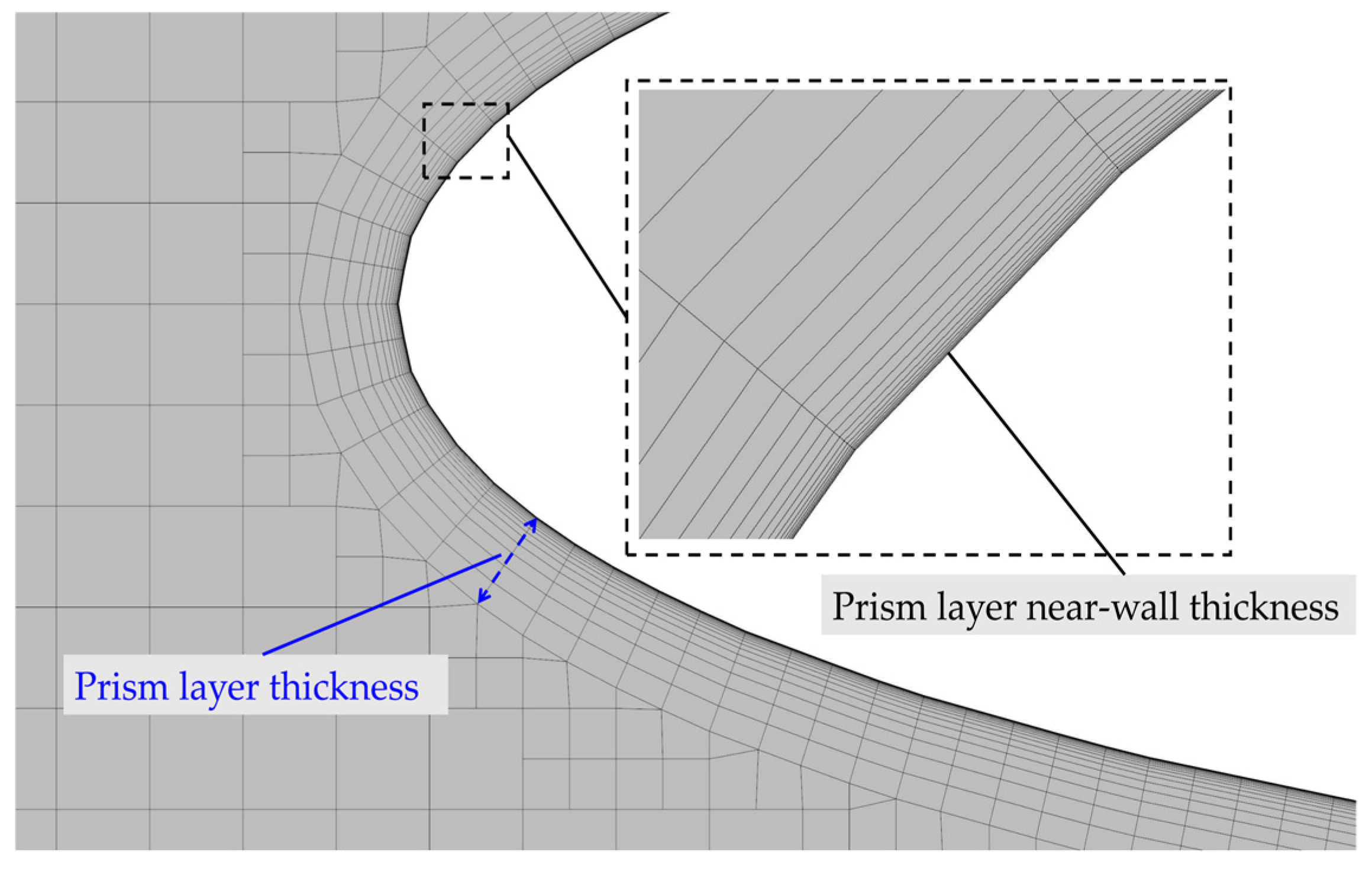
2.3.2. Mesh Generation and Convergence Test
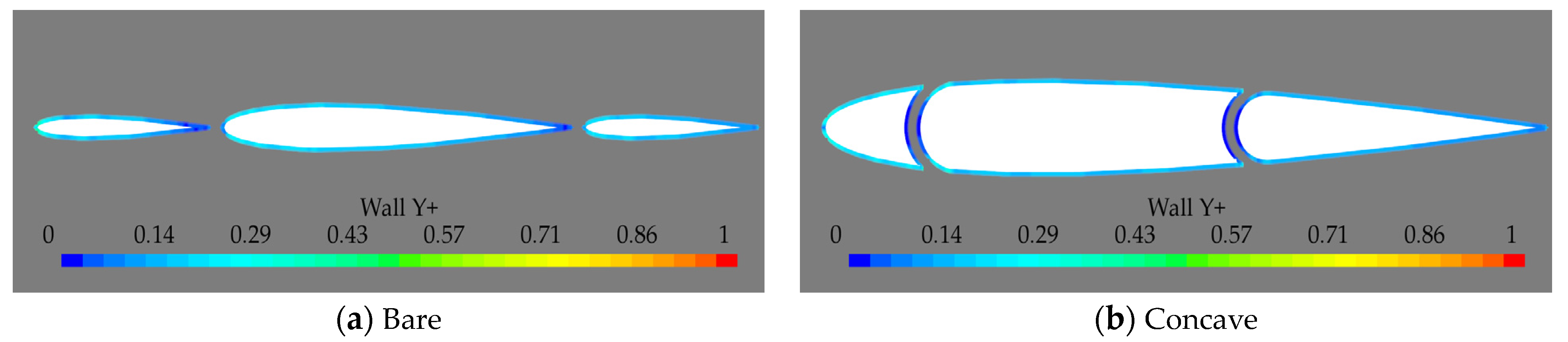
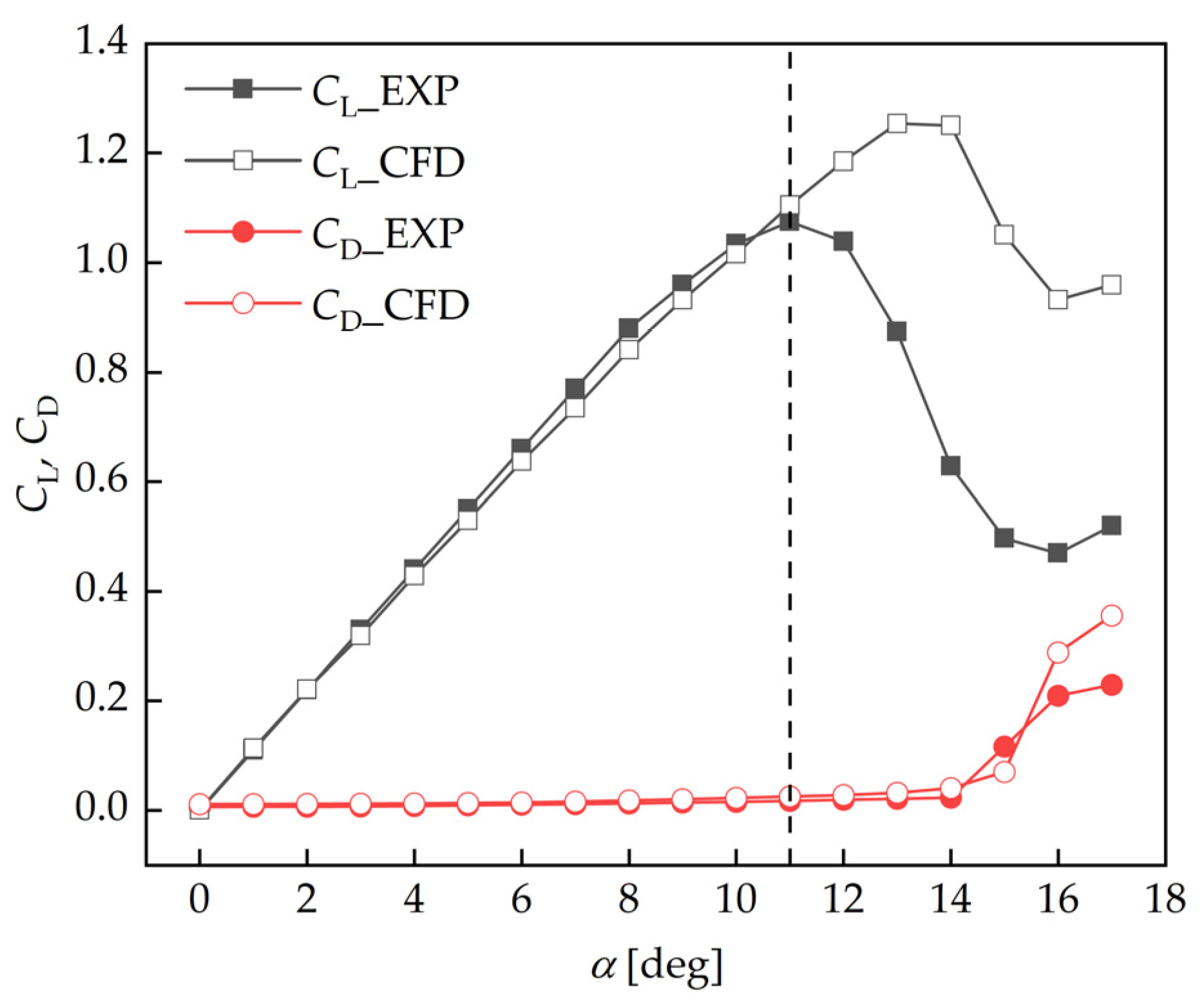
2.3.3. Validation
3. Numerical Results
3.1. Wingsails without Camber
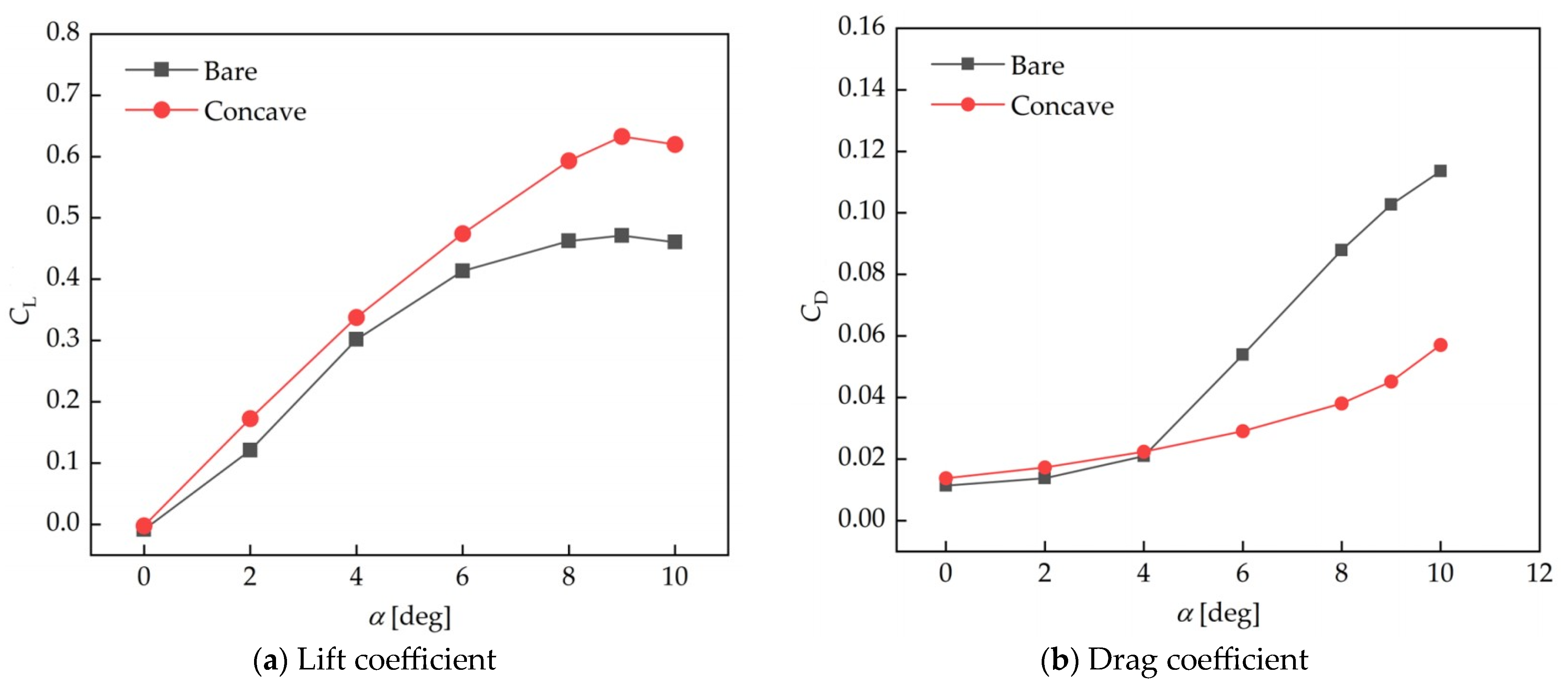
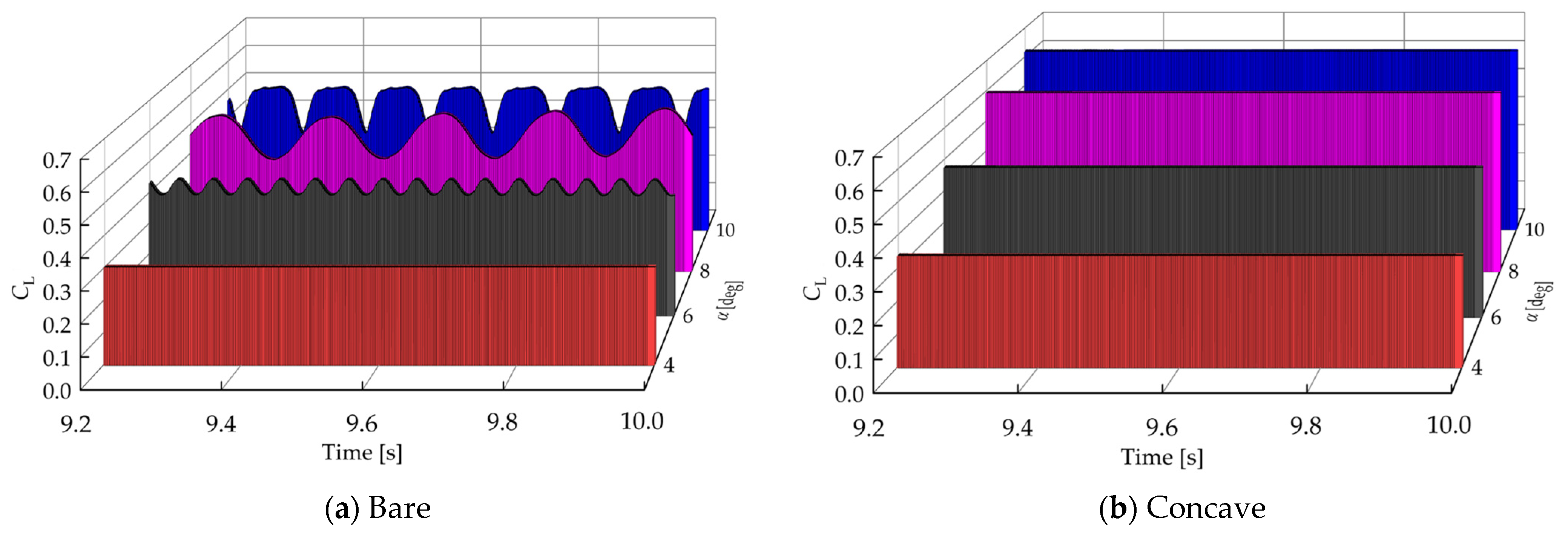
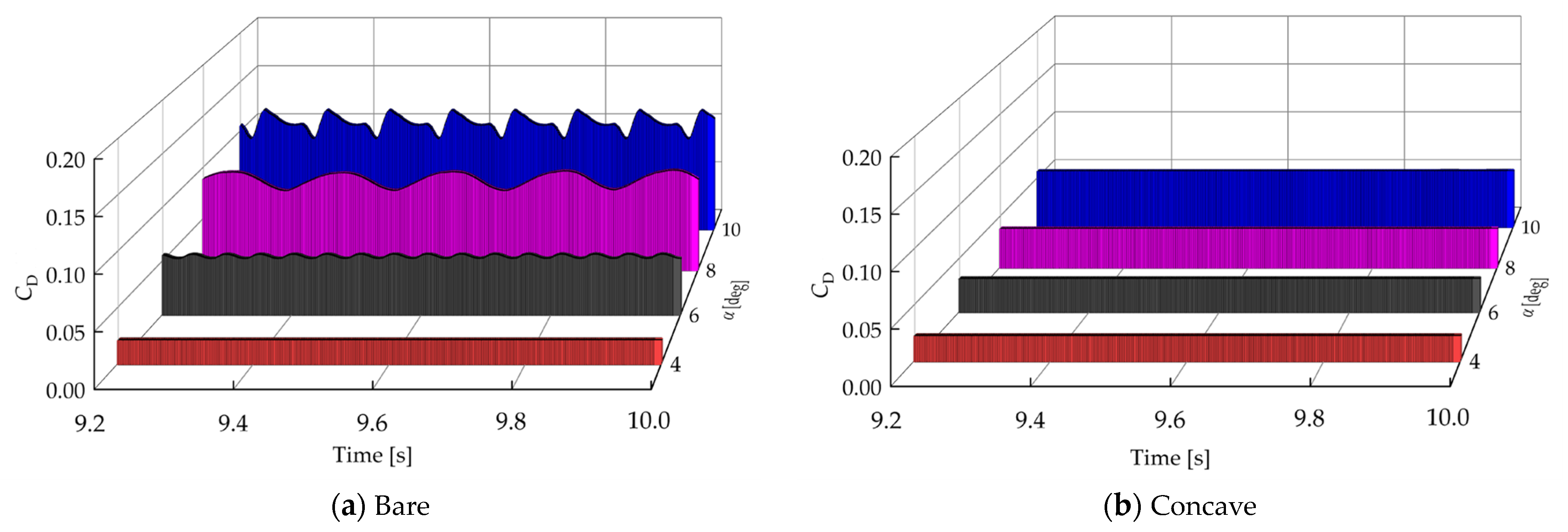
3.1.1. Lift and Drag Coefficient
3.1.2. Thrust Coefficient
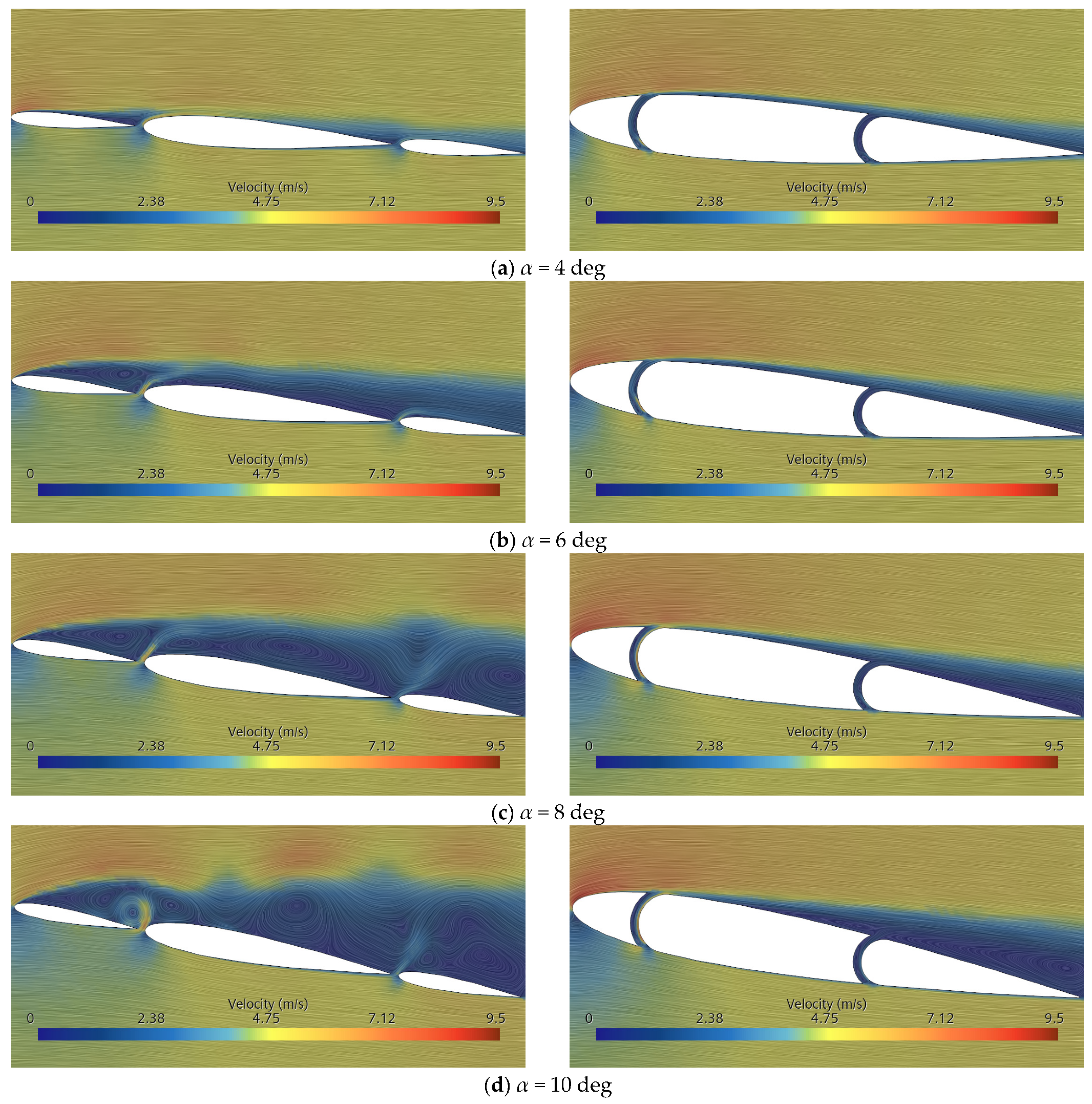
3.1.3. Flow Pattern
3.2. Wingsails with Camber
3.2.1. Definition of Camber
3.2.2. Individual Evaluation for Nose and Flap Cambers
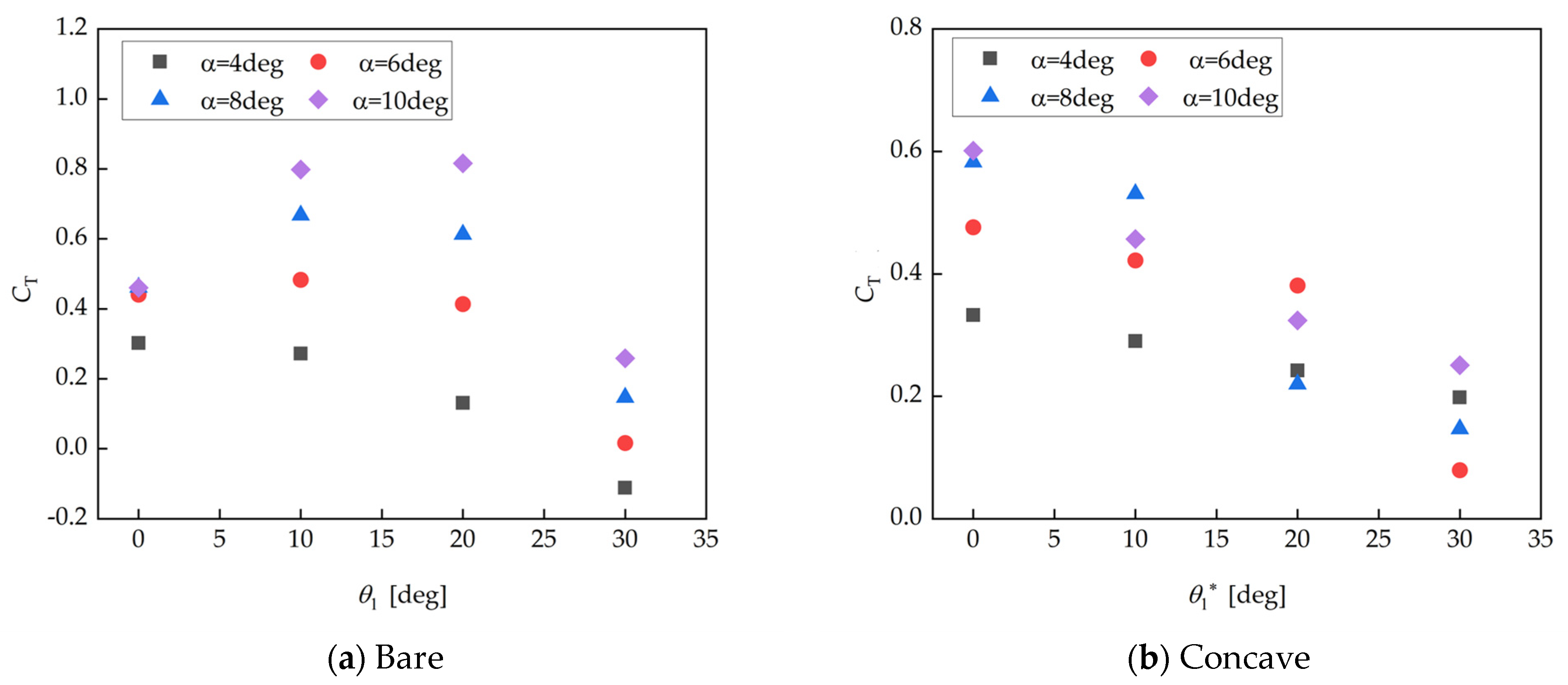
3.2.3. Parallel Evaluation for Nose and Flap Cambers
4. Conclusions
- In an unfolded state, the aerodynamic and thrust performance of the concave wingsail is superior to that of the bare wingsail. In an AOA range of 4 to 10 degrees, the concave wingsail has a higher lift coefficient and lower drag coefficient, which results in a higher thrust performance for the same AOA and AWA. In addition, the flow pattern on the surface of the concave wingsail is consistently stable, with no significant vortex shedding, which indicates that the thrust performance is more stable.
- When evaluating the effect of the nose and flap cambers individually, it is found that rotating only the flap can significantly increase the thrust coefficients of both the bare and concave wingsails. However, it should be noted that the thrust coefficients decrease when the nose and flap cambers increase to certain critical values. In summary, the suitable variation interval for the nose and flap cambers are 0 to 20 degrees and 40 to 60 degrees, respectively.
- The thrust performance of both wingsails is further improved in the fully folded condition, i.e., when both the nose and flap are rotated. The maximum thrust coefficient of the bare wingsail is 1.7, when the nose’s camber is equal to 20 degrees and the flap’s camber is equal to 50 degrees. As for the concave wing, the maximum thrust coefficient is 2.1, at which the nose’s camber is equal to 15 degrees and the flap’s camber is equal to 50 degrees. In particular, at an AOA of 8 degrees, the thrust coefficient of the concave wingsail is increased by 23.5% compared with the bare wingsail.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| c | total chord of the wingsail [m] |
| c1, c1* | chord of the nose of the wingsail [m] |
| c2, c2* | chord of the main body of the wingsail [m] |
| c3, c3* | chord of the flap of the wingsail [m] |
| g1, g1, g1*, g2* | gap of the wingsail [m] |
| Re | Reynolds number [-] |
| U | velocity of the inlet flow [m/s] |
| Δt | time step [s] |
| CL | lift coefficient [-] |
| CD | drag coefficient [-] |
| CT | thrust coefficient [-] |
| VA | apparent wind speed [m/s] |
| VS | sailing wind speed [m/s] |
| VT | true wind speed [m/s] |
| FL | lift force [N] |
| FD | drag force [N] |
| FT | thrust force [N] |
| α | angle of attack [deg] |
| β | angle of apparent wind [deg] |
| θ1, θ1* | camber angle of nose of the wingsail [deg] |
| θ2, θ2* | camber angle of flap of the wingsail [deg] |
| x1, x1* | pivot location of nose of the wingsail [-] |
| x2, x2* | pivot location of nose of the wingsail [-] |
References
- Joung, T.H.; Kang, S.G.; Lee, J.K.; Ahn, J. The IMO initial strategy for reducing Greenhouse Gas (GHG) emissions, and its follow-up actions towards 2050. J. Int. Marit. Saf. Environ. Aff. Ship. 2020, 4, 1–7. [Google Scholar] [CrossRef]
- Available online: https://www.dnv.com/news/imo-mepc-80-shipping-to-reach-net-zero-ghg-emissions-by-2050-245376 (accessed on 7 July 2023).
- Nyanya, M.N.; Vu, H.B. Wind Propulsion Optimisation and Its Integration with Solar Power. Ph.D. Thesis, World Maritime University, Malmö, Sweden, 2019. [Google Scholar]
- Julià Lluis, E. Concept Development of a Fossil Free Operated Cargo Ship. Ph.D Thesis, Chalmers University of Technology, Gothenburg, Sweden, 2019. [Google Scholar]
- Available online: https://www.offshore-energy.biz/dnv-greenlights-oceanbirds-wing-sail/ (accessed on 29 August 2023).
- Available online: https://www.prnewswire.com/news-releases/berge-bulk-unveils-the-worlds-most-powerful-sailing-cargo-ship-301955875.html (accessed on 16 October 2023).
- Furukawa, H.; Blakeley, A.W.; Flay, R.G.; Richards, P.J. Performance of wing sail with multi element by two-dimensional wind tunnel investigations. J. Fluid Sci. Technol. 2015, 10, JFST0019. [Google Scholar] [CrossRef]
- Schneider, A.; Arnone, A.; Savelli, M.; Ballico, A.; Scutellaro, P. On the use of CFD to assist with sail design. In Proceedings of the SNAME Chesapeake Sailing Yacht Symposium, Annapolis, MD, USA, 3 March 2003. [Google Scholar]
- Li, C.; Wang, H.; Sun, P. Numerical investigation of a two-element wingsail for ship auxiliary propulsion. J. Mar. Sci. Eng. 2020, 8, 333. [Google Scholar] [CrossRef]
- Li, C.; Wang, H.; Sun, P. Study on the Influence of Gradient Wind on the Aerodynamic Characteristics of a Two-Element Wingsail for Ship-Assisted Propulsion. J. Mar. Sci. Eng. 2023, 11, 134. [Google Scholar] [CrossRef]
- Blakeley, A.W.; Flay, R.G.J.; Richards, P.J. Design and optimisation of multi-element wing sails for multihull yachts. In Proceedings of the 18th Australasian Fluid Mechanics Conference, Launceston, Australia, 3–7 December 2012. [Google Scholar]
- Ma, R.; Wang, Z.; Wang, K.; Zhao, H.; Jiang, B.; Liu, Y.; Xing, H.; Huang, L. Evaluation Method for Energy Saving of Sail-Assisted Ship Based on Wind Resource Analysis of Typical Route. J. Mar. Sci. Eng. 2023, 11, 789. [Google Scholar] [CrossRef]
- Shen, X.; Avital, E.; Rezaienia, M.A.; Paul, G.; Korakianitis, T. Computational methods for investigation of surface curvature effects on airfoil boundary layer behavior. J. Algorithms Comput. Technol. 2017, 11, 68–82. [Google Scholar] [CrossRef]
- Bilandi, R.N.; Jamei, S.; Roshan, F.; Azizi, M. Numerical simulation of vertical water impact of asymmetric wedges by using a finite volume method combined with a volume-of-fluid technique. Ocean. Eng. 2018, 160, 119–131. [Google Scholar] [CrossRef]
- Hassan, G.E.; Hassan, A.; Youssef, M.E. Numerical investigation of medium range re number aerodynamics characteristics for NACA0018 airfoil. CFD Lett. 2014, 6, 175–187. [Google Scholar]
- Blount, H.; Portell Lasfuentes, J.M. CFD Investigation of Wind Powered Ships under Extreme Conditions. Master’s Thesis, Chalmers University of Technology, Gothenburg, Sweden, 2021. [Google Scholar]
- Sheldahl, R.E.; Klimas, P.C. Aerodynamic Characteristics of Seven Symmetrical Airfoil Sections through 180-Degrees Angle of Attack for Use in Aerodynamic Analysis of Vertical Axis Wind Turbines (No. SAND-80-2114); Sandia National Lab (SNL-NM): Albuquerque, NM, USA, 1981. [Google Scholar]


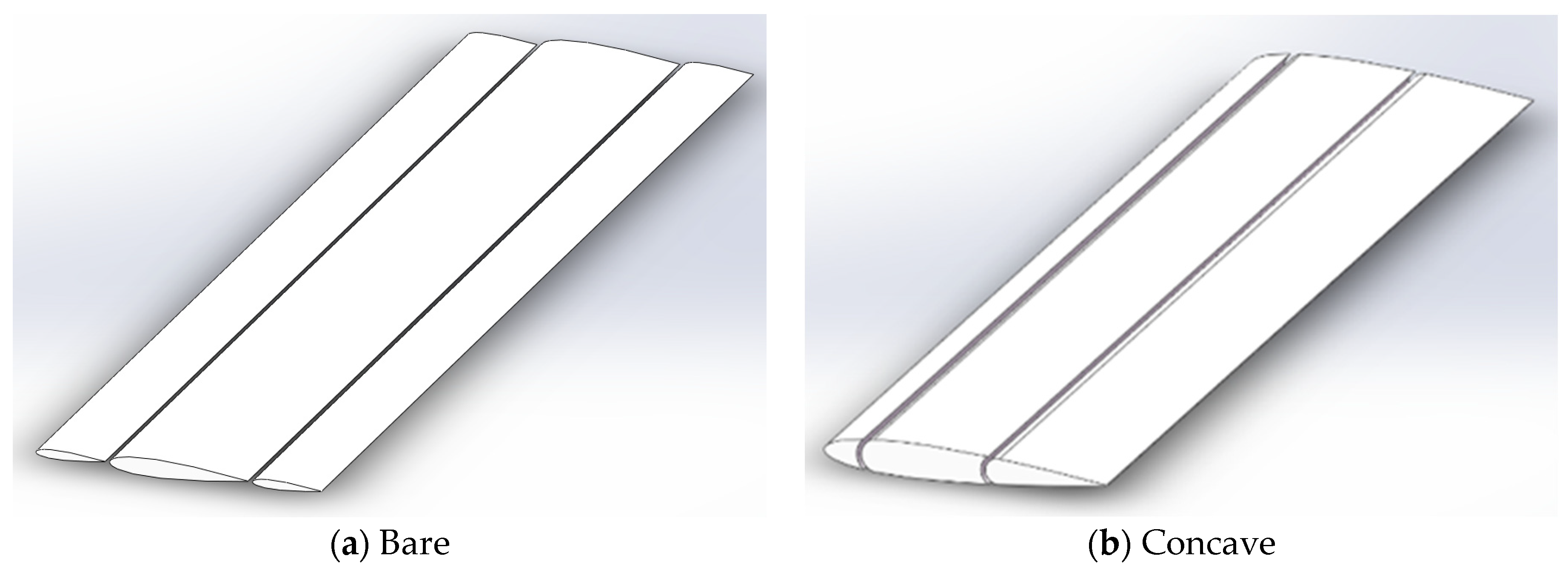
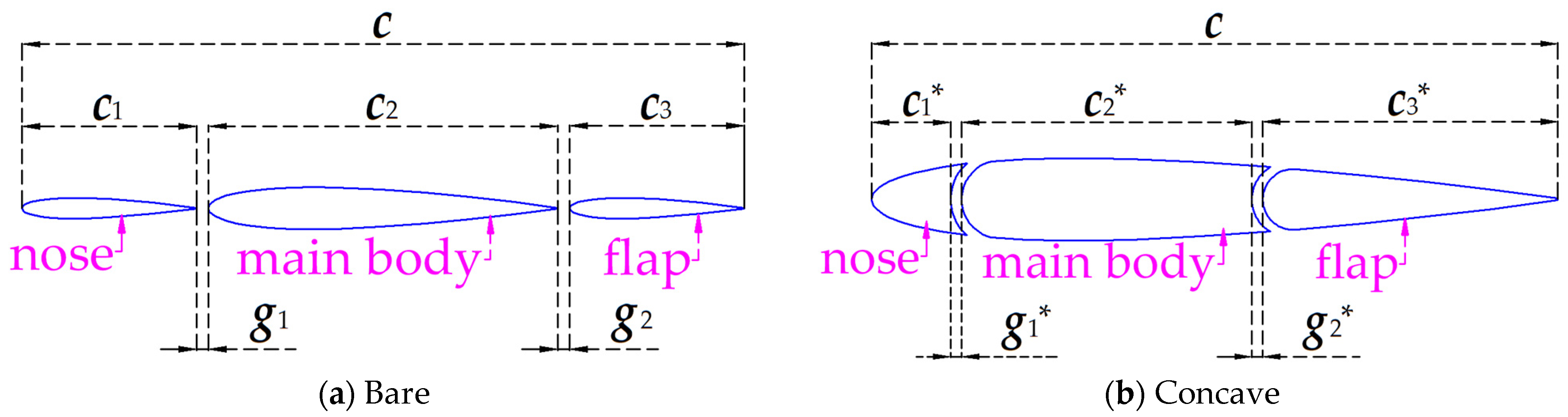








| Parameter | Value |
|---|---|
| c | 1 m |
| c1:c2:c3 | 1:2:1 |
| c1*:c2*:c3* | 1:3.7:3.7 |
| g1, g1, g1*, g2* | 0.016 m |
| Model | Case | Mesh Number [-] | CL | Difference of CL | CD | Difference of CD |
|---|---|---|---|---|---|---|
| bare | coarse | 79,775 | 0.112 | 7.44% | 0.015 | 7.14% |
| medium | 135,177 | 0.119 | 1.65% | 0.014 | 0 | |
| fine | 191,605 | 0.121 | —— | 0.014 | —— | |
| concave | coarse | 81,015 | 0.174 | 1.75% | 0.018 | 5.88% |
| medium | 137,130 | 0.172 | 0.58% | 0.017 | 0 | |
| fine | 193,963 | 0.171 | —— | 0.017 | —— |
| Parameter | Value |
|---|---|
| 1 m/s | |
| 2.1 | |
| 1.225 kg/m3 | |
| 5 m/s | |
| 1 m2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, Y.; Cao, C.; Cui, T.; Yang, H.; Tian, Z. Numerical Study on Auxiliary Propulsion Performance of Foldable Three-Element Wingsail Utilizing Wind Energy. Energies 2024, 17, 3833. https://doi.org/10.3390/en17153833
Jiang Y, Cao C, Cui T, Yang H, Tian Z. Numerical Study on Auxiliary Propulsion Performance of Foldable Three-Element Wingsail Utilizing Wind Energy. Energies. 2024; 17(15):3833. https://doi.org/10.3390/en17153833
Chicago/Turabian StyleJiang, Yongxu, Chenze Cao, Ting Cui, Hao Yang, and Zhengjun Tian. 2024. "Numerical Study on Auxiliary Propulsion Performance of Foldable Three-Element Wingsail Utilizing Wind Energy" Energies 17, no. 15: 3833. https://doi.org/10.3390/en17153833
APA StyleJiang, Y., Cao, C., Cui, T., Yang, H., & Tian, Z. (2024). Numerical Study on Auxiliary Propulsion Performance of Foldable Three-Element Wingsail Utilizing Wind Energy. Energies, 17(15), 3833. https://doi.org/10.3390/en17153833







