Droop Frequency Limit Control and Its Parameter Optimization in VSC-HVDC Interconnected Power Grids
Abstract
1. Introduction
2. Principles of VSC-HVDC Involved in Frequency Stabilization Control
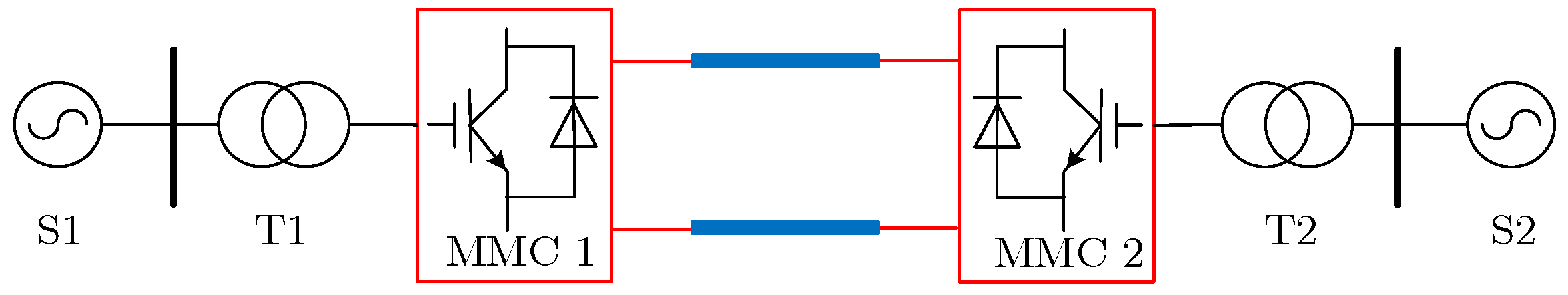
2.1. The Basic Structure of VSC-HVDC
2.2. VSC-HVDC in System Frequency Adjustment
2.3. The Role of FLC in VSC-HVDC
3. Additional Frequency Controller for VSC-HVDC Based on Droop Frequency Limit Control
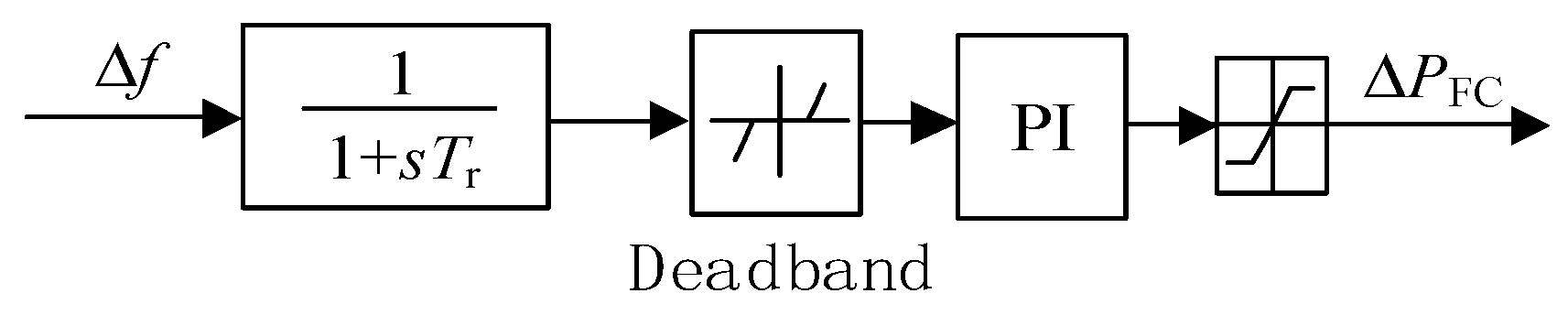
3.1. The Design of Additional Frequency Limit Controller
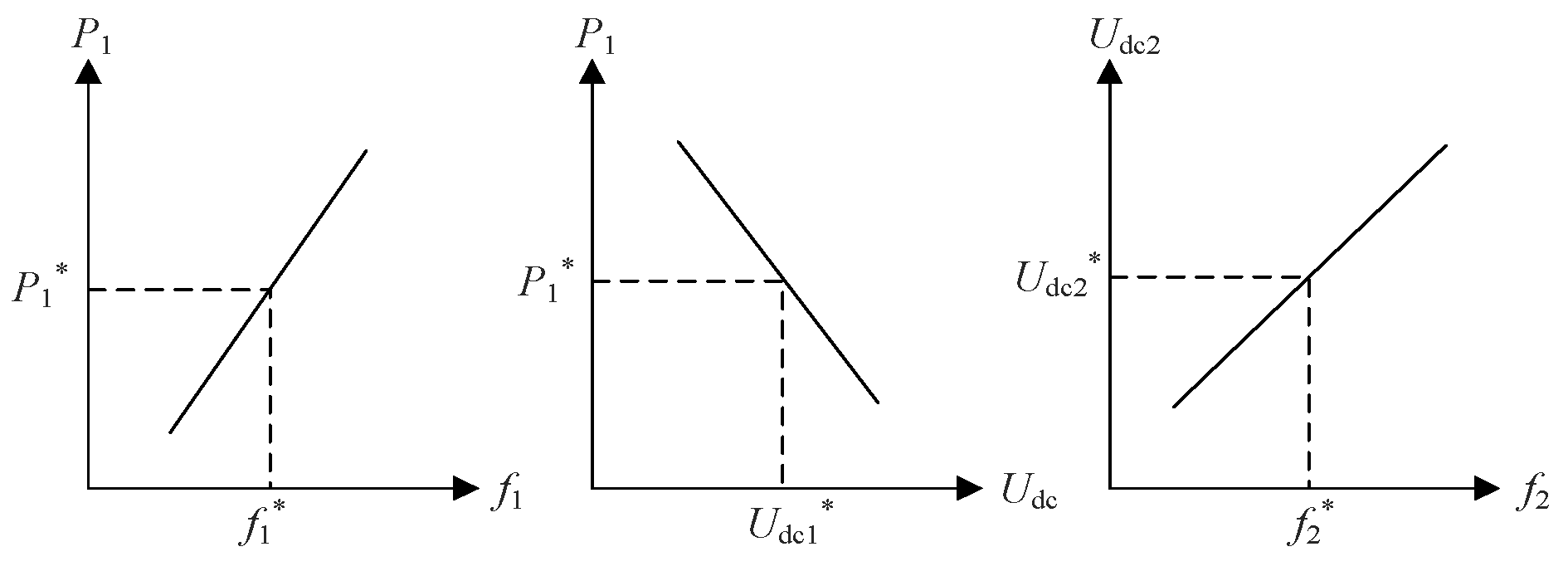
3.2. The Parameter Setting Principle of Additional Frequency Limit Controller
3.3. The Design of Controller Limiting
4. Parameter Optimization of a VSC-HVDC Additional Frequency Controller Based on a Two-Layer Optimization Approach
4.1. The Optimization Model of VSC-HVDC FLC Control Parameters
4.2. The Optimization Model of the VSC-HVDC FLC Deadband Parameter
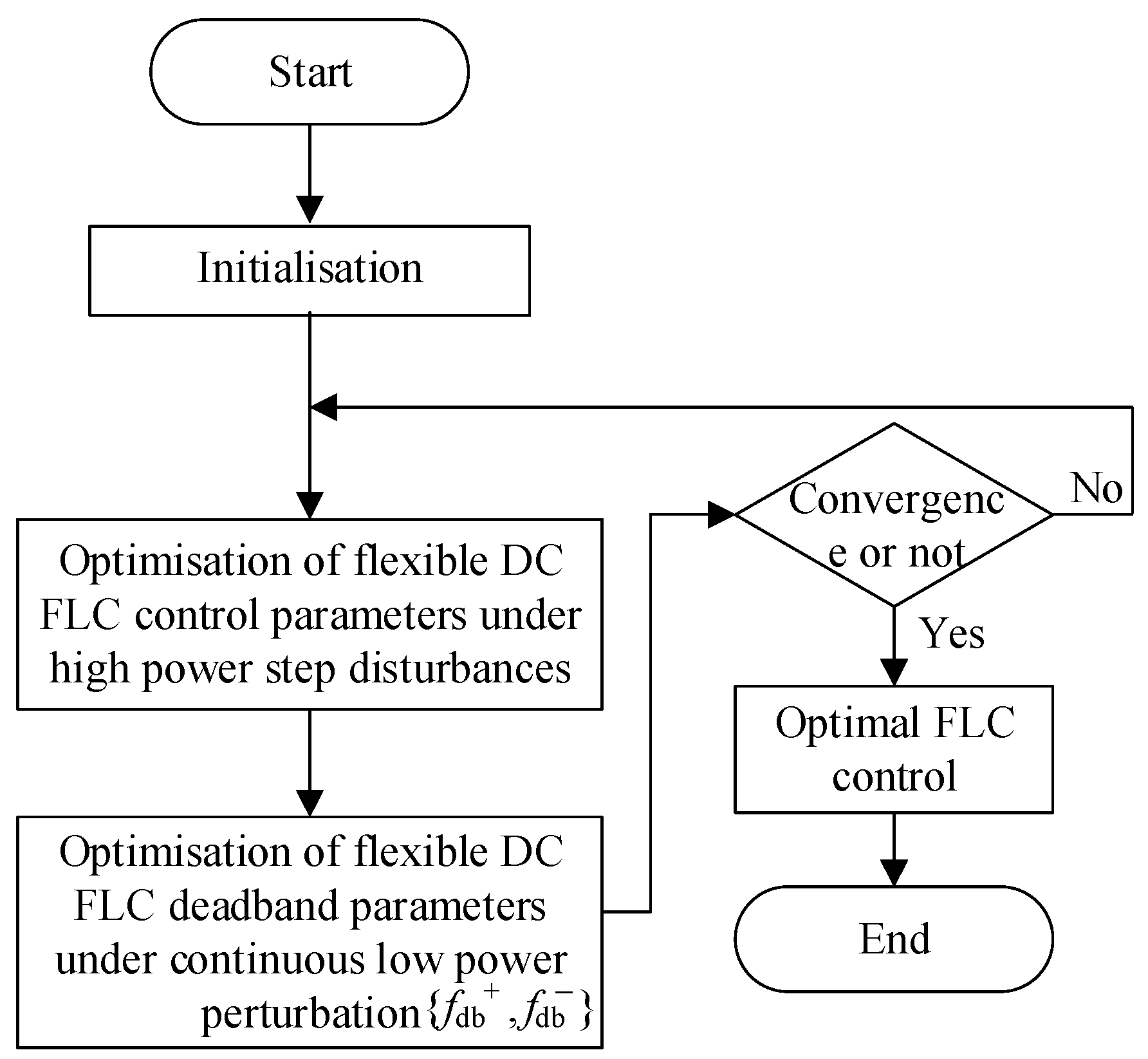
4.3. The Solution Methods of the Optimization Model
- (1)
- Layer 1 model solving
- (2)
- Layer 2 model solving
5. Case Studies
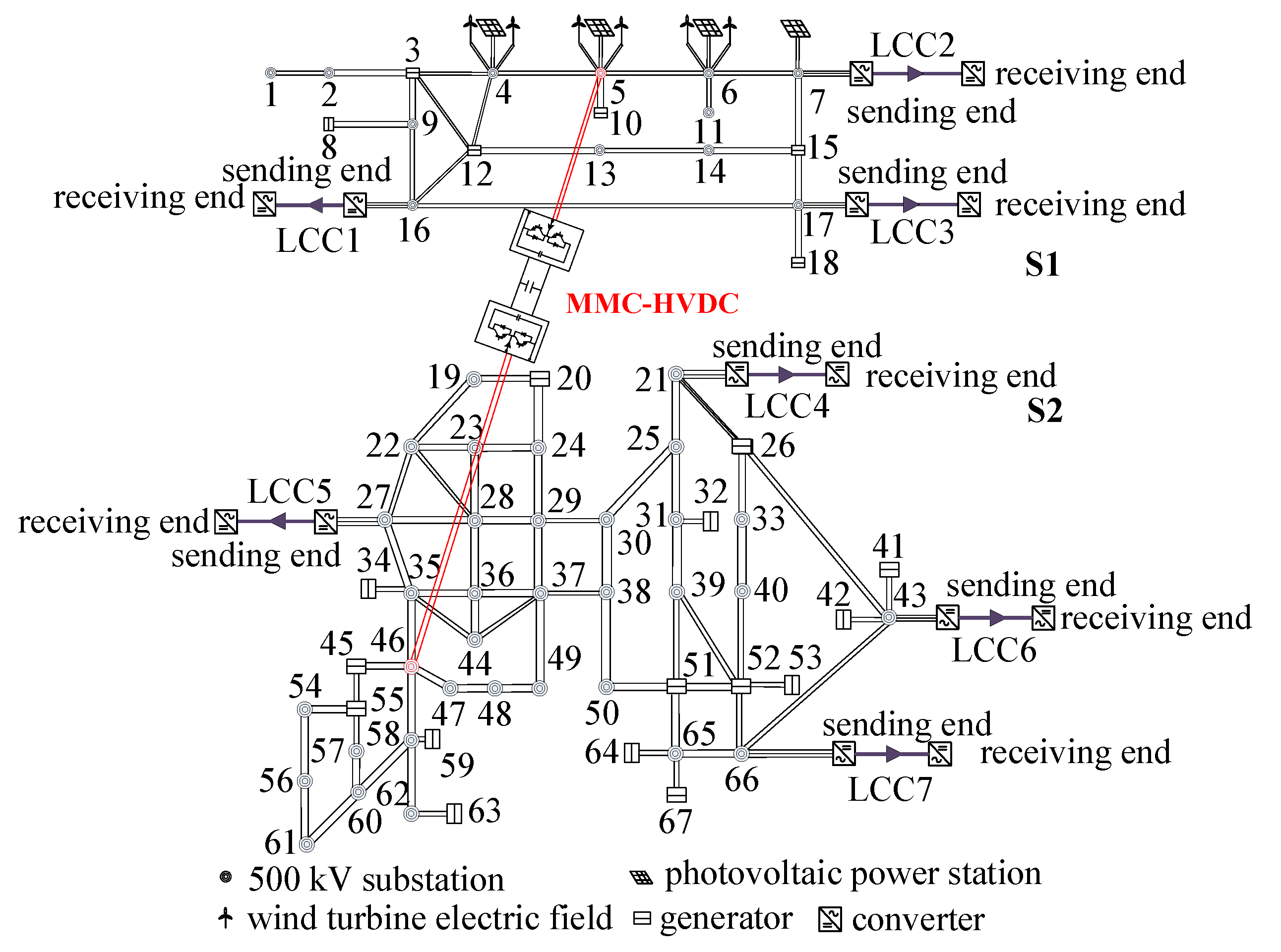
5.1. Introduction to the Test System
- (1)
- Introduction to System S1
- (2)
- Introduction to System S2
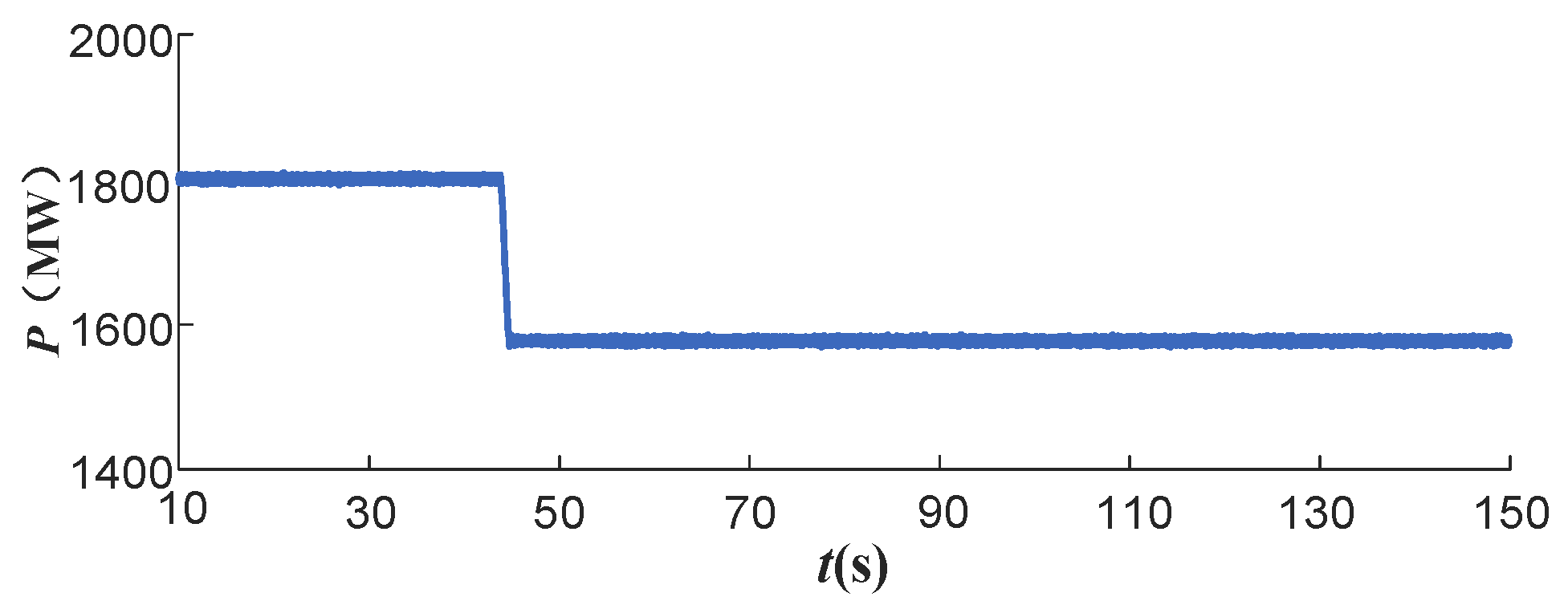
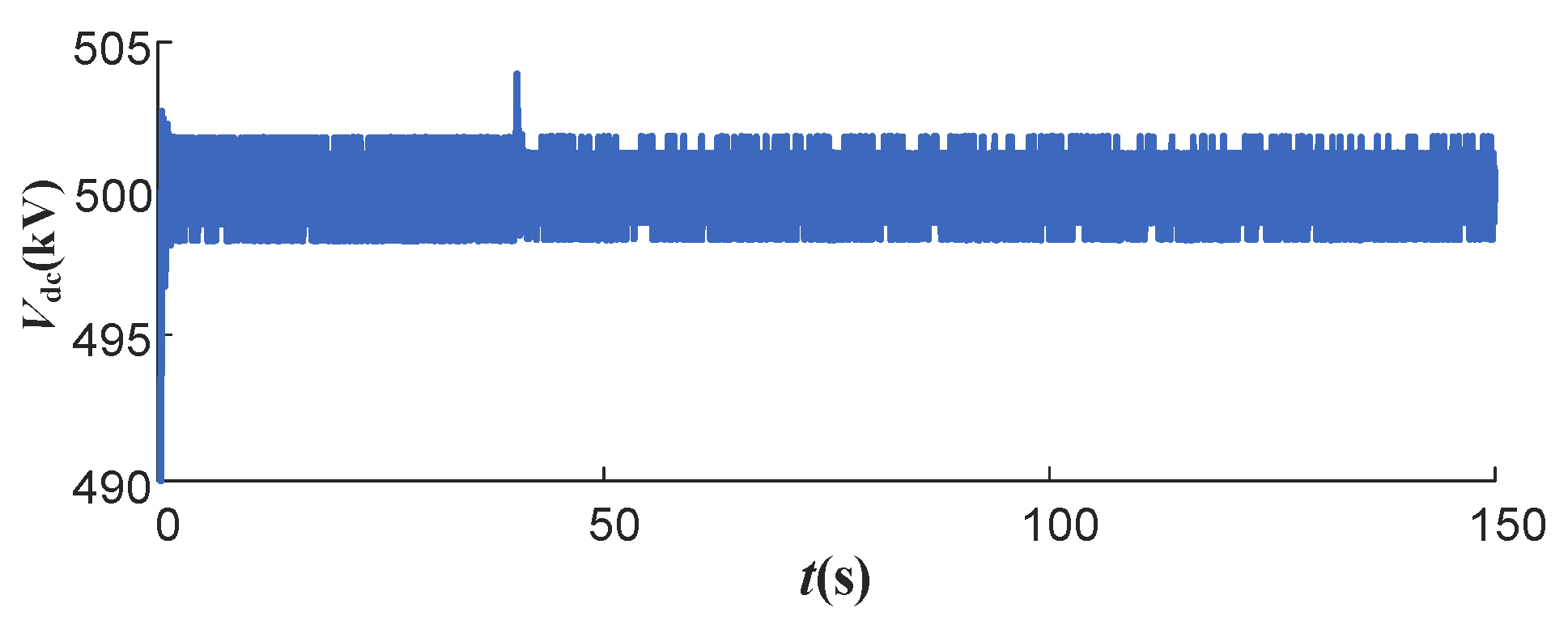
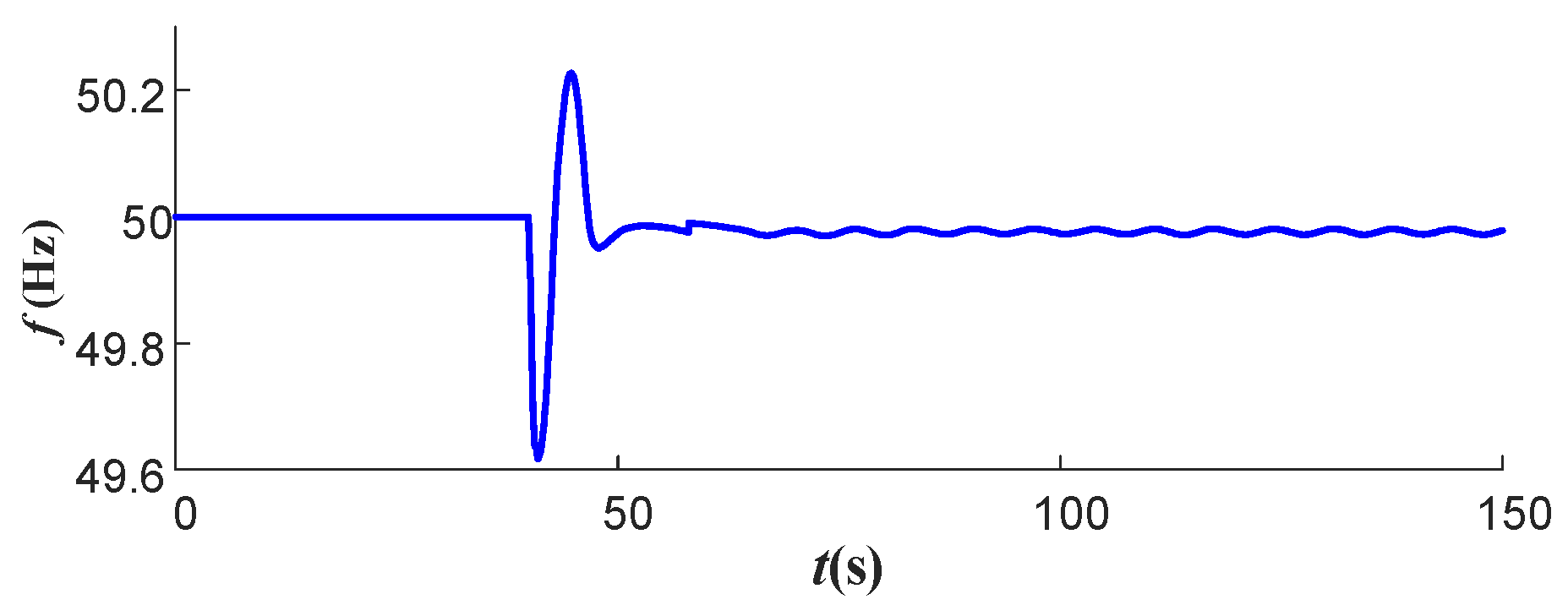
5.2. Analysis of the Low-Frequency Problem of System S1
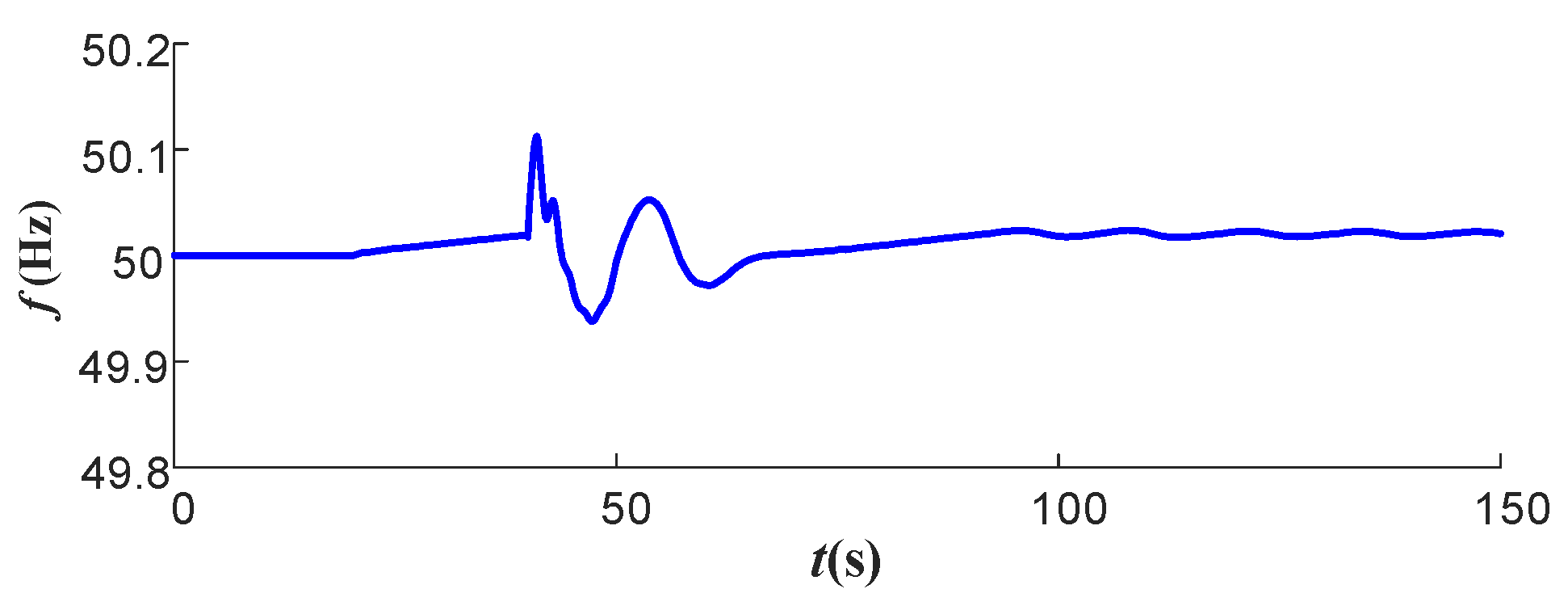
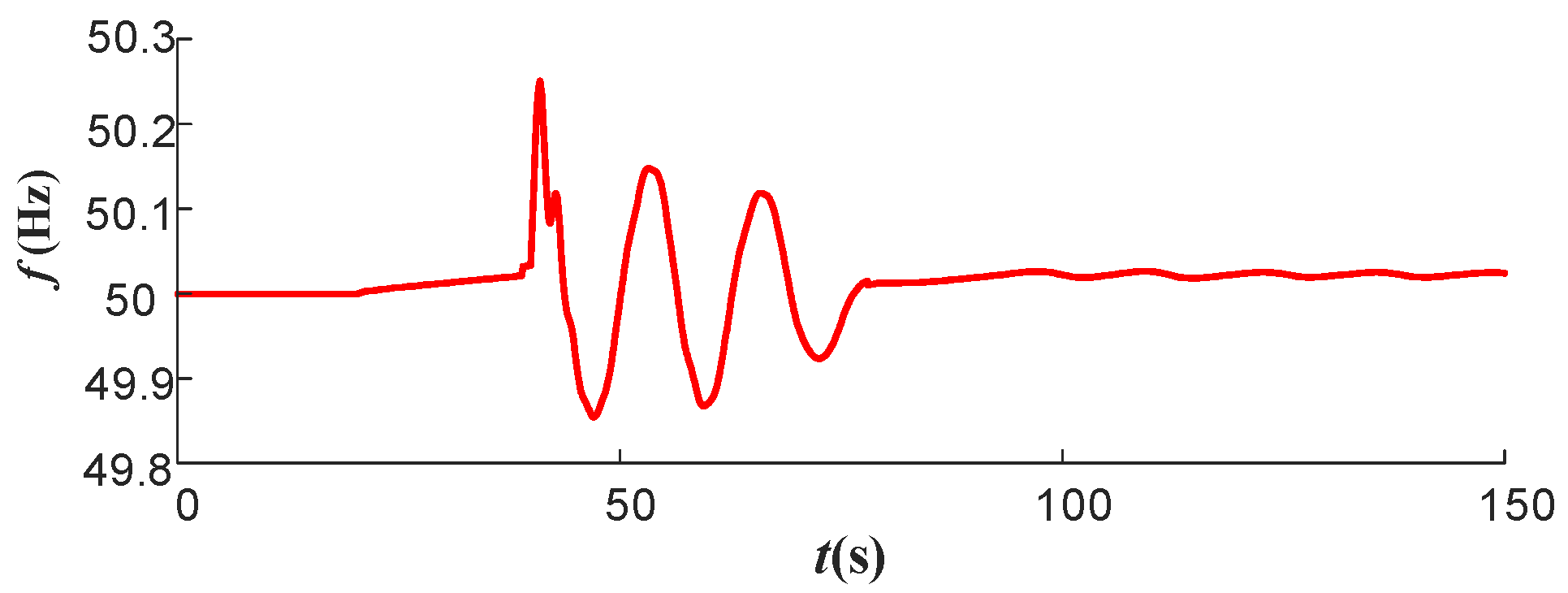
5.3. Analysis of Ultra-Low-Frequency Oscillations in System S2
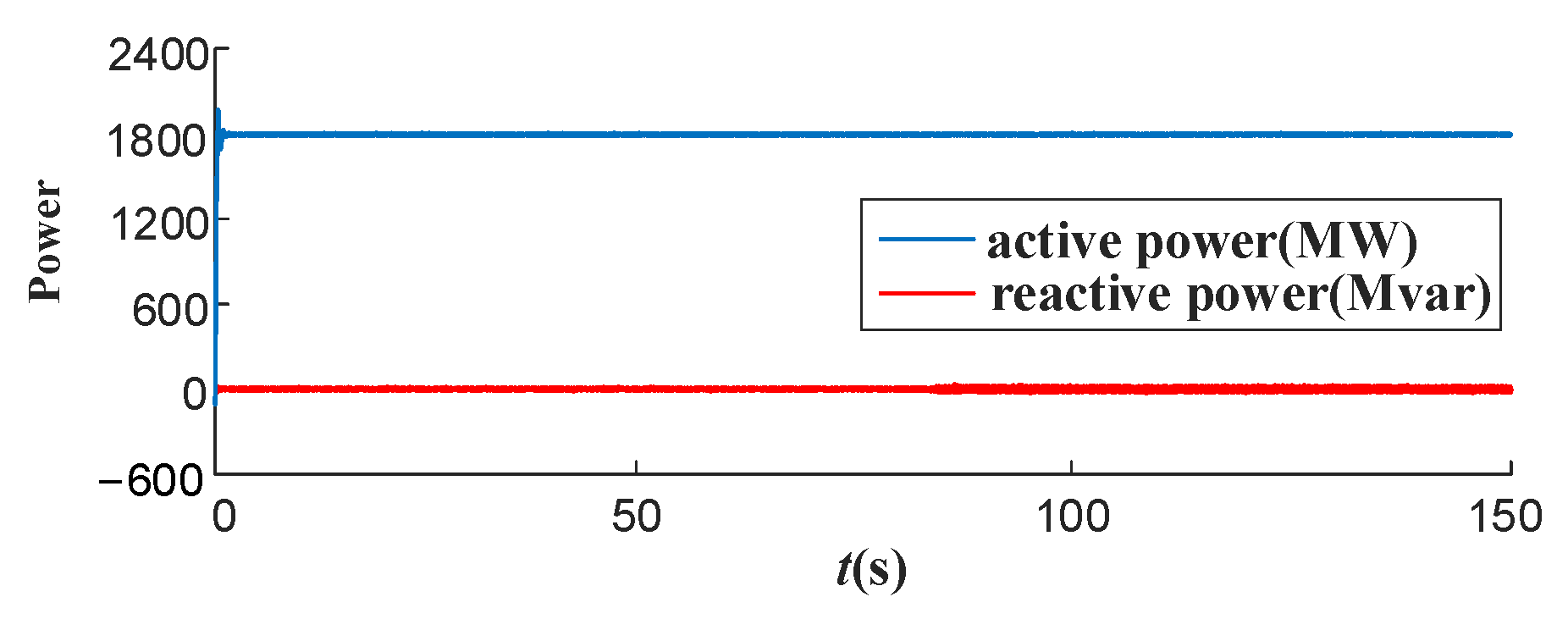
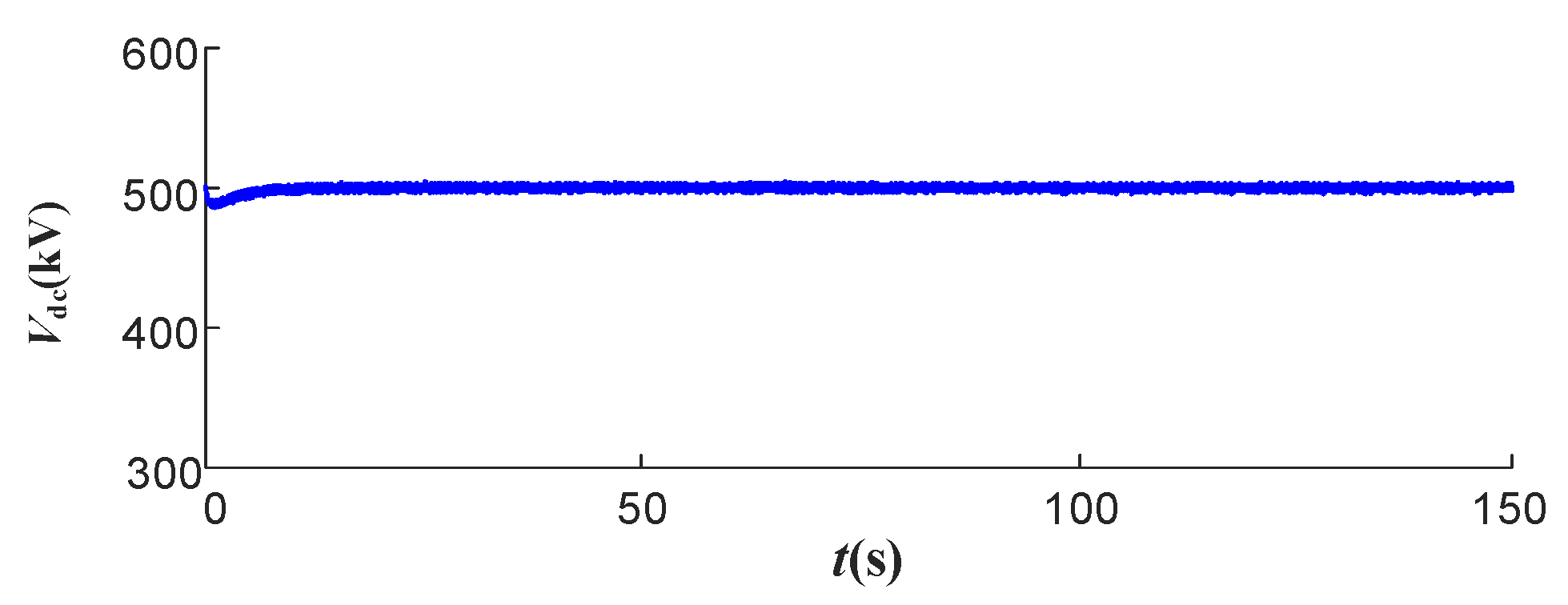
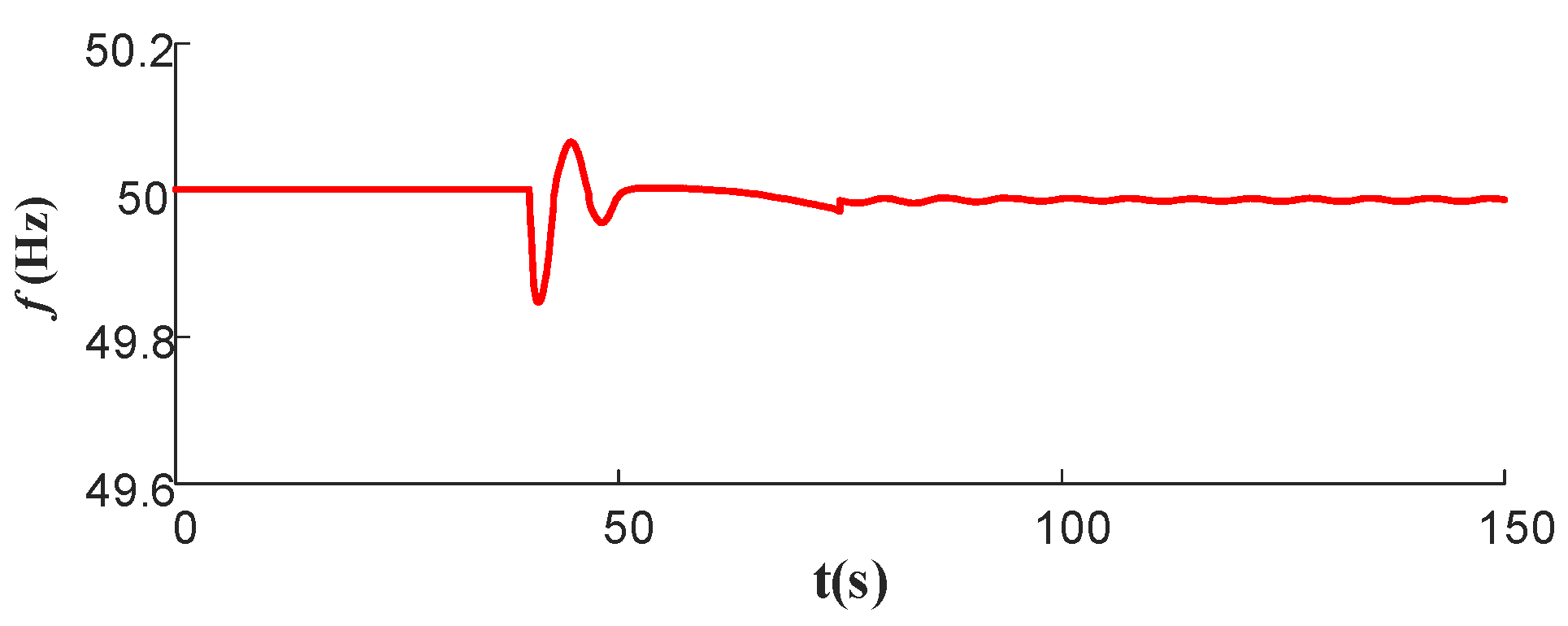
5.4. Analysis of Frequency Stabilization Problems in Flexible Interconnect Systems
5.4.1. Suppression of Low-Frequency Problems in System S1
5.4.2. Ultra-Low-Frequency Oscillation Suppression for System S2
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
| Voltage Level/kV | Number of Nodes | Type of Node |
|---|---|---|
| 500 | 13 | alternating current (AC) transmission network |
| 220 | 17 | Medium/high voltage side of transformer |
| 210 | 6 | DC rectifier side |
| 199 | 6 | DC inverter side |
| Type of Line | Number | Voltage Level/kV | Note |
|---|---|---|---|
| AC line | 23 | 500 kV | 11 AC power transmission grids |
| 220 kV | 12 renewable energy transmission lines | ||
| Two-winding transformers | 33 | 20/525 kV | 9 conventional unit step-up transformers |
| 0.4/38.5 kV | 12 renewable energy box-type transformers | ||
| 0.69/38.5 kV | |||
| 38.5/230 kV | 12 step-up transformers for renewable energy stations | ||
| Three-winding transformers | 12 | 525/230/37 kV | |
| DC line | 3 | rated voltage /kV | ±500 |
| rated power /MW | 5000 |
| Voltage Level/kV | Number of Nodes | Type of Node |
|---|---|---|
| 500 | 34 | alternating current (AC) transmission network |
| 220 | 35 | Medium/high voltage side of transformer |
| 210 | 8 | DC rectifier side |
| 199 | 8 | DC inverter side |
| Type of Line | Number | Voltage Level/kV | Note |
|---|---|---|---|
| AC line | 53 | 500 kV | 29 AC power transmission grids |
| 220 kV | 24 renewable energy transmission lines | ||
| Two-winding transformers | 62 | 20/525 kV | 14 conventional unit step-up transformers |
| 0.4/38.5 kV | 24 renewable energy box-type transformers | ||
| 0.69/38.5 kV | |||
| 38.5/230 kV | 24 step-up transformers for renewable energy stations | ||
| Three-winding transformers | 24 | 525/230/37 kV | |
| DC line | 4 | rated voltage /kV | ±500 |
| rated power /MW | 5000 |
| Parameter Name | Parameter Values on the Rectifier Side | Parameter Values on the Inverter Side |
|---|---|---|
| Rated capacity | 3000 MVA | 3000 MVA |
| d-axis outer ring controller reference value | = 1800 MW | = ±500 kV |
| q-axis outer ring controller reference | Q = 0 MVar | Q = 0 MVar |
| Inductors on bridge arms | 0.05 mH | 0.05 mH |
| Number of bridge arm submodules | 142 | 142 |
| Capacitance of bridge arm submodules | 0.015 F | 0.015 F |
| AC voltage at the PCC bus at both ends | 230 kV | 230 kV |
| Rated frequency of the AC grid at both ends | 50 Hz | 50 Hz |
| Rated voltage of the transformer | 230 kV/370 kV | 230 kV/370 kV |
| Standardized value of transformer leakage reactance | 0.4% | 0.4% |
| Initial voltage of the sub-module capacitor | 7.042 kV | 7.042 kV |
Appendix B
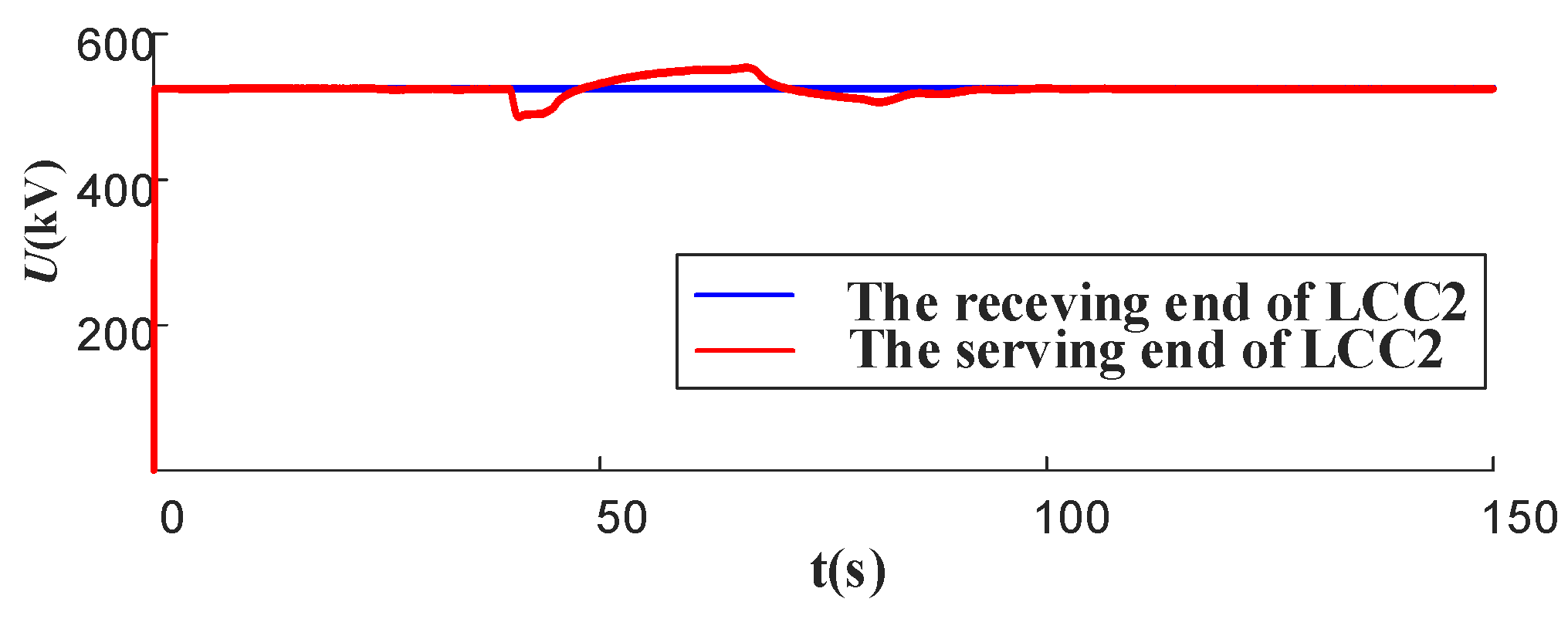
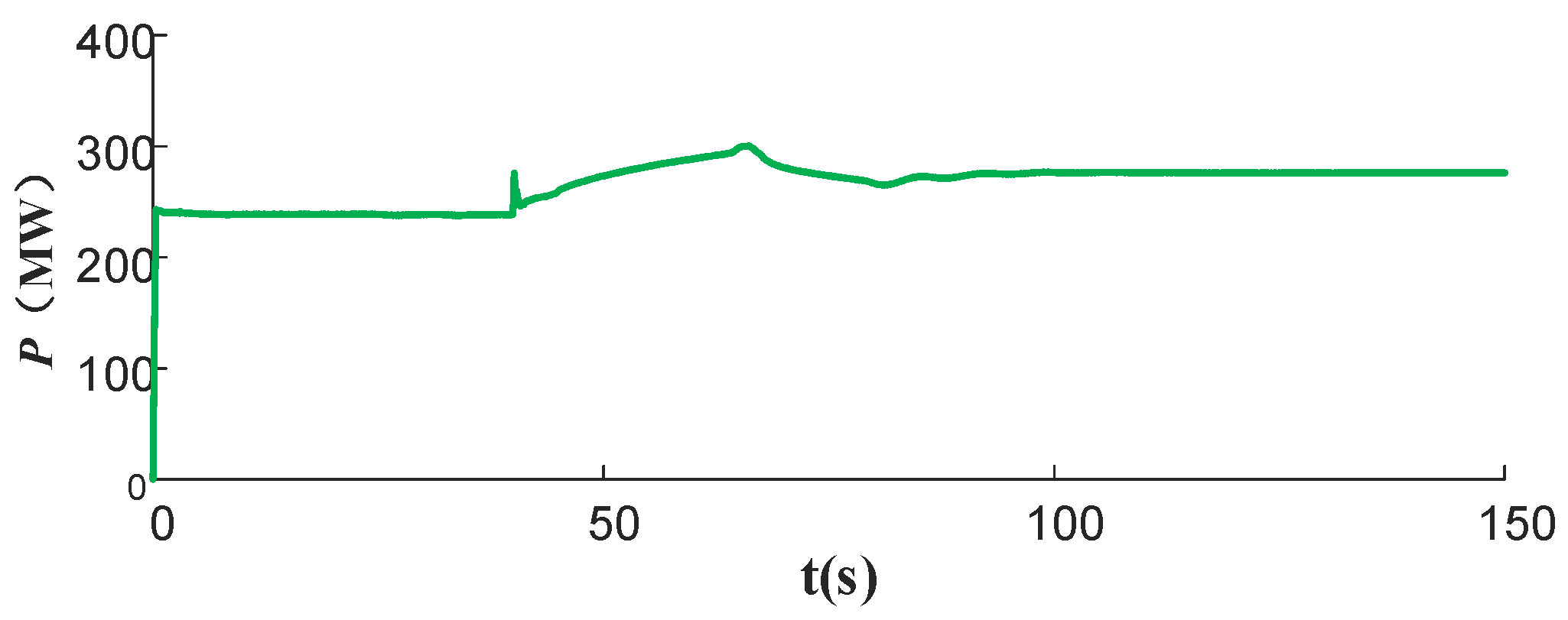
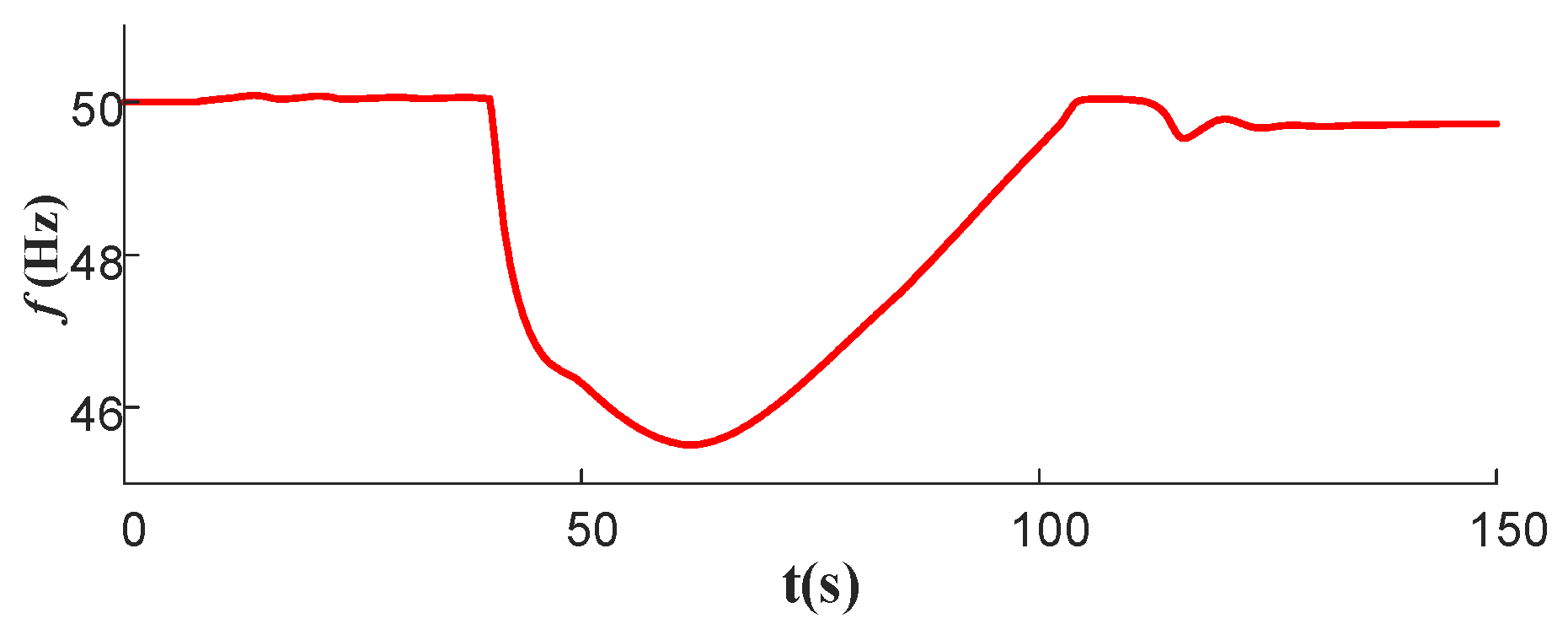
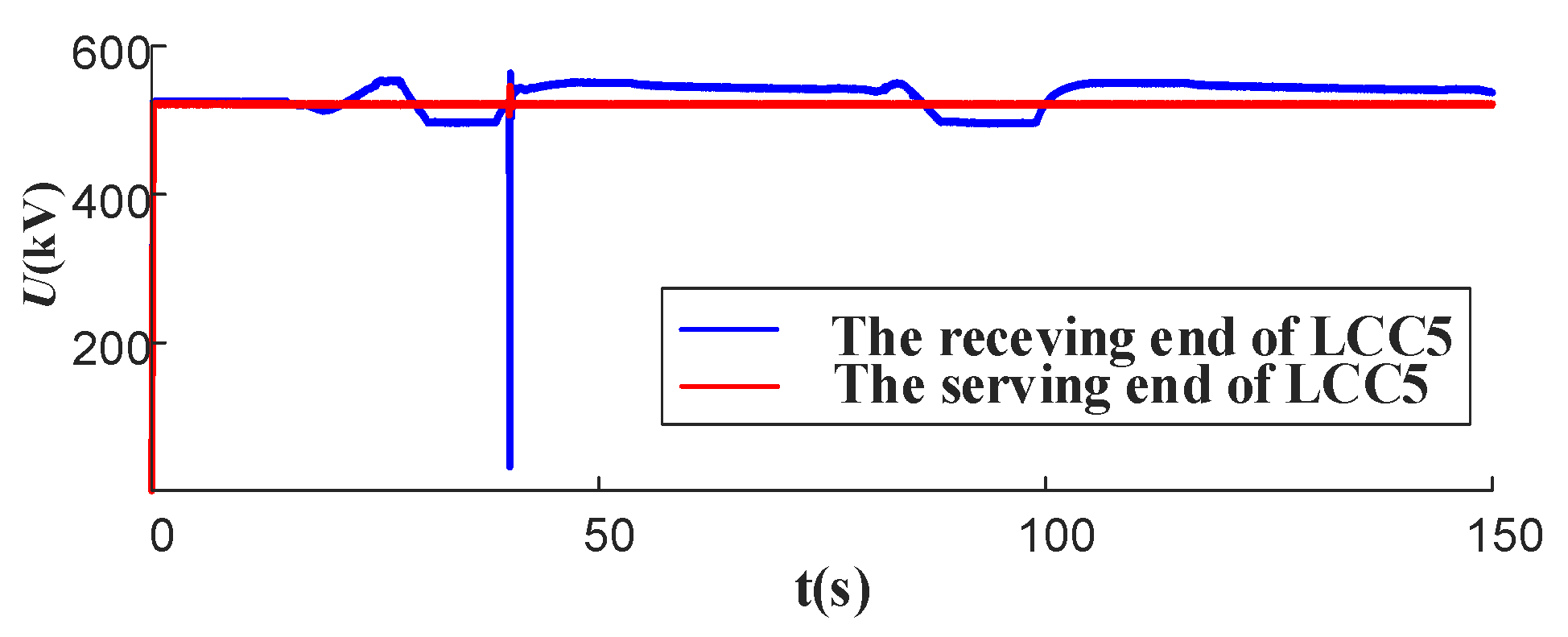

References
- Xu, Y.; Mili, L.; Sandu, A.; von Spakovsky, M.R.; Zhao, J. Propagating uncertainty in power system dynamic simulations using polynomial chaos. IEEE Trans. Power Syst. 2018, 34, 338–348. [Google Scholar] [CrossRef]
- Liu, Z.; Zhou, Y.; Li, J.; Meng, J.; Yu, X. The study on interconnection of northwest and southwest power grid in China. J. Glob. Energy Interconnect. 2023, 6, 341–352. [Google Scholar]
- Zhang, C.; Liu, L.; Cheng, H.; Liu, D.; Zhang, J.; Li, G. Frequency-constrained co-planning of generation and energy storage with high-penetration renewable energy. J. Mod. Power Syst. Clean Energy 2021, 9, 760–775. [Google Scholar]
- Liu, B.; Xiao, H.; Cai, Z.; Yang, Y. Study on frequency characteristics of receiving power system with large-scale offshore wind power generation. Energy Rep. 2023, 9, 596–607. [Google Scholar] [CrossRef]
- Li, Z.; Xue, Y.; Chen, N.; Lin, S. A coordinated frequency control of LCC-HVDC for quasi-synchronous operation of asynchronously interconnected power systems. In Proceedings of the 2023 Annual Meeting of CSEE Study Committee of HVDC and Power Electronics (HVDC 2023), Nanjing, China, 22–25 October 2023; pp. 162–168. [Google Scholar]
- Huang, J.; Chen, Y.; Gao, Q.; Zhang, Y.; Yang, R.; Yang, R.; Diao, H. Research on HVDC frequency limit control to improve frequency restoration in muti-HVDC asynchronous system. In Proceedings of the 8th Renewable Power Generation Conference (RPG 2019), Shanghai, China, 24–25 October 2019; pp. 1–6. [Google Scholar]
- Liu, C.; Liu, H.; Jiang, S.; Zheng, L. Dynamic Frequency Support and DC Voltage Regulation Approach for VSC-MTDC Systems. CSEE J. Power Energy Syst. 2023, 9, 645–658. [Google Scholar]
- Zhu, L.; He, Z.; Wu, X.; Yang, J.; Liu, D.; Kou, L. Influence of additional control strategy of back-to-back VSC-HVDC on system stability. Power Syst. Technol. 2018, 42, 2519–2527. [Google Scholar]
- Yuan, Z.C.; Wu, Z.L.; Jin, Q.; Jiang, S.G.; Huang, Y. Frequency stabilization control strategy with DC voltage secondary regulation of VSC-MTDC based interconnected systems. Autom. Electr. Power Syst. 2018, 42, 9–13+19. [Google Scholar]
- Chen, L.; Lu, X.M.; Chen, Y.P.; Min, Y.; Zhang, Y.; Tang, Z.Y. Analysis of ultra-low-frequency oscillations in multi-machine system and equivalent method. Autom. Electr. Power Syst. 2017, 41, 10–15. [Google Scholar]
- Chen, Y.; Xiao, L.; Gao, Q.; Zeng, K.; She, S.; Yang, R. Analysis of Frequency Oscillation Caused by AGC in Hydro-Dominant Power Grids using Piecewise Linearization Method. In Proceedings of the 2020 IEEE Power & Energy Society General Meeting (PESGM), Montreal, QC, Canada, 2–6 August 2020. [Google Scholar]
- Xing, C.; Liu, M.; Peng, J.; Wang, Y.; Zhou, Y.; Zheng, Z.; Gao, S.; Liao, J. FLC-Based Ultra-Low-Frequency Oscillation Suppression Scheme for Interconnected Power Grids. Energies 2024, 17, 1300. [Google Scholar] [CrossRef]
- You, G.; Guo, X.; Zhu, X.; Li, L.; Xiao, L.; Xu, Z.; Lu, S. Parameters tuning and coordination control of the frequency limit controller. J. Eng. 2019, 2019, 2102–2105. [Google Scholar] [CrossRef]
- Shi, H.; Chen, G.; Zhu, J.; Wang, Y.; Tai, K. FLC Dead band Optimization for HVDC System Stability Enhancement. In Proceedings of the 2020 IEEE Sustainable Power and Energy Conference (iSPEC), Chengdu, China, 23–25 November 2020; pp. 692–698. [Google Scholar]
- Li, J.; Xia, Y.; Yao, W.; Han, Q.; Wen, J. Additional frequency coordinated control for Chongqing-Hubei back to back VSC-HVDC and Longquan-Zhengping LCC-HVDC. South. Power Syst. Technol. 2023, 17, 134–144+152. [Google Scholar]
- Chen, Q.C.; Li, H.; Wu, W.C.; Zhang, Y.; Wang, F.; Zhang, W.Y. Optimization design of frequency limit controller of back-to-back VSC-HVDC. Power Syst. Technol. 2020, 44, 385–392. [Google Scholar]
- Yang, M.; Xiang, Q.; Wang, L.; Jiang, H.; Mo, Y.; Lin, K.; Liu, S. Application Analysis of FLC Function in Lu-xi VSC-HVDC System. Guangxi Electr. Power 2018, 41, 61–65. [Google Scholar]
- Liu, Y.; Shen, C.; Wang, Z.; Liu, F. Incentive mechanism design for emergency frequency control in multi-infeed hybrid AC-DC system. IEEE Trans. Power Syst. 2023, 39, 1867–1880. [Google Scholar] [CrossRef]
- Tang, H.; Wang, Y.; Wei, L.; Li, X. An additional frequency robust controller for HVDC transmission system under islanding operation. Power Syst. Technol. 2016, 40, 1066–1072. [Google Scholar]
- Liu, Q.; Yang, L.; Xin, Q.; Wang, Z.; Deng, J.; Wang, S.; Fu, C. Mechanism Analysis and Suppression Strategy of Ultra-low Frequency Oscillation in DC Asynchronous Networking. In Proceedings of the 16th Annual Conference of China Electrotechnical Society, Beijing, China, 24–26 September 2021; Lecture Notes in Electrical Engineering. Springer: Singapore, 2022; Volume 889. [Google Scholar]










Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, H.; Zhou, Y.; Gao, Y.; Gao, S. Droop Frequency Limit Control and Its Parameter Optimization in VSC-HVDC Interconnected Power Grids. Energies 2024, 17, 3851. https://doi.org/10.3390/en17153851
Jiang H, Zhou Y, Gao Y, Gao S. Droop Frequency Limit Control and Its Parameter Optimization in VSC-HVDC Interconnected Power Grids. Energies. 2024; 17(15):3851. https://doi.org/10.3390/en17153851
Chicago/Turabian StyleJiang, Han, Yichen Zhou, Yi Gao, and Shilin Gao. 2024. "Droop Frequency Limit Control and Its Parameter Optimization in VSC-HVDC Interconnected Power Grids" Energies 17, no. 15: 3851. https://doi.org/10.3390/en17153851
APA StyleJiang, H., Zhou, Y., Gao, Y., & Gao, S. (2024). Droop Frequency Limit Control and Its Parameter Optimization in VSC-HVDC Interconnected Power Grids. Energies, 17(15), 3851. https://doi.org/10.3390/en17153851







