Analysis of Vibration Electromechanical Response Behavior of Poly(Vinylidene Fluoride) Piezoelectric Films
Abstract
1. Introduction
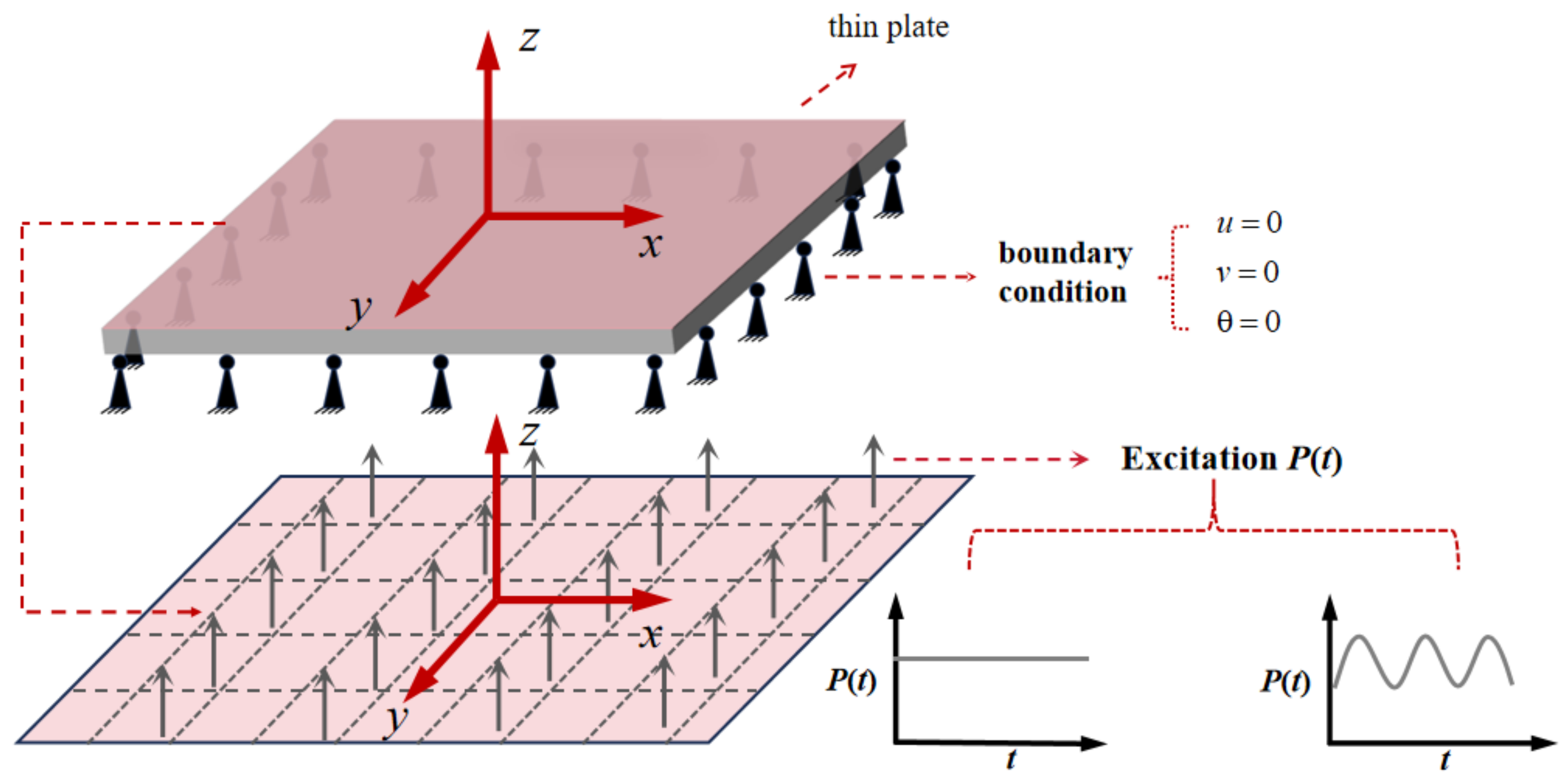
2. Theoretical Formulation
2.1. Mathematical Model
2.2. Equation of Motion
2.2.1. Static Loading Condition
2.2.2. Vibration Loading Condition
3. The Piezoelectric Film’s Electric Potential
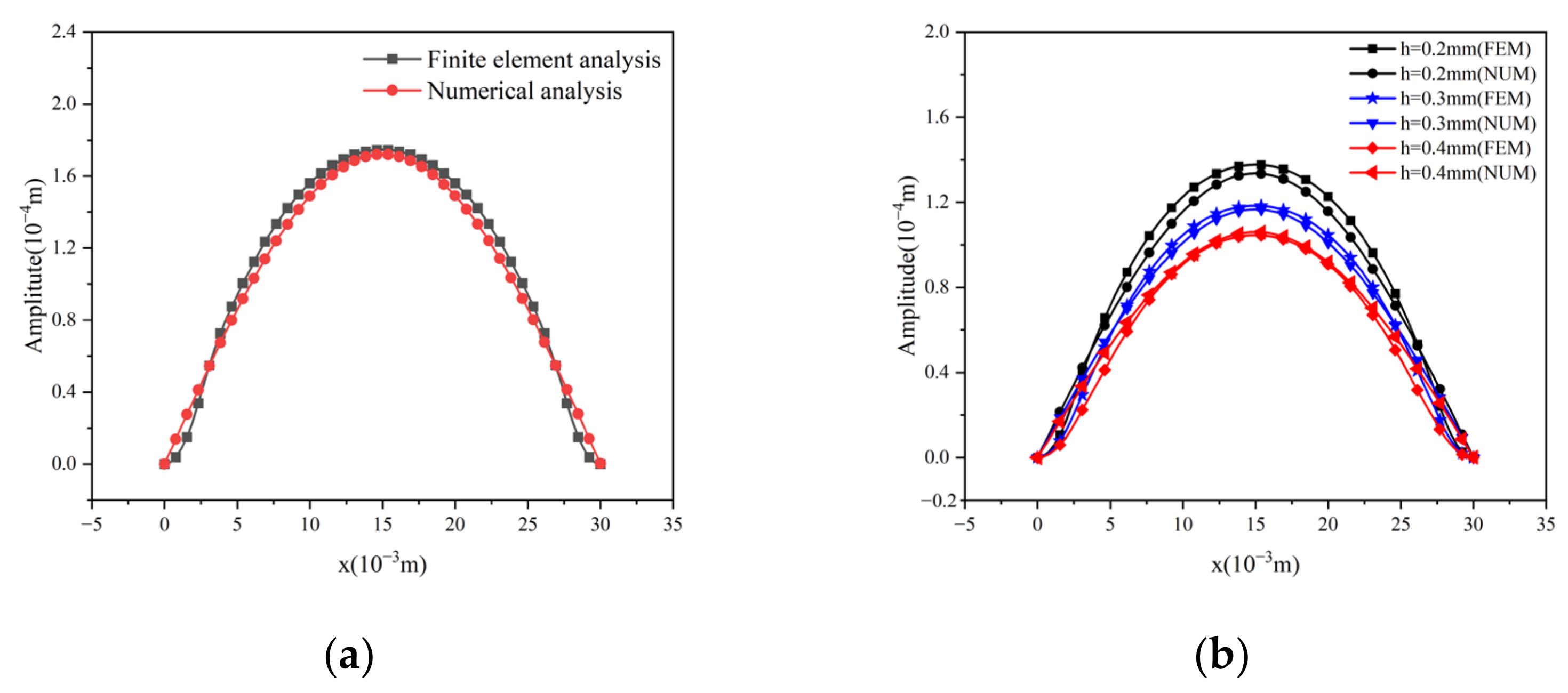
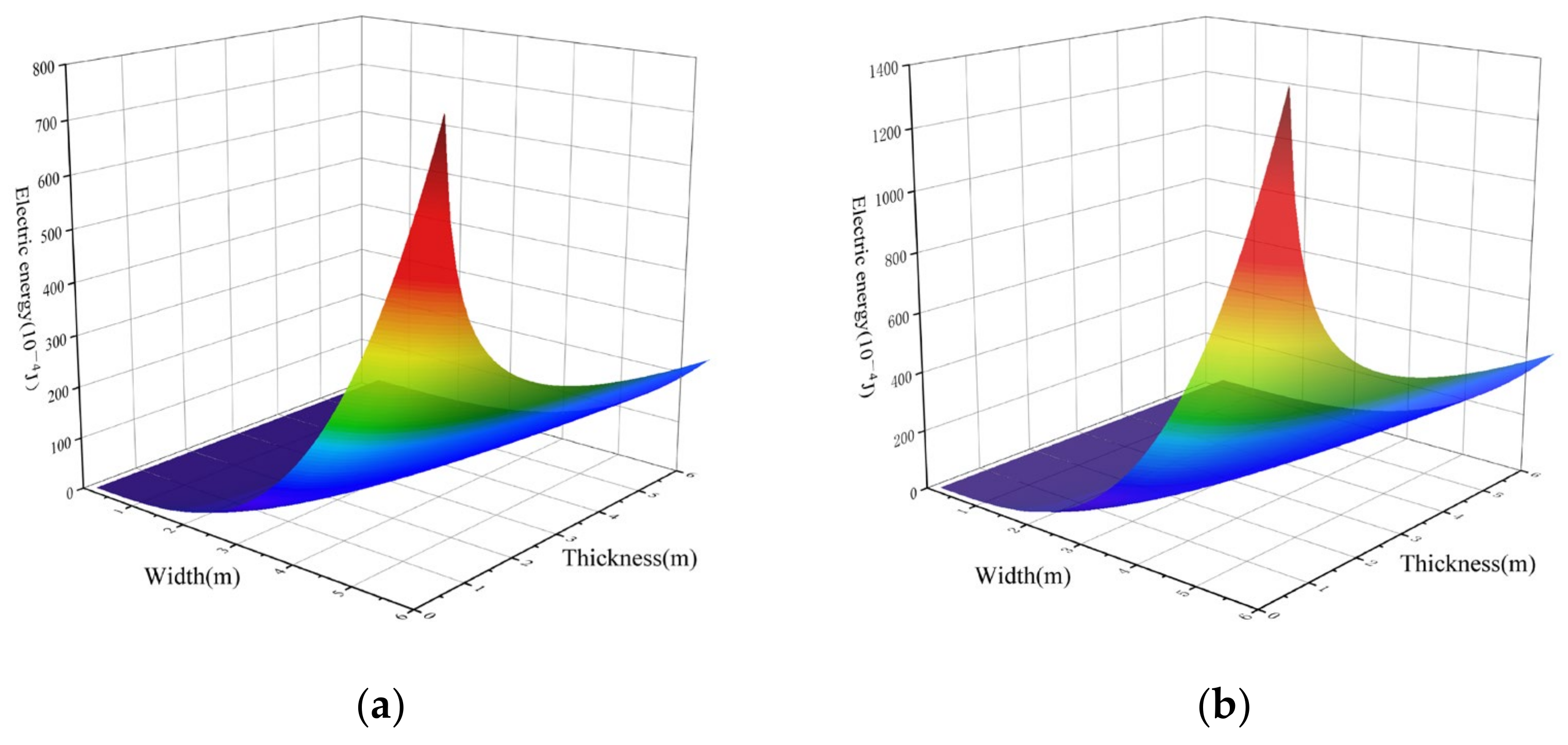
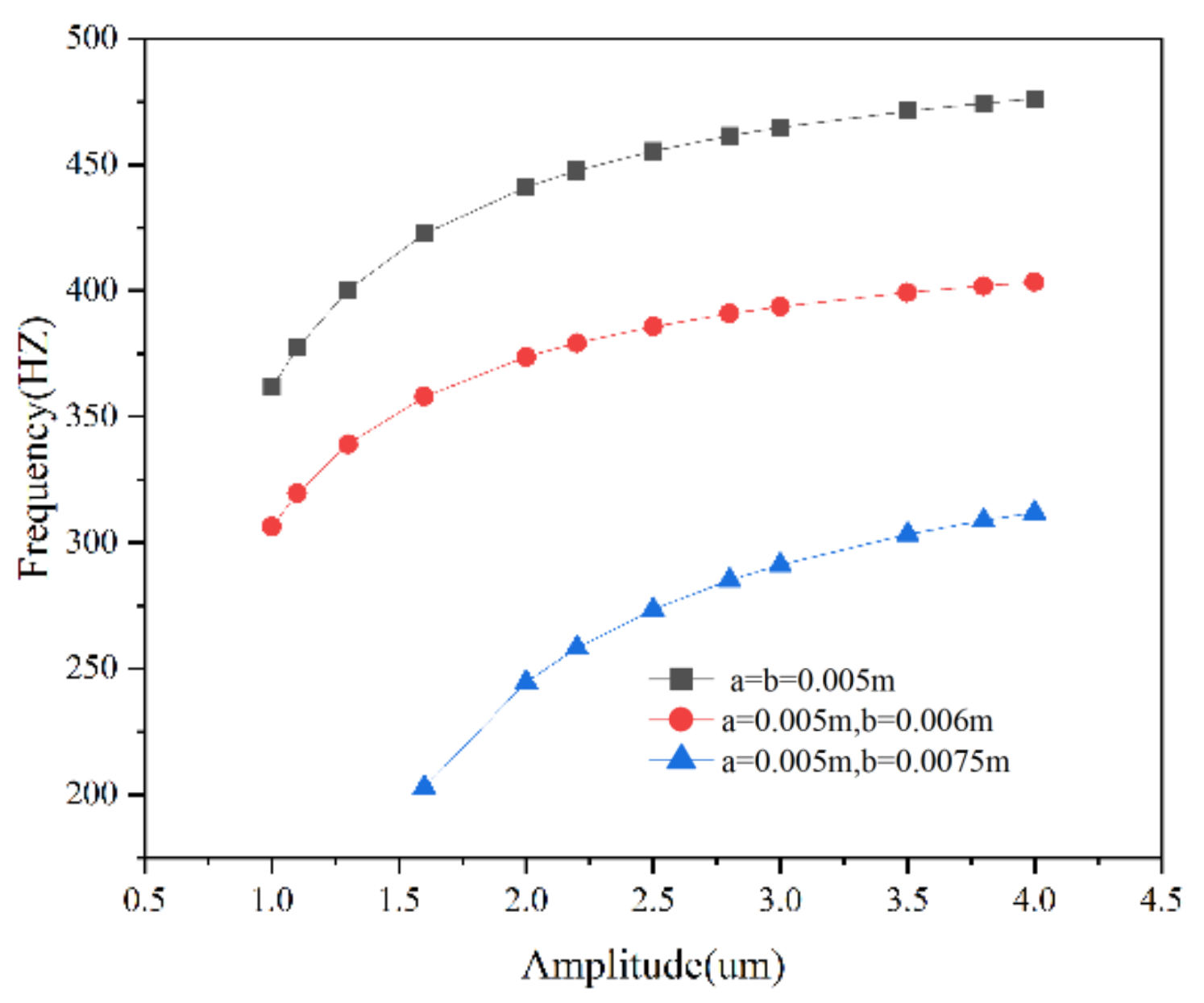
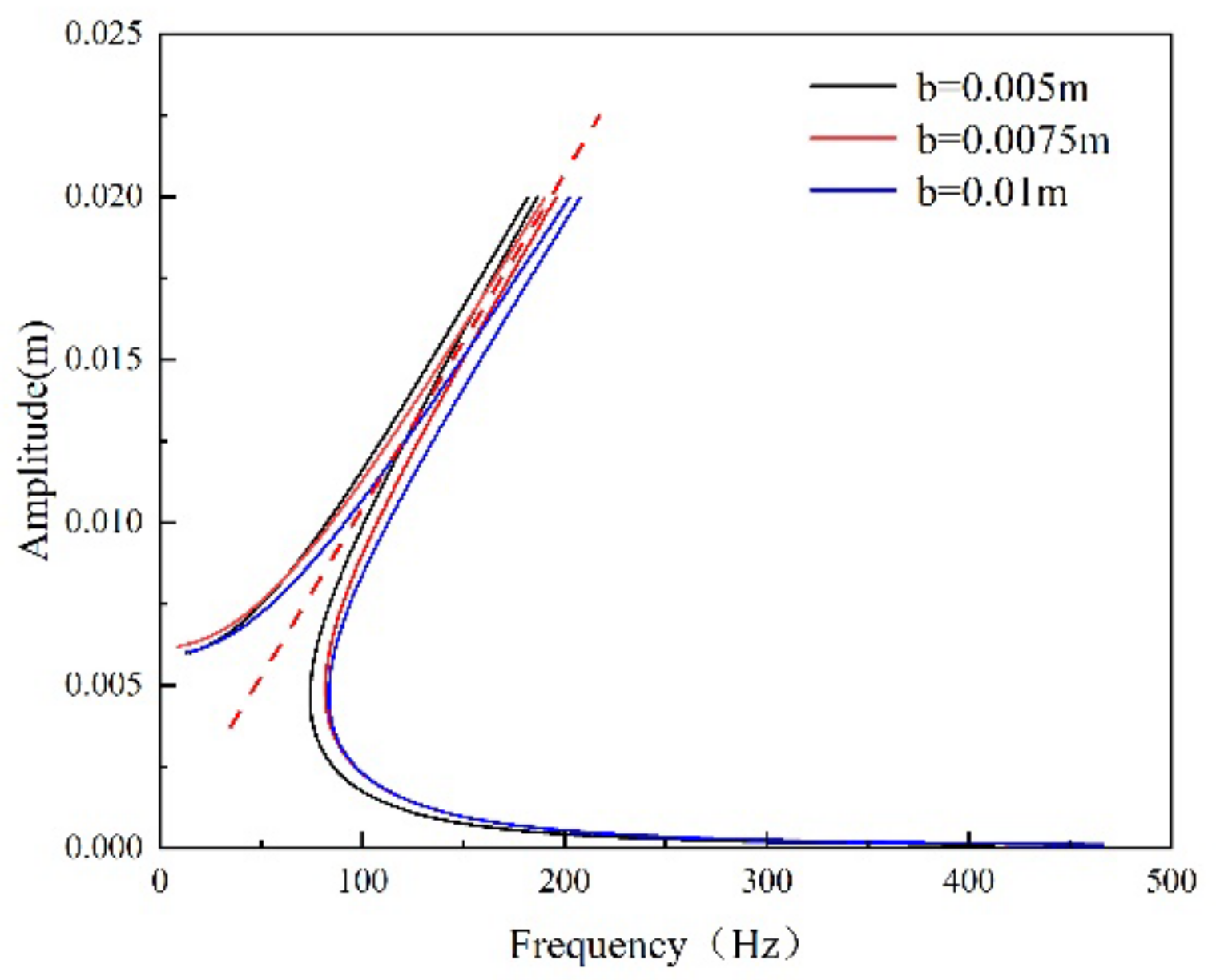
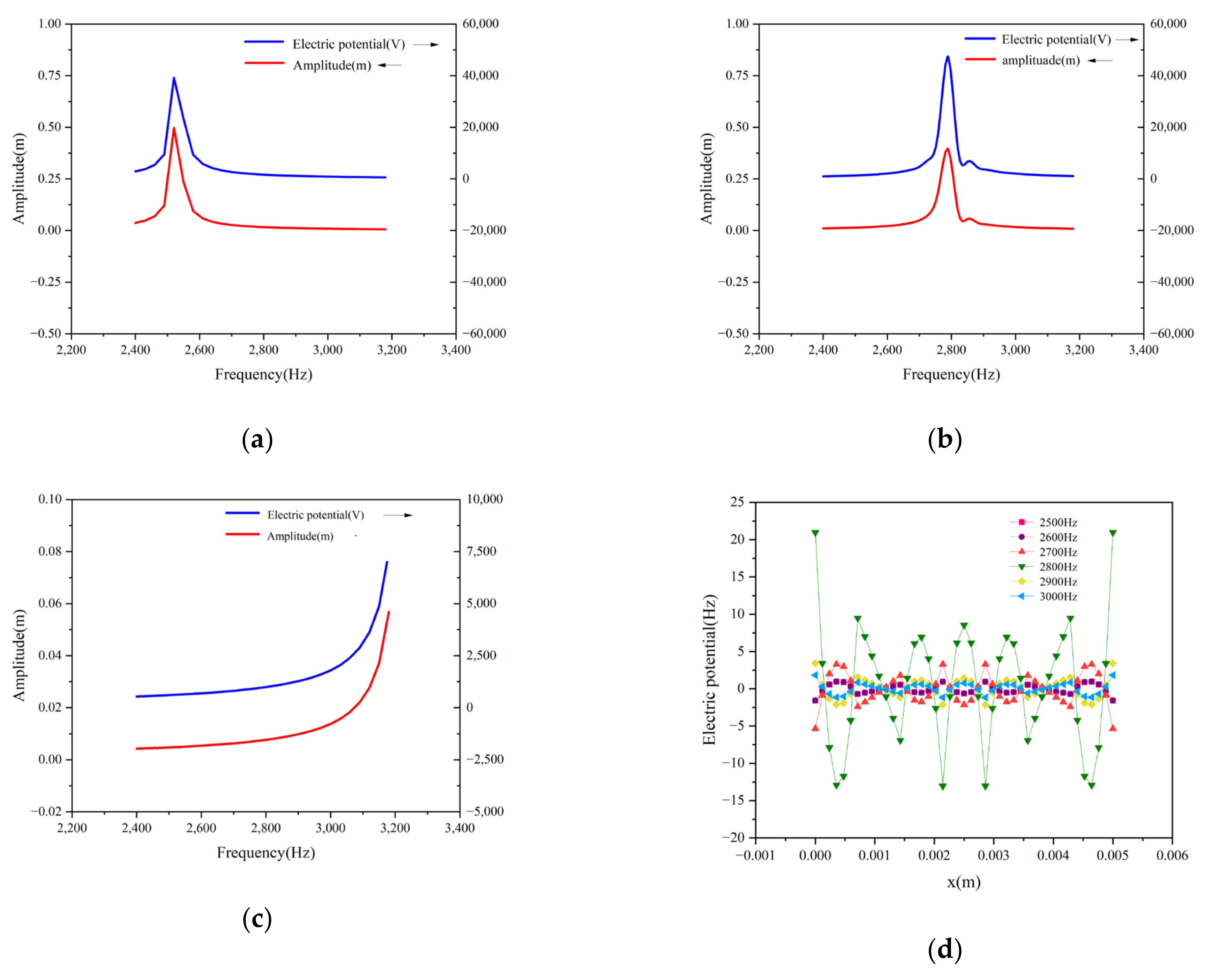
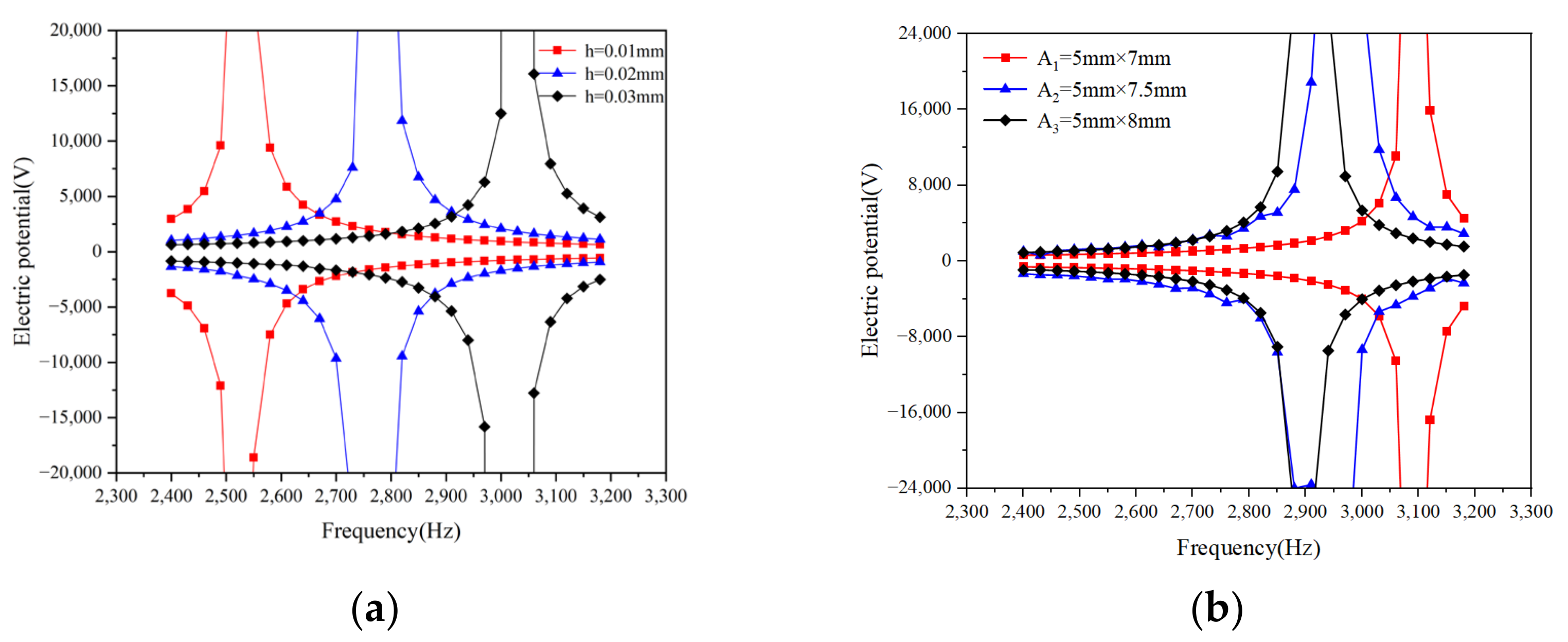
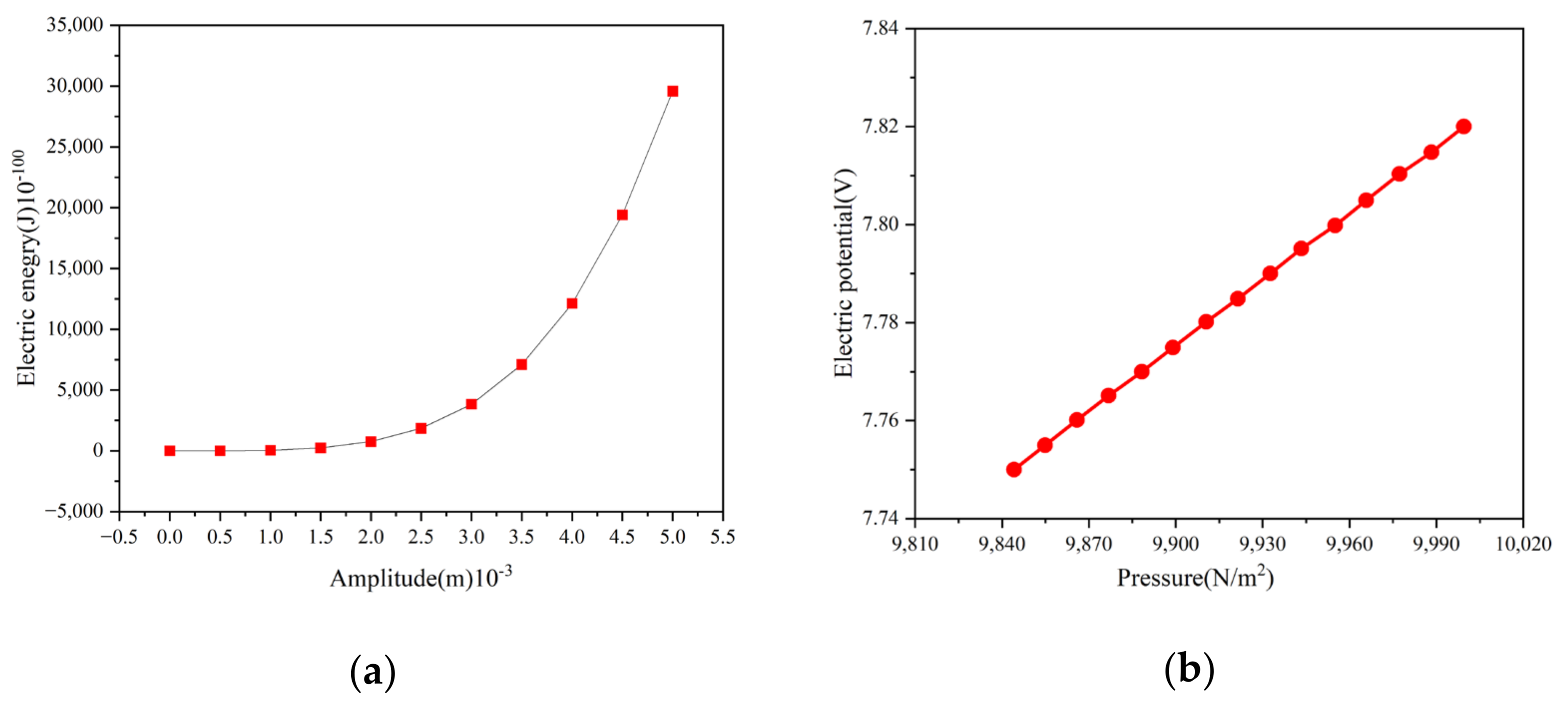
4. Simulation Results and Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Schwartz, G.; Tee, B.C.K.; Mei, J.; Appleton, A.L.; Kim, D.H.; Wang, H.; Bao, Z. Flexible polymer transistors with high pressure sensitivity for application in electronic skin and health monitoring. Nat. Commun. 2013, 4, 1859. [Google Scholar] [CrossRef] [PubMed]
- Mannsfeld, S.C.; Tee, B.C.; Stoltenberg, R.M.; Chen, C.V.H.; Barman, S.; Muir, B.V.; Sokolov, A.N.; Reese, C.; Bao, Z. Highly sensitive flexible pressure sensors with microstructured rubber dielectric layers. Nat. Commun. 2010, 9, 859–864. [Google Scholar] [CrossRef] [PubMed]
- Choong, C.L.; Shim, M.B.; Lee, B.S.; Jeon, S.; Ko, D.S.; Kang, T.H.; Bae, J.; Lee, S.H.; Byun, K.E.; Im, J. Highly stretchable resistive pressure sensors using a conductive elastomeric composite on a micropyramid array. Adv. Mater. 2014, 26, 3451–3458. [Google Scholar] [CrossRef]
- Xie, L.; Zhai, N.; Liu, Y.; Wen, Z.; Sun, X. Hybrid Triboelectric Nanogenerators: From Energy Complementation to Integration. Research 2021, 2021, 9143762. [Google Scholar] [CrossRef] [PubMed]
- Stadlober, B.; Zirkl, M.; Irimia-Vladu, M. Route towards sustainable smart sensors: Ferroelectric polyvinylidene fluoride-based materials and their integration in flexible electronics. Chem. Soc. Rev. 2019, 48, 1787–1825. [Google Scholar] [CrossRef] [PubMed]
- Ribeiro, C.; Sencadas, V.; Correia, D.M.; Lanceros-Méndez, S. Piezoelectric polymers as biomaterials for tissue engineering applications. Colloids Surf. B Biointerfaces 2015, 136, 46–55. [Google Scholar] [CrossRef] [PubMed]
- Giniyatova, S.; Dauletbekova, A.; Baimukhanov, Z.; Vlasukova, L.; Akilbekov, A.; Usseinov, A.; Kozlovskiy, A.; Akylbekovа, A.; Seitbayev, A.; Karipbayev, Z. Structure, electrical properties and luminescence of ZnO nanocrystals deposited in SiO2/Si track templates. Radiat. Meas. 2019, 125, 52–56. [Google Scholar] [CrossRef]
- Deore, S.; Navrotsky, A. Oxide melt solution calorimetry of sulfides: Enthalpy of formation of sphalerite, galena, greenockite, and hawleyite. Am. Mineral. 2006, 91, 400–403. [Google Scholar] [CrossRef]
- Ye, Z.G.; Rivera, J.P.; Schmid, H.; Haida, M.; Kohn, K. Magnetoelectric effect and magnetic torque of chromium chlorine boracite Cr3B7O13Cl. Ferroelectrics 1994, 161, 99–110. [Google Scholar] [CrossRef]
- Krambrock, K.; Pinheiro, M.; Guedes, K.; Medeiros, S.; Schweizer, S.; Spaeth, J.-M. Correlation of irradiation-induced yellow color with the O− hole center in tourmaline. Phys. Chem. Miner. 2004, 31, 168–175. [Google Scholar] [CrossRef]
- Kalendová, A.; Veselý, D.J.P.i.O.C. Study of the anticorrosive efficiency of zincite and periclase-based core–shell pigments in organic coatings. Prog. Org. Coat. 2009, 64, 5–19. [Google Scholar] [CrossRef]
- Xiao, X.; Liang, S.; Si, J.; Xu, Q.; Zhang, H.; Ma, L.; Yang, C.; Zhang, X. Performance of LiTaO3 crystals and thin films and their application. Crystals 2023, 13, 1233. [Google Scholar] [CrossRef]
- Tian, S.; Li, L.; Lu, X.; Yu, F.; Li, Y.; Jiang, C.; Duan, X.; Wang, Z.; Zhang, S.; Zhao, X. Electrical conduction mechanism of rare-earth calcium oxyborate high temperature piezoelectric crystals. Acta Mater. 2020, 183, 165–171. [Google Scholar] [CrossRef]
- Li, X.; Fenu, N.G.; Giles-Donovan, N.; Cochran, S.; Lucas, M. Can Mn: PIN-PMN-PT piezocrystal replace hard piezoceramic in power ultrasonic devices? Ultrasonics 2024, 138, 107257. [Google Scholar] [CrossRef] [PubMed]
- Lee, U.-W.; Baik, J.-H.; Lee, S.-G.; Kang, H.S. Transforming polymeric air filters into high-performance supercapacitor electrodes through carbonization and fluorination. Surf. Interfaces 2024, 46, 104061. [Google Scholar] [CrossRef]
- Kim, N.I.; Yarali, M.; Moradnia, M.; Aqib, M.; Liao, C.H.; AlQatari, F.; Nong, M.; Li, X.; Ryou, J.H. Piezoelectric Sensors Operating at Very High Temperatures and in Extreme Environments Made of Flexible Ultrawide-Bandgap Single-Crystalline AlN Thin Films. Adv. Funct. Mater. 2022, 33, 2212538. [Google Scholar] [CrossRef]
- Gu, Y.; Zhang, T.; Chen, H.; Wang, F.; Pu, Y.; Gao, C.; Li, S. Mini review on flexible and wearable electronics for monitoring human health information. Nanoscale Res. Lett. 2019, 14, 263. [Google Scholar] [CrossRef] [PubMed]
- Kalimuldina, G.; Turdakyn, N.; Abay, I.; Medeubayev, A.; Nurpeissova, A.; Adair, D.; Bakenov, Z. A review of piezoelectric PVDF film by electrospinning and its applications. Sensors 2020, 20, 5214. [Google Scholar] [CrossRef] [PubMed]
- Shin, Y.-H.; Jung, I.; Park, H.; Pyeon, J.J.; Son, J.G.; Koo, C.M.; Kim, S.; Kang, C.-Y. Mechanical fatigue resistance of piezoelectric PVDF polymers. Micromachines 2018, 9, 503. [Google Scholar] [CrossRef]
- Dong, B.; Zhao, R.; Yu, K. Nonlinear combined harmonic resonances of composite cylindrical shells operating in hygro-thermo-electro-magneto-mechanical fields. Compos. Struct. 2024, 331, 117877. [Google Scholar]
- Farokhi, H.; Jamia, N.; Jalali, H.; Taghipour, J.; Khodaparast, H.H.; Friswell, M.I. A nonlinear joint model for large-amplitude vibrations of initially curved panels: Reduced-order modelling and experimental validation. Mech. Syst. Signal Process. 2024, 211, 111239. [Google Scholar] [CrossRef]
- Covaci, C.; Gontean, A. Piezoelectric Energy Harvesting Solutions: A Review. Sensors 2020, 20, 3512. [Google Scholar] [CrossRef] [PubMed]
- Liu, B.; Jiang, Q.; Hu, Y.; Yang, J. High-frequency vibrations of piezoelectric plates driven by lateral electric fields. Int. J. Eng. Sci. 2011, 49, 1435–1442. [Google Scholar] [CrossRef]
- Beeby, S.P.; Tudor, M.J.; White, N.M. Energy harvesting vibration sources for microsystems applications. Meas. Sci. Technol. 2006, 17, R175. [Google Scholar] [CrossRef]
- Kim, H.S.; Kim, J.-H.; Kim, J. A review of piezoelectric energy harvesting based on vibration. Int. J. Precis. Eng. Manuf. 2011, 12, 1129–1141. [Google Scholar] [CrossRef]
- Arscott, S. Skate, overtravel, and contact force of tilted triangular cantilevers for microcantilever-based MEMS probe technologies. Sci. Rep. 2022, 12, 19386. [Google Scholar] [CrossRef] [PubMed]
- Jeung, W.K.; Kim, Y.J.; Choi, S.M. Large displacement out-of-plane bimorph actuator for optical application. Key Eng. Mater. 2006, 326–328, 1383–1386. [Google Scholar] [CrossRef]
- Hashmi, S.A.; Jayatissa, A.H. Modeling of piezoelectric response in PVDF for monitoring of mechanical bending in hinges. In Proceedings of the ASME International Mechanical Engineering Congress and Exposition, Chicago, IL, USA, 5–10 November 2006; pp. 497–502. [Google Scholar]
- Fu, Y.; Harvey, E.C.; Ghantasala, M.K.; Spinks, G.M. Design, fabrication and testing of piezoelectric polymer PVDF microactuators. Smart Mater. Struct. 2005, 15, S141. [Google Scholar] [CrossRef]
- Mo, C.; Radziemski, L.J.; Clark, W.W. Analysis of piezoelectric circular diaphragm energy harvesters for use in a pressure fluctuating system. Smart Mater. Struct. 2010, 19, 025016. [Google Scholar] [CrossRef]
- Mo, C.; Davidson, J.; Clark, W.W. Energy harvesting with piezoelectric circular membrane under pressure loading. Smart Mater. Struct. 2014, 23, 045005. [Google Scholar] [CrossRef]
- Viola, G.; Chang, J.; Steckler, F.; Rojac, T.; Fantuzzi, N.; Song, W. Electromechanical response of poly(vinylidenefluoride) thin films under acoustic stimuli. Mech. Adv. Mater. Struct. 2022, 31, 1377–1387. [Google Scholar] [CrossRef]
- Olha, H.; Yuriy, T.; Maryan, H. Local gradient theory of dielectrics incorporating polarization inertia and flexodynamic effect. Contin. Mech. Thermodyn. 2023, 35, 2125–2144. [Google Scholar]
- Qu, Y.L.; Zhang, G.Y.; Fan, Y.M.; Jin, F. A non-classical theory of elastic dielectrics incorporating couple stress and quadrupole effects: Part I—Reconsideration of curvature-based flexoelectricity theory. Math. Mech. Solids 2021, 26, 1647–1659. [Google Scholar] [CrossRef]
- Chen, W.; Liang, X.; Shen, S. Forced vibration of piezoelectric and flexoelectric Euler–Bernoulli beams by dynamic Green’s functions. Acta Mech. 2021, 232, 449–460. [Google Scholar] [CrossRef]
- Zhang, X.; Hu, K.; Li, H. Comparison of flexoelectric and piezoelectric ring energy harvester. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2019, 233, 3795–3803. [Google Scholar] [CrossRef]
- Wang, Y.J.; Chen, B. Simulating study of cochlear sensors based on PVDF organic polymer materials. Ferroelectrics 2023, 615, 358–368. [Google Scholar] [CrossRef]
- Wang, Q.; Tian, Y.; Yao, M.; Fu, J.; Wang, L.; Zhu, Y. Bimetallic Organic Frameworks of High Piezovoltage for Sono-Piezo Dynamic Therapy. Adv. Mater. 2023, 35, 2301784. [Google Scholar] [CrossRef] [PubMed]
- Iwai, H.; Wang, F. A study on comparing method of motion classification using muscle bulging for control of powered prosthetic hand. Electron. Commun. Jpn. 2023, 106, e12424. [Google Scholar] [CrossRef]
- Li, X.; Im, J.J. Development of an automatic blood pressure device based on korotkoff sounds. Int. J. Adv. Smart Converg. 2019, 8, 227–236. [Google Scholar]
- Kurishima, Y.; Hashimoto, H.; Okuyama, T.; Tanaka, M. Measurement system of swallowing motion by using a piezoelectric film sensor. Int. J. Appl. Electromagn. Mech. 2020, 64, 1173–1182. [Google Scholar] [CrossRef]
- Chu, Y.-F.; Lin, C.B.; Chen, C.S. Pressure measurement in a microchannel. Microsyst. Technol. 2017, 23, 3711–3718. [Google Scholar] [CrossRef]
- Velčić, I. On the general homogenization of von Kármán plate equations from three-dimensional nonlinear elasticity. Anal. Appl. 2017, 15, 1–49. [Google Scholar] [CrossRef]
- Tocci Monaco, G.; Fantuzzi, N.; Fabbrocino, F.; Luciano, R. Critical Temperatures for Vibrations and Buckling of Magneto-Electro-Elastic Nonlocal Strain Gradient Plates. Nanomaterials 2021, 11, 87. [Google Scholar] [CrossRef] [PubMed]
- Hosseini-Hashemi, S.; Azimzadeh-Monfared, M.; Rokni Damavandi Taher, H. A 3-D ritz solution for free vibration of circular/annular functionally graded plates integrated with piezoelectric layers. Int. J. Eng. Sci. 2010, 48, 1971–1984. [Google Scholar] [CrossRef]
- Zhang, Z.; Feng, C.; Liew, K.M. Three-dimensional vibration analysis of multilayered piezoelectric composite plates. Int. J. Eng. Sci. 2006, 44, 397–408. [Google Scholar] [CrossRef]
- Tiersten, H. Linear Piezoelectric Plate Vibrations: Elements of the Linear Theory of Piezoelectricity and the Vibrations Piezoelectric Plates; Springer: New York, NY, USA, 2013. [Google Scholar]
- Duan, W.H.; Quek, S.T.; Wang, Q. Free vibration analysis of piezoelectric coupled thin and thick annular plate. J. Sound Vib. 2005, 281, 119–139. [Google Scholar] [CrossRef]
- Zhao, F. Nonlinear solutions for circular membranes and thin plates. In Proceedings of the Modeling, Signal Processing, and Control for Smart Structures, San Diego, CA, USA, 30 April 2008; pp. 232–243. [Google Scholar] [CrossRef]
- Voorthuyzen, J.; Bergveld, P.J.S. The influence of tensile forces on the deflection of circular diaphragms in pressure sensors. Sens. Actuators 1984, 6, 201–213. [Google Scholar] [CrossRef]
- Timoshenko, S.; Woinowsky-Krieger, S. Theory of Plates and Shells; McGraw-hill: New York, NY, USA, 1959; Volume 2. [Google Scholar]
- Wilson, J.F. Dynamics of Offshore Structures; John Wiley & Sons: Hoboken, NJ, USA, 2003. [Google Scholar]




| PVDF | Numerical Value |
|---|---|
| Density ρ | 1780 kg/m3 |
| Elastic modulus Y | 3.3 GPa |
| Poisson’s ratio | 0.3 |
| −23 × 10−12 Pc/N | |
| 12 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, X.; Zuo, J.; Jiang, T.; Xiao, J.; Tong, J.; Huang, S.; Zhang, W. Analysis of Vibration Electromechanical Response Behavior of Poly(Vinylidene Fluoride) Piezoelectric Films. Energies 2024, 17, 3886. https://doi.org/10.3390/en17163886
Wang X, Zuo J, Jiang T, Xiao J, Tong J, Huang S, Zhang W. Analysis of Vibration Electromechanical Response Behavior of Poly(Vinylidene Fluoride) Piezoelectric Films. Energies. 2024; 17(16):3886. https://doi.org/10.3390/en17163886
Chicago/Turabian StyleWang, Xinyue, Jialin Zuo, Tianlin Jiang, Jinxin Xiao, Jie Tong, Shiqing Huang, and Wenhua Zhang. 2024. "Analysis of Vibration Electromechanical Response Behavior of Poly(Vinylidene Fluoride) Piezoelectric Films" Energies 17, no. 16: 3886. https://doi.org/10.3390/en17163886
APA StyleWang, X., Zuo, J., Jiang, T., Xiao, J., Tong, J., Huang, S., & Zhang, W. (2024). Analysis of Vibration Electromechanical Response Behavior of Poly(Vinylidene Fluoride) Piezoelectric Films. Energies, 17(16), 3886. https://doi.org/10.3390/en17163886







