The Effect of Carbon Nanotubes on the Viscosity and Surface Tension of Heat Transfer Fluids—A Review Paper

Abstract

The connection between surface tension and viscosity has been the subject of several pieces of research on nanofluids. Researchers have discovered differing relationships between these two suspension qualities in the literature. Surface tension and viscosity have been found to be correlated in certain research works but not in other. The behavior of these fluids may be influenced by several factors, including temperature, the presence of surfactants, and the functional groups on carbon nanotubes (CNTs). This study investigates the relationship between surface tension and viscosity in CNT-Nanofluids by reviewing earlier research on the impact of CNT addition on water’s intermolecular interactions. The findings show that depending on different aspects of the nanofluids, the connection is complicated and uncertain. The study shows that although temperature and the addition of carbon nanotubes affect both surface tension and viscosity, other studies only consider how these factors affect one of these qualities. We conclude that under certain heat transfer circumstances, there is no clear-cut relationship between surface tension and viscosity in CNT–water fluids.

1. Introduction

Nanofluids are nanometer-sized particle suspensions that drastically alter the characteristics of the base fluids. High thermal conductivity, variable surface tension, viscosity, and rheology are among the appealing qualities of Nanofluids. Carbon nanotubes (CNT) nanofluids have many applications based on the surface tension and viscosity properties. CNT improves thermal conductivity and controlled viscosity can make CNT nanofluids highly efficient in heat transfer applications [1]. In lubrication: As a result of enhanced viscosity and thermal stability, CNT nanofluids are used in lubrication systems, reducing wear and tear in machinery. The controlled surface tension of the CNT nanofluids is important in drug delivery and fluid behavior in biomedical applications. Surface tension of CNT nanofluids is influenced by the interaction between CNTs and the fluid molecules, particle dispersion, and surfactants. It plays an important role in processes involving fluid interfaces.

Viscosity normally increases with CNT concentration and depends on particle size, temperature, and dispersion quality. The higher viscosity can enhance heat transfer efficiency but may also increase the energy required for pumping [2]. These properties make CNT nanofluids highly versatile for heat transfer applications, advanced thermal management, and lubrication.

Various attempts have been made to comprehend the mechanisms underlying these property changes generated by the addition of nanoparticles, but these theories remain disputed due to a lack of direct nanoscale evidence. Surface tension is the propensity of liquid surfaces at rest to shrink to the smallest feasible surface area. The viscosity of carbon nanotube Nanofluids depends on factors like CNTs concentration, temperature, and fluids composition [3]. Principally, when the concentration of CNTs in water increases, viscosity also increases due to the interaction and entanglement of CNTs within the fluids [4]. Experimental studies specific to the CN–water system would provide more accurate insights into the viscosity of the resultant fluids. Functional groups of nanotubes can influence the viscosity of nanofluids. Functional groups alter the surface chemistry of CNTs, affecting their interactions with the surrounding fluids [5]. As an example, hydrophilic functional groups can enhance dispersion in water-based nanofluids, potentially reducing viscosity [6]. On the other hand, hydrophobic groups may lead to aggregation, increasing viscosity. The specific impact depends on the type, density, and distribution of the functional groups, making it essential to consider the characteristics of CNTs and the fluids investigation for detailed understanding. Surfactants can also influence the viscosity of CNT nanofluids [7]. Depending on the type and concentration of surfactant, the action can enhance CNT dispersion in the fluids, lowering aggregation and perhaps lowering viscosity [8]. However, the impact changes and excessive surfactant concentration may lead to undesirable effects, such as increased viscosity because of micelle formation or other interactions. It is imperative to tune the surfactant concentration to achieve the desired dispersion and viscosity properties for a given CNT’s nanofluid system [9].

This review is the first to the authors’ best knowledge which reviews the research done on both properties: surface tension and viscosity of CNT nanofluids. This review aims to highlight the behavior of viscosity and surface tension of nanofluids as a function of different operating parameters such as temperature and CNT concentration. The surface tension determines the behavior of liquids in a number of processes and phenomena like wetting of a solid by a liquid and viscosity on the other hand relates to shear rate [10]. Both properties affect fluids and suspensions, but what is the effect of adding carbon nanotubes (CNTs) to heat transfer fluids on these properties? Will these properties respond in the same manner, or will they behave differently? Understanding this will help study the impact on both heat and mass transfer of these fluids and provide insights into their behavior. Viscosity and surface tension in CNT nanofluids are interrelated and can influence fluid flow and heat transfer characteristics [7]. They are critical in the design of effective nanofluid systems, and any changes in key factors like CNT concentration, shape, or surface properties can lead to significant alterations in these parameters [11]. Starting with Su et al. [12] reviewed the factors affecting nanofluids’ surface tension. Bhuiyan [13] studied the effect of nanoparticle concentrations and their sizes on the surface tension of nanofluids. Boinovich [14] reviewed the effect of dispersed particles on surface tension, wetting, and spreading of nanofluids but not the viscosity. Rashid et al. [15] analyzed the heat transfer in various cavity geometries with and without nano-enhanced phase change material in a review paper without considering the relationship between surface tension and viscosity Gad et al. [16] conducted a comprehensive review on the nano-sized particles used with diesel and the effects on engine behavior. Rashid et al. [17] prepared a concise review about the progress in phase change nano-emulsions for energy applications. They found that viscosity, sub-cooling, and stability of CNTs are very related. Lu et al. [18] prepared a critical review of dynamic wetting by complex fluids. They summarize the recent studies of the time of dynamic wetting using the viewpoint of the fluids rather than the solid surfaces.

In this work, 567 papers about viscosity and surface tension published between 2022 and 2024 were surveyed [19]. Of these, 74 articles investigated the general concept of nanofluids without directly relating viscosity and surface tension. None of the papers specifically addressed the relationship between the viscosity and surface tension of nanofluids containing carbon nanotubes (CNTs). In this paper, the authors aim to establish a relationship between the viscosity and surface tension of CNT nanofluids, making this study unique. The reviewed articles were published in prestigious journals, such as Energy, Chemical Engineering, Advances in Colloid and Interface Science, Progress in Materials Science, Renewable Energy Reviews, Material Engineering, and others.

2. Theoretical Background of Surface Tension and Viscosity

2.1. Surface Tension

Surface tension allows objects with a higher density than water to float on the water’s surface without being immersed. In Figure 1, surface tension at liquid/air interfaces is affected by the higher attraction of molecules of the liquid to each other called (cohesion) than to the molecules in the air (due to a factor adhesion) [20]. There are two main systems in action here [21].

The first is an inward strain exerted on the surface molecules, which causes the liquid to constrict, and the second is a tangential force parallel to the liquid’s surface. The cohesion forces between the liquid molecules at the molecules as presented in Figure 1 and the adhesive forces between liquid glass and air system are called adhesive forces. Cohesive forces are those forces acting between molecules of the same kind, whereas adhesive forces are those acting between molecules of different types. The resultant forces of the cohesion of the liquid and its adhesion to the material of the container determine the degree of wetting.

The tangential force shown in Figure 1 above is known as surface tension. The liquid behaves as if its surface were covered by a stretched elastic membrane. However, these parallel pressures should not be pushed further apart since the tension in an elastic membrane is dictated by the degree of deformation of the membrane, whereas surface tension is a property of the liquid–air or liquid–vapor interaction. Surface tension is measured in terms of force per unit length or energy per unit area—both are equal [22]. However, when referring to energy per unit of area, the phrase surface energy is commonly used as a broad term in the sense that it also applies to solids.

When a molecule is placed distant from the surface, it is pushed equally in all directions by nearby liquid molecules resulting from cohesive forces with zero net force result. Because the molecules near the surface have adhesive forces and cohesive forces exerting on them, but the inside molecules have only cohesive forces between adjacent molecules, they are pulled inward. This generates internal stress and drives liquid surfaces to compress to the smallest possible area. Resulting from the cohesive structure of water molecules, there is also a tension parallel to the surface at the liquid–air contact that will resist an external force [23].

The equilibrium between the cohesiveness of the wetting degree, the contact angle, and the form of the meniscus can all be determined by the liquid and its adherence to the material of the vessel. When dominant cohesion presents (adhesion energy is less than half of cohesion energy), minimal wetting parents and the meniscus is convex at a vertical wall [24] (as in a glass vessel with mercury).

When adhesion prevails (adhesion energy exceeds cohesion energy), a concave meniscus occurs, like what happens with the water surface in a glass tube. The shape of liquid droplets is determined by surface tension. Although readily distorted, droplets of water are drawn into a spherical shape by the surface layer’s imbalance in cohesive forces. The drops of most of aqueous substances would be nearly spherical in the absence of any additional forces. The spherical form lowers the required “wall tension” of the surface layer, as per Laplace’s law [25]. Surface tension on a drop of water on a damask is high enough to keep the water from seeping through the fabric. Surface tension can also be explained in terms of energy. Compared to when it is isolated, a molecule in contact with another molecule has less energy. The boundary molecules have more energy than the inner molecules because they have fewer neighbors than the interior molecules do. The inside molecules have as many neighbors as possible. Lowering the number of molecules with greater energy barriers is necessary to lower the energy state of the liquid. A smaller surface area is the result of fewer border molecules [26]. The reduction of surface area causes a surface to assume a smooth form. The contour lines, which show the deformation in the water’s surface caused by the metal paper clip, are created by a grille in front of the light. In terms of equations, a liquid’s surface tension is the force per unit length defined as [27]:

$$\mathsf{\gamma }={\displaystyle \frac{\mathrm{F}}{2\mathrm{L}}}$$

(1)

where γ represents the Surface tension (N/m), F is the Force exerted on the fluids (N), L is the Surface unit length (m) as can be seen in Figure 2, The force required to expand the surface area down which is equal to the force (newtons) divided by the length of the container L (m) on both sides and the magnitude of this force is related to the surface tension this force is divided into two components and is half the total force, as the film has two surfaces contributing equally to the force. Hence, the force contributed by a single side (L) denoted F_s equals $\mathrm{F}/2.$ In Figure 2, the force exerted on the fluids inside a container is multiplied by unit distance dx (m), which is the displacement in the fluids because of surface tension, to give the work (N·m). Thus, multiplying both sides by $\mathrm{d}\mathrm{x}:$ [26] gives Equations (2) and (3):

$$\mathrm{F}·\mathrm{d}\mathrm{x}=\left({\displaystyle \frac{\mathrm{F}}{2\mathrm{L}}}\right)·\mathrm{d}\mathrm{x}$$

(2)

$$\mathrm{W}={\displaystyle \frac{\mathrm{F}\mathrm{d}\mathrm{x}}{2\mathrm{L}\mathrm{d}\mathrm{x}}}={\displaystyle \frac{\mathrm{F}\mathrm{d}\mathrm{x}}{\mathrm{d}\mathrm{A}}}$$

(3)

The conventional explanations suggest that this work on the fluids exerted by surface tension is stored as potential energy. As a result, surface tension will also be quantified in SI units as joules per square meter. As the mechanical systems seek the state with the lowest potential energy, a free droplet of liquid spontaneously acquires a spherical form, which has the smallest surface area for a given volume [29]. When an item is put into a liquid, its weight is referred to as F_w (which is the mass multiplied by the gravitational acceleration), this item addition un-presses the surface, and equalizes surface tension and downward forces. The surface tension forces on each side are denoted (F_s) and equal to half the total force on both sides of film, both parallel to the water’s surface at the locations it balances the item. However, a little movement in the item might cause it to sink. The surface tension diminishes as the angle of contact is deduced. The horizontal components of the two F_s, which are half the total force multiplied by the cosine of the contact angle theta arrows, act in opposite directions, canceling each other, while the vertical components point in the same direction and add up to balance F_w [27]. For this to occur, the object’s surface must be wettable, and its weight must be low enough for the surface tension to hold it. In Figure 3, m is the floating object mass, g is the acceleration because of gravity, and using the Equation (1) above, we obtain [29]:

$$weight=2{·F}_s·\mathit{sin}\theta , or m·g=2·\gamma ·L·sin\theta$$

(4)

Numerous variables related to the liquid’s characteristics, its surroundings, and the presence of other materials might affect surface tension. The following are the main variables that impact surface tension:

• The liquid molecular structure’s nature: Water and other liquids with strong intermolecular interactions, like hydrogen bonds, typically have higher surface tensions. Surface tension is lower in non-polar liquids with fewer intermolecular interactions.
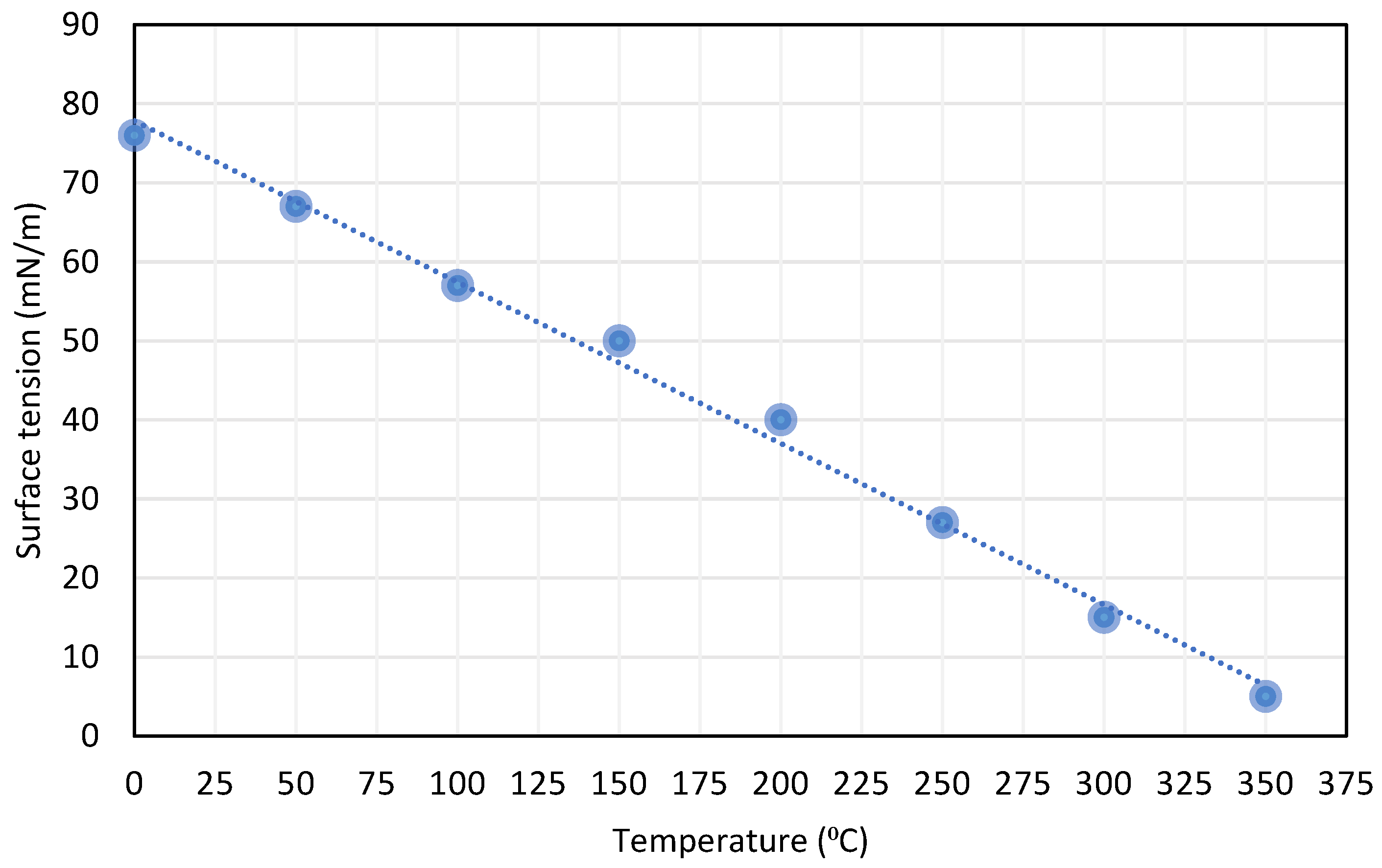
• Temperature: Molecules increase kinetic energy as the temperature rises, lowering the cohesive forces at the liquid’s surface. Tension typically falls as temperature rises.

When a number for an interface’s surface tension is given, the temperature must be explicitly indicated. The overall tendency is that surface tension falls with increasing temperature, eventually reaching zero at the critical point. They are only connected with the empirical formula called the Eötvös rule [27] and are shown in Equation (5) below:

$$\gamma V^{2/3}=k(T_c-T)$$

(5)

where γ is the surface tension, V is a substance’s molar volume, T_C is its critical temperature, and k is a constant that applies to practically all substances [5].

Common values are k = 2.1 × 10⁻⁷ J K⁻¹ mol^−2/3 [31].

V = 18 mL/mol [3].

T_C = 647 K (374 °C) can also be used for water [31].

Table 1. Common Liquids Surface tension values.

Liquid	Surface Tension γ (N/m)	Ref.
Water at 0 °C	0.0756	[26]
Water at 20 °C	0.0728	[26]
Water at 100 °C	0.0589	[26]
Soapy water (typical)	0.0370	[26]
Ethyl alcohol	0.0223	[32]
Mercury	0.465	[26]
Olive oil	0.032	[26]
Tissue fluids (typical)	0.050	[26]
Blood, whole at 37 °C	0.058	[26]
Blood plasma at 37 °C	0.073	[26]
Gold at 1070 °C	1.000	[26]
Benzene	0.0282	[32]
Oxygen at −193 °C	0.0157	[26]
Helium at −269 °C	0.00012	[26]

below lists common liquids’ surface tensions in N/m at different states. It is clearly shown that surface tension of water decreases from 0.0756 N/m (at 0 °C) to 0.0589 N/m (at 100 °C).

2.2. Vescosity

Fluids’ viscosity is a concept that describes a fluid’s resistance to deformation at a particular rate [32]. It relates to the colloquial sense of “thickness” in liquids. It quantifies the internal friction reactions within adjacent fluids layers with their relative motion [33]. Viscosity can be mathematically described as force multiplied by time divided by unit area. As a result, its SI units are newtons per square meter or pascal seconds [34].

Viscosity is affected by the state of the fluids, temperature, pressure, and rate of deformation. At very low temperatures, viscosity close to zero (no resistance to shear stress) is observed in superfluids; otherwise, the second law of thermodynamics requires all fluids to have positive viscosity [35,36]. Ideal or inviscid fluids have zero viscosity (are non-viscous).

The fact that both viscosity and liquid surface tension are related to fluid characteristics is one commonality between them. From then on, things become enigmatic. As previously mentioned, surface tension is the ability of a liquid’s surface to withstand force; it serves as a barrier to other substances, retaining the liquid molecules together. Unbalanced forces on surface molecules that draw toward the liquid’s center are the source of this omnipresent feature.

Viscosity-wise, the fluids are categorized into Newtonian and non-Newtonian fluids [37]:

• Newtonian fluids are those that respond to shear forces by moving the liquid in a straight-line fashion.
• Non-Newtonian fluids, on the other hand, obey a separate set of laws. The viscosity of shear-thinning fluids decreases as the pressure or force increases. Thixotropic fluids’ viscosity changes with time. Gels like ketchup, for example, are stable at repose but become fluids when disturbed.
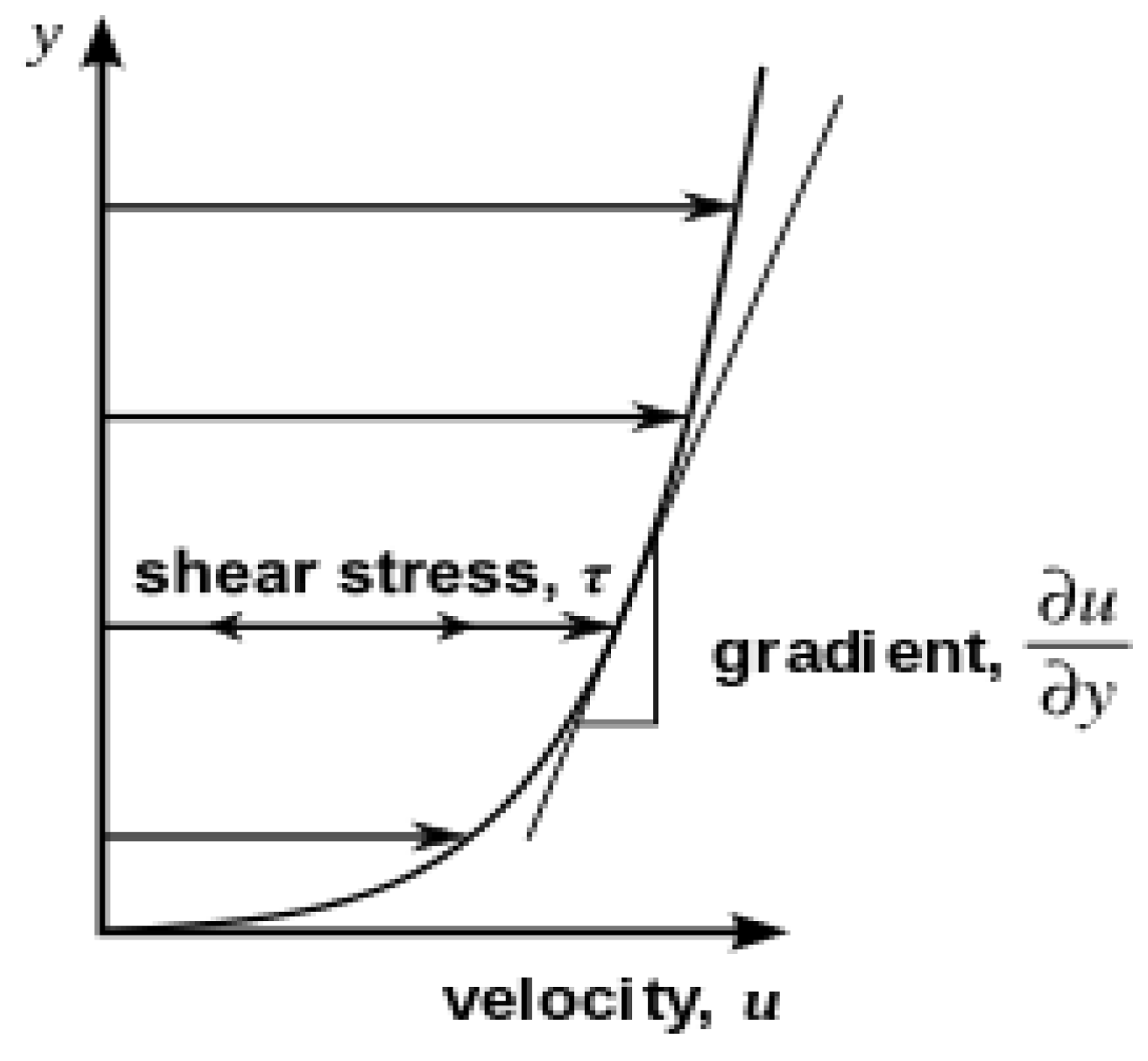
• The term viscosity is divided into two types: dynamic and kinematic viscosity. Materials science and engineering are interested in dynamic viscosity (forces or stresses involved in the deformation of a material). In a fluid such as water, the stresses caused by shearing the fluids do not depend on the distance sheared; rather, they depend on the fluid itself. This is what we mean by dynamic viscosity, and it can be presented by the following equation, named Newton’s Law of Viscosity [37].

  $$\mathrm{F}=\mathrm{\mu }{\displaystyle \frac{\mathrm{A}.\mathrm{d}\mathrm{u}}{\mathrm{d}\mathrm{y}}}$$

  (6)

  where du/dy is the rate of shear deformation, F is the shear force (N), A is the unit area (m²), µ is the dynamic viscosity (kg/m/s) or (Pa·s(, u is the velocity (m/s), and y is the separation distance (m). Figure 4 below shows the parallel flow corresponding to the shear stress proportional to the velocity gradient of the fluid’s shear rate deformation, dividing the force by the unit area to obtain τ (N/m²) as follows [38]:

  $$\tau =\mu {\displaystyle \frac{du}{dy}}$$

  (7)

In fluid dynamics, it is more acceptable to work in terms of kinematic viscosity or momentum diffusivity, defined as the ratio of dynamic viscosity ϻ over fluids density ρ. This is commonly represented by the Greek letter ν (m²/s).

A solid particle suspension’s effective viscosity, or ϻ_eff, can be expressed in terms of averaged stress components over a large volume relative to the distance between the suspended particles yet are small in macroscopic dimensions [40]. Under certain conditions, non-Newtonian behavior is typical. On the other hand, dilute systems behave in a Newtonian manner in steady flows, and effective viscosity can be readily obtained using equations for particle dynamics. In an environment with a volume percentage of φ ≲ 0.02 that is highly diluted, one can disregard interactions between suspended particles. In this case, it is possible to compute the flow field directly around each particle, and then combine the results to yield [40]:

$${\displaystyle \frac{{\mathrm{ϻ}}_{\mathrm{e}\mathrm{f}\mathrm{f}}}{{\mathrm{ϻ}}_0}}=1+2.5\mathrm{ø}$$

(8)

where ϻ₀ is the solvent viscosity, ϻ_eff is the suspension effective viscosity, and ø is the concentration of the CNTs presented in the base fluids in percentage weight. The linear dependence on the concentration is a consequence of neglecting inter-particle interactions. For dilute systems, the relation is as in Equation (9) [40]:

$${\displaystyle \frac{{\mathrm{ϻ}}_{\mathrm{e}\mathrm{f}\mathrm{f}}}{{\mathrm{ϻ}}_0}}=1+\mathrm{B}\mathrm{ø}$$

(9)

where the coefficient B may depend on the particle shape. However, experimental determination of the precise value is difficult: even the prediction (B = 5/2) for spheres has not been conclusively validated, with various experiments finding values B in the range (1.5 ≲ B ≲ 5). This deficiency has been attributed to the difficulty in controlling experimental conditions.

In higher-concentration suspensions, the effective viscosity develops a nonlinear dependency, indicating the importance of inter-particle interactions. There are several analytical and semi-empirical approaches for capturing this regime Equation (10) [40],

$${\displaystyle \frac{{\mathrm{ϻ}}_{\mathrm{e}\mathrm{f}\mathrm{f}}}{{\mathrm{ϻ}}_0}}=1+{\mathrm{B}}_1\mathsf{\phi }+{\mathrm{B}}_1{\mathrm{ø}}^2$$

(10)

B₁ is taken from experimental data or approximated from microscopic theory.

Casaos et al. [2] conducted a comparison between the experimental data obtained from a capillary viscometer and physical models that predict the features of nanoparticles, such as aspect ratio. He presented a universal curve that may predict the viscosity of any one-dimensional or two-dimensional nanoparticle in suspension, which leads to a basic knowledge of the motion modes for carbon particles.

Ahadian performed a presentation of his investigation for a procedure by which he predicted the viscosity and surface tension of liquids [23]. Depending on particle size, bulk density, packing density, surface free energy, and capillary rise time. The results showed that the artificial network approach used could predict the surface tension. Ghosh [41] found that the surface activity of these molecules is determined by the length of the hydrocarbon chain and the type of the head group(s). Amphiphiles with longer hydrocarbon chains were discovered to be more surface-active than those with shorter hydrocarbon tails. Amphiphiles with fluorocarbon chains were shown to be more surface active than those with hydrocarbon chains because the fluorocarbon chain is more hydrophobic than the hydrocarbon chain. Kumar [11] presented heat transfer results on single-walled CNT suspensions in a boiling environment and its effects. He found that the surface tension relaxation of the nanofluids has a strong function with material burnout. When CNTs are added to deionized water with surfactant, the surface tension is close to that of deionized water, which was explained by the adsorption of ionic surfactant (NADBS) on SWNTs, which resulted in the stabilization of the nanotubes in the solution. Ahmari [4] modified and developed a new imperial formula relating surface tension and viscosity, which is given by Equation (11).

$$\mathsf{\gamma }{\displaystyle \frac{({\mathrm{T}}_{\mathrm{c}}-{\mathrm{T}}_{\mathrm{m}})}{({\mathrm{T}}_{\mathrm{c}}-\mathrm{T})}}=\mathsf{\delta }(1-{\displaystyle \frac{\mathrm{B}}{\mathsf{\eta }}})$$

(11)

where γ is the surface tension, δ and β are constant; T_c critical temperature, T_m is the melting temperature T is the fluid’s temperature (kelvin), η is viscosity. The formula suggested that the surface tension and the viscosity are directly proportional to each other. The higher the viscosity is the higher the surface tension. The relation shows that both the viscosity and the surface tension are interrelated. Surface tension is a strong function of surfactants. The author emphasizes the fact that any relation between the surface tension and viscosity can be valid in the absence of the surface-active agent and capillarity.

3. Results and Discussion

It can be challenging to calculate the viscosity’s true value. Viscosity is all about dynamism and movement, in contrast to surface tension, which is a static phenomenon. Viscosity is a measure of the internal frictional force between adjacent fluid layers moving relative to one another [41]. A viscous fluid, for instance, flows more quickly toward the axis of a tube than it does toward its walls as it is pushed through. Experiments show that to maintain the flow, there must be some stress (such as a pressure difference between the tube’s ends). This is because a force is required to overcome the friction created by the flowing fluid layers. In a tube with a constant rate of flow, the viscosity of the fluids affects the strength of the compensating force.

3.1. Factors Affecting Surface Tension

3.1.1. Temperature

The temperature effect on the surface tension: As the temperature increases, the surface tension decreases in a linear relationship. The effectiveness of intermolecular attraction decreases because the kinetic energy increases due to temperature increase [42], as shown in Figure 5.

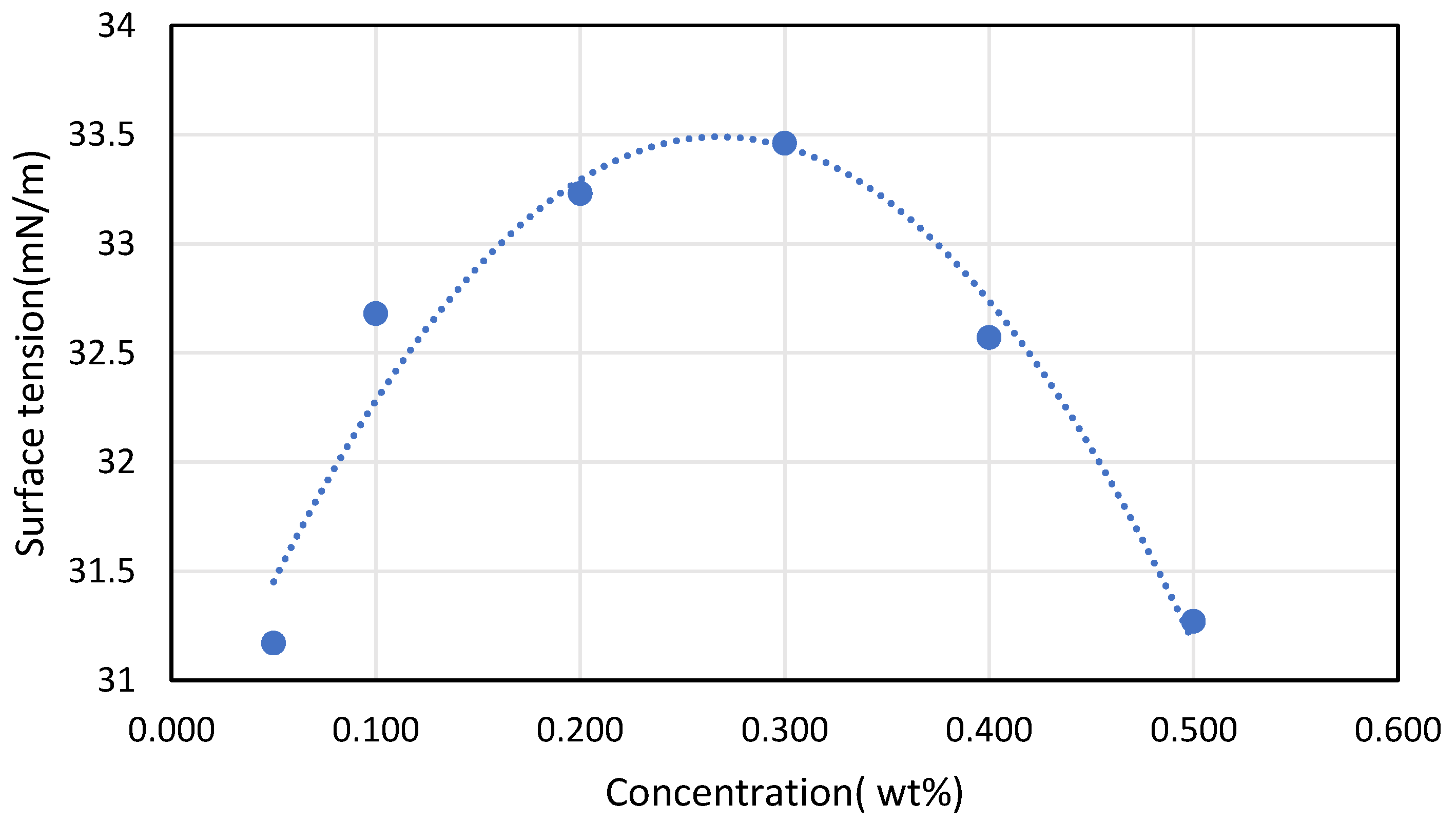
3.1.2. Concentration

The effect of solute concentration depends on the characteristics of the surface and the solute, solutes can have varying effects on surface tension: Most organic compounds found in the literature have little or no effect on surface tension [43], but most inorganic salts in water-air increase surface tension. Most inorganic acids undergo non-monotonic alteration when exposed to water-air [44]. As with most amphiphiles, such as alcohols in water–air, gradually reduce surface tension. Surfactants that create micelles reduce surface tension until a threshold concentration is reached, then have no effect [41].The fact that a solute might exist in a different concentration at the surface of a solvent than in its bulk complicates the effect. These differential changes depend on the solute-solvent mix [44].

Soleimani et al. [45] founded the opposite he found that the increase in CNTs concentration will increase the surface tension because of an increase in inter-molecular forces after that a drop in the surface tension will happen. This is presented in Figure 6, where the maximum concentration reached was 0.3% weight afterward the surface tension drops dramatically, which contradicts the before-mentioned reference. This leaves the concentration-surface tension relation undeterminable. Bhuiyan et al. [13] found that surface tension of the nanofluid enhances from 2.62% to 4.82% in comparison with the base fluids for concentration variation of 0.05 Vol % to 0.25 Vol % at 25 °C in his studying of the effect of nanoparticles concentration and their sizes on the surface tension of nanofluids.

Berrada et al. [46] found an opposite tendency regarding surface tension with MWCNT concentration for the two used base fluids: decreasing surface tension was observed with distilled water as base fluids and a significant increase in surface tension was observed with Tyfocor as a base fluid [46], this leads to the result that there is no solid understanding of surface tension concentration relation. In a study of the effect of volume concentration and temperature on viscosity and surface tension of graphene–water nanofluid for heat transfer applications. Berrada et al. [46] found a variation in variation in surface tension with temperature for various volume concentrations. The surface tension decreases with an increase in both volume concentration and temperature. The values of the surface tension of the nanofluid at 10 and 90 degrees centigrade were 65 and 52.2 mN/m, respectively, for 0.15 percent volume concentration, which were lower by 13.8 and 13.7 percent when compared with that of the deionized water [47].

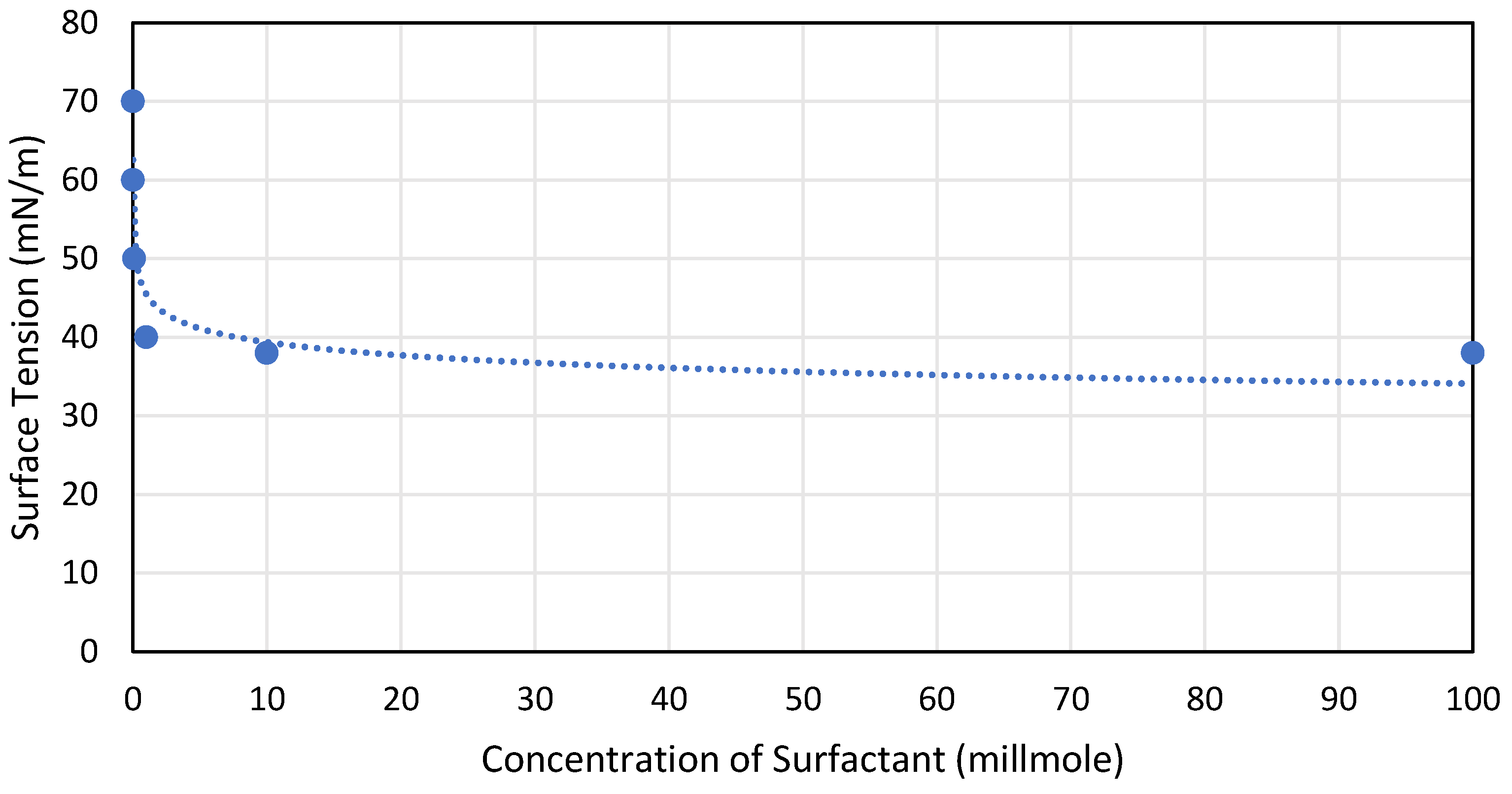
3.1.3. Surfactant

Surface tension is decreased when surfactants, or surface-active substances, such as detergents or soaps, are added to a liquid. The cohesive forces between molecules at the surface are lessened by surfactants as the molecule’s forces are leasing the surface tension becomes less, as can be seen in Figure 7. The surface tension is almost constant at 40 mN/m beyond 10 millimoles of surfactant.

Depending on their makeup, additional impurities can either raise or lower surface tension. For instance, salts can raise the surface tension of water, but alcohol lowers it [47]. The relation is clear that surface tension decreases as surfactant concentration increases. Some findings from the literature can be listed as follows:

➢

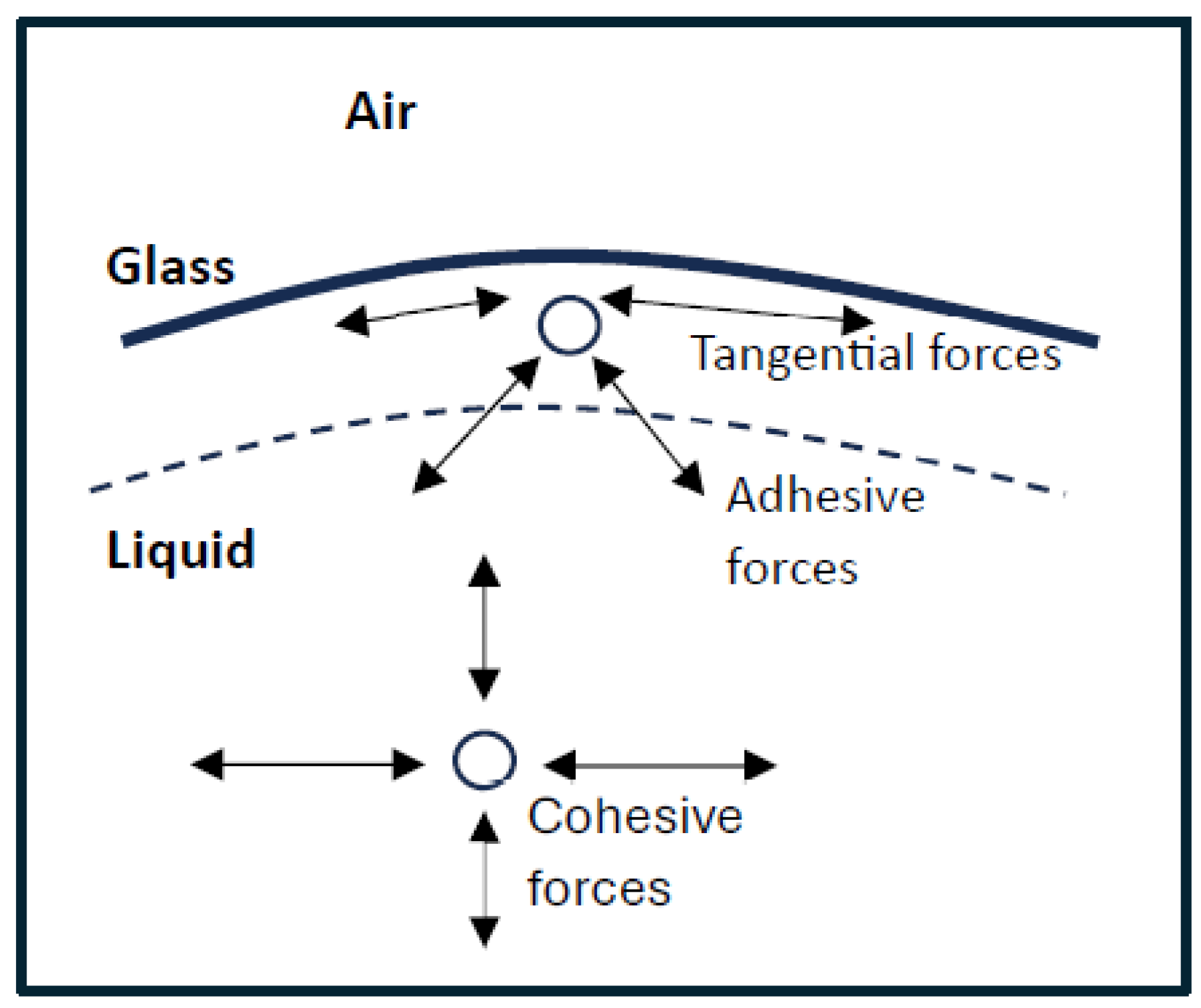
Forces’ interactions with molecules: Strong cohesive forces, like hydrogen bonds and van der Waals forces, between molecules in a liquid increase surface tension.

Adhesive forces between the liquid and the container of glass might affect the apparent surface tension of the liquid when it comes to capillary action, as an example.

➢

Dissolved gases: A liquid’s surface tension can be affected by the type and quantity of present gases dissolved in it [48].

➢

External pressure: surface tension is normally insensitive to changes in external high pressure can affect the surface tension noticeably. Vapor pressure of the liquid in volatile liquids tends to have higher vapor pressures because of their greater propensity to escape into the gas phase. This means that higher vapor pressure can decrease surface tension [49].

➢

Area of surface: Surface area can also affect surface tension. Based on the Laplace differential pressure between the inside and the outside of a curved surface that forms the boundary between two fluid regions. The pressure difference resulted from surface tension as an interface between liquid and air, or immiscible liquids’ surface curvatures in liquid droplets will affect surface tension; nevertheless, this is more of an issue to be considered rather than having a direct impact on the underlying surface tension, on the understanding of phenomena such as capillarity [4,50].

Figure 7. Effect of surfactant concentration on surface tension, reproduced from [51].

Figure 7. Effect of surfactant concentration on surface tension, reproduced from [51].

➢

Electric field influence: The surface tension of a liquid can be altered by the application of an electric field. This is especially important in electro-wetting, where an electric field alters a droplet’s surface tension on a surface [52].

➢

Temperature gradients: Temperature gradients are called the Marangoni Effect, which means differences in surface tension can induce fluid motion, or Marangoni flow when there is a temperature gradient across a liquid’s surface [46].

More results from the literature are presented in

Table 2. General results from the review.

Author	Findings
[46]	A decrease in surface tension with temperature for nanofluids was examined, and an opposite tendency regarding surface tension was obtained regarding surface tension with MWCNT concentration, the same for distilled water, but an increase was observed with Tyfocor anti frees as a base fluid instead of water.
[52]	Showed that the nanoparticle wettability is responsible for the modified surface tension. Because hydrophobic nanoparticles always tend to be on the free surface as they behave like surfactants and reduce surface tension.
[53]	Found a simple correlation that can predict the rheological behavior of graphene/water nanofluids and compared it to the experimental results which confine
[31]	Found that experimental results suggested adding CNTs to cementitious composites reduces the flow index while increasing the critical shear rate. CNTs with sub-micrometer lengths and tiny diameters provide high-yield stresses and low viscosities to cementitious composites.
[54]	Presented in his paper that the concentration increment of CNT will increase the viscosity as can be seen in Figure 8 Below. This makes sense as more particles are closing the gap between the molecules of the base fluids making them move tightly.
[55]	Presented that the relative viscosity (ηr) is a function of concentration (C) of MW CNTs at 5 different temperature settings. The fitting line was calculated with the Maron–Pierce model, and the relation was directly proportional.
[56]	Mentioned in his paper that the nanofluids have good properties such as high thermal conductivity and low viscosity with high photo-thermal properties that are attractive for thermal applications. This contradicts all the literature that says that the CNT increases the viscosity.
[56]	Mentioned that the nanofluids have a reduced pumping power as compared to pure liquid to achieve equivalent heat transfer intensification.
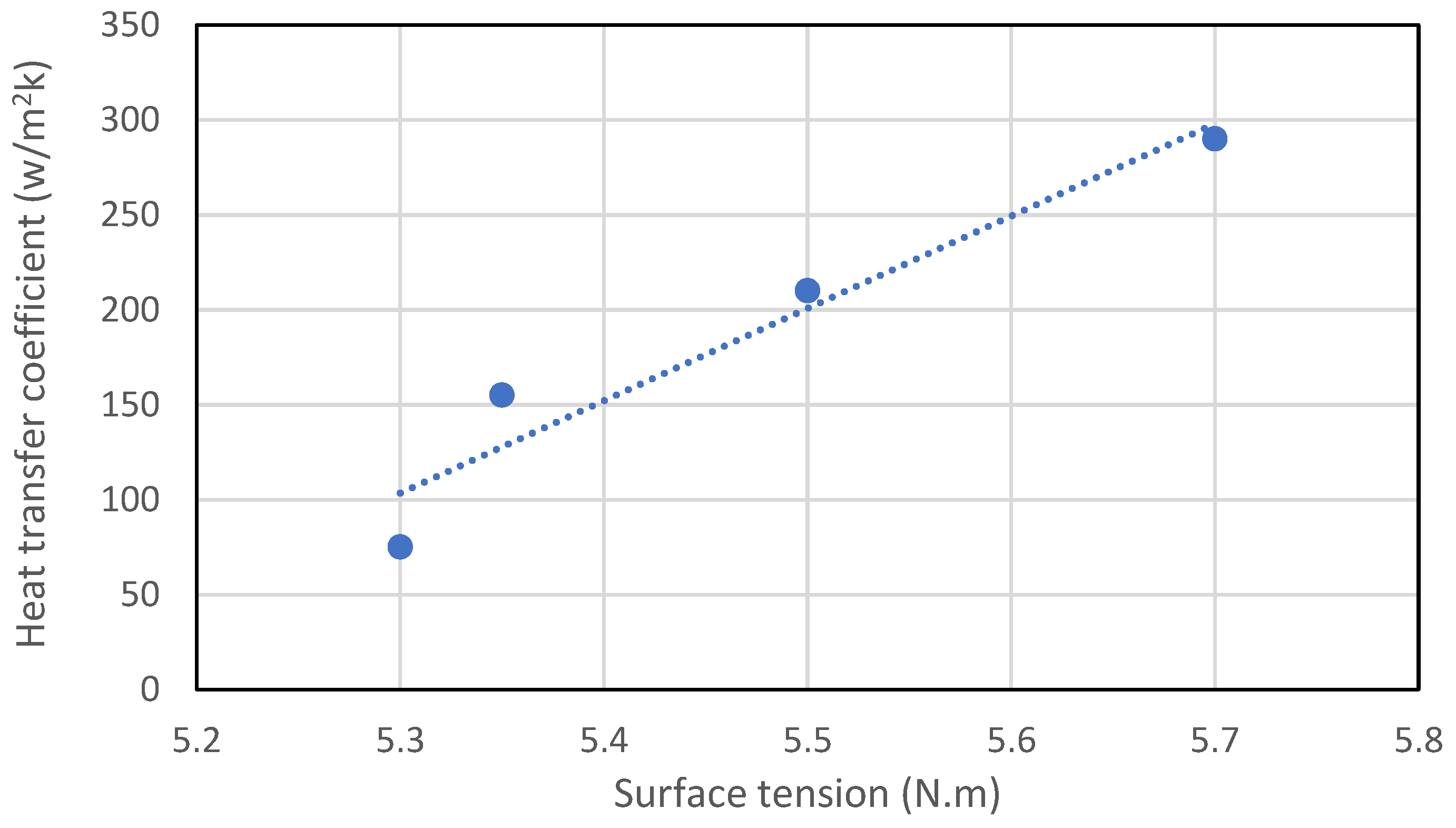
[57]	Shows that the surface tension relation with the heat transfer coefficient, which is linearly increasing, as in Figure 9.
[58]	Explores the properties of both viscosity and surface tension, he also highlighted the distinctions and occasional interactions between them.
[59]	Experienced the theoretical and experimental underpinning and observations of the relationship—or lack thereof—between the two properties.
[60]	In this book chapterthe auther discussed both surface tension and viscosity in depth, including their definitions, significance, and interactions.
[61]	This author discussed the nuances of viscosity and all fluid properties without establishing a strict connection to surface tension.
[62]	This author reviewed and discussed theoretical models and experiments regarding fluid properties, providing the complexities of the relationship between surface tension and viscosity.

:

4. Future Research Prospectives

About surface tension and viscosity of (CNT) nanofluids are critical in understanding their unique fluid dynamics and applications in mass and heat transfer, energy storage, and industrial processes. These properties, surface tension, and viscosity, play a pivotal role in the performance of CNT nanofluids in these contexts in terms of the following:

The relationship between surface tension and viscosity in CNT nanofluids offers a wide range of opportunities for future research, especially as they become more relevant to advanced industrial applications. Systematic experimental studies, enhanced modeling, and a focus on optimizing these properties for specific applications will drive innovation in the use of CNT nanofluids across various fields as listed in

Table 3. Findings on future research prospectives.

Item No.	Findings
1	CNT Characteristics on Surface Tension and Viscosity CNT Dimensions: Future research can systematically study how different CNT sizes impact both parameters and how these variables can be optimized for different applications [63]. Varying the diameter, length, and CNT aspect ratio lead to different surface tension and viscosity properties [63]. CNT Functionalization: Different types of functionalization impact on both surface tension and viscosity v can be explored. For instance, hydrophilic or hydrophobic groups could lead to significant variations in behavior. Functionalizing CNTs (adding chemical groups to their surface) alters their interaction with the base fluid. CNT Dispersion Stability: The dispersion quality of CNTs in the base fluid directly impacts both surface tension and viscosity. Poorly dispersed CNTs tend to aggregate, affecting the fluid’s bulk properties. Advanced dispersion techniques and stabilizing agents could provide key insights into achieving desired fluid properties. In order to have a stable suspension from CNT and liquid, there is a necessity to use an emulsifier. The type and concentration of the emulsifier depends on the type and concentration of CNT. These emulsifiers generally affect the viscosity and surface tension of the suspension, since the prepared suspension is intended to be used in heat and mass transfer applications one should be careful of selecting the emulsifier and its concentration so as not to affect the hydrodynamics in the system [7].
2	Temperature Effects on Surface Tension and Viscosity Thermal Behavior: CNT nanofluids are used often in heat transfer applications, where temperature significantly influences both viscosity and surface tension. Understanding of how temperature affects these two properties, specifically in CNT nanofluids, can optimize their use in thermal management systems. Experimental studies could examine temperature-dependent models for these fluids [64,65,66,67]. Critical Temperatures and Phase Changes: Exploring how do CNT nanofluids behave in phase change conditions (such as boiling, freezing) as in phase change material for thermal storage systems. This could reveal new insights of how viscosity and surface tension interact under extreme conditions.
3	Impact of Concentration on Surface Tension-Viscosity Relationship CNT Concentration: Byincreasing the concentration of CNTs in a base fluid, viscosity typically rises due to the formation of networks within the fluid. However, the effect of this increase on surface tension is less understood. A clearer understanding of the trade-offs between viscosity and surface tension in various concentrations and their combined influence on fluid behavior needs to be explored. Optimal Concentrations for Application: In certain applications a fine balance between surface tension and viscosity (e.g., in cooling systems or lubrication) may be required. Studying how concentration adjustment can achieve this balance for optimizing performance.
4	Interrelation Between Surface Tension and Viscosity in Dynamic Conditions Non-Newtonian Behavior: Many nanofluids exhibit non-Newtonian behavior, where the viscosity changes with shear rate. Research on how surface tension responds under such dynamic flow conditions (especially for CNT nanofluids) is still needed. Developing models that link these properties in dynamic environments is a key challenge. Capillarity and Fluid Transport: Surface tension-driven effects, such as capillarity, can be influenced by viscosity in systems where fluid transport through porous media or microchannels is important. Understanding viscosity role in limiting or enhancing capillary action through the surface tension-viscosity interaction could open new uses in microfluidics and in micro tubes and micro channels. This can be studied to find the surface tension effect on heat transfer in such systems.
5	Numerical and Computational Modeling Molecular Dynamics Simulations: Predictive modeling using molecular dynamics (MD) simulations can be used in understanding the interrelation of surface tension and viscosity at a molecular level in CNT nanofluids. Such models could be critical for predicting behavior under a wide range of conditions (e.g., temperature, pressure). Multi-Scale Modeling: Developing multi-scale models taking into consideration the gap between molecular-scale interactions (surface tension) and macro-scale fluid behavior (viscosity) could provide comprehensive tools for predicting the conduct of CNT nanofluids in real life applications.
6	Experimental Techniques for Simultaneous Measurement Advanced Rheological Techniques: These techniques will allow for better control and prediction of the fluid properties, refining experimental techniques for measuring surface tension and viscosity simultaneously, particularly in CNT nanofluids where CNTs are anisotropic and may align under certain conditions, will be critical. In Situ Monitoring: Techniques such as in situ measurements of surface tension and viscosity during phase changes also under dynamic flow conditions could be a gap for developing real-time data, useful for applications like enhanced oil recovery or coolant systems.
7	Applications and Industry-Specific Research Heat Transfer Applications: Future research may focus on fine-tuning viscosity and surface tension properties to enhance heat transfer efficiency while ensuring stability and fluidity. Understanding the interplay between surface tension and viscosity is crucial for optimizing CNT nanofluids in heat exchangers, electronic cooling systems, or solar energy collectors. Energy Storage and Lubrication Applications: In battery cooling or advanced lubricants, both low surface tension (better spreading) and controlled viscosity (low friction) are important. More future studies should be done to explore how these properties can be balanced to improve energy efficiency.
8	Environmental and Economic Considerations Biodegradable Base Fluids: The environmental impact of CNT nanofluids is becoming more important. Future research could explore sustainable or biodegradable base fluids and how they interact with CNTs focusing on whether the surface tension and viscosity of such fluids can be tailored in industrial use. Cost-Effectiveness: The challenge of scaling the production of CNT nanofluids while maintaining control over surface tension and viscosity is another research gab for the future. Finding cost-effective production methods without compromising performance could be crucial for widespread adoption.

.

Less pumping power can be achieved through less viscous fluids, which are also a contradiction to the formulas and literature. Because vast surface areas have tremendous activity, the nanoparticles are continually clashing owing to Brownian motion. Brownian motion is accelerated by conditions such as smaller particle size, decreased viscosity, and rising temperatures [64,65,66,67]. Because of Brownian motion, nanoparticles with extremely tiny diameters have greater kinetic energy. However, it still suffers from not remaining suspended in the base fluids for an extended period, which has an impact on nanofluid stability [68]. The use of surfactants and additives with nanofluids has the advantage of preventing nanoparticle precipitation in the base fluids. Other parameters, such as the viscosity and characteristics of the base fluids, influence clustering [69]. Some drawbacks with surfactants and pH regulators, which impact nanofluid stability, may occur at high temperatures, such as stabilizers breaking down, causing changes in liquid characteristics, viscosity, and liquid surface tension. Surfactant has a lower thermal conductivity than base fluids, which affects the thermal conductivity of Nanofluids [70]; utilizing surfactants at the optimal concentration is critical.

Viscosity is an important component that influences the behavior of nanofluids [71]. The viscosity of the fluids affects heat transfer properties, with increasing viscosity being the principal impediment to circulating Nanofluids in pump systems [31]. Increasing the nanoparticle concentration raises the viscosity of the nanofluids, which raises the pumping power owing to pressure drop [72]. Meanwhile, the addition of surfactants enhances the viscosity of nanofluids.

Said et al. [73] discovered that volume concentration and temperature influence viscosity when studying the thermo-physical characteristics of nanofluids. At the same time, the base fluids are the most critical component influencing nanofluid viscosity. Thus, the ideal nanofluid must have excellent heat conductivity and low viscosity. In contrast, the viscosity of nanofluids decreases with increasing temperature [74]. Increasing temperature adds to increased thermal conductivity. Increasing the temperature increases thermal conductivity, which decreases viscosity and minimizes nanoparticle agglomeration [5,23,75]. Thus, both Verma et al. [76] and Iranmanesh [77] found that increasing the temperature increased the thermal conductivity of nanofluids, but viscosity decreased [78].

Surface tension and viscosity are two important physical characteristics of the suspension that are altered when carbon nanotube CNTs are added to water. These characteristics are essential in many applications, including biological systems, coatings, and nanofluids for heat transfer.

CNT–Water Suspension Viscosity

➢

CNT Addition Effect on Viscosity:

The viscosity of the suspension usually increases when CNTs are added to water. This rise happens because CNTs, particularly when distributed well, form a fluid-resisting network structure. Multiple elements determine how much the viscosity increases. Viscosity increases more noticeably at higher CNT concentrations. Because more extensive network development occurs at higher CNT concentrations, viscosity is increased more significantly [78].

➢

CNTs’ large aspect ratio:

The ratio of their length to diameter contributes considerably to the rise in viscosity. Viscosity is often increased more by longer or higher aspect ratio carbon nanotubes CNTs than by shorter CNTs [79].

➢

Dispersion Quality:

Agglomerated CNTs contribute less effectively to the suspension’s network structure. Hence, well-dispersed CNTs cause a larger viscosity increase than poorly dispersed CNTs [80,81].

➢

Functionalization:

Functionalized CNTs may disperse more readily in water, producing a more uniform suspension with a possible increase in viscosity. Functionalization, however, may also lessen the propensity of CNTs to aggregate, perhaps leading to a lesser rise in viscosity in comparison to potentially leading to a reduced viscosity rise in comparison to unmodified CNTs at the same concentration [81].

➢

Temperature increases the effect on the viscosity of CNT Nanofluids:

As the temperature of the nanofluids increases, the viscosity decreases. The bonds between the particles and the fluids molecules weaken, thus decreasing viscosity. An example is that lubricants are less viscous when heated, so thicker oil is used for hot weather and less viscous oils are used for colder weather. Figure 10 describes the relation between viscosity and temperature.

➢

CNT–Water Nanofluid Surface Tension:

The force that makes a liquid behave like an elastic sheet that has been stretched is known as surface tension. It is essential to several processes, including capillary action, droplet stability, and wetting [45,58,83,84,85].

The water’s surface tension can change when CNTs are present:

CNT concentration: Surface tension can be influenced by CNT concentration like viscosity, but the relationship is more complicated and depends on the molecular interactions between CNTs and water.

The process of making something functional Depending on the type of treatment, CNTs that have been surface-treated or functionalized (e.g., with hydrophilic or hydrophobic groups) can either enhance or reduce the surface tension [84,86].

Temperature: Surface tension drops as the temperature rises. However, this behavior can be altered by the presence of CNTs [86,87].

Stability: Agglomerated CNTs can result in non-uniform surface characteristics, although well-dispersed CNTs can stabilize the surface tension [86,87].

5. Conclusions

This review looked at how surface tension and viscosity affect the characteristics of CNT nanofluids. These two features were chosen because the literature suggests a research need in understanding the impact of these parameters on applications and the usage of CNT nanofluids in heat transfer and other unit processes. Furthermore, there are few review studies that focus on these features in the context of CNT nanofluids. The search indicated that no studies have evaluated these attributes together or studied if there is a direct relation between them and essential operational parameters such as temperature and concentration. Only a few articles looked at viscosity, noting its evident influence on thermal characteristics, particularly heat and mass transfer, while a few others focused on surface tension, but the link is still unclear. This review compiles all findings into a detailed analysis.

The results show that surface tension refers to the tendency of liquid surfaces to contract to the smallest possible surface area. While viscosity of CNT Nanofluids depends on factors like CNT concentration, temperature, and fluid composition. The following conclusions can be drawn:

➢

There is an optimal concentration for surface tension, beyond which the surface tension will fall. As the concentration of CNT in nanofluids increases, the interaction and entanglement of CNTs within the fluids causes an increase in suspension viscosity. The most reasonable answer was this one. While some answers were contradicted and distinct, they lacked logical support.

➢

Because the forces or bonds between molecules drop as kinetic energy increases, increasing temperature lowers viscosity and surface tension.

➢

The use of surfactants during the creation of nanofluids typically results in a drop in surface tension since the molecules’ forces are reduced because of the surfactants. Simultaneously, the type and concentration of surfactant also affect the nanofluid’s viscosity.

➢

Certain other elements, such as surface area, stresses, and gas present, influence surface tension but not viscosity.

➢

There is no known influence of other parameters on surface tension, such as aspect ratio, dispersion quality, and functionalization.

➢

Certain articles mentioned a relationship between viscosity and surface tension. These relationships were complex and susceptible to many experimental setups, though. Functional groups of nanotubes, which may change the surface chemistry of CNTs and their interactions with surrounding fluids, were one of the influencing elements. These groups can also modify the viscosity of nanofluids.

➢

Hydrophilic functional groups can promote dispersion in water-based nanofluids in two distinct ways, which may result in a possible reduction in viscosity. On the other hand, adding hydrophobic groups might cause them to aggregate and increase viscosity. The impact is continuous with respect to the distribution and density of functional groups, highlighting the significance of investigating CNT properties.

➢

Adding CNTs can change surface properties and interrupt hydrogen bonding in water, affecting surface tension. The presence of CNTs in the fluids can affect viscosity, especially if they become entangled or form aggregates.

➢

To comprehend the relationship between surface tension and viscosity in CNT water fluids, experimental studies are recommended in this context.

➢

Surface tension affects how the fluid wets a surface or moves through capillary spaces. Higher surface tension can cause a higher resistance to movement, but this must be balanced with the viscosity, as higher viscosity will further resist flow.

➢

CNT nanofluids might sometimes exhibit shear-thinning behavior, where viscosity decreases with increasing shear rate. Surface tension can influence how the fluid spreads under these conditions, particularly in microfluidics or porous media.

➢

Surface tension can affect nanoparticle suspension stability leading to agglomeration if surface tension is too high, increasing viscosity and leading to inconsistent behavior.

In conclusion, the presence of a correlation between surface tension and viscosity remains uncertain. While one might expect that thicker fluids would have higher surface tension and thinner fluids lower surface tension, this is not the case. This research has shown that no conclusive correlation exists. Despite delving into more theoretical complexities, no clear relationship between surface tension and viscosity has been established yet. In summary, surface tension relates to a fluid’s steady state, while viscosity concerns its movement. Although there is some interaction between the two, there is no strict correlation.