Initial Occurrence State and Movability Evaluation of the Gulong Shale Oil Reservoir, Songliao Basin
Abstract
:1. Introduction
2. Methodology
2.1. Nano-Confined Phase State Calculation Method
2.2. Nuclear Magnetic Resonance Experimental Method
- (1)
- Measure the dry weight of the core by balance and test T2 spectrum of dry rock samples with a nuclear magnetic resonance instrument.
- (2)
- Saturate the shale core with oil samples, and maintain the saturation process for 15 days. Measure the quality of the shale core after saturation; obtain the NMR T2 spectrum and 2D T1-T2 spectrum of shale rock samples after saturation.
- (3)
- Centrifuge the saturated shale core at 2000 r/min, 6000 r/min, and 10,000 r/min (the maximum speed of the instrument), measure the weight, and obtain the NMR T2 spectrum after centrifugation.
2.3. Molecular Simulation Method
3. Results and Analysis
3.1. Phase Behavior in Shale Nanopores
3.2. Analysis of Initial Occurrence State
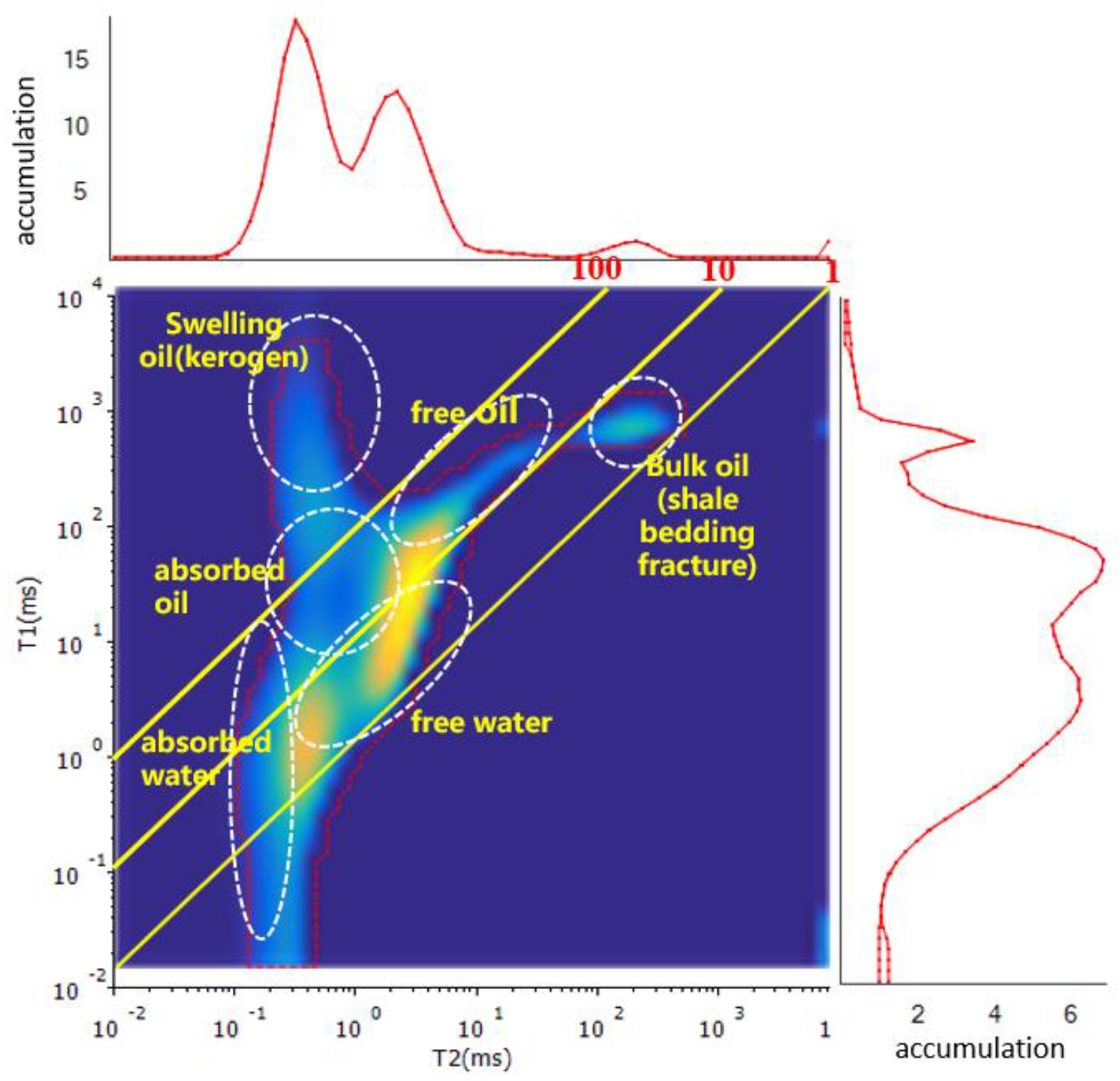
3.3. Analysis of Adsorbed Oil and Free Oil
3.4. Analysis of Movable Fluid Saturation
4. Conclusions
- (1)
- Considering the nano-confinement effects, the nanopores of the Gulong shale reservoir present the characteristics of a ‘condensate gas state in small pores and volatile oil state in large pore’.
- (2)
- The initial occurrence state of the Gulong shale reservoir is mainly the adsorbed state and free state. The adsorbed oil in inorganic pores accounts for about 24.4%, and the adsorbed oil in organic pores accounts for about 57.8%.
- (3)
- The minimum movable limit of the pore throat in the Gulong shale reservoir is determined by centrifugation and the nuclear magnetic resonance method. The movable porosity accounts for about 11% of the total porosity, and the movable fluid saturation is about 12%.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lu, S.; Huang, W.; Chen, F.; Li, J.; Wang, M.; Xue, H.; Wang, W.; Cai, X. Classification and evaluation criteria of shale oil and gas resources: Discussion and application. Pet. Explor. Dev. 2012, 39, 268–276. [Google Scholar] [CrossRef]
- Sun, L.; Liu, H.; He, W.; Li, G.; Zhang, S.; Zhu, R.; Jin, X.; Meng, S.; Jiang, H. An analysis of major scientific problems and research paths of Gulong shale oil in Daqing Oilfield, NE China. Pet. Explor. Dev. 2021, 48, 527–540. [Google Scholar] [CrossRef]
- Wang, Y.; Liang, J.; Zhang, J.; Zhao, B.; Zhao, Y.; Liu, X.; Xia, D. Resource potential and exploration direction of Gulong shale oil in Songliao Basin. Pet. Geol. Oilfield Dev. Daqing 2020, 39, 20–34. [Google Scholar]
- Zou, C.; Yang, Z.; Cui, J.; Zhu, R.; Hou, L.; Tao, S.; Yuan, X.; Wu, S.; Lin, S.; Wang, L.; et al. Formation mechanism, geological characteristics and development strategy of nonmarine shale oil in China. Pet. Explor. Dev. 2013, 40, 15–27. [Google Scholar] [CrossRef]
- He, W.; Meng, Q.; Feng, Z.; Zhang, J.; Wang, R. In-situ accumulation theory and exploration & development practice of Gulong shale oil in Songliao Basin. Shiyou Xuebao/Acta Pet. Sin. 2022, 43, 1–14. [Google Scholar] [CrossRef]
- Jiang, Z.; Zhang, W.; Liang, C.; Wang, Y.; Liu, H.; Chen, X. Characteristics and evaluation elements of shale oil reservoir. Shiyou Xuebao/Acta Pet. Sin. 2014, 35, 184–196. [Google Scholar] [CrossRef]
- Liu, H.; Zhang, S.; Bao, Y.; Fang, Z.; Yao, S.; Wang, Y. Geological characteristics and effectiveness of the shale oil reservoir in Dongying sag. Oil Gas Geol. 2019, 40, 512–523. [Google Scholar] [CrossRef]
- Wang, F.; Fu, Z.; Wang, J.; Tang, Z.; Jiang, R. Characteristics and classification evaluation of Gulong shale oil reservoir in Songliao Basin. Pet. Geol. Oilfield Dev. Daqing 2021, 40, 144–156. [Google Scholar]
- Tian, S. Microscopic Characteristics of Shale Reservoir Pores and Evaluation of Shale Oil Occurrence and Mobility. Ph.D. Thesis, China University of Petroleum (East China), Qingdao, China, 2019. [Google Scholar]
- Zhang, X. Research on New Methods for Characterizing Pore Structure of Shale Oil and Gas Reservoirs. Master’s Thesis, University of Chinese Academy of Sciences (Institute of Seepage Fluid Mechanics, Chinese Academy of Sciences), Beijing, China, 2021. [Google Scholar]
- Li, J. Study on the Occurrence Mechanism and Mobility of Shale Oil. Ph.D. Thesis, China University of Petroleum (East China), Qingdao, China, 2020. [Google Scholar]
- Lu, S.; Chen, F.; Xiao, H.; Li, J.; He, X. Quantitative evaluation of organic and inorganic pores in shale reservoirs-Taking the Lower Cambrian Niutitang Formation in Qiannan Depression as an example. In Proceedings of the 14th Annual Conference of the Chinese Society of Mineral and Rock Geochemistry, Nanjing, China, 21 April 2013; p. 1. [Google Scholar]
- O’Brien, N.R.; Cremer, M.D.; Canales, D.G. The Role of Argillaceous Rock Fabric in Primary Migration of Oil. GCAGS Trans. 2002, 52, 1103–1112. [Google Scholar]
- Zhang, W. Characteristics and evaluation factors of shale oil reservoirs in the third member of Paleogene Hetaoyuan Formation in Biyang Sag. Master’s Thesis, China University of Geosciences (Beijing), Beijing, China, 2014. [Google Scholar]
- Ke, S. Discussion on occurrence state and mobility of shale oil in Biyang sag. Pet. Geol. Eng. 2017, 31, 80–83. [Google Scholar]
- Ning, F.; Wang, X.; Hao, X.; Jiang, X.; Wang, Y.; Zhu, D.; Zhu, D. Study on evaluation method of shale oil sweet spot in Jiyang depression. Sci. Technol. Eng. 2015, 35, 11–16. [Google Scholar]
- Jarvie, D. Shale resource systems for oil and gas: Part 2: Shale-oil resource systems. AAPG Mem. 2012, 97, 89–119. [Google Scholar]
- Sun, J.; Chen, J.; Yang, Z.; Liu, X.; Liu, Y. Experimental Study of the NMR Characteristics of Shale Reservoir Rock. Sci. Technol. Rev. 2012, 30, 25–30. [Google Scholar]
- Zhou, S.; Liu, H.; Yan, G.; Xue, H.; Guo, W. NMR research of movable fluid and T_2 cutoff of marine shale in South China. Oil Gas Geol. 2016, 37, 612–616. [Google Scholar]
- Li, Z.; Zou, Y.-R.; Xu, X.-Y.; Sun, J.-N.; Li, M.; Peng, P.a. Adsorption of mudstone source rock for shale oil—Experiments, model and a case study. Org. Geochem. 2016, 92, 55–62. [Google Scholar] [CrossRef]
- Cao, H.; Zou, Y.-R.; Lei, Y.; Xi, D.; Wan, X.; Peng, P.A. Shale Oil Assessment for the Songliao Basin, Northeastern China, Using Oil Generation-Sorption Method. Energy Fuels 2017, 31, 4826–4842. [Google Scholar] [CrossRef]
- Xu, F.; Jiang, H.Q.; Liu, M.; Jiang, S.; Wang, Y.; Li, J.J. NMR-Based Analysis of Fluid Occurrence Space and Imbibition Oil Recovery in Gulong Shale. Processes 2023, 11, 1678. [Google Scholar] [CrossRef]
- Zhu, C.F.; Guo, W.; Li, Y.J.; Gong, H.J.; Sheng, J.J.; Dong, M.Z. Effect of occurrence states of fluid and pore structures on shale oil movability. Fuel 2021, 288, 119847. [Google Scholar] [CrossRef]
- Sun, X.; Du, H.; Wang, C.; Du, S.; Liu, G. Characterization of Shale Porosity and Permeability by Nuclear Magnetic Resonance. In Proceedings of the 2nd International Conference on Energy Utilization and Automation, ICEUA 2023, Virtual, Online, China, 10–12 February 2023. [Google Scholar]
- Zarragoicoechea, G.J.; Kuz, V.A. Critical shift of a confined fluid in a nanopore. Fluid Phase Equilibria 2004, 220, 7–9. [Google Scholar] [CrossRef]
- Guerillot, D.R. EOR Screening with an Expert System. In Proceedings of the Petroleum Computer Conference, San Jose, CA, USA, 27–29 June 1988. Article No. SPE-17791-MS. [Google Scholar] [CrossRef]
- Alvarado, V.; Manrique, E. Chapter 8: EOR’s Current Status. In Enhanced Oil Recovery; Alvarado, V., Manrique, E., Eds.; Gulf Professional Publishing: Boston, MA, USA, 2010; pp. 133–156. [Google Scholar] [CrossRef]
- Lyons, W. Working Guide to Reservoir Engineering; Gulf Professional Publishing: Houston, TX, USA, 2009. [Google Scholar]









| Components | Mole Fraction, % | Critical Temperature, K | Critical Pressure, MPa | Acentric Factor | Molecular Weight, g/mol | Critical Volume, m3/mol | Relative Density |
|---|---|---|---|---|---|---|---|
| N2 | 1.86 | 126.20 | 3.46 | 0.04 | 28.01 | 0.09 | 0.81 |
| CO2 | 3.98 | 304.19 | 7.382 | 0.23 | 44.01 | 0.09 | 0.82 |
| C1 | 54.84 | 190.56 | 4.599 | 0.01 | 16.04 | 0.10 | 0.30 |
| C2–C5 | 23.55 | 352.09 | 4.452 | 0.14 | 40.07 | 0.18 | 0.44 |
| C6–C10 | 4.59 | 582.04 | 2.805 | 0.32 | 111.18 | 0.43 | 0.75 |
| C11–C20 | 7.90 | 721.12 | 1.79 | 0.54 | 204.96 | 0.80 | 0.83 |
| C21–C30 | 2.94 | 833.44 | 1.257 | 0.80 | 327.19 | 1.31 | 0.88 |
| C31+ | 0.32 | 906.97 | 0.918 | 0.92 | 428.96 | 1.74 | 0.92 |
| Type of Porous Media | Range of Pore Radius | Fluid Distribution | Phase State |
|---|---|---|---|
| Nanopores | 2–50 nm | Hydrocarbon | Condensate gas state |
| Shale bedding fracture | 100 nm–5000 nm | Hydrocarbon, a small amount of fracturing fluid | Volatile oil state |
| Hydraulic fracture | >1 mm | Fracturing fluid, a small amount of hydrocarbon | Black oil state |
| Well Name | Subzone | T2 Cutoff Value Method—S1 | T2 Area Method—S2 | Mass of Cores Method—S3 | Movable Fluid Saturation S |
|---|---|---|---|---|---|
| GY-10HC | Q1 | 15.87% | 12.95% | 10.51% | 11.73% |
| Q3 | 16.34% | 12.72% | 10.32% | 11.52% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, G.; Cheng, L.; Jia, P.; Liu, Y.; Feng, H.; Kuang, T.; Wang, Q. Initial Occurrence State and Movability Evaluation of the Gulong Shale Oil Reservoir, Songliao Basin. Energies 2024, 17, 1358. https://doi.org/10.3390/en17061358
Zhao G, Cheng L, Jia P, Liu Y, Feng H, Kuang T, Wang Q. Initial Occurrence State and Movability Evaluation of the Gulong Shale Oil Reservoir, Songliao Basin. Energies. 2024; 17(6):1358. https://doi.org/10.3390/en17061358
Chicago/Turabian StyleZhao, Guozhong, Linsong Cheng, Pin Jia, Yong Liu, Haoran Feng, Tie Kuang, and Qingzhen Wang. 2024. "Initial Occurrence State and Movability Evaluation of the Gulong Shale Oil Reservoir, Songliao Basin" Energies 17, no. 6: 1358. https://doi.org/10.3390/en17061358
APA StyleZhao, G., Cheng, L., Jia, P., Liu, Y., Feng, H., Kuang, T., & Wang, Q. (2024). Initial Occurrence State and Movability Evaluation of the Gulong Shale Oil Reservoir, Songliao Basin. Energies, 17(6), 1358. https://doi.org/10.3390/en17061358





