Analysis of Thermal Management Strategies for 21700 Lithium-Ion Batteries Incorporating Phase Change Materials and Porous Copper Foam with Different Battery Orientations
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussions
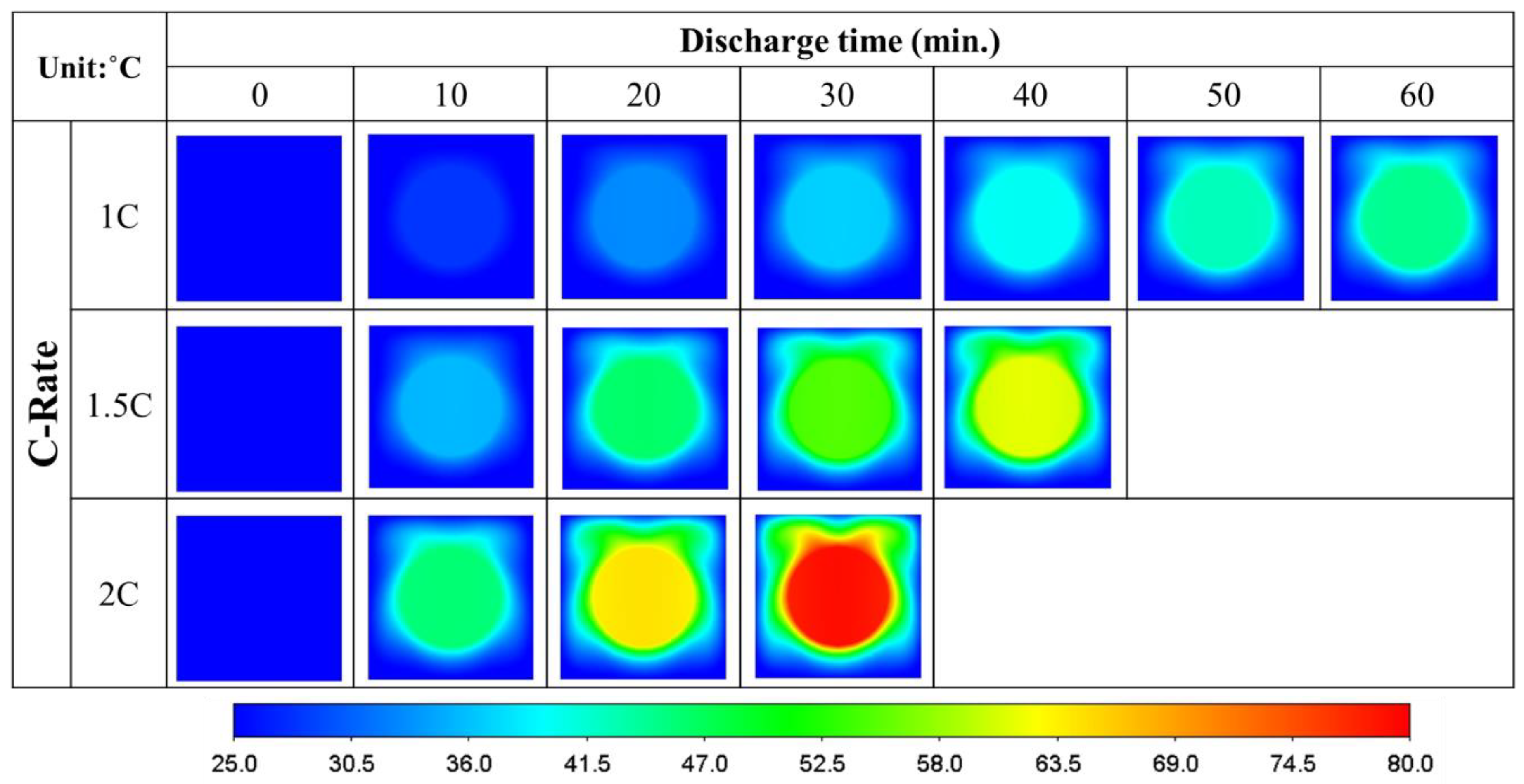
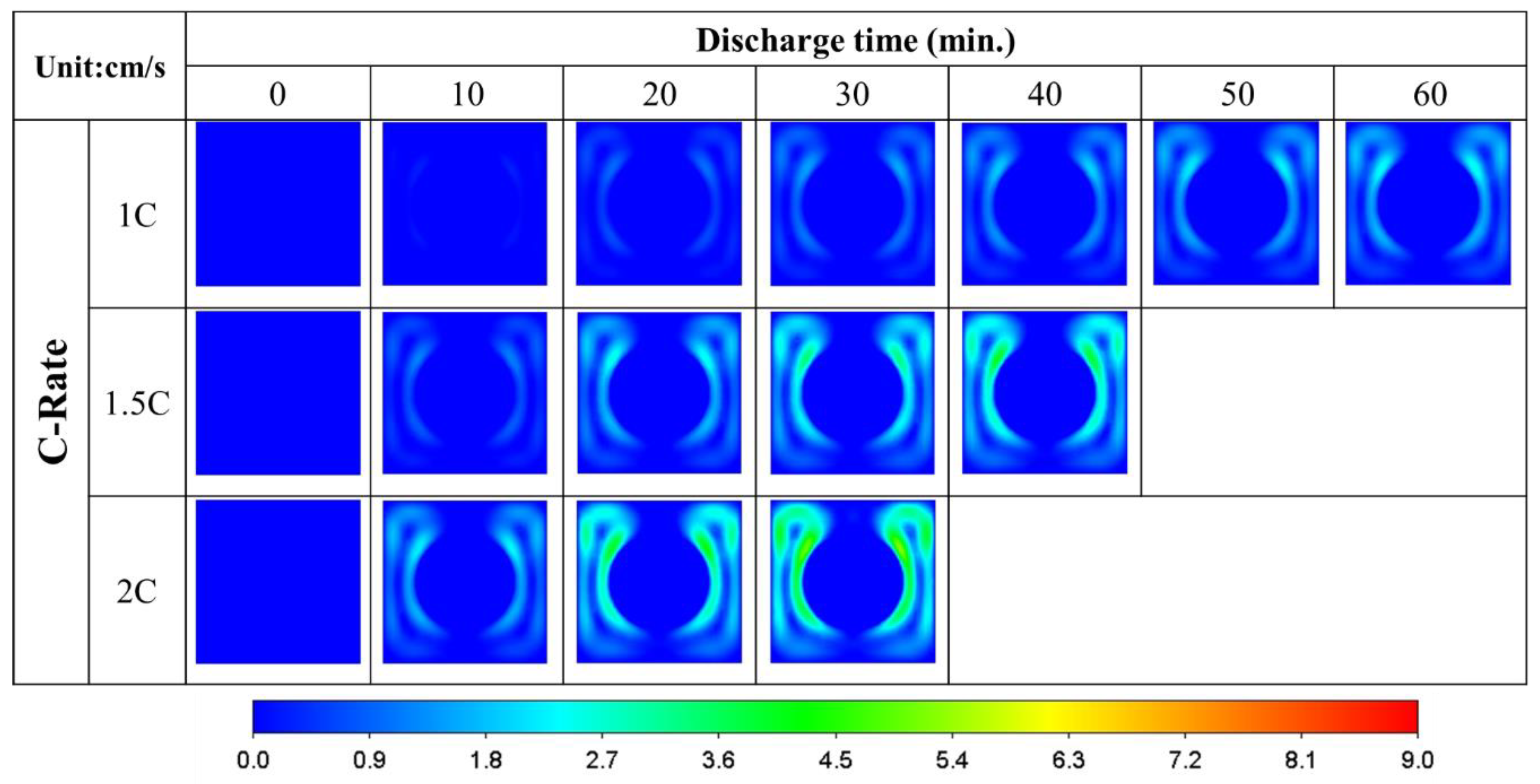
3.1. Single-Battery Model
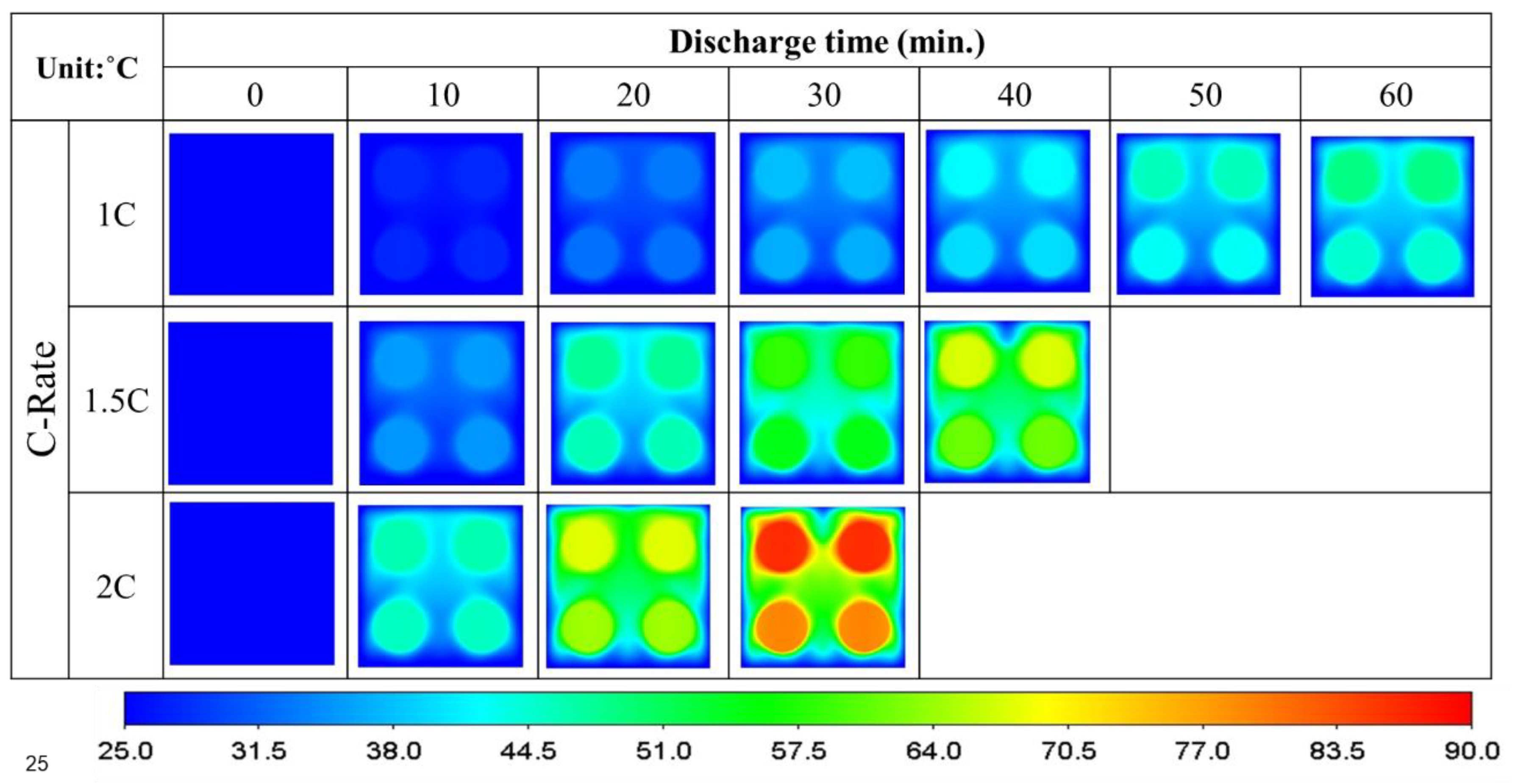
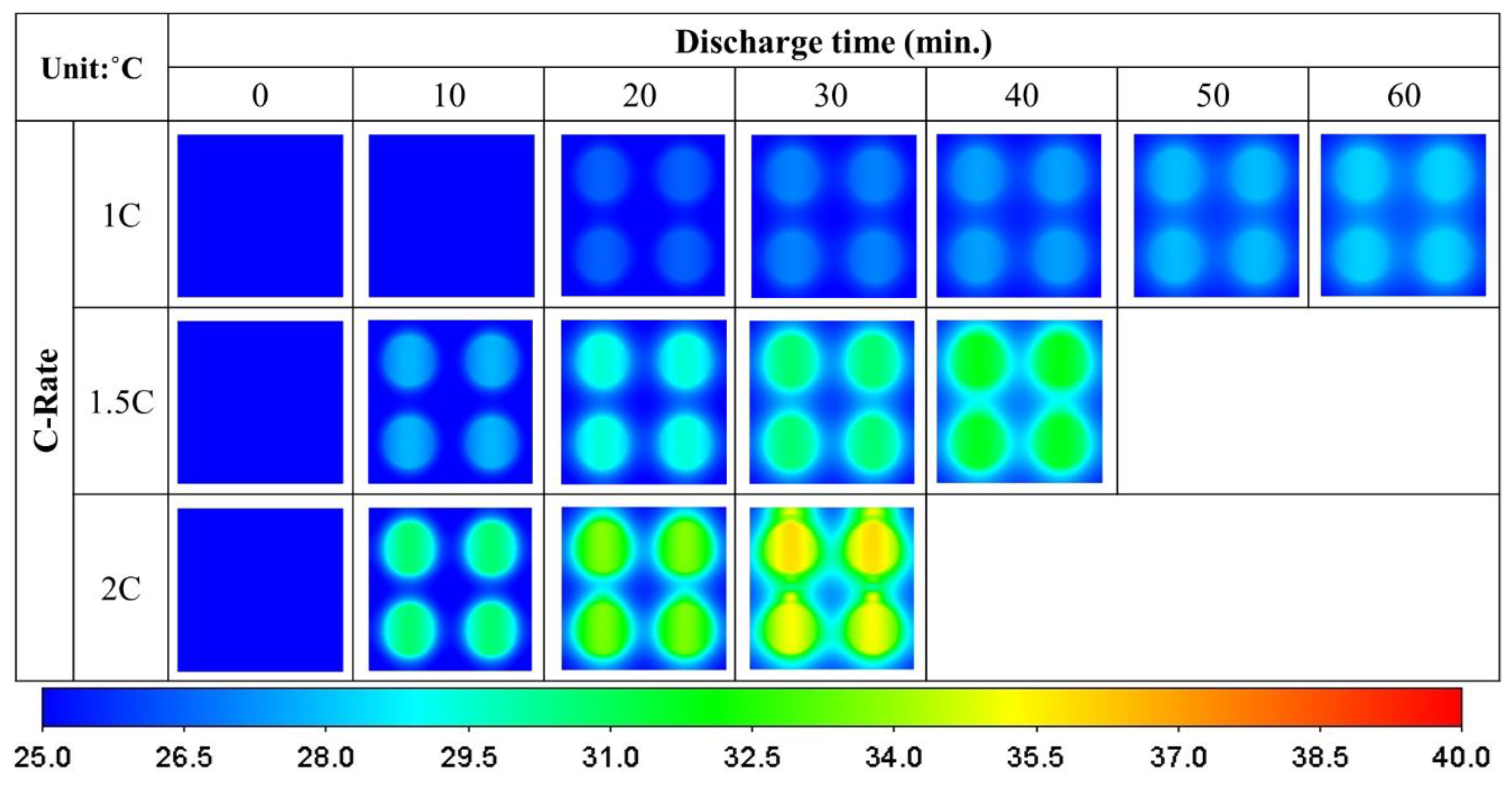
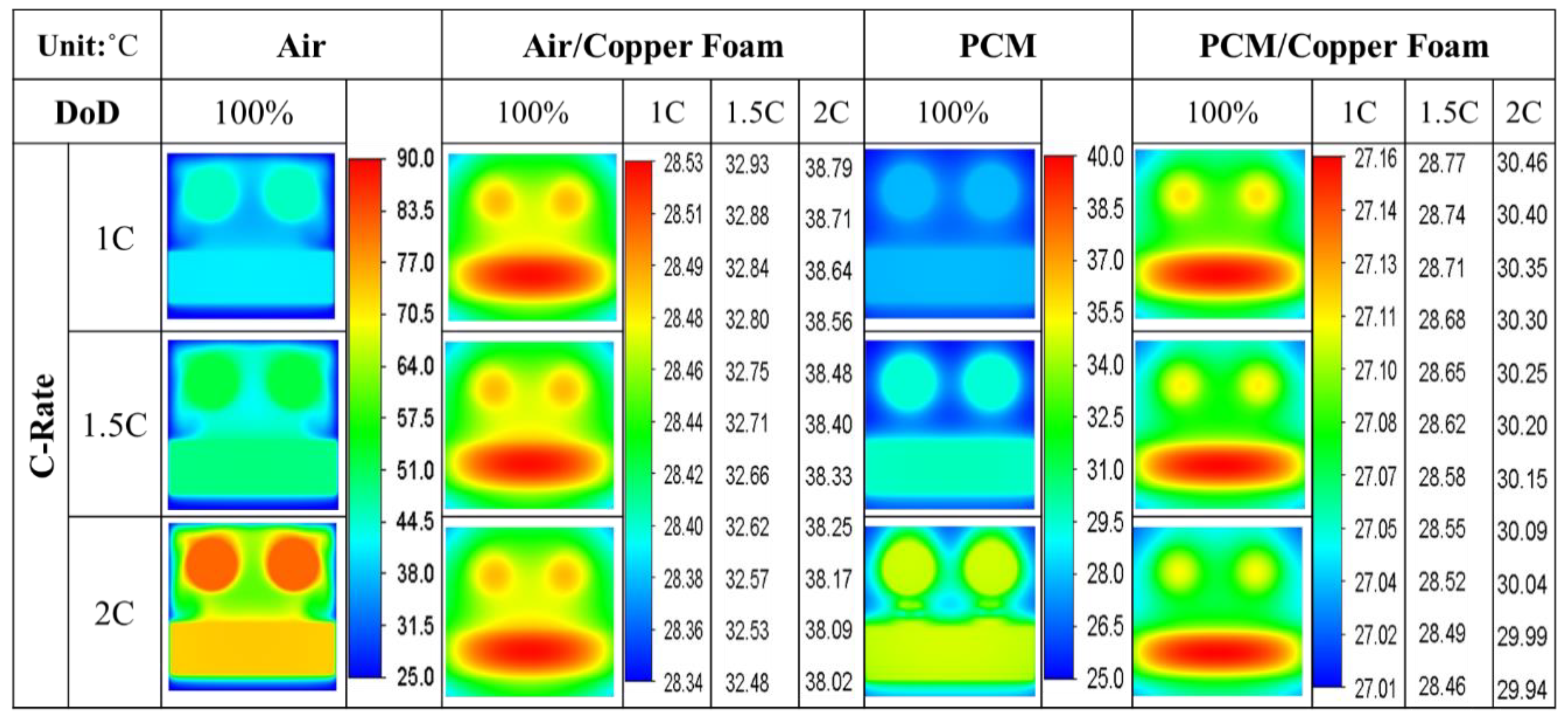
3.2. Unidirectional Four-Battery Model
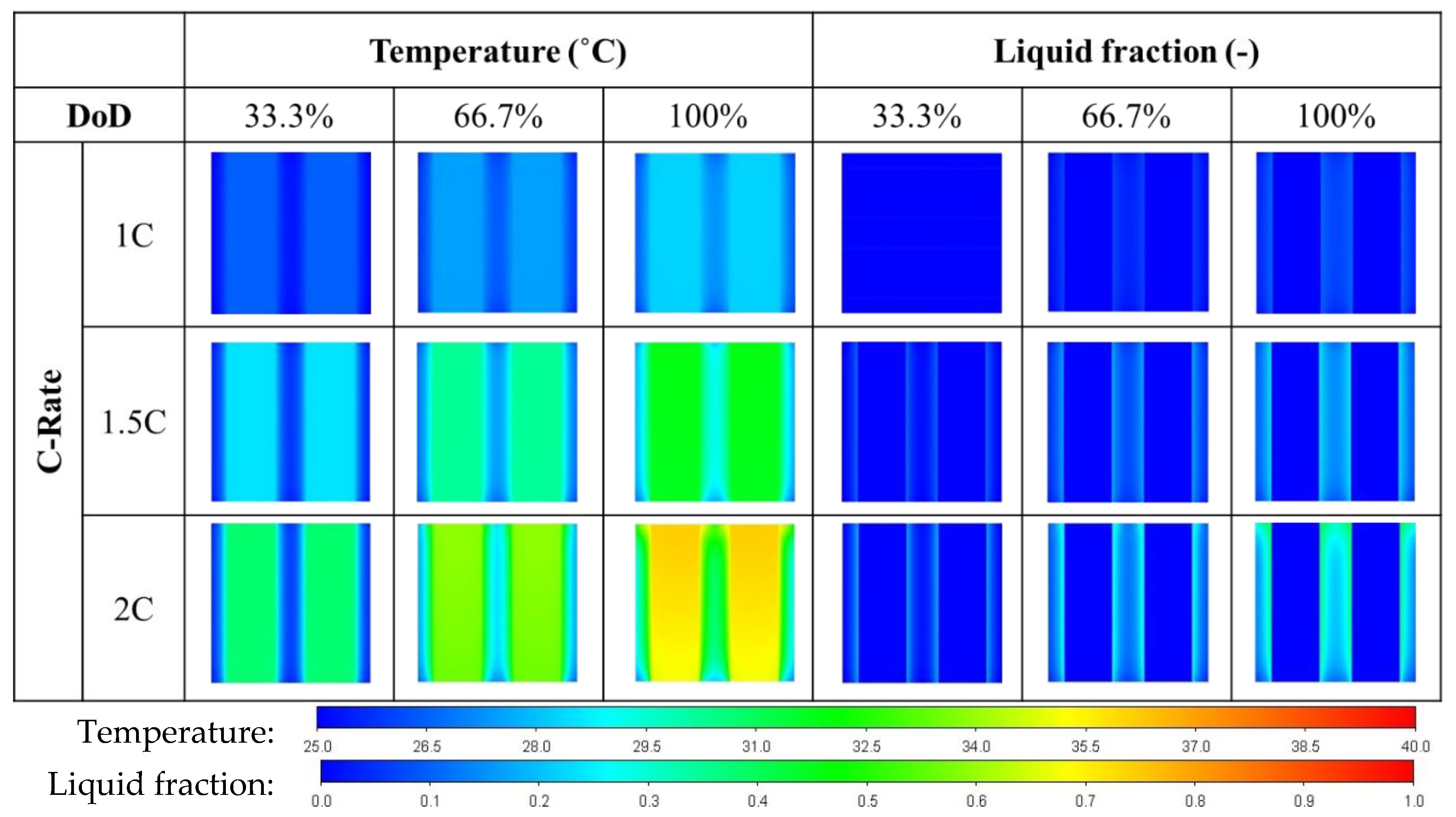
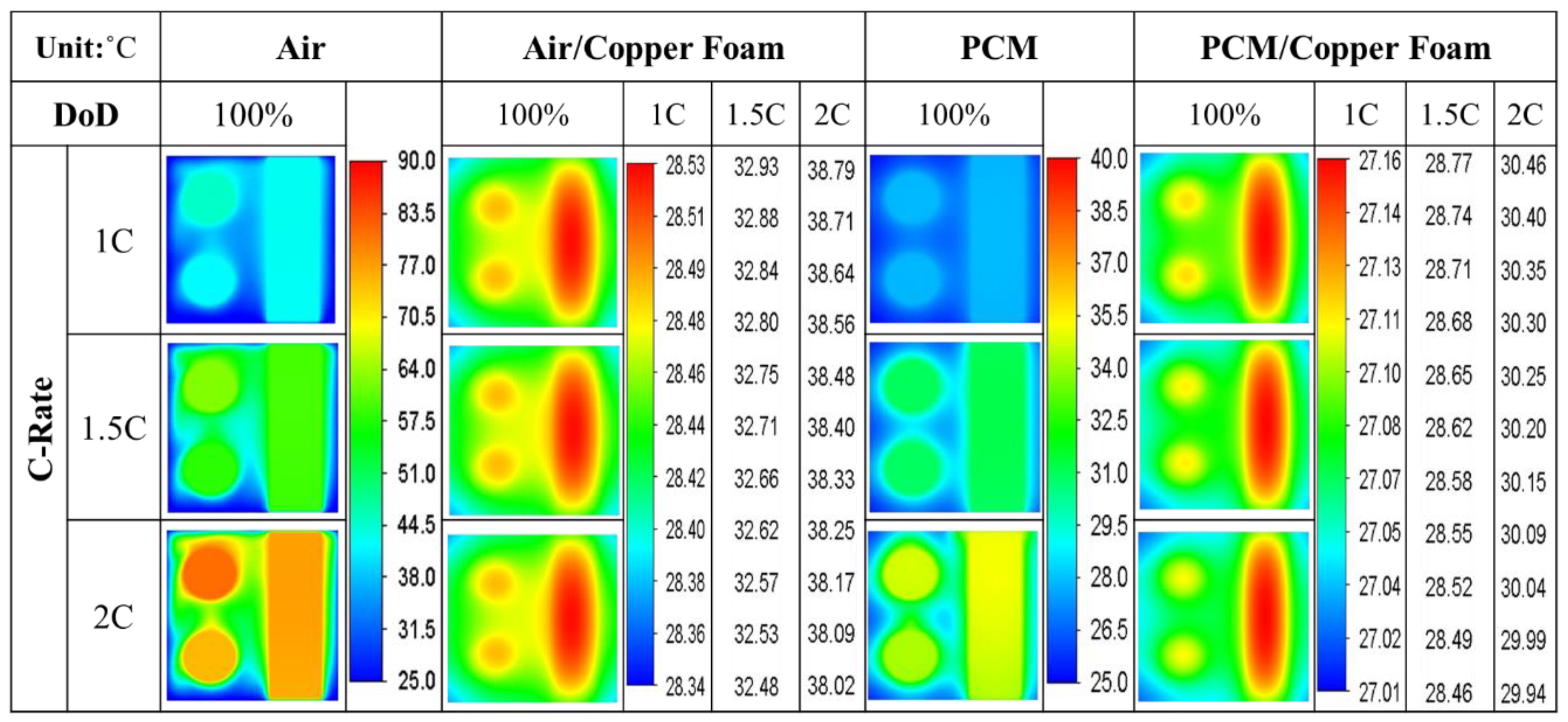
3.3. Crossover Four-Battery Model
3.4. Heat Flux on Air-Cooled Battery Surfaces
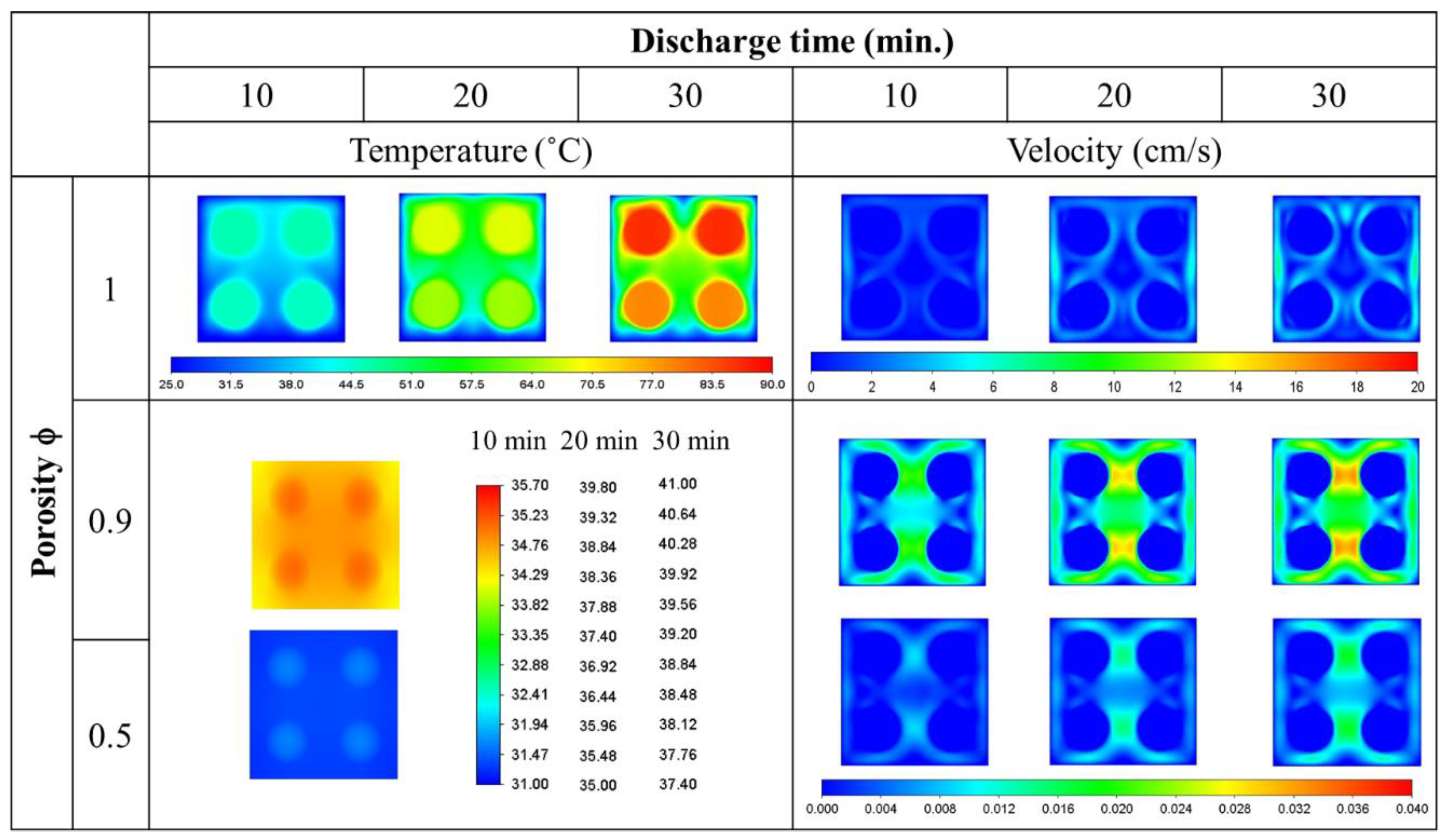
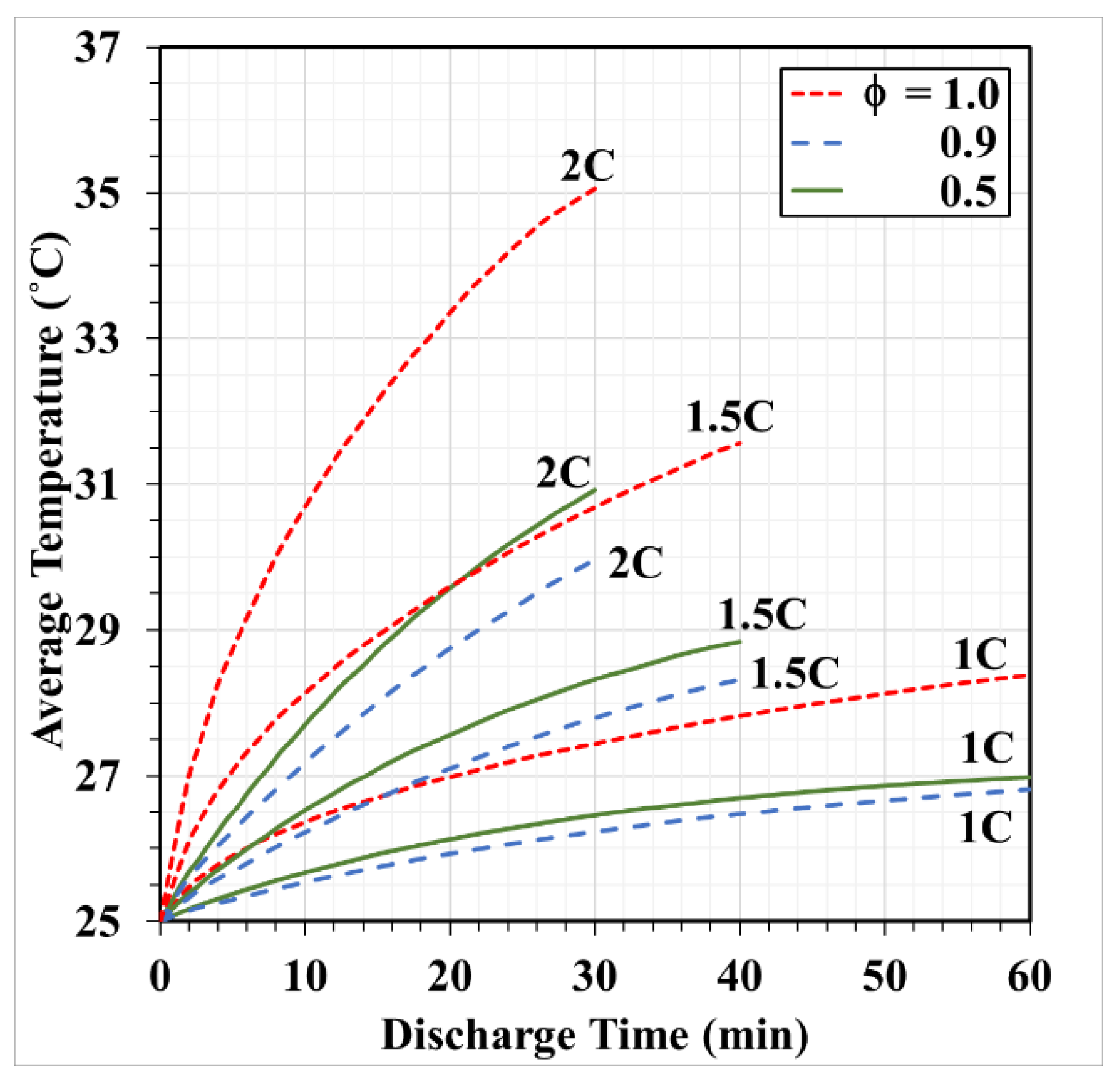
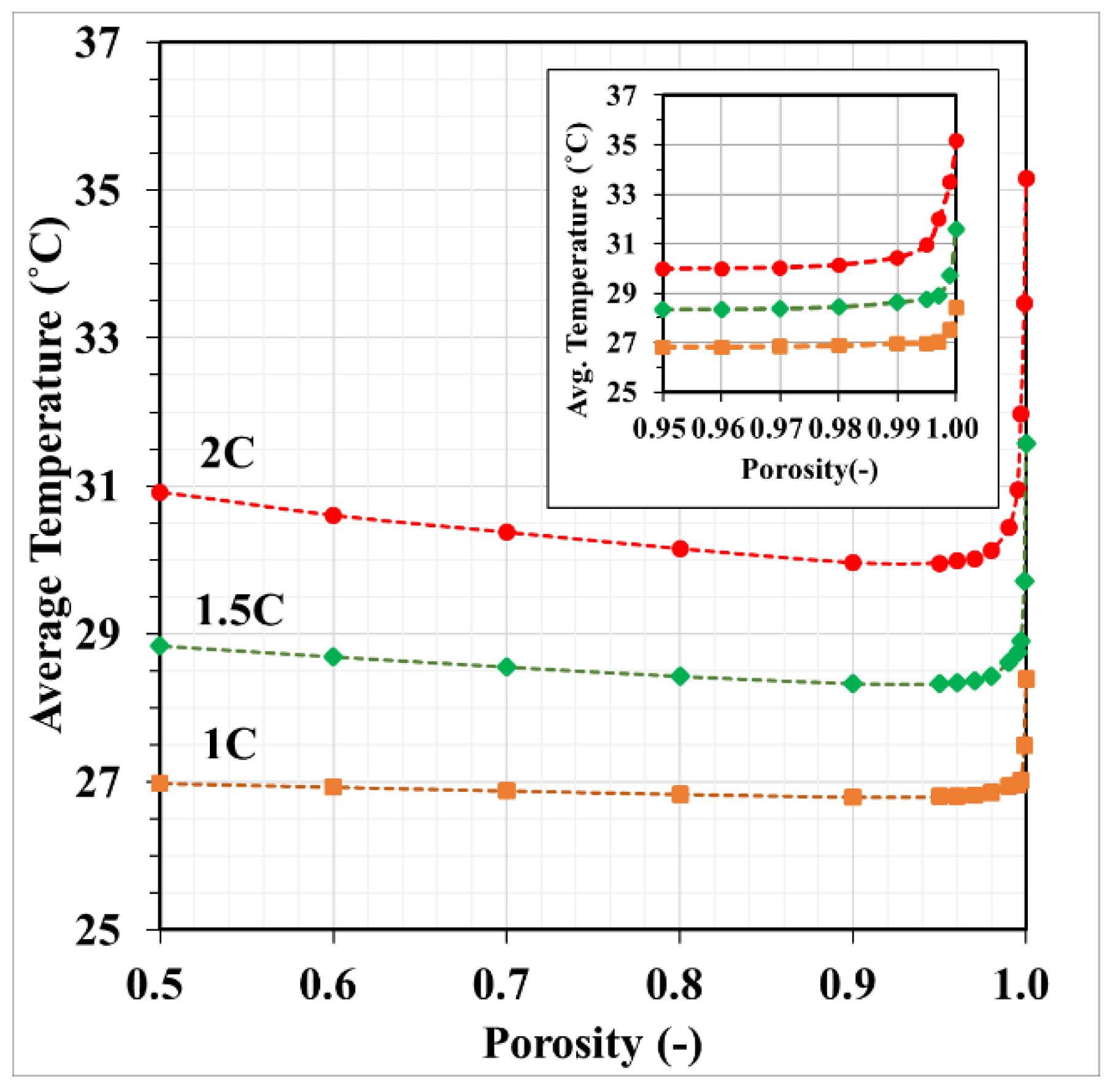
3.5. Foam Porosity on Battery Temperature
4. Conclusions
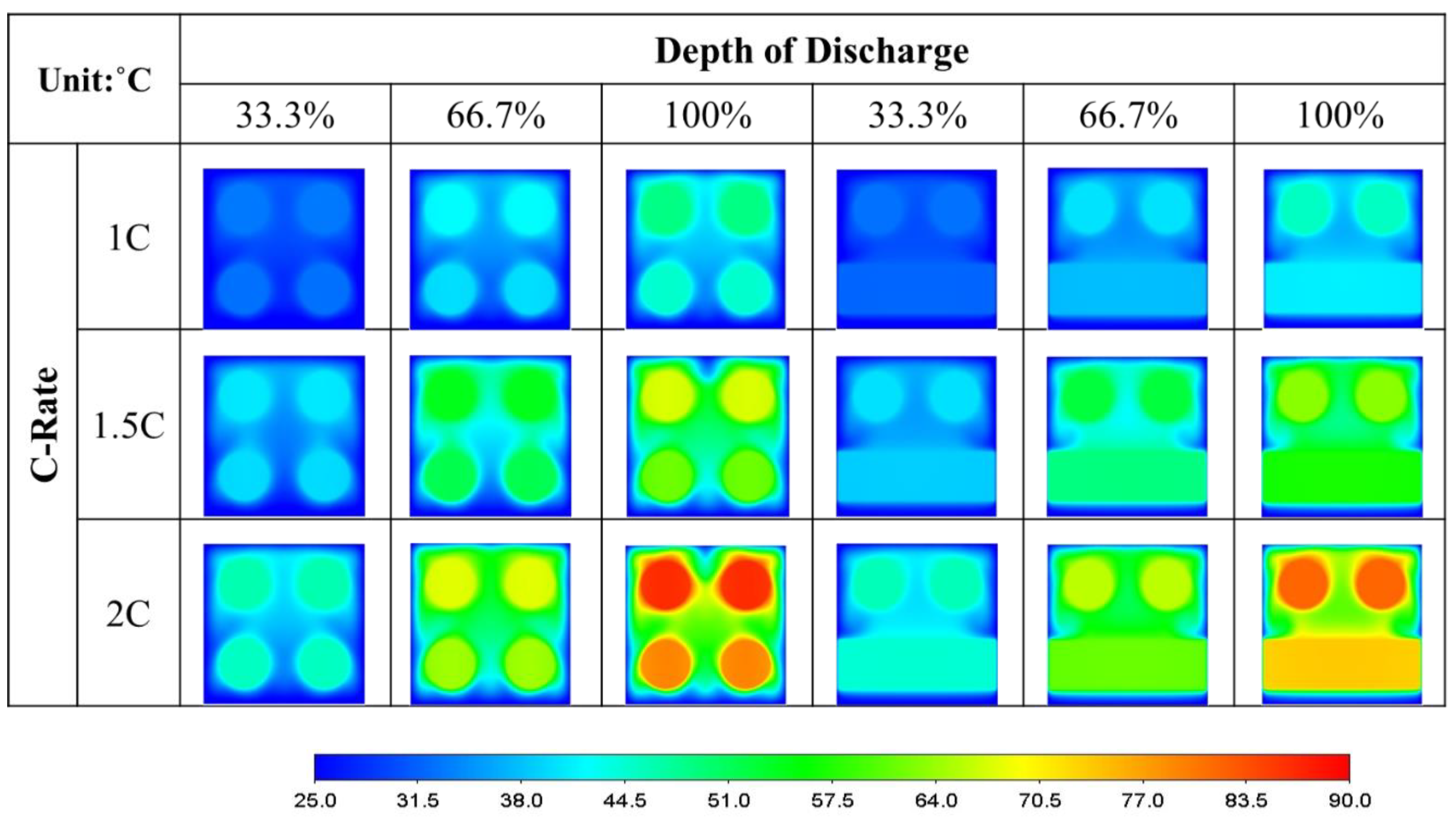
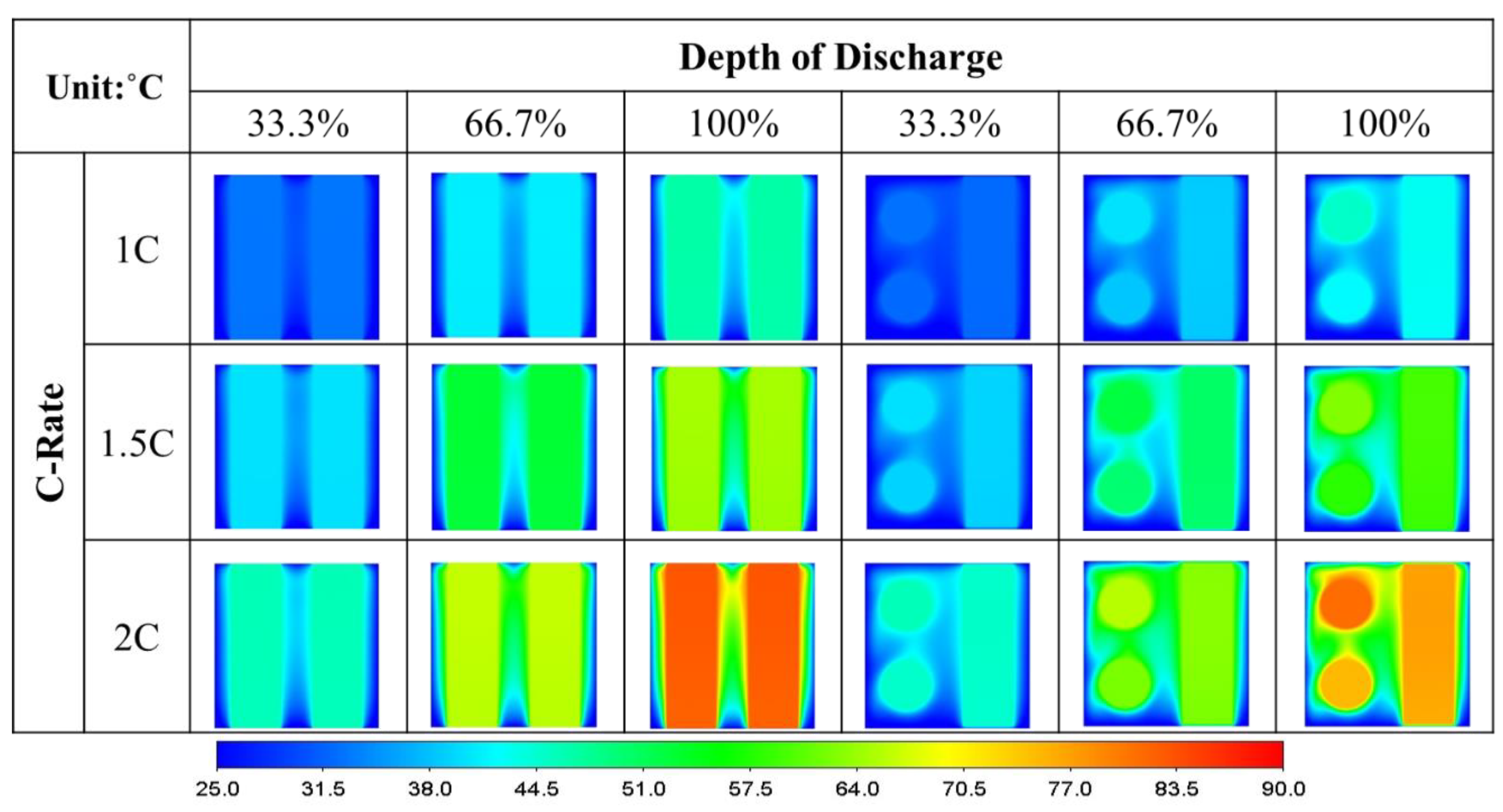
- Under the same discharge rate and cooling strategy, the orientation of the single-battery pack has an insignificant effect on the range of the battery temperature. However, the air flows faster in the battery pack when the battery is placed vertically at 8.1 cm/s compared to horizontally at 6 cm/s.
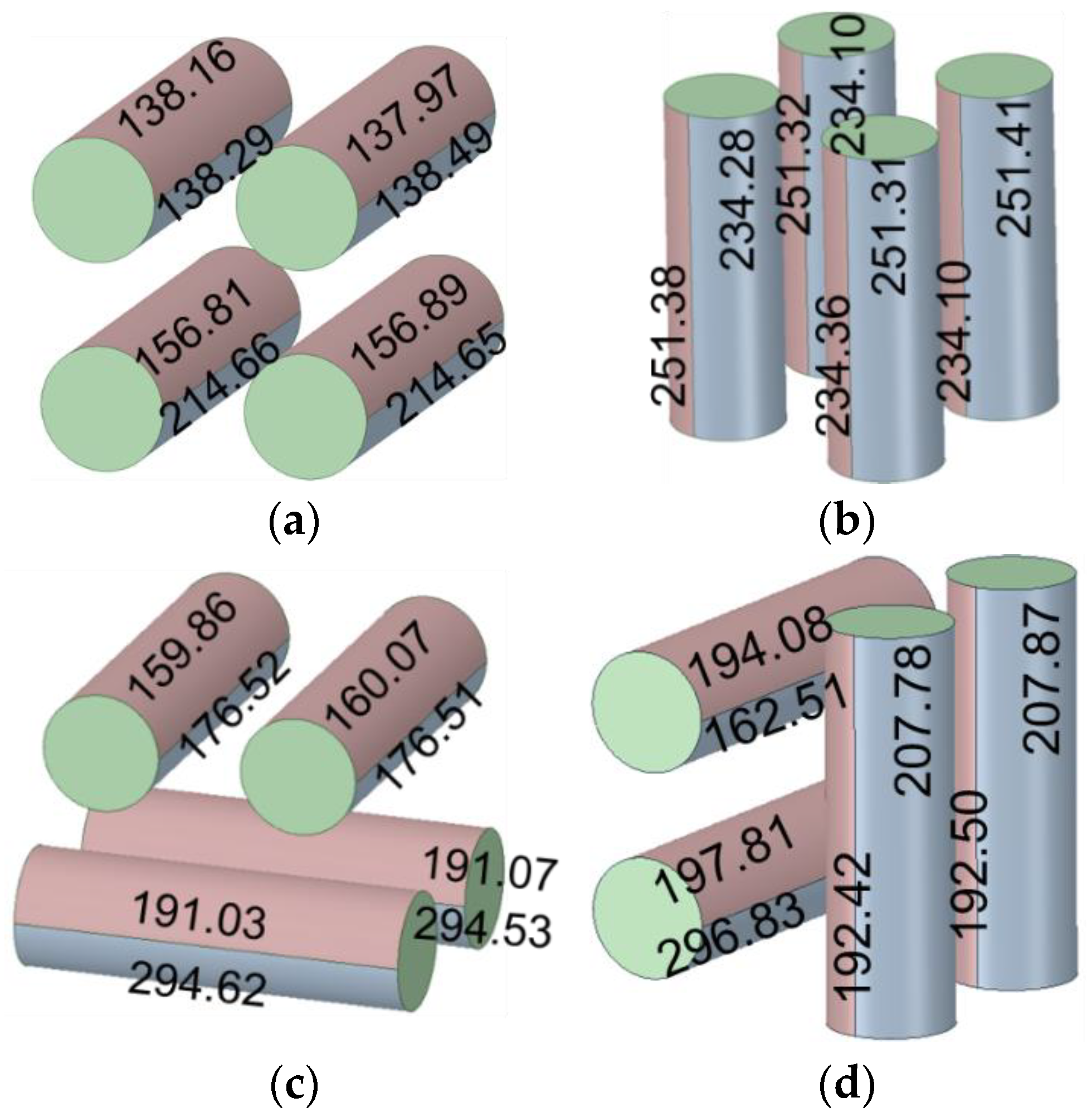
- The air-cooled lower horizontal batteries dissipate 34% more heat than the upper ones in a unidirectional arrangement. When arranged in a crossover configuration, the lower ones dissipate more heat than the upper ones by 44%.
- Arranging the air-cooled batteries vertically and in a crossover fashion yields both the lowest maximum battery temperature and volumetric average battery temperature. However, the smallest temperature difference and greatest surface heat flux can only be achieved if the batteries are placed vertically and unidirectionally.
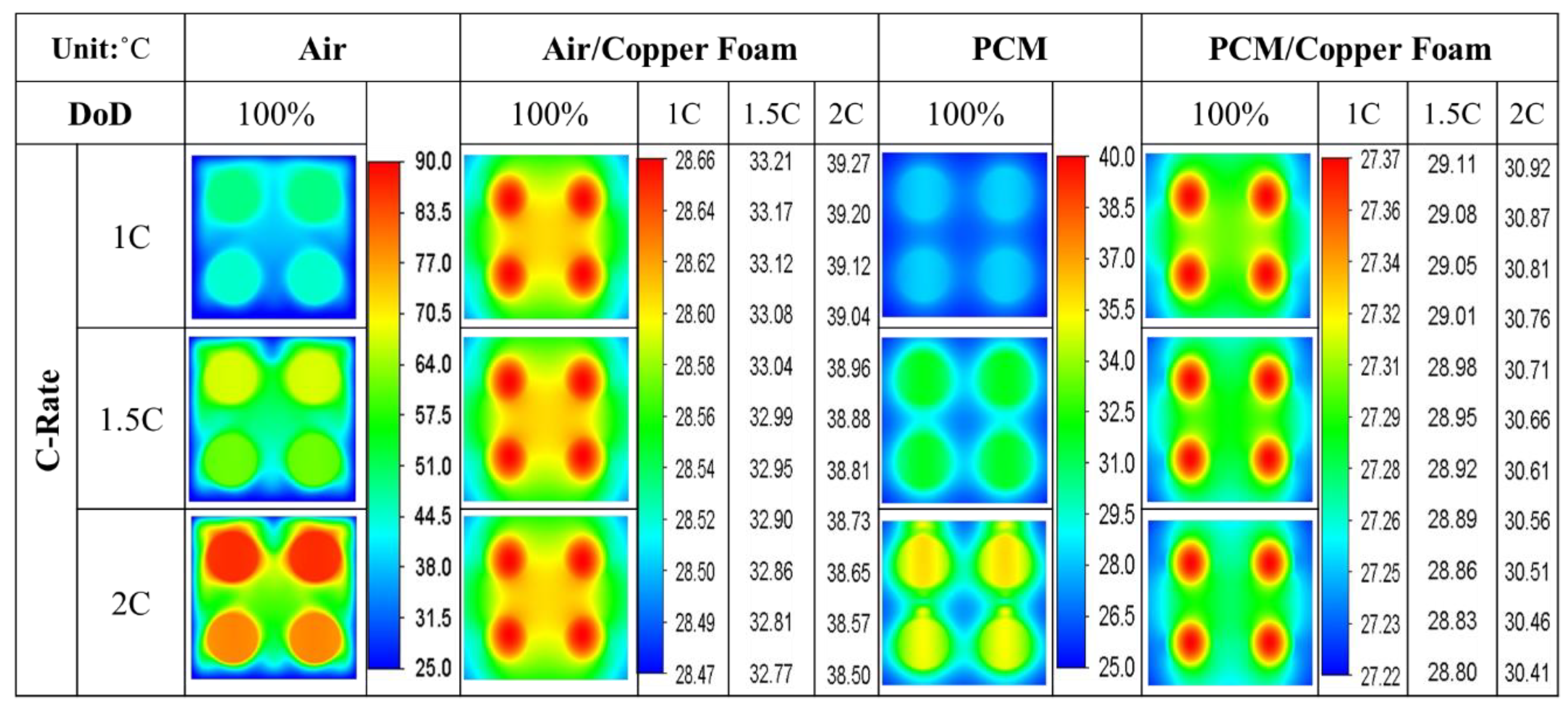
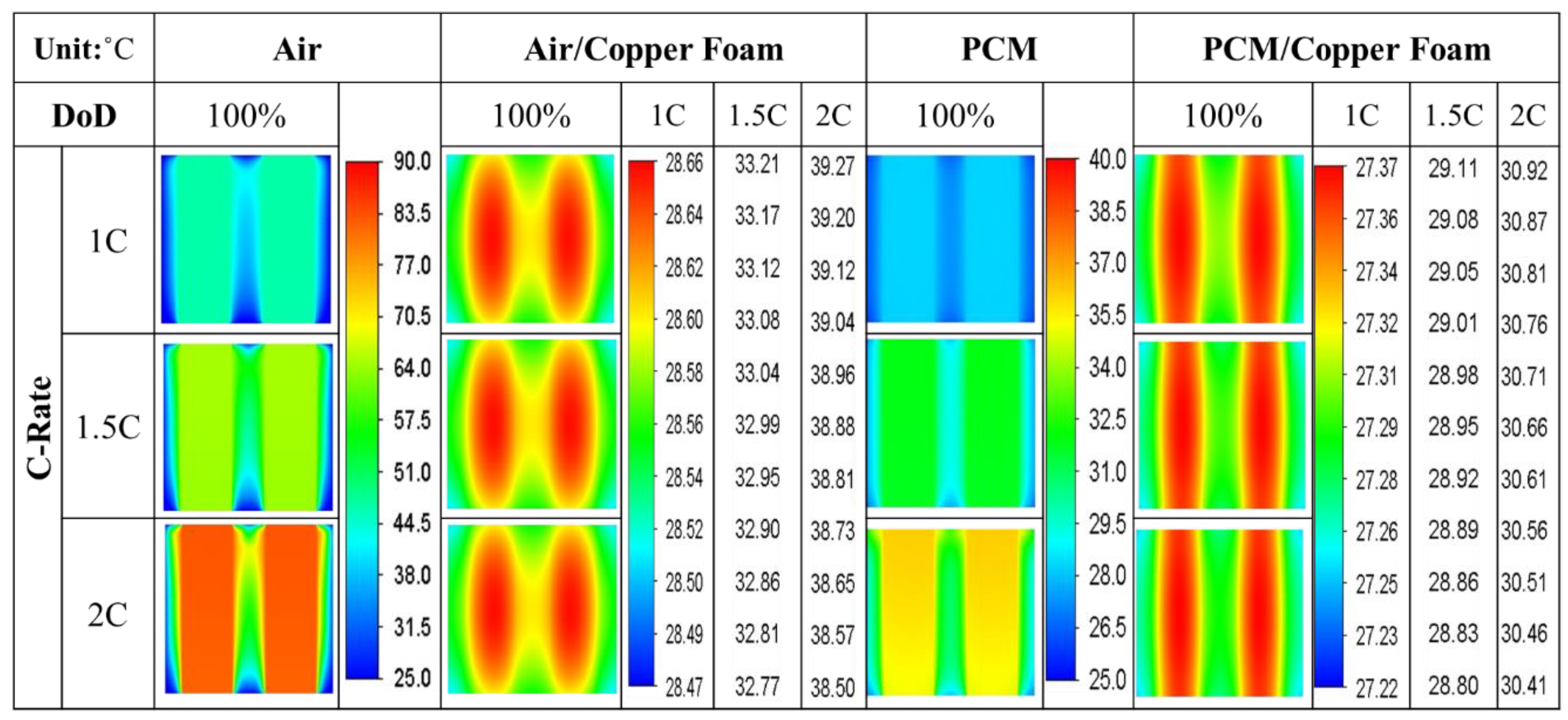
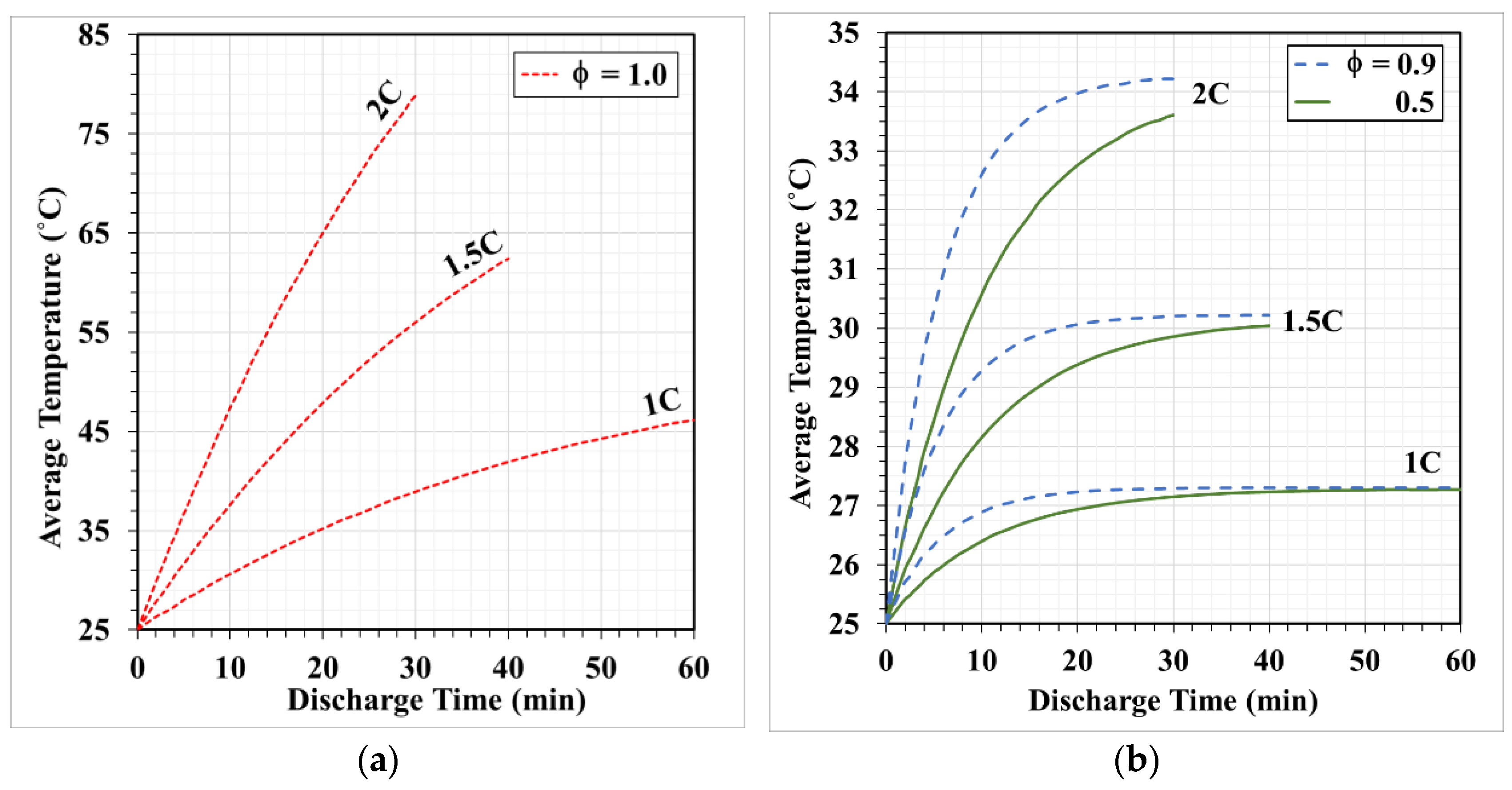
- The average temperature of a purely air-cooled 21700 battery may reach 79 °C under a 2C discharge. The installation of copper foam may reduce this temperature below 35 °C. If the air in the foam is entirely replaced with PCM, this temperature may be further reduced to approximately 31 °C.
- Air cooling is only acceptable if the battery discharge rate is less than 1C. If the battery continues discharging at a rate exceeding 1.5C until it is exhausted, either pure PCM or copper foam saturated with air should be used. If there is a need for high temperature uniformity, then copper foam saturated with PCM turns out to be a viable option.
- Copper foam saturated with PCM performs favorably in enhancing battery cooling effectiveness. The foam porosity is recommended to range between 0.90 and 0.97. Otherwise, the volumetric average temperature of the batteries may increase. When discharged at 2C, decreasing the foam porosity from 0.95 to 0.5 may increase the volumetric average battery temperature from 30 °C to 31 °C.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hwang, F.S.; Confrey, T.; Reidy, C.; Picovici, D.; Callaghan, D.; Culliton, D.; Nolan, C. Review of battery thermal management systems in electric vehicles. Renew. Sustain. Energy Rev. 2024, 192, 114171. [Google Scholar] [CrossRef]
- Khan, S.A.; Hussain, I.; Thakur, A.K.; Yu, S.; Lau, K.T.; He, S.; Dong, K.; Chen, J.; Li, X.; Ahmad, M.; et al. Advancements in battery thermal management system for fast charging/discharging applications. Energy Storage Mater. 2023, 65, 103144. [Google Scholar] [CrossRef]
- Bernardi, D.; Pawlikowski, E.; Newman, J. A general energy balance for battery systems. J. Electrochem. Soc. 1985, 132, 5–12. [Google Scholar] [CrossRef]
- Yao, T.P.; Forman, B.M.; Jiang, Z.; Cherbas, L.; Chen, J.D.; McKeown, M.; Cherbas, P.; Evans, R.M. Functional ecdysone receptor is the product of EcR and Ultraspiracle genes. Nature 1993, 366, 476–479. [Google Scholar] [CrossRef] [PubMed]
- Al-Hallaj, S.; Maleki, H.; Hong, J.S.; Selman, J.R. Thermal modeling and design considerations of lithium-ion batteries. J. Power Sources 1999, 83, 1–8. [Google Scholar] [CrossRef]
- Sato, N. Thermal behavior analysis of lithium-ion batteries for electric and hybrid vehicles. J. Power Sources 2001, 99, 70–77. [Google Scholar] [CrossRef]
- Jeon, D.H.; Baek, S.M. Thermal modeling of cylindrical lithium ion battery during discharge cycle. Energy Convers. Manag. 2011, 52, 2973–2981. [Google Scholar] [CrossRef]
- Liu, G.M.; Ouyang, M.G.; Lu, L.G.; Li, J.Q.; Han, X.B. Analysis of the heat generation of lithium-ion battery during charging and discharging considering different influencing factors. J. Therm. Anal. Calorim. 2013, 116, 1001–1010. [Google Scholar] [CrossRef]
- Jarrett, A.; Kim, I.Y. Design optimization of electric vehicle battery cooling plates for thermal performance. J. Power Source 2011, 196, 10359–10368. [Google Scholar] [CrossRef]
- Tran, T.H.; Harmand, S.; Desmet, B.; Filangi, S. Experimental investigation on the feasibility of heat pipe cooling for HEV/EV lithium-ion battery. Appl. Therm. Eng. 2014, 63, 551–558. [Google Scholar] [CrossRef]
- Zhou, R.; Gu, J.J.; Liu, J. An experimental study of heat pipe thermal management system with wet cooling method for lithium-ion batteries. J. Power Source 2015, 273, 1089–1097. [Google Scholar] [CrossRef]
- Wang, Z.W.; Zhang, H.Y.; Xia, X. Experimental investigation on the thermal behavior of cylindrical battery with composite paraffin and fin structure. Int. J. Heat Mass Transf. 2017, 109, 958–970. [Google Scholar] [CrossRef]
- Ye, X.; Zhao, Y.H.; Quan, Z.H. Experimental study on heat lithium-ion battery based on micro heat pipe array (MHPA). Appl. Therm. Eng. 2018, 130, 74–82. [Google Scholar] [CrossRef]
- Yu, Z.; Zhang, J.; Pan, W. A review of battery thermal management systems about heat pipe and phase change materials. J. Energy Storage 2023, 62, 106827. [Google Scholar] [CrossRef]
- Han, U.; Jun, Y.J.; Choi, H.; Lee, H. Thermal performance analysis and optimization of heat pipe-assisted hybrid fin structure for lithium battery thermal management for extreme thermal conditions. Int. Commun. Heat Mass Transf. 2023, 149, 107128. [Google Scholar] [CrossRef]
- Lin, X.W.; Li, Y.B.; Wu, W.T.; Zhou, Z.F.; Chen, B. Advances on two-phase heat transfer for lithium-ion battery thermal management. Renew. Sustain. Energy Rev. 2024, 189, 114052. [Google Scholar] [CrossRef]
- Chaudhari, J.; Singh, G.K.; Rathod, M.K.; Ali, H.M. Experimental and computational analysis on lithium-ion battery thermal management system utilizing air cooling with radial fins. J. Therm. Anal. Calorim. 2024, 149, 203–218. [Google Scholar] [CrossRef]
- Lyu, Y.; Siddique, A.R.M.; Majid, S.H.; Biglarbegian, M.; Gadsden, S.A.; Mahmud, S. Electric vehicle battery thermal management system with thermoelectric cooling. Energy Rep. 2019, 5, 822–827. [Google Scholar] [CrossRef]
- Lyu, Y.; Siddique, A.R.M.; Gadsden, S.A.; Mahmud, S. Experimental investigation of thermoelectric cooling for a new battery pack design in a copper holder. Results Eng. 2021, 10, 100214. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, C.F.; Zhou, J.G.; Xiong, X.; Wang, Y.P. Thermal performance of battery thermal management system using fins to enhance the combination of thermoelectric Cooler and phase change Material. Appl. Energy 2022, 322, 119503. [Google Scholar] [CrossRef]
- Hameed, M.M.; Mansor, M.B.; Azau, M.A.M.; Alshara, A.K. Computational design and analysis of LiFePO4 battery thermal management system (BTMS) using thermoelectric cooling/thermoelectric generator (TEC–TEG) in electric vehicles (EVs). J. Energy Storage 2023, 72, 108394. [Google Scholar] [CrossRef]
- Park, H. A design of air flow configuration for cooling lithium-ion battery in hybrid electric vehicles. J. Power Source 2013, 239, 30–36. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, J. Design a J-type air-based battery thermal management system through surrogate-based optimization. Appl. Energy 2019, 252, 113426. [Google Scholar] [CrossRef]
- Zhang, F.; Lin, A.; Wang, P.; Liu, P. Optimization design of a parallel air-cooled battery thermal management system with spoilers. Appl. Therm. Eng. 2021, 182, 116062. [Google Scholar] [CrossRef]
- Chen, J.; Zhao, X.; Wang, B.; Zhang, C.; Xuan, D. Multiobjective optimization of air-cooled battery thermal management system based on heat dissipation model. Ionics 2021, 27, 1307–1322. [Google Scholar] [CrossRef]
- Yang, C.; Xi, H.; Wang, M. Structure optimization of air cooling battery thermal management system based on lithium-ion battery. J. Energy Storage 2023, 59, 106538. [Google Scholar] [CrossRef]
- Kumar, R.; Singh, L.K.; Gupta, A.K. Evaluating air-cooling performance of lithium-ion-battery module with various cell arrangements. Energy Technol. 2024, 12, 2301061. [Google Scholar] [CrossRef]
- Huang, C.-R.; Leong, J.C. Model simplification of lithium-ion battery module heating for electric vehicle. In Proceedings of the 471st International Conference on Heat Transfer and Fluid Flow (ICHTFF), Houston, TX, USA, 23–24 January 2019. [Google Scholar]
- Falcone, M.; Palka Bayard De Volo, E.; Hellany, A.; Rossi, C.; Pulvirenti, B. Lithium-ion battery thermal management systems: A survey and new CFD results. Batteries 2021, 7, 86. [Google Scholar] [CrossRef]
- Jeon, H.; Hong, S.; Yun, J.; Han, J. Cooling strategy optimization of cylindrical lithium-ion battery pack via multi-counter cooling channels. Energies 2023, 16, 7860. [Google Scholar] [CrossRef]
- Isfahani, M.S.; Gharehghani, A.; Saeedipour, S.; Rabiei, M. PCM/metal foam and microchannels hybrid thermal management system for cooling of Li-ion battery. J. Energy Storage 2023, 72, 108789. [Google Scholar] [CrossRef]
- Kumar, K.; Sarkar, J.; Mondal, S.S. Multi-scale-multi-domain simulation of novel microchannel-integrated cylindrical Li-ion battery thermal management: Nanoparticle shape effect. J. Energy Storage 2024, 84, 110824. [Google Scholar] [CrossRef]
- Li, W.; Wang, Y.; Yang, W.; Zhang, K. Design and optimization of an integrated liquid cooling thermal management system with a diamond-type channel. Therm. Sci. Eng. Prog. 2024, 47, 102325. [Google Scholar] [CrossRef]
- Coşkun, T.; Çetkin, E. Cold plate enabling air and liquid cooling simultaneously: Experimental study for battery pack thermal management and electronic cooling. Int. J. Heat Mass Transf. 2023, 217, 124702. [Google Scholar] [CrossRef]
- Shahid, S.; Agelin-Chaab, M. Investigation of heat transfer enhancement techniques on a scalable novel hybrid thermal management strategy for lithium-ion battery packs. Batteries 2024, 10, 32. [Google Scholar] [CrossRef]
- Tousi, M.; Sarchami, A.; Kiani, M.; Najafi, M.; Houshfar, E. Numerical study of novel liquid-cooled thermal management system for cylindrical Li-ion battery packs under high discharge rate based on AgO nanofluid and copper sheath. J. Energy Storage 2021, 41, 102910. [Google Scholar] [CrossRef]
- Kiani, M.; Omiddezyani, S.; Nejad, A.M.; Ashjaee, M.; Houshfar, E. Novel hybrid thermal management for Li-ion batteries with nanofluid cooling in the presence of alternating magnetic field: An experimental study. Case Stud. Therm. Eng. 2021, 28, 101539. [Google Scholar] [CrossRef]
- Liao, G.; Wang, W.; Zhang, F.; Jiaqiang, E.; Chen, J.; Leng, E. Thermal performance of lithium-ion battery thermal management system based on nanofluid. Appl. Therm. Eng. 2022, 216, 118997. [Google Scholar] [CrossRef]
- Guo, Z.; Wang, Y.; Zhao, S.; Zhao, T.; Ni, M. Investigation of battery thermal management system with considering effect of battery aging and nanofluids. Int. J. Heat Mass Transf. 2023, 202, 123685. [Google Scholar] [CrossRef]
- Khateeb, S.A.; Amiruddin, S.; Farid, M.; Selman, J.R.; Al-Hallaj, S.A. Thermal management of Li-ion battery with phase change material for electric scooters: Experimental validation. J. Power Source 2005, 142, 345–353. [Google Scholar] [CrossRef]
- Duan, X.; Naterer, G.F. Heat transfer in phase change materials for thermal management of electric vehicle battery modules. Int. J. Heat Mass Transf. 2010, 53, 5176–5182. [Google Scholar] [CrossRef]
- Li, W.Q.; Qu, Z.G.; He, Y.L.; Tao, Y.B. Experimental study of a passive thermal management system for high-powered lithium-ion batteries using porous metal foam saturated with phase materials. J. Power Source 2014, 255, 9–15. [Google Scholar] [CrossRef]
- Wilke, S.; Schweitzer, B.; Khateeb, S.; Al-Hallaj, S.A. Preventing thermal runaway propagation in lithium ion battery packs using a phase change composite material: An experimental study. J. Power Source 2017, 340, 51–59. [Google Scholar] [CrossRef]
- Nasajpour-Esfahani, N.; Garmestani, H.; Rozati, M.; Smaisim, G.F. The role of phase change materials in lithium-ion batteries: A brief review on current materials, thermal management systems, numerical methods, and experimental models. J. Energy Storage 2023, 63, 107061. [Google Scholar] [CrossRef]
- Talele, V.; Thorat, P.; Gokhale, Y.P.; Desai, H. Technical review on battery thermal management system for electric vehicle application. In Energy Storage Systems; Springer: Singapore, 2023; pp. 177–225. [Google Scholar]
- Lakhotia, V.K.; Kumar, R.S. Review on various types of battery thermal management systems. J. Therm. Anal. Calorim. 2023, 148, 12335–12368. [Google Scholar] [CrossRef]
- Zhou, R.; Chen, Y.; Zhang, J.; Guo, P. Research progress in liquid cooling technologies to enhance the thermal management of LIBs. Mater. Adv. 2023, 4, 4011–4040. [Google Scholar] [CrossRef]
- Chen, M.; Yu, Y.; Ouyang, D.; Weng, J.; Zhao, L.; Wang, J.; Chen, Y. Research progress of enhancing battery safety with phase change materials. Renew. Sustain. Energy Rev. 2024, 189, 113921. [Google Scholar] [CrossRef]
- Sabbah, R.; Kizilel, R.; Selman, J.R.; Al-Hallaj, S.A. Active (air-cooled) vs. passive (phase change material) thermal management of high power lithium-ion packs: Limitation of temperature rise and uniformity of temperature distribution. J. Power Source 2008, 182, 630–638. [Google Scholar] [CrossRef]
- Kizilel, R.; Sabbah, R.; Selman, J.R.; Al-Hallaj, S.A. An alternative cooling system to enhance the safety of Li-ion battery packs. J. Power Source 2009, 194, 1105–1112. [Google Scholar] [CrossRef]
- Ramandi, M.Y.; Dincer, I.; Naterer, G.F. Heat transfer and thermal management of electric vehicle batteries with phase change materials. Heat Mass Transf. 2011, 47, 777–788. [Google Scholar] [CrossRef]
- Ling, Z.Y.; Chen, J.J.; Fang, X.M.; Zhang, Z.G.; Xu, T.; Gao, X.N.; Wang, S.F. Experimental and numerical investigation of the application of phase change materials in a simulative power batteries thermal management system. Appl. Energy 2014, 121, 104–113. [Google Scholar] [CrossRef]
- Jiang, G.W.; Huang, J.H.; Fu, Y.S.; Cao, M.; Liu, M.C. Thermal optimization of composite phase change material/expanded graphite for Li-ion battery thermal management. Appl. Therm. Eng. 2016, 108, 1119–1125. [Google Scholar] [CrossRef]
- Bai, F.F.; Chen, M.B.; Song, W.J.; Feng, Z.P.; Li, Y.L.; Ding, Y.L. Thermal management performances of PCM/water cooling-plate using for lithium-ion battery module based on non-uniform internal heat source. Appl. Therm. Eng. 2017, 126, 17–27. [Google Scholar] [CrossRef]
- Zhao, C.; Li, Y.; Liu, Y.; Zhu, D.; Ma, M.; Yu, W. Polyurethane foam skeleton-based phase change hydrogel for efficient battery thermal management with favorable antivibration performance. ACS Appl. Mater. Interfaces 2023, 15, 49653–49664. [Google Scholar] [CrossRef] [PubMed]
- Kiani, M.; Ansari, M.; Arshadi, A.A.; Houshfar, E.; Ashjaee, M. Hybrid thermal management of lithium-ion batteries using nanofluid, metal foam, and phase change material: An integrated numerical–experimental approach. J. Therm. Anal. Calorim. 2020, 141, 1703–1715. [Google Scholar] [CrossRef]
- Liu, H.; Shi, G.; Mei, J.; Li, Q.; Wang, Z. Experimental study on the thermal management performance of lithium-ion battery using phase-change material at various ambient temperatures. Energy Technol. 2024, 12, 2300790. [Google Scholar] [CrossRef]
- Huo, Z.; Hong, X.; Li, Y.; Chen, Z.; Ruan, D. Numerical study of paraffin and glass fiber composites for thermal management of lithium-ion battery packs. Asia-Pac. J. Chem. Eng. 2024, 19, e2989. [Google Scholar] [CrossRef]
- Li, Z.R.; Liang, G.N.; Ding, Y.D.; Liao, Q.; Zhu, X.; Cheng, M. Experimental study on the thermal management performance of lithium-ion battery with PCM combined with 3-D finned tube. Appl. Therm. Eng. 2024, 245, 122794. [Google Scholar] [CrossRef]
- Sadrameli, S.M.; Azizi, Y. Thermal management of a LiFEPO4 battery pack in a cold temperature environment using phase change materials (PCMs). JOM 2024, 76, 853–862. [Google Scholar] [CrossRef]
- Temel, U.N.; Kilinc, F.; Coskun, S. Thermal protection performances of the macro and/or nano enhanced PCM in a representative battery pack. Nanosc. Microsc. Therm. 2022, 26, 52–66. [Google Scholar] [CrossRef]
- Jilte, R.; Afzal, A.; Panchal, S. A novel battery thermal management system using nano-enhanced phase change materials. Energy 2021, 219, 119564. [Google Scholar] [CrossRef]
- Mohammad, M.H.; Sepehr, M.; Majid, S. Battery thermal management with thermal energy storage composites of PCM, metal foam, fin and nanoparticle. J. Energy Storage 2020, 28, 101235. [Google Scholar]
- Yousefi, E.; Khaboshan, H.N.; Jaliliantabar, F.; Abdullah, A.A. The effect of different enclosure materials and NePCMs on performance of battery thermal management system. Mater. Today Proc. 2023, 75, 1–9. [Google Scholar] [CrossRef]
- Malekipour, M.; Sabzpooshani, M.; Houshfar, E. Investigation on the effect of addition of nano-titanium oxide particles to phase change material in a hybrid system for battery cooling under constant heat flux. MEJ 2021, 53, 4379–4396. [Google Scholar]
- Jindal, P.; Sharma, P.; Kundu, M.; Singh, S.; Shukla, D.K.; Pawar, V.J.; Wei, Y.; Breedon, P. Computational fluid dynamics (CFD) analysis of graphene nanoplatelets for the cooling of a multiple tier Li-ion battery pack. Therm. Sci. Eng. Prog. 2022, 31, 101282. [Google Scholar] [CrossRef]
- Faraji, H.; Yıldız, Ç.; Arshad, A.; Arıcı, M.; Choukairy, K.; El Alami, M. Passive thermal management strategy for cooling multiple portable electronic components: Hybrid nanoparticles enhanced phase change materials as an innovative solution. J. Energy Storage 2023, 70, 108087. [Google Scholar] [CrossRef]
- Weragoda, D.M.; Tian, G.; Cai, Q.; Zhang, T.; Lo, K.H.; Gao, Y. Conceptualization of a novel battery thermal management system based on capillary-driven evaporative cooling. Therm. Sci. Eng. Prog. 2024, 47, 102320. [Google Scholar] [CrossRef]
- Haghighi, E.B.; Moghaddam, M. Analyzing thermal management methods of Li-ion battery modules. In Proceedings of the IEEE International Telecommunications Energy Conference (INTELEC), Turino, Italy, 7–11 October 2018. [Google Scholar]
- Singh, L.K.; Kumar, R.; Gupta, A.K.; Sharma, A.K.; Panchal, S. Computational study on hybrid air-PCM cooling inside lithium-ion battery packs with varying number of cells. J. Energy Storage 2023, 67, 107649. [Google Scholar] [CrossRef]
- Fan, R.; Zheng, N.; Sun, Z. Evaluation of fin intensified phase change material systems for thermal management of Li-ion battery modules. Int. J. Heat Mass Transf. 2021, 166, 120753. [Google Scholar] [CrossRef]
- Sun, Z.; Fan, R.; Zheng, N. Thermal management of a simulated battery with the compound use of phase change material and fins: Experimental and numerical investigations. Int. J. Therm. Sci. 2021, 165, 106945. [Google Scholar] [CrossRef]
- Weng, J.; Ouyang, D.; Yang, X.; Chen, M.; Zhang, G.; Wang, J. Optimization of the internal fin in a phase-change-material module for battery thermal management. Appl. Therm. Eng. 2020, 167, 114698. [Google Scholar] [CrossRef]
- Chen, H.; Abidi, A.; Hussein, A.K.; Younis, O.; Degani, M.; Heidarshenas, B. Investigation of the use of extended surfaces in paraffin wax phase change material in thermal management of a cylindrical lithium-ion battery: Applicable in the aerospace industry. J. Energy Storage 2022, 45, 103685. [Google Scholar] [CrossRef]
- Dagdevir, T.; Ding, Y. Numerical investigation of battery thermal management by using helical fin and composite phase change material. J. Energy Storage 2024, 75, 109674. [Google Scholar] [CrossRef]
- Choudhari, V.G.; Dhoble, A.S.; Panchal, S. Numerical analysis of different fin structures in phase change material module for battery thermal management system and its optimization. Int. J. Heat Mass Transf. 2020, 163, 120434. [Google Scholar] [CrossRef]
- Mansir, I.B.; Sinaga, N.; Farouk, N.; Aljaghtham, M.; Diyoke, C.; Nguyen, D.D. Numerical simulation of dimensions and arrangement of triangular fins mounted on cylindrical lithium-ion batteries in passive thermal management. J. Energy Storage 2022, 50, 104392. [Google Scholar] [CrossRef]
- Zhang, F.; Lu, F.; Liang, B.; Zhu, Y.; Gou, H.; Xiao, K.; He, Y. Thermal performance analysis of a new type of branch-fin enhanced battery thermal management PCM module. Renew. Energy 2023, 206, 1049–1063. [Google Scholar] [CrossRef]
- Liu, H.; Jin, C.; Li, H.; Ji, Y. A numerical study of PCM battery thermal management performance enhancement with fin structures. Energy Rep. 2023, 9, 1793–1802. [Google Scholar] [CrossRef]
- Wu, C.; Qiu, C.; Yuan, X.; Yuan, N.; Zhang, B.; Li, Y.; Qin, L.; Shi, H. Numerical study and optimization of battery thermal management systems (BTMS) Based on Fin-Phase change material (PCM) in variable gravity environments. Appl. Therm. Eng. 2024, 224, 122777. [Google Scholar] [CrossRef]
- Rashidi, S.; Ijadi, A.; Dadashi, Z. Potentials of porous materials for temperature control of lithium-ion batteries. J. Energy Storage 2022, 51, 104457. [Google Scholar] [CrossRef]
- Ranjbaran, Y.S.; Haghparast, S.J.; Shojaeefard, M.H.; Molaeimanesh, G.R. Numerical evaluation of a thermal management system consisting PCM and porous metal foam for Li-ion batteries. J. Therm. Anal. Calorim. 2020, 141, 1717–1739. [Google Scholar] [CrossRef]
- Bamdezh, M.A.; Molaeimanesh, G.R.; Zanganeh, S. Role of foam anisotropy used in the phase-change com-posite material for the hybrid thermal management system of lithium-ion battery. J. Energy Storage 2020, 32, 101778. [Google Scholar] [CrossRef]
- Sudhakaran, S.; Terese, M.; Mohan, Y.; Thampi, A.D.; Rani, S. Influence of various parameters on the cooling performance of battery thermal management systems based on phase change materials. Appl. Therm. Eng. 2023, 222, 119936. [Google Scholar] [CrossRef]
- Liu, G.; Xiao, T.; Wei, P.; Meng, X.; Yang, X.; Yan, J.; He, Y.L. Experimental and numerical studies on melt-ing/solidification of PCM in a horizontal tank filled with graded metal foam. Sol. Energy Mater. Sol. Cells 2023, 250, 112092. [Google Scholar] [CrossRef]
- Kurşun, B.; Toklu, E.; Polat, F.; Balta, M. The effect of outer container geometry on the thermal management of lithium-ion batteries with a combination of phase change material and metal foam. J. Energy Storage 2024, 80, 110227. [Google Scholar] [CrossRef]
- Nield, D.A.; Bejan, A. Convection in Porous Media, 3rd ed.; Springer: New York, NY, USA, 2006. [Google Scholar]
- Du Plessis, P.; Montillet, A.; Comiti, J.; Legrand, J. Pressure drop prediction for flow through high porosity metallic foams. Chem. Eng. Sci. 1994, 49, 3545–3553. [Google Scholar] [CrossRef]
- Yang, X.H.; Lu, T.J.; Kim, T. A simplistic model for the tortuosity in two-phase close-celled porous media. J. Phys. D Appl. 2013, 46, 125305. [Google Scholar] [CrossRef]
- Kitamura, K.; Kami-iwa, F.; Misumi, T. Heat transfer and fluid flow of natural convection around large horizontal cylinders. Int. J. Heat Mass Transf. 1999, 42, 4093–4106. [Google Scholar] [CrossRef]

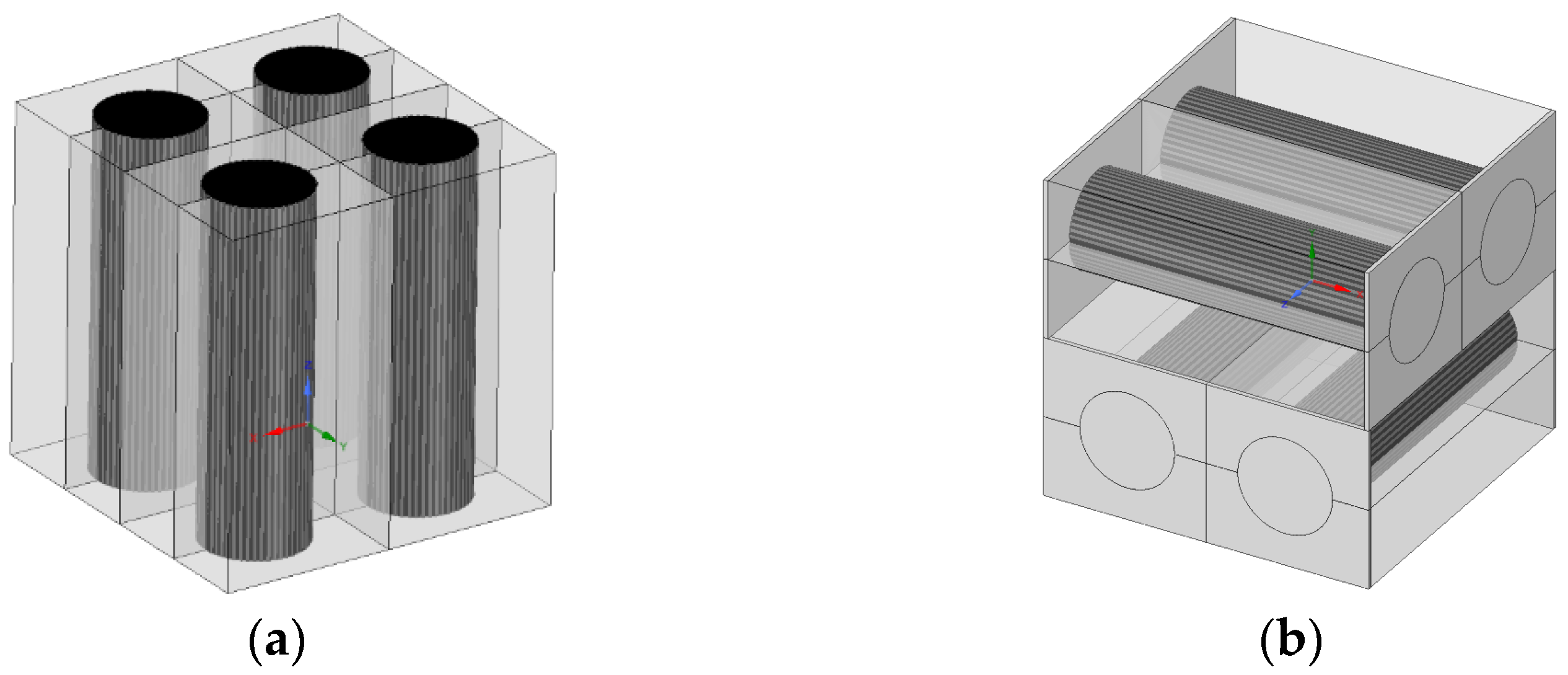
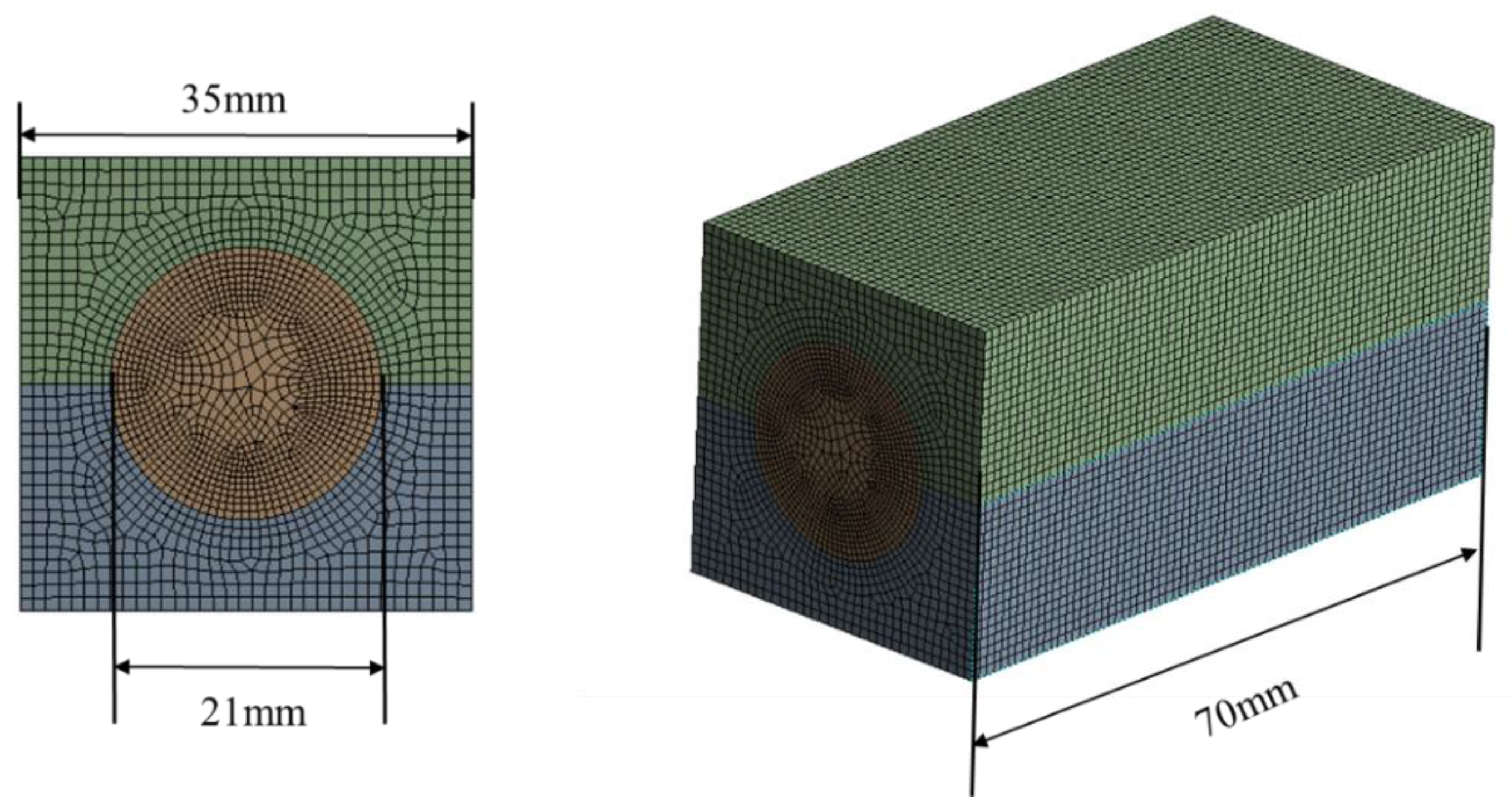




| Specification | Value |
|---|---|
| Rated discharge capacity (1C-rate) | 3.2 Ah |
| Nominal voltage | 3.56 V |
| Rated discharge energy | 11.4 Wh |
| Density | 2560 kg/m3 |
| Heat capacity | 1000 J/kg·K |
| Radial thermal conductivity | 1 W/m·K |
| Axial thermal conductivity | 25 W/m·K |
| Tangential thermal conductivity | 25 W/m·K |
| Internal resistance | 53 mΩ |
| Material Parameters | Solid (≤25 °C) | Liquid (≥50 °C) |
|---|---|---|
| Density ρ (kg/m3) | 910 | 769 |
| Thermal conductivity λ (W/m·K) | 0.423 | 0.146 |
| Specific heat capacity Cp (J/kg·K) | 1926 | 2400 |
| Thermal expansion coefficient β (1/K) | – | 8.161 × 10−4 |
| Reference temperature Tref (°C) | – | 50 |
| Melting point Tm (°C) | 36.4 | – |
| Latent heat of fusion L (kJ/kg) | 248 | |
| Material Parameters | Pure Copper | Air |
|---|---|---|
| Density ρ (kg/m3) | 8978 | varies 1 |
| Thermal conductivity λ (W/m·K) | 387.6 | 0.0242 |
| Specific heat capacity Cp (J/kg·K) | 381 | 1006.43 |
| Dynamic viscosity μ (kg/m·s) | – | 1.7894 × 10−5 |
| C-Rate | Heat Generation Rate (W) |
|---|---|
| 0.5 | 0.16 |
| 1 | 0.65 |
| 1.5 | 1.47 |
| 2 | 2.61 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, C.-L.; Leong, J.C. Analysis of Thermal Management Strategies for 21700 Lithium-Ion Batteries Incorporating Phase Change Materials and Porous Copper Foam with Different Battery Orientations. Energies 2024, 17, 1553. https://doi.org/10.3390/en17071553
Wang C-L, Leong JC. Analysis of Thermal Management Strategies for 21700 Lithium-Ion Batteries Incorporating Phase Change Materials and Porous Copper Foam with Different Battery Orientations. Energies. 2024; 17(7):1553. https://doi.org/10.3390/en17071553
Chicago/Turabian StyleWang, Chen-Lung, and Jik Chang Leong. 2024. "Analysis of Thermal Management Strategies for 21700 Lithium-Ion Batteries Incorporating Phase Change Materials and Porous Copper Foam with Different Battery Orientations" Energies 17, no. 7: 1553. https://doi.org/10.3390/en17071553
APA StyleWang, C.-L., & Leong, J. C. (2024). Analysis of Thermal Management Strategies for 21700 Lithium-Ion Batteries Incorporating Phase Change Materials and Porous Copper Foam with Different Battery Orientations. Energies, 17(7), 1553. https://doi.org/10.3390/en17071553








