Design and Analysis of a Linear Electric Generator for Harvesting Vibration Energy
Abstract
:1. Introduction
1.1. Background
1.2. Motivations and Contributions
- Detailed theoretical finite element modelling and simulation using Flux by Cedrat. The magnetic flux within and between the stator and mover cores was modelled to analyse machine reluctance. Air gap reluctance was reduced through the use of flanges on the stator teeth.
- Simulation studies were performed for various excitation modes in both static and parametric analyses. The modes studied include no mover coil excitation, a DC-excited mover coil, and an AC-excited mover coil.
- Construction and design of a practical linear generator were performed once the proposed design was verified. Calculations for the mechanical properties of the machine were undertaken to obtain the spring rate which would provide the desired resonant frequency.
- Verification and analysis of the hardware through the use of empirical testing with the use of a pneumatic actuator.
1.3. Paper Structure
2. Theoretical Aspects
2.1. Electromagnetism
2.2. Mechanics
3. Machine Design
4. Simulations
4.1. Modelling of the Generator
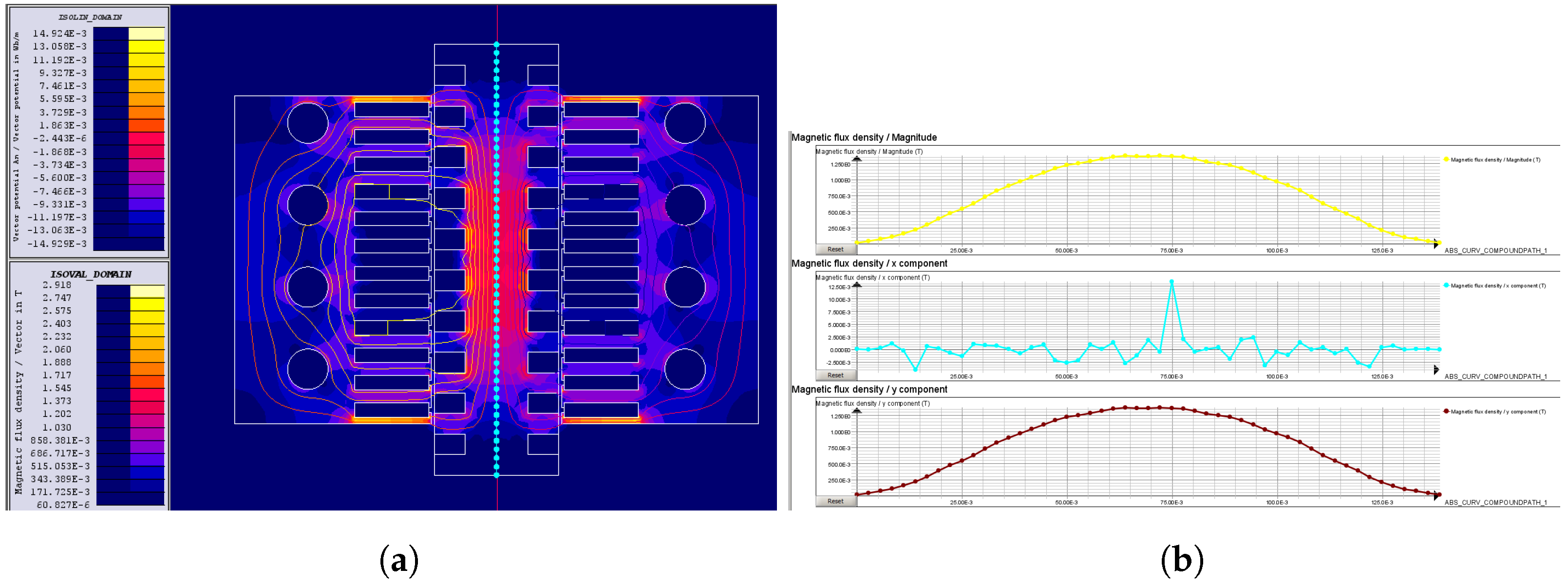
4.2. Magnetostatic Analysis
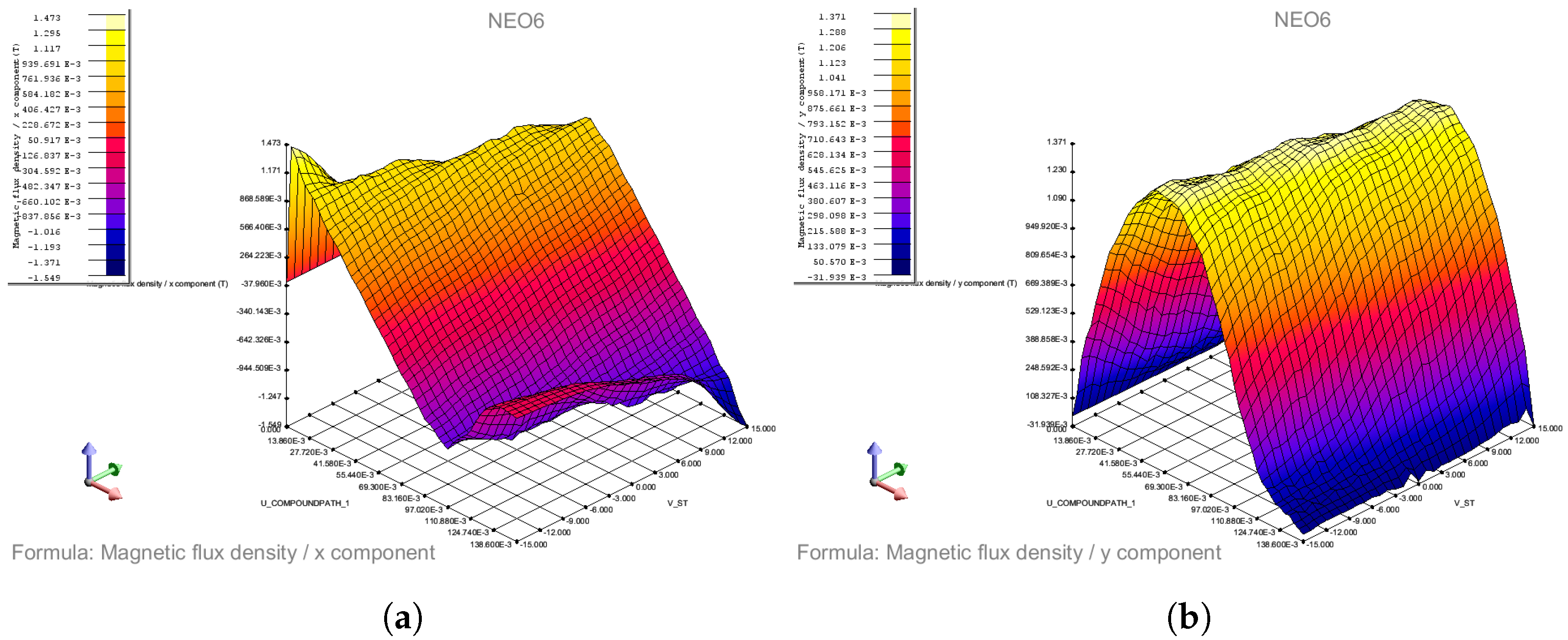
4.3. Parametric Analysis
4.3.1. No Mover Excitation
4.3.2. DC Excited Mover
4.3.3. AC Excited Mover
5. Hardware Tests
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ball, A.; Billing, J.; McCluskey, C.; Pham, P.; Pittman, O.; Lawson, S.; Ahmad, S.; Starr, A.; Rousseau, J.; Lambert, N. Australian Energy Statistics; Australian Government Department of Environment and Energy: Canberra, Australia, 2018. [Google Scholar]
- Höök, M.; Tang, X. Depletion of fossil fuels and anthropogenic climate change—A review. Energy Policy 2013, 52, 797–809. [Google Scholar] [CrossRef]
- Kulkarni, S.S.; Kulkarni, R.K. A Comprehensive Review of Energy Harvesting Techniques and its Potential Applications. Int. J. Comput. Appl. 2016, 139, 14–19. [Google Scholar]
- Ballo, A.; Bottaro, M.; Grasso, A.D. A Review of Power Management Integrated Circuits for Ultrasound-Based Energy Harvesting in Implantable Medical Devices. Appl. Sci. 2021, 11, 2487. [Google Scholar] [CrossRef]
- Hadas, Z.; Vetiska, V.; Vetiska, J.; Krejsa, J. Analysis and efficiency measurement of electromagnetic vibration energy harvesting system. Microsyst. Technol. 2016, 22, 1767–1779. [Google Scholar] [CrossRef]
- Aliabadi, M.H.; Hosseinian, S.H.; Moghani, S.J.; Abedi, M. Multisided linear induction generator, analytical modeling, 3-D finite element analysis and experimental test. Elektron. Elektrotech. 2013, 19, 8–14. [Google Scholar]
- Li, Z.; Yang, Z.; Naguib, H.; Zu, J. Design and Studies on a Low-Frequency Truss-Based Compressive-Mode Piezoelectric Energy Harvester. IEEE/ASME Trans. Mechatron. 2018, 23, 2849–2858. [Google Scholar] [CrossRef]
- Thein, C.K.; Foong, F.M.; Shu, Y.C. Damping ratio and power output prediction of an electromagnetic energy harvester designed through finite element analysis. Sens. Actuators A Phys. 2019, 286, 220–231. [Google Scholar] [CrossRef]
- Zhou, S.; Cao, J.; Inman, D.J.; Lin, J.; Liu, S.; Wang, Z. Broadband tristable energy harvester: Modeling and experiment verification. Appl. Energy 2014, 133, 33–39. [Google Scholar] [CrossRef]
- Thein, C.K.; Foong, F.M.; Shu, Y.C. Spring amplification and dynamic friction modelling of a 2DOF/2SDOF system in an electromagnetic vibration energy harvester—Experiment, simulation, and analytical analysis. Mech. Syst. Signal Process. 2019, 132, 232–252. [Google Scholar] [CrossRef]
- Vo, N.Y.P.; Le, T.D. Adaptive pneumatic vibration isolation platform. Mech. Syst. Signal Process. 2021, 133, 2019. [Google Scholar] [CrossRef]
- Zhao, L.C.; Zou, H.-X.; Zhao, Y.-J.; Wu, Z.-Y.; Liu, F.-R.; Wei, K.-X.; Zhang, W.-M. Hybrid energy harvesting for self-powered rotor condition monitoring using maximal utilization strategy in structural space and operation process. Appl. Energy 2022, 314, 118983. [Google Scholar] [CrossRef]
- Chen, G.; Au, C.; Chen, J. Textile Triboelectric Nanogenerators for Wearable Pulse Wave Monitoring. Trends Biotechnol. 2021, 39, 1078–1092. [Google Scholar] [CrossRef] [PubMed]
- Nguyen, H.T.; Genov, D.A.; Bardaweel, H. Vibration energy harvesting using magnetic spring based nonlinear oscillators: Design strategies and insights. Appl. Energy 2020, 269, 115102. [Google Scholar] [CrossRef]
- Gu, Y.; Liu, W.; Zhao, C.; Wang, P. A goblet-like non-linear electromagnetic generator for planar multi-directional vibration energy harvesting. Appl. Energy 2020, 266, 114846. [Google Scholar] [CrossRef]
- Cawthorne, W.R.; Famouri, P.; Chen, J.; Clark, N.N.; McDaniel, T.I.; Atkinson, R.J.; Nandkumar, S.; Atkinson, C.M.; Petreanu, S. Development of a linear alternator-engine for hybrid electric vehicle applications. IEEE Trans. Veh. Technol. 1999, 48, 1797–1802. [Google Scholar] [CrossRef]
- Ngwaka, U.; Jia, B.; Lawrence, C.; Wu, D.; Smallbone, A.; Roskilly, A.P. The characteristics of a Linear Joule Engine Generator operating on a dry friction principle. Appl. Energy 2019, 37, 49–59. [Google Scholar] [CrossRef]
- Boldea, I.; Nasar, S.A. Linear Electric Actuators and Generators; IEEE: New York, NY, USA, 1997. [Google Scholar]
- Prudell, J.; Stoddard, M.; Amon, E.; Brekken, T.K.A.; Jouanne, A.V. A permanent-magnet tubular linear generator for ocean wave energy conversion. IEEE Trans. Ind. Appl. 2010, 46, 2392–2400. [Google Scholar] [CrossRef]
- Shin, J.S.; Watanabe, R.; Koseki, T.; Kim, H.J. Practical design approach of a transverse flux linear synchronous motor for compact size, small mover weight, high efficiency, and low material cost. IEEE Trans. Magn. 2015, 51, 8200504. [Google Scholar]
- Tang, Y.; Paulides, J.J.H.; Motoasca, T.E.; Lomonova, E.A. Flux-switching machine with DC excitation. IEEE Trans. Magn. 2012, 48, 3583–3586. [Google Scholar] [CrossRef]
- Li, J.; Li, W.; Li, R.; Ming, Z. A Five-Phase Doubly Fed Doubly Salient HTS Linear Motor for Vertical Transportation. IEEE Trans. Appl. Supercond. 2018, 28, 5203405. [Google Scholar] [CrossRef]
- Farrok, O.; Islam, M.R.; Guo, Y.; Zhu, J.; Xu, W. A Novel Design Procedure for Designing Linear Generators. IEEE Trans. Ind. Electron. 2018, 65, 1846–1854. [Google Scholar] [CrossRef]
- Farrok, O.; Islam, M.R.; Sheikh, M.R.I.; Guo, Y.; Zhu, J.G. Design and Analysis of a Novel Lightweight Translator Permanent Magnet Linear Generator for Oceanic Wave Energy Conversion. IEEE Trans. Magn. 2017, 53, 8207304. [Google Scholar] [CrossRef]
- Farrok, O.; Islam, M.R.; Sheikh, M.R.I.; Guo, Y.; Zhu, J.G. A Split Translator Secondary Stator Permanent Magnet Linear Generator for Oceanic Wave Energy Conversion. IEEE Trans. Ind. Electron. 2018, 65, 7600–7608. [Google Scholar] [CrossRef]
- Jun, J.; Shin, Y.; Kim, J.H. Linear electric generator with Halbach array to self-charge a smartphone. J. Vibroeng. 2016, 18, 587–594. [Google Scholar]
- Seo, U.J.; Riemer, B.; Appunn, R.; Hameyer, K. Design considerations of a linear generator for a range extender application. Arch. Electr. Eng. 2015, 64, 581–592. [Google Scholar] [CrossRef]
- Iacchetti, M.F.; Shuttleworth, R.; Zhang, M. Volt-ampere ratings in electronically tuned linear alternators for thermoacoustic engines. IET Renew. Power Gener. 2018, 12, 1256–1262. [Google Scholar] [CrossRef]
- Benamor, A.; Benchouia, M.T.; Srairi, K.; Benbouzid, M.E.H. A novel rooted tree optimization apply in the high order sliding mode control using super-twisting algorithm based on DTC scheme for DFIG. Int. J. Electr. Power Energy Syst. 2019, 108, 293–302. [Google Scholar] [CrossRef]
- Ayyarao, T.S.L.V. Modified vector controlled DFIG wind energy system based on barrier function adaptive sliding mode control. Prot. Control. Mod. Power Syst. 2019, 4, 4. [Google Scholar] [CrossRef]
- Jabr, H.M.; Lu, D.; Kar, N.C. Design and implementation of neuro-fuzzy vector control for wind-driven doubly-fed induction generator. IEEE Trans. Sustain. Energy 2011, 2, 404–413. [Google Scholar] [CrossRef]
- Cho, S.J.; Kim, J.H. Linear electromagnetic electric generator for harvesting vibration energy at frequencies more than 50 Hz. Adv. Mech. Eng. 2017, 9. [Google Scholar] [CrossRef]
- Kimoulakis, N.M.; Kladas, A.G.; Tegopoulos, J.A. Cogging force minimization in a coupled permanent magnet linear generator for sea wave energy extraction applications. IEEE Trans. Magn. 2009, 45, 1246–1249. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, K.; Zhou, M. Suppression of thrust fluctuation of doubly-fed linear motor. J. Mod. Transp. 2012, 20, 103–107. [Google Scholar] [CrossRef]
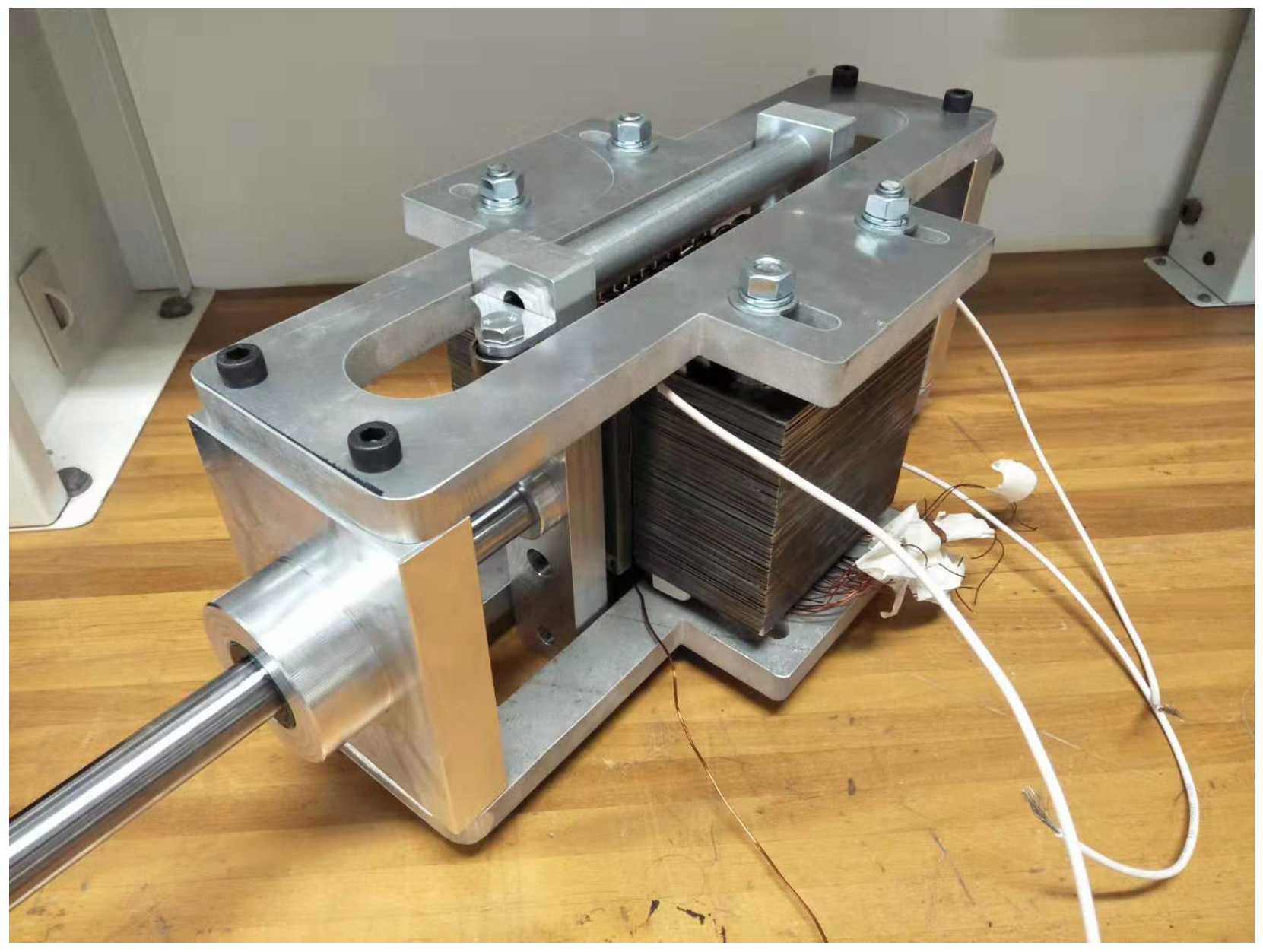
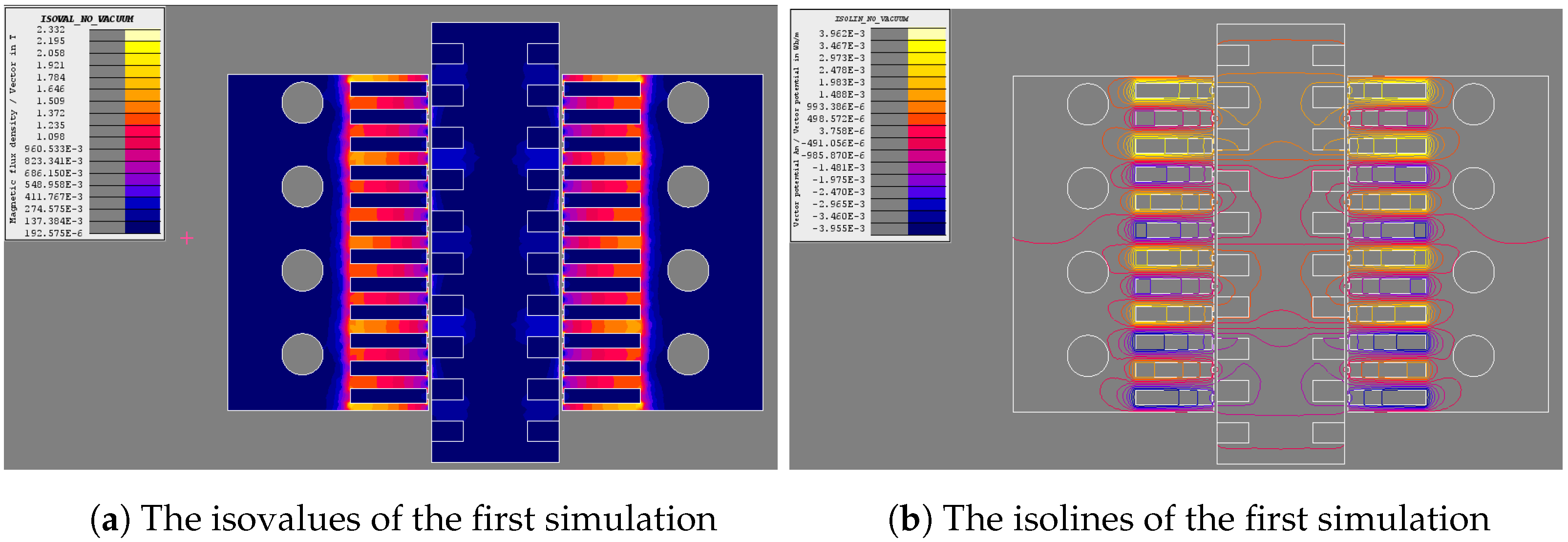
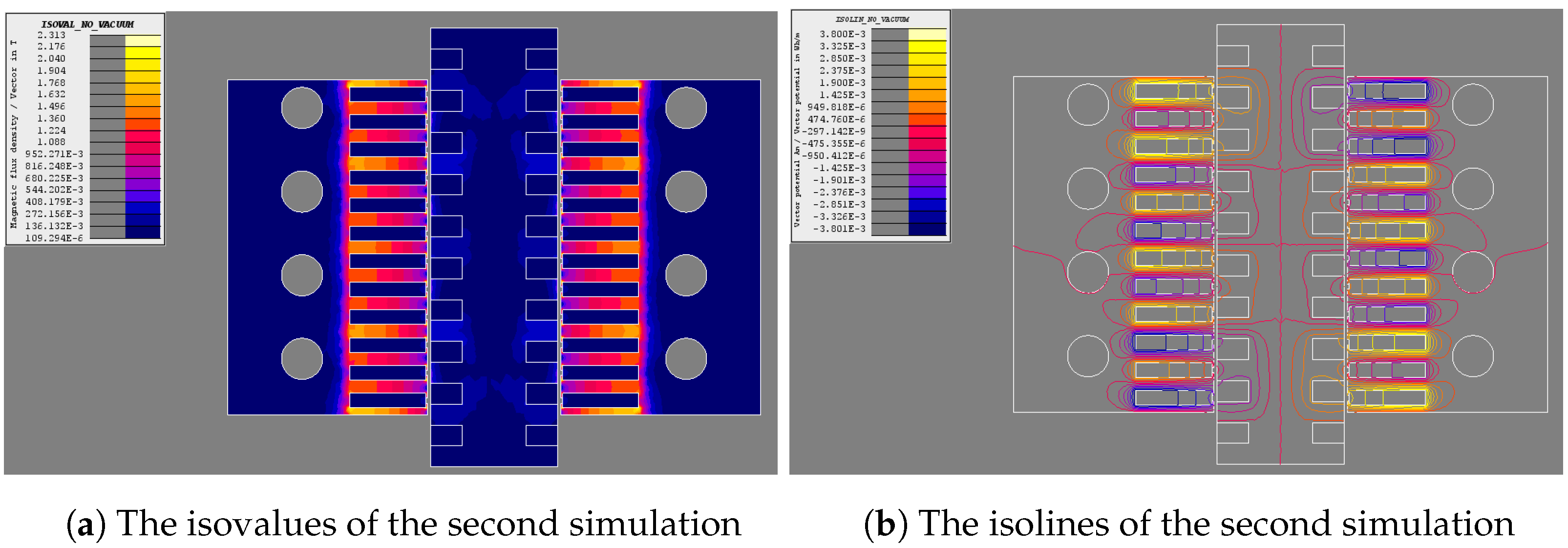
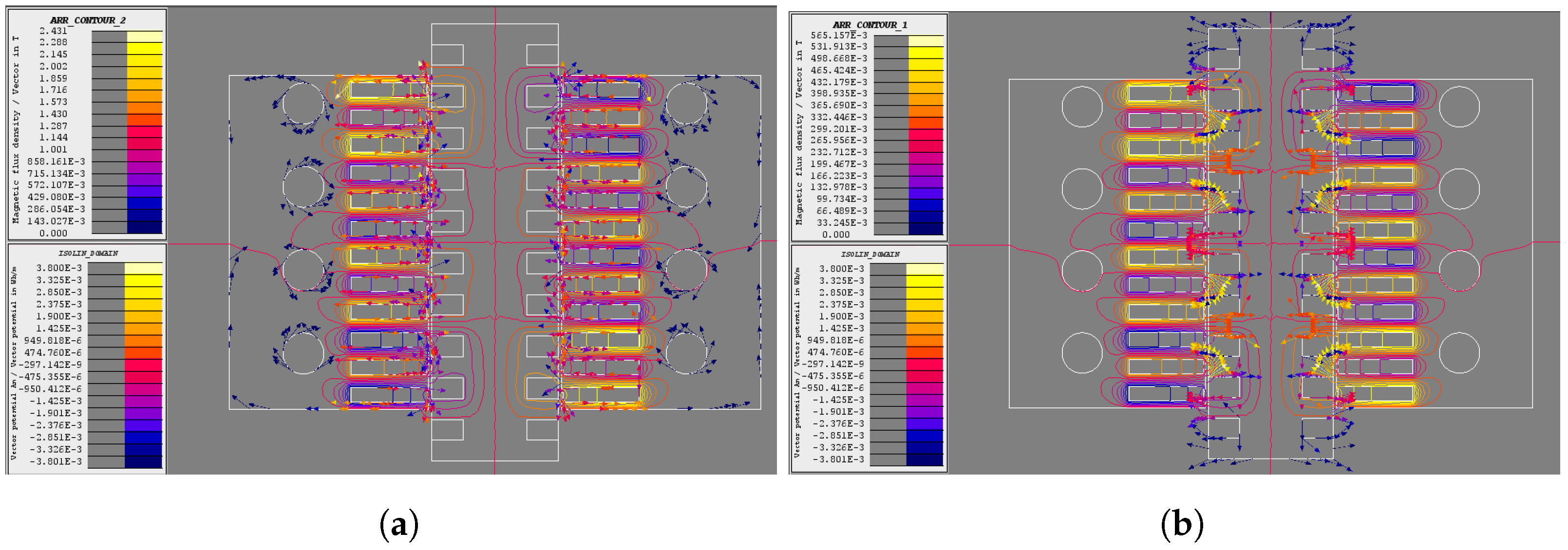


| Parameter | Value | Unit |
|---|---|---|
| Number of stator teeth | 13 | |
| Number of stator slots | 12 | |
| Stator pole pitch | 8.8 | mm |
| Depth of stator slot | 24 | mm |
| Number of mover teeth | 11 | |
| Number of mover slots | 10 | |
| Mover pole pitch | 13.2 | mm |
| Width of mover tooth | 6.6 | mm |
| Width of mover slot | 6.6 | mm |
| Depth of mover slot | 10 | mm |
| Mover length | 138.6 | mm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Then, J.; Agalgaonkar, A.P.; Safaei, F.; Muttaqi, K.M. Design and Analysis of a Linear Electric Generator for Harvesting Vibration Energy. Energies 2024, 17, 1715. https://doi.org/10.3390/en17071715
Then J, Agalgaonkar AP, Safaei F, Muttaqi KM. Design and Analysis of a Linear Electric Generator for Harvesting Vibration Energy. Energies. 2024; 17(7):1715. https://doi.org/10.3390/en17071715
Chicago/Turabian StyleThen, Joshua, Ashish P. Agalgaonkar, Farzad Safaei, and Kashem M. Muttaqi. 2024. "Design and Analysis of a Linear Electric Generator for Harvesting Vibration Energy" Energies 17, no. 7: 1715. https://doi.org/10.3390/en17071715
APA StyleThen, J., Agalgaonkar, A. P., Safaei, F., & Muttaqi, K. M. (2024). Design and Analysis of a Linear Electric Generator for Harvesting Vibration Energy. Energies, 17(7), 1715. https://doi.org/10.3390/en17071715





