System Analysis and Comparison Between a 2 MW Conventional Liquid Cooling System and a Novel Two-Phase Cooling System for Fuel Cell-Powered Aircraft
Abstract
1. Introduction
1.1. Background
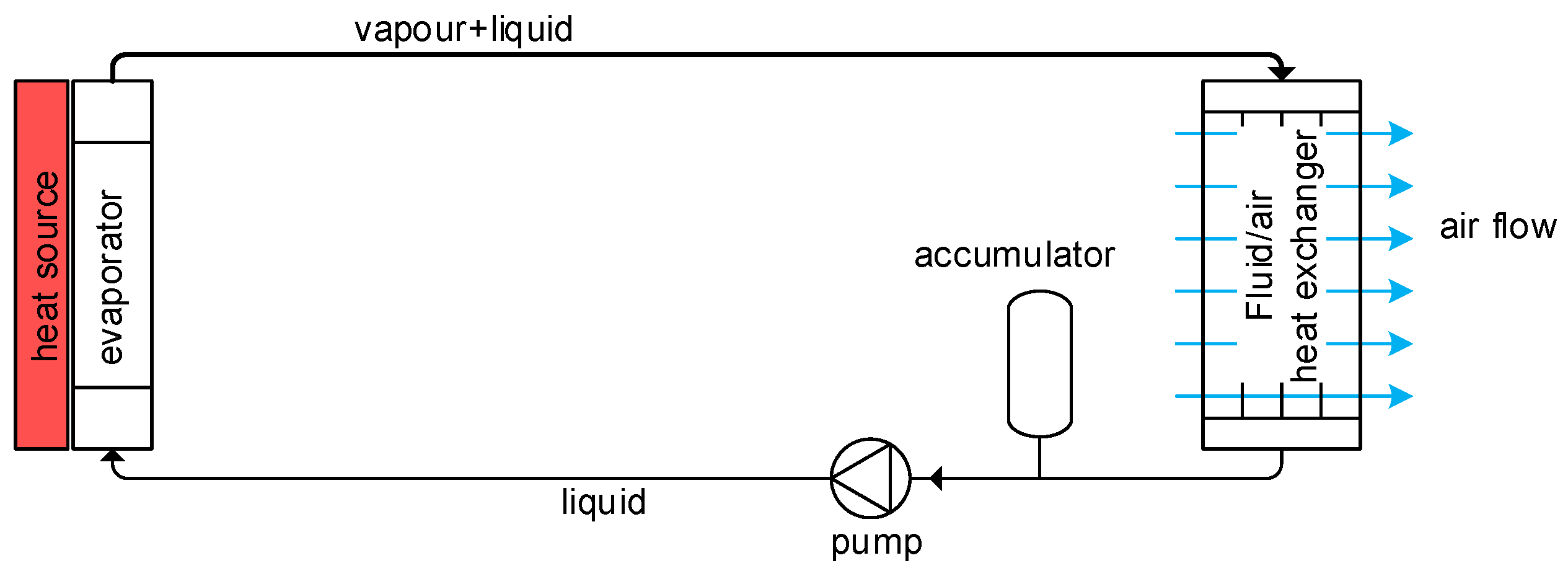
1.2. What Is a Two-Phase Pumped Cooling System?
- The required mass flow is an order of magnitude smaller. This results in much lower electrical power consumption of the pump and a much smaller pump mass. Also, the piping diameter can be smaller, which reduces the overall mass of the system.
- Freezing of the fluid under low ambient temperatures (−55 °C) is not possible, since the freezing points of fluids that are used for two-phase cooling are much lower (typically lower than −80 °C) than the freezing point of EGW (approximately −45 °C).
- Due to the low freezing point and high heat transfer coefficient of two-phase fluids, it is easier to use the waste heat from the fuel cell to warm liquid H2 before it enters the fuel cell.
- The heat transfer coefficient for evaporating or condensing flow is typically much higher than the heat transfer coefficient for liquid flow. This results in a smaller temperature difference between the fluid and the heat exchanger walls
1.3. Heat Source
1.4. Heat Sink
2. Analysis Methods
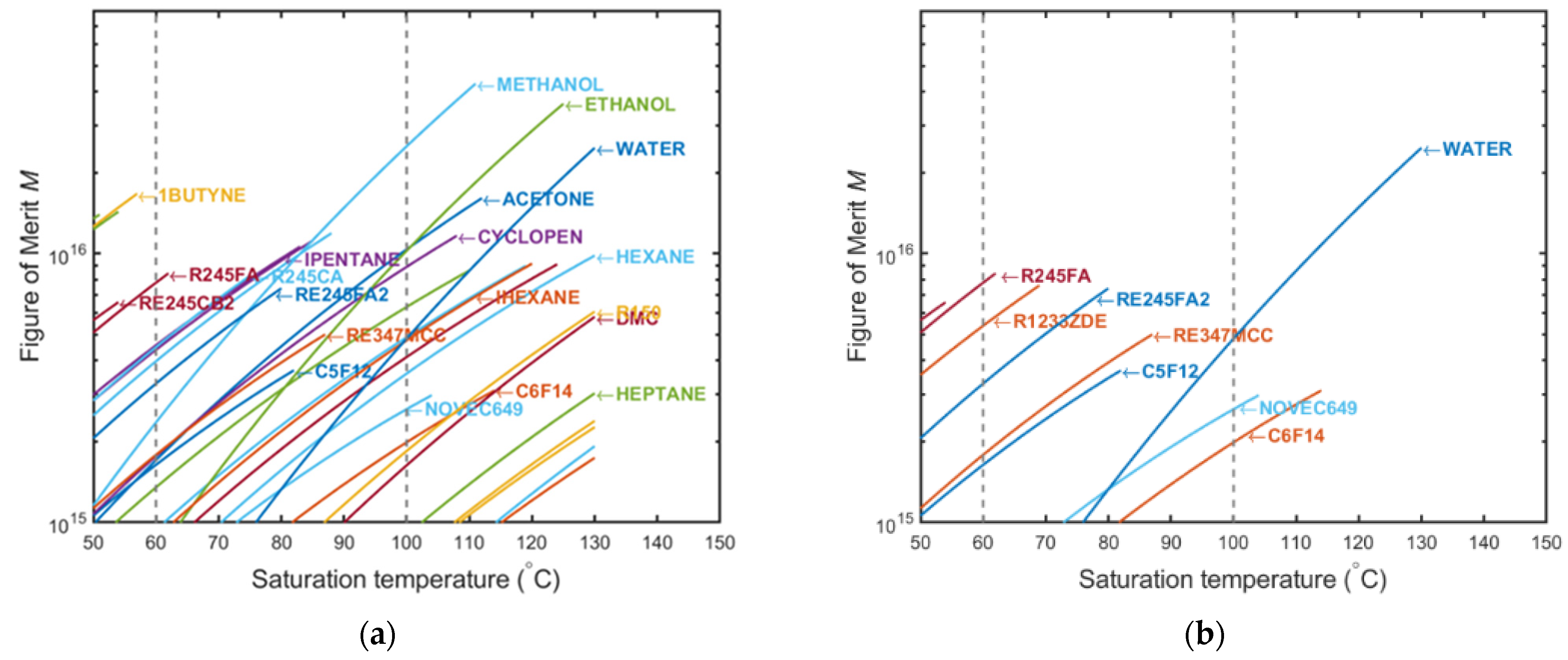
2.1. Fluid Preselection with “Figure of Merit”
| Methanol | RE347mcc (HFE-7000) | Water | Novec 649 | Fluorinert 72 (C6F14) | |
|---|---|---|---|---|---|
| NFPA (health/flammability) [15] | 1-3 | 3 1-0 | 0-0 | 3 1-0 | 1-0 |
| Global warming potential | ~0 | 530 | ~0 | 1 | 9300 |
| Saturation pressure at 95 °C (bar) | 3.0 | 6.0 | 0.8 | 3.9 | 3.1 |
| Saturation pressure at 60 °C (bar) | 0.85 | 2.4 | 0.20 | 1.5 | 1.1 |
| Saturation pressure at 20 °C (bar) | 0.13 | 0.59 | 0.02 | 0.33 | 0.24 |
| Triple point (°C) | −97 | −122 | 0 | −108 | −86 |
| Heat of evaporation hlv (kJ/kg) | 1035 | 104 | 2270 | 72 | 73 |
| Specific heat Cp (kJ/kgK) | 3.1 | 1.4 | 4.2 | 1.2 | 1.2 |
| Liquid density ρl (kg/m3) | 717 | 1184 | 962 | 1361 | 1446 |
| Density ratio ρl/ρv | 208 | 24 | 1915 | 28 | 36 |
| Conductivity kl (W/m K) | 0.19 | 0.05 | 0.68 | 0.05 | 0.06 |
| ∂Tsat/∂psat (°C/bar) | 10.1 | 6.8 | 31.8 | 9.9 | 12.2 |
2.2. System Analysis Tool
- Total heat rejection during take-off: 2 MW.
- Maximum power consumption (fans and pumps): 100 kW.
- Air temperature during take-off: 30 °C.
3. Results for Two-Phase Methanol
4. Results for Two-Phase Water
- FLUID.name = “water” instead of “methanol” (see Appendix A).
- constant.d_twophase = 178e-3 instead of 114e-3.
- constant.d_liquid = constant.d_twophase*0.25 instead of constant.d_liquid = constant.d_twophase*0.4.
5. Results for Liquid EGW Mixture
- FLUID.cooling_type = 1 instead of 2 (2 = two-phase cooling, 1 = liquid cooling).
- FLUID.name = “water-eglycol” (40–60% mixture) instead of “methanol”.
- constant.d_twophase = 97e-3 instead of 114e-3.
- The size of the air HX in the direction of the airflow is increased by 10%: HX.L = 0.21*1.1 instead of 0.21.
- The width of the air HX is increased by 15%: HX.W = 0.75*1.154 instead of 0.75.
6. Summary of Analysis Results
7. Discussion
- The freezing point of methanol is lower (−97 °C, see Table 2).
- In a heat exchanger with two-phase methanol, there is a high heat transfer coefficient between the methanol vapor and liquid, and this prevents the liquid from freezing.
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Input for System Analysis
Appendix A.1. Simulation Parameters
| %% Simulation settings FLUID.cooling_type = 2; % 2 = two-phase, 1 = liquid FLUID.name = 'methanol'; % Fluid in system, if mixture (only for liquid cooling): always split by: - FLUID.mix = [0.4 0.6]; % fraction of mixture (only for liquid cooling): first fraction is for first fluid in FLUID.name system = 'Airbus'; % Geometry of system %%Initial values and conditions constant.P(1:4) = 500e3; % Heat input per Fuel Cell branch [W] constant.nrBranch = length(constant.P);% number of evaporator branches constant.nrCondens = 4; % number of condensers constant.nrCondensAir = 20; %non-physical division of condenser in several parallel tubes (for the cross-air heat exchanger). constant.NRiter = 150; % NR of iterations constant.CalcParallel = 0; % if 1, calculate distribution, if 0, assume equal distribution over parallel branches constant.dP_cor = 1; % Two-phase frictional pressure drop correlation. 1 = Muller-Steinhagen&Heck, 2 = Friedel constant.Xexit = 0.3; % vapour mass fraction after evaporator constant.DTpreheat = 15; %For liquid, this is the temperature increase due to the heat load, for two-phase, this determines the preheater power (if present in system) constant.Tkelvin = 273.15; % zero degrees Celsius [K] constant.Tsp = 95 + constant.Tkelvin; % set-point temperature [K] constant.Tsink = 30 + constant.Tkelvin; % heat sink (i.e. space or ambient air) temperature [K] constant.Tinit = constant.Tsink; % initial temperature of the loop [K] constant.pump_eff = 0.5; % adiabatic efficiency of pump [-] constant.fan_eff = 0.75; % adiabatic efficiency of pump [-] constant.Recuperator = 0; % 1 = with recuperator, 0 = without recuperator %% accu properties accu.Tmax = 110 + constant.Tkelvin; % maximum temperature used for strength and mass calculation accu.Hratio = 1.5; % ratio between the height of the cylindrical part and the diameter of the accumulator, e.g., Hratio = H/D accu.material = 'aluminium'; accu.rho = 2700; % density [kg/m^3] accu.factor_proof = 3; % factor for proof pressure accu.factor_burst = 4; % factor for burst pressure accu.Smargin = 1.25; %additional margin factor for proof and burst calculation accu.Syield = 83e6; %yield strength [N/m2] accu.Sultimate = 110e6; %ultimate strength [N/m2] %% ambient air input air.InputType = 1; % if InputType = 1, air mass flow is input. if InputType = 2, air mass flow is calculated from air speed air.m = 44.7/4; % air massflow [kg/s] (only for air.InputType = 1) air.U = 230/3.6; % flight speed in [m/s] (only for air.InputType = 2) air.pin = 1e5; % ambient air pressure [Pa] air.Tin = constant.Tsink; % Ambient air temperature; HX inlet air temperature air.Tout = air.Tin + 30; % Initial guess for HX outlet air temperature %% Finned air HX properties HX.L = 0.21; % Length of HX [m]: from air inlet to air outlet HX.Wlayer = 0.75*1.03; % Width of HX layer of fins [m] HX.NRlayer = 44; % number of layers of fins HX.W = HX.Wlayer*HX.NRlayer;%: width of HX times number of layers of fins HX.Hfin = 15e-3; % Height of one fin [m] HX.tfin = 0.15e-3; % Thickness of one fin [m] HX.dfin = 0.82e-3; % Distance between two fins [m] HX.k = 167; % Thermal conductivity of material btwn condenser-tube and airHX [W/mK] HX.rho = 2700; % Density of material btwn condenser-tube and airHX [kg/m3] HX.K_inlet = 0.0; % Minor pressure loss at air inlet of HX HX.K_outlet = 0.0; % Minor pressure loss at air inlet of HX |
Appendix A.2. Geometry Parameters
| %########################################################################% %# #% %# Geometry of Airbus two-phase cooling MPL #% %# #% %########################################################################% %# #% %# Components are structured like this: #% %# <ID> = Identifier number #% %# <Name> = Component name #% %# 'L' = Length of component [m] #% %# 'D' = Diameter of component [m] #% %# 'D_restriction' = Diameter of restriction [m], 0 for no restriction #% %# 'nParTube' = Number of parallel tubes [-] #% %# 'Shape' = Geometrical shape of tubes. 0 for round, 1 for square #% %# 'Diab' = 0 for none, 1 for evaporator, 2 for HX, #% %# 3 for condenser, 4 for single-phase #% %# 'mass' = Mass of component [kg] #% %# 'Cp' = Specific heat of material [J kg-1 K-1] #% %# 'K' = minor loss coefficient #% %# 'nElm' = Number of elements per component #% %# 'e' = Pipe inner roughness #% %########################################################################% constant.d_twophase = 114e-3; % [m] inner diameter of two-phase tubing if FLUID.cooling_type = =1 constant.d_liquid = constant.d_twophase; % [m] inner diameter of liquid tubing else constant.d_liquid = constant.d_twophase*0.4; % [m] inner diameter of liquid tubing end d_condenser = 1.4e-3; % [m] inner diameter of condenser tubes d_factor = constant.d_twophase/114e-3; % scaling factor for diameter of condenser, etc. restriction_factor = 1; %% Minor pressure loss constants % From: Y. A. Çengel, Fundamentals of thermal-fluid Sciences (McGraw-Hill, New York, 2012). K_elbow = 1.1; K_union = 0.08; K_tee_b = 2; % Branch flow K_tee_l = 0.9; % Line flow K_long_bend = 0.3; K_inlet = 0.5; % inlet loss K_outlet = 1; % outlet loss nC = 0; % Start counting the number of components %% Tube from pump to Evap nC = nC + 1; name = 'Tube_PtoE'; ID.(name) = nC; C(nC) = struct('ID',name, 'L',5, 'D',constant.d_liquid,'D_restriction',0.0e-3, 'nParTube',1, 'K',K_outlet + K_long_bend*2,'e',2e-6, 'Shape',0, 'Diab',0, 'mass',1, 'Cp',1); for i = 1:constant.nrBranch %% START evaporator branches d_restriction = constant.d_liquid*restriction_factor; % add restriction to inlet of each branch %d_restriction = 0; %% tube to Evaporator that contains the restriction nC = nC + 1; name = 'Tube_toE'; ID.(name)(i) = nC; C(nC) = struct('ID',[name '' int2str(i)], 'L',0.1, 'D',constant.d_liquid,'D_restriction',d_restriction, 'nParTube',1, 'K',K_outlet,'e',2e-6, 'Shape',1, 'Diab',0, 'mass',1, 'Cp',1); %% Evaporator nC = nC + 1; name = 'Evaporator'; ID.(name)(i) = nC; % cooling channels in FC biploar plates C(nC) = struct('ID',[name '' int2str(i)], 'L',0.370, 'D',0.62e-3,'D_restriction',0, 'nParTube',88*500*5, 'K',0,'e',2e-6, 'Shape',1, 'Diab',1, 'mass',40, 'Cp',900); %% tube from Evaporator nC = nC + 1; name = 'Tube_fromE'; ID.(name)(i) = nC; C(nC) = struct('ID',[name '' int2str(i)], 'L',0.1, 'D',constant.d_twophase,'D_restriction',0, 'nParTube',1, 'K',K_outlet,'e',2e-6, 'Shape',1, 'Diab',0, 'mass',1, 'Cp',1); end ID.twophase_start = ID.Evaporator(1); %% Tube from Evaporator to Condenser nC = nC + 1; name = 'Tube_EtoC'; ID.(name) = nC; C(nC) = struct('ID',name, 'L',10, 'D',constant.d_twophase,'D_restriction',0, 'nParTube',1, 'K',(K_inlet + K_outlet + K_long_bend*2)*1,'e',2e-6, 'Shape',0, 'Diab',0, 'mass',1, 'Cp',1); for i = 1:constant.nrCondens %% START branches %% tube to condenser nC = nC + 1; name = 'Tube_toC'; ID.(name)(i) = nC; C(nC) = struct('ID',[name '' int2str(i)], 'L',0.1, 'D',constant.d_twophase,'D_restriction',0, 'nParTube',1, 'K',K_outlet,'e',2e-6, 'Shape',1, 'Diab',0, 'mass',1, 'Cp',1); for j = 1:constant.nrCondensAir %% Condenser nC = nC + 1; name = 'Condenser'; ID.(name)(i,j) = nC; C(nC) = struct('ID',[name '_' int2str(i) '_' int2str(j)], 'L',HX.Wlayer, 'D',d_condenser*d_factor,'D_restriction',0, 'nParTube',HX.L*HX.NRlayer/(d_condenser*d_factor)/1.1/constant.nrCondensAir, 'K',0,'e',2e-6, 'Shape',0, 'Diab',3, 'mass',80, 'Cp',900); end %% tube to condenser nC = nC + 1; name = 'Tube_fromC'; ID.(name)(i) = nC; C(nC) = struct('ID',[name '' int2str(i)], 'L',0.1, 'D',constant.d_liquid,'D_restriction',0, 'nParTube',1, 'K',K_outlet,'e',2e-6, 'Shape',1, 'Diab',0, 'mass',1, 'Cp',1); end ID.twophase_end = ID.Condenser(end,end); %% Tube from condenser to accu nC = nC + 1; name = 'Tube_CtoA'; ID.(name) = nC; C(nC) = struct('ID',name, 'L',5, 'D',constant.d_liquid,'D_restriction',0, 'nParTube',1, 'K',(K_inlet + K_tee_l + K_long_bend*2)*0,'e',2e-6, 'Shape',0, 'Diab',0, 'mass',1, 'Cp',1); ID.preAccu = nC; % index for last component before accu %% Tube from accu to pump nC = nC + 1; name = 'Tube_AtoP'; ID.(name) = nC; C(nC) = struct('ID',name, 'L',1, 'D',constant.d_liquid,'D_restriction',0, 'nParTube',1, 'K',(K_inlet + K_tee_l + K_long_bend*2)*1,'e',2e-6, 'Shape',0, 'Diab',0, 'mass',1, 'Cp',1); |
Appendix B. Mass Estimation
Appendix B.1. Accumulator Vessel
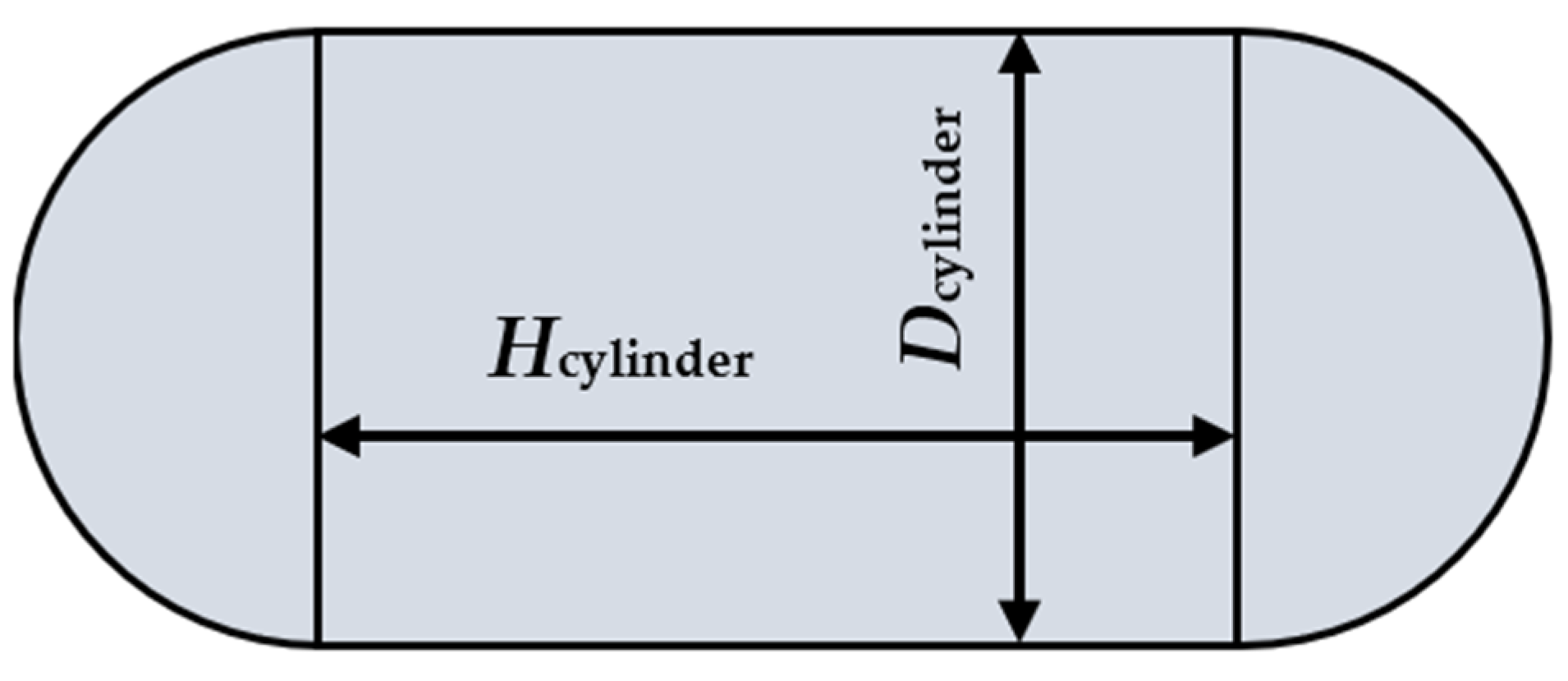
Appendix B.2. Tubing
Appendix B.3. Pump
Appendix B.4. Fluid
Appendix B.5. Ram Air Heat Exchanger
References
- Li, X.; Ye, T.; Meng, X.; He, D.; Li, L.; Song, K.; Jiang, J.; Sun, C. Advances in the Application of Sulfonated Poly(Ether Ether Ketone) (SPEEK) and Its Organic Composite Membranes for Proton Exchange Membrane Fuel Cells (PEMFCs). Polymers 2024, 16, 2840. [Google Scholar] [CrossRef] [PubMed]
- Shang, Z.; Hossain, M.; Wycisk, R.; Pintauro, P.N. Poly(phenylene sulfonic acid)-expanded polytetrafluoroethylene composite membrane for low relative humidity operation in hydrogen fuel cells. J. Power Sources 2022, 535, 231375. [Google Scholar] [CrossRef]
- Wang, Y.; Chen, K.S.; Mishler, J.; Cho, S.C.; Cordobes Adroher, X. A review of polymer electrolyte membrane fuel cells: Technology, applications, and needs on fundamental research. Appl. Energy 2011, 88, 4. [Google Scholar] [CrossRef]
- Revolutionising Aviation: Propelling Towards Decarbonised Skies with H2-Fuelled FC. Available online: https://brava-project.eu/ (accessed on 7 January 2025).
- van Es, J.; Pauw, A.; van Donk, G.; van Gerner, H.J.; Laudi, E.; He, Z.; Gargiulo, C.; Verlaat, B. AMS02 Tracker Thermal Control Cooling System commissioning and operational results. In Proceedings of the 43rd International Conference on Environmental Systems, Vail, CO, USA, 14–18 July 2013. [Google Scholar]
- Gorbenko, G.O.; Gakal, P.H.; Turna, R.Y.; Hodunov, A. Retrospective Review of a Two-Phase Mechanically Pumped Loop for Spacecraft Thermal Control Systems. J. Mech. Eng. 2021, 24, 27–37. [Google Scholar] [CrossRef]
- van Gerner, H.J.; Kunst, R.; van den Berg, T.H.; van Es, J.; Tailliez, A.; Walker, A.; Ortega, C.; Centeno, M.; Roldan, N.; Castaneda, C.; et al. Development and Testing of a Two-Phase Mechanically Pumped Loop for Active Antennae. In Proceedings of the 52nd International Conference on Environmental Systems, Calgary, AB, Canada, 12–16 July 2023. [Google Scholar]
- van Gerner, H.J.; de Smit, M.; van Es, J.; Migneau, M. Lightweight Two-Phase Pumped Cooling System with Aluminium Components produced with Additive Manufacturing. In Proceedings of the 49th International Conference on Environmental Systems, Boston, MA, USA, 7–11 July 2019. [Google Scholar]
- van Gerner, H.J.; Cao, C.; Pedroso, D.A.; te Nijenhuis, A.K.; Castro, I.; Dsouza, H. Two-phase pumped cooling system for power electronics; analyses and experimental results. In Proceedings of the 30th International Workshop on Thermal Investigations of ICs and Systems (THERMINIC), Toulouse, France, 25–27 September 2024. [Google Scholar]
- Tang, X.; Zhang, Y.; Xu, S. Experimental study of PEM fuel cell temperature characteristic and corresponding automated optimal temperature calibration model. Energy 2023, 283, 128456. [Google Scholar] [CrossRef]
- EKPO Fuel Cell Stacks. Available online: https://www.now-gmbh.de/en/projectfinder/h2sky/ (accessed on 22 October 2024).
- Neto, D.M.; Oliveira, M.C.; Alves, J.L.; Menezes, L.F. Numerical study on the formability of metallic bipolar plates for Proton Exchange Membrane (PEM) Fuel Cells. Metals 2019, 9, 810. [Google Scholar] [CrossRef]
- van Gerner, H.J.; van Benthem, R.C.; van Es, J.; Schwaller, D.; Lapensée, S. Fluid selection for space thermal control systems. In Proceedings of the 44th International Conference on Environmental Systems, Tucson, AZ, USA, 13–14 July 2014. [Google Scholar]
- Lemmon, E.; Huber, M.; McLinden, M. NIST Standard Reference Database 23: Reference Fluid Thermodynamic and Transport Properties-REFPROP, version 9.1; National Institute of Standards and Technology: Gaithersburg, MD, USA; Standard Reference Data Program: Gaithersburg, MD, USA, 2013. [Google Scholar]
- NFPA 704 Standard System for the Identification of the Hazards of Materials for Emergency Response. Available online: https://www.nfpa.org/Codes-and-Standards/All-Codes-and-Standards/List-of-Codes-and-Standards. (accessed on 18 February 2021).
- van Gerner, H.J.; Braaksma, N. Transient modelling of pumped two-phase cooling systems: Comparison between experiment and simulation, ICES-2016-004. In Proceedings of the 46th International Conference on Environmental Systems, Vienna, Austria, 10–14 July 2016. [Google Scholar]
- van Gerner, H.J.; Bolder, R.; van Es, J. Transient modelling of pumped two-phase cooling systems: Comparison between experiment and simulation with R134a, ICES-2017-037. In Proceedings of the 47th International Conference on Environmental Systems, Charleston, South Carolina, 16–20 July 2017. [Google Scholar]
- Donders, S.N.L.; Banine, V.Y.; Moors, J.H.J.; Verhagen, M.C.M.; Frijns, O.V.W.; van Donk, G.; van Gerner, H.J. Thermal Conditioning System for Thermal Conditioning a Part of a Lithographic Apparatus and a Thermal Conditioning Method. Patent US8610089, 2013. Available online: https://patents.google.com/patent/US8610089B2/en (accessed on 7 January 2025).
- Van Gerner, H.J.; Luten, T.; Scholten, S.; Mühlthaler, G.; Buntz, M.B. Test results for a novel 20 kW two-phase pumped cooling for aerospace applications. Aerospace, 2024; submitted. [Google Scholar]
- PFAS Ban Affects Most Refrigerant Blends. Available online: https://www.coolingpost.com/world-news/pfas-ban-affects-most-refrigerant-blends/ (accessed on 20 April 2023).
- ECHA, European Chemical Agency. Submitted Restrictions Under Consideration. Available online: https://echa.europa.eu/restrictions-under-consideration/-/substance-rev/72301/term (accessed on 24 January 2024).
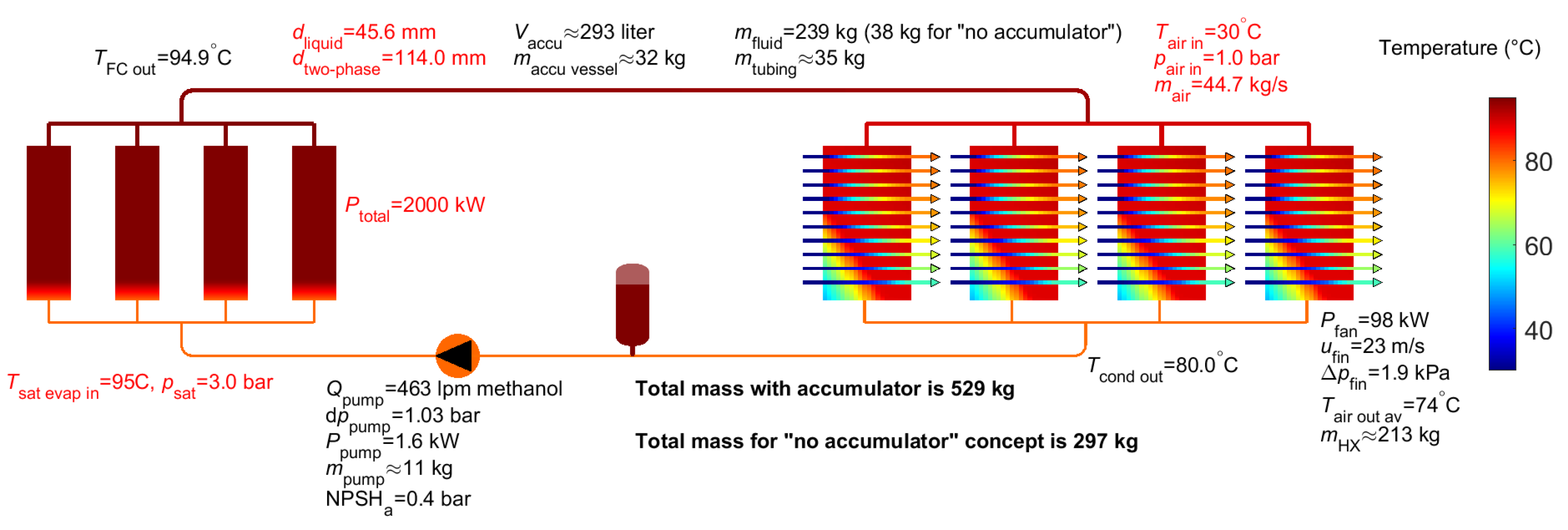

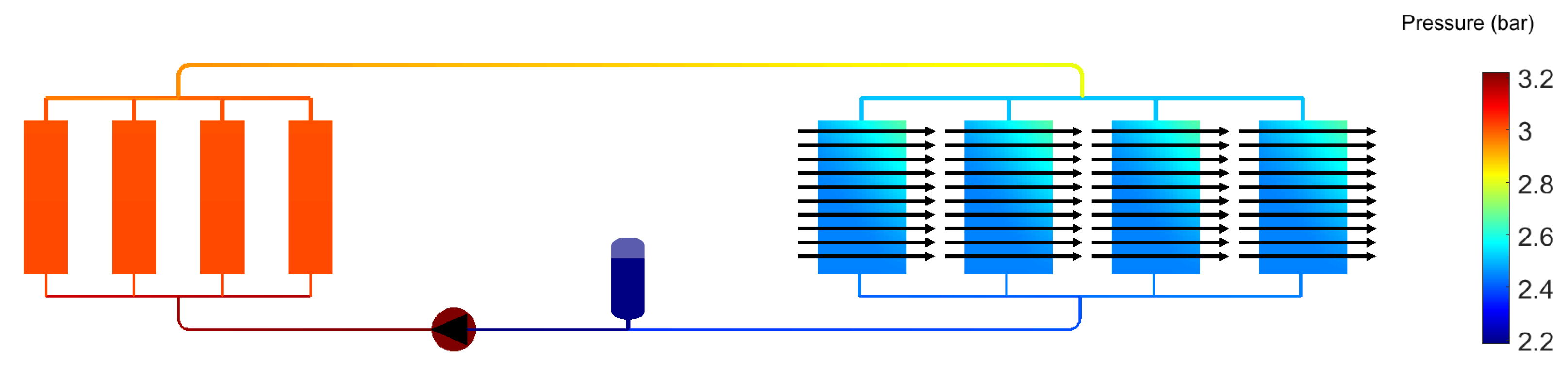
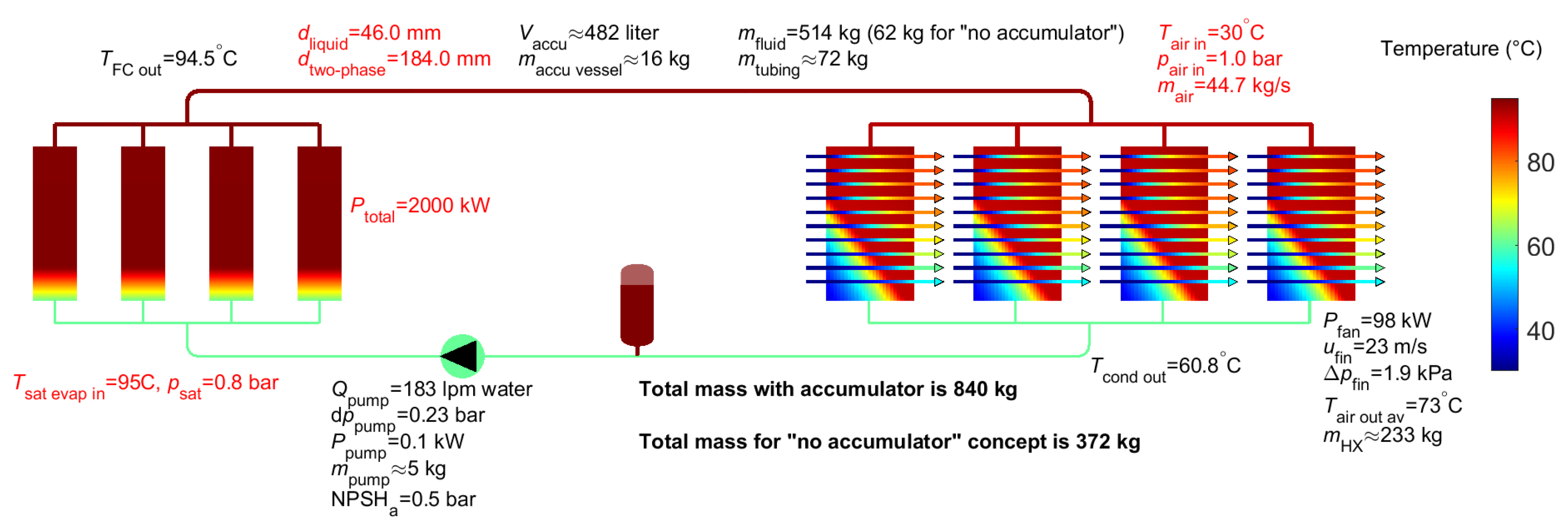
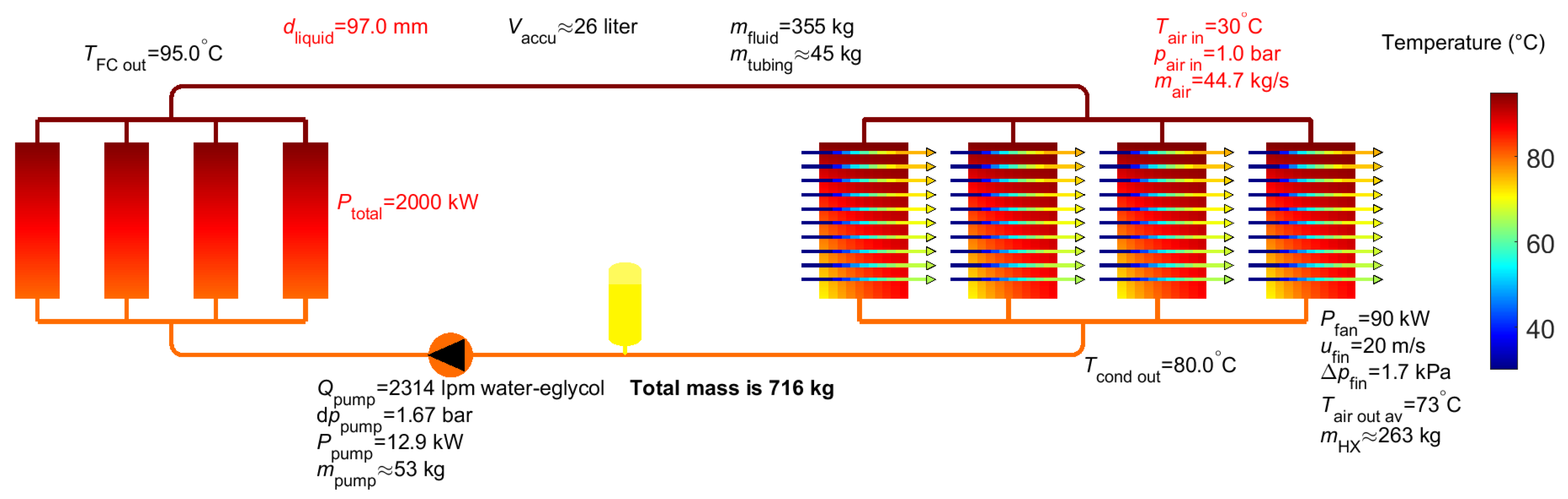
| Methanol | Water | Novec 649 | Fluorinert 72 (C6F14) | Liquid EGW | |
|---|---|---|---|---|---|
| Accumulator volume 2 | 293 L (0 L) | 482 L (0 L) | 476 L (0 L) | 542 L (0 L) | 26 L |
| Pump flow | 463 lpm | 183 lpm | 1741 lpm | 1635 lpm | 2314 lpm |
| Pump power | 1.6 kW | 0.1 kW | 6.6 kW | 4.2 kW | 12.9 kW |
| Triple point (°C) | −97 °C | 0 °C | −108 °C | −86 °C | ~−48 °C |
| Mass fluid 2 | 239 kg (38 kg) | 514 kg (61 kg) | 810 kg (260 kg) | 992 kg (311 kg) | 355 kg |
| Mass tubing | 35 kg | 72 kg | 75 kg | 91 kg | 45 kg |
| Mass pump | 11 kg | 5 kg | 40 kg | 38 kg | 53 kg |
| Mass accumulator 2 | 32 kg (0 kg) | 16 kg (0 kg) | 61 kg (0 kg) | 56 kg (0 kg) | neglected |
| Mass air heat exchanger | 213 kg | 233 kg | 226 kg | 232 kg | 263 kg |
| Total mass 2 | 529 kg (297 kg) | 840 kg (372 kg) | 1212 kg (601 kg) | 1409 kg (671 kg) | 716 kg |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
van Gerner, H.J.; Luten, T.; Resende, W.; Mühlthaler, G.; Buntz, M.-B. System Analysis and Comparison Between a 2 MW Conventional Liquid Cooling System and a Novel Two-Phase Cooling System for Fuel Cell-Powered Aircraft. Energies 2025, 18, 849. https://doi.org/10.3390/en18040849
van Gerner HJ, Luten T, Resende W, Mühlthaler G, Buntz M-B. System Analysis and Comparison Between a 2 MW Conventional Liquid Cooling System and a Novel Two-Phase Cooling System for Fuel Cell-Powered Aircraft. Energies. 2025; 18(4):849. https://doi.org/10.3390/en18040849
Chicago/Turabian Stylevan Gerner, Henk Jan, Tim Luten, William Resende, Georg Mühlthaler, and Marcus-Benedict Buntz. 2025. "System Analysis and Comparison Between a 2 MW Conventional Liquid Cooling System and a Novel Two-Phase Cooling System for Fuel Cell-Powered Aircraft" Energies 18, no. 4: 849. https://doi.org/10.3390/en18040849
APA Stylevan Gerner, H. J., Luten, T., Resende, W., Mühlthaler, G., & Buntz, M.-B. (2025). System Analysis and Comparison Between a 2 MW Conventional Liquid Cooling System and a Novel Two-Phase Cooling System for Fuel Cell-Powered Aircraft. Energies, 18(4), 849. https://doi.org/10.3390/en18040849






