Experimental Study on the Influence of Ion Components in Geothermal Water on Scaling Behavior
Abstract
:1. Introduction
2. Corrosion and Scaling Trend Assessment Using Index Models
3. Experimental
3.1. Materials
3.1.1. Simulated Geothermal Water Composition
3.1.2. Sample Preparation
3.2. Test Methods
4. Results and Discussion
4.1. Effect of Major Cations on Scale Deposition
4.1.1. Effect of Ca2+ on Scale Deposition
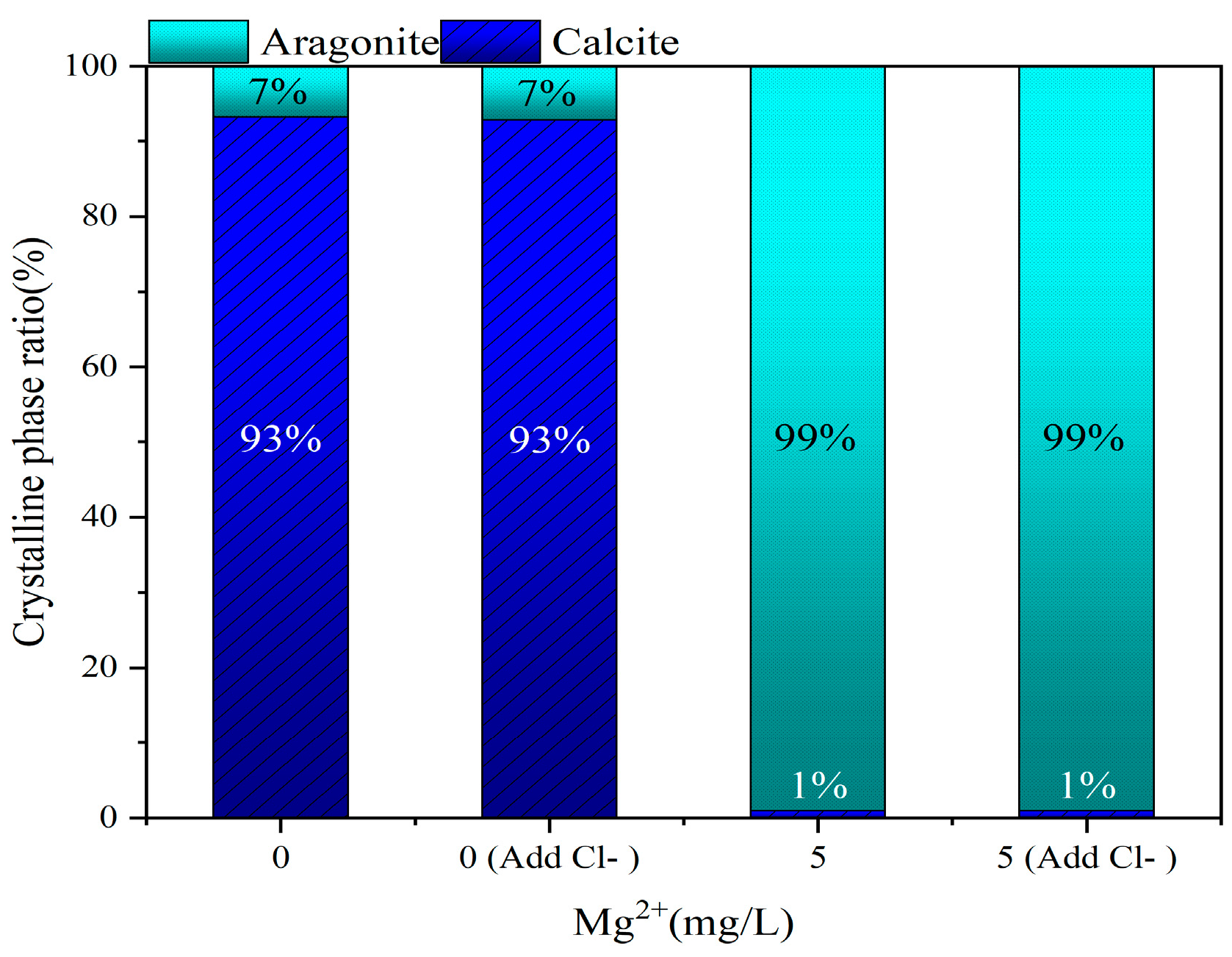
4.1.2. Effect of Mg2+ on Scale Deposition
4.2. Effect of Anions on Scale Deposition
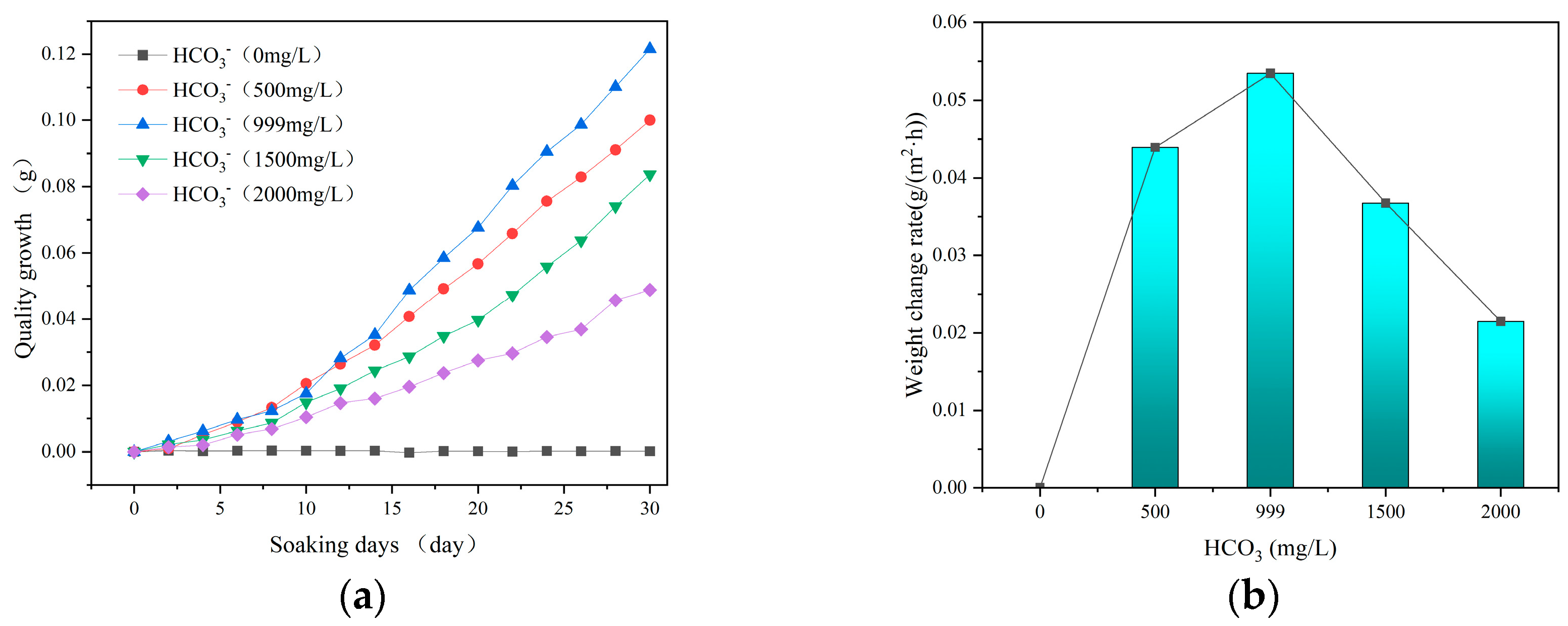
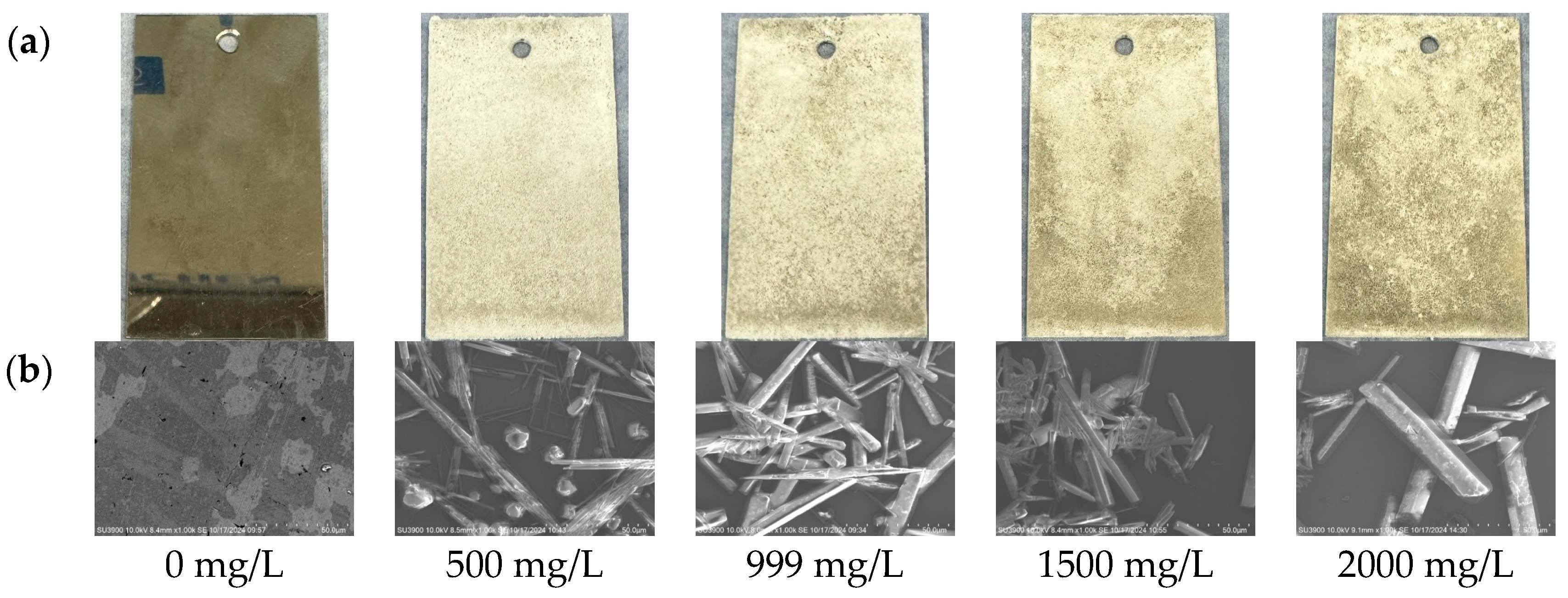
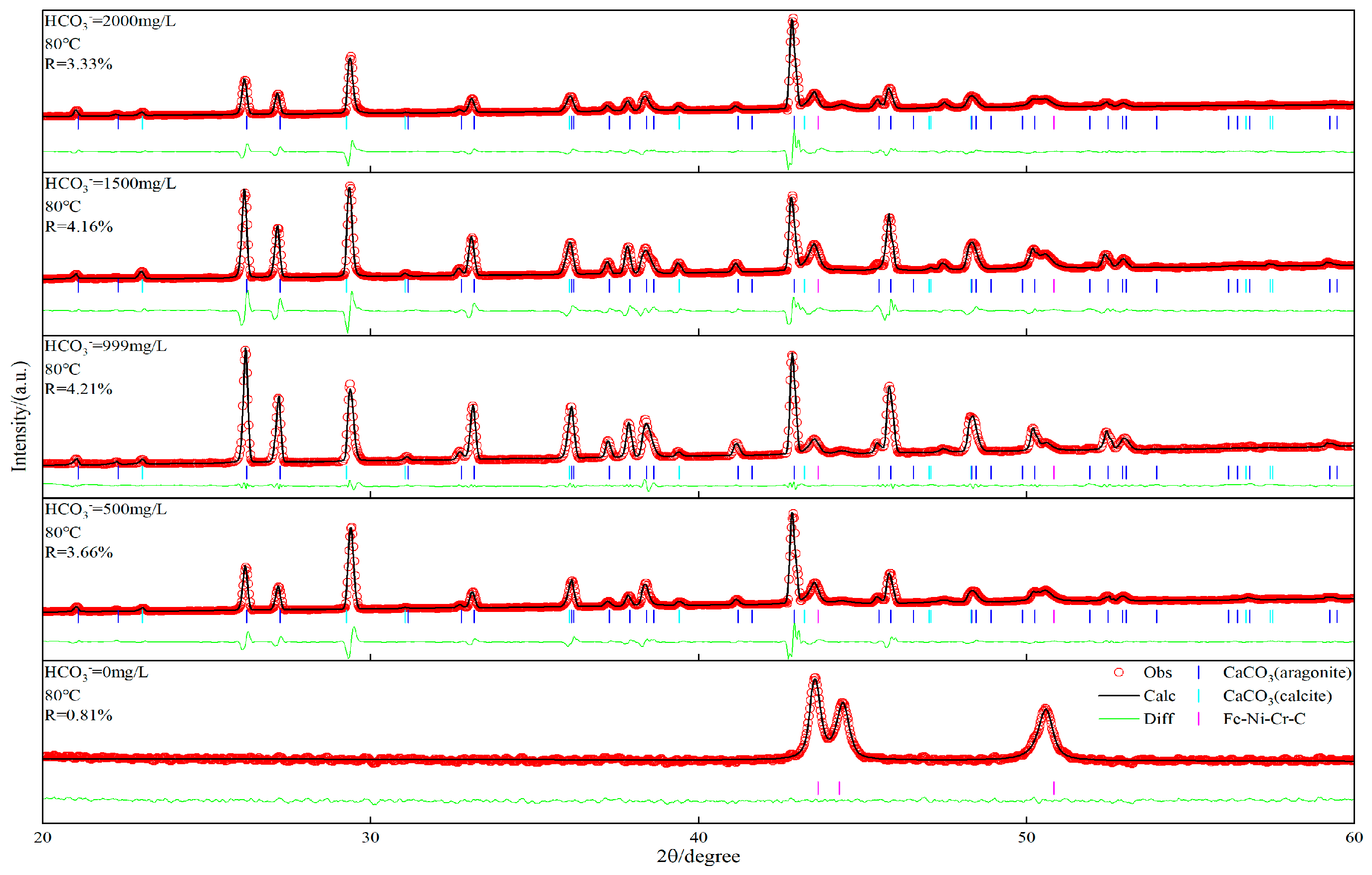
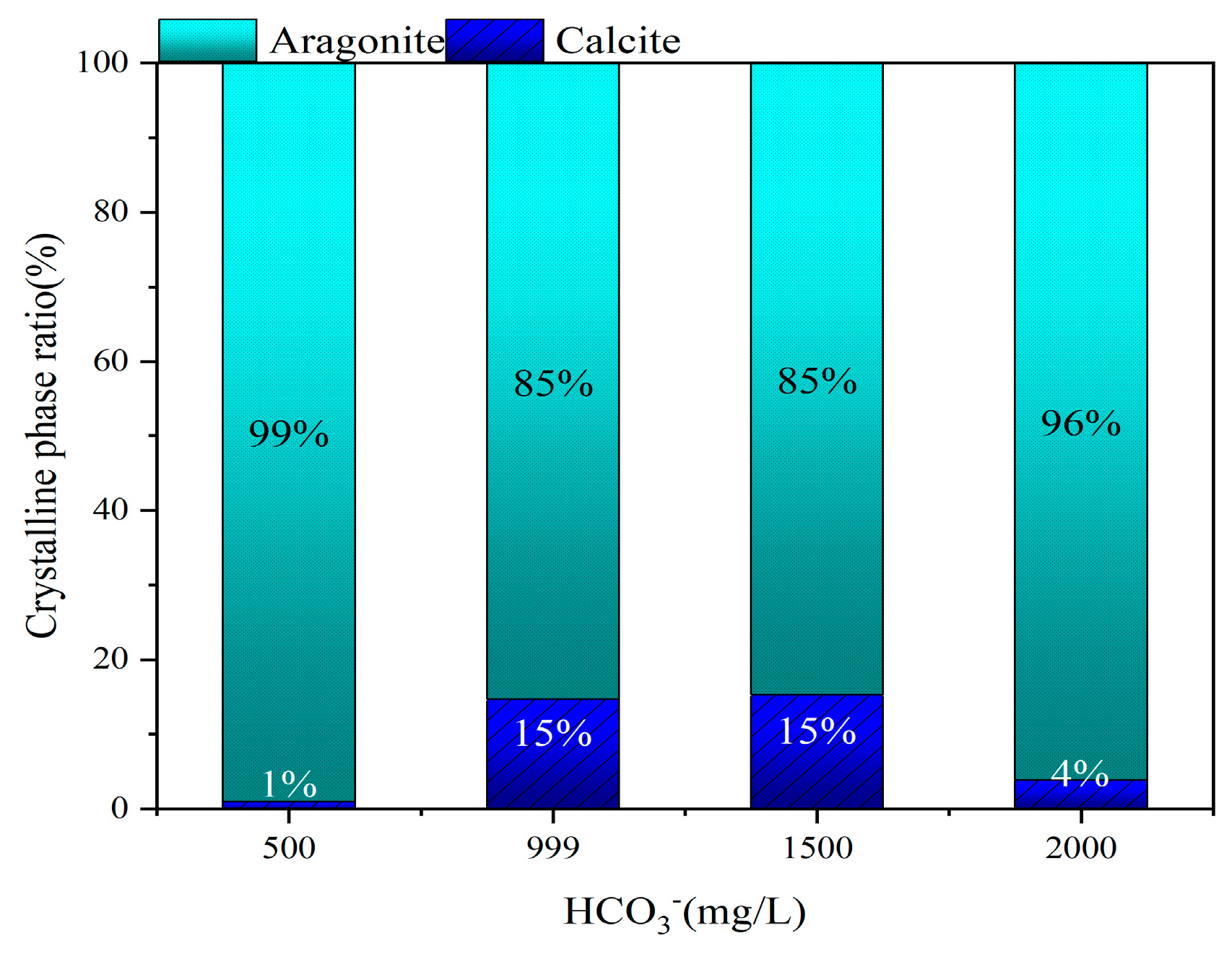
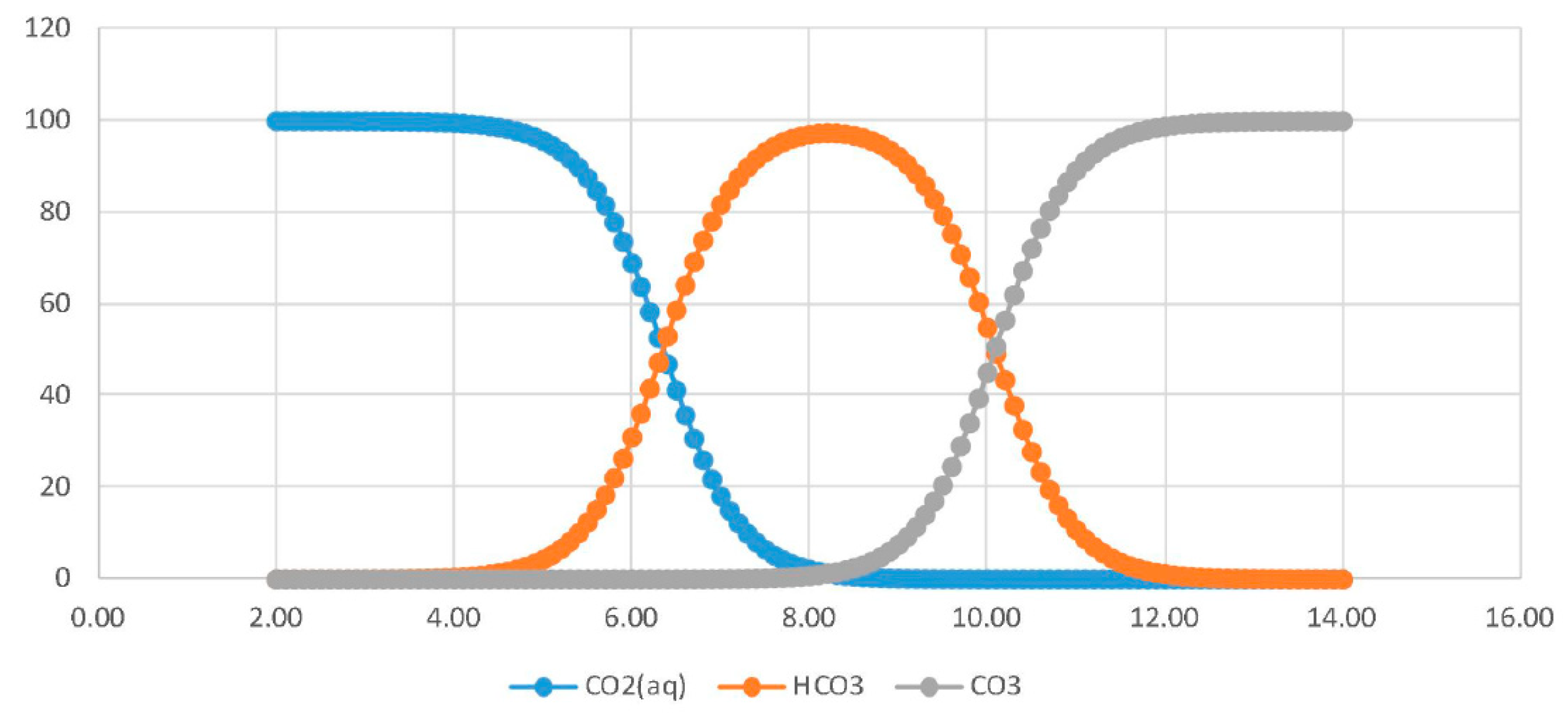
4.2.1. Effect of HCO3− on Scale Deposition
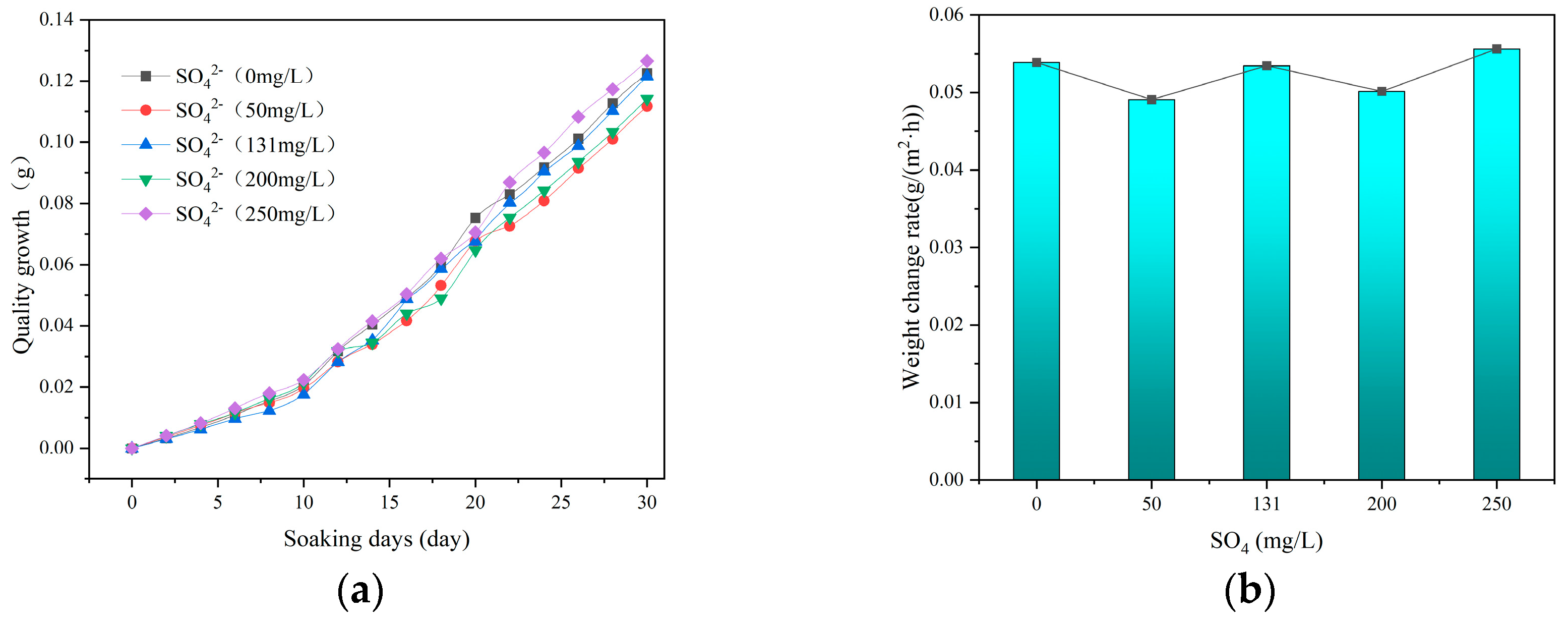
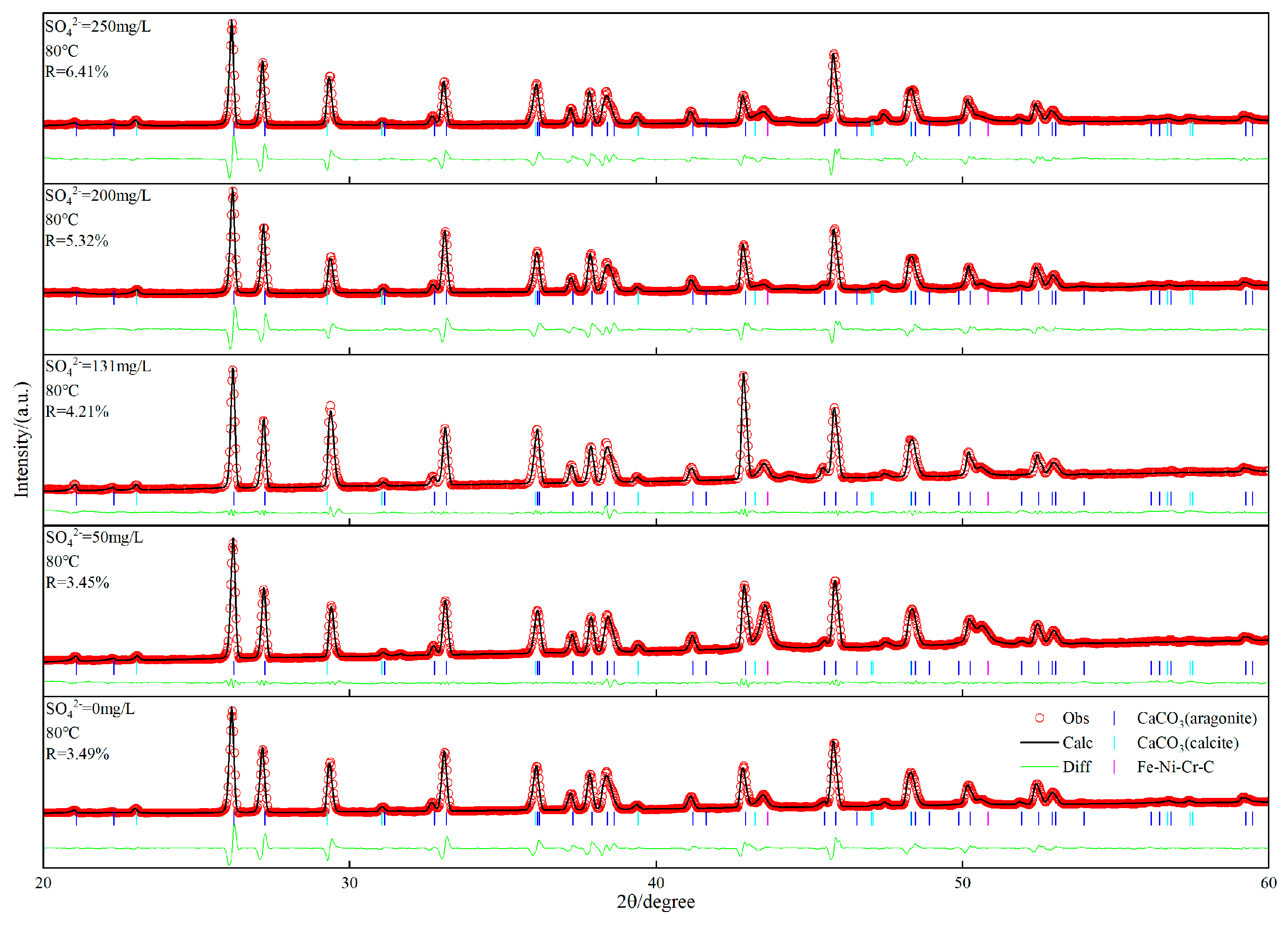
4.2.2. Effect of SO42− on Scale Deposition
4.2.3. Effect of SiO32− on Scale Deposition
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Penot, C.; Martelo, D.; Paul, S. Corrosion and scaling in geothermal heat exchangers. Appl. Sci. 2023, 13, 11549. [Google Scholar] [CrossRef]
- Wan, Z.; Zhao, Y.; Kang, J. Forecast and evaluation of hot dry rock geothermal resource in China. Renew. Energy 2005, 30, 1831–1846. [Google Scholar] [CrossRef]
- El Haj Assad, M.; Bani-Hani, E.; Khalil, M. Performance of geothermal power plants (single, dual, and binary) to compensate for LHC-CERN power consumption: Comparative study. Geotherm. Energy 2017, 5, 17. [Google Scholar] [CrossRef]
- van der Zwaan, B.; Dalla Longa, F. Integrated assessment projections for global geothermal energy use. Geothermics 2019, 82, 203–211. [Google Scholar] [CrossRef]
- Zhao, H.; Huang, Y.; Deng, S.; Wang, L.; Peng, H.; Shen, X.; Ling, D.; Liu, L.; Liu, Y. Research progress on scaling mechanism and anti-scaling technology of geothermal well system. J. Dispers. Sci. Technol. 2023, 44, 1657–1670. [Google Scholar] [CrossRef]
- Keserovic, A.; Bäßler, R. Suitability of UNS S31603 steel for geothermal brines in volcanic areas-Influence of different physicochemical conditions on its corrosion behavior. Geothermics 2015, 53, 479–487. [Google Scholar] [CrossRef]
- Shannon, D.W. Economic Impact of Corrosion and Scaling Problems in Geothermal Energy Systems; Battelle Pacific Northwest Labs: Richland, WA, USA, 1975. [Google Scholar]
- Tardiff, G.E. Using Salton Sea geothermal brines for electrical power: A review of progress in chemistry and materials technology 1976 status. In Proceedings of the 12th Intersociety Energy Conversion Engineering Conference, Washington, DC, USA, 28 August–2 September 1977. [Google Scholar]
- Yang, J.; Li, C.; Pan, Y.; Huang, H. The failure mechanism of the 316 SS heat exchanger tube in the geothermal water environment. Materials 2022, 15, 8103. [Google Scholar] [CrossRef] [PubMed]
- Ledésert, B.A.; Hébert, R.L.; Mouchot, J.; Bosia, C.; Ravier, G.; Seibel, O.; Dalmais, É.; Ledésert, M.; Trullenque, G.; Sengelen, X.; et al. Scaling in a geothermal heat exchanger at soultz-sous-forêts (Upper Rhine Graben, France): A XRD and SEM-EDS characterization of sulfide precipitates. Geosciences 2021, 11, 271. [Google Scholar] [CrossRef]
- Morake, J.B.; Mutua, J.M.; Ruthandi, M.M.; Olakanmi, E.O.; Botes, A. Failure analysis of corroded heat exchanger CuNi tubes from a geothermal plant. Eng. Fail. Anal. 2023, 153, 107543. [Google Scholar] [CrossRef]
- Epstein, N. Fouling in heat exchangers. In International Heat Transfer Conference Digital Library; Begel House Inc.: Danbury, CT, USA, 1978. [Google Scholar]
- Wu, K.-H.; Zhu, L.-Q.; Li, W.-P.; Liu, H.-C. Effect of Ca2+ and Mg2+ on corrosion and scaling of galvanized steel pipe in simulated geothermal water. Corros. Sci. 2010, 52, 2244–2249. [Google Scholar] [CrossRef]
- Kim, W.T.; Bai, C.; Cho, Y.I. A study of CaCO3 fouling with a microscopic imaging technique. Int. J. Heat Mass Transf. 2002, 45, 597–607. [Google Scholar] [CrossRef]
- Bansal, B. Crystallisation Fouling in Plate Heat Exchangers. Doctoral Dissertation, ResearchSpace@Auckland, Auckland, New Zealand, 1994. [Google Scholar]
- Pääkkönen, T.; Ojaniemi, U.; Pättikangas, T.; Manninen, M.; Muurinen, E.; Keiski, R.; Simonson, C. CFD modelling of CaCO3 crystallization fouling on heat transfer surfaces. Int. J. Heat Mass Transf. 2016, 97, 618–630. [Google Scholar] [CrossRef]
- Lu, A.Y.-T.; Harouaka, K.; Paudyal, S.; Ko, S.; Dai, C.; Gao, S.; Deng, G.; Zhao, Y.; Wang, X.; Mateen, S.; et al. Kinetics of barium sulfate deposition and crystallization process in the flowing tube. Ind. Eng. Chem. Res. 2020, 59, 7299–7309. [Google Scholar] [CrossRef]
- Waly, T.; Kennedy, M.D.; Witkamp, G.-J.; Amy, G.; Schippers, J.C. The role of inorganic ions in the calcium carbonate scaling of seawater reverse osmosis systems. Desalination 2012, 284, 279–287. [Google Scholar] [CrossRef]
- SMuryanto, A.P.; Bayuseno, W.; Sediono, W. Mangestiyono, Influence of flow rates and copper (II) ions on the kinetics of gypsum scale formation in pipes. Int. J. Technol. 2014, 4, 217–223. [Google Scholar] [CrossRef]
- Wang, G.; Zhu, L.; Liu, H.; Li, W. Galvanic corrosion of Ni-Cu-Al composite coating and its anti-fouling property for metal pipeline in simulated geothermal water, Surf. Coat. Technol. 2012, 206, 3728–3732. [Google Scholar] [CrossRef]
- Cai, Y.; Quan, X.; Li, G.; Gao, N. Anticorrosion and scale behaviors of nanostructured ZrO2–TiO2 coatings in simulated geothermal water. Ind. Eng. Chem. Res. 2016, 55, 11480–11494. [Google Scholar] [CrossRef]
- Tai, C.; Chien, W. Interpreting the effects of operating variables on the induction period of CaCl2-Na2CO3 system by a cluster coagulation model. Chem. Eng. Sci. 2003, 58, 3233–3241. [Google Scholar] [CrossRef]
- Lei, B.; Li, M.; Zhao, Z.; Wang, L.; Li, Y.; Wang, F. Corrosion mechanism of an Al–BN abradable seal coating system in chloride solution. Corros. Sci. 2014, 79, 198–205. [Google Scholar] [CrossRef]
- Zeppenfeld, K. Prevention of CaCO3 scale formation by trace amounts of copper(II) in comparison to zinc (II). Desalination 2010, 252, 60–65. [Google Scholar] [CrossRef]
- Benslimane, S.; Bouhidel, K.E.; Ferfache, A.; Farhi, S. Mechanistic study of the synergetic inhibiting effects of Zn2+, Cu2+ and Mg2+ ions on calcium carbonate precipitation. Water Res. 2020, 186, 116323. [Google Scholar] [CrossRef]
- Zhang, F.; Liu, M.; Zhang, S.; Zhao, Q. Corrosion and fouling of different coatings in geothermal water. Acta Energiae Solaris Sin. 2015, 36, 510–516. [Google Scholar]
- Gallup, D.L.; Sugiaman, F.; Capuno, V.; Manceau, A. Laboratory investigation of silica removal from geothermal brines to control silica scaling and produce usable silicates. Appl. Geochem. 2003, 18, 1597–1612. [Google Scholar] [CrossRef]
- Song, J.; Liu, M.; Sun, X. Model analysis and experimental study on scaling and corrosion tendencies of aerated geothermal water. Geothermics 2020, 85, 101766. [Google Scholar] [CrossRef]
- Langelier, W.F. Chemical equilibria in water treatment. J. Am. Water Work. Assoc. 1946, 36, 169–178. [Google Scholar] [CrossRef]
- Ryznar, J.W. A new index for determining amount of calcium carbonate scale formed by a water. J. Am. Water Work. Assoc. 1944, 36, 472–483. [Google Scholar] [CrossRef]
- Larson, T.E.; Skold, R.V. Laboratory studies relating mineral quality of water to corrosion of steel and cast iron. Corrosion 1958, 14, 43–46. [Google Scholar] [CrossRef]
- Gryta, M. Alkaline scaling in the membrane distillation process. Desalination 2008, 228, 128–134. [Google Scholar] [CrossRef]
- Chen, T.; Neville, A.; Yuan, M. Assessing the effect of Mg2+ on CaCO3 scale formation–bulk precipitation and surface deposition. J. Cryst. Growth 2005, 275, e1341–e1347. [Google Scholar] [CrossRef]
- Wright, K.C.; Kim, H.S.; Cho, D.J.; Rabinovich, A.; Fridman, A.; Cho, Y.I. New fouling prevention method using a plasma gliding arc for produced water treatment. Desalination 2014, 345, 64–71. [Google Scholar] [CrossRef]
- Minkowicz, L.; Dagan, A.; Uvarov, V.; Benny, O. Controlling calcium carbonate particle morphology, size, and molecular order using silicate. Materials 2021, 14, 3525. [Google Scholar] [CrossRef] [PubMed]
- Fan, R.; Short, M.D.; Zeng, S.-J.; Qian, G.; Li, J.; Schumann, R.C.; Kawashima, N.; Smart, R.S.C.; Gerson, A.R. The formation of silicate-stabilized passivating layers on pyrite for reduced acid rock drainage. Environ. Sci. Technol. 2017, 51, 11317–11325. [Google Scholar] [CrossRef] [PubMed]
- Nie, Y.; Ma, S.; Tian, M.; Zhang, Q.; Huang, J.; Cao, M.; Li, Y.; Sun, L.; Pan, J.; Wang, Y.; et al. Superhydrophobic silane-based surface coatings on metal surface with nanoparticles hybridization to enhance anticorrosion efficiency, wearing resistance and antimicrobial ability. Surf. Coat. Technol. 2021, 410, 126966. [Google Scholar] [CrossRef]

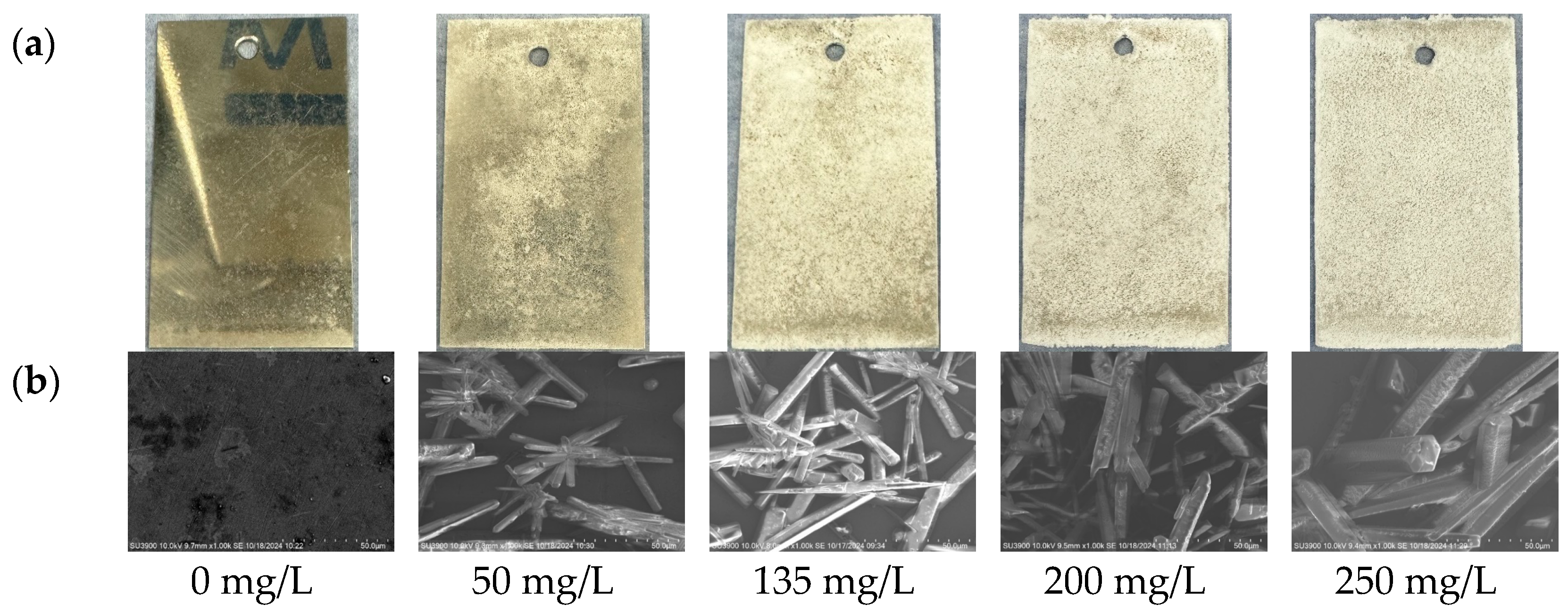
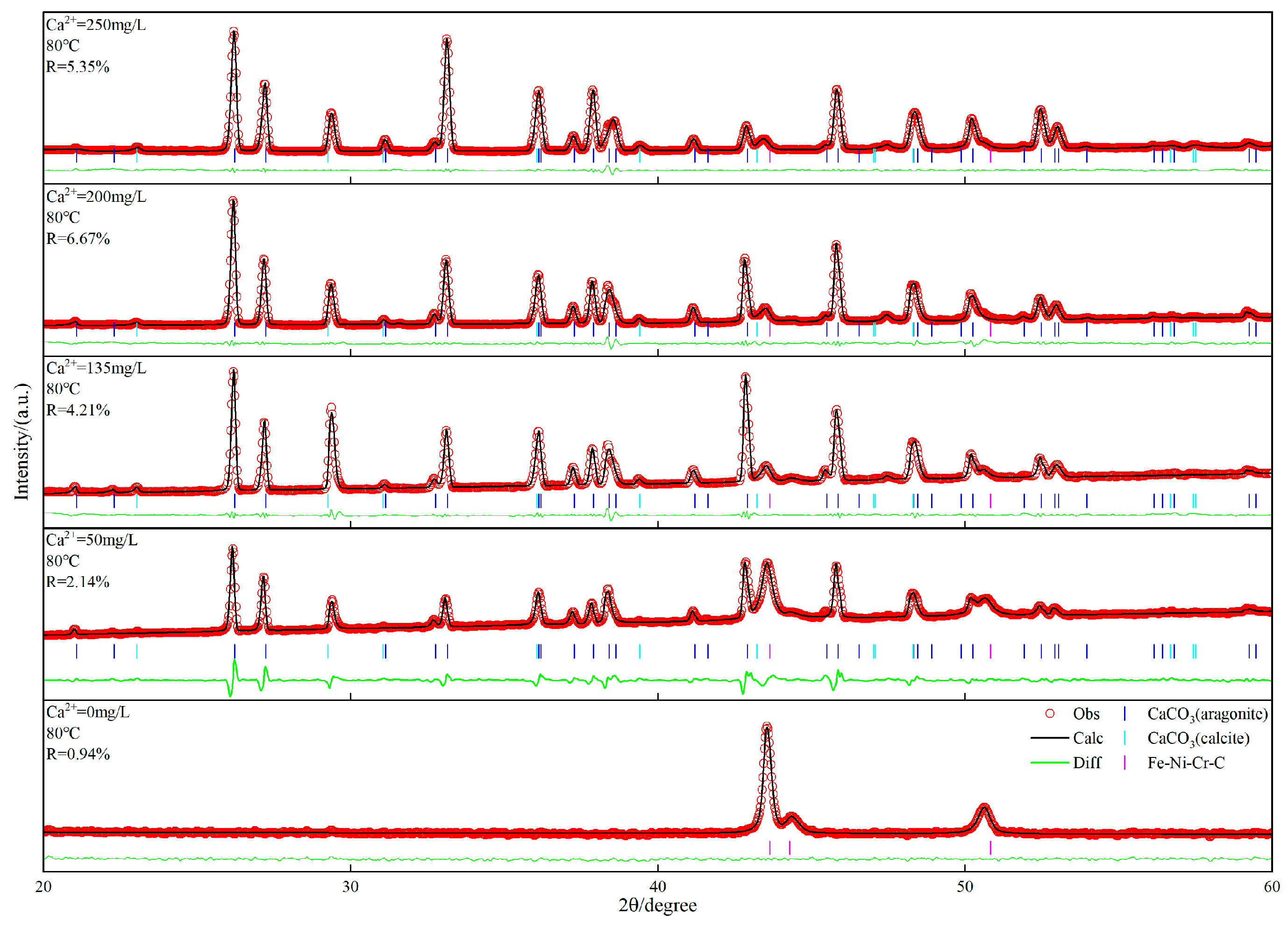
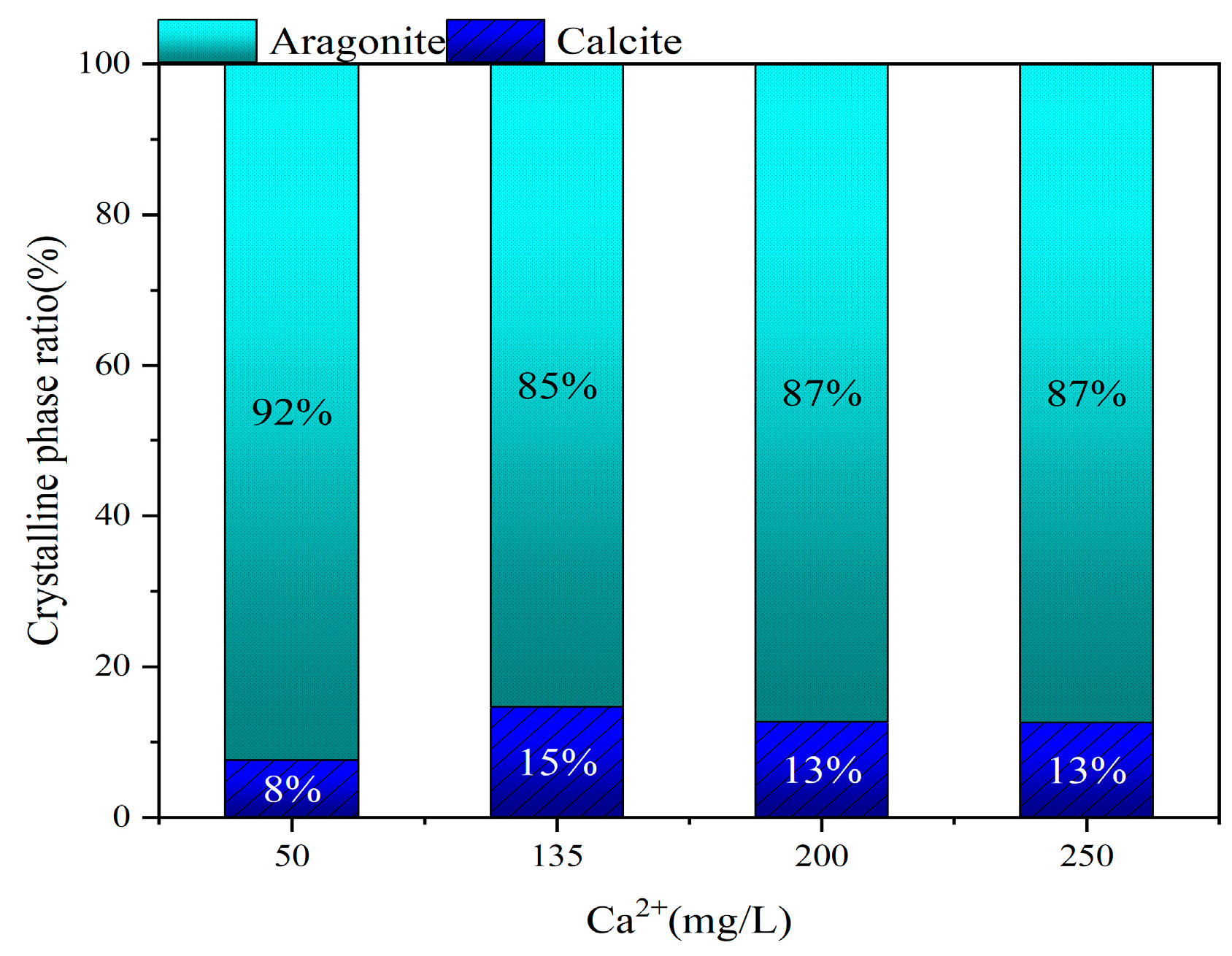

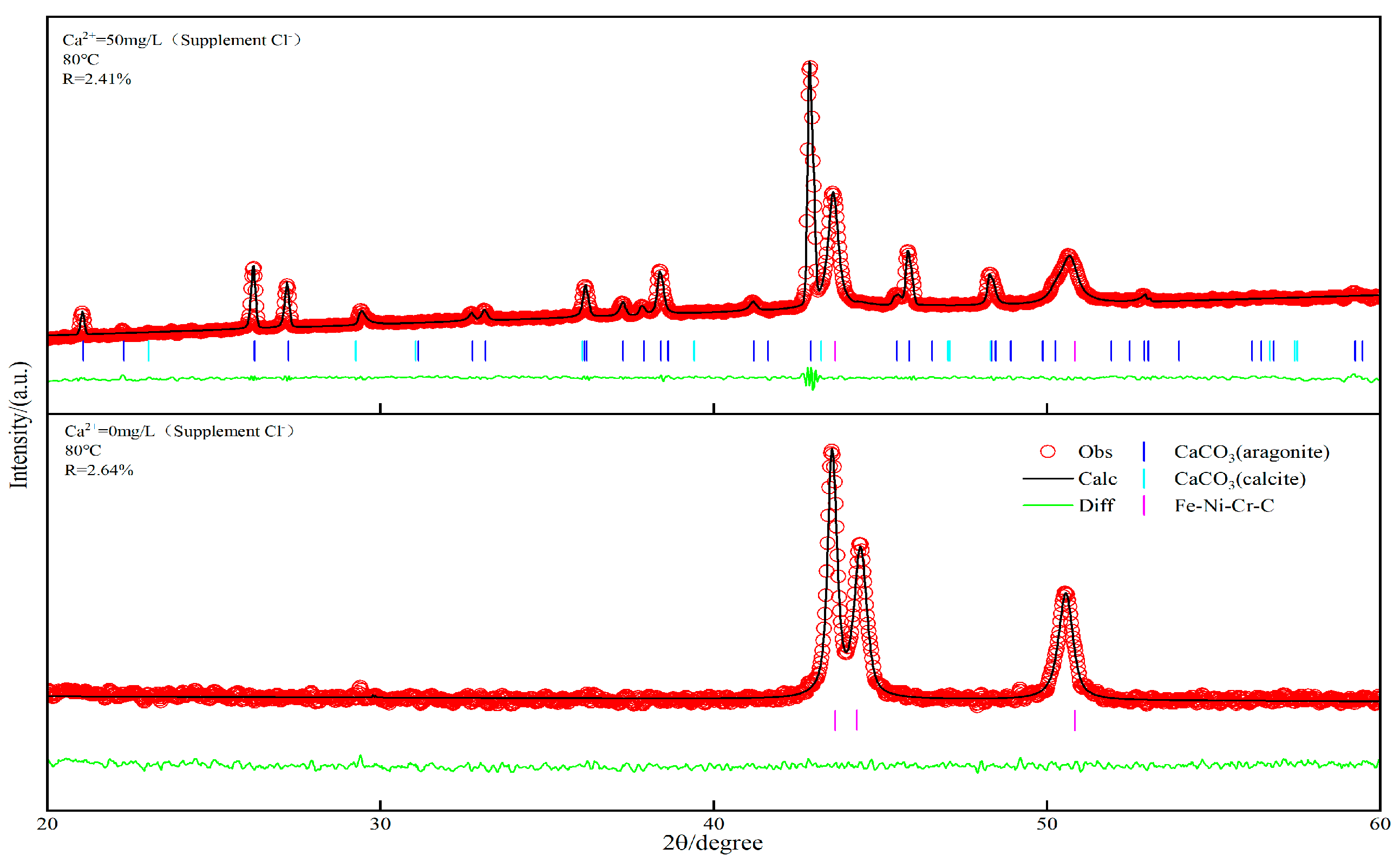
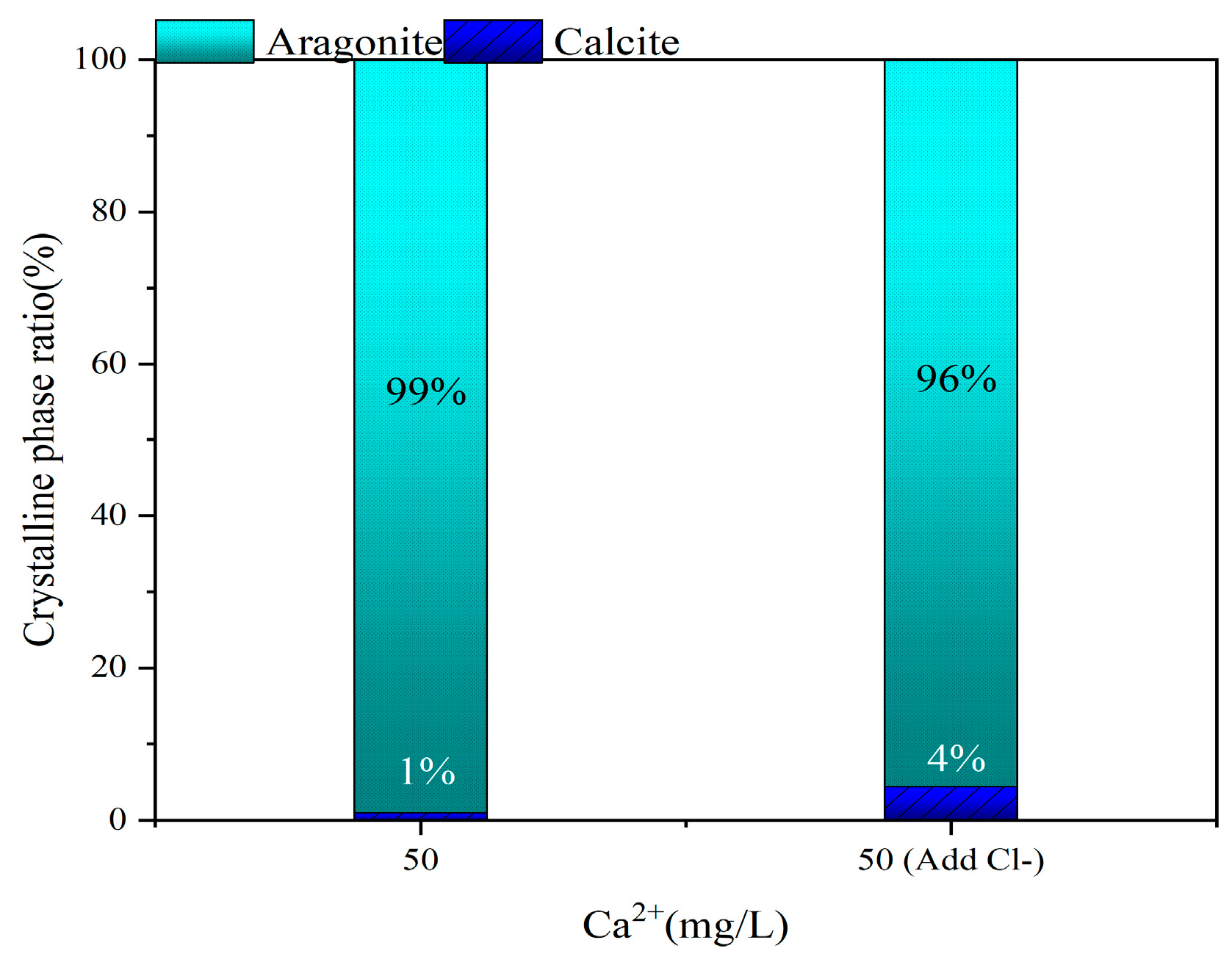

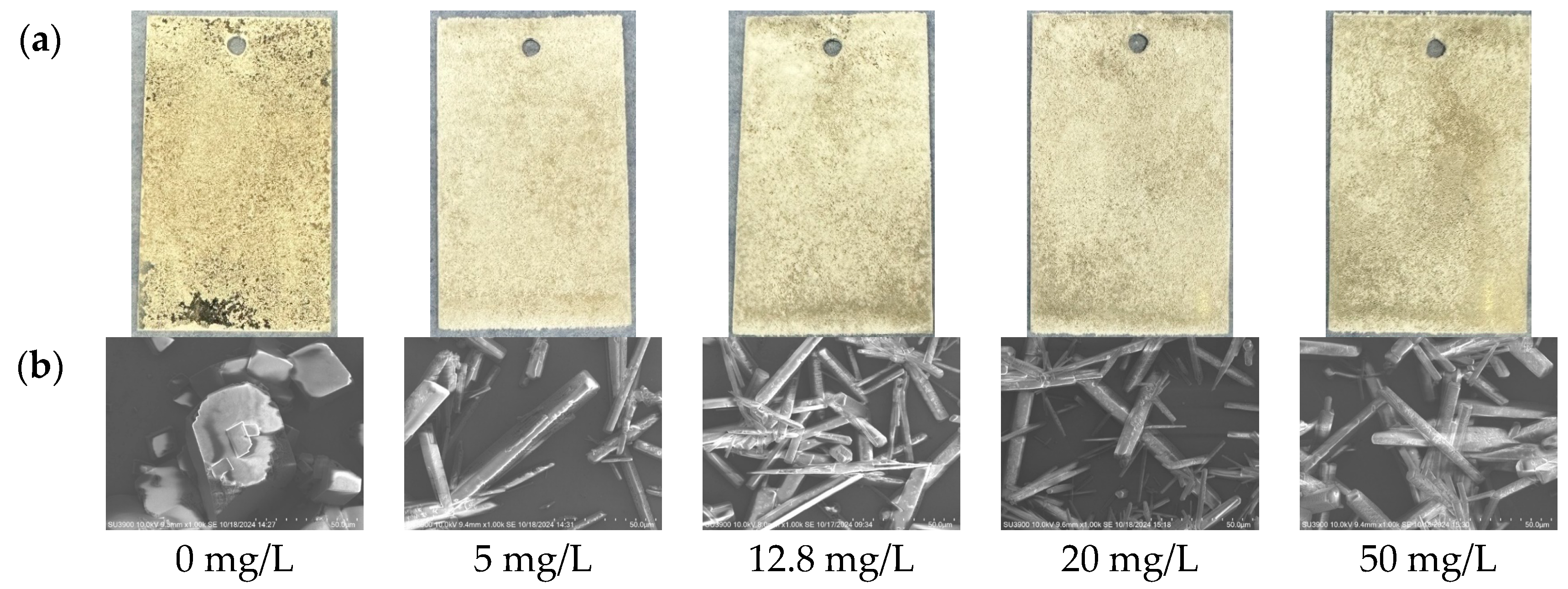
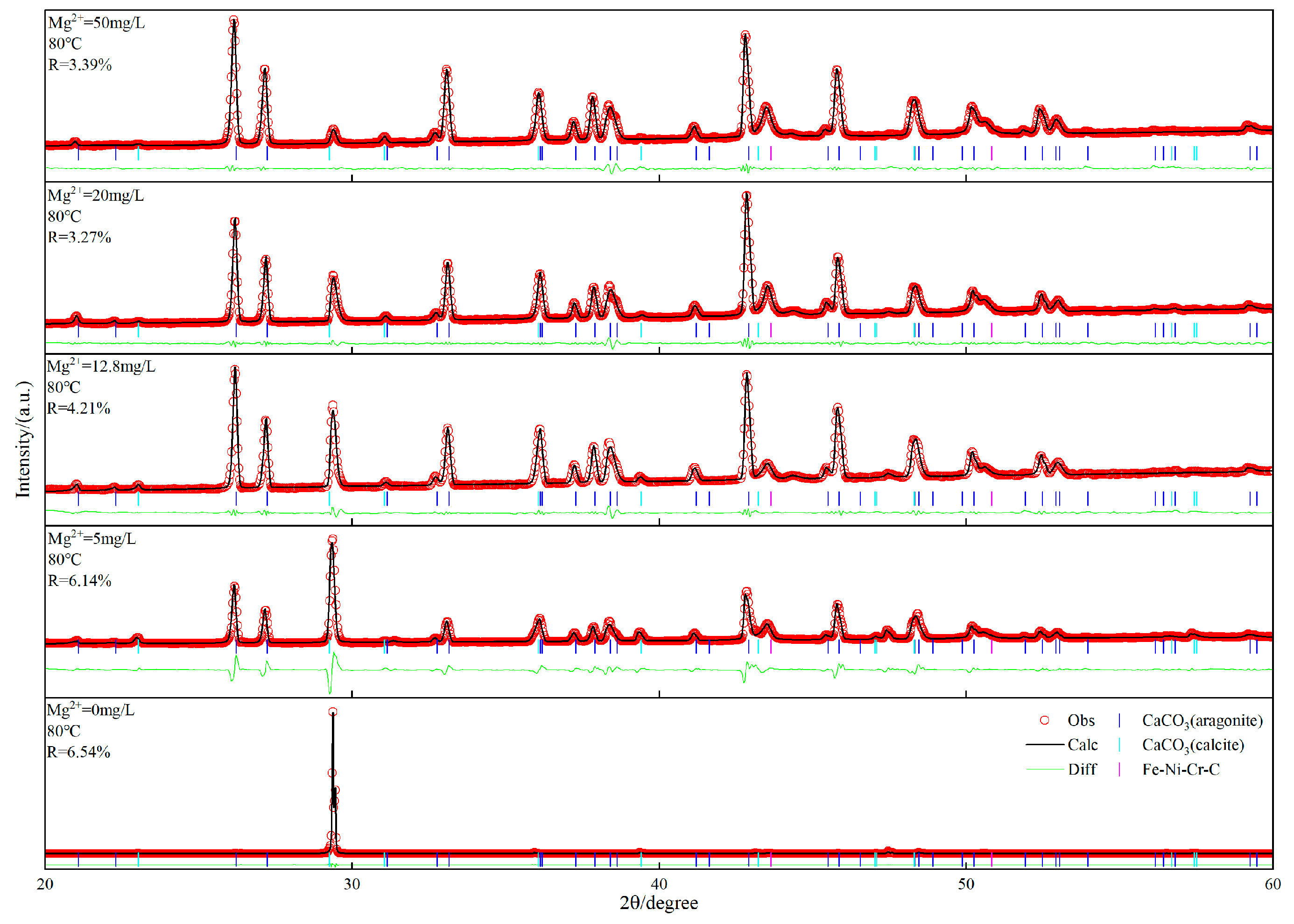


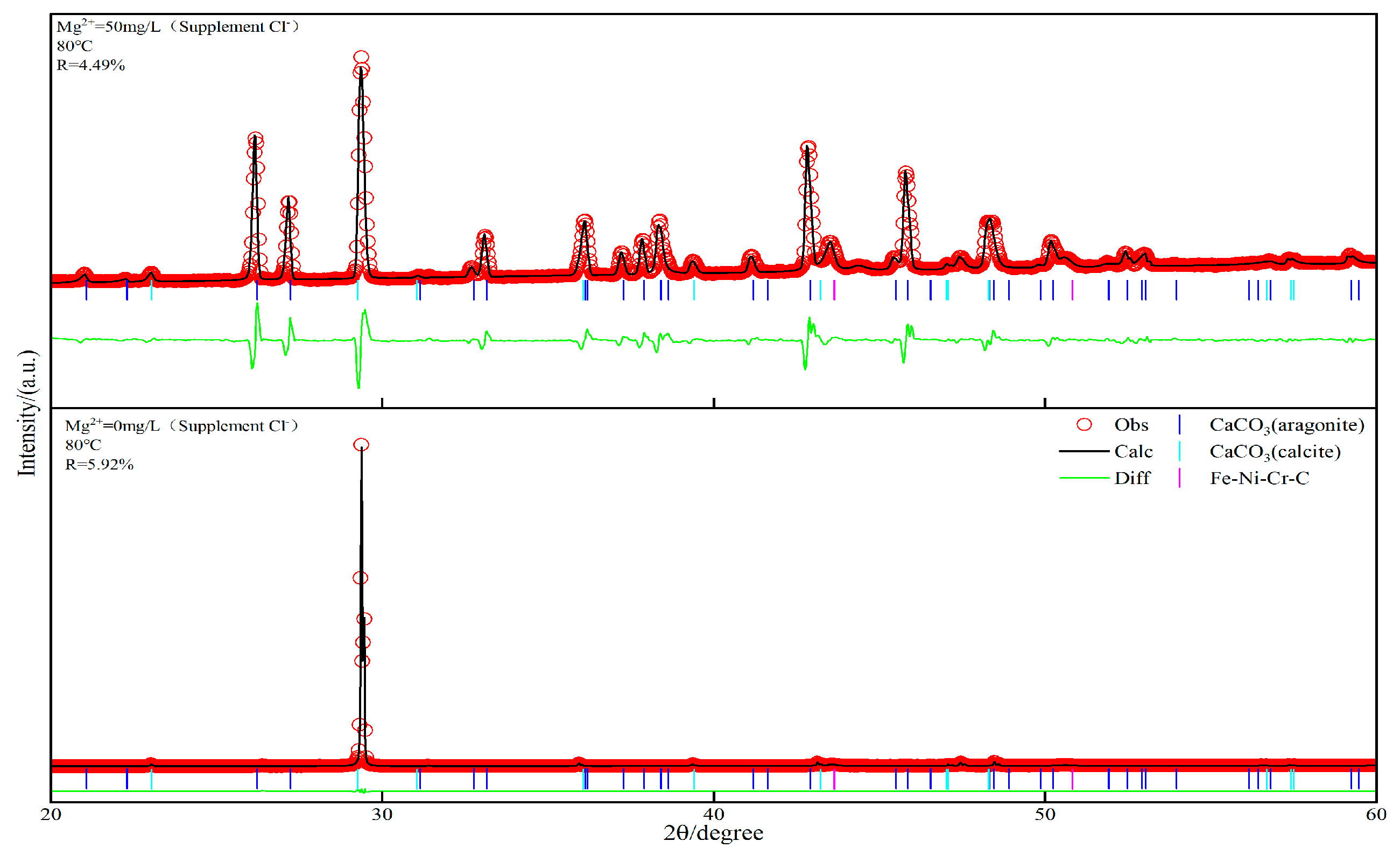

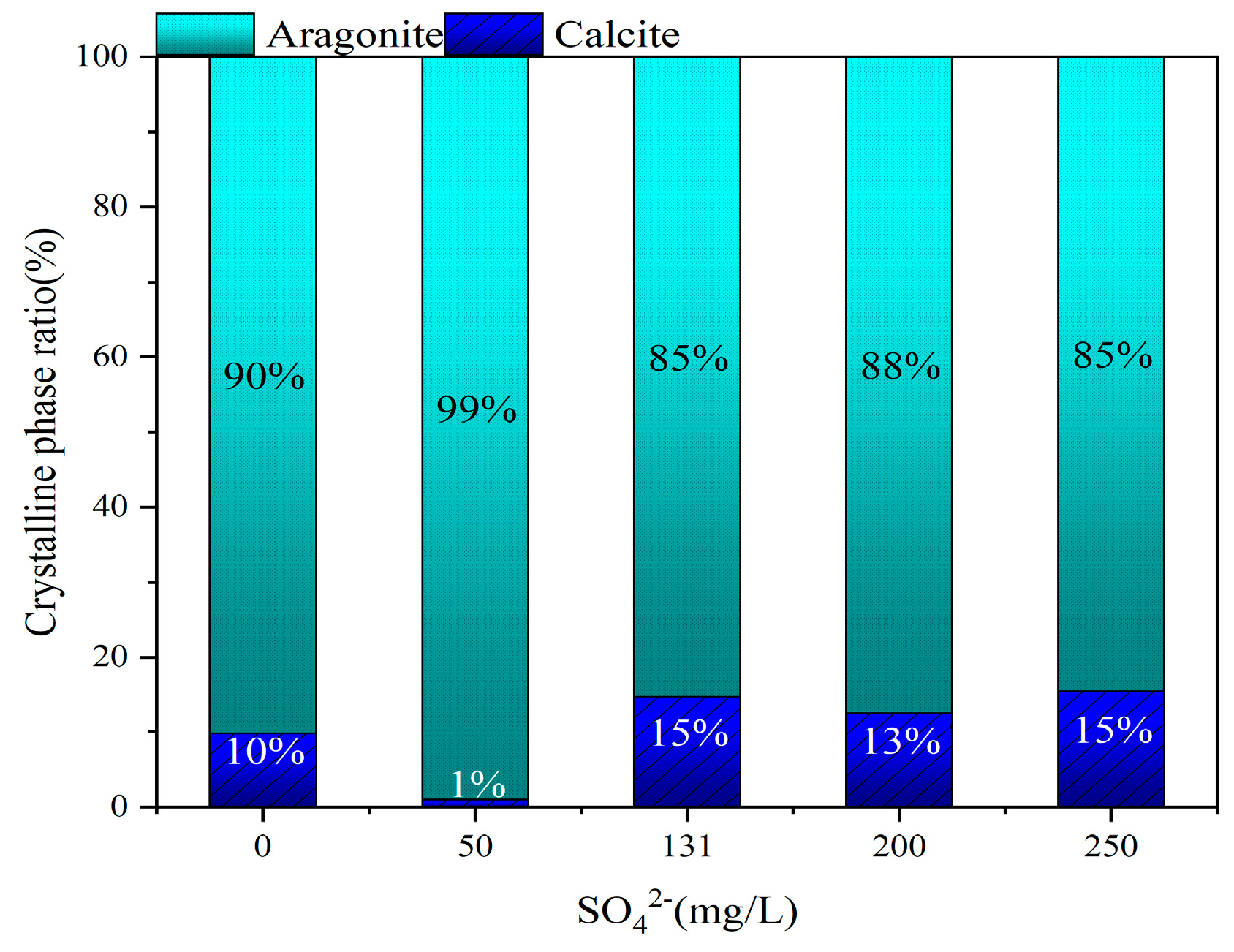

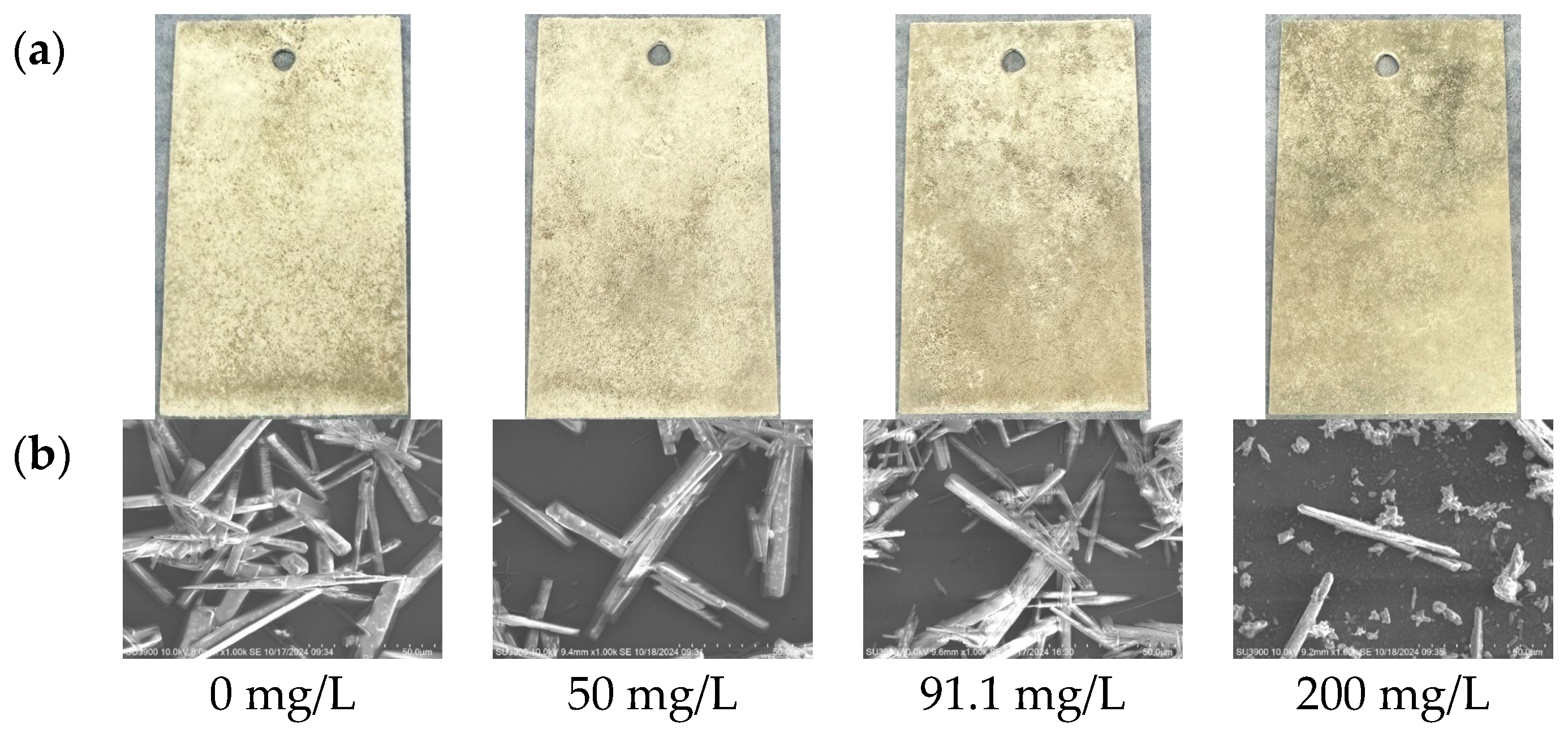

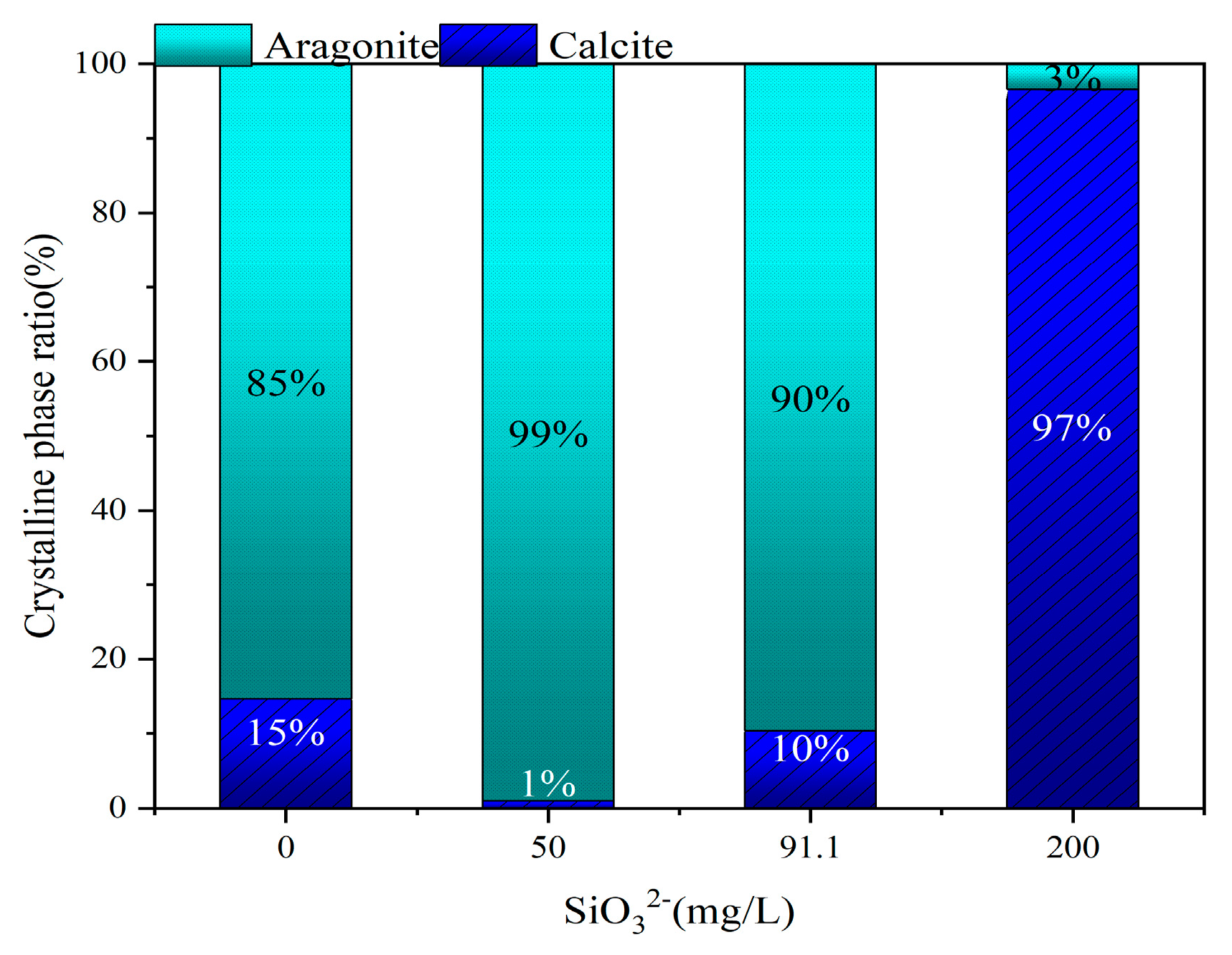
| NO. | WT | pH | TDS | Cl− | SO42− | HCO3− | Ca2+ | Mg2+ | Na+ | K+ | SiO2 |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1# | 78 | 7.22 | 1560 | 276.4 | 131 | 999 | 135 | 12.8 | 449 | 42.5 | 91.1 |
| 2# | 85.9 | 7.49 | 597 | 12.5 | 216 | 300 | 46.2 | 6.72 | 159 | 13.3 | 120 |
| 3# | 54.9 | 6.94 | 729 | 12.5 | 126 | 610 | 103 | 18.6 | 140 | 10 | 111 |
| 4# | 56.8 | 7.33 | 832 | 8.9 | 309 | 444 | 133 | 24.4 | 133 | 9.9 | 70.3 |
| 5# | 53.6 | 7.74 | 607 | 8.9 | 112 | 490 | 36.7 | 9.85 | 172 | 14.9 | 83.5 |
| 6# | 55.4 | 7.39 | 488 | 48.2 | 52 | 398 | 49.6 | 13.9 | 115 | 15.2 | 50.8 |
| NO. | Langelier Saturation Index Model | Ryznar Stability Index Model | Larson Index Model | |||
|---|---|---|---|---|---|---|
| Langelier Index | Scaling Tendency | Ryznar Index | Scaling Tendency | Larson Index | Corrosion (Scaling) Tendency | |
| 1# | 1.58 | Severe scaling | 4.06 | Severe scaling | 0.64 | Slight corrosive (Generally stable) |
| 2# | 0.99 | Slight scaling | 5.5 | Moderate scaling | 0.98 | Slight corrosive (Generally stable) |
| 3# | 0.67 | Slight scaling | 5.6 | Moderate scaling | 0.3 | Non-corrosive (Potential for scaling) |
| 4# | 1.06 | Severe scaling | 5.2 | Moderate scaling | 0.92 | Slight corrosive (Generally stable) |
| 5# | 0.91 | Slight scaling | 5.93 | Moderate scaling | 0.32 | Non-corrosive (Potential for scaling) |
| 6# | 0.65 | Slight scaling | 6.1 | Slight scaling | 0.37 | Non-corrosive (Potential for scaling) |
| Control Group | Composition | |||||||
|---|---|---|---|---|---|---|---|---|
| CaCl2 2H2O | MgCl2 6H2O | NaHCO3 | Na2SO4 | KCl | Na2SiO3 9H2O | NaCl | ||
| baseline | 495.2 | 107.1 | 1375.5 | 193.7 | 81 | / | / | |
| Ca2+ group (concentration, mg/L) | 0 | / | - | - | - | - | / | / |
| 0 | / | - | - | - | - | / | 393.8 | |
| 50 | 183 | - | - | - | - | / | / | |
| 50 | 183 | - | - | - | - | / | 247.9 | |
| 200 | 734.8 | - | - | - | - | / | / | |
| 250 | 918.4 | - | - | - | - | / | / | |
| Mg2+ group (concentration, mg/L) | 0 | - | / | - | - | - | / | / |
| 0 | - | / | - | - | - | / | 61.6 | |
| 5 | - | 41.8 | - | - | - | / | / | |
| 5 | - | 41.8 | - | - | - | / | 37.5 | |
| 20 | - | 167.3 | - | - | - | / | / | |
| 50 | - | 418.2 | - | - | - | / | / | |
| HCO3− group (concentration, mg/L) | 0 | - | - | - | - | - | / | / |
| 500 | - | - | 688.5 | - | - | / | / | |
| 1500 | - | - | 2065.4 | - | - | / | / | |
| 2000 | - | - | 2753.9 | - | - | / | / | |
| SO42− group (concentration, mg/L) | 0 | - | - | - | / | - | / | / |
| 50 | - | - | - | 73.9 | - | / | / | |
| 200 | - | - | - | 295.7 | - | / | / | |
| 250 | - | - | - | 369.6 | - | / | / | |
| SiO32− group (concentration, mg/L) | 50 | - | - | - | - | - | 185.2 | / |
| 91.1 | - | - | - | - | - | 339.6 | / | |
| 200 | - | - | - | - | - | 747.6 | / | |
| Grade | Chemical Composition (%) | |||||||
|---|---|---|---|---|---|---|---|---|
| C | Mn | Si | P | S | Ni | Cr | N | |
| SS304 | 0.053 | 0.85 | 0.43 | 0.032 | 0.007 | 8.00 | 18.12 | 0.061 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Y.; Li, Z.; Wang, H. Experimental Study on the Influence of Ion Components in Geothermal Water on Scaling Behavior. Energies 2025, 18, 946. https://doi.org/10.3390/en18040946
Yang Y, Li Z, Wang H. Experimental Study on the Influence of Ion Components in Geothermal Water on Scaling Behavior. Energies. 2025; 18(4):946. https://doi.org/10.3390/en18040946
Chicago/Turabian StyleYang, Yansong, Zhouhang Li, and Hua Wang. 2025. "Experimental Study on the Influence of Ion Components in Geothermal Water on Scaling Behavior" Energies 18, no. 4: 946. https://doi.org/10.3390/en18040946
APA StyleYang, Y., Li, Z., & Wang, H. (2025). Experimental Study on the Influence of Ion Components in Geothermal Water on Scaling Behavior. Energies, 18(4), 946. https://doi.org/10.3390/en18040946






