Topological Design of a Lightweight Sandwich Aircraft Spoiler
Abstract
:1. Introduction
2. Materials and Methods
2.1. Topology Optimization Method
2.2. Design Problem Definition
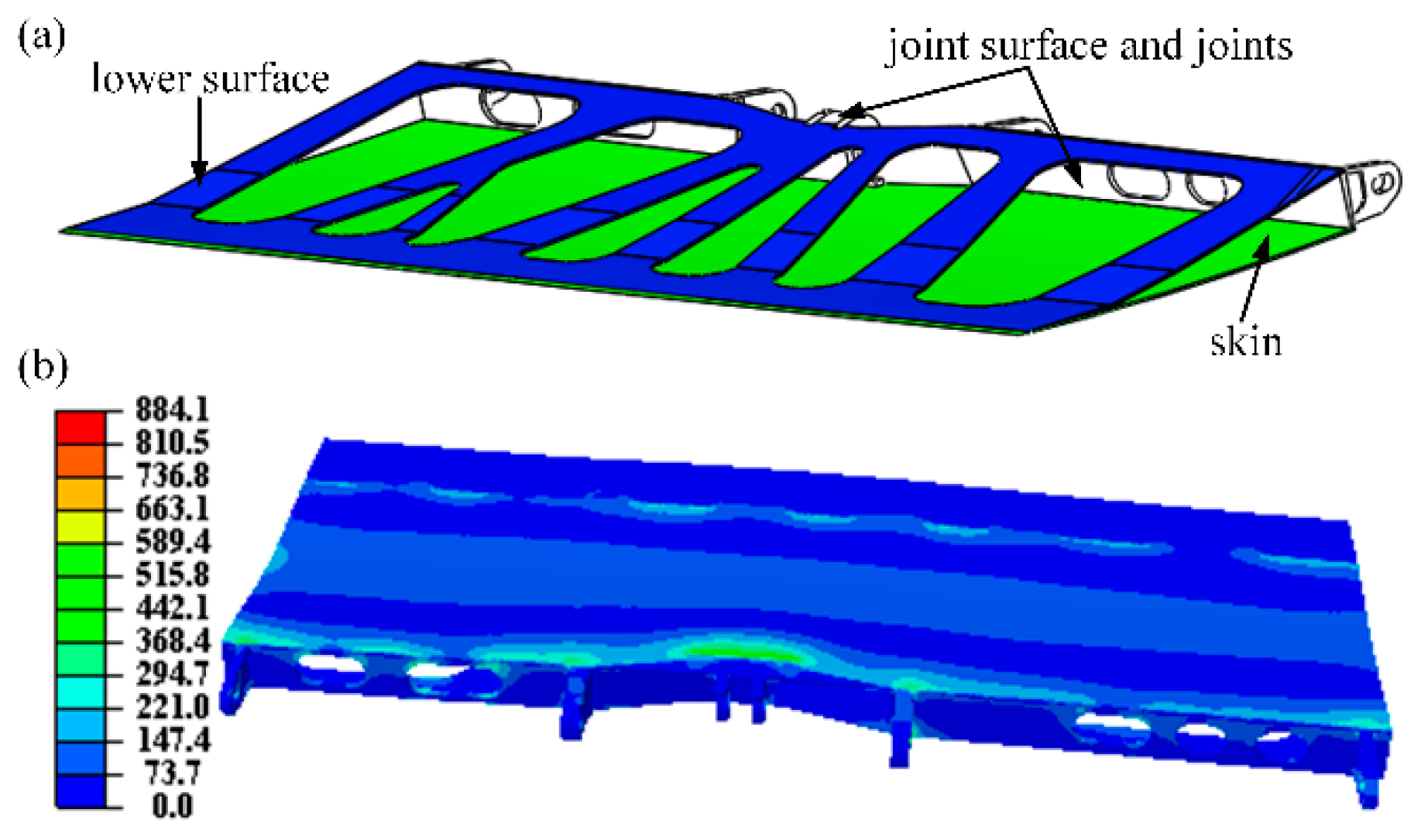
3. Results and Discussions
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Wang, X.L.; Wang, F.X.; Li, Y.L. Aerodynamic characteristics of high-lift devices with downward deflection of spoiler. J. Aircr. 2011, 48, 730–735. [Google Scholar] [CrossRef]
- Zhu, J.H.; Zhang, W.H.; Xia, L. Topology optimization in aircraft and aerospace structures design. Arch. Comput. Methods Eng. 2016, 23, 595–622. [Google Scholar] [CrossRef]
- Sigmund, O.; Maute, K. Topology optimization approaches. Struct. Multidiscip. Optim. 2013, 48, 1031–1055. [Google Scholar] [CrossRef]
- Huang, X.; Xie, Y.M. A further review of ESO type methods for topology optimization. Struct. Multidiscip. Optim. 2010, 41, 671–683. [Google Scholar] [CrossRef]
- Zhu, B.; Chen, Q.; Li, H.; Zhang, H.; Zhang, X. Design of planar large-deflection compliant mechanisms with decoupled multi-input-output using topology optimization. J. Mech. Robot. 2019, 11, 031015. [Google Scholar] [CrossRef]
- Liu, J.; Ma, Y. A survey of manufacturing oriented topology optimization methods. Adv. Eng. Softw. 2016, 100, 161–175. [Google Scholar] [CrossRef]
- Zegard, T.; Paulino, G.H. Bridging topology optimization and additive manufacturing. Struct. Multidiscip. Optim. 2016, 53, 175–192. [Google Scholar] [CrossRef]
- Guo, X.; Zhou, J.; Zhang, W.; Du, Z.; Liu, C.; Liu, Y. Self-supporting structure design in additive manufacturing through explicit topology optimization. Comput. Methods Appl. Mech. Eng. 2017, 323, 27–63. [Google Scholar] [CrossRef] [Green Version]
- Zhang, K.; Cheng, G.; Xu, L. Topology optimization considering overhang constraint in additive manufacturing. Comput. Struct. 2019, 212, 86–100. [Google Scholar] [CrossRef]
- Liu, Y.; Li, Z.; Wei, P.; Chen, S. Generating support structures for additive manufacturing with continuum topology optimization methods. Rapid Prototyp. J. 2019, 25, 232–246. [Google Scholar] [CrossRef]
- Mei, J.; Liu, J.; Liu, J. A novel fabrication method and mechanical behavior of all-composite tetrahedral truss core sandwich panel. Compos. Part A Appl. Sci. Manuf. 2017, 102, 28–39. [Google Scholar] [CrossRef]
- Wei, K.; Yang, Q.; Ling, B.; Xie, H.; Qu, Z.; Fang, D. Mechanical responses of titanium 3D kagome lattice structure manufactured by selective laser melting. Extrem. Mech. Lett. 2018, 23, 41–48. [Google Scholar] [CrossRef]
- Liu, J.; Chen, T.; Zhang, Y.; Wen, G.; Qing, Q.; Wang, H.; Sedaghati, R.; Xie, Y.M. On sound insulation of pyramidal lattice sandwich structure. Compos. Struct. 2019, 208, 385–394. [Google Scholar] [CrossRef]
- Yin, H.; Huang, X.; Scarpa, F.; Wen, G.; Chen, Y.; Zhang, C. In-plane crashworthiness of bio-inspired hierarchical honeycombs. Compos. Struct. 2018, 192, 516–527. [Google Scholar] [CrossRef] [Green Version]
- Liu, J.; Fan, X.; Wen, G.; Qing, Q.; Wang, H.; Zhao, G. A novel design framework for structures/materials with enhanced mechanical performance. Materials 2018, 11, 576. [Google Scholar] [CrossRef] [PubMed]
- Birman, V.; Kardomateas, G.A. Review of current trends in research and applications of sandwich structures. Compos. Part B Eng. 2018, 142, 221–240. [Google Scholar] [CrossRef]
- Caliri, M.F., Jr.; Ferreira, A.J.; Tita, V. A review on plate and shell theories for laminated and sandwich structures highlighting the Finite Element Method. Compos. Struct. 2016, 156, 63–77. [Google Scholar] [CrossRef]
- Remouchamps, A.; Bruyneel, M.; Fleury, C.; Grihon, S. Application of a bi-level scheme including topology optimization to the design of an aircraft pylon. Struct. Multidiscip. Optim. 2011, 44, 739–750. [Google Scholar] [CrossRef] [Green Version]
- Zhu, J.H.; Gu, X.J.; Zhang, W.H.; Beckers, P. Structural design of aircraft skin stretch-forming die using topology optimization. J. Comput. Appl. Math. 2013, 246, 278–288. [Google Scholar] [CrossRef]
- Liu, S.; Li, Q.; Chen, W.; Hu, R.; Tong, L. H-DGTP—A Heaviside-function based directional growth topology parameterization for design optimization of stiffener layout and height of thin-walled structures. Struct. Multidiscip. Optim. 2015, 52, 903–913. [Google Scholar] [CrossRef]
- Krog, L.; Tucker, A.; Kemp, M.; Boyd, R. Topology optimisation of aircraft wing box ribs. In Proceedings of the 10th AIAA/ISSMO Multidisciplinary Analysis and Optimization Conference, Albany, NY, USA, 30 August–1 September 2004. [Google Scholar]
- Maute, K.K.; Reich, G.W. Integrated multidisciplinary topology optimization approach to adaptive wing design. J. Aircr. 2006, 43, 253–263. [Google Scholar] [CrossRef]
- Zhu, J.; Zhang, W.; Beckers, P. Integrated layout design of multi-component system. Int. J. Numer. Methods Eng. 2009, 78, 631–651. [Google Scholar] [CrossRef]
- Xia, L.; Zhu, J.; Zhang, W. Sensitivity analysis with the modified Heaviside function for the optimal layout design of multi-component systems. Comput. Methods Appl. Mech. Eng. 2012, 241, 142–154. [Google Scholar] [CrossRef]
- Yang, K.; Zhu, J.; Wu, M.; Zhang, W. Integrated optimization of actuators and structural topology of piezoelectric composite structures for static shape control. Comput. Methods Appl. Mech. Eng. 2018, 334, 440–469. [Google Scholar] [CrossRef]
- Roy, S.; Crossley, W.A.; Stanford, B.; Moore, K.T.; Gray, J.S. A Mixed Integer Efficient Global Optimization Algorithm with Multiple Infill Strategy-Applied to a Wing Topology Optimization Problem. In Proceedings of the AIAA Scitech 2019 Forum, San Diego, CA, USA, 7–11 January 2019; p. 2356. [Google Scholar]
- Dubois, A.; Farhat, C.; Abukhwejah, A.H.; Shageer, H.M. Parameterization Framework for the MDAO of Wing Structural Layouts. AIAA J. 2018, 56, 1627–1638. [Google Scholar] [CrossRef]
- Bhattacharyya, A.; Conlan-Smith, C.; James, K.A. Design of a Bi-stable Airfoil with Tailored Snap-through Response Using Topology Optimization. Comput. Aided Des. 2019, 108, 42–55. [Google Scholar] [CrossRef]
- Guo, X.; Zhang, W.; Zhong, W. Doing topology optimization explicitly and geometrically—A new moving morphable components based framework. J. Appl. Mech. 2014, 81, 081009. [Google Scholar] [CrossRef]
- Zhang, W.; Yuan, J.; Zhang, J.; Guo, X. A new topology optimization approach based on Moving Morphable Components (MMC) and the ersatz material model. Struct. Multidiscip. Optim. 2016, 53, 1243–1260. [Google Scholar] [CrossRef]
- Zhang, W.; Chen, J.; Zhu, X.; Zhou, J.; Xue, D.; Lei, X.; Guo, X. Explicit three dimensional topology optimization via Moving Morphable Void (MMV) approach. Comput. Methods Appl. Mech. Eng. 2017, 322, 590–614. [Google Scholar] [CrossRef] [Green Version]
- Costa, J.C.A., Jr.; Alves, M.K. Layout optimization with h-adaptivity of structures. Int. J. Numer. Methods Eng. 2003, 58, 83–102. [Google Scholar] [CrossRef]
- Stainko, R. An adaptive multilevel approach to the minimal compliance problem in topology optimization. Commun. Numer. Methods Eng. 2006, 22, 109–118. [Google Scholar] [CrossRef]
- Wang, Y.; Kang, Z.; He, Q. An adaptive refinement approach for topology optimization based on separated density field description. Comput. Struct. 2013, 117, 10–22. [Google Scholar] [CrossRef]
- Wang, H.; Liu, J.; Wen, G. An efficient evolutionary structural optimization method with smooth edges based on the game of building blocks. Eng. Optim. 2019. [Google Scholar] [CrossRef]
- Wang, H.; Liu, J.; Wen, G. An adaptive mesh-adjustment strategy for continuum topology optimization to achieve manufacturable structural layout. Int. J. Numer. Methods Eng. 2019, 117, 1304–1322. [Google Scholar] [CrossRef]
- Liu, C.; Zhu, Y.; Sun, Z.; Li, D.; Du, Z.; Zhang, W.; Guo, X. An efficient moving morphable component (MMC)-based approach for multi-resolution topology optimization. Struct. Multidiscip. Optim. 2018, 58, 2455–2479. [Google Scholar] [CrossRef] [Green Version]
- Leader, M.K.; Chin, T.W.; Kennedy, G.J. High-resolution topology optimization with stress and natural frequency constraints. AIAA J. 2019. [Google Scholar] [CrossRef]
- Wei, P.; Li, Z.; Li, X.; Wang, M.Y. An 88-line MATLAB code for the parameterized level set method based topology optimization using radial basis functions. Struct. Multidiscip. Optim. 2018, 58, 831–849. [Google Scholar] [CrossRef]
- Zhu, B.; Zhang, X.; Liu, M.; Chen, Q.; Li, H. Topological and shape optimization of flexure hinges for designing compliant mechanisms using the level set method. Chin. J. Mech. Eng. 2019, 32, 13. [Google Scholar] [CrossRef]
- ABAQUS User’s Manual, version 6.14; Dassault Systèmes Simulia Corp.: Providence, RI, USA, 2014.
- SolidWorks User’s Guide, version 2014; SolidWorks Corp.: Waltham, MA, USA, 2014.
- Svanberg, K. The method of moving asymptotes—A new method for structural optimization. Int. J. Numer. Methods Eng. 1987, 24, 359–373. [Google Scholar] [CrossRef]
- Zuo, W.; Saitou, K. Multi-material topology optimization using ordered SIMP interpolation. Struct. Multidiscip. Optim. 2017, 55, 477–491. [Google Scholar] [CrossRef]
- Rong, J.H.; Tang, Z.L.; Xie, Y.M.; Li, F.Y. Topological optimization design of structures under random excitations using SQP method. Eng. Struct. 2013, 56, 2098–2106. [Google Scholar] [CrossRef]
- Long, K.; Wang, X.; Liu, H. Stress-constrained topology optimization of continuum structures subjected to harmonic force excitation using sequential quadratic programming. Struct. Multidiscip. Optim. 2019, 59, 1747–1759. [Google Scholar] [CrossRef]
- Liu, J.; Wen, G.; Xie, Y.M. Layout optimization of continuum structures considering the probabilistic and fuzzy directional uncertainty of applied loads based on the cloud model. Struct. Multidiscip. Optim. 2016, 53, 81–100. [Google Scholar] [CrossRef]
- Xie, Y.M.; Steven, G.P. A simple evolutionary procedure for structural optimization. Comput. Struct. 1993, 49, 885–896. [Google Scholar] [CrossRef]
- Hu, Z.; Gadipudi, V.K.; Salem, D.R. Topology optimization of lightweight lattice structural composites inspired by cuttlefish bone. Appl. Compos. Mater. 2019, 26, 15–27. [Google Scholar] [CrossRef]
- Aage, N.; Andreassen, E.; Lazarov, B.S.; Sigmund, O. Giga-voxel computational morphogenesis for structural design. Nature 2017, 550, 84. [Google Scholar] [CrossRef]
- Xue, X.; Pereira, A.; Vincze, G.; Wu, X.; Liao, J. Interfacial Characteristics of Dissimilar Ti6Al4V/AA6060 Lap Joint by Pulsed Nd: YAG Laser Welding. Metals 2019, 9, 71. [Google Scholar] [CrossRef]




| Material | Titanium Alloy | Aluminum Alloy |
|---|---|---|
| Density (kg/m3) | 4500 | 2760 |
| Tensile strength (MPa) | 900 | 450 |
| Compressive strength (MPa) | 880 | 270 |
| Modulus of elasticity (GPa) | 108 | 68 |
| Poisson’s ratio | 0.33 | 0.33 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, J.; Ou, H.; He, J.; Wen, G. Topological Design of a Lightweight Sandwich Aircraft Spoiler. Materials 2019, 12, 3225. https://doi.org/10.3390/ma12193225
Liu J, Ou H, He J, Wen G. Topological Design of a Lightweight Sandwich Aircraft Spoiler. Materials. 2019; 12(19):3225. https://doi.org/10.3390/ma12193225
Chicago/Turabian StyleLiu, Jie, Haifeng Ou, Junfeng He, and Guilin Wen. 2019. "Topological Design of a Lightweight Sandwich Aircraft Spoiler" Materials 12, no. 19: 3225. https://doi.org/10.3390/ma12193225
APA StyleLiu, J., Ou, H., He, J., & Wen, G. (2019). Topological Design of a Lightweight Sandwich Aircraft Spoiler. Materials, 12(19), 3225. https://doi.org/10.3390/ma12193225






