The Influence of Single-Walled Carbon Nanotubes on the Dynamic Properties of Nematic Liquid Crystals in Magnetic Field
Abstract
:1. Introduction
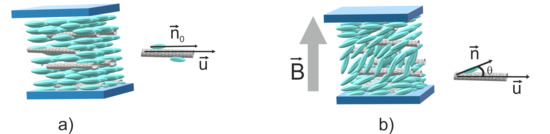
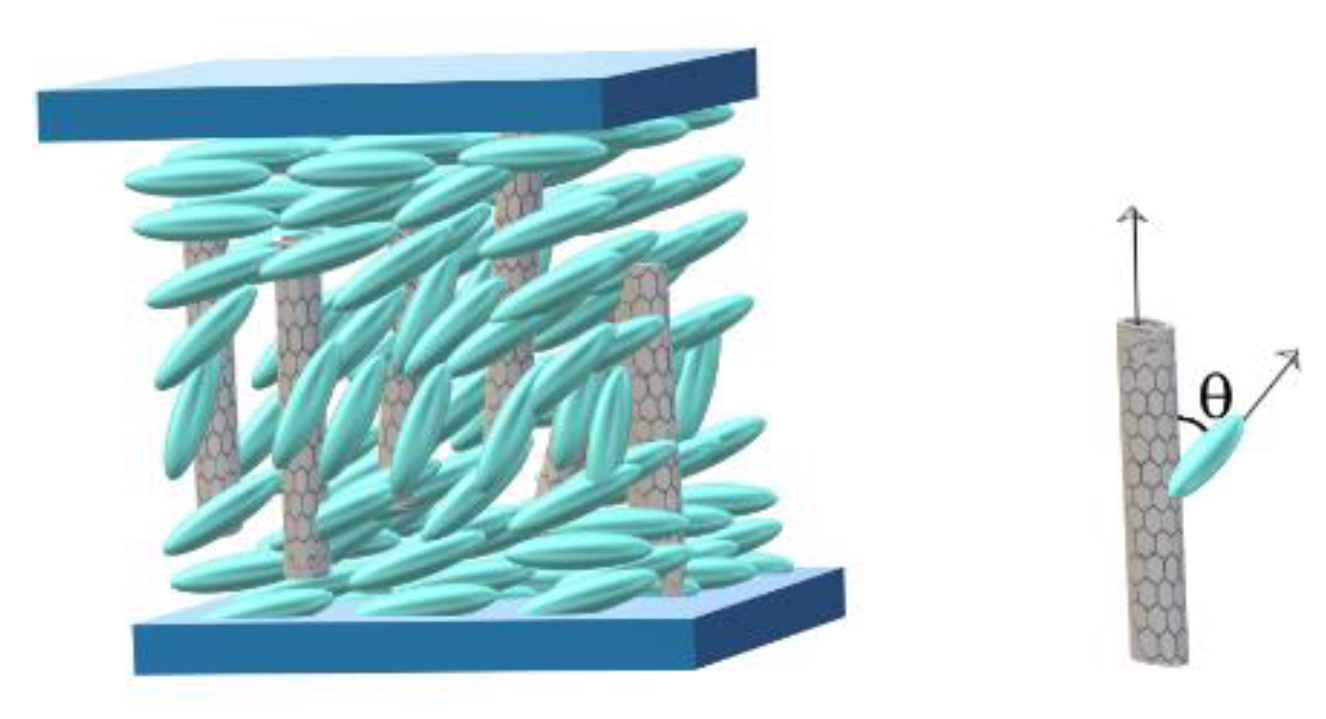
2. Molecular Interaction with the Inserted Carbon Nanotubes and Critical Field for Freedericksz Transition
3. Dynamic Behavior of Nematic Liquid Crystal with the Insertiona of Carbon Nanotubes
4. Set-Up and Procedure
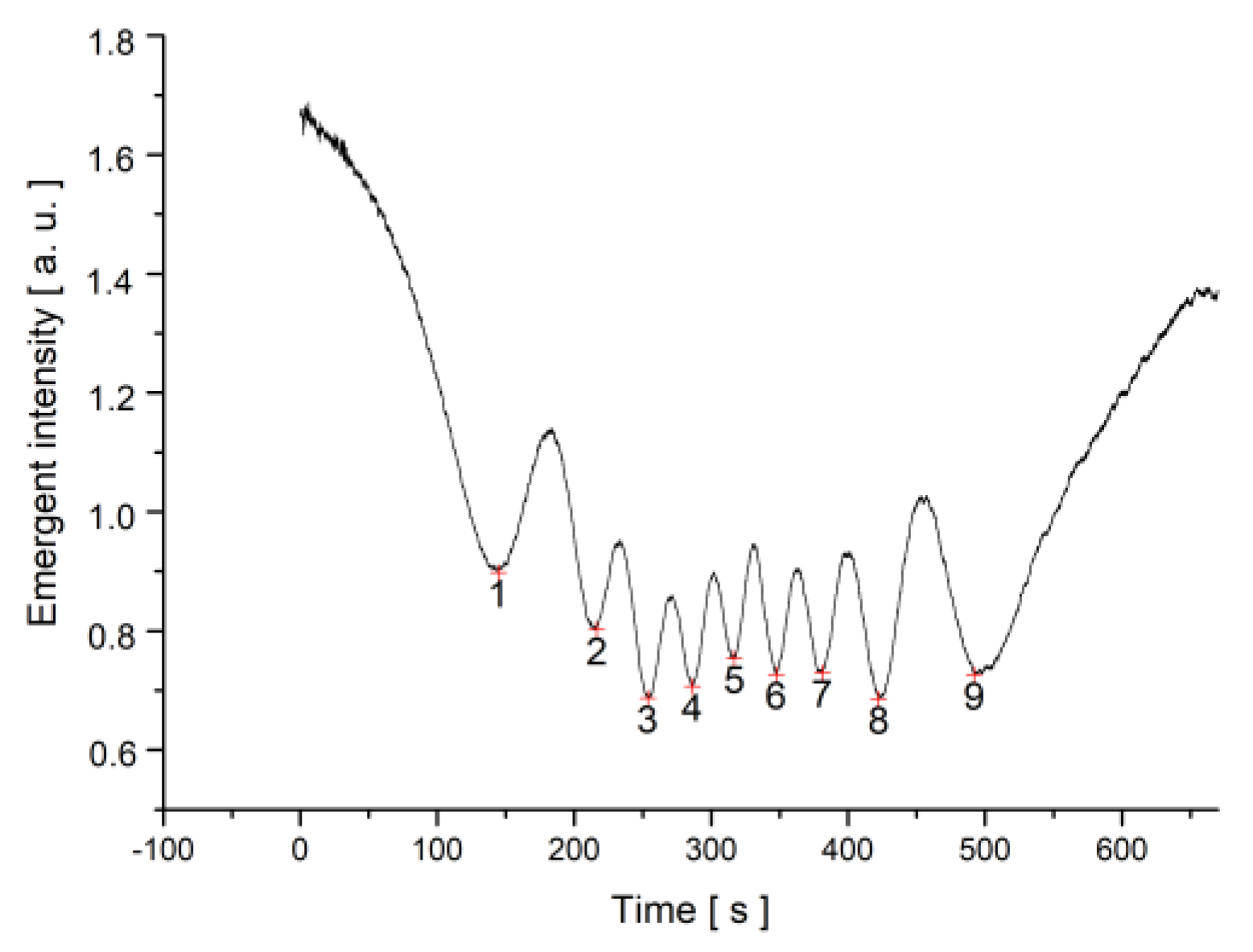
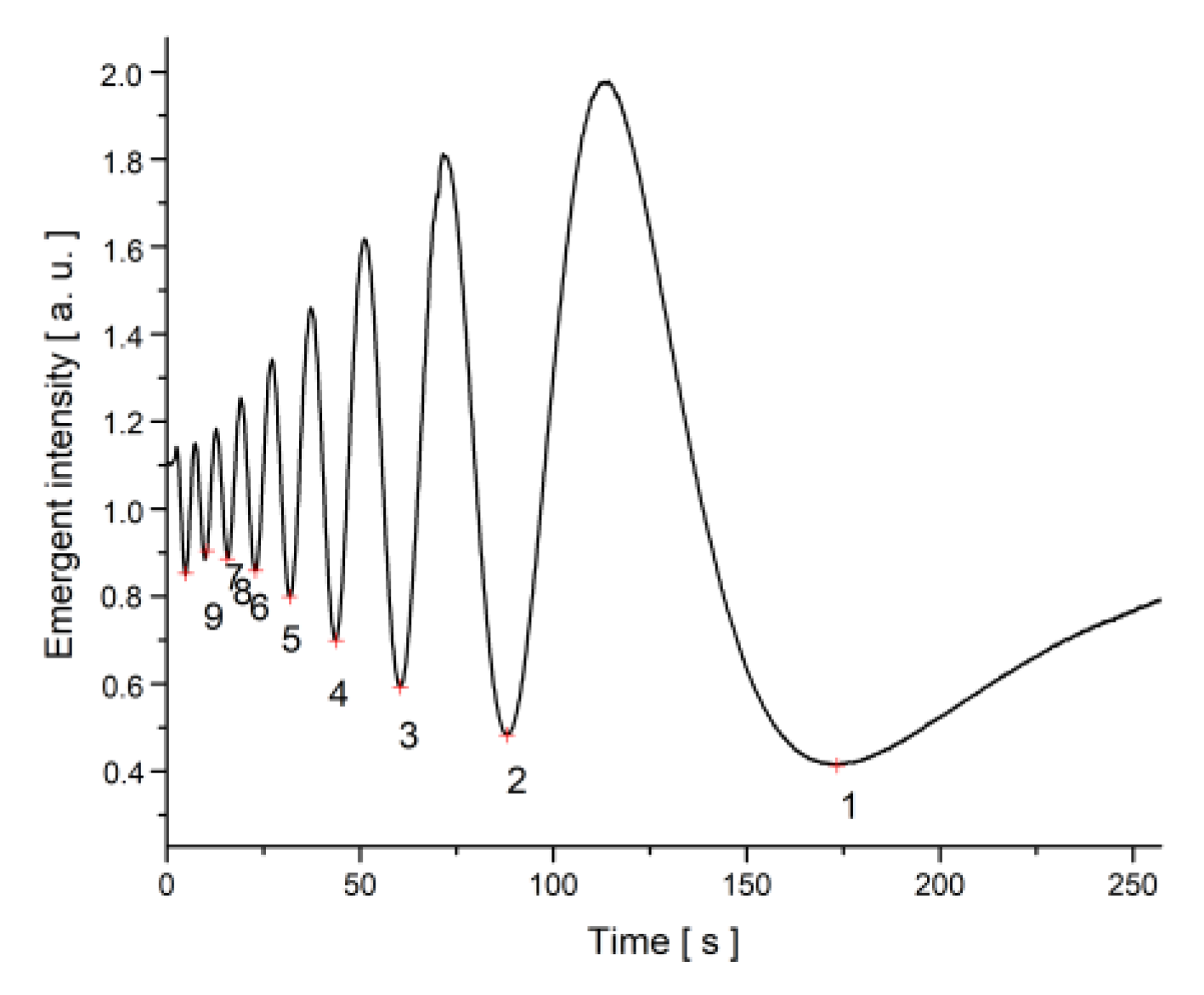
5. Results and Discussions
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Saravanan, M. Director deformation in a nematic liquid crystal with a ferromagnetic nanoparticle suspension. Chin. J. Phys. 2019, 59, 426–433. [Google Scholar] [CrossRef]
- Kyrou, C.; Kralj, S.; Panagopoulou, M.; Raptis, Y.; Nounesis, G.; Lelidis, I. Impact of spherical nanoparticles on nematic order parameters. Phys. Rev. E 2018, 97, 042701. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Saravanan, M.; Ameya Jagtap, D.; Murthy Vasudeva, A.S. Perturbed soliton and director deformation in a ferronematic liquid crystal. Chaos Solitons Fractals 2018, 106, 220–226. [Google Scholar]
- Romero-Hasler, P.; Martinez-Miranda, L.J.; Meneses-Franco, A. TiO2 nanoparticle—Liquid crystal interaction with smectogenic monomers and their electropolymerised polymers. Liq. Cryst. 2019. [CrossRef]
- Dehghani, Z.; Dalir, N.; Nadafan, M.; Ara, M.H.M.; Iranizad, E. Investigation of electrical and nonlinear optical properties of colloidal composite nematic liquid crystal. J. Mol. Liq. 2017, 225, 502–509. [Google Scholar] [CrossRef]
- Yadav, S.; Singh, S. Carbon nanotube dispersion in nematic liquid crystals: An overview. Prog. Mater. Sci. 2016, 80, 38–76. [Google Scholar] [CrossRef]
- Garbovskiy, Y.; Glushchenko, I. Nano-objects and ions in liquid crystals: Ion trapping effect and related phenomena. Crystals 2015, 5, 501–533. [Google Scholar] [CrossRef]
- Lisetski, L.N.; Fedoryako, A.P.; Samoilov, A.N.; Minenko, S.S.; Soskin, M.S.; Lebovka, N.I. Nano-objects and ions in liquid crystals: Ion trapping effect and related phenomena. Eur. Phys. J. E 2014, 37, 68. [Google Scholar] [CrossRef]
- Atasiei, R.; Dascalu, C.; Raicopol, M. The time dependence of the electric charge in a nematic cell aligned with doped polypyrrole. Univ. Politeh. Buchar. Ser. A 2013, 74, 293–298. [Google Scholar]
- Staic, M.; Petrescu-Nita, A. Symmetry group of two special types of carbon nanotori. Acta Crystallogr. 2013, 69, 435–439. [Google Scholar] [CrossRef]
- Chang, C.; Zhao, Y.; Liu, Y.; An, L. Liquid crystallinity of carbon nanotubes. RSC Adv. 2018, 8, 15780–15795. [Google Scholar] [CrossRef] [Green Version]
- Liu, X.; Li, X. Study on Tunable Self-Collimation in Photonic Crystals. J. Nanoelectron. Optoelectron. 2017, 12, 575–579. [Google Scholar] [CrossRef]
- Kedzierski, K.; Rytel, K.; Barszcz, B.; Gronostaj, A.; Majchrzycki, L. On the temperature dependent electrical resistivity of CNT layers in view of Variable Range Hopping models. Org. Electron. 2017, 43, 253–261. [Google Scholar] [CrossRef]
- Bale, S.; Liyana-Arachchi, T.; Hung, F. Molecular dynamics simulation of single-walled carbon nanotubes inside liquid crystals. Mol. Simul. 2016, 42, 1242–1248. [Google Scholar] [CrossRef]
- Denkta, C.; Ocak, H.; Okutan, M.; Yildiz, A.; Bilgin Eranc, B.; Köysal, O. Effect of multi wall carbon nanotube on electrical properties 4-[4-((S)-Citronellyloxy) benzoyloxy]benzoic acid liquid crystal host. Compos. Part B Eng. 2015, 82, 173–177. [Google Scholar] [CrossRef]
- Volpati, D.; Massey, M.K.; Johnson, D.W.; Kotsialos, A.; Qaiser, F.; Pearson, C.; Cole- man, K.S.; Tiburzi, G.; Zeze, D.A.; Petty, M.C. Exploring the alignment of carbon nanotubes dispersed in a liquid crystal matrix using coplanar electrodes. J. Appl. Phys. 2015, 117, 125303. [Google Scholar] [CrossRef] [Green Version]
- Petrov, D.A.; Zakhlevnykh, A.N. Statistical theory of magnetic field induced phase transitions in negative diamagnetic anisotropy liquid crystals doped with carbon nanotubes. J. Mol. Liq. 2019, 287, 110901. [Google Scholar] [CrossRef]
- Petrescu, E.; Cirtoaje, C. Dynamic behavior of a nematic liquid crystal with added carbon nanotubes in an electric field. Beilstein J. Nanotechnol. 2018, 9, 233–241. [Google Scholar] [CrossRef]
- Barar, A.; Manaila-Maximean, D.; Vladescu, M.; Schiopu, P. Simulation of charge carrier transport mechanisms for quantum dot-sensitized solar cell structures. Univ. Politeh. Buchar. Ser. A 2019, 81, 265–270. [Google Scholar]
- Petrov, D.A.; Zakhlevnykh, A.N.; Mantsurov, A.V. Ordering of a Liquid-Crystal Suspension of Carbon Nanotubes in a Magnetic Field. J. Exp. Theor. Phys. 2018, 127, 357–369. [Google Scholar] [CrossRef]
- Zakhlevnykh, A.N.; Petrov, D.A.; Skokov, P.K. Influence of Ferromagnetic Carbon Nanotubes on Magnetic Transitions in Liquid Crystals. J. Exp. Theor. Phys. 2018, 18, 767–777. [Google Scholar] [CrossRef]
- Petrov, D.A.; Skokov, P.K.; Zakhlevnykh, A.N.; Makarov, D.V. Magnetic segregation effect in liquid crystals doped with carbon nanotubes. Beilstein J. Nanotechol. 2019, 10, 1464–1474. [Google Scholar] [CrossRef]
- Dierking, I.; Scalia, G.; Morales, P.; LeClere, D. Aligning and Reorienting Carbon Nanotubes with Nematic Liquid Crystals. Adv. Mater. 2004, 16, 865–869. [Google Scholar] [CrossRef]
- Lynch, M.; Patrick, D. Organizing Carbon Nanotubes with Liquid Crystals. Nano Lett. 2002, 2, 1194–1201. [Google Scholar] [CrossRef]
- van der Schoot, P.; Popa-Nita, V.; Kralj, S. Alignment of Carbon Nanotubes in Nematic Liquid Crystals. J. Phys. Chem. B 2008, 1812, 4512–4518. [Google Scholar] [CrossRef]
- Li, R.J.; Cao, L.C. Progress in Phase Calibration for Liquid Crystal Spatial Light Modulators. Appl. Sci. 2019, 9, 2012. [Google Scholar] [CrossRef] [Green Version]
- Chang, C.M.; Lin, Y.H.; Srivastava, A.K.; Chigrinov, V.G. An optical system via liquid crystal photonic devices for photobiomodulation. Sci. Rep. 2018, 8, 4251. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Otón, J.M.; Otón, E.; Quintana, X.; Geday, M.A. Liquid-crystal phase-only devices. J. Mol. Liq. 2018, 267, 469–483. [Google Scholar] [CrossRef]
- Zhang, Z.C.; You, Z.; Chu, D.P. Fundamentals of phase-only liquid crystal on silicon (LCOS) devices. Light Sci. Appl. 2014, 3, e213. [Google Scholar] [CrossRef]
- Caño-Garcia, M.; Elmogi, A.; Mattelin, M.A.; Missinne, J.; Geday, M.A.; Otón, J.M.; Van Steenberge, G.; Quintana, X. All-organic switching polarizer based on polymer waveguides and liquid crystals. Opt. Express 2018, 26, 9584–9594. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cirtoaje, C.; Petrescu, E. Measurement of magnetic anisotropy of multiwalled carbon nanotubes in nematic host. Phys. E Low-Dimens. Syst. Nanostruct. 2016, 84, 244–248. [Google Scholar] [CrossRef]
- Burylov, S.V.; Zakhlevnykh, A.N. Orientational energy of anisometric particles in liquid-crystalline suspensions. Phys. Rev. E 2013, 88, 012511. [Google Scholar] [CrossRef] [PubMed]
- Pieranski, P.; Brochard, F.; Guyon, E. Static and dynamic behavior of a nematic liquid crystal in a magnetic field. Part II: Dynamics. J. Phys. 1973, 34, 35–48. [Google Scholar]
- Iacobescu, G.; Paun, A.L.; Cirtoaje, C. Magnetically induced Freedericksz transition and relaxation phenomena in nematic liquid crystals doped with azo-dyes. J. Magn. Magn. Mater. 2008, 431, 62–65. [Google Scholar] [CrossRef]
- Petrescu, E.; Motoc, C.; Manaila, D. A dynamical method for determining the elastic constants and relaxation parameters in nematic liquid crystals. Mod. Phys. Lett. B 2000, 14, 139–146. [Google Scholar] [CrossRef]
- Cirtoaje, C.; Motoc, C.; Petrescu, E.; Bena, R. New magnetic methods for determination of elastic constants and rotational viscosity coefficient in nematic liquid crystals. Univ. Politeh. Buchar. Ser. A 2010, 72, 233–246. [Google Scholar]
- Basu, R.; Garvey, A. Insulator-to-conductor transition in liquid crystal-carbon nanotube nanocomposites. J. Appl. Phys. 2016, 120, 164309. [Google Scholar] [CrossRef]






| B(T) | LC | LC+SWCNT | ||
|---|---|---|---|---|
| 0.1035 ± 0.00517 | 33.5 ± 1.8 | 36.9 ± 1.9 | 37.6 ± 1.9 | 39.5 ± 1.9 |
| 0.1074 ± 0.00537 | 28.6 ± 1.7 | 30.2 ± 1.5 | 31.5 ± 1.6 | 32.0 ± 1.6 |
| 0.1114 ± 0.00557 | 25.7 ± 1.7 | 25.4 ± 1.3 | 28.9 ± 1.5 | 26.6 ± 1.3 |
| 0.1153 ± 0.00577 | 25.2 ± 1.6 | 21.8 ± 1.1 | 26.0 ± 1.3 | 22.7 ± 1.4 |
| B(T) | LC | LC+SWCNT | ||
|---|---|---|---|---|
| Theor (s) | ||||
| 0.1035 ± 0.00517 | 63.3 ± 3.2 | 62.3 ± 3.1 | 80.0 ± 4.0 | 78.2 ± 3.9 |
| 0.1074 ± 0.00537 | 64.1 ± 3.2 | 81.3 ± 4.1 | ||
| 0.1114 ± 0.00557 | 62.9 ± 3.1 | 80.0 ± 4.0 | ||
| 0.1153 ± 0.00577 | 63.7 ± 3.2 | 80.4 ± 4.0 | ||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cirtoaje, C.; Petrescu, E. The Influence of Single-Walled Carbon Nanotubes on the Dynamic Properties of Nematic Liquid Crystals in Magnetic Field. Materials 2019, 12, 4031. https://doi.org/10.3390/ma12244031
Cirtoaje C, Petrescu E. The Influence of Single-Walled Carbon Nanotubes on the Dynamic Properties of Nematic Liquid Crystals in Magnetic Field. Materials. 2019; 12(24):4031. https://doi.org/10.3390/ma12244031
Chicago/Turabian StyleCirtoaje, Cristina, and Emil Petrescu. 2019. "The Influence of Single-Walled Carbon Nanotubes on the Dynamic Properties of Nematic Liquid Crystals in Magnetic Field" Materials 12, no. 24: 4031. https://doi.org/10.3390/ma12244031
APA StyleCirtoaje, C., & Petrescu, E. (2019). The Influence of Single-Walled Carbon Nanotubes on the Dynamic Properties of Nematic Liquid Crystals in Magnetic Field. Materials, 12(24), 4031. https://doi.org/10.3390/ma12244031