p-n Control of AlMgB14-Based Thermoelectric Materials by Metal Site Occupancy
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
3.1. Rietveld Analysis
3.2. Thermoelectric Properties
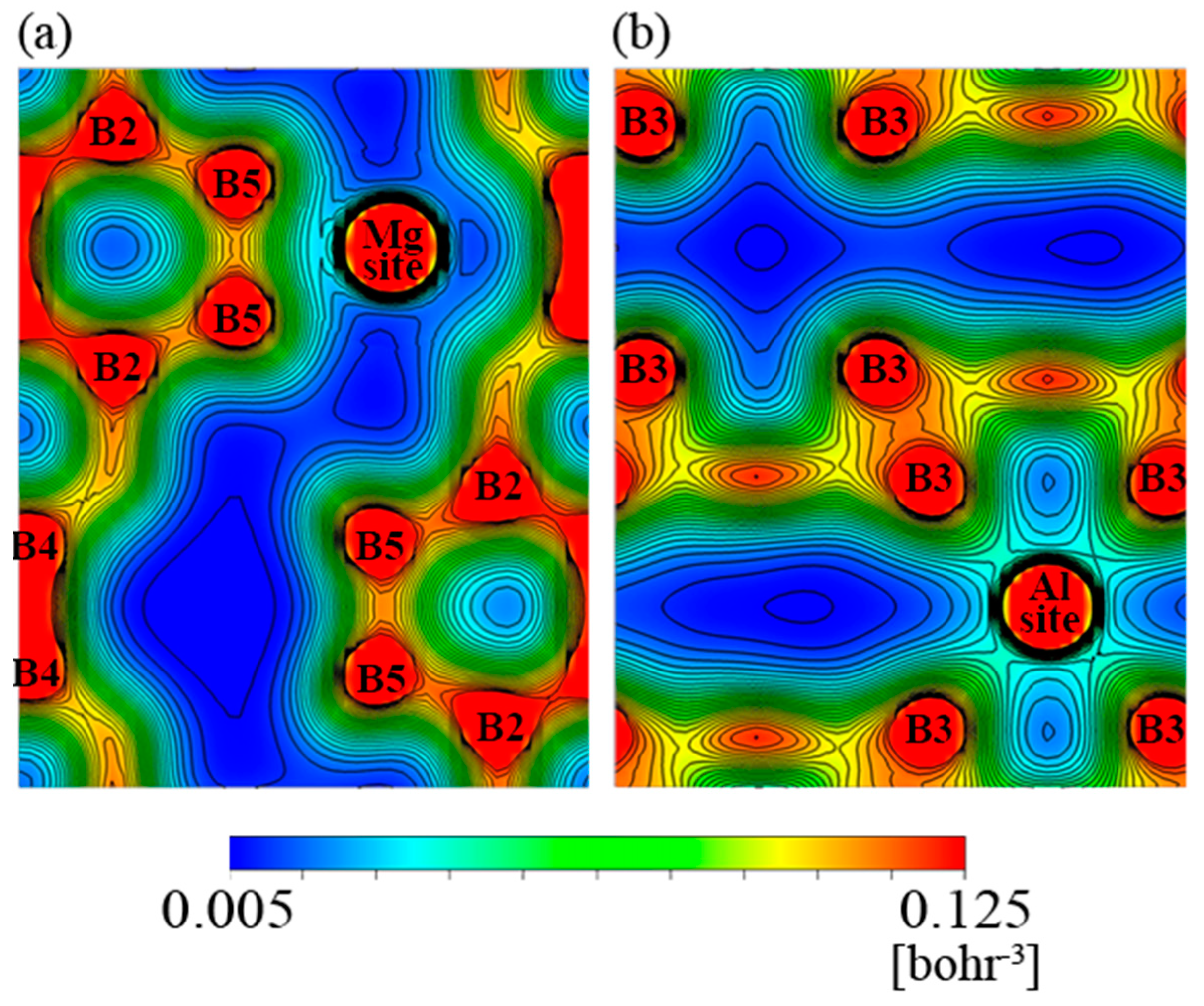
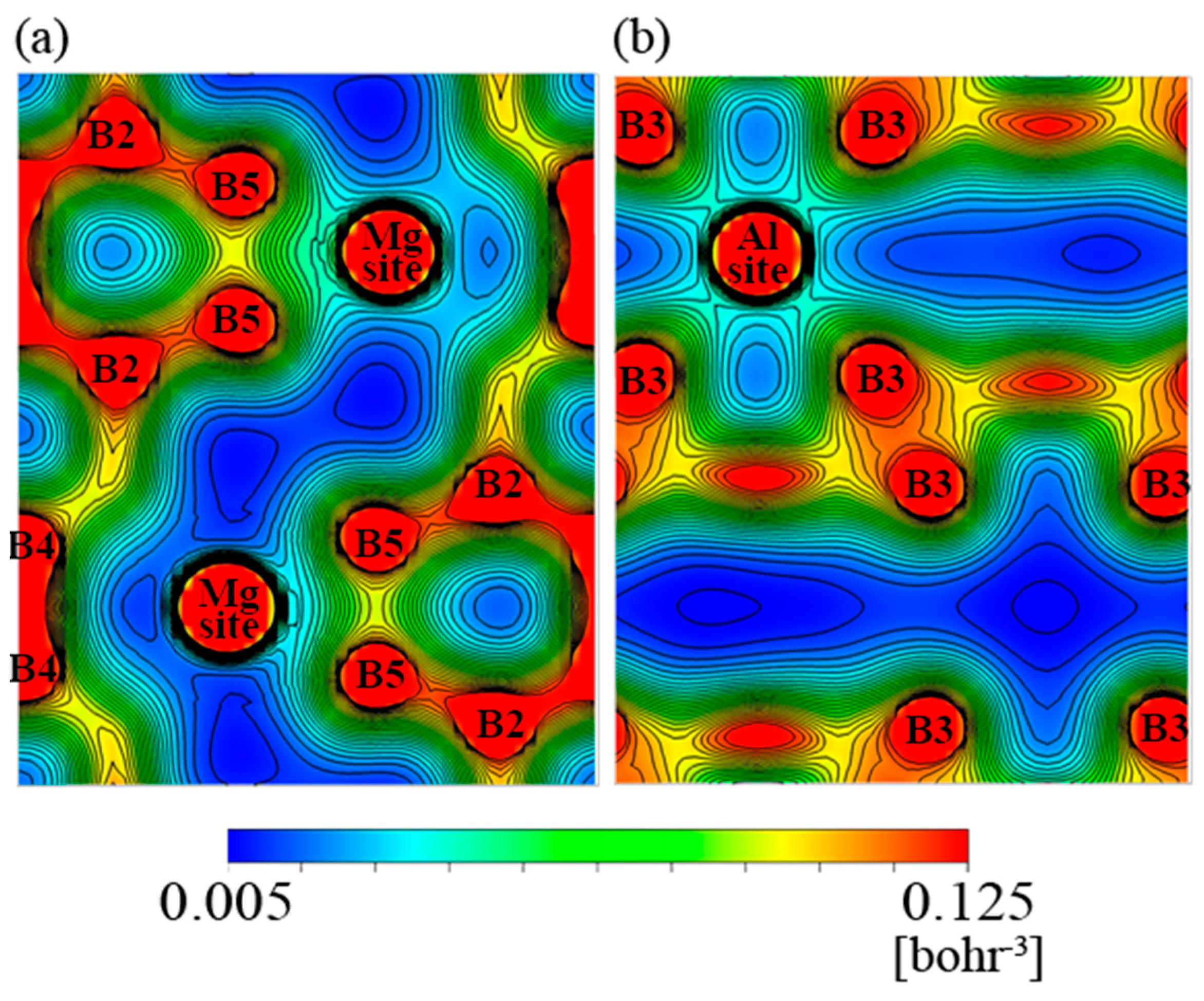
3.3. Results of First-Principle Band Calculation
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Killander, A.; Bass, J.C. A Stove-Top Generator for Cold Areas. In Proceedings of the 15th International Conference on Thermoelectrics, Pasadena, CA, USA, 26–29 March 1996; pp. 390–393. [Google Scholar]
- Qiu, K.; Hayden, A.C.S. Development of a thermoelectric self-powered residential heating system. J. Power. Sources 2008, 180, 884–889. [Google Scholar] [CrossRef]
- Dan, D.; Zhou, Y.; Liu, J. Liquid metal based thermoelectric generation system for waste heat recovery. Renew. Energy 2011, 36, 3530–3536. [Google Scholar]
- Rowe, D.M. Modules, Systems, and Applications in Thermoelectrics; CRC Press: London, UK, 2012. [Google Scholar]
- Koumoto, K.; Mori, T. Thermoelectric Nanomaterials: Materials Design and Applications; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Ioffe, A.F. Semiconductor Thermoelements, and Thermoelectric; Cool-ingInfosearch Ltd.: London, UK, 1957. [Google Scholar]
- Rowe, D.M. CRC Handbook of Thermoelectrics; CRC Press: Boca Raton, FL, USA, 1995. [Google Scholar]
- Omer, S.A.; Infield, D.G. Design optimization of thermoelectric devices for solar power generation. Sol. Energy Mater. Sol. Cells 1998, 53, 67–82. [Google Scholar] [CrossRef]
- Takeda, M.; Fukuda, T.; Domingo, F.; Miura, T. Thermoelectric properties of some metal borides. J. Solid State Chem. 2004, 177, 471–475. [Google Scholar] [CrossRef]
- Kim, H.K.; Nakayama, T.; Shimizu, J.; Kimura, K. Effects of Metal Doping on Thermoelectric Properties of Arc-Melted and Hot-Pressed β-Rhombohedral Boron. Mater. Trans. 2008, 49, 593–599. [Google Scholar] [CrossRef]
- Mori, T.; Shishido, T.; Nakajima, K. Doping Effects in Rare-Earth Borides. J. Electron. Mater. 2009, 38, 1098–1103. [Google Scholar] [CrossRef]
- Sologub, O.; Mori, T. Structural and thermoelectric properties of Y1−xB22+yC2−yN. J. Phys. Chem. Solids 2013, 74, 1109–1114. [Google Scholar] [CrossRef]
- Nakayama, T.; Shimizu, J.; Kimura, K. Thermoelectric Properties of Metal-Doped β-Rhombohedral Boron. J. Solid State Chem. 2000, 154, 13–19. [Google Scholar] [CrossRef]
- Werheit, H. Present knowledge of electronic properties and charge transport of icosahedral boron-rich solids. J. Phys. Conf. Ser. 2009, 176, 012019. [Google Scholar] [CrossRef]
- Wood, C.; Emin, D. Conduction Mechanism in boron carbide. Phys. Rev. B 1984, 29, 4582–4587. [Google Scholar] [CrossRef]
- Bouchacourt, M.; Thevenot, F. The correlation between the thermoelectric properties and stoichiometry in the boron carbide phase B4C-B10.5C. Mater. Sci. 1985, 20, 1237–1247. [Google Scholar] [CrossRef]
- Werheit, H. Boron-rich solids: a chance for high-efficiency high-temperature thermoelectric energy conversion. Mater. Sci. Eng. 1995, B29, 228–232. [Google Scholar] [CrossRef]
- Emin, D. Unusual properties of icosahedral boron-rich solids. J. Solid. State Chem. 2006, 179, 2791–2798. [Google Scholar] [CrossRef]
- Mori, T.; Nishimura, T.; Yamaura, K.; Takayama-Muromachi, E. High temperature thermoelectric properties of a homologous series of n-type boron icosahedra compounds: A possible counterpart to p-type boron carbide. J. Appl. Phys. 2007, 101, 093714. [Google Scholar] [CrossRef]
- Emin, D. Icosahedral Boron-Rich Solids. Phys. Today 1987, 40, 55. [Google Scholar] [CrossRef]
- Mori, T. High temperature thermoelectric properties of B12 icosahedral cluster-containing rare earth boride crystals. J. Appl. Phys. 2005, 97, 093703. [Google Scholar] [CrossRef]
- Kim, H.; Kimura, K. Vanadium Concentration Dependence of Thermoelectric Properties of β-Rhombohedral Boron Prepared by Spark Plasma Sintering. Mater. Trans. 2011, 52, 41–48. [Google Scholar] [CrossRef]
- Uehara, M.; Shiraishi, R.; Nogami, A.; Enomoto, N.; Hojo, J. SiC-B4C composites for synergistic enhancement of thermoelectric property. J. Eur. Ceram. Soc. 2004, 24, 409–412. [Google Scholar] [CrossRef]
- Hossain, M.A.; Tanaka, I.; Tanaka, T.; Khan, A.U.; Mori, T. YB48 the metal rich boundary of YB66; crystal growth and thermoelectric properties. J. Phys. Chem. Solids 2015, 87, 221–227. [Google Scholar] [CrossRef]
- Golikova, A.; Higashi, I. MgAlB14: Structure and Doping. JJAP Ser. 1994, 10, 52–53. [Google Scholar]
- Sasaki, H.; Fujima, T.; Miura, S.; Fujima, T.; Takagi, K. Thermoelectric properties of higher boride-intermetallics composite materials made from MgAlB14 by spark plasma sintering. J. Solid. State Chem. 2012, 14, 1698–1701. [Google Scholar] [CrossRef]
- Fujima, T.; Arimatsu, H.; Miura, S.; Yokoyama, S.; Takagi, K. n-p Type variation in thermoelectric AlMgB14–based materials by raw material mixture ratio. Solid State Sci. 2015, 47, 51–54. [Google Scholar] [CrossRef]
- Izumi, F.; Momma, K. Three-Dimensional Visualization in Powder Diffraction. Solid State Phenom. 2007, 130, 15–20. [Google Scholar] [CrossRef]
- Blaha, P.; Schwarz, K.; Madsen, G.K.H.; Kvasnicka, D.; Luitz, J.; Laskowski, R.; Tran, F.; Marks, L.D. WIEN2k, An Augmented Plane Wave + Local Orbitals Program for Calculating Crystal Properties; Karlheinz Schwarz, Techn. Universität Wien: Vienna, Austria, 2001. [Google Scholar]
- Mott, N.F.; Jones, H. The Theory of the Properties of Metals and Alloys; Oxford Univ. Press: London, UK, 1939; pp. 305–314. [Google Scholar]
- Sahara, R.; Mori, T.; Maruyama, S.; Miyazaki, Y.; Hayashi, K.; Kajitani, T. Theoretical and experimental investigation of the excellent p–n control in yttrium aluminoborides. Sci. Technol. Adv. Mater. 2014, 15, 35012–35019. [Google Scholar] [CrossRef] [PubMed]
- Maruyama, S.; Miyazaki, Y.; Hayashi, K.; Kajitani, T.; Mori, T. Excellent p-n control in a high temperature thermoelectric boride. Appl. Phys. Lett. 2012, 101, 152101. [Google Scholar] [CrossRef]
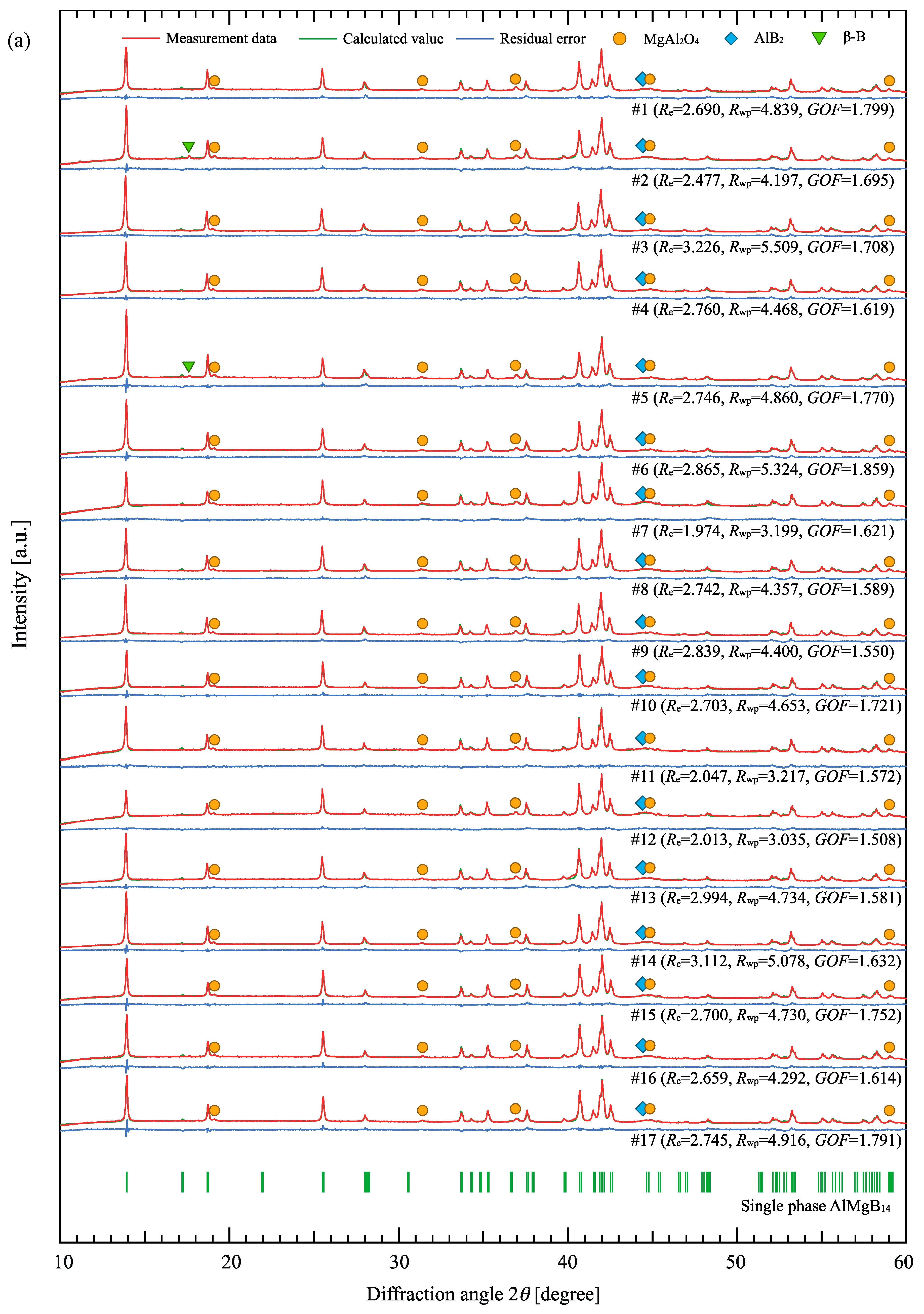
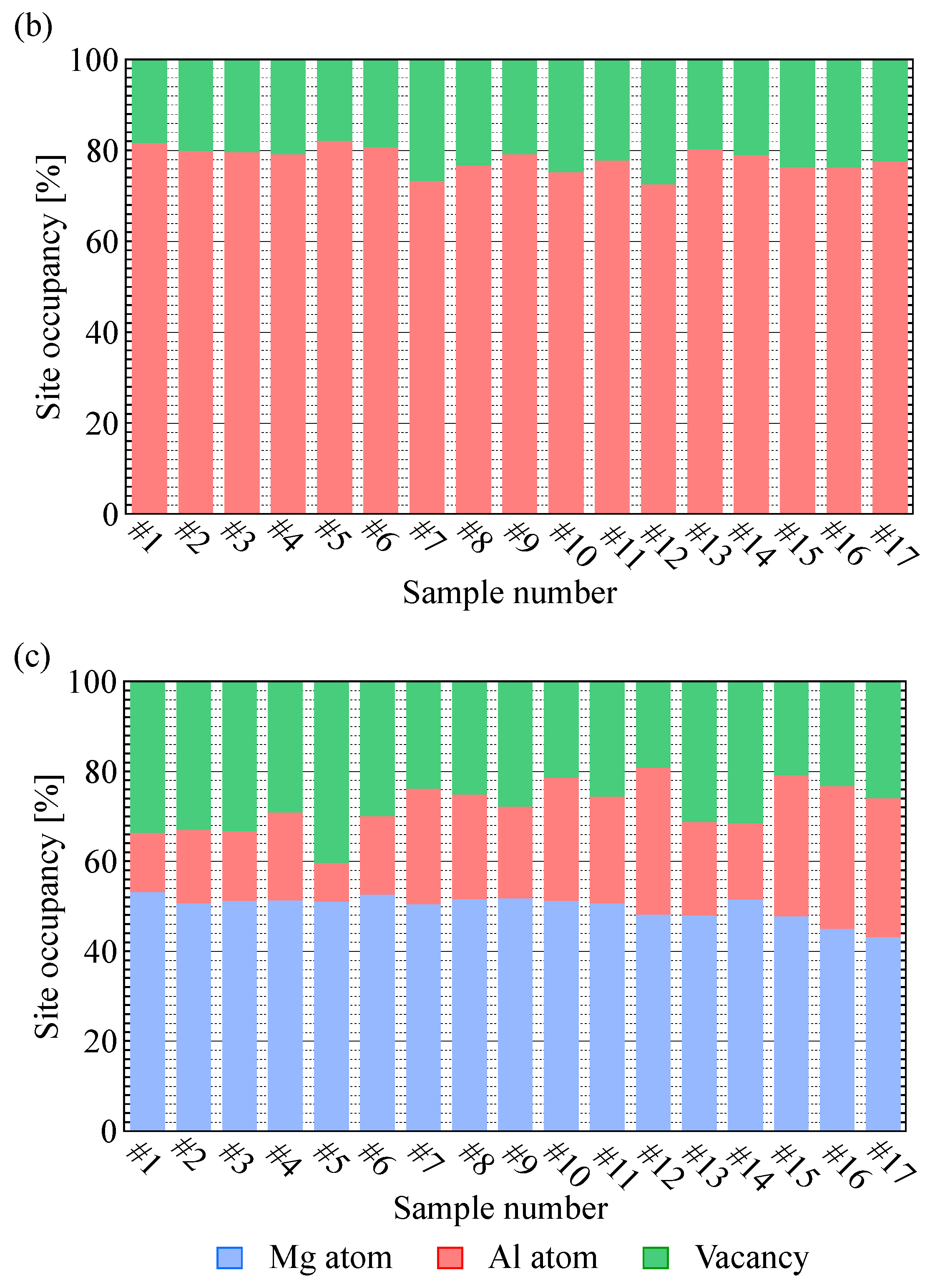
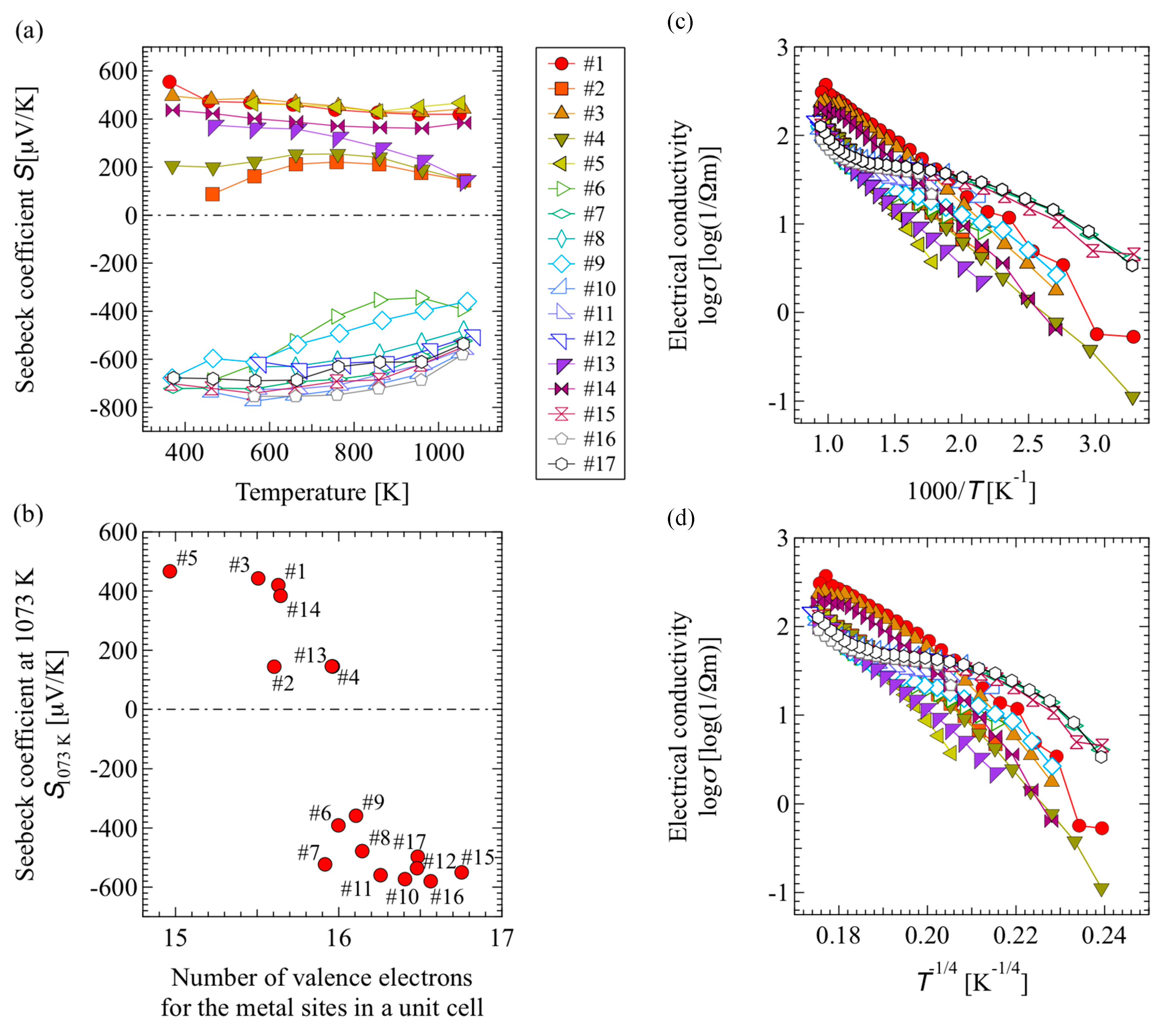
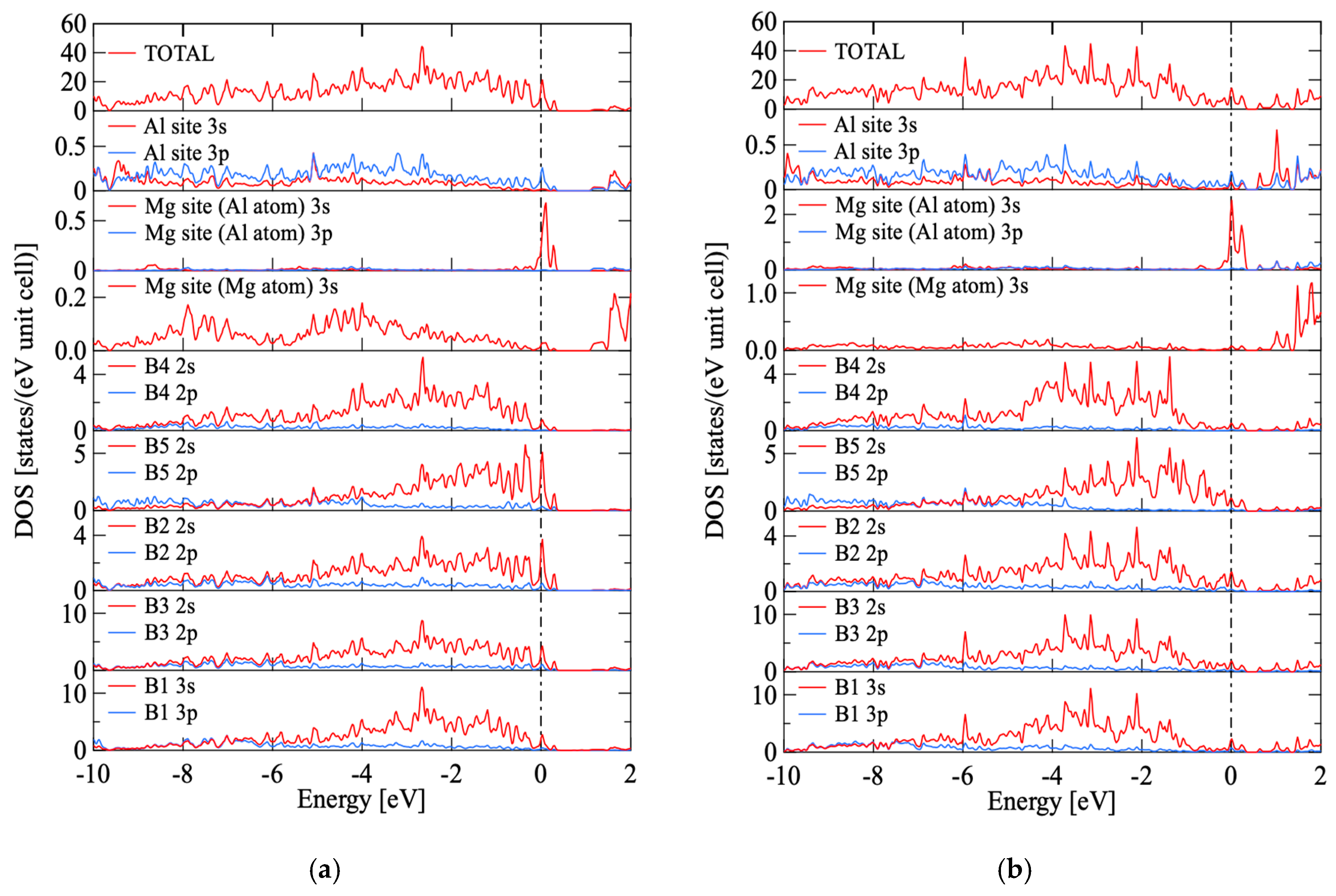
| Sample | Raw Material Mixture Ration [Molar Ration] | ||||
|---|---|---|---|---|---|
| Number | Al | : | Mg | : | B |
| #1 | 0.98 | : | 1.06 | : | 14 |
| #2 | 1.00 | : | 0.99 | : | 14 |
| #3 | 1.01 | : | 1.02 | : | 14 |
| #4 | 1.01 | : | 1.11 | : | 14 |
| #5 | 1.02 | : | 0.92 | : | 14 |
| #6 | 1.02 | : | 0.96 | : | 14 |
| #7 | 1.02 | : | 1.01 | : | 14 |
| #8 | 1.02 | : | 1.06 | : | 14 |
| #9 | 1.04 | : | 1.04 | : | 14 |
| #10 | 1.04 | : | 1.12 | : | 14 |
| #11 | 1.04 | : | 1.16 | : | 14 |
| #12 | 1.10 | : | 1.06 | : | 14 |
| #13 | 1.12 | : | 0.99 | : | 14 |
| #14 | 1.12 | : | 1.11 | : | 14 |
| #15 | 1.14 | : | 1.06 | : | 14 |
| #16 | 1.18 | : | 1.06 | : | 14 |
| #17 | 1.22 | : | 1.06 | : | 14 |
| (a) | |||||||
| #5 | Lattice Constant [Å] | a | 5.85063 | ||||
| b | 10.32511 | ||||||
| c | 8.11847 | ||||||
| Crystal Structure Parameter | Site | Fractional Coordinate | Atom | Occupancy [%] | |||
| x | y | z | |||||
| B1 | 0.15985 | 0.56183 | 0.16043 | B | 100.00 | ||
| B2 | 0 | 0.08655 | 0.17090 | B | 100.00 | ||
| B3 | 0.25153 | 0.07959 | 0.04495 | B | 100.00 | ||
| B4 | 0 | 0.66767 | 0.01982 | B | 100.00 | ||
| B5 | 0 | 0.15787 | 0.37898 | B | 100.00 | ||
| Al | 1/4 | 1/4 | 1/4 | Al | 82.12 | ||
| Mg | 0 | 1/4 | 0.64651 | Mg | 51.07 | ||
| Al | 8.54 | ||||||
| (b) | |||||||
| #15 | Lattice Constant [Å] | a | 5.85399 | ||||
| b | 10.30819 | ||||||
| c | 8.11090 | ||||||
| Crystal Structure Parameter | Site | Fractional Coordinate | Atom | Occupancy [%] | |||
| x | y | z | |||||
| B1 | 0.16209 | 0.56242 | 0.16089 | B | 100.00 | ||
| B2 | 0 | 0.08605 | 0.17424 | B | 100.00 | ||
| B3 | 0.24768 | 0.07860 | 0.04583 | B | 100.00 | ||
| B4 | 0 | 0.66614 | 0.01407 | B | 100.00 | ||
| B5 | 0 | 0.15344 | 0.38171 | B | 100.00 | ||
| Al | 1/4 | 1/4 | 1/4 | Al | 76.37 | ||
| Mg | 0 | 1/4 | 0.63967 | Mg | 47.77 | ||
| Al | 31.39 | ||||||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fujima, T.; Shimizu, N.; Arimatsu, H. p-n Control of AlMgB14-Based Thermoelectric Materials by Metal Site Occupancy. Materials 2019, 12, 632. https://doi.org/10.3390/ma12040632
Fujima T, Shimizu N, Arimatsu H. p-n Control of AlMgB14-Based Thermoelectric Materials by Metal Site Occupancy. Materials. 2019; 12(4):632. https://doi.org/10.3390/ma12040632
Chicago/Turabian StyleFujima, Takuya, Natsuki Shimizu, and Hideki Arimatsu. 2019. "p-n Control of AlMgB14-Based Thermoelectric Materials by Metal Site Occupancy" Materials 12, no. 4: 632. https://doi.org/10.3390/ma12040632
APA StyleFujima, T., Shimizu, N., & Arimatsu, H. (2019). p-n Control of AlMgB14-Based Thermoelectric Materials by Metal Site Occupancy. Materials, 12(4), 632. https://doi.org/10.3390/ma12040632





