Hot Deformation Behavior of a New Al–Mn–Sc Alloy
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
3.1. Microstructure of As-Cast Al–Mn–Sc Alloy
3.2. Hot Deformation Behavior
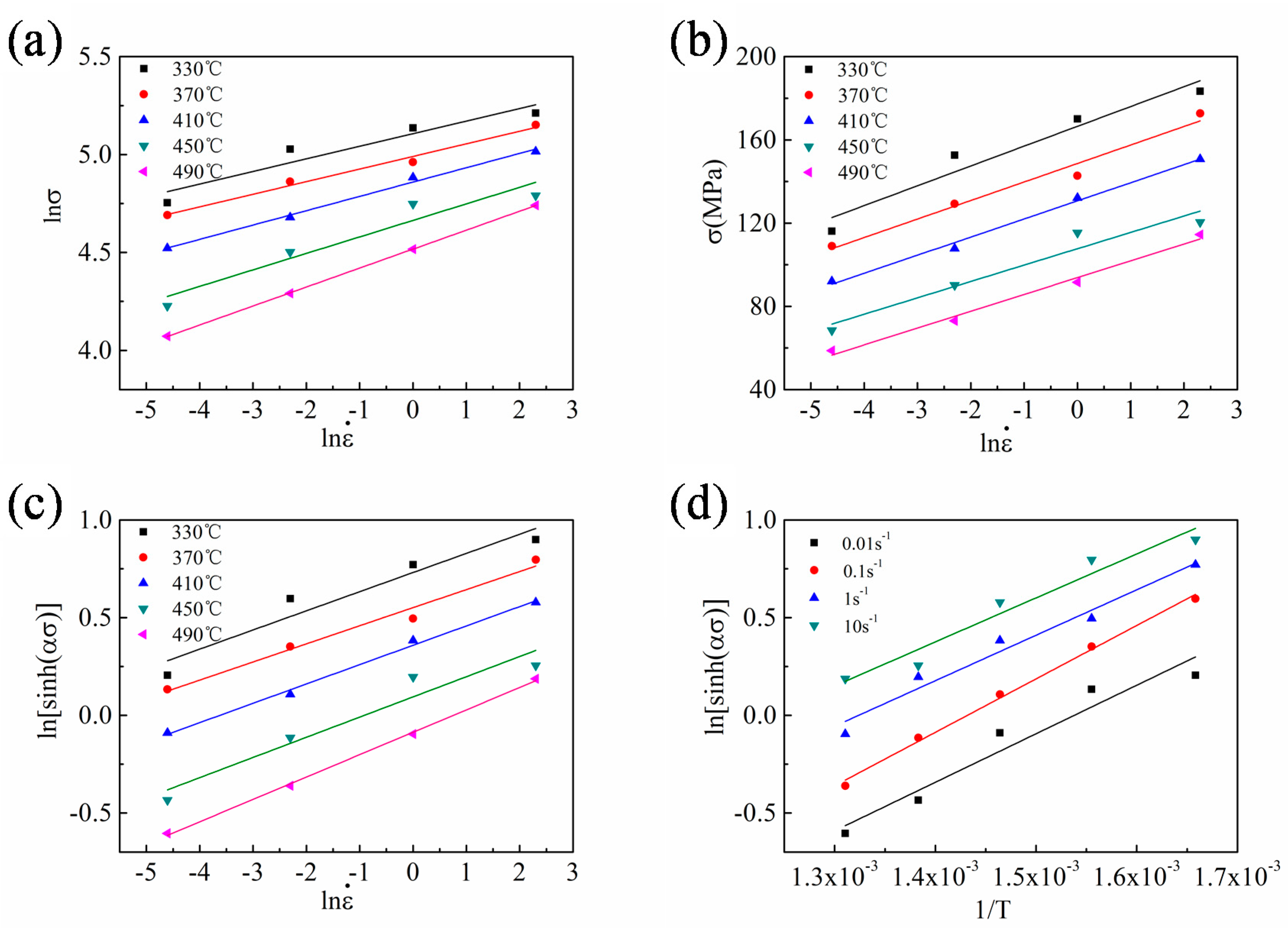
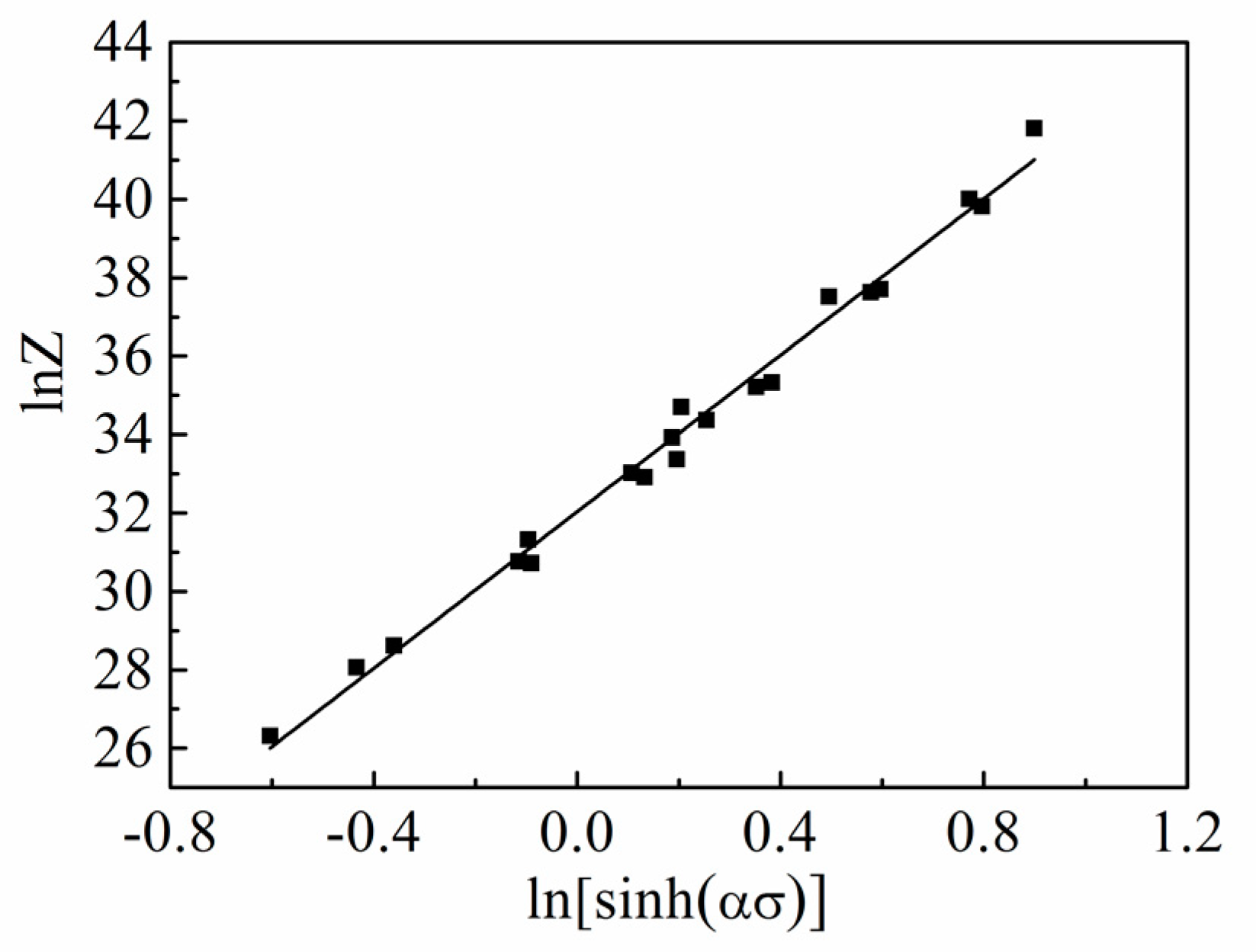
3.3. Constitutive Equations
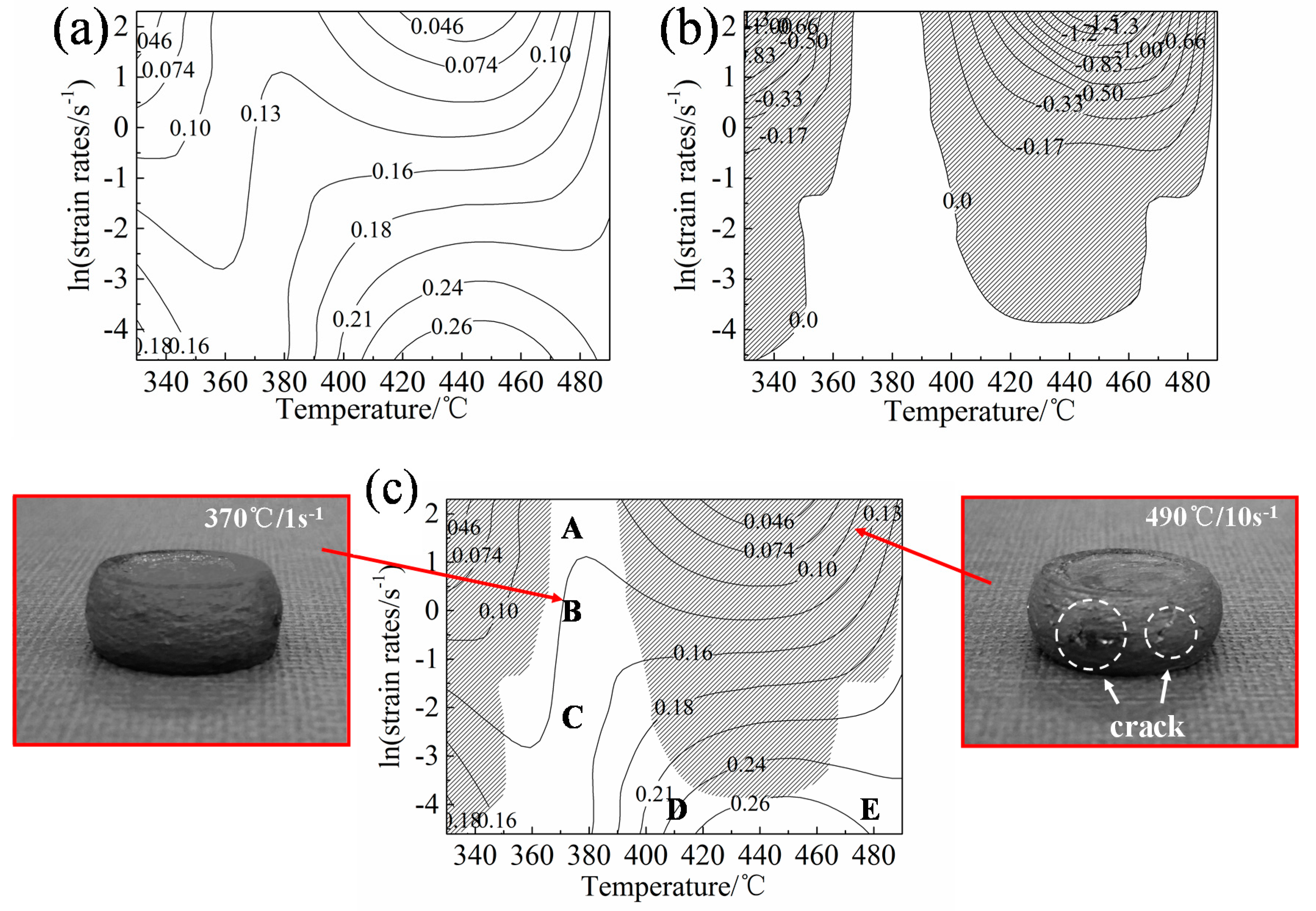
3.4. Processing Map
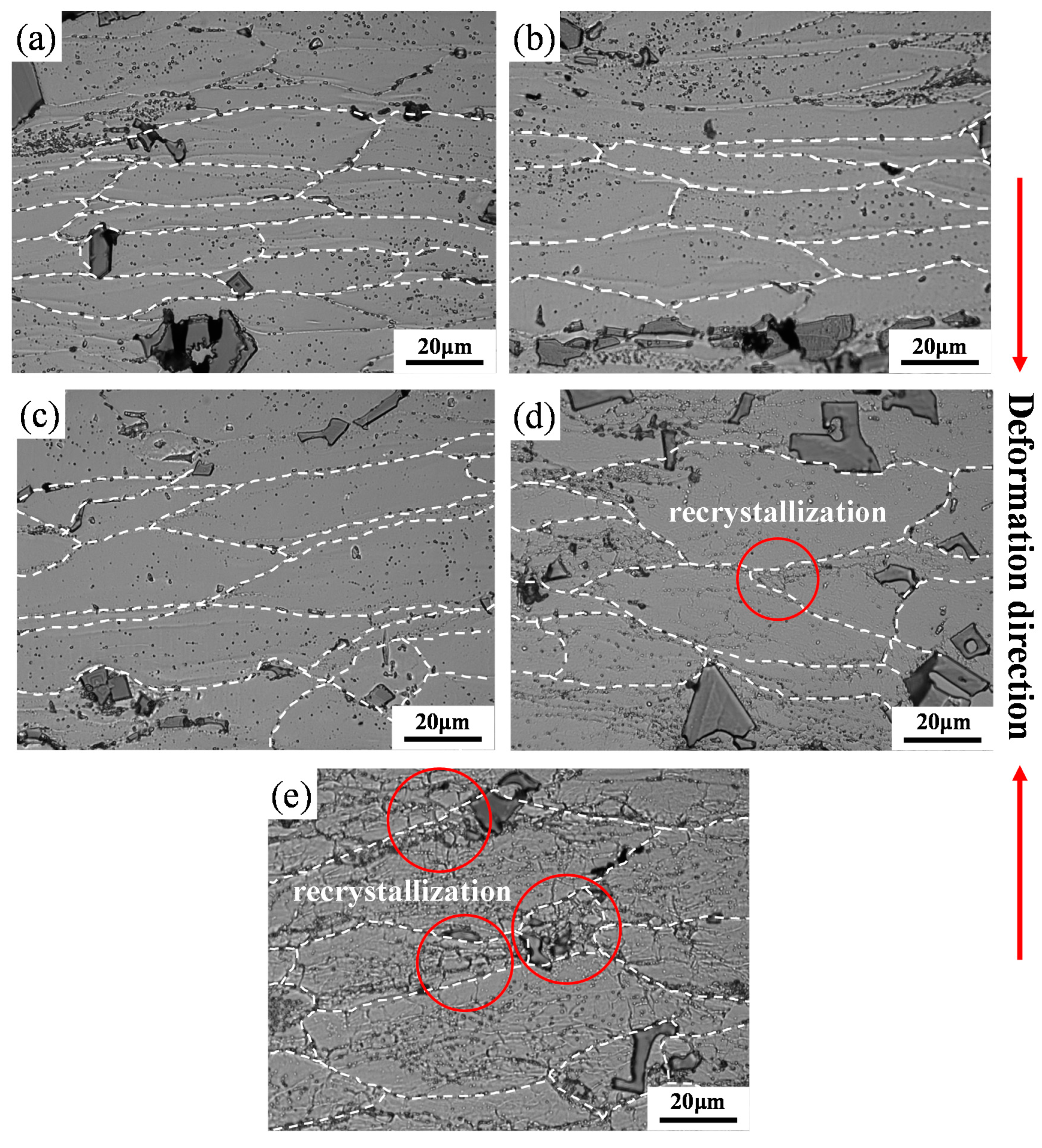
3.5. Microstructure Analysis
4. Conclusions
- (1)
- The dynamic softening is sufficient at higher temperatures and lower strain rates. In general, the flow stress decreases with increasing deformation temperatures and decreasing strain rates. The deformation behavior satisfies the hyperbolic sinusoidal constitutive law with an activation energy Q at 200.581 kJ·mol−1 and a structural factor A at 8.171 × 1013.
- (2)
- On the basis of DMM, a hot processing map is established at a 0.4 strain. The optimal processing range is between 420–475 °C and 0.01–0.022 s−1, in which a stable flow and a high power dissipation are achieved.
- (3)
- In hot deformation, a high lnZ (low deformation temperatures and high strain rates) suggests a dynamic recovery dominated mechanism and a low lnZ indicates a dynamic recrystallization dominated mechanism.
Author Contributions
Funding
Conflicts of Interest
References
- Pantelakis, S.G.; Alexopoulos, N.D. Assessment of the ability of conventional and advanced wrought aluminum alloys for mechanical performance in light-weight applications. Mater. Des. 2008, 29, 80–91. [Google Scholar] [CrossRef]
- Mostafaei, M.A.; Kazeminezhad, M. Hot deformation behavior of hot extruded Al-6Mg alloy. Mater. Sci. Eng. A 2012, 535, 216–221. [Google Scholar] [CrossRef]
- Zhang, J.; Chen, B.Q.; Zhang, B.X. Effect of initial microstructure on the hot compression deformation behavior of a 2219 aluminum alloy. Mater. Des. 2012, 34, 15–21. [Google Scholar] [CrossRef]
- Chamanfar, A.; Alamoudi, M.T.; Nanninga, N.E.; Misiolek, W.Z. Analysis of flow stress and microstructure during hot compression of 6099 aluminum alloy (AA6099). Mater. Sci. Eng. A 2019, 743, 684–696. [Google Scholar] [CrossRef]
- Mandal, P.K.; Anant, R.; Kumar, R.; Muthaiah, V.M.R. Effect of scandium on ageing kinetics in cast Al-Zn-Mg alloys. Mater. Sci. Eng. A 2017, 696, 257–266. [Google Scholar] [CrossRef]
- Wang, H.S.; Jiang, B.; Zhang, J.Y.; Wang, N.H.; Yi, D.Q.; Wang, B.; Liu, H.Q. The precipitation behavior and mechanical properties of cast Al-4.5Cu-3.5Zn-0.5Mg alloy. J. Alloys Compd. 2018, 768, 707–713. [Google Scholar] [CrossRef]
- Jia, Q.B.; Rometsch, P.; Kürnsteiner, P.; Chao, Q.; Huang, A.J.; Weyland, M.; Bourgeois, L.; Wu, X.H. Selective laser melting of a high strength Al-Mn-Sc alloy: Alloy design and strengthening mechanisms. Acta Mater. 2019, 171, 108–118. [Google Scholar] [CrossRef]
- Deng, Y.; Yin, Z.M.; Huang, J.W. Hot deformation behavior and microstructural evolution of homogenized 7050 aluminum alloy during compression at elevated temperature. Mater. Sci. Eng. A 2011, 528, 1780–1786. [Google Scholar] [CrossRef]
- Murty, S.V.S.N.; Sarkar, A.; Narayanan, P.R.; Venkitakrishnan, P.V.; Mukhopadhyay, J. Microstructure and micro-texture evolution during large strain deformation of aluminium alloy AA 2219. Mater. Sci. Eng. A 2016, 677, 41–49. [Google Scholar] [CrossRef]
- Son, K.T.; Kim, M.H.; Kim, S.W.; Lee, J.W.; Hyun, S.K. Evaluation of hot deformation characteristics in modified AA5052 using processing map and activation energy map under deformation heating. J. Alloys Compd. 2018, 740, 96–108. [Google Scholar] [CrossRef]
- Askariani, S.A.; Mohammad, S.; Pishbin, H. Hot deformation behavior of Mg-4Li-1Al alloy via hot compression tests. J. Alloys Compd. 2016, 688, 1058–1065. [Google Scholar] [CrossRef]
- Lu, S.H.; Wu, D.; Chen, R.S.; Han, E. The effect of twinning on dynamic recrystallization behavior of Mg-Gd-Y alloy during hot compression. J. Alloys Compd. 2019, 803, 277–290. [Google Scholar] [CrossRef]
- Liu, G.; Xie, W.; Hadadzadeh, A.; Wei, G.B.; Ma, Z.D. Hot deformation behavior and processing map of a superlight dual- phase Mg-Li alloy. J. Alloys Compd. 2018, 766, 460–469. [Google Scholar] [CrossRef]
- Lin, X.J.; Dong, F.Y.; Zhang, Y.; Yuan, X.G.; Huang, H.J.; Zheng, B.W.; Wang, L.; Wang, X.; Luo, L.S.; Su, Y.Q.; et al. Hot-deformation behaviour and hot-processing map of melt-hydrogenated Ti–6Al–4V/(TiB+TiC). Int. J. Hydrogen Energy 2019, 44, 8641–8649. [Google Scholar] [CrossRef]
- Jha, J.S.; Toppo, S.P.; Singh, R.; Tewari, A.; Mishra, S.K. Flow stress constitutive relationship between lamellar and equiaxed microstructure during hot deformation of Ti-6Al-4V. J. Mater. Process. Technol. 2019, 270, 216–227. [Google Scholar] [CrossRef]
- Long, S.; Xia, Y.F.; Hu, J.C.; Zhang, J.S.; Zhou, J.; Zhang, P.; Cui, M.L. Hot deformation behavior and microstructure evolution of Ti-6Cr-5Mo-5V-4Al alloy during hot compression. Vacuum 2019, 160, 171–180. [Google Scholar] [CrossRef]
- Ren, F.C.; Chen, F.; Chen, J.; Tang, X.Y. Hot deformation behavior and processing maps of AISI 420 martensitic stainless steel. J. Manuf. Process. 2018, 31, 640–649. [Google Scholar] [CrossRef]
- Wu, Z.Q.; Tang, Y.B.; Chen, W.; Lu, L.W.; Li, E.; Li, Z.C.; Ding, H. Exploring the in fluence of Al content on the hot deformation behavior of Fe- Mn-Al-C steels through 3D processing map. Vacuum 2019, 159, 447–455. [Google Scholar] [CrossRef] [Green Version]
- Yang, P.R.; Cai, M.H.; Wu, C.F.; Su, J.H.; Guo, X.P. Strain-rate dependent hot deformation behavior and mechanism of interphase- precipitated Ti-Mo-xNb steels: Physical modeling and characterization. Mater. Sci. Eng. A 2018, 729, 230–240. [Google Scholar] [CrossRef]
- Røyset, J.; Ryum, N. Scandium in aluminum alloys. J. Int. Mater. Rev. 2005, 50, 19–44. [Google Scholar] [CrossRef]
- Forbord, B.; Lefebvre, W.; Danoix, F.; Hallem, H.; Marthinsen, K. Three dimensional atom probe investigation on the formation of Al3(Sc,Zr)-dispersoids in aluminium alloys. Scr. Mater. 2004, 51, 333–337. [Google Scholar] [CrossRef] [Green Version]
- Kreiner, G.; Franzen, H.F. The crystal structure of λ-Al4Mn. J. Alloys Compd. 1997, 261, 83–104. [Google Scholar] [CrossRef]
- Jia, Q.B.; Rometsch, P.; Cao, S.; Zhang, K.; Huang, A.J.; Wu, X.H. Characterisation of AlScZr and AlErZr alloys processed by rapid laser melting. Scr. Mater. 2018, 151, 42–46. [Google Scholar] [CrossRef]
- Niu, J.T. Physical Simulation Technology in Materials and Thermal Processing; National Defence Industry Press: Beijing, China, 1999; p. 32. [Google Scholar]
- Huang, H.F.; Jiang, F.; Zhou, J.; Wei, L.L.; Zhong, M.C.; Liu, X.T. Hot deformation behavior and microstructural evolution of as-homogenized Al-6Mg-0.4Mn-0.25Sc-0.1Zr alloy during compression at elevated temperature. J. Alloys Compd. 2015, 644, 862–872. [Google Scholar] [CrossRef]
- Sun, Y.; Cao, Z.H.; Wan, Z.P.; Hu, L.X.; Ye, W.H.; Li, N.K.; Fan, C.L. 3D processing map and hot deformation behavior of 6A02 aluminum alloy. J. Alloys Compd. 2018, 742, 356–368. [Google Scholar] [CrossRef]
- Mirzadeh, H.; Cabrera, J.M.; Najafizadeh, A. Constitutive relationships for hot deformation of austenite. Acta Mater. 2011, 59, 6441–6448. [Google Scholar] [CrossRef]
- Sellars, C.M.; McTegart, W.J. On the mechanism of hot deformation. Acta Metal. 1966, 9, 1136–1138. [Google Scholar] [CrossRef]
- Zener, C.; Hollomon, J.H. Effect of strain rate upon plastic flow of steel. J. Appl. Phys. 1944, 15, 22–32. [Google Scholar] [CrossRef]
- Caillard, D.; Martin, J.L. Thermally Activated Mechanisms in Crystal Plasticity; M. Pergamon Press: Oxford, UK, 2003; pp. 1–280. [Google Scholar]
- Wang, S.; Hou, L.G.; Luo, J.R.; Zhang, J.S.; Zhuang, L.Z. Characterization of hot workability in AA 7050 aluminum alloy using activation energy and 3-D processing map. J. Mater. Process. Technol. 2015, 225, 110–121. [Google Scholar] [CrossRef]
- Li, Z.; Chen, Y.B.; Wei, S.Z.; Xiao, F.N.; Siyal, S.H.; Xu, L.J. Flow behavior and processing map for hot deformation of W-1.5ZrO2 alloy. J. Alloys Compd. 2019, 802, 118–128. [Google Scholar] [CrossRef]
- Liu, Y.; Geng, C.; Lin, Q.Q.; Xiao, Y.F.; Xu, J.R.; Kang, W. Study on hot deformation behavior and intrinsic workability of 6063 aluminum alloys using 3D processing map. J. Alloys Compd. 2017, 713, 212–221. [Google Scholar] [CrossRef]
- Prasad, Y.V.R.K.; Seshacharyulu, T. Modelling of hot deformation for microstructural control. Metall. Rev. 1998, 43, 243–258. [Google Scholar] [CrossRef]
- Prasad, Y. Author’s reply: Dynamic materials model: Basis and principles. Metall. Mater. Trans. A 1996, 27, 235–236. [Google Scholar] [CrossRef]
- He, H.L.; Yi, H.L.; Cui, J.D.; Huang, S.Q. Hot deformation characteristics and processing parameter optimization of 2219 Al alloy using constitutive equation and processing map. Vacuum 2019, 160, 293–302. [Google Scholar] [CrossRef]
- Jeong, H.T.; Han, S.H.; Kim, W.J. Effects of large amounts of Mg (5–13 wt%) on hot compressive deformation behavior and processing maps of Al-Mg alloys. J. Alloys Compd. 2019, 788, 1282–1299. [Google Scholar] [CrossRef]
- Maizza, G.; Pero, R.; Richetta, M.; Montanari, R. Continuous dynamic recrystallization (CDRX) model for aluminum alloys. J. Mater. Sci. 2018, 53, 4563–4573. [Google Scholar] [CrossRef]
- Wang, K.K.; Li, X.P.; Li, Q.L.; Shu, G.G.; Tang, G.Y. Hot deformation behavior and microstructural evolution of particulate-reinforced AA6061/B4C composite during compression at elevated temperature. Mater. Sci. Eng. A 2017, 696, 248–256. [Google Scholar] [CrossRef]
- Mazurina, I.; Sakai, T.; Miura, H.; Sitdikov, O.; Kaibyshev, R. Grain refinement in aluminum alloy 2219 during ECAP at 250 °C. Mater. Sci. Eng. A 2008, 473, 297–305. [Google Scholar] [CrossRef]
- He, G.A.; Liu, F.; Si, J.Y.; Yang, C.; Jiang, L. Characterization of hot compression behavior of a new HIPed nickel-based P/M superalloy using processing maps. Mater. Des. 2015, 87, 256–265. [Google Scholar] [CrossRef]
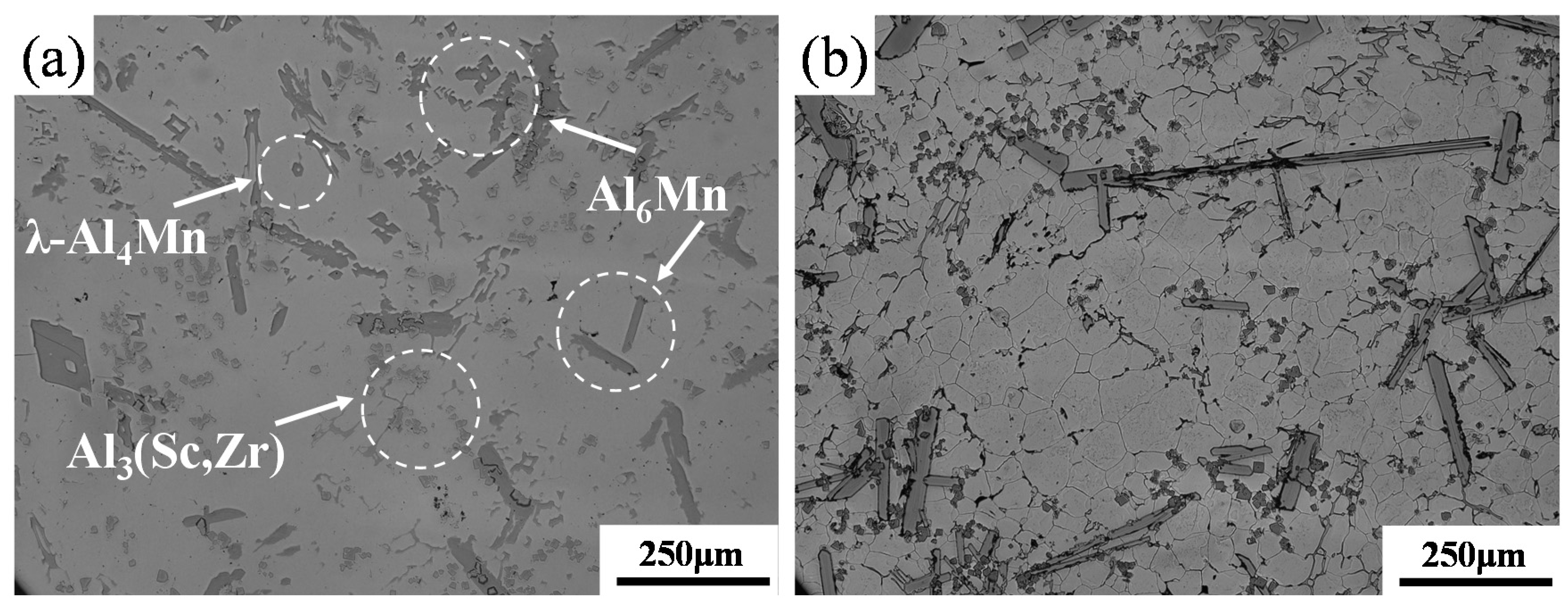
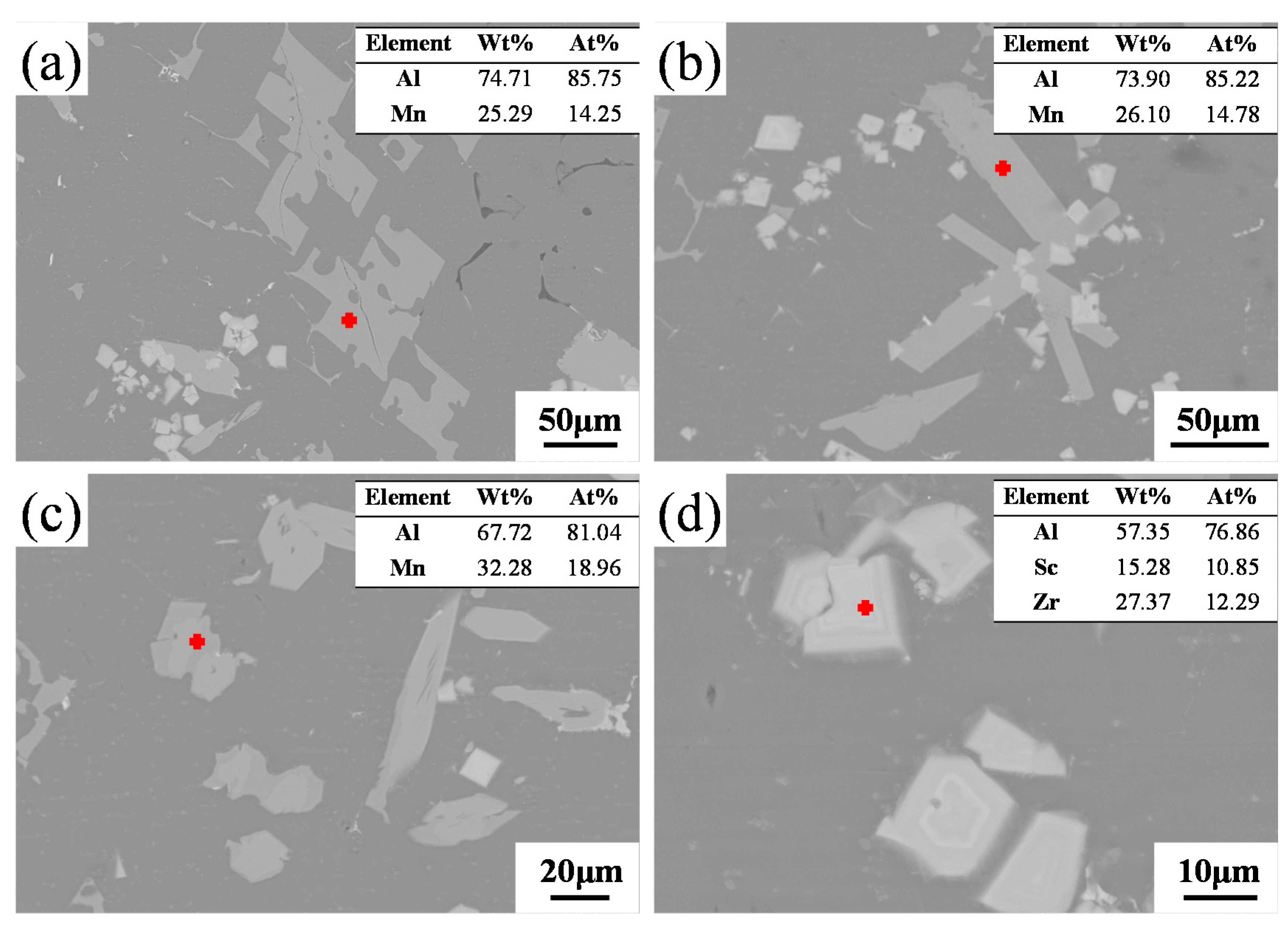

| Mn | Mg | Sc | Zr | Si | Fe | Al |
|---|---|---|---|---|---|---|
| 4.3–4.7 | 1.4–1.6 | 0.65–0.85 | 0.7–0.8 | <0.1 | <0.1 | Bal |
| Strain Rate/s−1 | Temperature/°C | ||||
|---|---|---|---|---|---|
| 330 | 370 | 410 | 450 | 490 | |
| 0.01 | 116 | 110 | 92 | 68 | 59 |
| 0.1 | 153 | 129 | 108 | 90 | 73 |
| 1 | 170 | 143 | 132 | 115 | 92 |
| 10 | 183 | 173 | 151 | 120 | 115 |
| Strain Rate/s−1 | Temperature/°C | ||||
|---|---|---|---|---|---|
| 330 | 370 | 410 | 450 | 490 | |
| 0.01 | 210.439 | 222.745 | 208.569 | 199.904 | 180.28 |
| 0.1 | 231.688 | 245.236 | 229.629 | 220.089 | 198.486 |
| 1 | 197.168 | 208.697 | 195.415 | 187.297 | 168.913 |
| 10 | 190.941 | 202.106 | 189.244 | 181.382 | 163.578 |
| Regions in PM | Temperature/°C | Strain Rate/s−1 | LnZ |
|---|---|---|---|
| A | 370 | 10 | 39.823 |
| B | 370 | 1 | 37.520 |
| C | 370 | 0.1 | 35.217 |
| D | 410 | 0.01 | 30.718 |
| E | 490 | 0.01 | 26.317 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kang, W.; Yang, Y.; Cao, S.; Li, L.; Xin, S.; Wang, H.; Cao, Z.; Liang, E.; Zhang, X.; Huang, A. Hot Deformation Behavior of a New Al–Mn–Sc Alloy. Materials 2020, 13, 22. https://doi.org/10.3390/ma13010022
Kang W, Yang Y, Cao S, Li L, Xin S, Wang H, Cao Z, Liang E, Zhang X, Huang A. Hot Deformation Behavior of a New Al–Mn–Sc Alloy. Materials. 2020; 13(1):22. https://doi.org/10.3390/ma13010022
Chicago/Turabian StyleKang, Weiqi, Yi Yang, Sheng Cao, Lei Li, Shewei Xin, Hao Wang, Zhiqiang Cao, Enquan Liang, Xi Zhang, and Aijun Huang. 2020. "Hot Deformation Behavior of a New Al–Mn–Sc Alloy" Materials 13, no. 1: 22. https://doi.org/10.3390/ma13010022
APA StyleKang, W., Yang, Y., Cao, S., Li, L., Xin, S., Wang, H., Cao, Z., Liang, E., Zhang, X., & Huang, A. (2020). Hot Deformation Behavior of a New Al–Mn–Sc Alloy. Materials, 13(1), 22. https://doi.org/10.3390/ma13010022






