Reliability and Lifetime Assessment of Glider Wing’s Composite Spar through Accelerated Fatigue Life Testing
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
3.1. Static Mechanical Proprieties
3.2. Accelerated Fatigue Life Testing of the GFRP-Foam Specimens
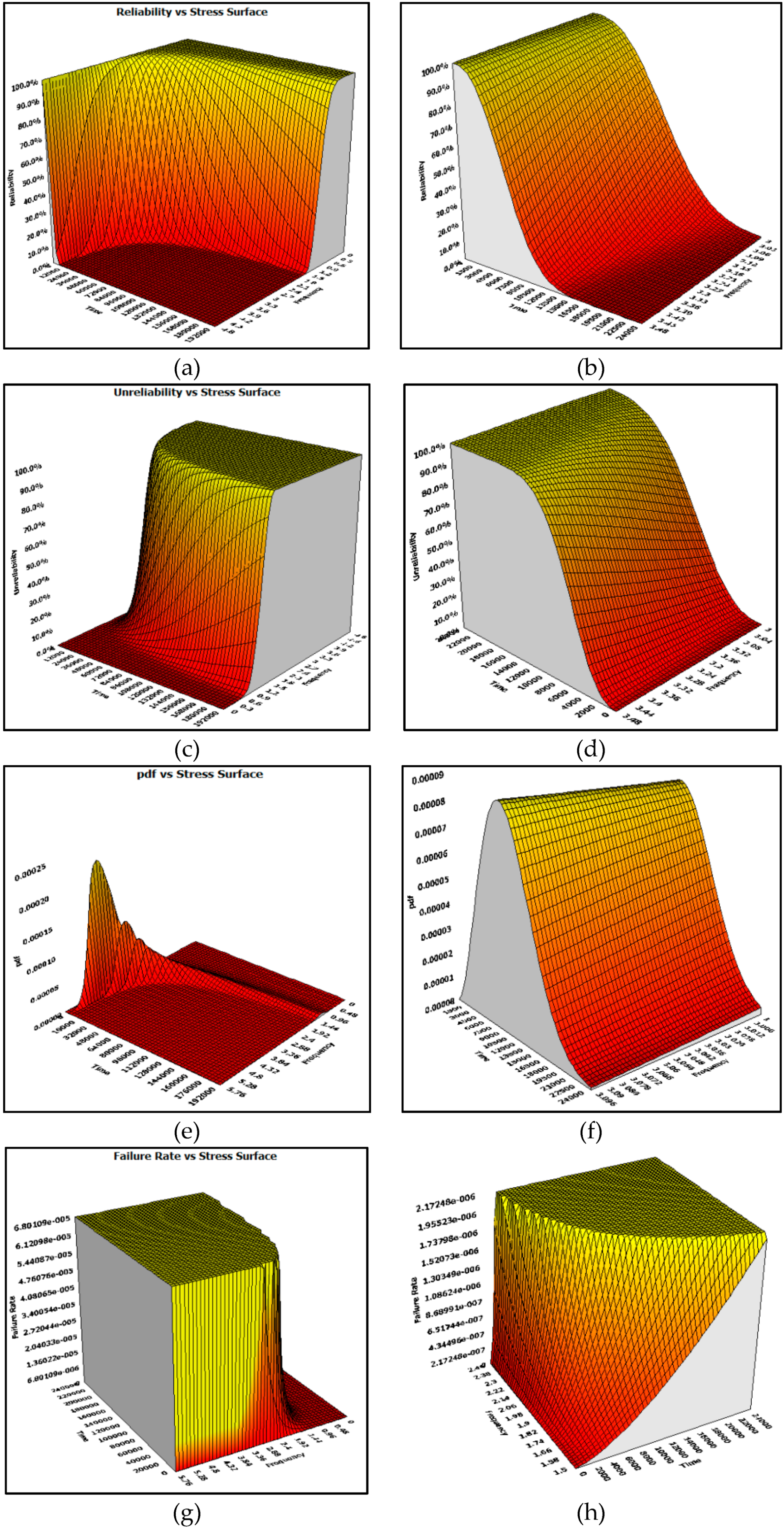
3.3. Reliability Analysis of Accelerated Life-Test Data
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Zaharia, S.M. Reliability, Maintenance and Testing of Aerospace Systems; Lambert Academic Publishing: Riga, Latvia, 2019; pp. 25–26. [Google Scholar]
- Nelson, W. Accelerated Testing: Statistical Models, Test Plans, and Data Analysis; John Wiley & Sons, Inc.: New York, NY, USA, 2004; pp. 15–17. [Google Scholar]
- Sha, N. Statistical Inference for Progressive Stress Accelerated Life Testing with Birnbaum-Saunders Distribution. Stats 2018, 1, 189–203. [Google Scholar] [CrossRef] [Green Version]
- Kim, S.K.; Lim, H.; Sung, S.-I.; Kim, Y.S. Planning of Partially Accelerated Degradation Test with Two Stress Variables for Practical Lifetime Estimation. Appl. Sci. 2018, 8, 2162. [Google Scholar] [CrossRef] [Green Version]
- Hu, C.H.; Plante, R.D.; Tang, J. Statistical equivalency and optimality of simple step-stress accelerated test plans for the exponential distribution. Nav. Res. Logist. 2013, 60, 19–30. [Google Scholar] [CrossRef]
- Escobar, L.A.; Meeker, W.Q. A Review of Accelerated Test Models. Stat. Sci. 2006, 21, 552–577. [Google Scholar] [CrossRef] [Green Version]
- Nakada, M.; Miyano, Y. Accelerated testing for long-term fatigue strength of various FRP laminates for marine use. Compos. Sci. Technol. 2009, 69, 805–813. [Google Scholar] [CrossRef]
- Chen, Y.; Davalos, J.F.; Ray, I.; Kim, H.Y. Accelerated aging tests for evaluations of durability performance of FRP reinforcing bars for concrete structures. Compos. Struct. 2007, 78, 101–111. [Google Scholar] [CrossRef]
- Zaharia, S.M.; Morariu, C.O.; Nedelcu, A.; Pop, M.A. Experimental Study of Static and Fatigue Behavior of CFRP-Balsa Sandwiches under Three-point Flexural Loading. BioResources 2017, 12, 2673–2689. [Google Scholar] [CrossRef] [Green Version]
- He, Q.; Chen, W.; Pan, J.; Qian, P. Improved step stress accelerated life testing method for electronic product. Microelectron. Reliab. 2012, 52, 2773–2780. [Google Scholar]
- Cai, M.; Yang, D.; Tian, K.; Zhang, P.; Chen, X.; Liu, L.; Zhang, G. Step-stress accelerated testing of high-power LED lamps based on subsystem isolation method. Microelectron. Reliab. 2015, 55, 1784–1789. [Google Scholar] [CrossRef]
- Cai, M.; Yang, D.; Zheng, J.; Mo, Y.; Huang, J.; Xu, J.; Chen, W.; Zhang, G.; Chen, X. Thermal degradation kinetics of LED lamps in step-up-stress and step-down-stress accelerated degradation testing. Appl. Eng. 2016, 107, 918–926. [Google Scholar] [CrossRef]
- Qian, C.; Fan, J.; Fang, J.; Yu, C.; Ren, Y.; Fan, X.; Zhang, G. Photometric and Colorimetric Assessment of LED Chip Scale Packages by Using a Step-Stress Accelerated Degradation Test (SSADT) Method. Materials 2017, 10, 1181. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Tseng, S.T.; Wen, Z.C. Step-Stress Accelerated Degradation Analysis for Highly Reliable Products. J. Qual. Technol. 2000, 32, 209–216. [Google Scholar] [CrossRef]
- Coutu, R.A.; Lake, R.A.; Christiansen, B.D.; Heller, E.R.; Bozada, C.A.; Poling, B.S.; Via, G.D.; Theimer, J.P.; Tetlak, S.E.; Vetury, R.; et al. Benefits of Considering More than Temperature Acceleration for GaN HEMT Life Testing. Electronics 2016, 5, 32. [Google Scholar] [CrossRef] [Green Version]
- Yang, X.; Sun, B.; Wang, Z.; Qian, C.; Ren, Y.; Yang, D.; Feng, Q. An Alternative Lifetime Model for White Light Emitting Diodes under Thermal–Electrical Stresses. Materials 2018, 11, 817. [Google Scholar] [CrossRef] [Green Version]
- Hernández-López, A.M.; Aguilar-Garib, J.A.; Guillemet-Fritsch, S.; Nava-Quintero, R.; Dufour, P.; Tenailleau, C.; Durand, B.; Valdez-Nava, Z. Reliability of X7R Multilayer Ceramic Capacitors During High Accelerated Life Testing (HALT). Materials 2018, 11, 1900. [Google Scholar] [CrossRef] [Green Version]
- Coro, A.; Abasolo, M.; Aguirrebeitia, J.; López de Lacalle, L.N. Inspection scheduling based on reliability updating of gas turbine welded structures. Adv. Mech. Eng. 2019, 11, 1–20. [Google Scholar] [CrossRef] [Green Version]
- Coro, A.; Macareno, L.M.; Aguirrebeitia, J.; López de Lacalle, L.N. A Methodology to Evaluate the Reliability Impact of the Replacement of Welded Components by Additive Manufacturing Spare Parts. Metals 2019, 9, 932. [Google Scholar] [CrossRef] [Green Version]
- Wang, L.; Li, Y. Boundary for aviation bearing accelerated life test based on quasi-dynamic analysis. Tribol. Int. 2017, 116, 414–421. [Google Scholar] [CrossRef]
- Lok, S.; Paul, J.; Upendranath, V. Prescience life of landing gear using multiaxial fatigue numerical analysis. Procedia Eng. 2014, 86, 775–779. [Google Scholar] [CrossRef] [Green Version]
- Zhang, M.; Meng, Q.; Hu, W.; Shi, S.; Hu, M.; Zhang, X. Damage mechanics method for fatigue life prediction of PitchChange-Link. Int. J. Fatigue 2010, 32, 1683–1688. [Google Scholar] [CrossRef]
- Shahani, A.R.; Mohammadi, S. Damage tolerance approach for analyzing a helicopter main rotor blade. Eng. Fail. Anal. 2015, 57, 56–71. [Google Scholar] [CrossRef]
- Van der Ven, H.; Bakker, R.J.J.; van Tongeren, J.H.; Bos, M.J.; Münninghoff, N. A modelling framework for the calculation of structural loads for fatigue life prediction of helicopter airframe components. Aerosp. Sci. Technol. 2012, 23, 26–33. [Google Scholar] [CrossRef]
- Özsoy, S.; Çelik, M.; Kadıoğlu, F.S. An accelerated life test approach for aerospace structural components. Eng. Fail. Anal. 2008, 15, 946–957. [Google Scholar] [CrossRef]
- Zaharia, S.M. The methodology of fatigue lifetime prediction and validation based on accelerated reliability testing of the rotor pitch links. Eksploat. Niezawodn. 2019, 21, 638–644. [Google Scholar] [CrossRef]
- Zaharia, S.M.; Martinescu, I. Management of accelerated reliability testing. Teh. Vjesn. 2016, 23, 1447–1455. [Google Scholar]
- Zaharia, S.M.; Martinescu, I.; Morariu, C.O. Life time prediction using accelerated test data of the specimens from mechanical element. Eksploat. Niezawodn. 2012, 14, 99–106. [Google Scholar]
- Orkisz, M.; Święch, L.; Zacharzewski, J. Fatigue tests of motor glider wing’s composite spar. Eksploat. Niezawodn. 2012, 14, 228–232. [Google Scholar]
- Święch, Ł. Calibration of a Load Measurement System for an Unmanned Aircraft Composite Wing Based on Fibre Bragg Gratings and Electrical Strain Gauges. Aerospace 2020, 7, 27. [Google Scholar] [CrossRef] [Green Version]
- Petrašinović, N.; Petrašinović, D.; Rašuo, B.; Milković, D. Aircraft duraluminum wing spar fatigue testing. FME Trans. 2017, 45, 531–536. [Google Scholar] [CrossRef] [Green Version]
- Eldwaib, K.A.; Grbović, A.; Sedmak, A.; Kastratović, G.; Petrasinović, D.; Sedmak, S. Fatigue life estimation of damaged integral wing spar using XFEM. Teh. Vjesn. 2018, 25, 1837–1842. [Google Scholar]
- Grbović, A.; Rasuo, B. FEM based fatigue crack growth predictions for spar of light aircraft under variable amplitude loading. Eng. Fail. Anal. 2012, 26, 50–64. [Google Scholar]
- Rasuo, B.; Grbović, A.; Petrasinović, D. Investigation of fatigue life of 2024-T3 aluminum spar using Extended Finite Element Method (XFEM). SAE Int. J. Aerosp. 2013, 6, 408–416. [Google Scholar] [CrossRef]
- IAR Ghimbav. Composite Glider Flight Manual; IAR Ghimbav: Brașov, Romania, 2000. [Google Scholar]
- Divinycell F. Available online: http://www.diabgroup.com/Products-and-services/Core-Material/Divinycell-F (accessed on 4 April 2020).
- Interglas Technologies. Available online: https://www.pd-interglas.com/en/index.php (accessed on 4 April 2020).
- Miyano, Y.; Nakada, M.; Nishigaki, K. Prediction of long-term fatigue life of quasi-isotropic CFRP laminates for aircraft use. Int. J. Fatigue 2006, 28, 1217–1225. [Google Scholar] [CrossRef]
- Miyano, Y.; Nakada, M.; Ichimura, J.; Hayakawa, E. Accelerated testing for long-term strength of innovative CFRP laminates for marine use. Compos. Part B Eng. 2008, 39, 5–12. [Google Scholar] [CrossRef]
- Rajaneesh, A.; Satrio, W.; Chai, G.B.; Sridhar, I. Long-term life prediction of woven CFRP laminates under three point flexural fatigue. Compos. Part B Eng. 2016, 91, 539–547. [Google Scholar] [CrossRef]
- ReliaSoft Corp. Accelerated Life Testing Reference eBook. Available online: http://reliawiki.org/index.php/ReliaWiki:Books/Accelerated_Life_Testing_Reference_eBook (accessed on 4 April 2020).
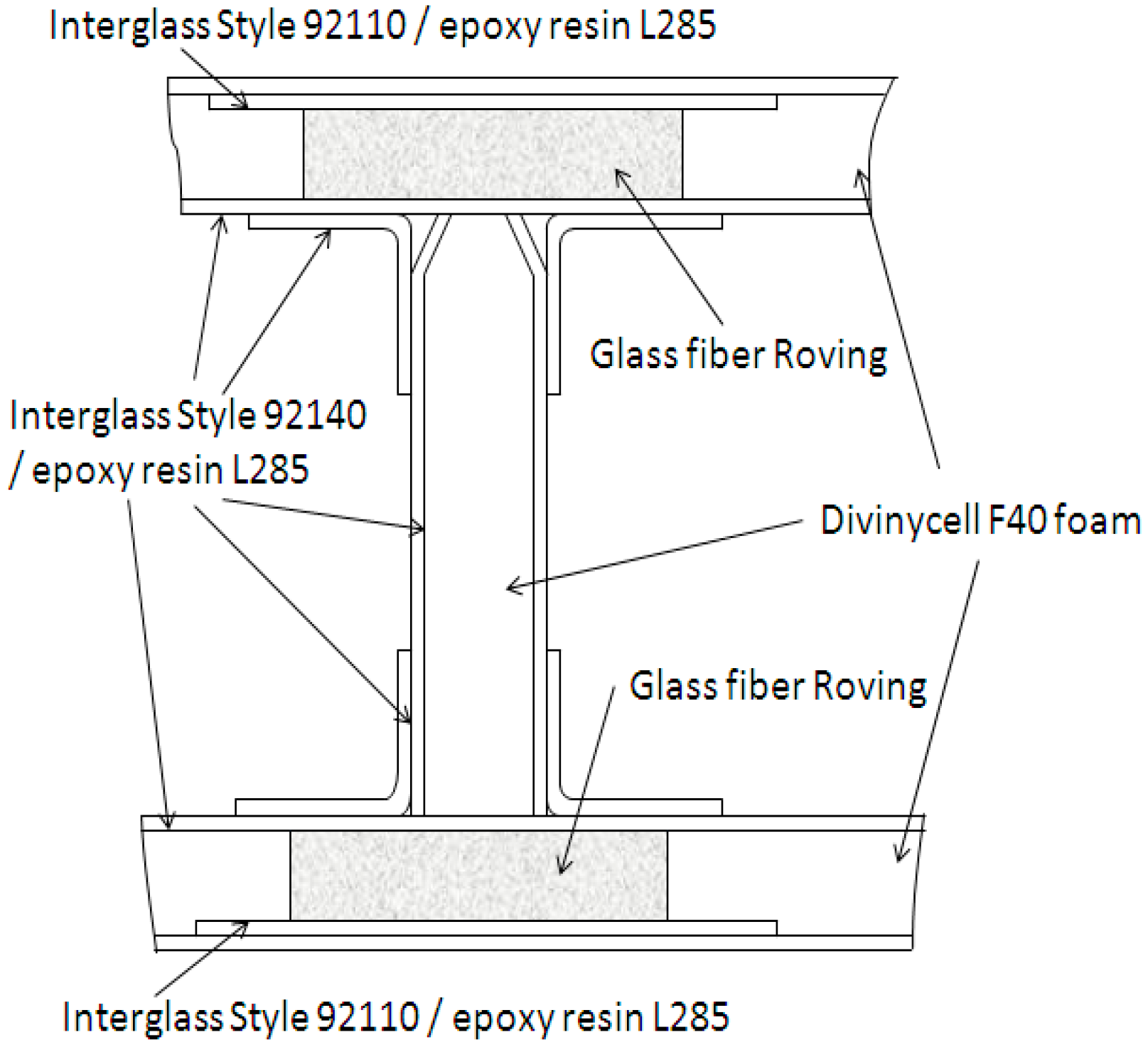







| Material | Length L (mm) | Thickness d (mm) | Width b (mm) | Span Length S (mm) | Core Thickness c (mm) | Facing Thickness t (mm) |
|---|---|---|---|---|---|---|
| GFRP-Foam | 150 | 10 | 15 | 110 | 9 | 0.5 |
| Material | Mean (μ) | Standard Deviation (s) | Coefficient of Variation (δ)% |
|---|---|---|---|
| GFRP-Foam—Bending Strength (MPa) | 26.5 | 3.3 | 12.452 |
| GFRP-Foam Bending Modulus (GPa) | 2.8 | 0.4 | 14.285 |
| Core shear ultimate strength (MPa) | 0.843 | 0.105 | 12.455 |
| Core shear yield stress (MPa) | 0.069 | 0.009 | 13.043 |
| Facing stress (MPa) | 92.8 | 11.6 | 12.5 |
| Specimen No. | The Number of Cycles to Failure in Accelerated Conditions | Accelerated Frequency Level (Hz) |
|---|---|---|
| 1 | 20,756 | 2 |
| 2 | 22,321 | 2 |
| 3 | 24,759 | 2 |
| 4 | 26,098 | 2 |
| 5 | 27,479 | 2 |
| 6 | 14,389 | 3 |
| 7 | 16,342 | 3 |
| 8 | 17,743 | 3 |
| 9 | 19,902 | 3 |
| 10 | 21,872 | 3 |
| 11 | 2763 | 4 |
| 12 | 3473 | 4 |
| 13 | 4093 | 4 |
| 14 | 5764 | 4 |
| 15 | 6034 | 4 |
| 16 | 1234 | 5 |
| 17 | 1456 | 5 |
| 18 | 1789 | 5 |
| 19 | 2021 | 5 |
| 20 | 2341 | 5 |
| Specimen No. | The Number of Cycles to Failure in Accelerated Testing Conditions | Accelerated Frequency Level (Hz) | Acceleration Factor | The Number of Cycles to Failure in Normal Testing Conditions |
|---|---|---|---|---|
| 1 | 20,756 | 2 | 2.504 | 51,973 |
| 2 | 22,321 | 55,892 | ||
| 3 | 24,759 | 61,997 | ||
| 4 | 26,098 | 65,349 | ||
| 5 | 27,479 | 68,807 | ||
| 6 | 14,389 | 3 | 9.133 | 131,415 |
| 7 | 16,342 | 149,251 | ||
| 8 | 17,743 | 162,047 | ||
| 9 | 19,902 | 181,765 | ||
| 10 | 21,872 | 199,757 | ||
| 11 | 2763 | 4 | 22.87 | 63,190 |
| 12 | 3473 | 79,428 | ||
| 13 | 4093 | 93,607 | ||
| 14 | 5764 | 131,823 | ||
| 15 | 6034 | 137,998 | ||
| 16 | 1234 | 5 | 46.614 | 57,522 |
| 17 | 1456 | 67,870 | ||
| 18 | 1789 | 83,392 | ||
| 19 | 2021 | 94,207 | ||
| 20 | 2341 | 109,123 |
| The Number of Cycles to Failure in Normal Testing Conditions | Reliability R (t) | Unreliability F (t) | Pdf f(t) × 10−6 | Failure Rate λ(t) × 10−6 |
|---|---|---|---|---|
| 51,973 | 0.965 | 0.035 | 5.67 | 21.7 |
| 55,892 | 0.917 | 0.083 | 6.15 | 23.8 |
| 57,522 | 0.868 | 0.132 | 6.35 | 24.7 |
| 61,997 | 0.819 | 0.181 | 6.85 | 27.2 |
| 63,190 | 0.770 | 0.230 | 6.98 | 27.9 |
| 65,349 | 0.721 | 0.279 | 7.20 | 29.1 |
| 67,870 | 0.672 | 0.328 | 7.44 | 30.6 |
| 68,807 | 0.622 | 0.378 | 7.52 | 31.2 |
| 79,428 | 0.573 | 0.427 | 8.29 | 37.8 |
| 83,392 | 0.524 | 0.476 | 8.48 | 40.4 |
| 93,607 | 0.475 | 0.525 | 8.70 | 47.4 |
| 94,207 | 0.426 | 0.574 | 8.70 | 47.9 |
| 109,123 | 0.377 | 0.623 | 8.32 | 59.0 |
| 131,415 | 0.327 | 0.673 | 6.61 | 77.5 |
| 131,823 | 0.278 | 0.722 | 6.57 | 77.9 |
| 137,998 | 0.229 | 0.771 | 5.96 | 83.5 |
| 149,251 | 0.180 | 0.82 | 4.80 | 94.0 |
| 162,047 | 0.131 | 0.869 | 3.53 | 106.7 |
| 181,765 | 0.082 | 0.918 | 1.95 | 127.8 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zaharia, S.M.; Pop, M.A.; Udroiu, R. Reliability and Lifetime Assessment of Glider Wing’s Composite Spar through Accelerated Fatigue Life Testing. Materials 2020, 13, 2310. https://doi.org/10.3390/ma13102310
Zaharia SM, Pop MA, Udroiu R. Reliability and Lifetime Assessment of Glider Wing’s Composite Spar through Accelerated Fatigue Life Testing. Materials. 2020; 13(10):2310. https://doi.org/10.3390/ma13102310
Chicago/Turabian StyleZaharia, Sebastian Marian, Mihai Alin Pop, and Răzvan Udroiu. 2020. "Reliability and Lifetime Assessment of Glider Wing’s Composite Spar through Accelerated Fatigue Life Testing" Materials 13, no. 10: 2310. https://doi.org/10.3390/ma13102310
APA StyleZaharia, S. M., Pop, M. A., & Udroiu, R. (2020). Reliability and Lifetime Assessment of Glider Wing’s Composite Spar through Accelerated Fatigue Life Testing. Materials, 13(10), 2310. https://doi.org/10.3390/ma13102310






