Axial Behavior of Reinforced UHPC-NSC Composite Column under Compression
Abstract
1. Introduction
2. Experiment
2.1. Specimen Design
2.2. Material Properties
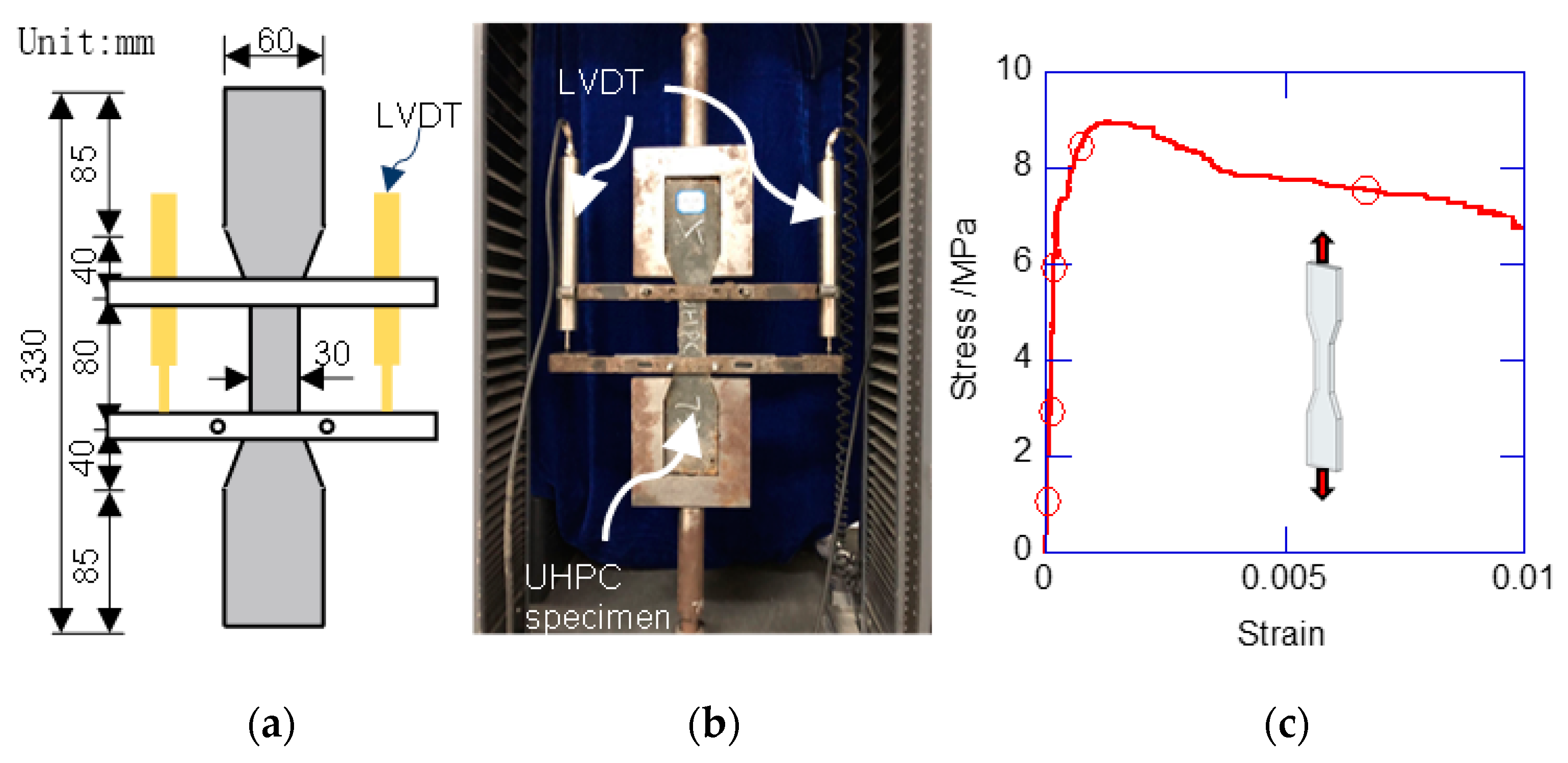
2.3. Loading and Measurement System
3. Results
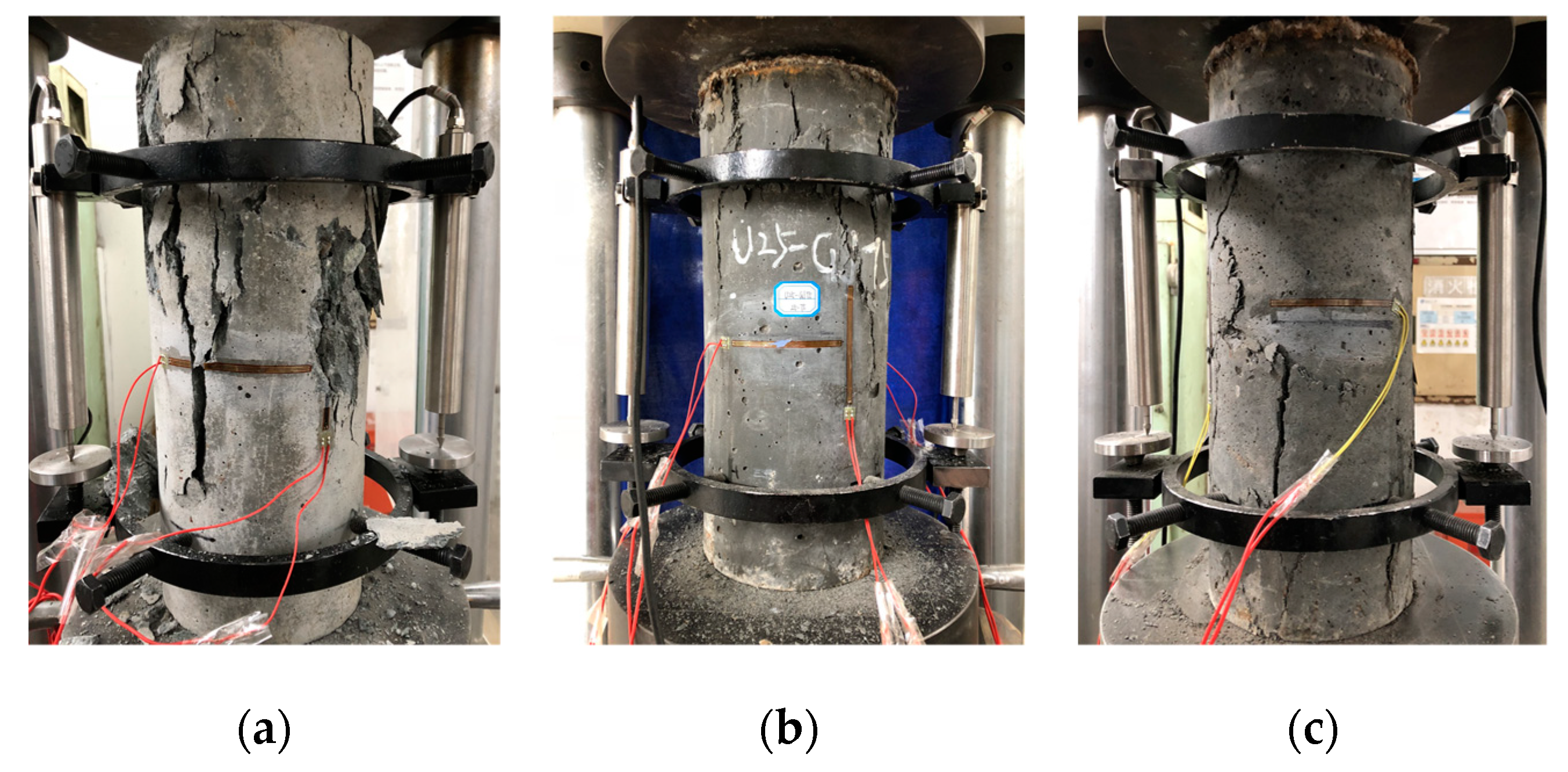
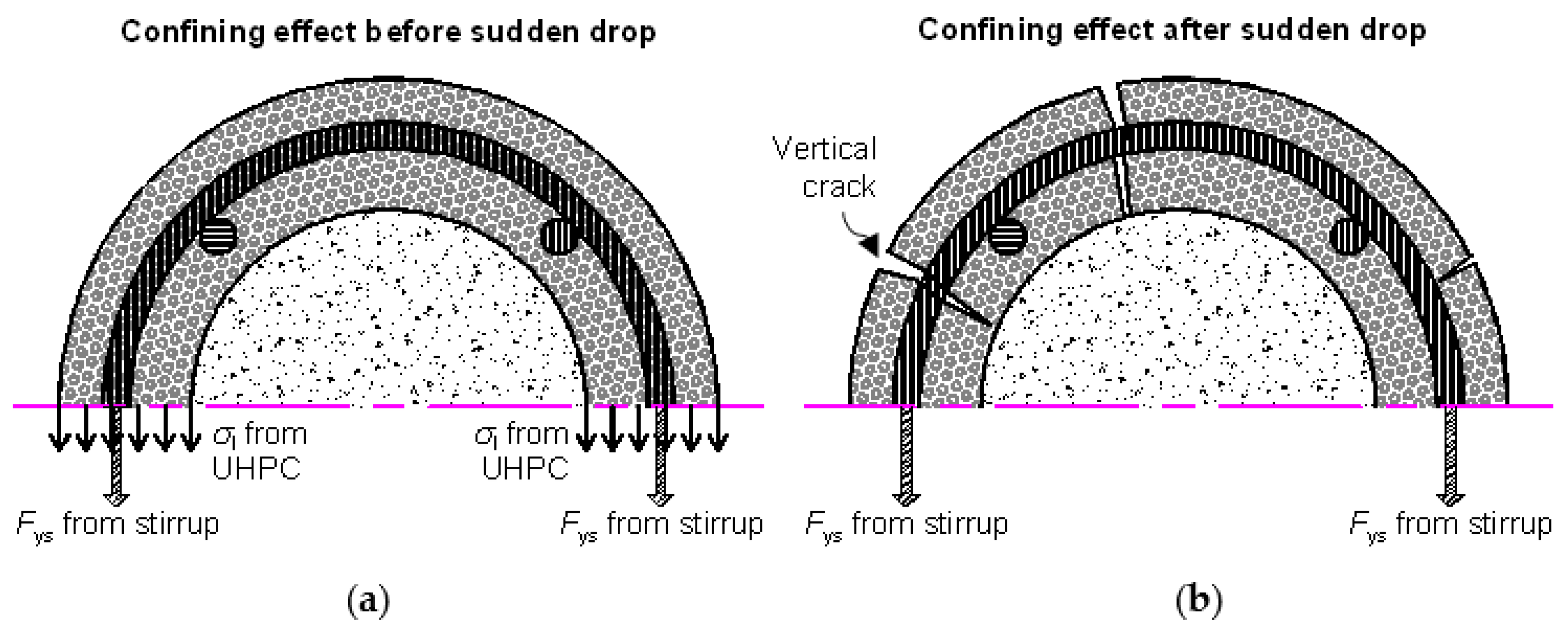
3.1. Damage and Failure Mode
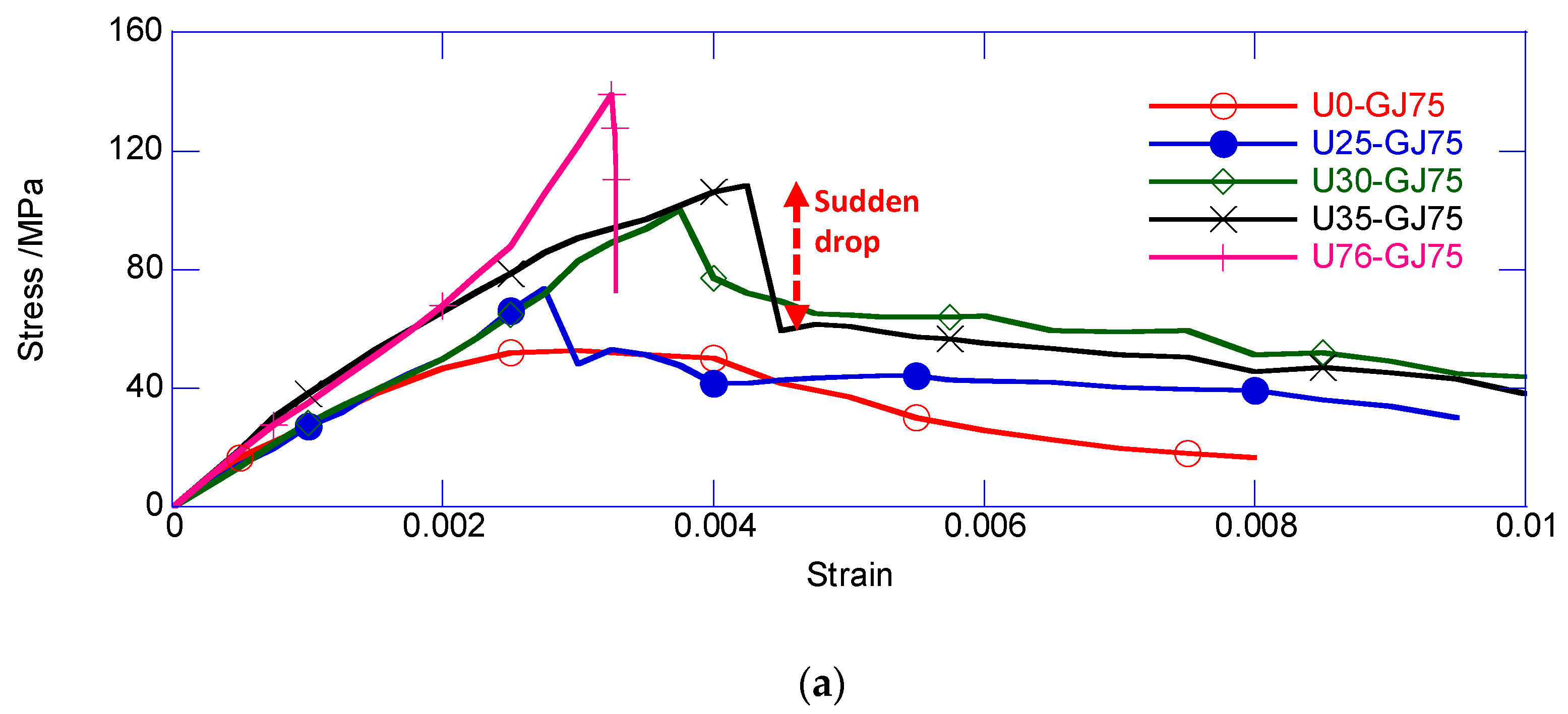
3.2. Strain–Stress Relationship
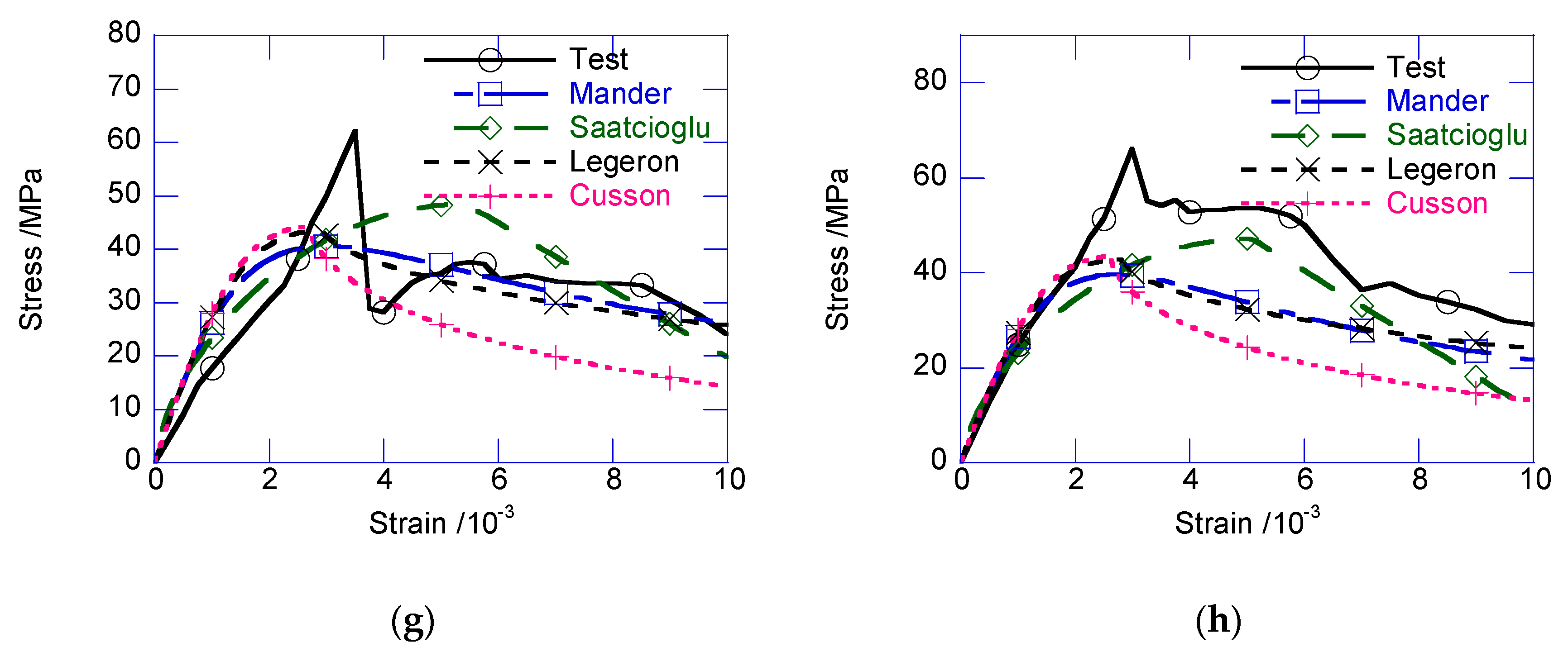
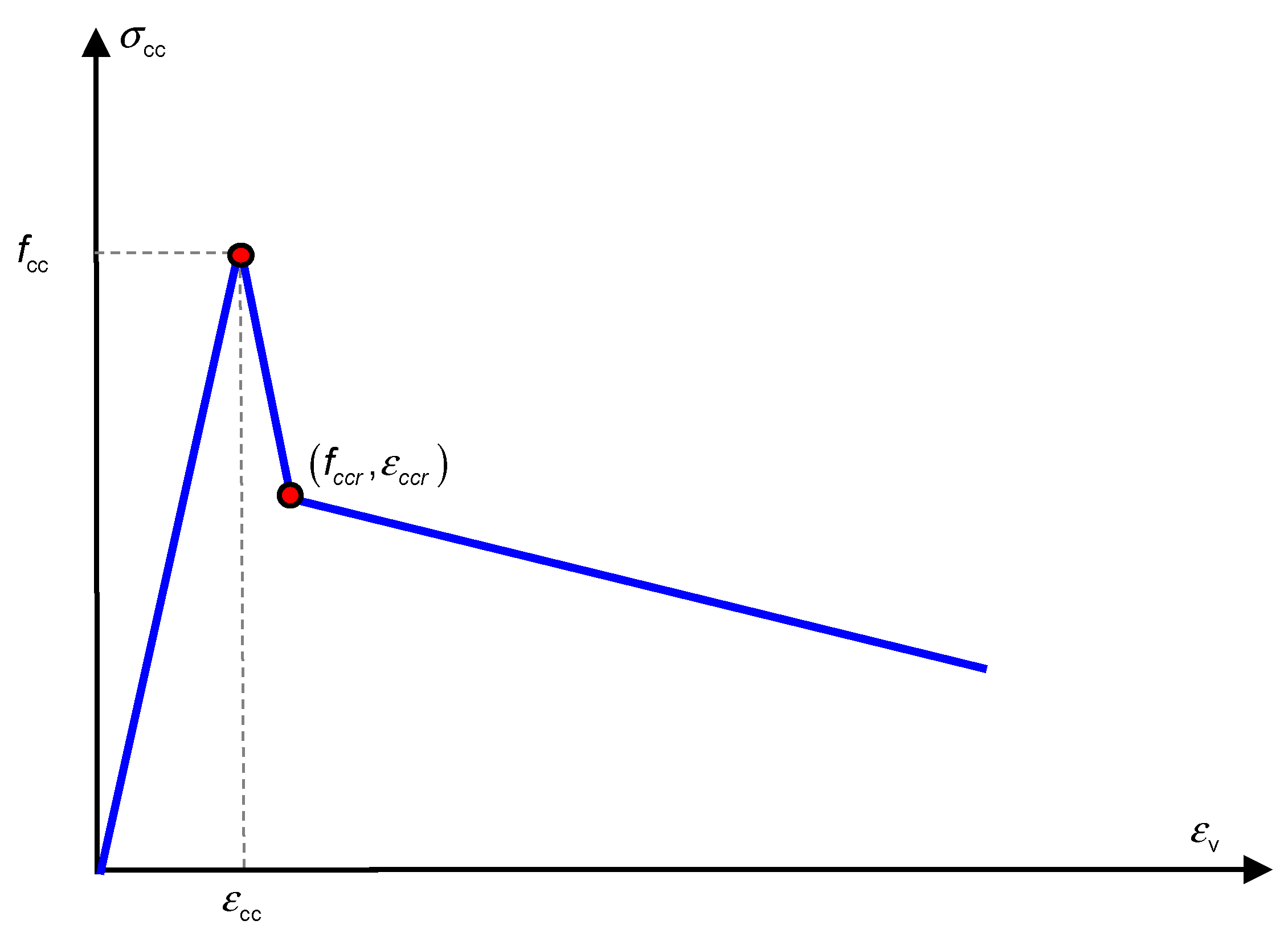
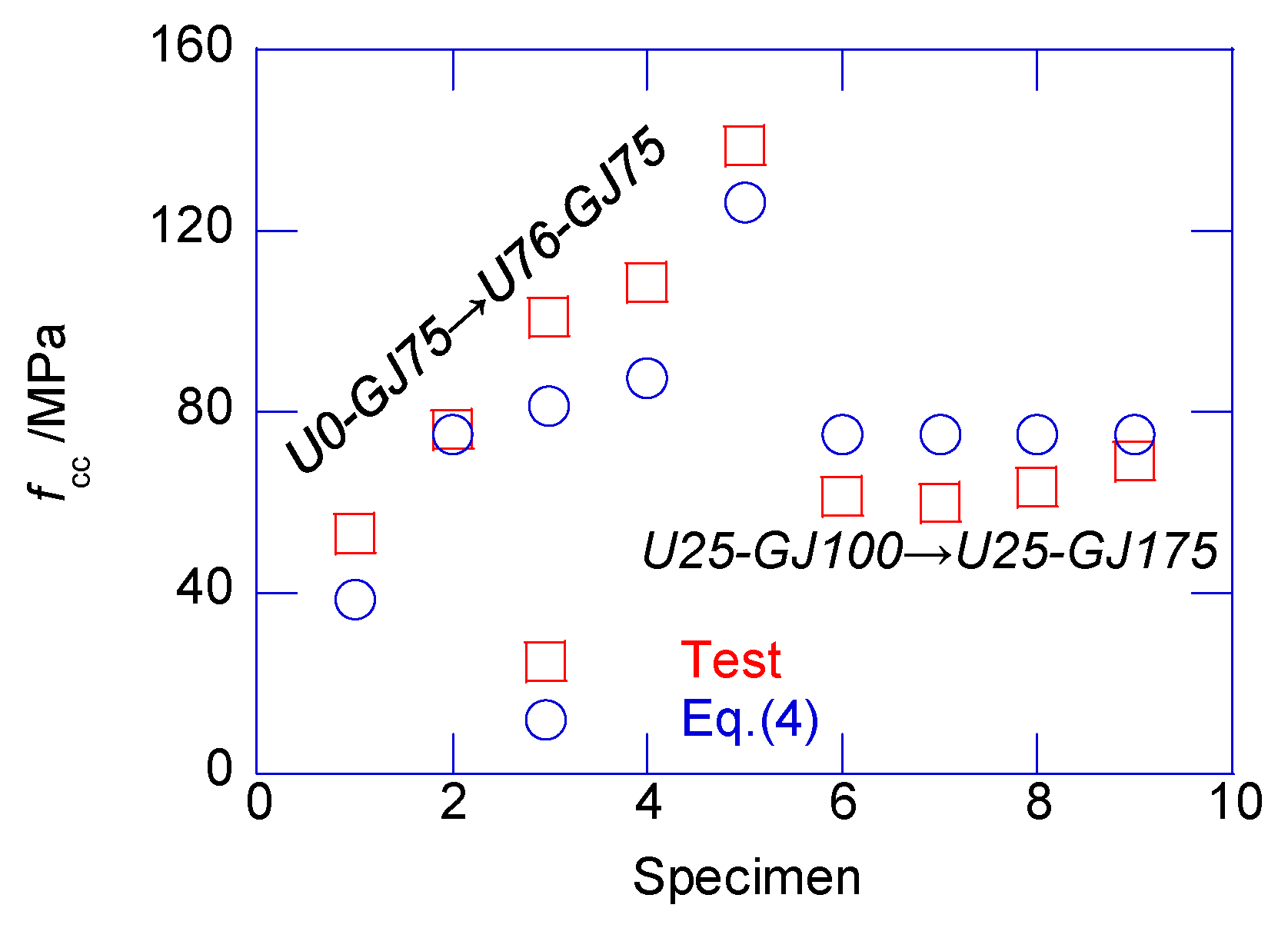
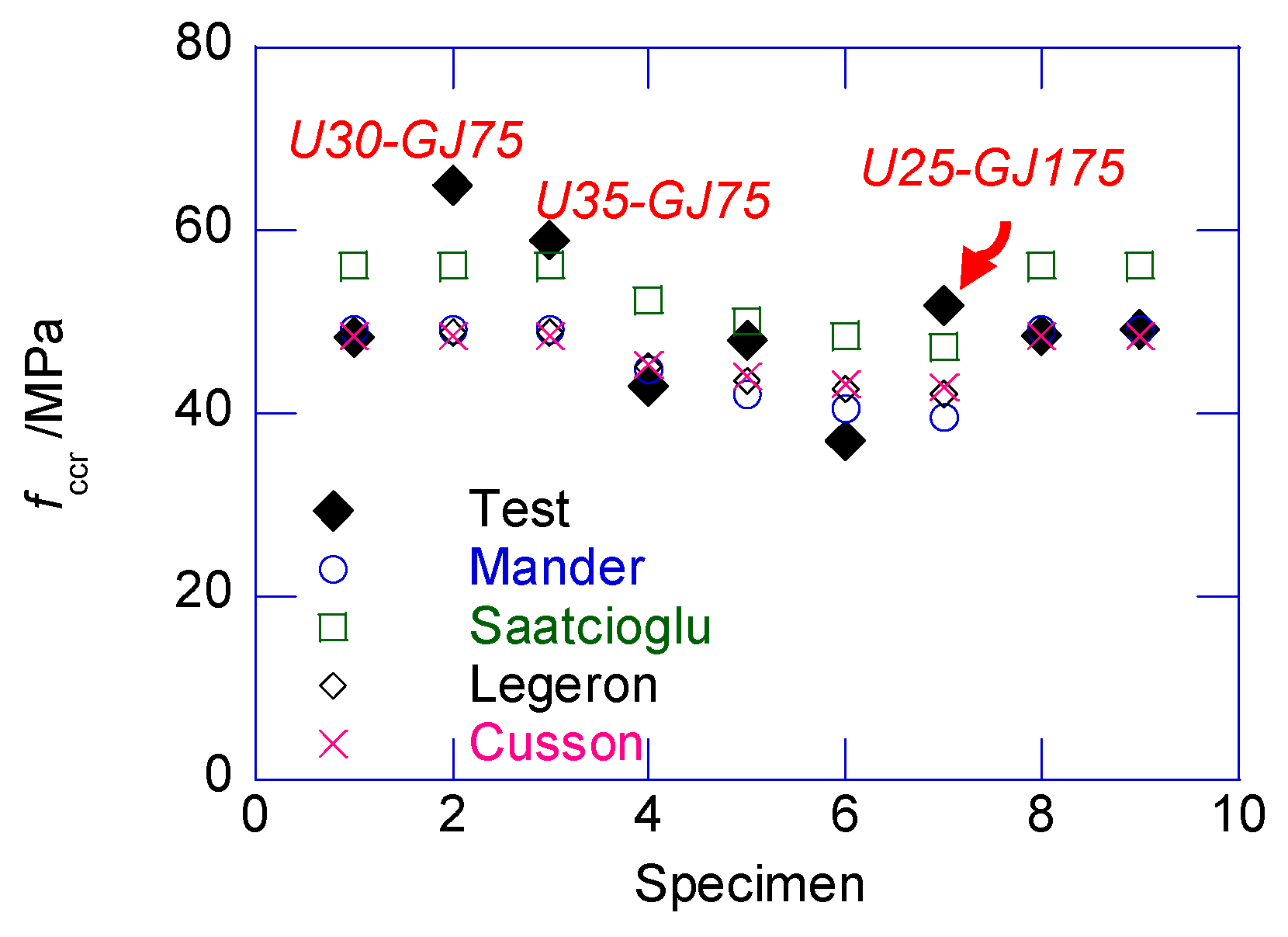
4. Modified Confined Model
5. Conclusions
- The peak confined strength of the reinforced UHPC-NSC composite column occurred at the compressive strain of the UHPC material. The confinement from the stirrup rarely enhanced the deformation of the exterior UHPC cover.
- The distinctive sudden decrease was caused by the loss of confining stress from the exterior UHPC cover. After the sudden decrease, the mechanical status of the composite column degenerated into the reinforced NSC column, wherein the confining stress was mainly provided by the stirrup.
- The confinement from the stirrup did not contribute to the compressive strength of the exterior UHPC cover, and the proposed trilinear model achieved acceptable accuracy, compared with the test results.
- Due to the excessively large spacing of stirrups in the small scaled specimens, the confinement from stirrup was inadequate. Further sophisticated tests for the reinforced UHPC-NSC composite column with the consideration of size effect must be conducted to independently investigate the load sharing and deformation status.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Zhao, C.; Wang, K.; Xu, R.; Deng, K.; Cui, B. Development of fully prefabricated steel-UHPC composite deck system. J. Struct. Eng. 2019, 145, 04019051. [Google Scholar] [CrossRef]
- Vítek, J.L.; Coufal, R.; Čítek, D. UHPC–development and testing on structural elements. Procedia Eng. 2013, 65, 218–223. [Google Scholar] [CrossRef]
- Wang, K.; Zhao, C.; Wu, B.; Deng, K.; Cui, B. Fully-scale test and analysis of fully dry-connected prefabricated steel-UHPC composite beam under hogging moments. Eng. Struct. 2019, 197, 109380. [Google Scholar] [CrossRef]
- Zhao, C.; Wang, K.; Zhou, Q.; Deng, K.; Cui, B. Full-scale test and simulation on flexural behavior of dovetail-shaped reactive powder–concrete wet joint in a composite deck system. J. Bridge Eng. 2018, 23, 04018051. [Google Scholar] [CrossRef]
- Zeng, X.; Deng, K.; Liang, H.; Xu, R.; Zhao, C.; Cui, B. Uniaxial behavior and constitutive model of reinforcement confined coarse aggregate UHPC. Eng. Struct. 2020, 207, 110261. [Google Scholar] [CrossRef]
- Lubkowski, Z.A.; Duan, X. EN1998 Eurocode 8: Design of structures for earthquake resistance. In Proceedings of the Institution of Civil Engineers—Civil Engineering; Thomas Telford Ltd: London, UK, 2001; Volume 144, pp. 55–60. [Google Scholar]
- Hajek, P.; Kynclova, M.; Fiala, C. Timber–UHPC composite floor structures—Environmental study. In Proceedings of the 3rd International Symposium on UHPC, Kassel, Germany, 5–7 March 2012; pp. 674–679. [Google Scholar]
- Maalej, M.; Leong, K.S. Engineered cementitious composites for effective FRP-strengthening of RC beams. Compos. Sci. Technol. 2005, 65, 1120–1128. [Google Scholar] [CrossRef]
- Maalej, M.; Lin, V.W.J.; Nguyen, M.P.; Quek, S.T. Engineered cementitious composites for effective strengthening of unreinforced masonry walls. Eng. Struct. 2010, 32, 2432–2439. [Google Scholar] [CrossRef]
- Li, F.; Feng, Z.; Deng, K.; Yu, Y.; Hu, Z.; Jin, H. Axial behavior of reinforced PP-ECC column and hybrid NSC-ECC column under compression. Eng. Struct. 2019, 195, 223–230. [Google Scholar] [CrossRef]
- Caluk, N.; Mantawy, I.; Azizinamini, A. Durable bridge columns using stay-in-place UHPC shells for accelerated bridge construction. Infrastructures 2019, 4, 25. [Google Scholar] [CrossRef]
- Ministry of Housing and Urban-Rural Construction of the People’s Republic of China; General Administration of Quality Supervision, Inspection, Quarantine of the People’s Republic of China. GB50010 C S. Code for Design of Concrete Structures; GB50010-2010; China Building Industry Press: Beijing, China, 2010. (In Chinese) [Google Scholar]
- Zhong, W.Z.; Song, S.C.; Chen, G.; Huang, X.C.; Huang, P. Stress field of orthotropic cylinder subjected to axial compression. Appl. Math. Mech. 2010, 31, 305–316. [Google Scholar] [CrossRef]
- Mander, J.B.; Priestley, M.J.N.; Park, R. Theoretical stress-strain model for confined concrete. J. Struct. Eng. 1988, 114, 1804–1826. [Google Scholar] [CrossRef]
- Saatcioglu, M.; Razvi, S.R. Strength and ductility of confined concrete. J. Struct. Eng. 1992, 118, 1590–1607. [Google Scholar] [CrossRef]
- Légeron, F.; Paultre, P. Uniaxial confinement model for normal-and high-strength concrete columns. J. Struct. Eng. 2003, 129, 241–252. [Google Scholar] [CrossRef]
- Cusson, D.; Paultre, P. Stress-strain model for confined high-strength concrete. J. Struct. Eng. 1995, 121, 468–477. [Google Scholar] [CrossRef]






| Specimen | Type | tu/mm | s/mm | ρv | Remark |
|---|---|---|---|---|---|
| U0-GJ75 | NSC | 0 | 75 | 1.26% | Benchmark |
| U25-GJ75 | Composite | 25 | 75 | 1.26% | Thickness of UHPC cover |
| U30-GJ75 | 30 | 75 | 1.26% | ||
| U35-GJ75 | 35 | 75 | 1.26% | ||
| U76-GJ75 | UHPC | - | 75 | 1.26% | |
| U25-GJ100 | Composite | 25 | 100 | 0.94% | Volumetric stirrup ratio |
| U25-GJ125 | 25 | 125 | 0.76% | ||
| U25-GJ150 | 25 | 150 | 0.63% | ||
| U25-GJ175 | 25 | 175 | 0.54% | ||
| U25-GJ0 | 25 | - | 0.0% | ||
| U25-GJ75-L | 25 | 75 | 1.26% | Construction method | |
| U35-GJ75-L | 35 | 75 | 1.26% |
| Matrix (kg/m3) | Performance Indicators of Steel Fibers | ||||
|---|---|---|---|---|---|
| Cement: 680 Steel fiber: 100 Water: 160 Concrete Admixtures: 18 | Volume ratio | Length (mm) | Density (kg/m3) | Diameter (μm) | Tensile modulus of elasticity (Gpa) |
| 2% | 12 | 0.78 | 20 | 200 | |
| Material | E/GPa | fti/MPa | ftu/MPa | /10−3 | fco/MPa | /10−3 |
|---|---|---|---|---|---|---|
| UHPC | 43.38 | 7.8 | 9.29 | 1.08 | 126.45 | 3.08 |
| NSC | 29.51 | - | - | - | 38.63 | 2.01 |
| Specimen | fcc/MPa | fccr/MPa | (fcc−fccr)/MPa | Esd/GPa | |||
|---|---|---|---|---|---|---|---|
| U0-GJ75 | 53.20 | 2.76 | - | - | - | - | - |
| U25-GJ75 | 76.16 | 2.88 | 48.27 | 2.90 | 27.89 | 0.02 | −2.44 |
| U30-GJ75 | 101.04 | 3.82 | 65.08 | 4.07 | 35.96 | 0.25 | −4.71 |
| U35-GJ75 | 108.80 | 4.20 | 59.23 | 4.47 | 49.57 | 0.27 | −3.91 |
| U76-GJ75 | 138.63 | 3.26 | - | - | - | - | - |
| U25-GJ100 | 61.34 | 2.98 | 43.08 | 3.43 | 18.26 | 0.45 | −2.73 |
| U25-GJ125 | 59.90 | 3.56 | 48.01 | 3.81 | 11.89 | 0.25 | −4.85 |
| U25-GJ150 | 63.43 | 3.47 | 37.08 | 3.57 | 26.35 | 0.10 | −0.71 |
| U25-GJ175 | 69.06 | 3.15 | 51.89 | 3.29 | 17.17 | 0.14 | −4.50 |
| U25-GJ0 | 67.77 | 2.66 | 43.04 | 2.67 | 24.73 | 0.01 | −4.52 |
| U25-GJ75-L | 72.99 | 3.67 | 48.46 | 3.71 | 24.53 | 0.04 | −2.75 |
| U35-GJ75-L | 97.43 | 3.27 | 49.13 | 4.13 | 48.30 | 0.86 | −3.16 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, F.; Hexiao, Y.; Gao, H.; Deng, K.; Jiang, Y. Axial Behavior of Reinforced UHPC-NSC Composite Column under Compression. Materials 2020, 13, 2905. https://doi.org/10.3390/ma13132905
Li F, Hexiao Y, Gao H, Deng K, Jiang Y. Axial Behavior of Reinforced UHPC-NSC Composite Column under Compression. Materials. 2020; 13(13):2905. https://doi.org/10.3390/ma13132905
Chicago/Turabian StyleLi, Fuhai, Yunfeng Hexiao, Hao Gao, Kailai Deng, and Yilin Jiang. 2020. "Axial Behavior of Reinforced UHPC-NSC Composite Column under Compression" Materials 13, no. 13: 2905. https://doi.org/10.3390/ma13132905
APA StyleLi, F., Hexiao, Y., Gao, H., Deng, K., & Jiang, Y. (2020). Axial Behavior of Reinforced UHPC-NSC Composite Column under Compression. Materials, 13(13), 2905. https://doi.org/10.3390/ma13132905






