Acoustic Emission Monitoring of High-Strength Concrete Columns Subjected to Compressive Axial Loading
Abstract
:1. Introduction
2. Experimental Program
2.1. Material Properties
2.1.1. Concrete
2.1.2. Steel Reinforcement
2.2. Test Set-Up and Instrumentation
3. Test Results
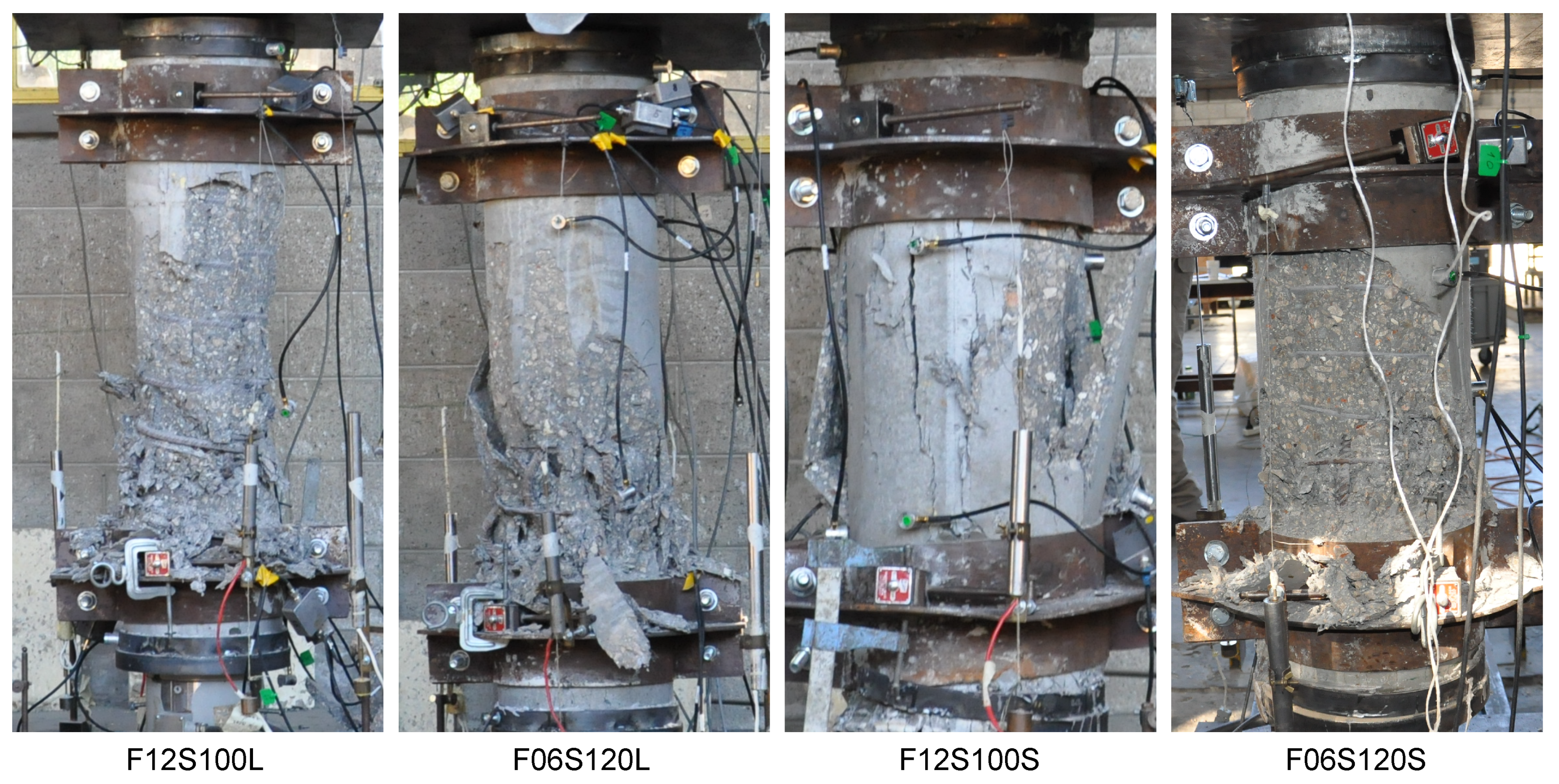
3.1. General
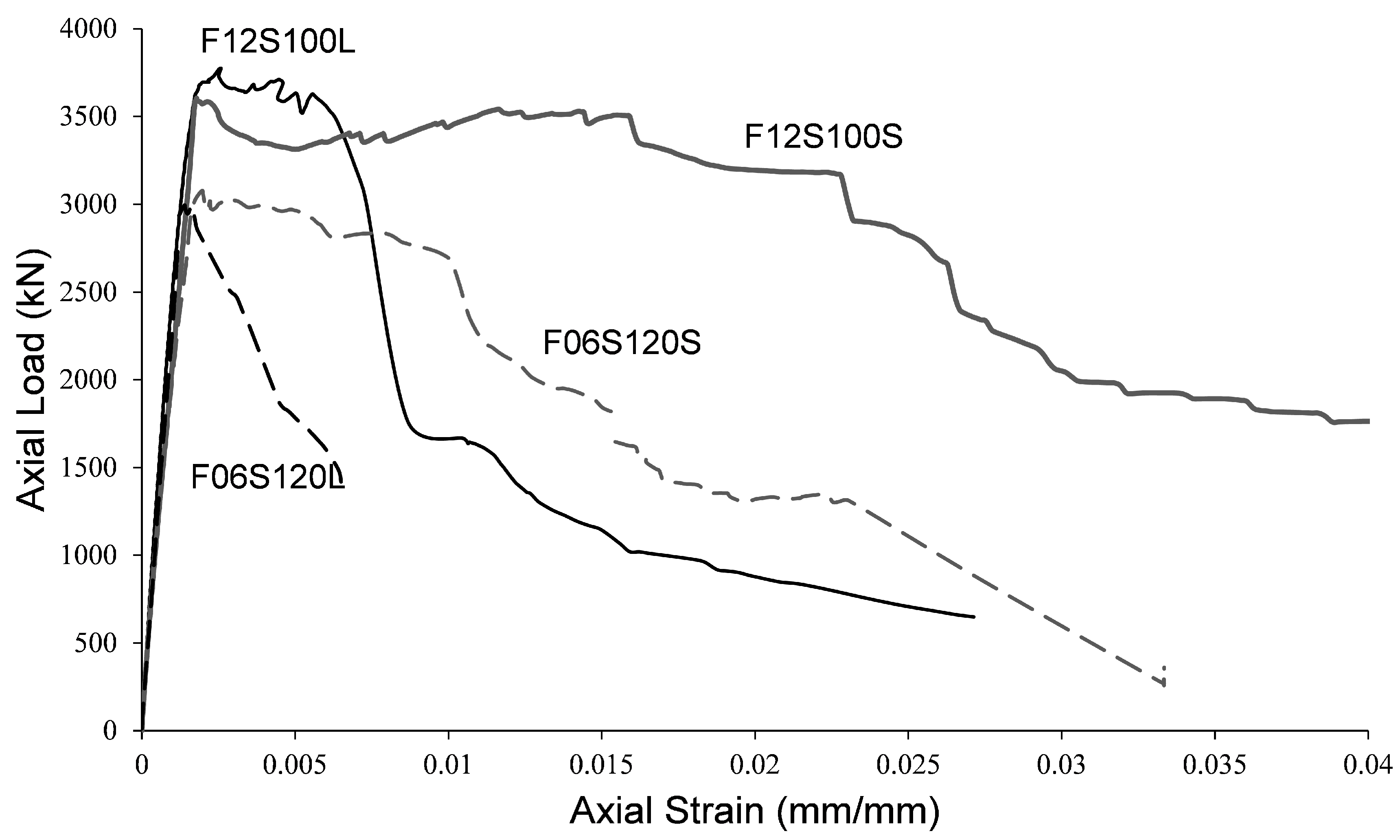
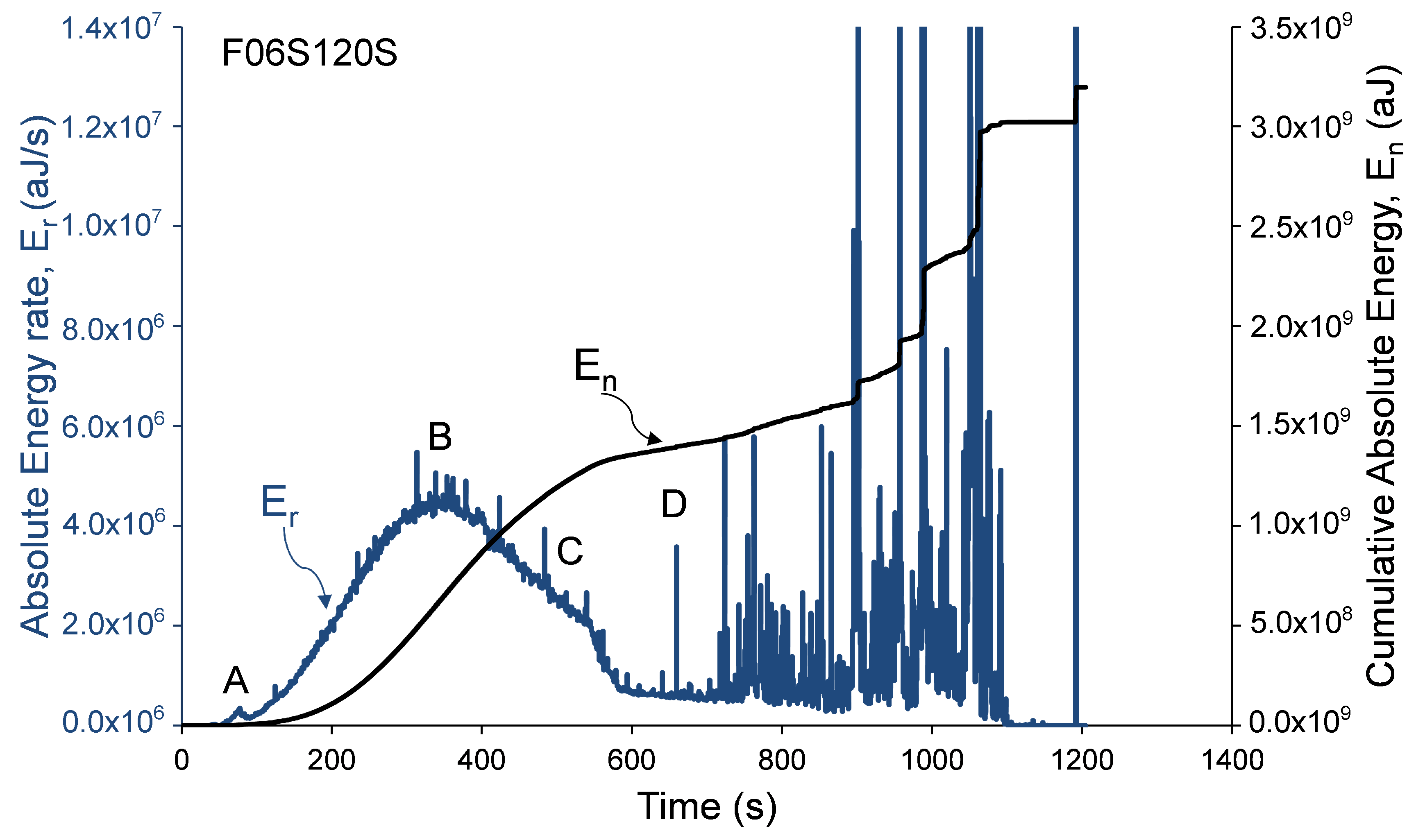
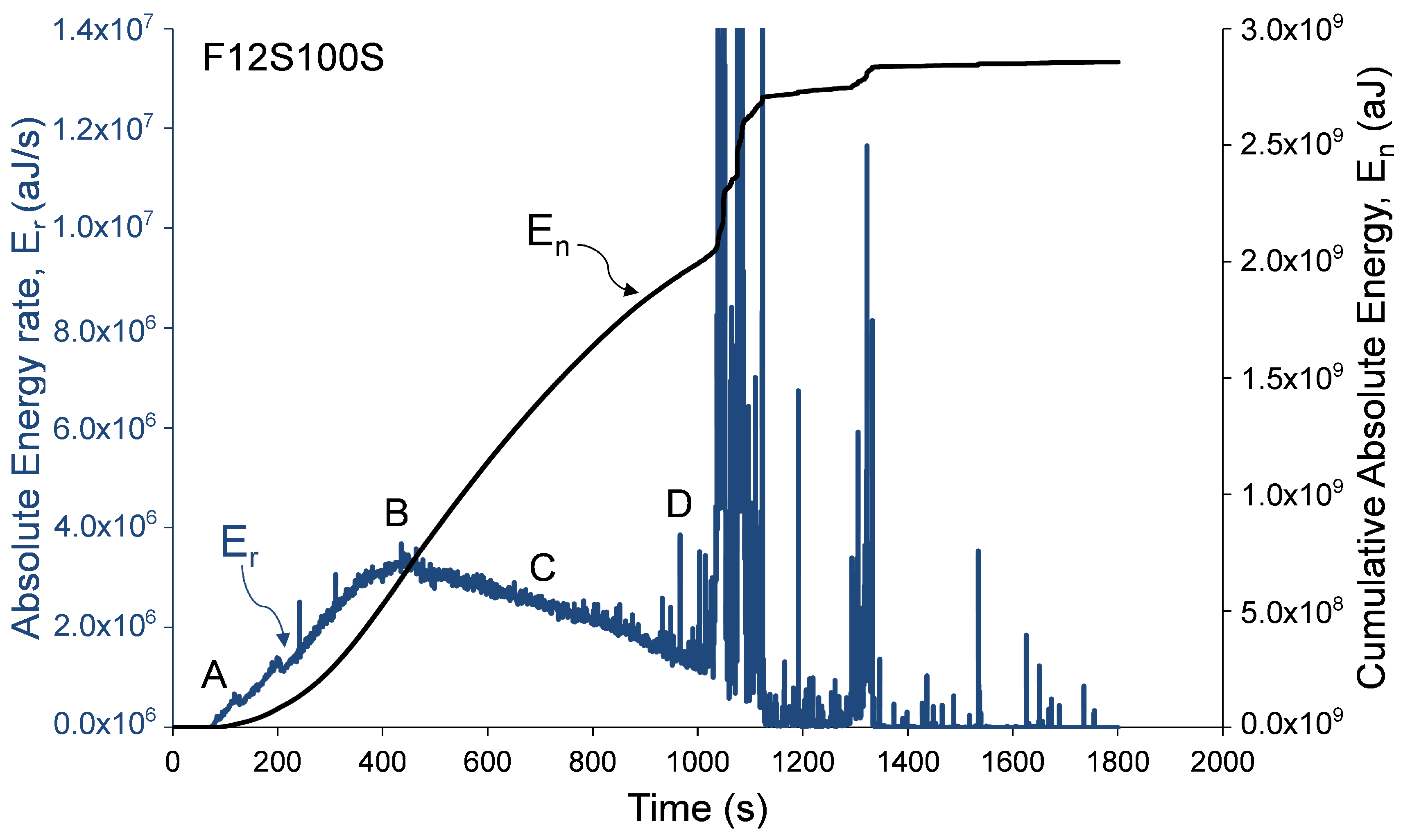
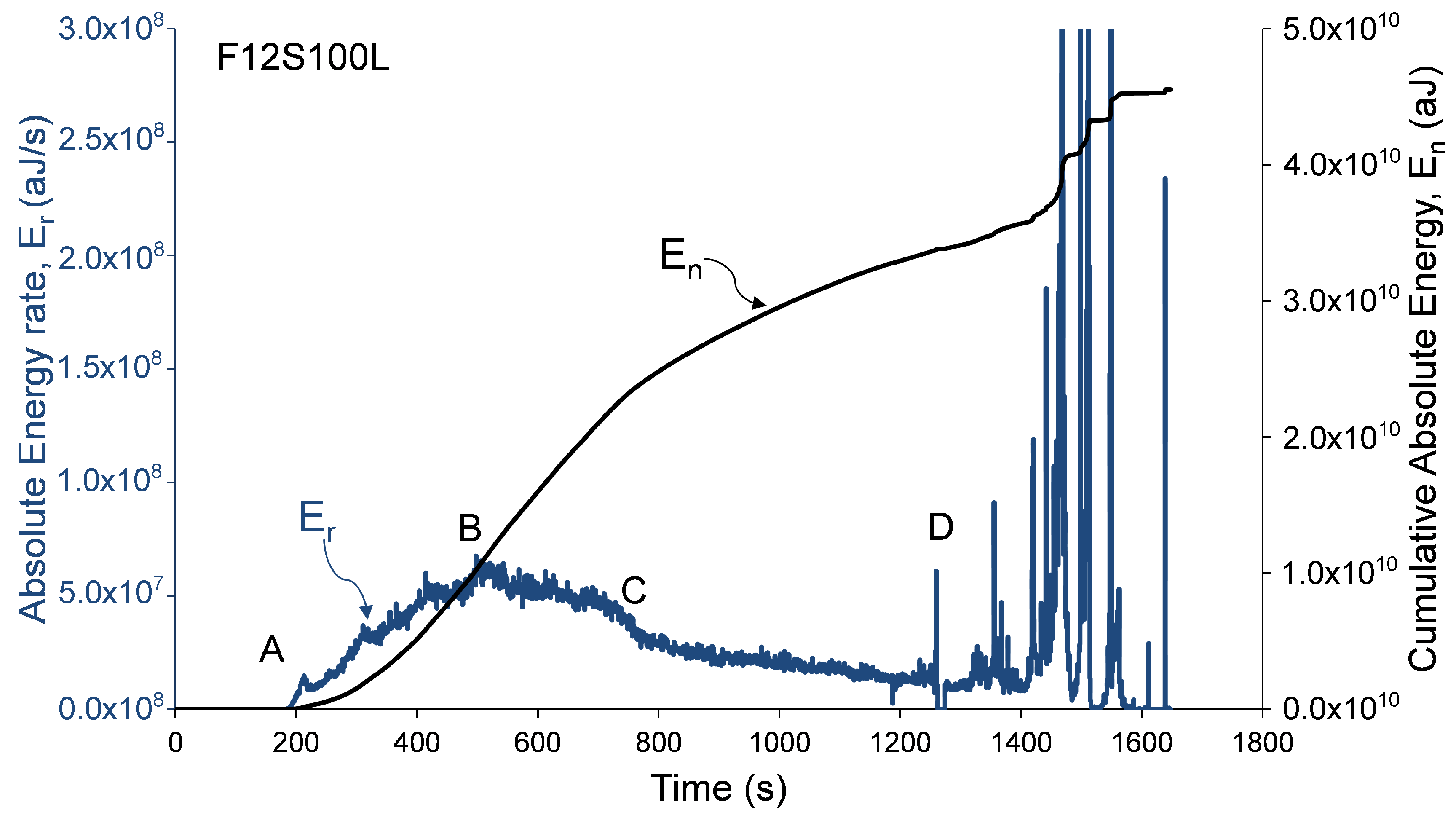
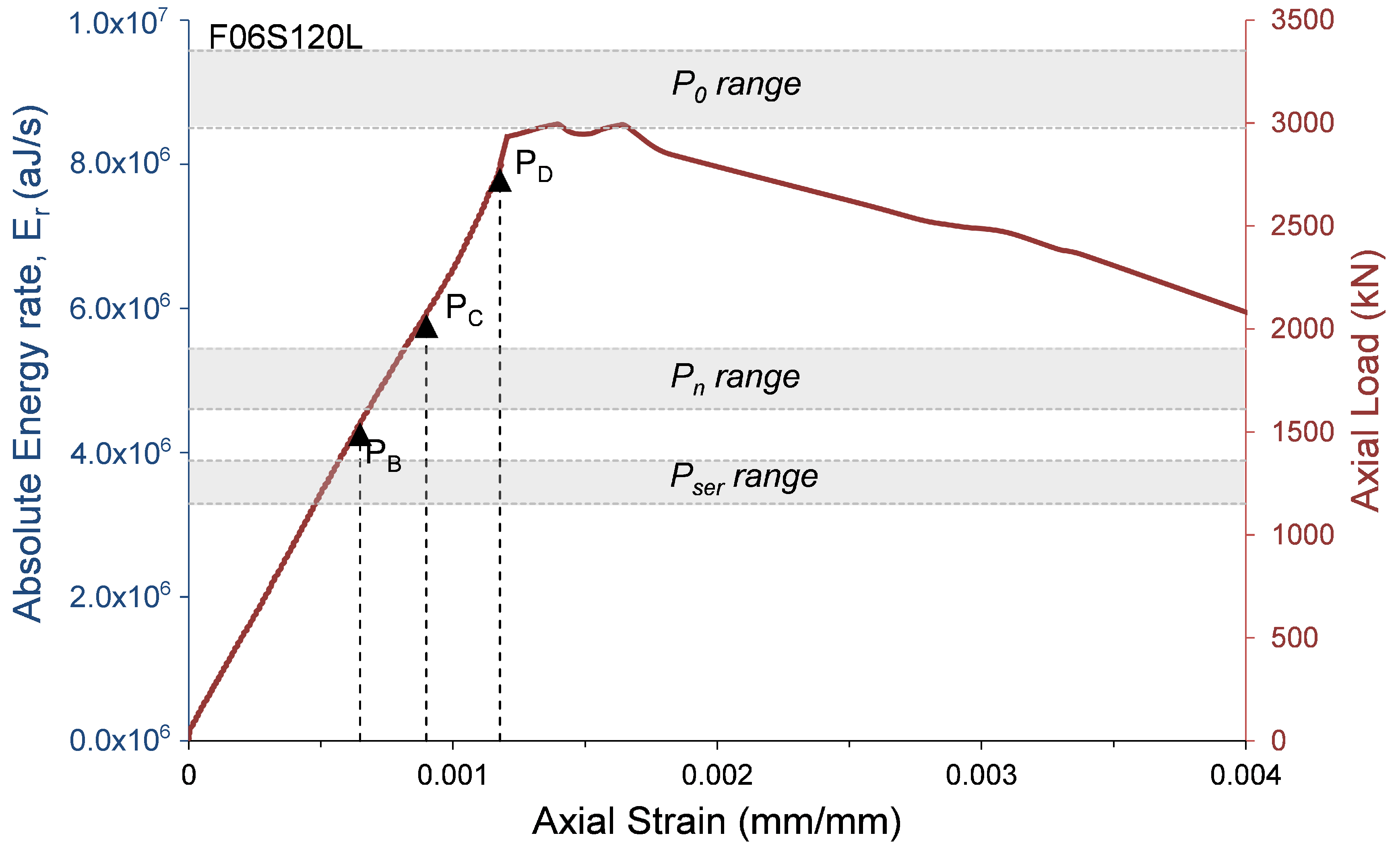
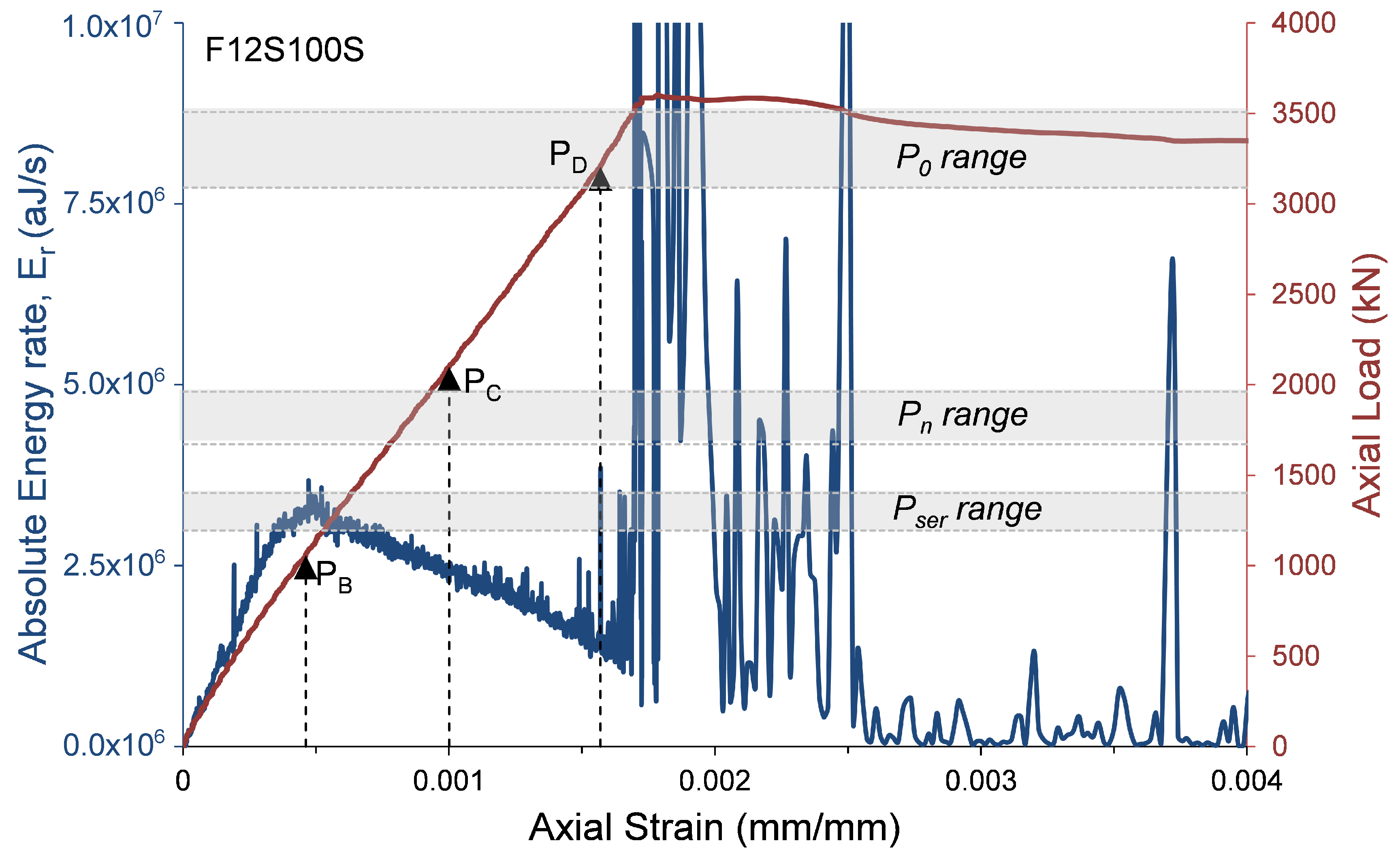
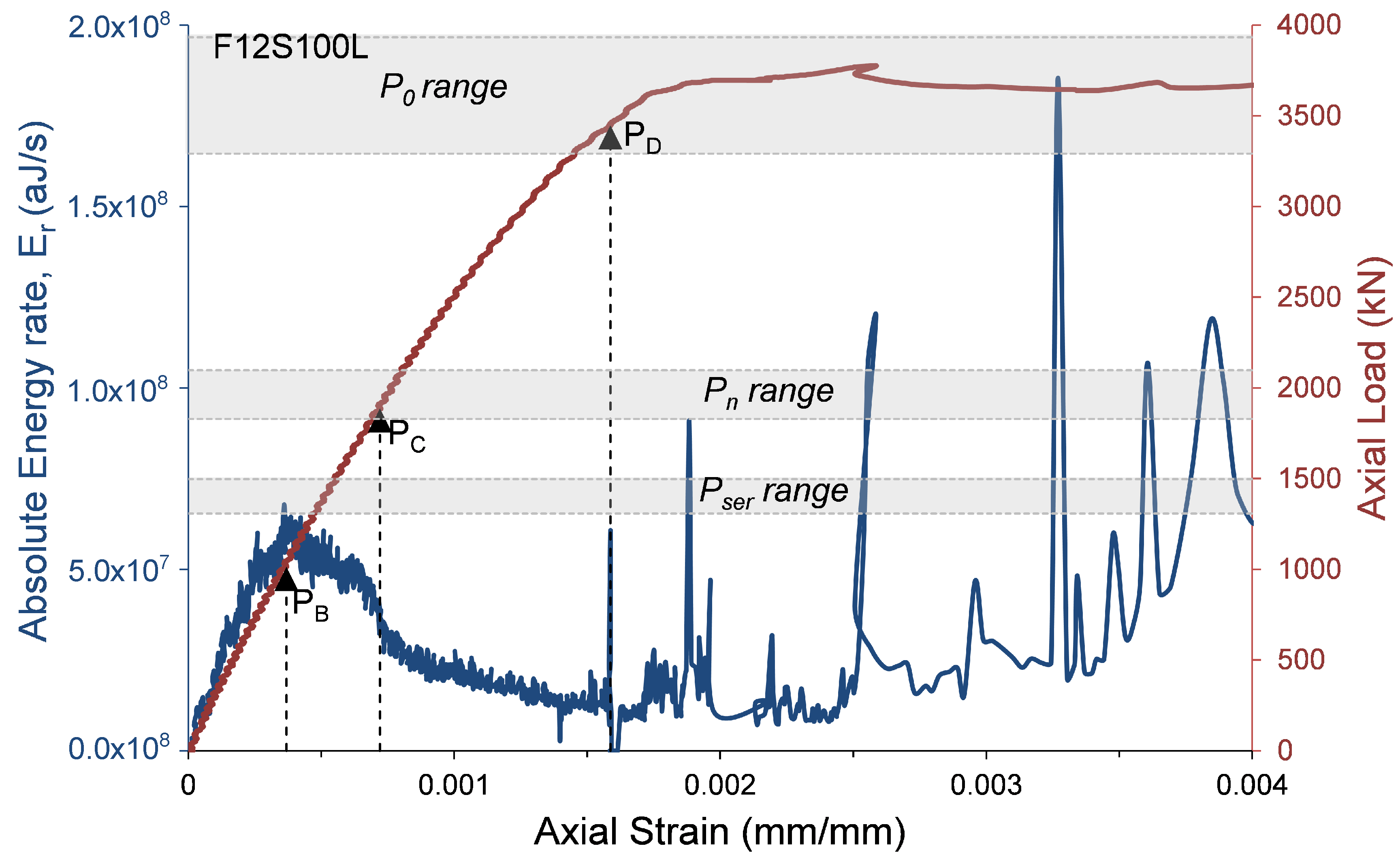
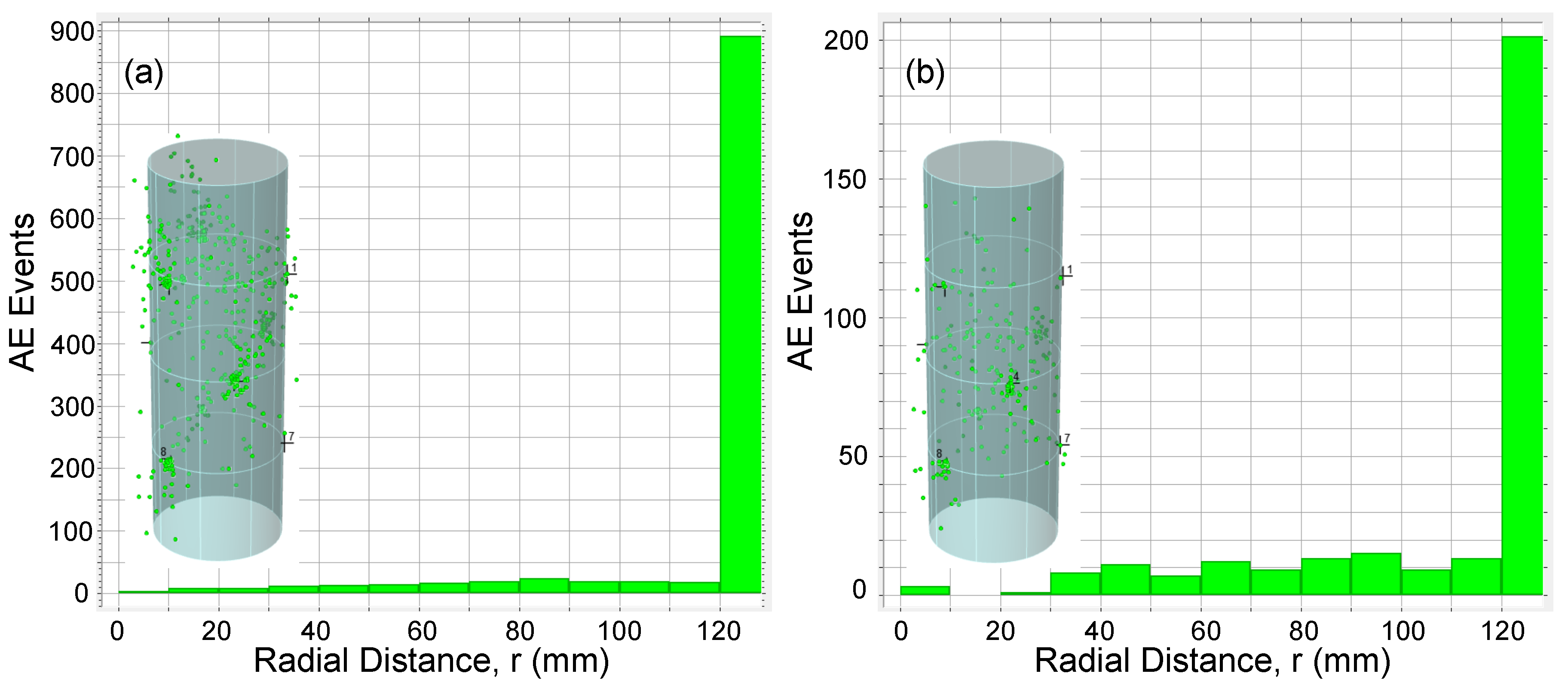
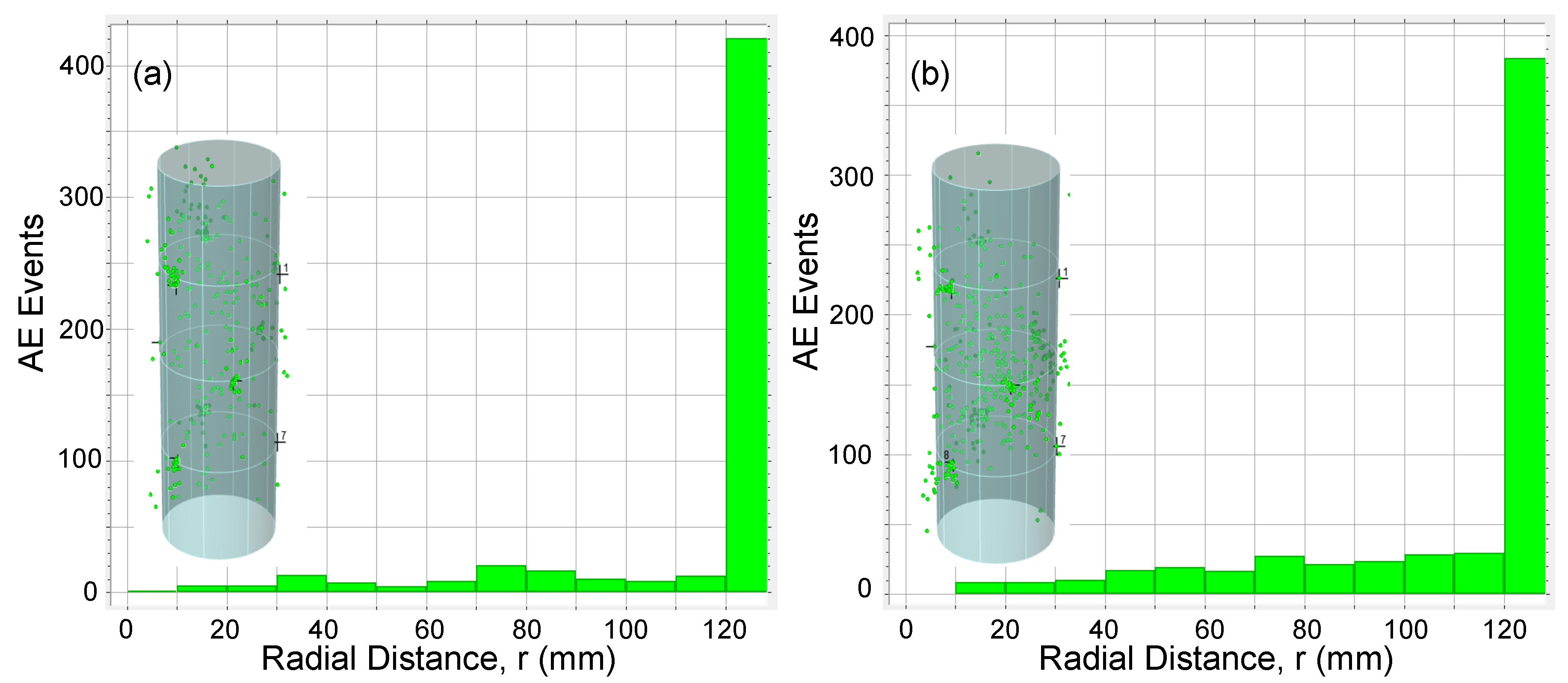
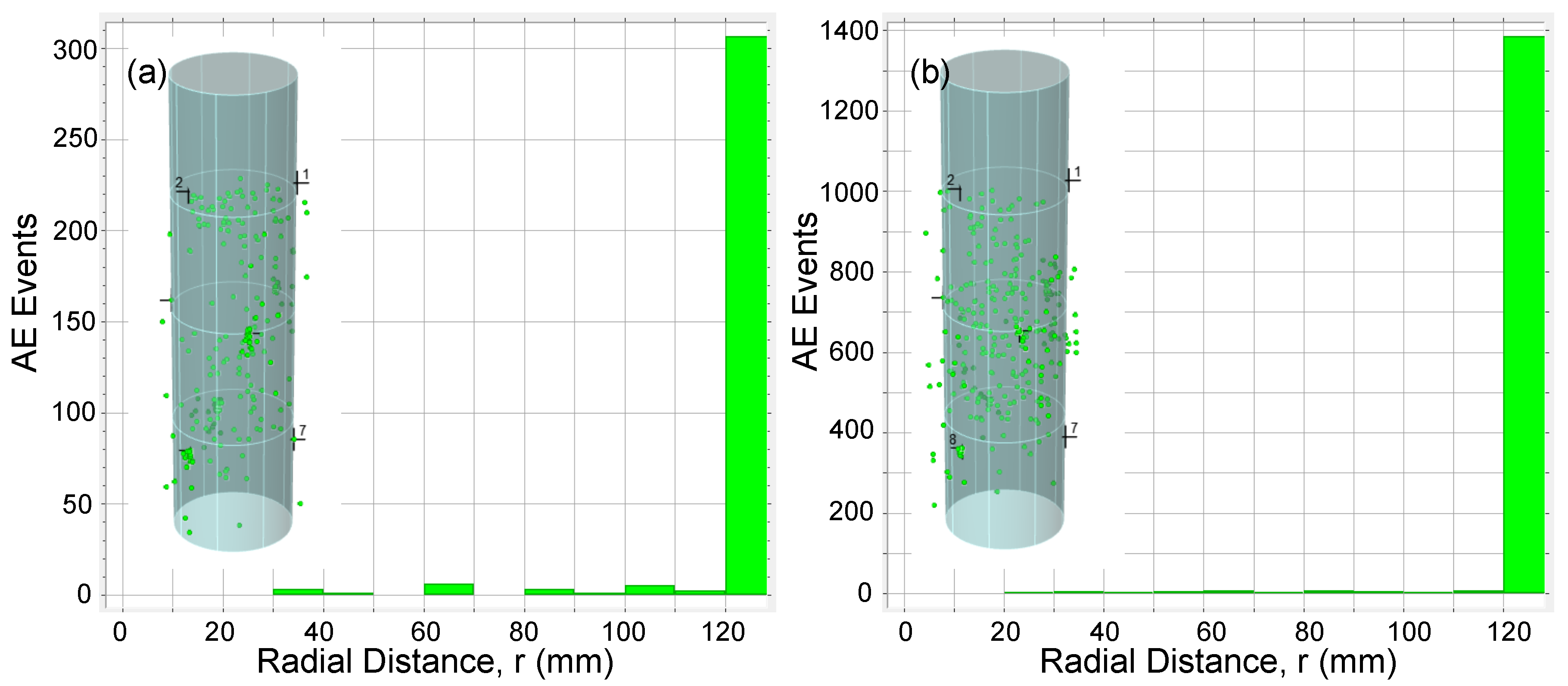
3.2. Acoustic Emission and Compressive Behavior
4. Conclusions
- The AE distributions in the columns are categorized by three stages defined by the initiation of AE activity, the peak AE absolute energy rate distribution, the beginning of steady damage accumulation, and the first AE macro event and initiation of macro-damage development.
- The levels of loads reached at the peak AE energy distribution are similar to the levels of the assumed serviceability axial compressive strengths of the tested specimens.
- The levels of loads reached at the beginning of steady damage accumulation are similar to the levels of the design axial compressive strengths of the tested specimens.
- The levels of loads reached at the first AE macro event are similar to the lower range levels of the nominal axial compressive strengths of the tested specimens.
- The majority of AE events are located at the concrete cover and especially those associated with the macro-cracking.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| AE | Acoustic Emission |
| TSR | Transverse steel reinforcement |
| NSC | Normal-strength concrete |
| HSC | High-strength concrete |
| RC | Reinforced-concrete |
References
- Eid, R.; Kovler, K.; David, I.; Khoury, W.; Miller, S. Behavior and design of high-strength circular reinforced concrete columns subjected to axial compression. Eng. Struct. 2018, 173, 472–480. [Google Scholar] [CrossRef]
- Paultre, P.; Khayat, K.H.; Langlois, Y.; Trudel, A.; Cusson, D. Structural performance of some special concretes. In Proceedings of the 4th International Sumposium on Utilization of High-Strength/High-performance Concrete, Paris, France, 29–31 May 1996; pp. 787–796. [Google Scholar]
- Foster, S.J.; Liu, J.; Sheikh, S.A. Cover spalling in HSC columns loaded in concentric compression. ASCE J. Struct. Eng. 1998, 124, 1431–1437. [Google Scholar] [CrossRef]
- Khaliq, W.; Kodur, V. Effectiveness of polypropylene and steel fibers in enhancing fire resistance of high-strength concrete columns. ASCE J. Struct. Eng. 2018, 144, 04017224. [Google Scholar] [CrossRef]
- Hager, I.; Mróz, K. Role of polypropylene fibres in concrete spalling risk mitigation in fire and test methods of fibres effectiveness evaluation. Materials 2019, 12, 3869. [Google Scholar] [CrossRef] [Green Version]
- Bangi, M.R.; Horiguchi, T. Effect of fibre type and geometry on maximum pore pressures in fibre-reinforced high strength concrete at elevated temperatures. Cem. Concr. Res. 2012, 42, 459–466. [Google Scholar] [CrossRef]
- Zhu, Y.K.; Tian, G.Y.; Lu, R.S.; Zhang, H. A review of optical NDT technologies. Sensors 2011, 11, 7773–7798. [Google Scholar] [CrossRef] [Green Version]
- Maj, M.; Ubysz, A.; Hammadeh, H.; Askifi, F. Non-destructive testing of technical conditions of RC industrial tall chimneys subjected to high temperature. Materials 2019, 12, 2027. [Google Scholar] [CrossRef] [Green Version]
- Schabowicz, K. Non-destructive testing of materials in civil engineering. Materials 2019, 12, 3237. [Google Scholar] [CrossRef] [Green Version]
- Kobaka, J.; Katzer, J.; Ponikiewski, T. A combined electromagnetic induction and radar-based test for quality control of steel fibre reinforced concrete. Materials 2019, 12, 3507. [Google Scholar] [CrossRef] [Green Version]
- Ahn, E.; Kim, H.; Sim, S.H.; Shin, S.W.; Shin, M. Principles and applications of ultrasonic-based nondestructive methods for self-healing in cementitious materials. Materials 2017, 10, 278. [Google Scholar] [CrossRef] [Green Version]
- Climent, M.A.; Miró, M.; Carbajo, J.; Poveda, P.; De Vera, G.; Ramis, J. Use of non-linear ultrasonic techniques to detect cracks due to steel corrosion in reinforced concrete structures. Materials 2019, 12, 813. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Xiao, M.; Ju, F.; Ning, P.; Li, K. Mechanical and acoustic emission behavior of gangue concrete under uniaxial compression. Materials 2019, 12, 3318. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Abarkane, C.; Rescalvo, F.J.; Donaire-Ávila, J.; Galé-Lamuela, D.; Benavent-Climent, A.; Molina, A.G. Temporal acoustic emission index for damage monitoring of RC structures subjected to bidirectional seismic loadings. Materials 2019, 12, 2804. [Google Scholar] [CrossRef] [Green Version]
- Mirmiran, A.; Shahawy, M.; El Echary, H. Acoustic emission monitoring of hybrid FRP-concrete columns. ASCE J. Eng. Mech. 1999, 125, 899–905. [Google Scholar] [CrossRef]
- Ma, G.; Li, H. Acoustic emission monitoring and damage assessment of FRP-strengthened reinforced concrete columns under cyclic loading. Constr. Build. Mater. 2017, 144, 86–98. [Google Scholar] [CrossRef]
- Puri, S.; Weiss, J. Assessment of localized damage in concrete under compression using acoustic emission. ASCE J. Mater. Civ. Eng. 2006, 18, 325–333. [Google Scholar] [CrossRef]
- Benavent-Climent, A.; Castro, E.; Gallego, A. AE monitoring for damage assessment of RC exterior beam-column subassemblages subjected to cyclic loading. Struct. Health Monit. 2009, 8, 175–189. [Google Scholar] [CrossRef]
- Benavent-Climent, A.; Gallego, A.; Vico, J.M. An acoustic emission energy index for damage evaluation of reinforced concrete slabs under seismic loads. Struct. Health Monit. 2011, 11, 69–81. [Google Scholar] [CrossRef]
- Du, F.; Li, D.; Shan, B.; Wang, Y. Failure behavior monitoring and evaluation of steel-confined reinforced concrete columns by acoustic emission under quasi-static loading. Latin Am. J. Solids Struct. 2018, 15, 1–18. [Google Scholar] [CrossRef]
- Li, D.; Chen, Z.; Feng, Q.; Wang, Y. Damage analysis of CFRP-confined circular concrete-filled steel tubular columns by acoustic emission techniques. Smart Mater. Struct. 2015, 24, 085017. [Google Scholar] [CrossRef]
- Aldahdooh, M.A.A.; Bunnori, N.M.; Johari, M.M. Damage evaluation of reinforced concrete beams with varying thickness using the acoustic emission technique. Constr. Build. Mater. 2013, 44, 812–821. [Google Scholar] [CrossRef]
- European Standard 1992-1-1. Eurocode 2: Design of Concrete Structures. Part 1-1: General Rules and Rules for Buildings; European Committee for Standardization: Brussels, Belgium, 2004. [Google Scholar]
- Canadian Standard Association (CSA) A23.3. Design of Concrete Structures; CSA Group: Toronto, ON, Canada, 2014. [Google Scholar]
- Standards New Zealand (NZS) 3101. Concrete Structures Standard, Part 1—The Design of Concrete Structures; Standards New Zealand: Wellington, New Zealand, 2006. [Google Scholar]
- Eid, R.; Cohen, A.; Guma, R.; Ifrach, E.; Levi, N.; Zvi, A. High-strength concrete circular columns with TRC-TSR dual internal confinement. Buildings 2019, 9, 218. [Google Scholar] [CrossRef] [Green Version]
- American Concrete Institute ACI 318. Building Code Requirements for Structural Concrete; American Concrete Institute: Farmington Hills, MI, USA, 2019. [Google Scholar]
- Razvi, S.R.; Saatcioglu, M. Strength and deformability of confined high-strength concrete columns. ACI Struct. J. 1994, 91, 678–687. [Google Scholar]
- Azizinamini, A.; Kuska, S.S.B.; Brungardt, P.; Hatfield, E. Seismic Behavior of Square High-Strength Concrete Columns. ACI Struct. J. 1994, 91, 336–345. [Google Scholar]
- Ozbakkaloglu, T.; Saatcioglu, M. Rectangular stress block for high-strength concrete. ACI Struct. J. 2004, 101, 475–483. [Google Scholar]
- Bae, S.; Bayrak, O. Examination of stress block Parameters for high-strength concrete in the context of ACI 318 Code. In Proceedings of the Reinforced Concrete Columns with High Strength Concrete and Steel Reinforcement, SP-293, Farmington Hills, MI, USA, 9 January 2013; pp. 59–77. [Google Scholar]
- American Concrete Institute ACI 441.1R-18. Report on Equivalent Rectangular Concrete Stress Block and Transverse Reinforcement for High-Strength Concrete Columns; American Concrete Institute: Farmington Hills, MI, USA, 2018. [Google Scholar]
- Vallen Systeme GmbH. Vallen AE-Suite Software, Version R2019.0926.2; Vallen Systeme GmbH: Icking, Germany, 2019.
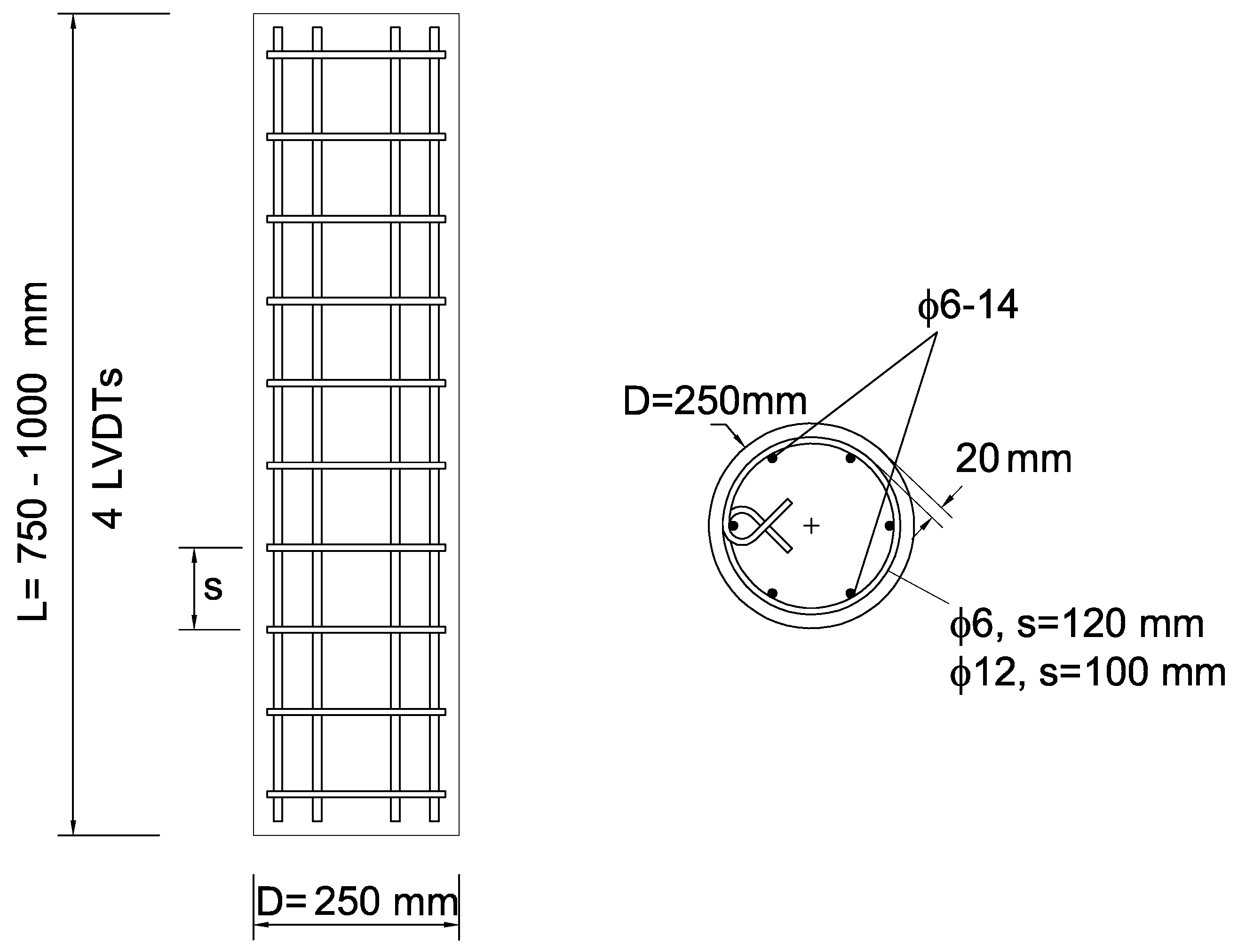




| Longitudinal Reinforcement | Transverse Reinforcement | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Specimen No. | MPa | mm | mm | MPa | % | mm | mm | MPa | % | |
| 1 | F06S120S | 84.8 | 250 | 750 | 433 | 1.88 | 6 | 120 | 288 | 0.44 |
| 2 | F06S120L | 72.1 | 250 | 1000 | 433 | 1.88 | 6 | 120 | 288 | 0.44 |
| 3 | F12S100S | 75.9 | 250 | 750 | 433 | 1.88 | 12 | 100 | 435 | 2.03 |
| 4 | F12S100L | 86.3 | 250 | 1000 | 433 | 1.88 | 12 | 100 | 435 | 2.03 |
| Variable | (kg/m) |
|---|---|
| Cement | 460 |
| Fine aggregate—quartz sand | 220 |
| Intermediate aggregate (9 mm) | 900 |
| Coarse aggregate (19 mm) | 550 |
| High-Range Water Reducer | 9 |
| Fly Ash | 100 |
| Polypropylene Fibers | 1 |
| Water | 150 |
| Slump (mm) | 175 |
| Water to cementitious materials w/cm | 0.27 |
| Specimen | * | ** | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| (kN) | (mm/mm) | (mm/mm) | (kN) | (kN) | (kN) | (kN) | (kN) | (kN) | (kN) | (kN) | (kN) | |
| F06S120S | 3079 | 0.0020 | 0.0168 | 36 | 154 | 1249 | 2054 | 2636 | 3268 | 3872 | 3352 | 3463 |
| (0.05) | (0.41) | (0.67) | (0.86) | (1.06) | (1.26) | (1.09) | (1.12) | |||||
| F06S120L | 2994 | 0.0014 | 0.0065 | 13 | 89 | 1549 | 2074 | 2783 | 3026 | 3352 | 2976 | 3114 |
| (0.03) | (0.52) | (0.69) | (0.93) | (1.01) | (1.12) | (0.99) | (1.04) | |||||
| F12S100S | 3604 | 0.0018 | 0.0384 | 109 | 87 | 1057 | 2100 | 3206 | 3105 | 3507 | 3091 | 3202 |
| (0.02) | (0.29) | (0.58) | (0.89) | (0.86) | (0.97) | (0.86) | (0.89) | |||||
| F12S100L | 3774 | 0.0026 | 0.0087 | 30 | 20 | 1014 | 1889 | 3448 | 3292 | 3933 | 3395 | 3517 |
| (0.01) | (0.27) | (0.50) | (0.91) | (0.87) | (1.04) | (0.90) | (0.93) |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Eid, R.; Muravin, B.; Kovler, K. Acoustic Emission Monitoring of High-Strength Concrete Columns Subjected to Compressive Axial Loading. Materials 2020, 13, 3114. https://doi.org/10.3390/ma13143114
Eid R, Muravin B, Kovler K. Acoustic Emission Monitoring of High-Strength Concrete Columns Subjected to Compressive Axial Loading. Materials. 2020; 13(14):3114. https://doi.org/10.3390/ma13143114
Chicago/Turabian StyleEid, Rami, Boris Muravin, and Konstantin Kovler. 2020. "Acoustic Emission Monitoring of High-Strength Concrete Columns Subjected to Compressive Axial Loading" Materials 13, no. 14: 3114. https://doi.org/10.3390/ma13143114
APA StyleEid, R., Muravin, B., & Kovler, K. (2020). Acoustic Emission Monitoring of High-Strength Concrete Columns Subjected to Compressive Axial Loading. Materials, 13(14), 3114. https://doi.org/10.3390/ma13143114





