Effect of Porosity on Functional Properties of Lead-Free Piezoelectric BaZr0.15Ti0.85O3 Porous Ceramics
Abstract
1. Introduction
2. Materials and Methods
2.1. Sample Processing and Preparation
2.2. Experimental Details
3. Results and Discussion
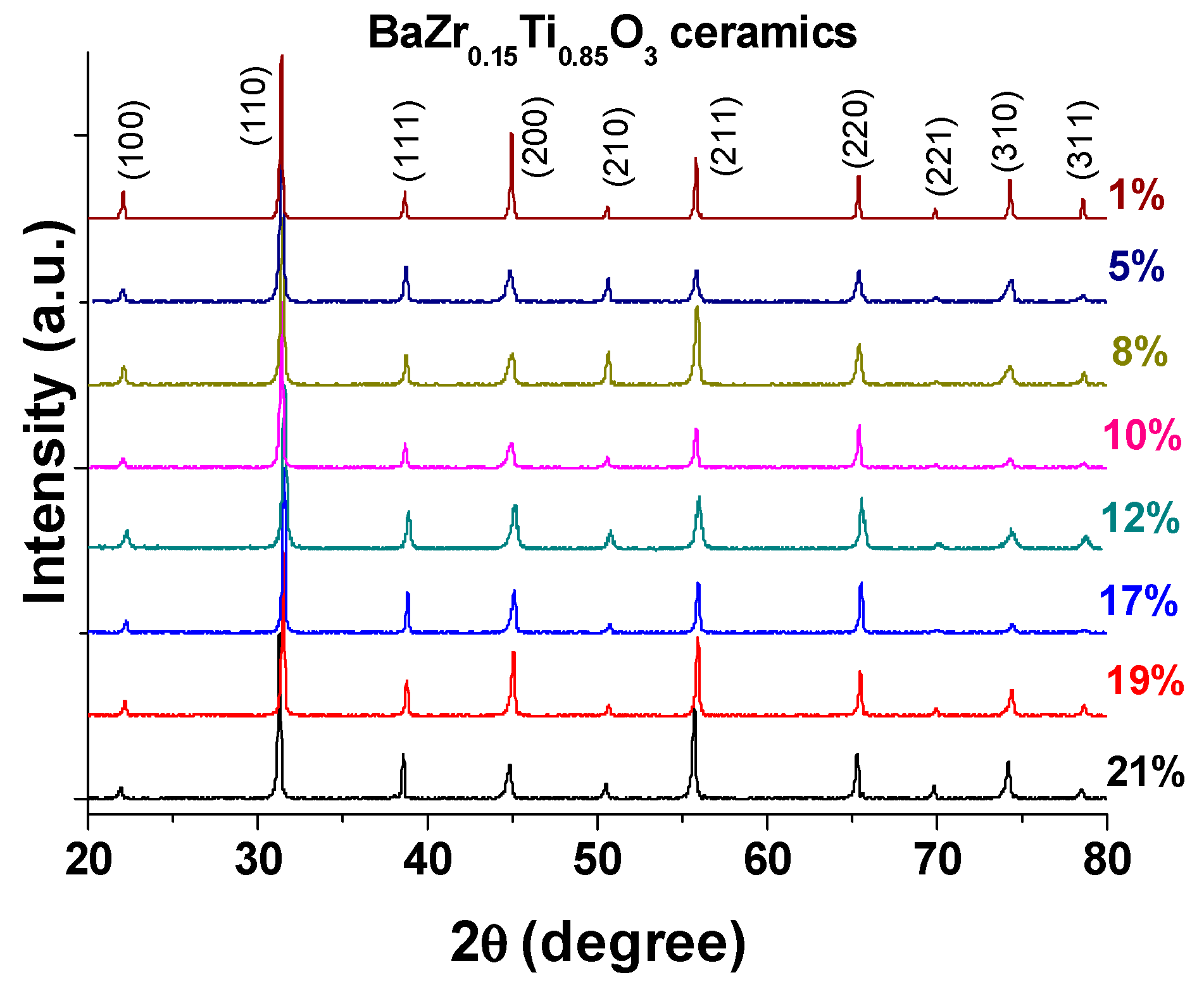
3.1. Phase and Microstructural Characterisation
3.2. Low-Field Dielectric Properties
3.3. High-Field Properties
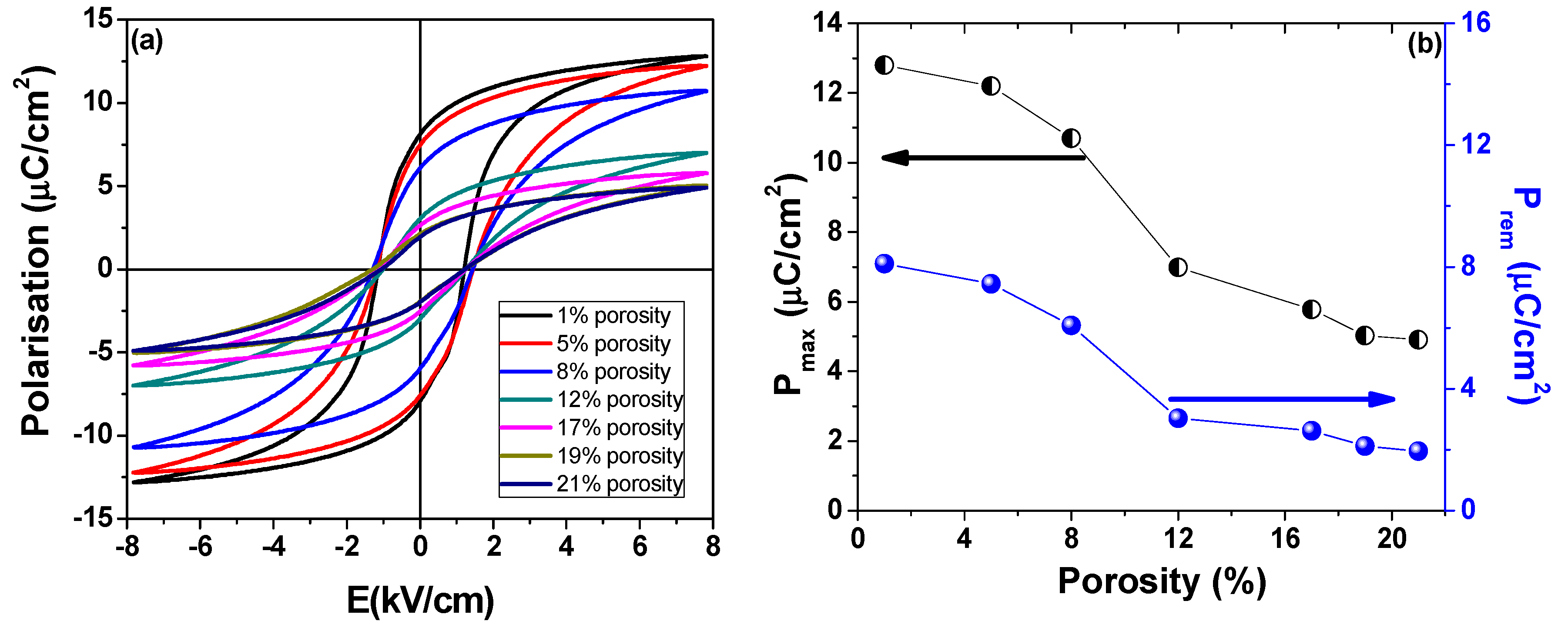
3.3.1. P(E) Hysteresis Loops
3.3.2. DC Tunability
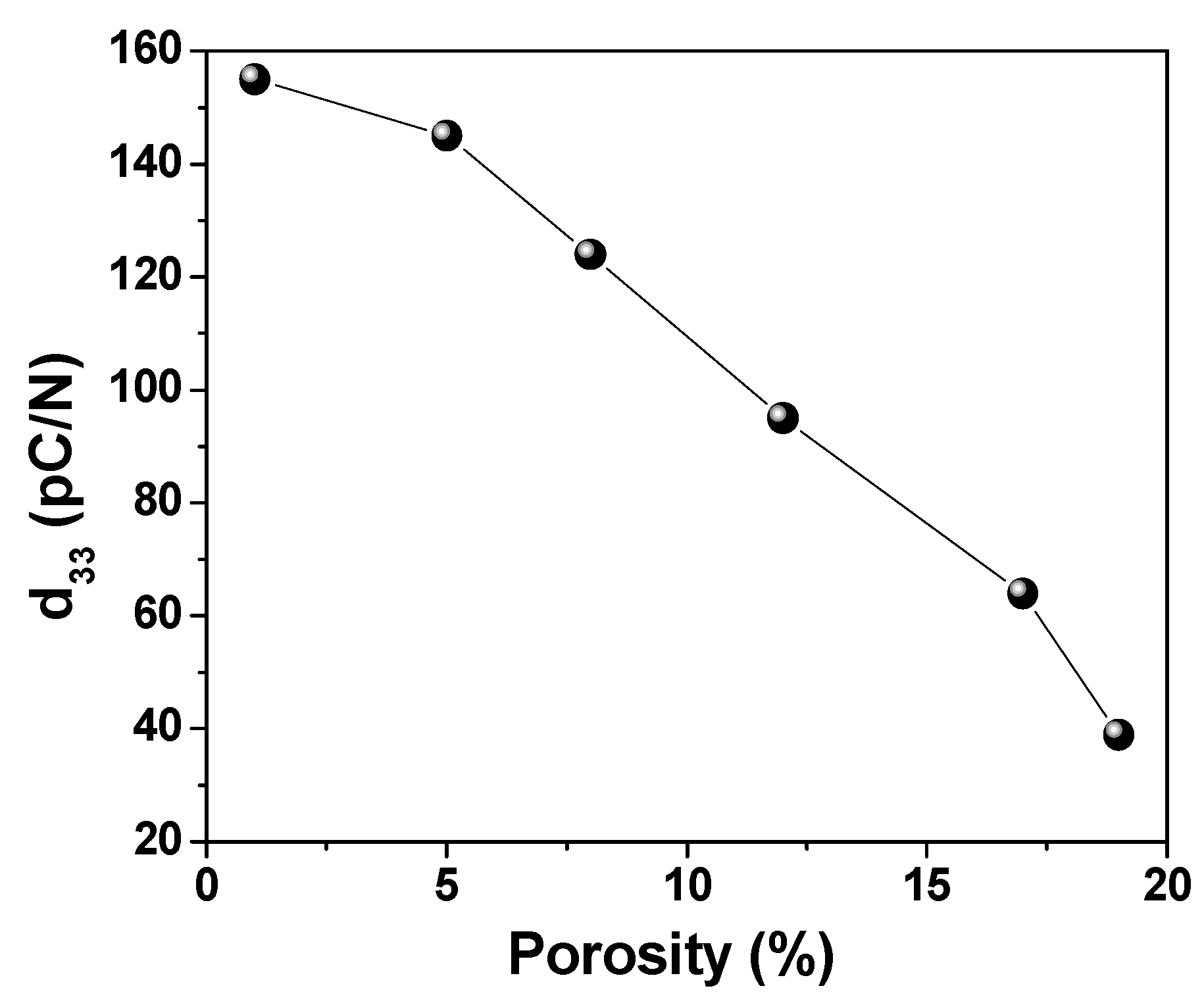
3.3.3. Piezoelectric Characteristics
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Xu, Y. Ferroelectric Materials and Their Applications; Elsevier: North Holland, The Netherlands, 1991. [Google Scholar]
- Khachaturyan, R.; Zhukov, S.; Schultheiß, J.; Galassi, C.; Reimuth, C.; Koruza, J.; Seggern, H.V.; Genenko, Y.A. Polarization-switching dynamics in bulk ferroelectrics with isometric and oriented anisometric pores. J. Phys. Appl. Phys. 2016, 50, 045303. [Google Scholar] [CrossRef]
- Kar-Gupta, R.; Venkatesh, T.A. Electromechanical response of porous piezoelectric materials. Acta Mater. 2006, 54, 4063–4078. [Google Scholar] [CrossRef]
- Kar-Gupta, R.; Venkatesh, T.A. Electromechanical response of piezoelectric composites: Effects of geometric connectivity and grain size. Acta Mater. 2008, 56, 3810–3823. [Google Scholar] [CrossRef]
- Zhang, Y.; Xie, M.; Roscow, J.; Bao, Y.; Zhou, K.; Zhang, D.; Bowen, C.R. Enhanced pyroelectric and piezoelectric properties of PZT with aligned porosity for energy harvesting applications. J. Mater. Chem. 2017, 5, 6569–6580. [Google Scholar] [CrossRef] [PubMed]
- Castro, A.; Ferreira, P.; Rodriguez, B.J.; Vilarinho, P.M. The role of nanoporosity on the local piezo and ferroelectric properties of lead titanate thin films. J. Mater. Chem. C 2015, 3, 1035–1043. [Google Scholar] [CrossRef]
- Okazaki, K.; Nagata, K. Effects of grain size and porosity on electrical and optical properties of PLZT ceramics. J. Am. Ceram. Soc. 1973, 56, 82–86. [Google Scholar] [CrossRef]
- Bakaric, T.; Rojac, T.; Abellard, A.P.; Malic, B.; Levassort, F.; Kuscer, D. Effect of pore size and porosity on piezoelectric and acoustic properties of Pb(Zr0.53Ti0.47)O3 ceramics. Adv. Appl. Ceram. 2016, 115, 66–71. [Google Scholar] [CrossRef]
- Padurariu, C.; Padurariu, L.; Curecheriu, L.; Ciomaga, C.; Horchidan, N.; Galassi, C.; Mitoseriu, L. Role of the pore interconnectivity on the dielectric, switching and tunability properties of PZTN ceramics. Ceram. Int. 2017, 43, 5767–5773. [Google Scholar] [CrossRef]
- Ming, C.; Yang, T.; Luan, K.; Chen, L.; Wang, L.; Zeng, J.; Li, Y.; Zhang, W.; Chen, L.-Q. Microstructural effects on effective piezoelectric responses of textured PMN-PT ceramics. Acta Mater. 2018, 145, 62–70. [Google Scholar] [CrossRef]
- Geis, S.; Fricke, J.; Lobmann, P. Electrical properties of PZT aerogels. J. Eur. Ceram. Soc. 2002, 22, 1155–1161. [Google Scholar] [CrossRef]
- Stancu, V.; Lisca, M.; Boerasu, I.; Pintilie, L.; Kosec, M. Effects of porosity on ferroelectric properties of Pb(Zr0.2Ti0.8)O3 films. Thin Solid Films 2007, 515, 6557–6561. [Google Scholar] [CrossRef]
- Bosse, P.W.; Challagulla, K.S.; Venkatesh, T.A. Effects of foam shape and porosity aspect ratio on the electromechanical properties of 3-3 piezoelectric foams. Acta Mater. 2012, 60, 6464–6475. [Google Scholar] [CrossRef]
- Challagulla, K.S.; Venkatesh, T.A. Electromechanical response of piezoelectric foams. Acta Mater. 2012, 60, 2111–2127. [Google Scholar] [CrossRef]
- Jiang, Q.Y.; Cross, L.E. Effects of porosity on electric fatigue behaviour in PLZT and PZT ferroelectric ceramics. J. Mater. Sci. 1993, 28, 4536–4543. [Google Scholar] [CrossRef]
- Padurariu, L.; Curecheriu, L.P.; Mitoseriu, L. Nonlinear dielectric properties of paraelectric-dielectric composites described by a 3D Finite Element Method based on Landau-Devonshire theory. Acta Mater. 2016, 103, 724–734. [Google Scholar] [CrossRef]
- Padurariu, L.; Curecheriu, L.; Galassi, C.; Mitoseriu, L. Tailoring non-linear dielectric properties by local field engineering in anisotropic porous ferroelectric structures. Appl. Phys. Lett. 2012, 100, 252905. [Google Scholar] [CrossRef]
- Wang, W.; Wang, L.D.; Li, W.L.; Xu, D.; Hou, Y.F.; Fei, W.D. Effect of pore content on diffuse phase transition behaviour of porous 0.5 BaZr0.2Ti0.8O3- 0.5 Ba0.7Ca0.3TiO3 ceramics. J. Alloy Comp. 2015, 624, 284–289. [Google Scholar] [CrossRef]
- Stanculescu, R.; Ciomaga, C.E.; Padurariu, L.; Galizia, P.; Horchidan, N.; Capiani, C.; Galassi, C.; Mitoseriu, L. Study of the role of porosity on the functional properties of (Ba,Sr)TiO3 ceramics. J. Alloy Comp. 2015, 643, 79–87. [Google Scholar] [CrossRef]
- Stanculescu, R.; Horchidan, N.; Galassi, C.; Asandulesa, M.; Padurariu, L.; Ciomaga, C.E.; Mitoseriu, L. Porous (Ba,Sr)TiO3 ceramics for tailoring dielectric and tunability properties: Modelling and experiment. Proc. Appl. Ceram. 2017, 11, 235–246. [Google Scholar] [CrossRef]
- Ye, Z.-G. Handbook of Advanced Dielectric, Piezoelectric and Ferroelectric Materials: Synthesis, Properties and Applications; Ye, Z.-G., Ed.; Elsevier: Sawston Cambridge, UK, 2008. [Google Scholar]
- Dobal, P.S.; Dixit, A.; Katiyar, R.S.; Yu, Z.; Guo, R.; Bhalla, A.S. Micro-Raman scattering and dielectric investigations of phase transitions behavior in the BaTiO3-BaZrO3 system. J. Appl. Phys. 2001, 89, 8085. [Google Scholar] [CrossRef]
- Canu, G.; Confalonieri, G.; Deluca, M.; Curecheriu, L.; Buscaglia, M.T.; Asandulesa, M.; Horchidan, N.; Dapiaggi, M.; Mitoseriu, L.; Buscaglia, V. Structure-property correlations and origin of relaxor behaviour in BaCexTi1-xO3. Acta Mater. 2018, 152, 258–268. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, G.; Zeng, T.; Liang, R.; Dong, X. Electric field-dependent dielectric properties and high tunbaility of porous Ba0.5Sr0.5TiO3 ceramics. J. Am. Ceram. Soc. 2007, 90, 1327–1330. [Google Scholar] [CrossRef]
- Tufescu, F.M.; Curecheriu, L.; Ianculescu, A.; Ciomaga, C.E.; Mitoseriu, L. High-voltage tunability measurements of the BaZrxTi1-xO3 ferroelectric ceramics. J. Optoel. Adv. Mater. 2008, 10, 1894. [Google Scholar]
- Laulhe, C.; Hippert, F.; Kreisel, J.; Maglione, M.; Simon, A.; Hazemann, J.L.; Nassif, V. EXAFS study of lead-free relaxor ferroelectric of BaTi1-xZrxO3 at Zr K edge. Phys. Rev. B 2006, 74, 014106. [Google Scholar] [CrossRef]
- Mao, C.; Yan, S.; Cao, S.; Yao, C.; Cao, F.; Wang, G.; Dong, X.; Hu, X.; Yang, C. Effect of grain size on phase transition, dielectric and pyroelectric properties of BST ceramics. J. Eur. Ceram. Soc. 2014, 34, 2933–2939. [Google Scholar] [CrossRef]
- Gheorghiu, F.; Gheorghiu, F.; Padurariu, L.; Airimioaei, M.; Curecheriu, L.; Ciomaga, C.E.; Padurariu, C.; Galassi, C.; Mitoseriu, L. Porosity dependent properties of Nb-doped Pb(Zr,Ti)O3 ceramics. J. Am. Ceram. Soc. 2017, 100, 647–658. [Google Scholar] [CrossRef]
- Mahesh, M.L.V.; Bhanu Prasad, V.V.; James, A.R. Enhanced dielectric and ferroelectric properties of the lead-free Ba(Zr0.15Ti0.85)O3 ceramics compacted by cold isostatic pressing. J. Alloy Compd. 2014, 611, 43–49. [Google Scholar] [CrossRef]
- Deluca, M.; Vasilescu, C.A.; Ianculescu, A.C.; Berger, D.C.; Ciomaga, C.E.; Curecheriu, L.P.; Stoleriu, L.; Gajovic, A.; Mitoseriu, L.; Galassi, C. Investigation of the composition-dependent properties of BaTi1-xZrxO3 ceramics prepared by the modified Pechini method. J. Eur. Ceram. Soc. 2012, 32, 3551–3566. [Google Scholar] [CrossRef]
- Yu, Z.; Ang, C.; Guo, R.; Bhalla, A.S. Piezoelectric and strain properties of Ba(Ti1-xZrx)O3 ceramics. J. Appl. Phys. 2002, 92, 1489–1492. [Google Scholar] [CrossRef]






| Sample | Tm (°C) | T0 (°C) | εm | C (×105 °C) |
|---|---|---|---|---|
| 1% porosity | 60 | 66 | 27,363 | 1.49 |
| 5% porosity | 62.5 | 67 | 30,714 | 1.45 |
| 8% porosity | 62.5 | 67 | 20,469 | 1.33 |
| 10% porosity | 62.5 | 62 | 12,972 | 1.23 |
| 12% porosity | 62.5 | 65 | 12,779 | 1.12 |
| 17% porosity | 62.5 | 66 | 12,557 | 1.06 |
| 19% porosity | 65 | 67 | 13,175 | 0.92 |
| 21% porosity | 60 | 69 | 12,971 | 0.92 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Curecheriu, L.; Lukacs, V.A.; Padurariu, L.; Stoian, G.; Ciomaga, C.E. Effect of Porosity on Functional Properties of Lead-Free Piezoelectric BaZr0.15Ti0.85O3 Porous Ceramics. Materials 2020, 13, 3324. https://doi.org/10.3390/ma13153324
Curecheriu L, Lukacs VA, Padurariu L, Stoian G, Ciomaga CE. Effect of Porosity on Functional Properties of Lead-Free Piezoelectric BaZr0.15Ti0.85O3 Porous Ceramics. Materials. 2020; 13(15):3324. https://doi.org/10.3390/ma13153324
Chicago/Turabian StyleCurecheriu, Lavinia, Vlad Alexandru Lukacs, Leontin Padurariu, George Stoian, and Cristina Elena Ciomaga. 2020. "Effect of Porosity on Functional Properties of Lead-Free Piezoelectric BaZr0.15Ti0.85O3 Porous Ceramics" Materials 13, no. 15: 3324. https://doi.org/10.3390/ma13153324
APA StyleCurecheriu, L., Lukacs, V. A., Padurariu, L., Stoian, G., & Ciomaga, C. E. (2020). Effect of Porosity on Functional Properties of Lead-Free Piezoelectric BaZr0.15Ti0.85O3 Porous Ceramics. Materials, 13(15), 3324. https://doi.org/10.3390/ma13153324







