Mechanical Performance of Metallic Bone Screws Evaluated Using Bone Models
Abstract
:1. Introduction
2. Experimental Method
2.1. Metallic Medical Bone Screws
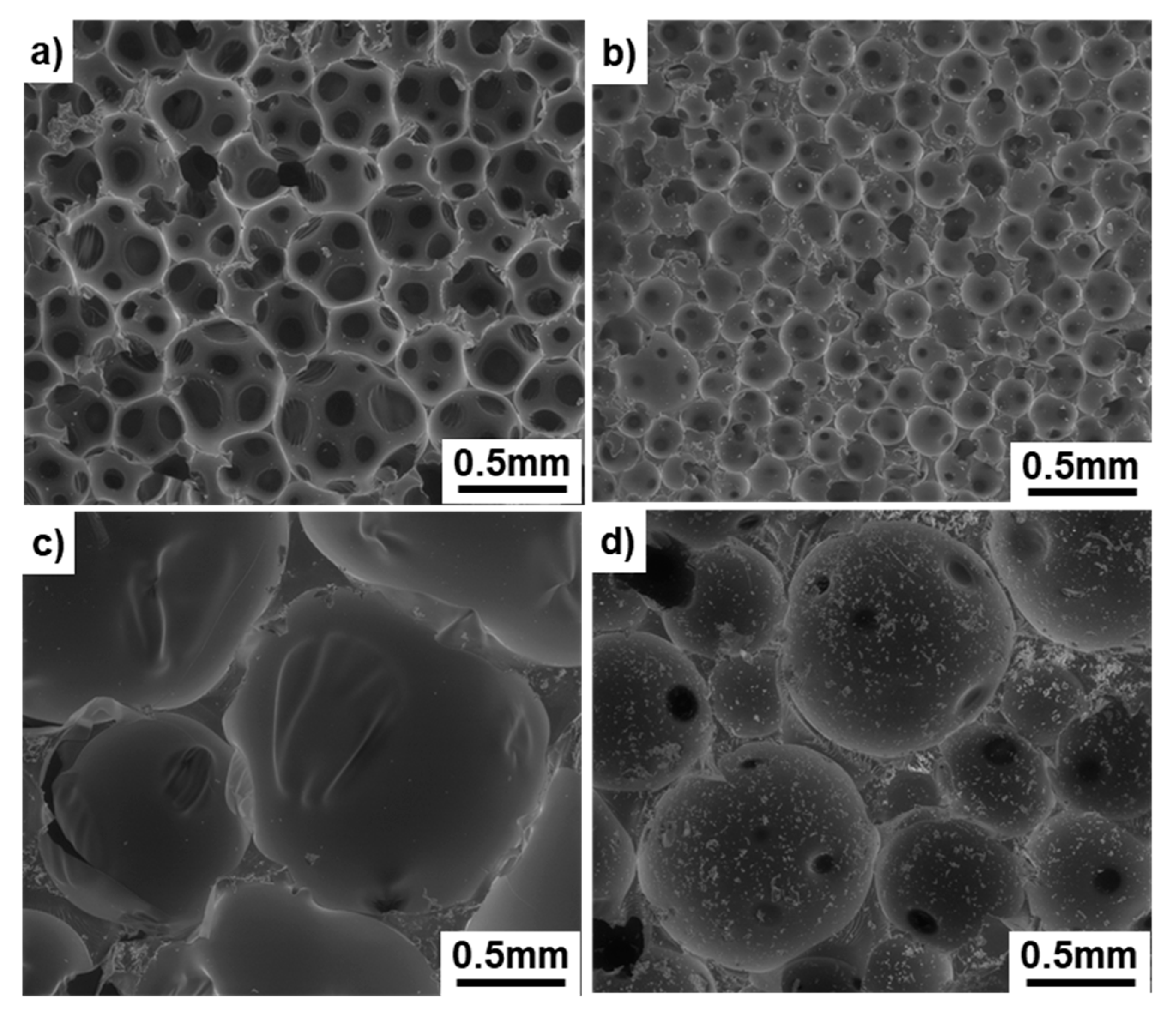
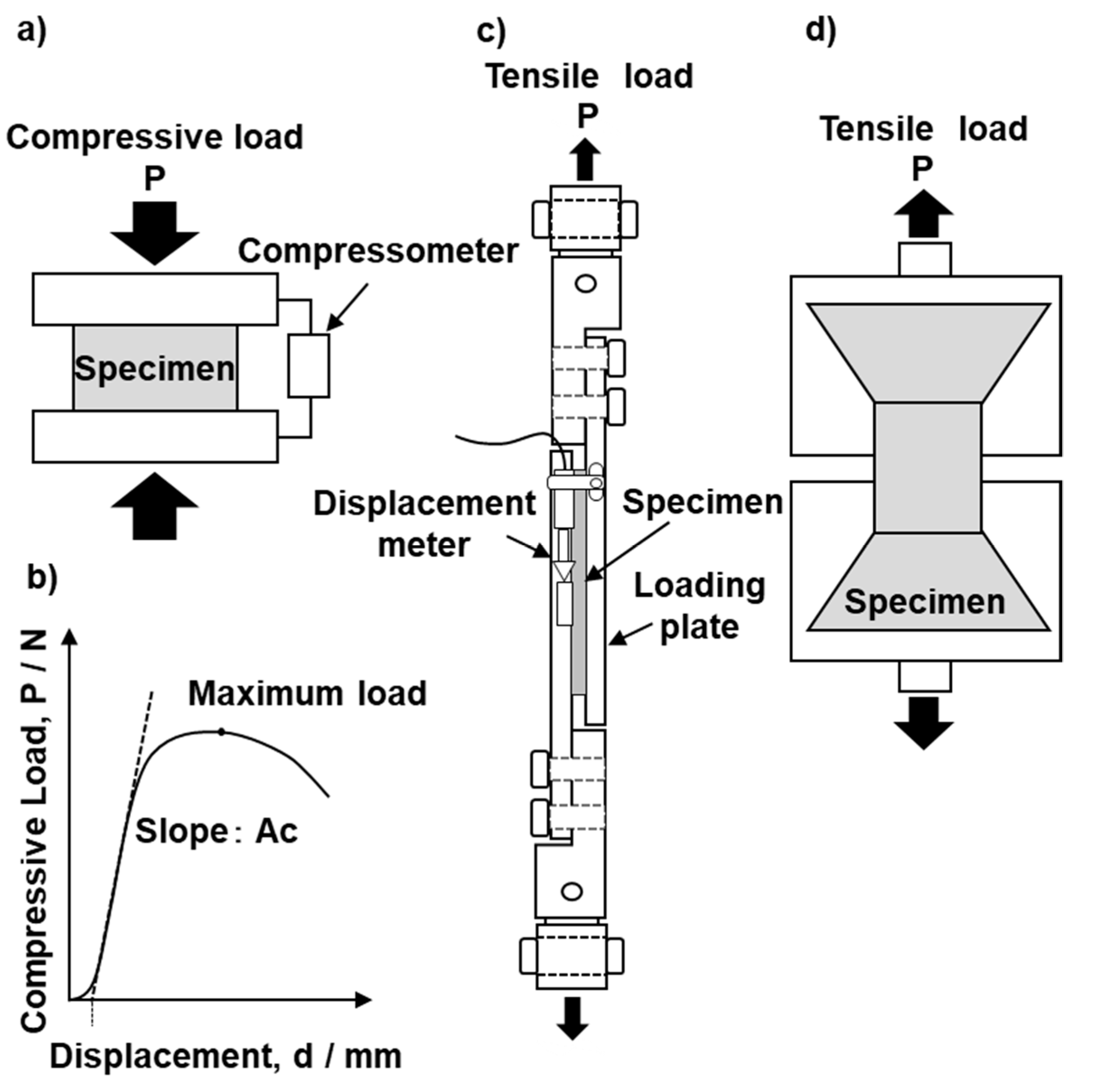
2.2. Mechanical Tests of Bone Models
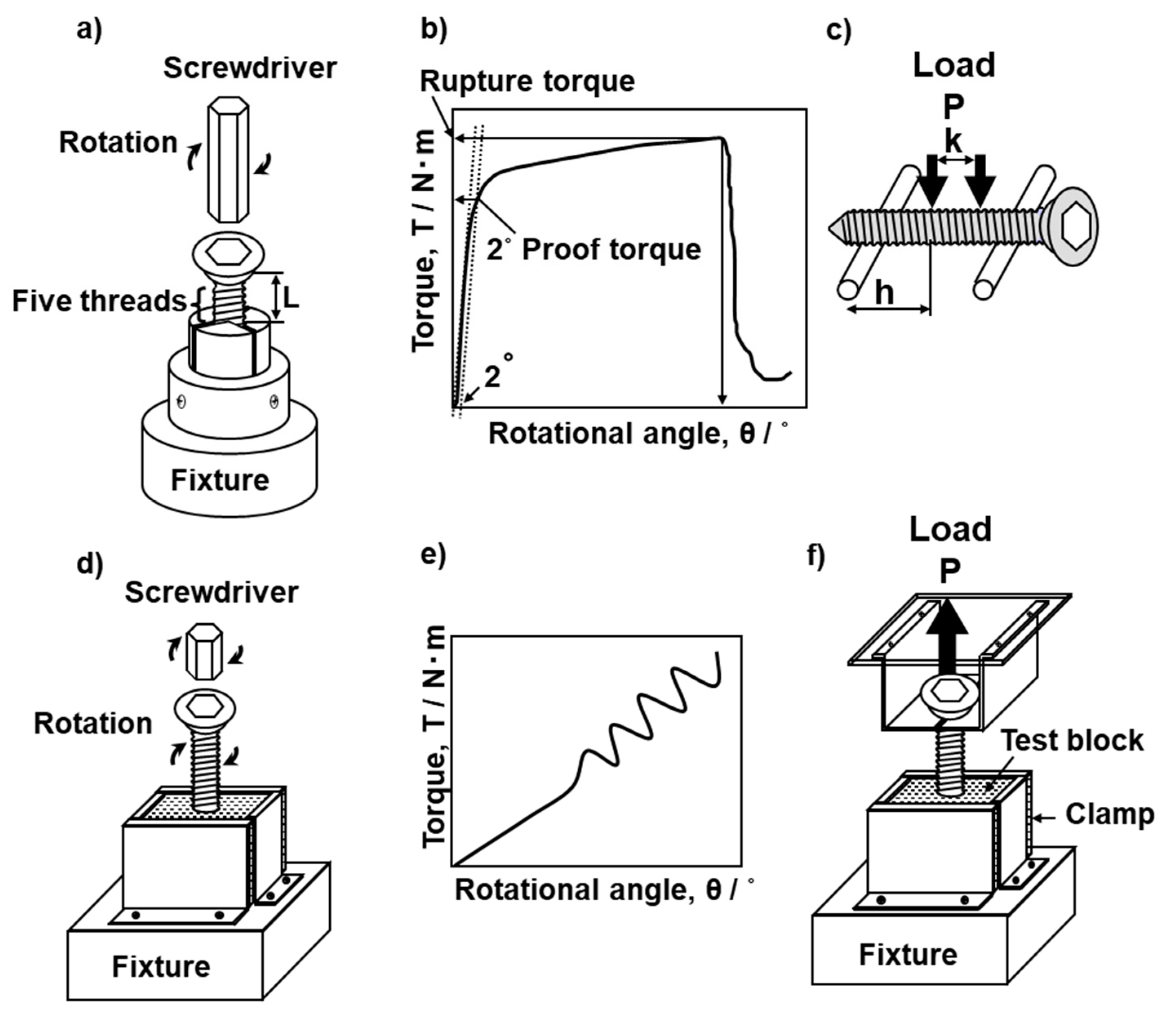
2.3. Mechanical Performance Tests of Bone Screws
2.4. Driving Torque and Screw Pullout Tests
2.5. Statistical Analysis
3. Results and Discussion
3.1. Mechanical Properties of Metallic Screws
3.2. Mechanical Properties of Bone Models
3.3. Screw Driving Torque and Pullout Properties
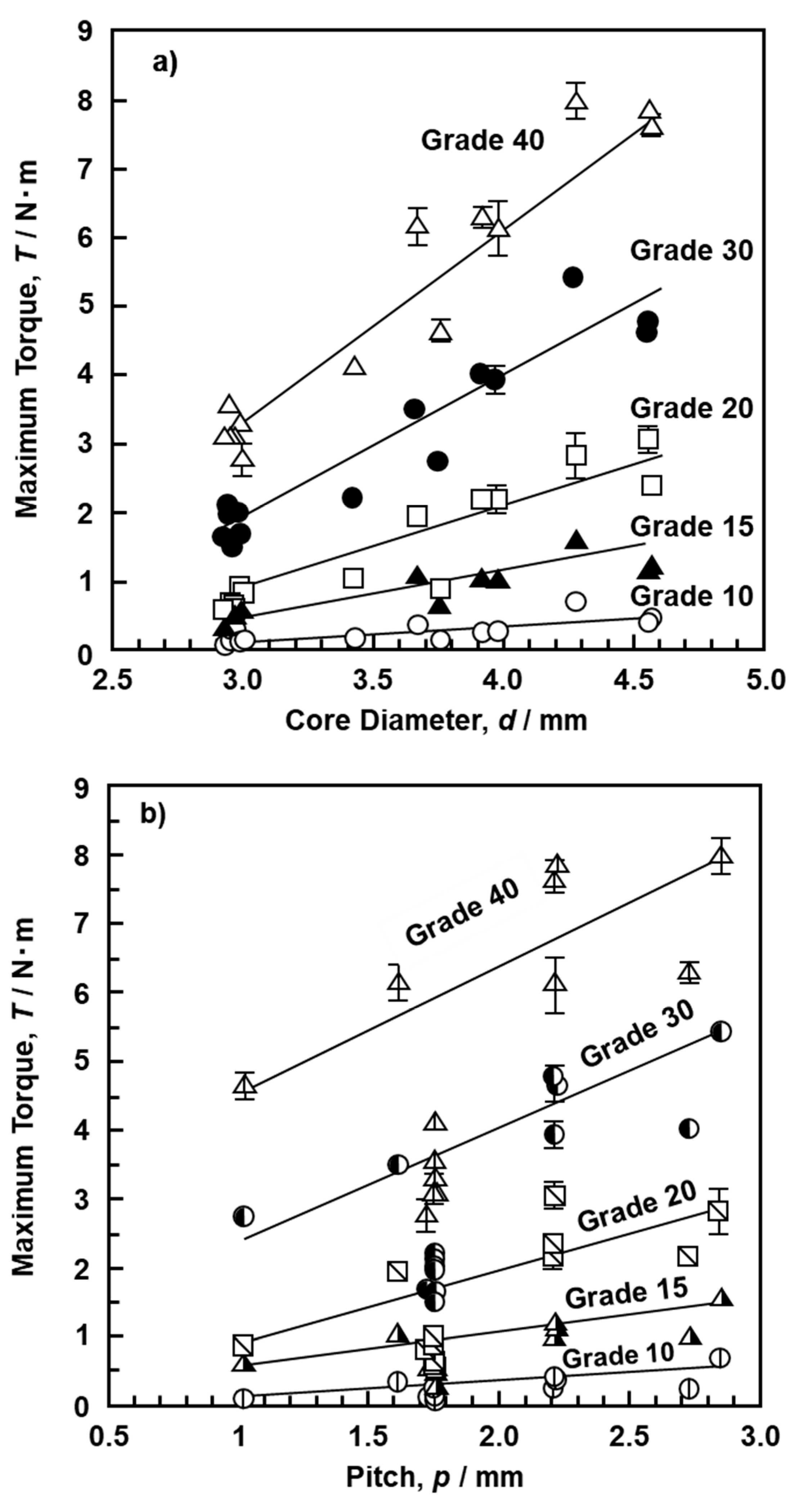
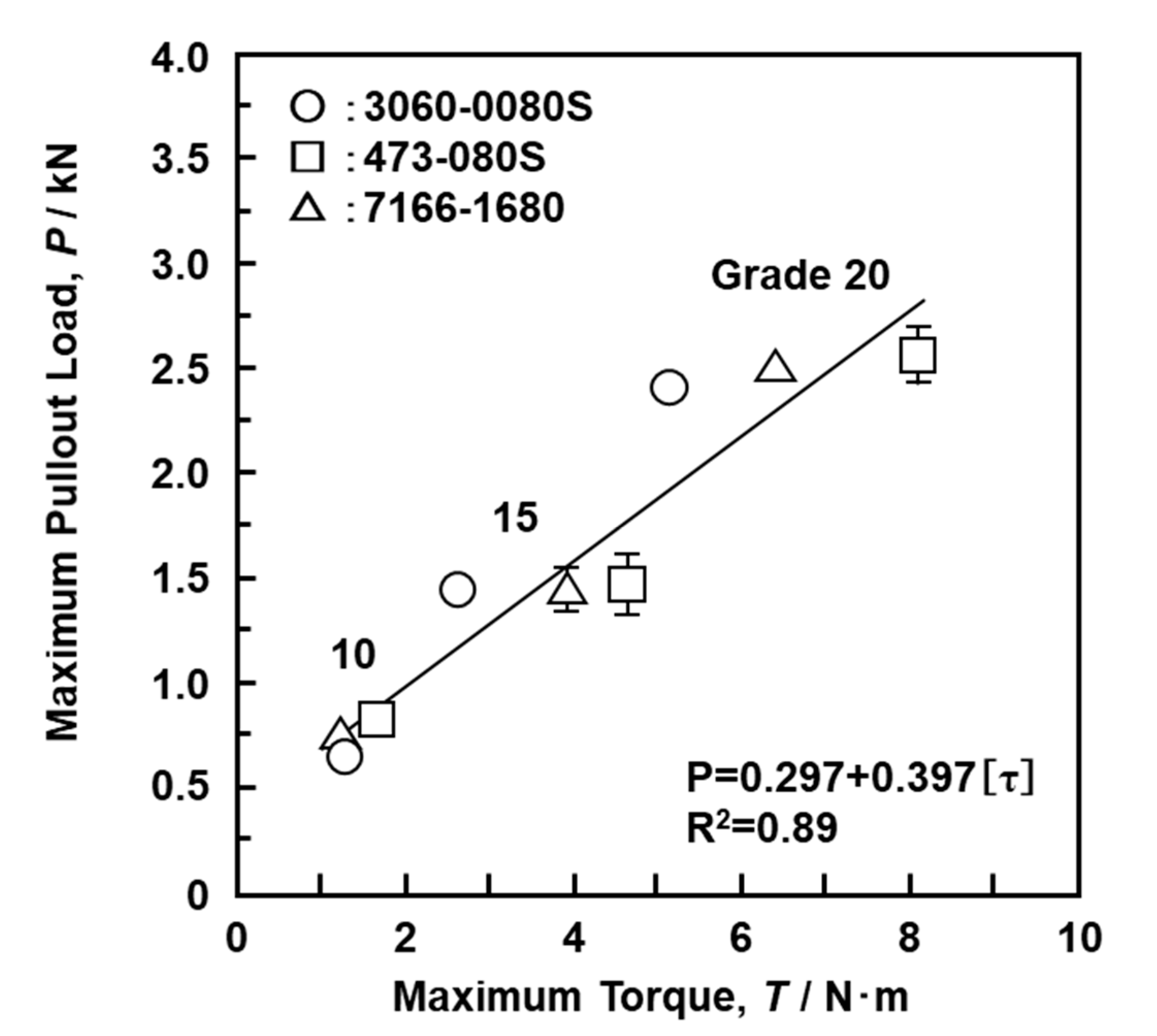
- Grade 15 SRPF: T = 0.00841S – 0.270, R2 = 0.93
- Grade 20 SRPF: T = 0.0209S – 1.168, R2 = 0.95
- Grade 20 CRPF: T = 0.0101S – 0.591, R2 = 0.93
4. Conclusions
- (1)
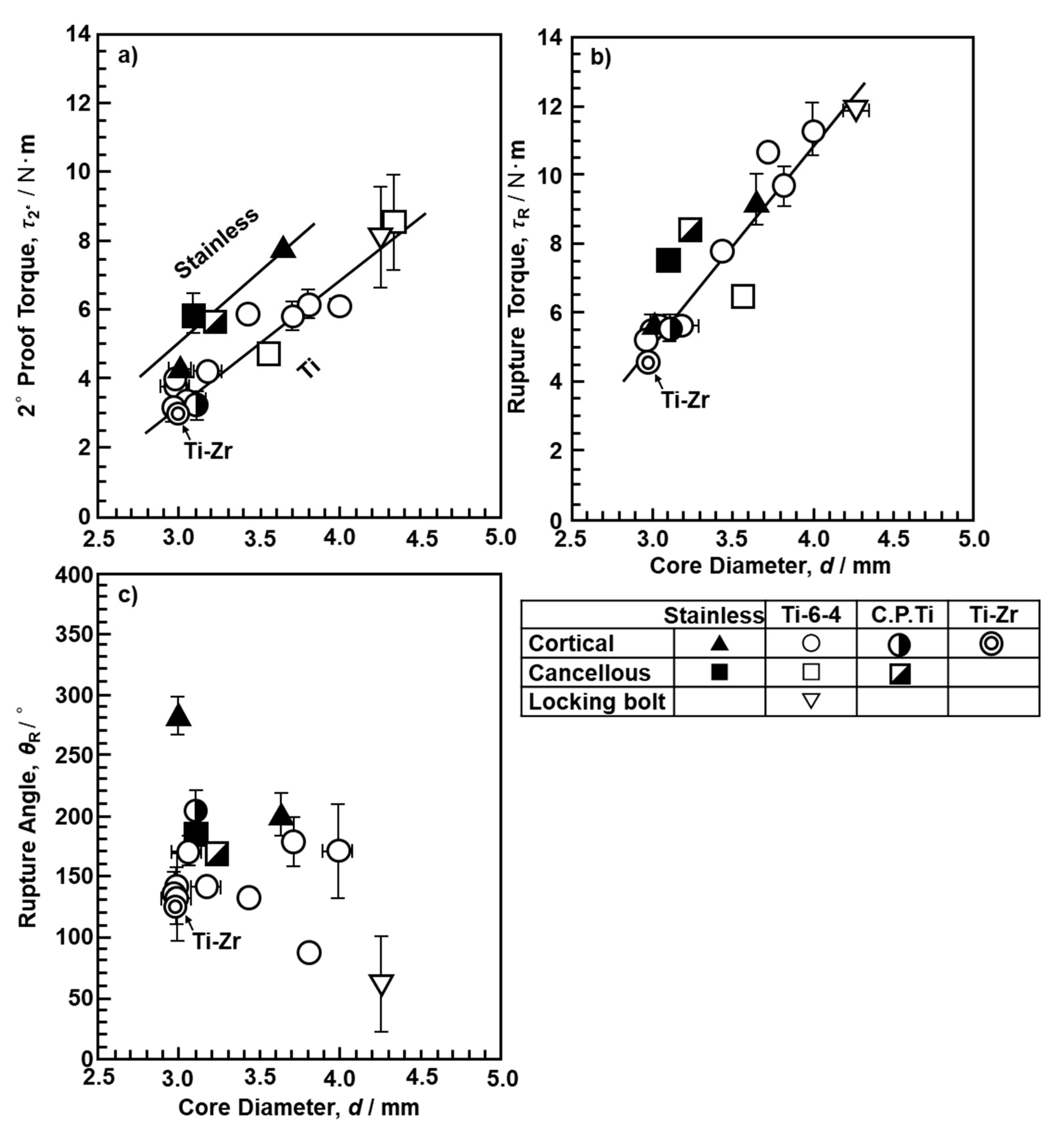
- The 2° yield torque and rupture torque obtained by the torsional breaking test of metallic screws increased with the core diameter of the screws. Using the maximum torsional moment and rupture angle, we estimated the maximum surface shearing stress (τmax) and torsional rigidity (G) for cortical, cancellous, and locking bolt screws.
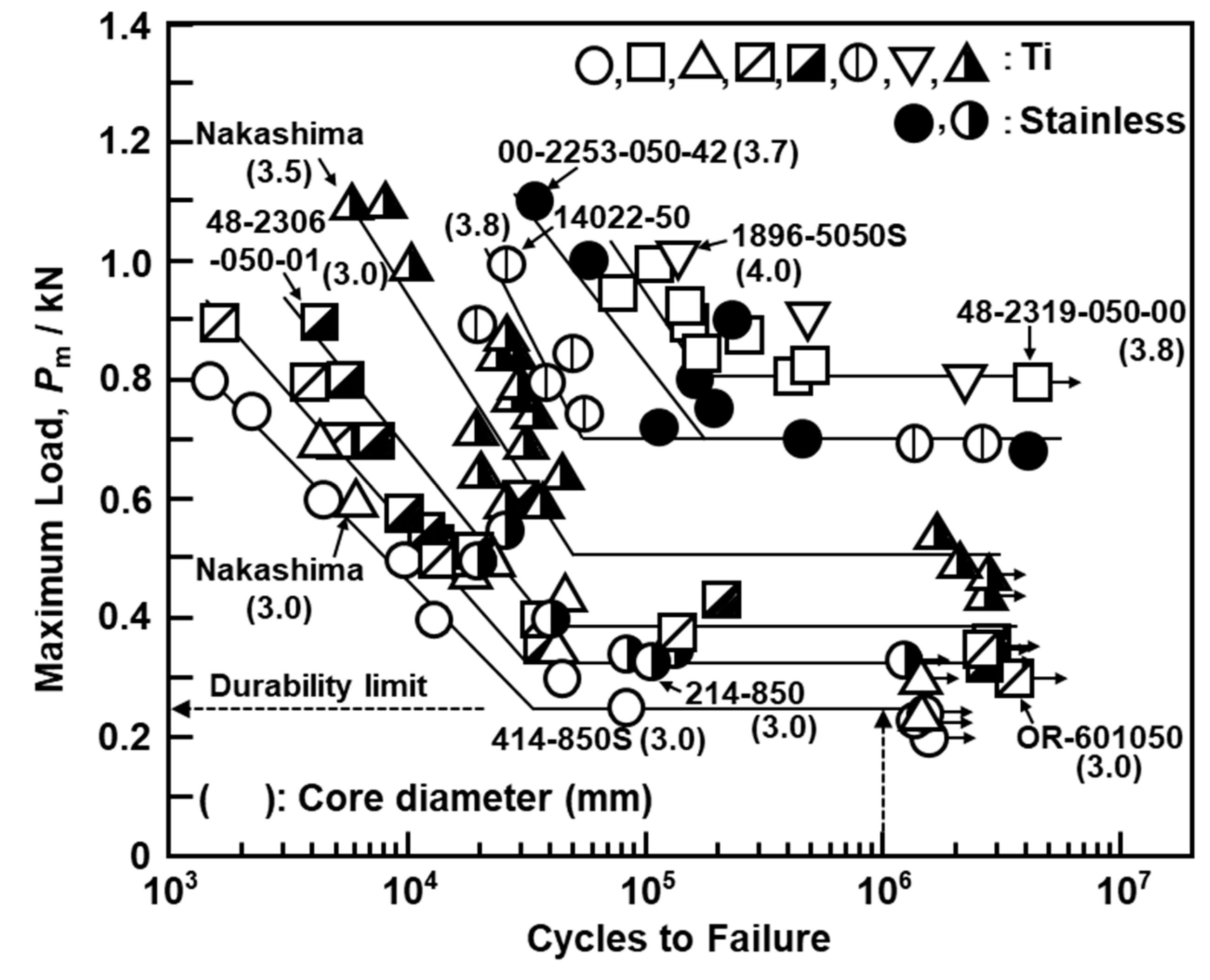
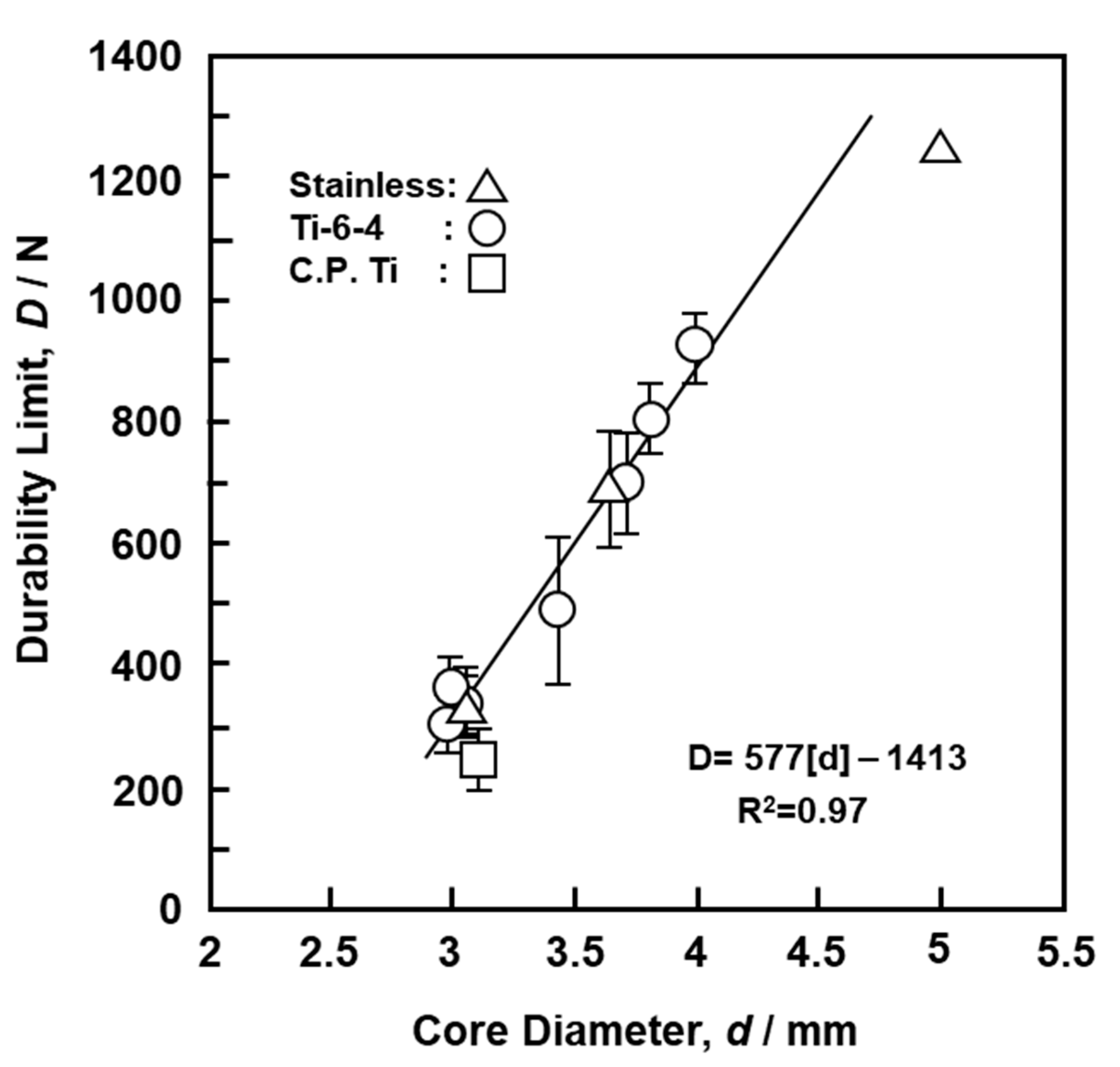
- (2)
- The durability limit of screws increased with increasing core diameter. The maximum surface stress (σD) values of C.P. Ti, Ti-6-4 alloy, and stainless-steel screws calculated from the durability limit were 69, 82, and 70% of the ultimate tensile strength (σUTS) for osteosynthesis devices, respectively.
- (3)
- The compressive, tensile, and shear strengths of the bone model increased with increasing density of the bone model. The strength and modulus obtained for SRPF and CRPF lay on the same straight line. Among the three strengths, the rate of increase in compressive strength with the increase in density was the highest.
- (4)
- The maximum driving torque increased linearly with increasing effective surface area of the screws.
- (5)
- The maximum pullout load of the screws increased linearly with increasing density of the bone model and number of rotations. Screws with low driving torque and high pullout load were considered to have excellent fixation and are a target for development.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Okazaki, Y.; Gotoh, E.; Mori, J. Strength–durability correlation of osteosynthesis devices made by 3D layer manufacturing. Materials 2019, 12, 436. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kincaid, B.; Schroder, L.; Mason, L. Measurement of orthopedic cortical bone screw insertion performance in cadaver bone and model materials. Exp. Mech. 2007, 47, 595–607. [Google Scholar] [CrossRef]
- Pena, F.; Grontvedt, T.; Brown, G.A.; Aune, A.K.; Engebretsen, L. Comparison of failure strength between metallic and absorbable interference screws. Am. J. Sports Med. 1996, 24, 329–334. [Google Scholar] [CrossRef] [PubMed]
- ASTM. Standard Specification and Test. Methods for Metallic Medical Bone Screws; ASTM F 543; ASTM International: West Conshohocken, PA, USA, 2009. [Google Scholar]
- JIS. Mechanical Testing Methods for Metallic Bone Screws; JIS T 0311; Japanese Standards Association: Tokyo, Japan, 2020. [Google Scholar]
- ASTM. Standard Specification for Rigid Polyurethane Foam for Use as a Standard Material for Testing Orthopaedic Devices and Instruments; ASTM F 1839; ASTM International: West Conshohocken, PA, USA, 2016. [Google Scholar]
- ASTM. Standard Test. Method for Compressive Properties of Rigid Cellular Plastics; ASTM D 1621; ASTM International: West Conshohocken, PA, USA, 2000. [Google Scholar]
- ASTM. Standard Test. Method for Shear Properties of Sandwich Core Materials; ASTM C 273/C273M; ASTM International: West Conshohocken, PA, USA, 2011. [Google Scholar]
- ASTM. Standard Test. Method for Tensile and Tensile Adhesion Properties of Rigid Cellular Plastic; ASTM D 1623; ASTM International: West Conshohocken, PA, USA, 2003. [Google Scholar]
- ASTM. Standard Test. Method for Tensile Properties of Plastics; ASTM D 638; ASTM International: West Conshohocken, PA, USA, 2014. [Google Scholar]
- ISO. Implants for Surgery—Metallic Materials—Part. 1: Wrought Stainless steel; ISO 5832-1; International Organization for Standardization: Geneva, Switzerland, 2016. [Google Scholar]
- ISO. Implants for Surgery—Metallic Materials—Part. 2: Unalloyed Titanium; ISO 5832-2; International Organization for Standardization: Geneva, Switzerland, 2018. [Google Scholar]
- ISO. Implants for Surgery—Metallic Materials—Part. 3: Wrought Titanium 6-Aluminium 4-Vanadium Alloy; ISO 5832-3; International Organization for Standardization: Geneva, Switzerland, 2016. [Google Scholar]
- Okazaki, Y. On the effects of hot forging and hot rolling on the microstructural development and mechanical response of a biocompatible Ti alloy. Materials 2012, 5, 1439–1461. [Google Scholar] [CrossRef]
- Okazaki, Y. Comparison of fatigue properties and fatigue crack growth rates of various implantable metals. Materials 2012, 5, 2981–3005. [Google Scholar] [CrossRef] [Green Version]
- Okazaki, Y.; Gotoh, E. Metal ion effects on different types of cell line, metal ion incorporation into L929 and MC3T3-E1 cells, and activation of macrophage-like J774.1 cells. Mater. Sci. Eng. C 2013, 33, 1993–2001. [Google Scholar] [CrossRef] [PubMed]
- Okazaki, Y.; Gotoh, E. Comparison of fatigue strengths of biocompatible Ti-15Zr-4Nb-4Ta alloy and other titanium materials. Mater. Sci. Eng. C 2011, 31, 325–333. [Google Scholar] [CrossRef]
- Okazaki, Y. Development of low-cost manufacturing process and effects of adding small amounts of Ta, O, and N on the physical and mechanical properties of highly biocompatible Ti alloys. Mater. Trans. 2019, 60, 1769–1778. [Google Scholar] [CrossRef]
- JIS. Titanium Materials for Surgical Implant. Applications Part. 4: Wrought Titanium 15-Zirconium 4-Niobium Alloy; JIS T 7401-4; Japanese Standards Association: Tokyo, Japan, 2020. [Google Scholar]
- ASTM. Test. Method for Apparent Density of Rigid Cellular Plastics; ASTM D 1622; ASTM International: West Conshohocken, PA, USA, 2020. [Google Scholar]
- ASTM. Standard Guide for Preparation of Metal. Surfaces for Adhesive Bonding; ASTM D 2651; ASTM International: West Conshohocken, PA, USA, 2016. [Google Scholar]
- JIS. Testing Methods for Bending Properties of Metallic Osteosynthesis; JIS T 0312; Japanese Standards Association: Tokyo, Japan, 2020. [Google Scholar]
- JSMS Committee on Fatigue and Reliability Engineering. Standard Evaluation Method of Fatigue Reliability for Metallic Materials—Standard Regression Method of SN Curves; JSMS-SD-06-08; JSMS Committee on Fatigue and Reliability Engineering: Tokyo, Japan, 2008. [Google Scholar]
- Kuroki, K.; Tomota, Y. Material Mechanics; Morikita Publishing: Tokyo, Japan, 2014. [Google Scholar]
- ISO. Implants for Surgery–Metal. Bone Screws with Conical Under-Surface of Head–Dimensions; ISO 9268; International Organization for Standardization: Geneva, Switzerland, 1968. [Google Scholar]
- Yakacki, C.M.; Griffis, J.; Poukalova, M.; Gall, K. Bearing area: A new indication for suture anchor pullout strength? J. Orthop. Res. 2009, 27, 1048–1054. [Google Scholar] [CrossRef] [PubMed]
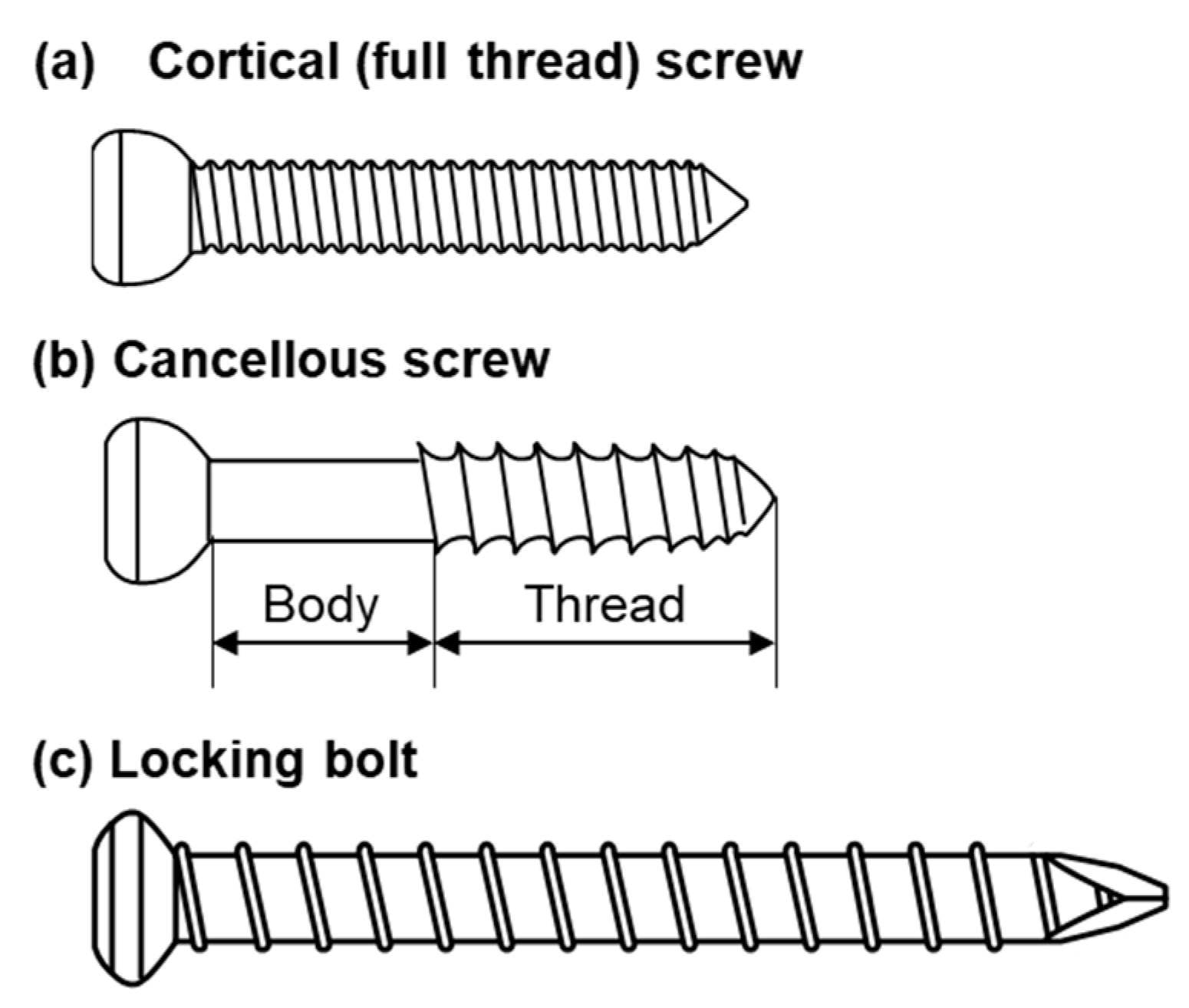

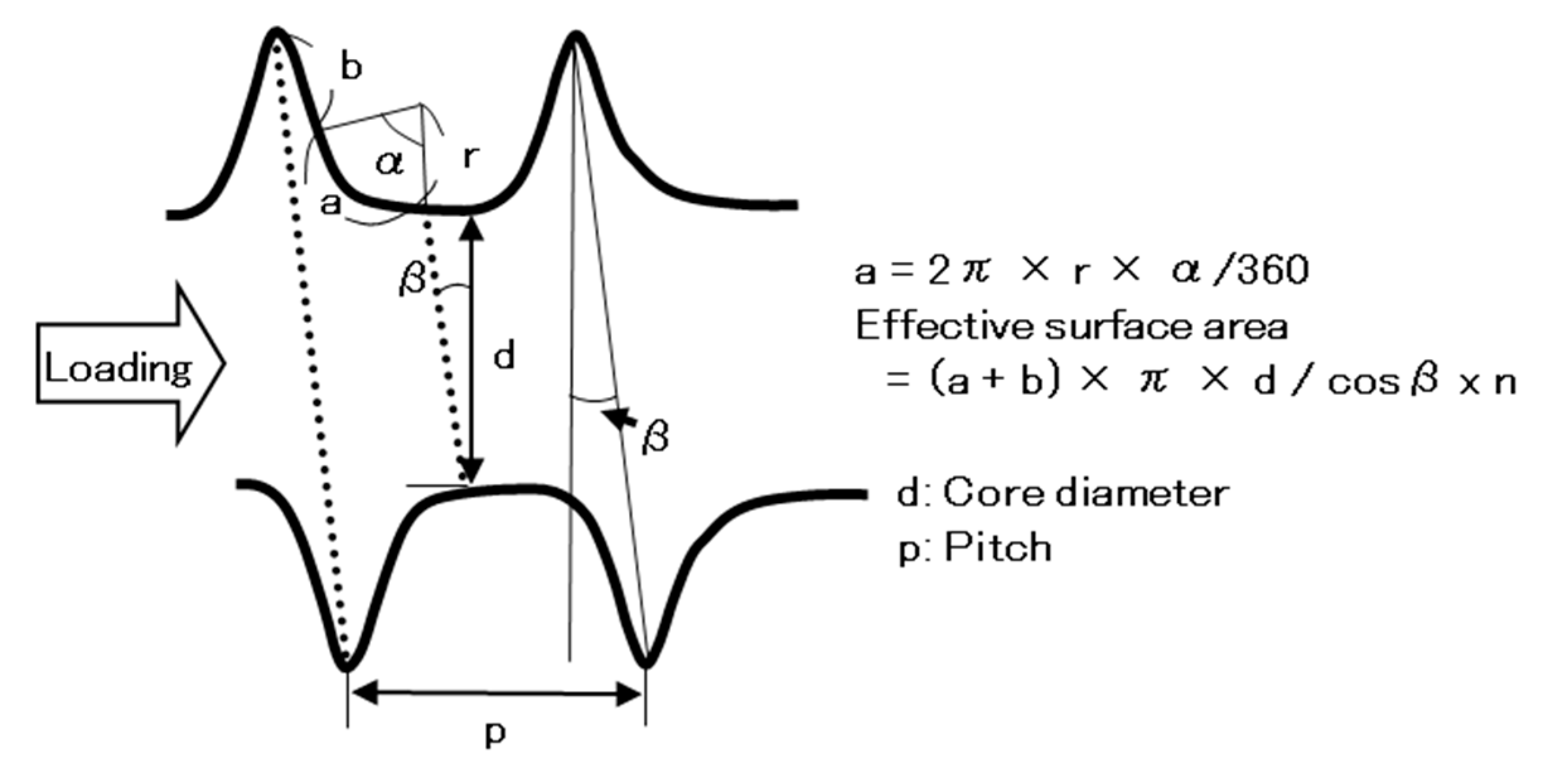



| Supplier | Length (mm) | Length of Threaded Portion (mm) | Thread dia. (mm) | Core dia. (mm) | Pitch (mm) | |
|---|---|---|---|---|---|---|
| (1) Self-tapping fully threaded cortical screws | ||||||
| (i) Depuy Synthes | ||||||
| C.P. Ti | 414-040 | 40 | 35 | 4.5 | 3.0 | 1.75 |
| 414-850S | 50 | 45 | 4.4 | 3.0 | 1.75 | |
| Ti-6-4 | 480-990VS | 36 | 26 | 3.9 | 3.0 | 0.70 |
| Stainless | 214-850 | 50 | 45 | 4.4 | 3.0 | 1.75 |
| 280-990 | 36 | 26 | 3.9 | 3.0 | 0.70 | |
| (ii) Zimmer Biomet | ||||||
| Ti-6-4 | 48-2319-038-00 | 38 | 33 | 4.6 | 3.8 | 1.02 |
| 48-2319-050-00 | 50 | 45 | 4.6 | 3.8 | 1.02 | |
| 48-2306-050-01 | 50 | 43 | 4.4 | 3.0 | 1.72 | |
| Stainless | 00-2253-050-42 | 50 | 45 | 4.2 | 3.7 | 1.61 |
| 00-2253-050-55 | 50 | 45 | 5.5 | 5.0 | 1.60 | |
| (iii) Mizuho | ||||||
| Ti-6-4 | 01-810-34 | 34 | 29 | 4.5 | 3.0 | 1.75 |
| 01-810-36 | 36 | 31 | 4.5 | 3.0 | 1.75 | |
| 01-810-38 | 38 | 32 | 4.5 | 3.0 | 1.75 | |
| 01-810-46 | 46 | 41 | 4.5 | 3.0 | 1.75 | |
| 01-810-48 | 48 | 43 | 4.5 | 3.0 | 1.75 | |
| Ti-Zr | 01-810-46 | 46 | 41 | 4.5 | 3.0 | 1.75 |
| (iv) Stryker | ||||||
| Ti-6-4 | OR-601050 | 50 | 44 | 4.5 | 2.9 | 1.75 |
| 1896-5050S | 50 | 46 | 5.0 | 3.9 | 1.75 | |
| (v) MDM | ||||||
| Ti-6-4 | 14022-50 | 50 | 45 | 4.6 | 3.7 | 1.00 |
| 14224-50 | 50 | 43 | 5.0 | 3.6 | 1.83 | |
| (vi) Teijin Nakashima Medical | ||||||
| Ti-6-4 | B30 | 50 | 45 | 4.5 | 2.9 | 1.75 |
| B35 | 50 | 45 | 4.5 | 3.4 | 1.75 | |
| (vii) Meira | ||||||
| Ti-6-4 | 035A-001-050 | 50 | 45 | 3.6 | 2.5 | 1.24 |
| (2) Cannulated cancellous screw (partially threaded) | ||||||
| (i) Depuy Synthes | ||||||
| C.P. Ti | 417-050 | 50 | 32 | 6.5 | 3.1 | 2.76 |
| Stainless | 217-050 | 50 | 32 | 6.5 | 3.0 | 2.75 |
| (ii) Zimmer Biomet | ||||||
| Ti-6-4 | 47-2483-095-60 | 95 | 32 | 6.0 | 4.7 | 1.72 |
| (iii) MDM | ||||||
| Ti-6-4 | 14225-50 | 50 | 20 | 5.0 | 3.6 | 1.73 |
| (iv) Teijin Nakashima Medical | ||||||
| Ti-6-4 | B050 | 50 | 30 | 6.0 | 4.3 | 1.76 |
| (v) Meira | ||||||
| Ti-6-4 | 005A-340-070 | 70 | 25 | 4.2 | 2.8 | 1.50 |
| (3) Locking bolts used in intramedullary femoral nails | ||||||
| Depuy Synthes | ||||||
| Ti-6-4 | 459-300VS | 30 | 25 | 4.8 | 4.2 | 2.78 |
| Ti-6-4 | 459-500VS | 50 | 45 | 4.8 | 4.2 | 2.78 |
| (4) Pedicle screws | ||||||
| (i) Robert Reid | ||||||
| Ti-6-4 | ISOLA 2226-2440 | 45 | 38 | 5.5 | 4.0 | 2.21 |
| ISOLA 2226-2840 | 45 | 38 | 6.3 | 4.6 | 2.21 | |
| ISOLA 2230-07R | 55 | 48 | 6.3 | 4.6 | 2.22 | |
| (ii) Medtronic | ||||||
| Ti-6-4 | 8695540 | 45 | 40 | 5.6 | 3.9 | 2.73 |
| 86946540 | 45 | 40 | 6.6 | 4.3 | 2.85 | |
| (iii) Zimmer Biomet | ||||||
| Ti-6-4 | 3306-4540 | 40 | 32 | 4.5 | 3.2 | 2.37 |
| 3306-5540 | 40 | 32 | 5.5 | 4.0 | 2.54 | |
| Solid Rigid Polyurethane Foam (SRPF) | ||||||||
|---|---|---|---|---|---|---|---|---|
| Grade | 5 | 10 | 15 | 20 | 25 | 30 | 35 | 40 |
| Nominal density (kg/m3) | 80.1 | 160.2 | 240.3 | 320.4 | 400.5 | 480.6 | 560.6 | 640.8 |
| Cellular rigid polyurethane foam (CRPF) | ||||||||
| Grade | 7.5 | 10 | 15 | 20 | − | − | − | − |
| Nominal density (kg/m3) | 120.15 | 160.2 | 240.3 | 320.4 | − | − | − | − |
| Material | Torsional | Durability | Tensile [1] | ||||
|---|---|---|---|---|---|---|---|
| τ2°/MPa | τmax/MPa | G/MPa | σD/MPa | σ0.2/MPa | σUTS/MPa | σD/σUTS (%) | |
| (1) Full-thread cortical screws | |||||||
| C.P.Ti | 552 | 948 | 1616 | 498 | 568 | 725 | 69 |
| Ti-6-4 | 650 ± 95 | 986 ± 67 | 2140 ± 50 | 804 ± 77 | 892 | 990 | 82 |
| Stainless | 824 ± 5 | 1012 ± 53 | 1421 ± 80 | 732 ± 133 | 876 | 1051 | 70 |
| Ti-Zr | 598 | 870 | 2698 | - | - | - | - |
| (2) Cannulated cancellous screws | |||||||
| C.P.Ti | 849 | 1259 | 4889 | - | - | - | - |
| Ti-6-4 | 535 ± 2 | 730 | - | - | - | - | - |
| Stainless | 1013 | 1304 | - | - | - | - | - |
| (3) Locking bolt | |||||||
| Ti-6-4 | 535 | 776 | 3254 | - | - | - | - |
| Grade | Density/kg·m−3 | Compressive | Shear | ||
|---|---|---|---|---|---|
| Strength/MPa | Modulus/MPa | Strength/MPa | Modulus/MPa | ||
| 5 | 72−88 | 0.4−1.0 | 12−28 | 0.4−0.9 | 5.5−10 |
| 10 | 144−176 | 1.7−2.8 | 46−78 | 1.2−2.0 | 15−30 |
| 12 | 173−212 | 2.5−4.0 | 65−100 | 1.6−2.6 | 20−40 |
| 15 | 216−265 | 3.8−6.1 | 98−170 | 2.2−3.5 | 27−60 |
| 20 | 289−353 | 6.5−10 | 170−270 | 3.4−5.4 | 40−90 |
| 25 | 361−441 | 10−16 | 250−390 | 4.0−7.3 | 56−130 |
| 30 | 433−529 | 14−23 | 360−550 | 5.0−9.5 | 72−200 |
| 35 | 505−617 | 18−31 | 470−800 | 7−12 | 90−250 |
| 40 | 577−705 | 25−40 | 600−1100 | 8−15 | 110−300 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Okazaki, Y.; Hayakawa, E.; Tanahashi, K.; Mori, J. Mechanical Performance of Metallic Bone Screws Evaluated Using Bone Models. Materials 2020, 13, 4836. https://doi.org/10.3390/ma13214836
Okazaki Y, Hayakawa E, Tanahashi K, Mori J. Mechanical Performance of Metallic Bone Screws Evaluated Using Bone Models. Materials. 2020; 13(21):4836. https://doi.org/10.3390/ma13214836
Chicago/Turabian StyleOkazaki, Yoshimitsu, Emiko Hayakawa, Kazumasa Tanahashi, and Jun Mori. 2020. "Mechanical Performance of Metallic Bone Screws Evaluated Using Bone Models" Materials 13, no. 21: 4836. https://doi.org/10.3390/ma13214836
APA StyleOkazaki, Y., Hayakawa, E., Tanahashi, K., & Mori, J. (2020). Mechanical Performance of Metallic Bone Screws Evaluated Using Bone Models. Materials, 13(21), 4836. https://doi.org/10.3390/ma13214836





