Dynamic Fracture and Fragmentation Characteristics of Metal Cylinder and Rings Subjected to Internal Explosive Loading
Abstract
1. Introduction
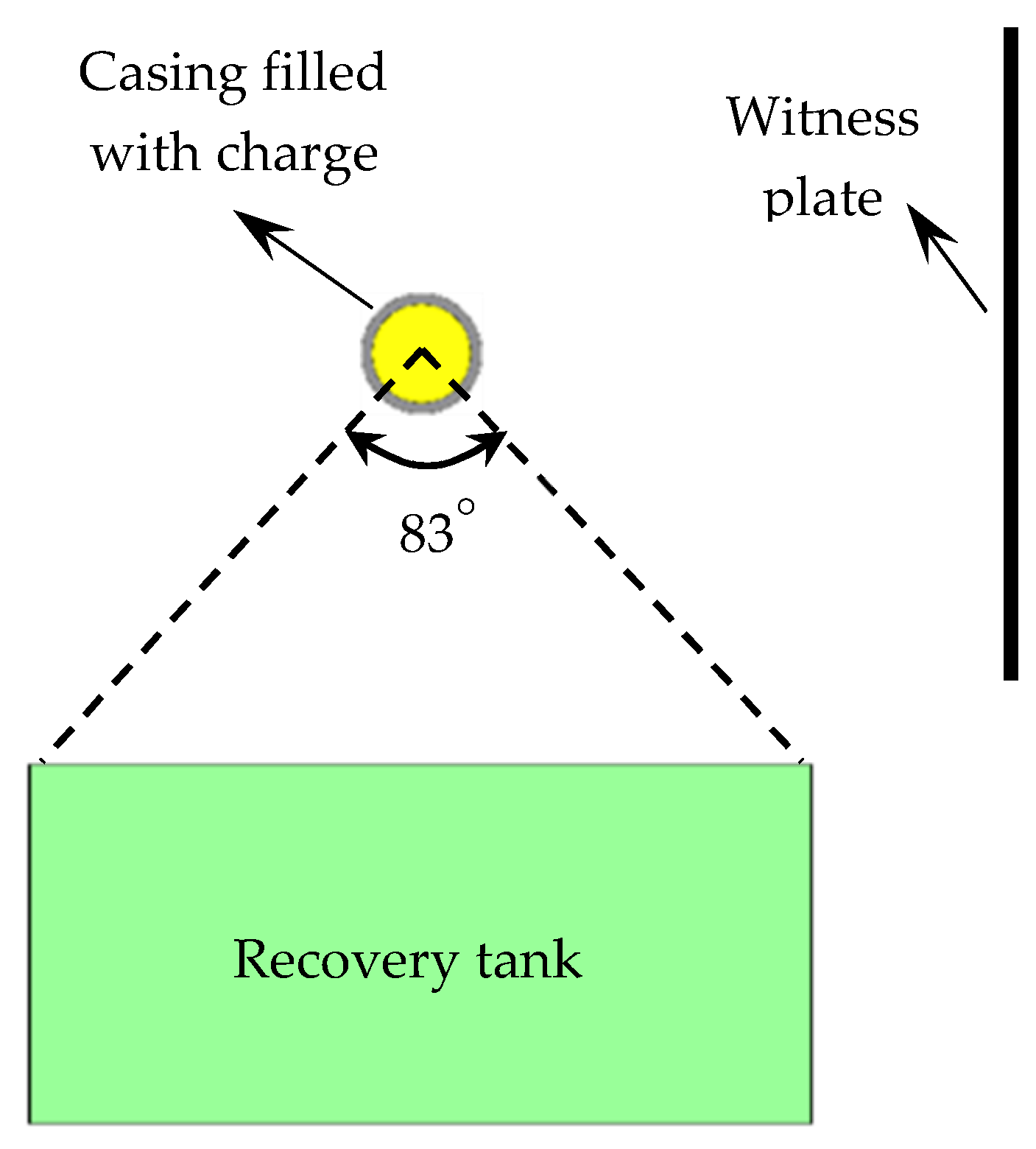
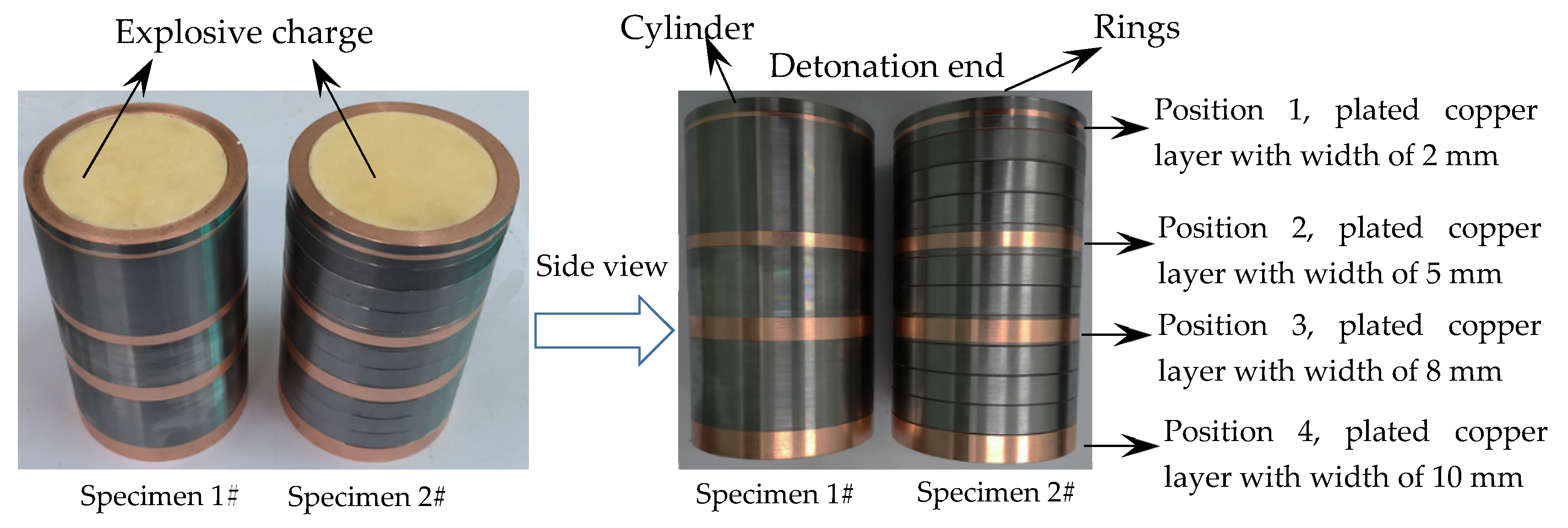
2. Experimental Design
3. Experimental Results and Discussion
3.1. Witness Plate
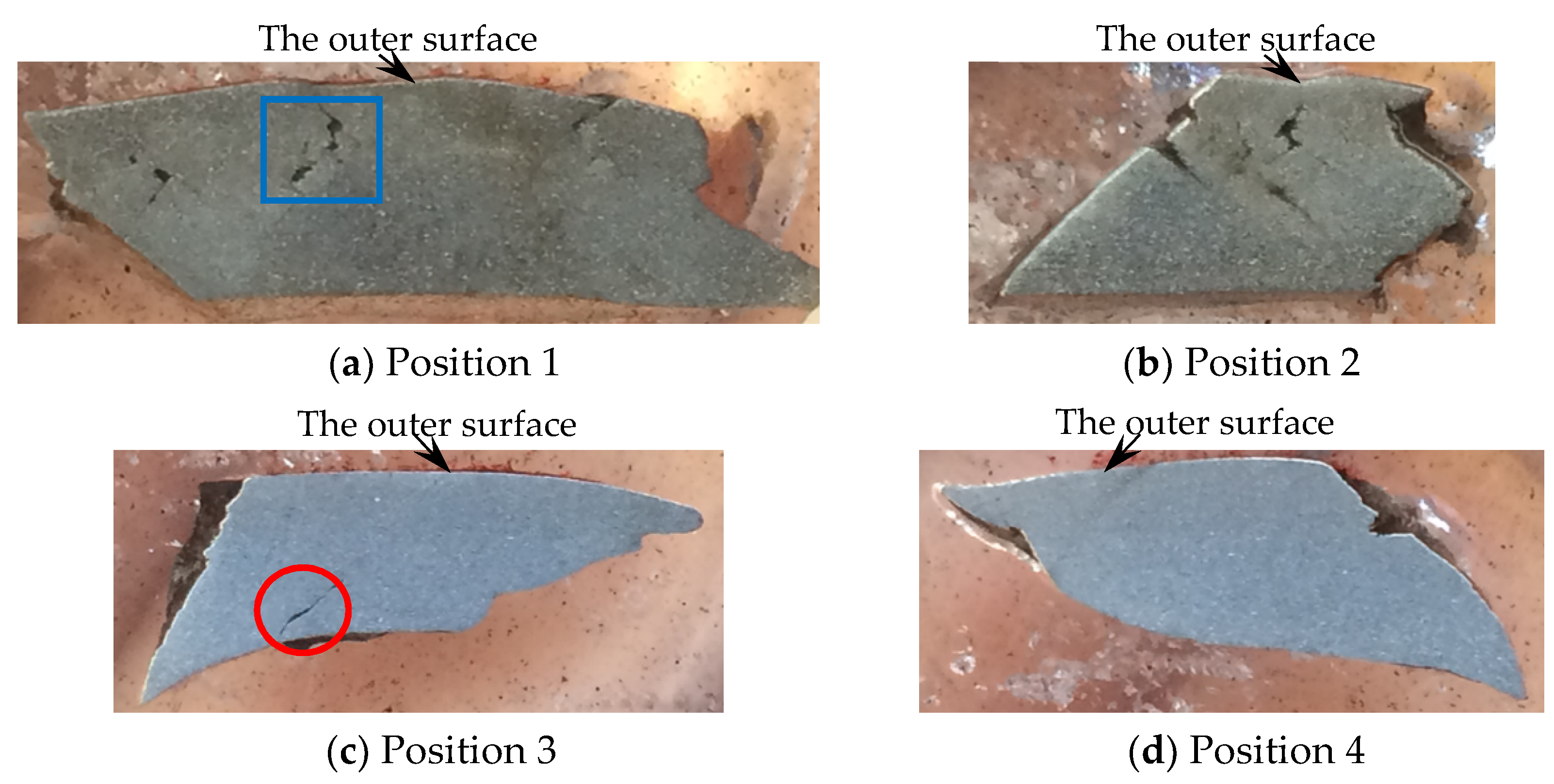
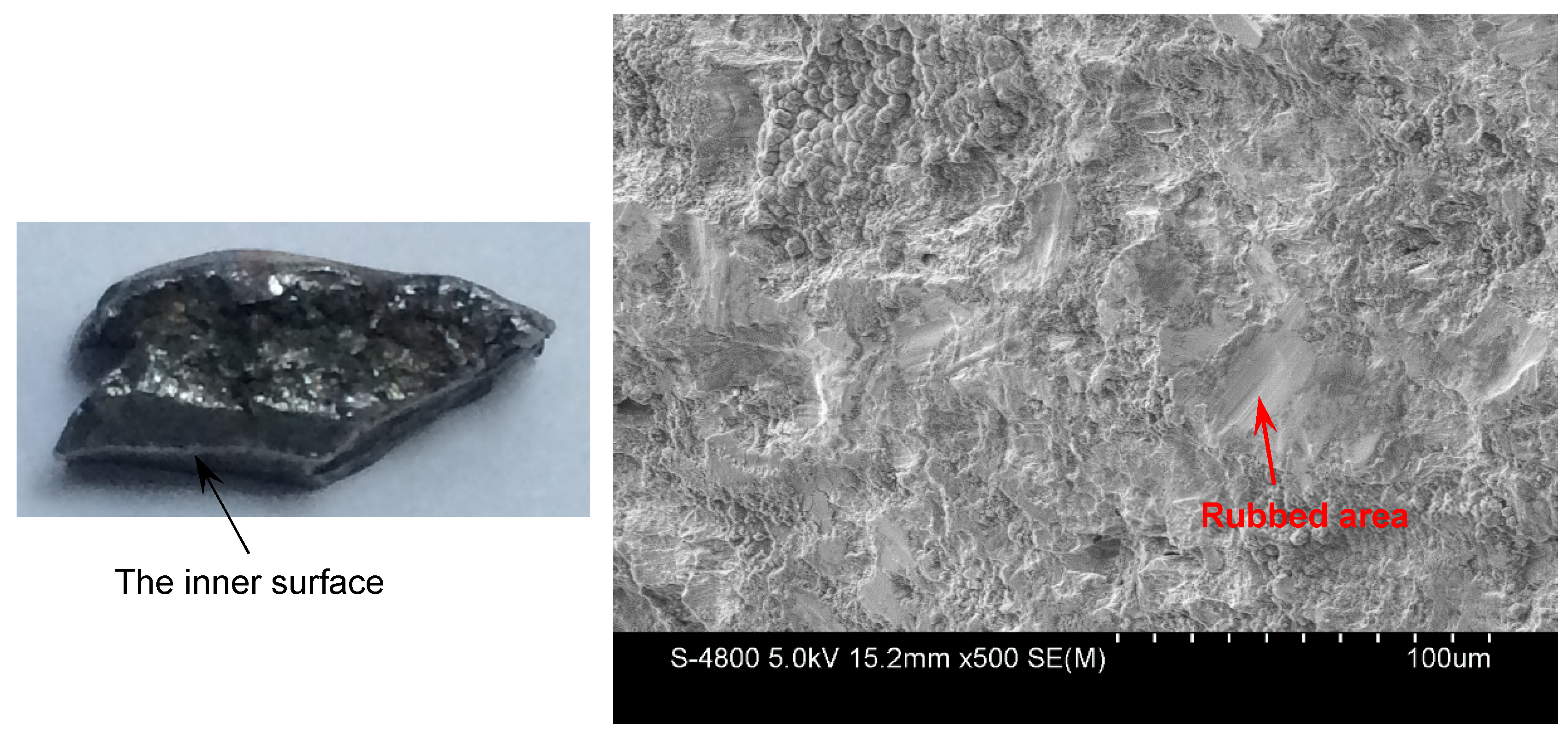
3.2. Fracture Mechanism
3.3. Fragment Mass and Size
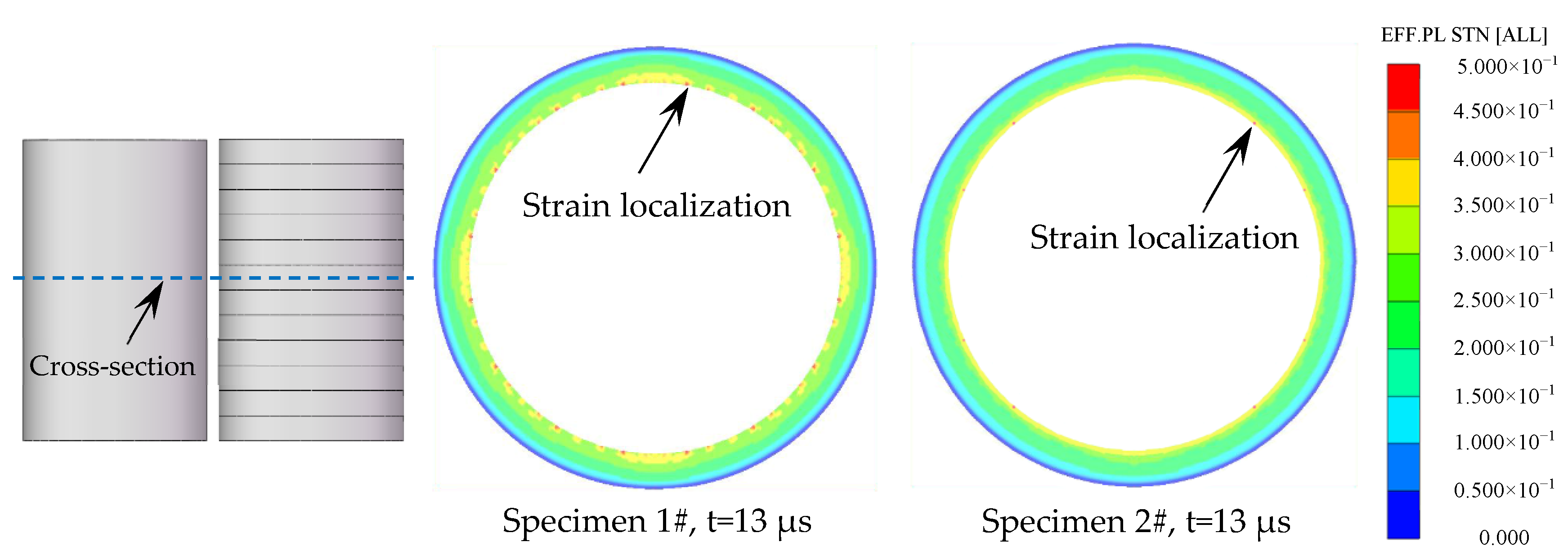
4. Numerical Simulation
5. Conclusions
- (1)
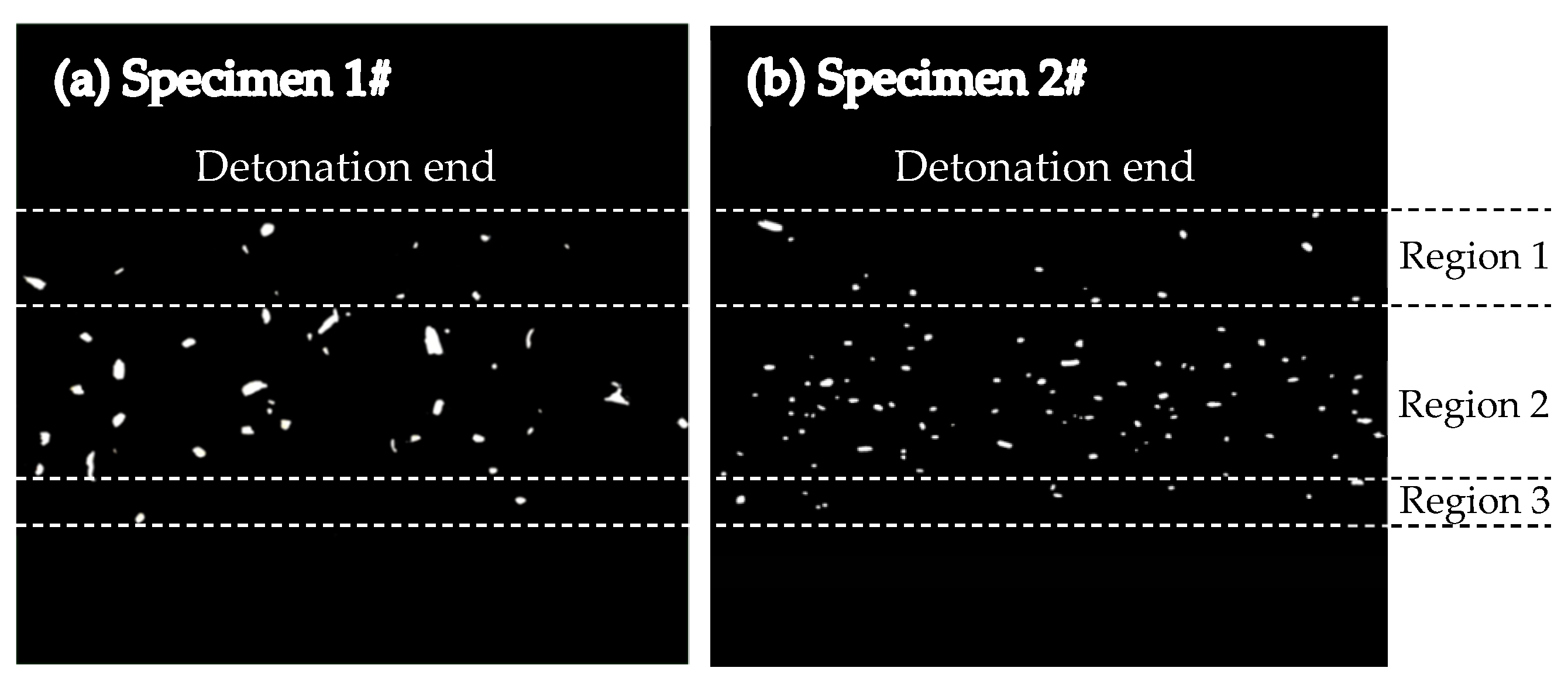
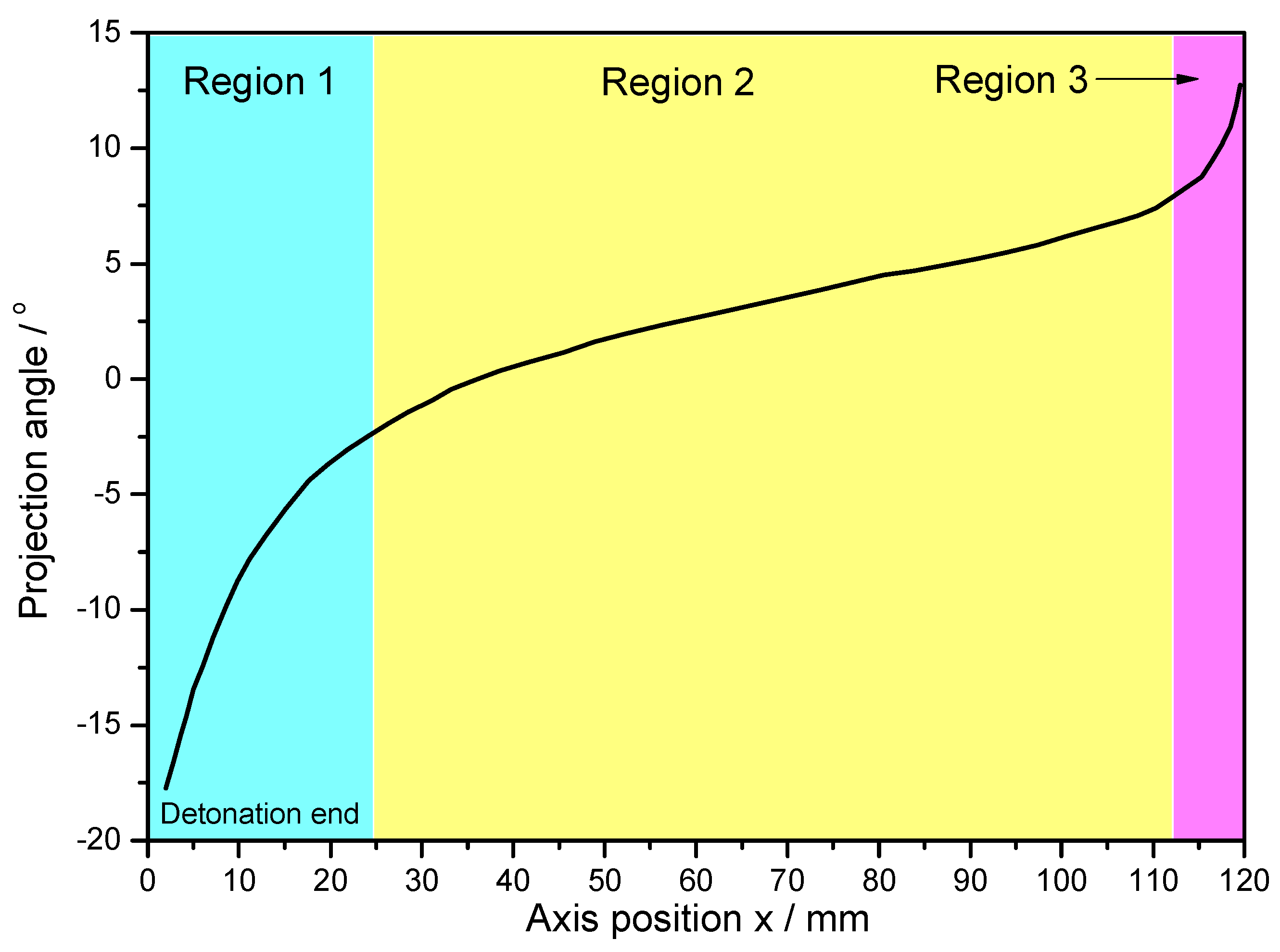
- The similar site distributions of the holes in witness plates suggest that the projection angle axial distribution of the fragments for the metal cylinder was similar to that of the fragments for the metal rings. The fragments from the two ends of the metal cylinder were relatively small, and the metal ring at the non-detonation end of the metal rings was split along the axis of the warhead, which is due to the radical changes of both the fragment velocity and the fragment projection angle along the axis of the casings at the ends.
- (2)
- For the metal cylinder, the fracture surfaces were typically oriented at ~45° with respect to either the inner or outer fragment surfaces. The adiabatic shear band played a key role in the fracture process of the metal cylinder, and it preferred to initiate near the inner surface and propagated towards the outer surface along the maximum shear stress direction. The adiabatic shear band could maintain a long time before it converged into a crack, and the material at two sides of the adiabatic shear band moved a long distance along the adiabatic shear band during this time, which made the inner surface of the fragment step-like. The morphologies of the fracture surfaces of fragments from different positions of metal cylinder were similar, and the fracture surfaces were very smooth, which was caused by the adiabatic shear bands.
- (3)
- For the metal rings, the deformation and fracture characteristics were significantly different from those of the metal cylinder. Some fractures were typically oriented at ~45° with respect to either the inner or outer fragment surfaces, and some fractures were perpendicular to the outer surface. Large and small voids formed within some fragments by brittle fracture or ductile fracture. During the expansion of the metal rings, the adiabatic shear band had little chance to initiate because the metal rings could deform uniformly with much fewer strain localizations due to their much lower length, but some slightly deformed grains formed near the inner surface, which made the inner surface of the fragment step-like. The morphologies of the fracture surfaces of fragments from different positions of the metal rings were similar, and dimples were found in the fracture surfaces, which shows that it was a kind of ductile fracture.
- (4)
- The mass distribution of the fragments from the metal rings was more uniform than that of the fragments from the metal cylinder. The average thicknesses of the fragments from different positions of the metal cylinder and rings were at the same level. The average widths of the plated copper layers for the metal cylinder were slightly larger than their original ones because tensile stress existed between the fragments along the axis of the cylinder during its expansion. In contrast, the average widths of the plated copper layers for the metal rings were smaller than their original ones, because the metal rings were disconnected in the axial direction of the warhead.
- (5)
- Under current experimental conditions, the circumferential rupture strains for different positions of the metal rings were at the same level (~78%), and those of the metal cylinder were much lower (~41%). The metal cylinder was broken when it expanded to ~1.41 times its original radius, and the metal rings were broken when they expanded to ~1.78 times their original radius.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Gurney, R.W. The Initial Velocities of Fragments from Bombs, Shells and Grenades; Ballistic Research Laboratories: Aberdeen, MD, USA, 1943. [Google Scholar]
- Zhang, Q.; Miao, C.Q.; Lin, D.C.; Bai, C.H. Relation of fragment with air shock wave intensity for explosion in a shell. Int. J. Impact Eng. 2003, 28, 1129–1141. [Google Scholar] [CrossRef]
- Elek, P.; Jaramaz, S.; Mickovic, D. Modeling of the metal cylinder acceleration under explosive loading. Sci. Tech. Rev. 2013, 63, 39–46. [Google Scholar]
- An, X.Y.; Dong, Y.X.; Liu, J.Y.; Tian, C. General formula to calculate the fragment velocity of warheads with hollow core. Int. J. Impact Eng. 2018, 113, 1–8. [Google Scholar] [CrossRef]
- Hennequin, E. Influence of edge effects on the initial velocities of fragments from a warhead. In Proceedings of the 9th international symposium on ballistics, Shrivenham, UK, 29 April–1 May 1986. [Google Scholar]
- Charron, Y.J. Estimation of velocity distribution of fragmenting warheads using a modified Gurney method. Master’s Thesis, Air Force Institute of Technology, Dayton, OH, USA, 1979. [Google Scholar]
- Huang, G.Y.; Li, W.; Feng, S.S. Axial distribution of fragment velocities from cylindrical casing under explosive loading. Int. J. Impact Eng. 2015, 76, 20–27. [Google Scholar] [CrossRef]
- Grisaro, H.; Dancygier, A.N. Numerical study of velocity distribution of fragments caused by explosion of a cylindrical cased charge. Int. J. Impact Eng. 2015, 86, 1–12. [Google Scholar] [CrossRef]
- Ning, J.G.; Duan, Y.; Xu, X.; Ren, H.L. Velocity characteristics of fragments from prismatic casing under internal explosive loading. Int. J. Impact Eng. 2017, 109, 29–38. [Google Scholar] [CrossRef]
- An, X.Y.; Liu, J.Y.; Ye, P.; Tian, C.; Feng, S.S.; Dong, Y.X. Axial distribution characteristics of fragments of the warhead with a hollow core. Int. J. Impact Eng. 2018, 122, 10–22. [Google Scholar] [CrossRef]
- Mott, N.F. Fragmentation of shell cases. Proc. R. Soc. Lond. Ser. A 1947, 189, 300–308. [Google Scholar]
- Grady, D.E.; Olsen, M.L. Statistics and energy based theory of dynamic fragmentation. Int. J. Impact Eng. 2003, 29, 293–306. [Google Scholar] [CrossRef]
- Grady, D. Fragmentation of Rings and Shells; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Taylor, G.I. The fragmentation of tubular bombs. Advis. Counc. Sci. Res. Tech. Dev. 1963, 5, 202–320. [Google Scholar]
- Hoggatt, C.R.; Recht, R.F. Fracture behavior of tubular bombs. J. Appl. Phys. 1968, 39, 1856–1862. [Google Scholar] [CrossRef]
- Martineau, R.L.; Anderson, C.A. Expansion of cylinder shells subjected to internal explosive detonations. Exp. Mech. 2000, 40, 219–225. [Google Scholar] [CrossRef]
- Hu, H.B.; Tang, T.G.; Hu, B.Y.; Wang, D.S. Longitudinal propagation of fracture surface in cylindrical metal shells under explosive loading. Shock Compress. Condens. Matter 2007, 955, 541–5444. [Google Scholar]
- Goto, D.M.; Becker, R.; Orzechowski, T.J.; Springer, H.K.; Sunwoo, A.J.; Syn, C.K. Investigation of the fracture and fragmentation of explosively driven rings and cylinders. Int. J. Impact Eng. 2008, 35, 1547–1556. [Google Scholar] [CrossRef]
- Tang, T.G.; Li, Q.Z.; Sun, X.L.; Sun, Z.F.; Jin, S.; Gu, Y. Strain rate effects of expanding fracture of 45 steel cylinder shells driven by detonation. Explos. Shock Waves 2006, 26, 129. [Google Scholar]
- Liu, M.T.; Ren, G.W.; Fan, C.; Tang, T.G.; Wang, X.Y.; Hu, H.B. Experimental and Numerical Studies on the Expanding Fracture Behavior of an Explosively Driven 1045 Steel Cylinder. Int. J. Impact Eng. 2017, 109, 240–252. [Google Scholar] [CrossRef]
- Avriel, E.; Lovinger, Z.; Nemirovsky, R.; Rittel, D. Investigating the strength of materials at very high strain rates using electro-magnetically driven expanding cylinders. Mech. Mater. 2018, 117, 165–180. [Google Scholar] [CrossRef]
- Singh, M.; Suneja, H.R.; Bola, M.S.; Prakash, S. Dynamic tensile deformation and fracture of metal cylinders at high strain rates. Int. J. Impact Eng. 2002, 27, 939–954. [Google Scholar] [CrossRef]
- Arnold, W.; Rottenkolber, E. Fragment mass distribution of metal cased explosive charges. Int. J. Impact Eng. 2008, 35, 1393–1398. [Google Scholar] [CrossRef]
- König, P.J. A Correction for Ejection Angles of Fragments from Cylindrical Wareheads. Propellants Explos. Pyrotech. 1987, 12, 154–157. [Google Scholar] [CrossRef]
- Wang, L.; Han, F.; Zhou, Q. The projection angles of fragments from a cylindrical casing filled with charge initiated at one end. Int. J. Impact Eng. 2017, 103, 138–148. [Google Scholar] [CrossRef]
- Taylor, G. Analysis of the explosion of a long cylindrical bomb detonated at one end. Sci. Pap. GI Taylor. 1941, 2, 277–286. [Google Scholar]
- Johnson, G.R.; Cook, W.H. A constitutive model and data for metals subjected to large strain, high strain rates and high temperatures. In Proceedings of the 7th international symposium on ballistic, The Hague, The Netherlands, 19–21 April 1983. [Google Scholar]
- Li, Y.X.; Li, Y.; Yang, M.S.; Wei, F.Z.; Yuan, Q.L.; Cui, F.K. Determination of 40Cr Johnson-Cook Dynamic Constitutive Equation for Cold roll-beating Forming Process. Chem. Eng. Trans. 2015, 46, 1159–1164. [Google Scholar]
- ANSYS AUTODYN. ANSYS Inc.: USA. Available online: https://www.ansys.com/products/structures/ansys-autodyn (accessed on 31 December 2019).
- Kasvayee, K.A.; Ghassemali, E.; Salomonsson, K.; Sujakhu, S.; Castagne, S.; Jarfors, A.E.W. Strain localization and crack formation effects on stress-strain response of ductile iron. Mater. Sci. Eng. A 2017, 702, 265–271. [Google Scholar] [CrossRef]
- Abuzaid, W.; Sehitoglu, H.; Lambros, J. Plastic strain localization and fatigue micro-crack formation in Hastelloy X. Mater. Sci. Eng. A 2013, 561, 507–519. [Google Scholar] [CrossRef]










| Density (g/cm3) | Yield Strength (MPa) | Elastic Modulus (GPa) | Hardness | Poisson Ratio |
|---|---|---|---|---|
| 7.87 | 785 | 211 | 207 HB | 0.3 |
| No. | Position | Average Thickness (mm) | Average Width of the Plated Copper Layers (mm) | Circumferential Rupture Strain (%) | Ratio of Rupture Radius to Original Radius |
|---|---|---|---|---|---|
| 1# | 1 | 4.71 | ----- | ----- | ----- |
| 2 | 4.59 | 5.07 | 39.7 | 1.397 | |
| 3 | 4.48 | 8.14 | 42.7 | 1.427 | |
| 4 | 4.64 | ----- | ----- | ----- | |
| 2# | 1 | 4.82 | ----- | ----- | ----- |
| 2 | 4.47 | 4.12 | 76.4 | 1.764 | |
| 3 | 4.48 | 6.43 | 80.5 | 1.805 | |
| 4 | 5.05 | ----- | ----- | ----- |
| No. | Length of Charge (mm) | Length of Rings (mm) | Diameter of Charge (mm) | Outside Diameter of Casing (mm) |
|---|---|---|---|---|
| 3#-1 | 120 | 120 | 60 | 80 |
| 3#-2 | 120 | 10 | 60 | 80 |
| 4#-1 | 120 | 120 | 60 | 66 |
| 4#-2 | 120 | 10 | 60 | 66 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
An, X.; Ye, P.; Liu, J.; Tian, C.; Feng, S.; Dong, Y. Dynamic Fracture and Fragmentation Characteristics of Metal Cylinder and Rings Subjected to Internal Explosive Loading. Materials 2020, 13, 778. https://doi.org/10.3390/ma13030778
An X, Ye P, Liu J, Tian C, Feng S, Dong Y. Dynamic Fracture and Fragmentation Characteristics of Metal Cylinder and Rings Subjected to Internal Explosive Loading. Materials. 2020; 13(3):778. https://doi.org/10.3390/ma13030778
Chicago/Turabian StyleAn, Xuanyi, Ping Ye, Jiayun Liu, Chao Tian, Shunshan Feng, and Yongxiang Dong. 2020. "Dynamic Fracture and Fragmentation Characteristics of Metal Cylinder and Rings Subjected to Internal Explosive Loading" Materials 13, no. 3: 778. https://doi.org/10.3390/ma13030778
APA StyleAn, X., Ye, P., Liu, J., Tian, C., Feng, S., & Dong, Y. (2020). Dynamic Fracture and Fragmentation Characteristics of Metal Cylinder and Rings Subjected to Internal Explosive Loading. Materials, 13(3), 778. https://doi.org/10.3390/ma13030778




