In Situ Electrochemical Study of the Growth Kinetics of Passive Film on TC11 Alloy in Sulfate Solution at 300 °C/10 MPa
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
3.1. Long-Term OCP Tests
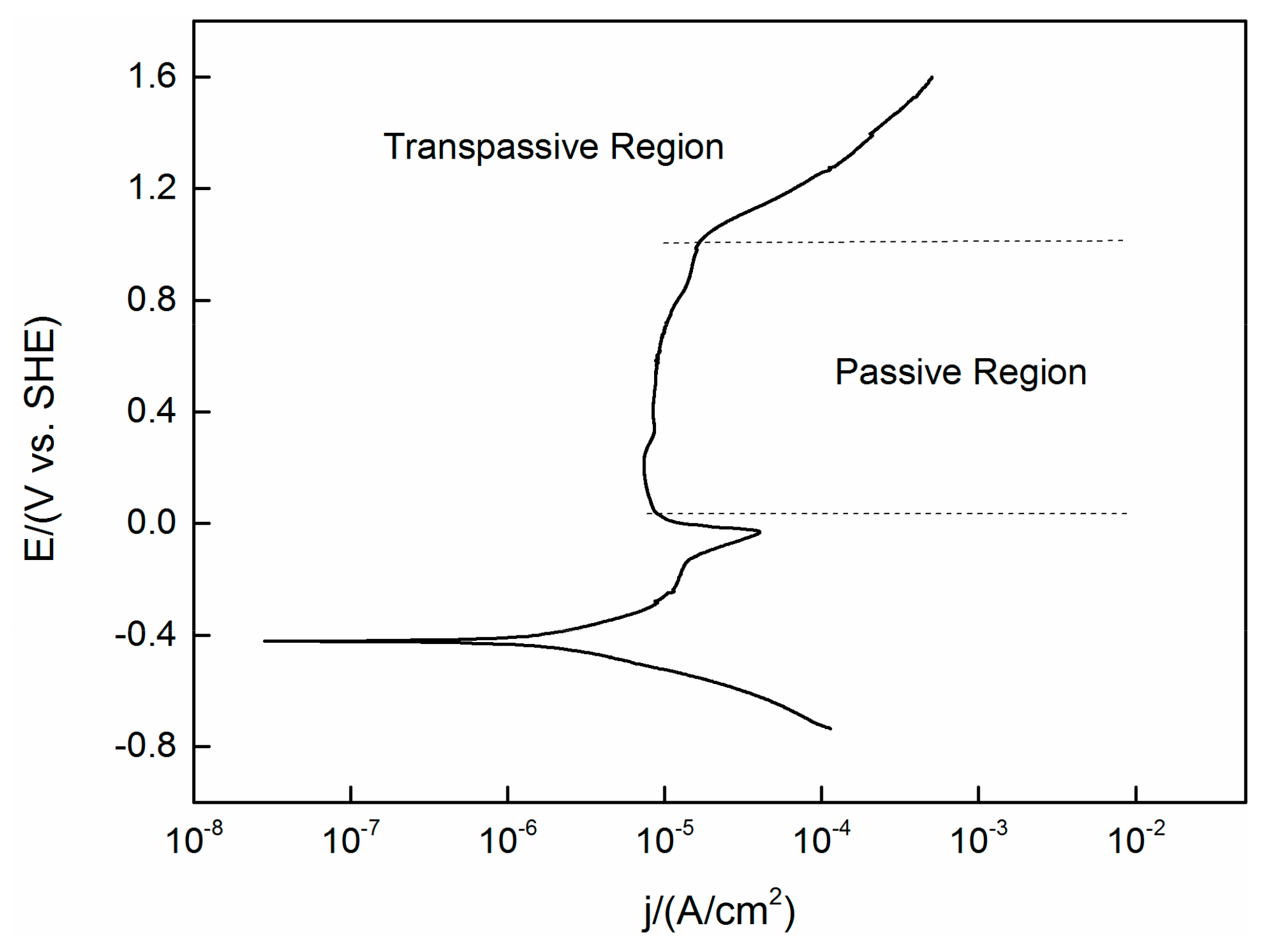
3.2. Polarization Resistance
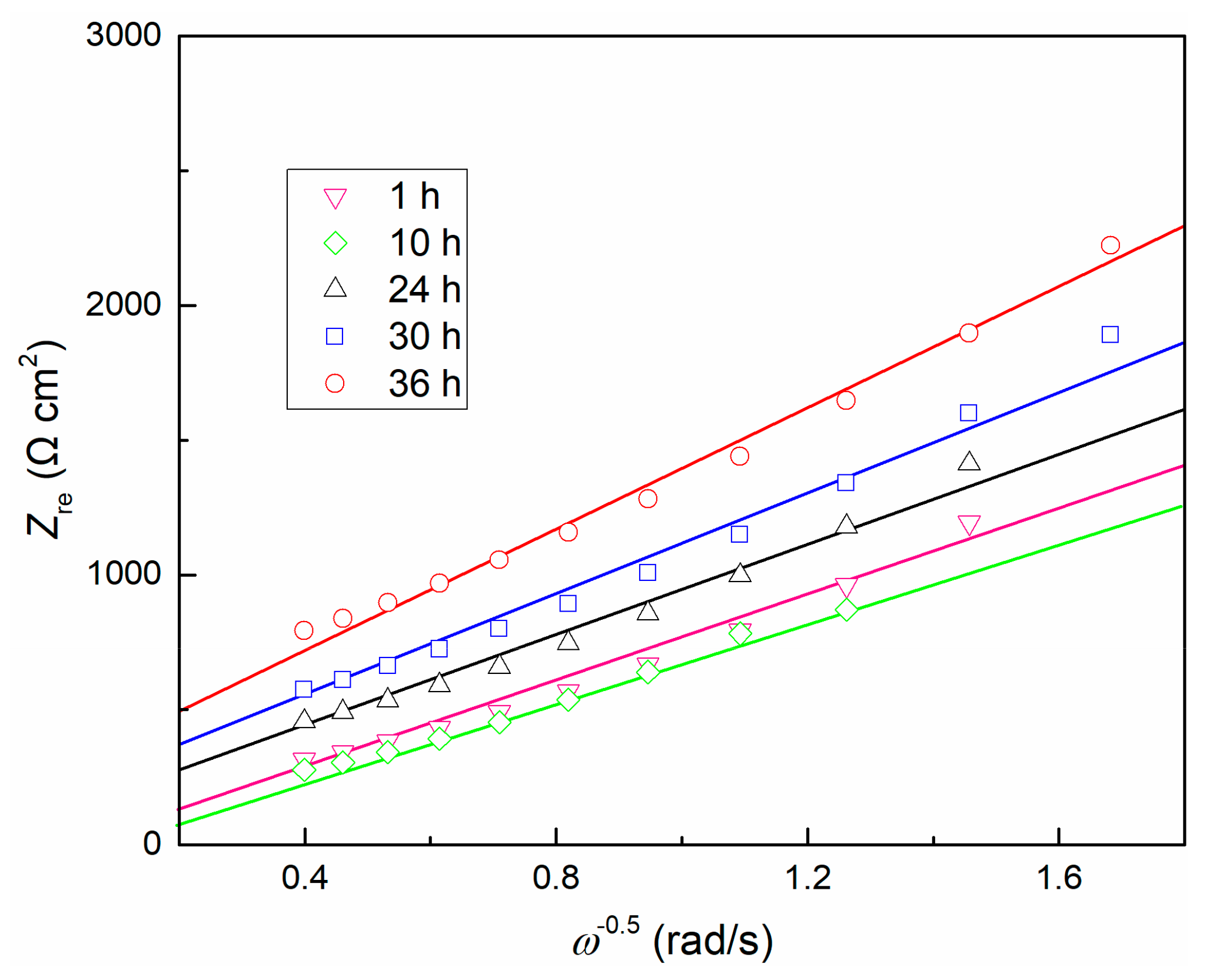
3.3. Electrochemical Impedance Spectroscopy
3.3.1. EIS Analysis Using Equivalent Circuit
3.3.2. EIS Analysis Using the Point Defect Model
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- El-Bagoury, N.; Ahmed, S.I.; Abu Ali, O.A.; El-Hadad, S.; Fallatah, A.M.; Mersal, G.A.M.; Ibrahim, M.M.; Wysocka, J.; Ryl, J.; Boukherroub, R.; et al. The influence of microstructure on the passive layer chemistry and corrosion resistance for some titanium-based alloys. Materials 2019, 12, 20. [Google Scholar] [CrossRef]
- De Assis, S.L.; Wolynec, S.; Costa, I. Corrosion characterization of titanium alloys by electrochemical techniques. Electrochim. Acta 2006, 51, 1815–1819. [Google Scholar] [CrossRef]
- Wang, K. The use of titanium for medical applications in the USA. Mater. Sci. Eng. A 1996, 213, 134–137. [Google Scholar] [CrossRef]
- Oberwinkler, B.; Riedler, M.; Eichlseder, W. Importance of local microstructure for damage tolerant light weight design of Ti–6Al–4V forgings. Int. J. Fatigue 2010, 32, 808–814. [Google Scholar] [CrossRef]
- Knobbe, H.; Köster, P.; Christ, H.-J.; Fritzen, C.-P.; Riedler, M. Initiation and propagation of short fatigue cracks in forged Ti6Al4V. Proced. Eng. 2010, 2, 931–940. [Google Scholar] [CrossRef]
- Boyer, R.R. An overview on the use of titanium in the aerospace industry. Mater. Sci. Eng. A 1996, 213, 103–114. [Google Scholar] [CrossRef]
- Jackson, M.; Dring, K. A review of advances in processing and metallurgy of titanium alloys. Mater. Sci. Technol. 2006, 22, 881–887. [Google Scholar] [CrossRef]
- Ansari, G.; Fattah-alhosseini, A. On the passive and semiconducting behavior of severely deformed pure titanium in Ringer’s physiological solution at 37°C: A trial of the point defect model. Mater. Sci. Eng. C 2017, 75, 64–71. [Google Scholar] [CrossRef] [PubMed]
- Fernandez-Domene, R.M.; Blasco-Tamarit, E.; Garcia-Garcia, D.M.; Anton, J.G. Passivity breakdown of titanium in LiBr solutions. J. Electrochem. Soc. 2014, 161, C25–C35. [Google Scholar] [CrossRef]
- Manjaiah, M.; Laubscher, R.F. Effect of anodizing on surface integrity of Grade 4 titanium for biomedical applications. Surf. Coat. Tech. 2017, 310, 263–272. [Google Scholar] [CrossRef]
- Wang, L.; Yu, H.; Wang, S.; Chen, B.; Wang, Y.; Fan, W.; Sun, D. Quantitative analysis of local fine structure on diffusion of point defects in passive film on Ti. Electrochim. Acta 2019, 314, 161–172. [Google Scholar] [CrossRef]
- Zha, L.; Li, H.P.; Wang, N.; Lin, S.; Xu, L.P. Electrochemical corrosion behavior of TC11 alloy in sulfate solution at high temperature and high pressure. Int. J. Electrochem. Sci. 2017, 12, 5704–5722. [Google Scholar] [CrossRef]
- Zha, L.; Li, H.P.; Wang, N. Electrochemical characterization of anodic oxide film on TC11 alloy in sulfate solution at high temperature and high pressure. Int. J. Electrochem. Sci. 2019, 14, 4546–4556. [Google Scholar] [CrossRef]
- Nickchi, T.; Alfantazi, A. Kinetics of passive film growth on Alloy 800 in the presence of hydrogen peroxide. Electrochim. Acta 2011, 58, 743–749. [Google Scholar] [CrossRef]
- Xu, J.; Wu, X.; Han, E.-H. The evolution of electrochemical behaviour and oxide film properties of 304 stainless steel in high temperature aqueous environment. Electrochim. Acta 2012, 71, 219–226. [Google Scholar] [CrossRef]
- De Araújo Figueiredo, C.; Bosch, R.-W.; Vankeerberghen, M. Electrochemical investigation of oxide films formed on nickel alloys 182, 600 and 52 in high temperature water. Electrochim. Acta 2011, 56, 7871–7879. [Google Scholar]
- Bosch, R.W.; Vankeerberghen, M. In-pile electrochemical tests of stainless steel under PWR conditions: Interpretation of electrochemical impedance spectroscopy data. Electrochim. Acta 2007, 52, 7538–7544. [Google Scholar] [CrossRef]
- Macák, J.; Sajdl, P.; Kučera, P.; Novotný, R.; Vošta, J. In situ electrochemical impedance and noise measurements of corroding stainless steel in high temperature water. Electrochim. Acta 2006, 51, 3566–3577. [Google Scholar] [CrossRef]
- Bojinov, M.; Kinnunen, P.; Lundgren, K.; Wikmark, G. A mixed-conduction model for the oxidation of stainless steel in a high-temperature electrolyte. J. Electrochem. Soc. 2005, 152, B250–B261. [Google Scholar] [CrossRef]
- Betova, I.; Bojinov, M.; Kinnunen, P.; Lundgren, K.; Saario, T. Mixed-conduction model for stainless steel in a high-temperature electrolyte: Estimation of Kinetic Parameters of Inner Layer Constituents. J. Electrochem. Soc. 2008, 155, C81–C92. [Google Scholar] [CrossRef]
- Vetter, K.J.; Gorn, F. Kinetics of layer formation and corrosion processes of passive iron in acid solutions. Electrochim. Acta 1973, 18, 321–326. [Google Scholar] [CrossRef]
- Cabrera, N.; Mott, N.F. Theory of the oxidation of metals. Rep. Prog. Phys. 1949, 12, 163–184. [Google Scholar] [CrossRef]
- Macdonald, D.D. The point-defect model for the passive state. J. Electrochem. Soc. 1992, 139, 3434–3449. [Google Scholar] [CrossRef]
- Macdonald, D.D.; Sikora, E.; Sikora, J. The kinetics of growth of the passive film on tungsten in acidic phosphate solutions. Electrochim. Acta 1998, 43, 2851–2861. [Google Scholar] [CrossRef]
- Kirchheim, R. Growth kinetics of passive films. Electrochim. Acta 1987, 32, 1619–1629. [Google Scholar] [CrossRef]
- Macdonald, D.D. The history of the point defect model for the passive state: A brief review of film growth aspects. Electrochim. Acta 2011, 56, 1761–1772. [Google Scholar] [CrossRef]
- Wang, W.; Alfantazi, A. An electrochemical impedance spectroscopy and polarization study of the role of crystallographic orientation on electrochemical behavior of niobium. Electrochim. Acta 2014, 131, 79–88. [Google Scholar] [CrossRef]
- Chao, C.Y.; Lin, L.F.; Macdonald, D.D. A point defect model for anodic passive films: I. Film growth kinetics. J. Electrochem. Soc. 1981, 128, 1187–1194. [Google Scholar] [CrossRef]
- Nicic, I.; Macdonald, D.D. The passivity of Type 316L stainless steel in borate buffer solution. J. Nucl. Mater. 2008, 379, 54–58. [Google Scholar] [CrossRef]
- Sharifi-Asl, S.; Taylor, M.L.; Lu, Z.; Engelhardt, G.R.; Kursten, B.; Macdonald, D.D. Modeling of the electrochemical impedance spectroscopic behavior of passive iron using a genetic algorithm approach. Electrochim. Acta 2013, 102, 161–173. [Google Scholar] [CrossRef]
- Wang, M.; Zhou, Z.; Wang, Q.; Wu, L.; Wang, Z.; Zhang, X. Long term semiconducting and passive film properties of a novel dense FeCrMoCBY amorphous coating by atmospheric plasma spraying. Appl. Surf. Sci. 2019, 495, 143600. [Google Scholar] [CrossRef]
- Mao, F.; Dong, C.; Sharifi-Asl, S.; Lu, P.; Macdonald, D.D. Passivity Breakdown on Copper: Influence of Chloride Ion. Electrochim. Acta 2014, 144, 391–399. [Google Scholar] [CrossRef]
- Boissy, C.; Alemany-Dumont, C.; Normand, B. EIS evaluation of steady-state characteristic of 316L stainless steel passive film grown in acidic solution. Electrochem. Commun. 2013, 26, 10–12. [Google Scholar] [CrossRef]
- Niu, W.; Lillard, R.S.; Li, Z.; Ernst, F. Properties of the passive film formed on interstitially hardened AISI 316L stainless steel. Electrochim. Acta 2015, 176, 410–419. [Google Scholar] [CrossRef]
- Gao, S.; Dong, C.; Luo, H.; Xiao, K.; Li, X. Electrochemical behavior and nonlinear Mott-Schottky characterization of a stainless steel passive film. Anal. Lett. 2014, 47, 1162–1181. [Google Scholar] [CrossRef]
- Jinlong, L.; Hongyun, L. Comparison of corrosion properties of passive films formed on phase reversion induced nano/ultrafine-grained 321 stainless steel. Appl. Surf. Sci. 2013, 280, 124–131. [Google Scholar] [CrossRef]
- Meng, G.; Shao, Y.; Zhang, T.; Zhang, Y.; Wang, F. Synthesis and corrosion property of pure Ni with a high density of nanoscale twins. Electrochim. Acta 2008, 53, 5923–5926. [Google Scholar] [CrossRef]
- Jinlong, L.; Tongxiang, L.; Chen, W.; Wenli, G. Investigation of passive films formed on the surface of Alloy 690 in borate buffer solution. J. Nucl. Mater. 2015, 465, 418–423. [Google Scholar] [CrossRef]
- Macdonald, D.D.; Scott, A.C.; Wentrcek, P. External reference electrodes for use in high temperature aqueous systems. J. Electrochem. Soc. 1979, 126, 908–911. [Google Scholar] [CrossRef]
- Fattah-alhosseini, A.; Ansari, A.R.; Mazaheri, Y.; Keshavarz, M.K. Effect of immersion time on the passive and electrochemical response of annealed and nano-grained commercial pure titanium in Ringer’s physiological solution at 37 °C. Mater. Sci. Eng. C 2017, 71, 771–779. [Google Scholar] [CrossRef]
- Jelliti, S.; Richard, C.; Retraint, D.; Roland, T.; Chemkhi, M.; Demangel, C. Effect of surface nanocrystallization on the corrosion behavior of Ti–6Al–4V titanium alloy. Surf. Coat. Tech. 2013, 224, 82–87. [Google Scholar] [CrossRef]
- Yue, Y.; Liu, C.; Asselin, E.; Shi, P.; Jiang, M. Kinetics of passive film growth on 304 stainless steel in H2SO4 pickling solution under chemical oxidation. Corrosion 2018, 74, 705–714. [Google Scholar] [CrossRef]
- Cook, W.G.; Olive, R.P. Pourbaix diagrams for chromium, aluminum and titanium extended to high-subcritical and low-supercritical conditions. Corros. Sci. 2012, 58, 291–298. [Google Scholar] [CrossRef]
- Priyantha, N.; Jayaweera, P.; Macdonald, D.D.; Sun, A. An electrochemical impedance study of Alloy 22 in NaCl brine at elevated temperature. I. Corrosion behavior. J. Electroanal. Chem. 2004, 572, 409–419. [Google Scholar] [CrossRef]
- Nickchi, T.; Alfantazi, A. Electrochemical corrosion behaviour of Incoloy 800 in sulphate solutions containing hydrogen peroxide. Corros. Sci. 2010, 52, 4035–4045. [Google Scholar] [CrossRef]
- Mao, F.; Yao, J.; Zhou, Y.; Dong, C.; Kursten, B.; Macdonald, D.D. Determining the electric-field strength in a passive film via photo-induced electric fields. Corros. Sci. 2019, 154, 239–245. [Google Scholar] [CrossRef]
- Macdonald, D.D. Theory of steady-state passive films. J. Electrochem. Soc. 1990, 137, 2395–2402. [Google Scholar] [CrossRef]
- Macdonald, D.D. Steady-state passive films. J. Electrochem. Soc. 1992, 139, 170–177. [Google Scholar] [CrossRef]
- Zhang, L.; Macdonald, D.D.; Sikora, E.; Sikora, J. On the kinetics of growth of anodic oxide films. J. Electrochem. Soc. 1998, 145, 898–905. [Google Scholar] [CrossRef]
- Bojinov, M.; Fabricius, G.; Laitinen, T.; Mäkelä, K.; Saario, T.; Sundholm, G. Coupling between ionic defect structure and electronic conduction in passive films on iron, chromium and iron—Chromium alloys. Electrochim. Acta 2000, 45, 2029–2048. [Google Scholar] [CrossRef]
- Krishnamurthy, B.; White, R.E.; Ploehn, H.J. Electric field strength effects on time-dependent passivation of metal surfaces. Electrochim. Acta 2002, 47, 2505–2513. [Google Scholar] [CrossRef]
- Chao, C.Y.; Lin, L.F.; Macdonald, D.D. A point defect model for anodic passive films: III. Impedance response. J. Electrochem. Soc. 1982, 129, 1874–1879. [Google Scholar] [CrossRef]


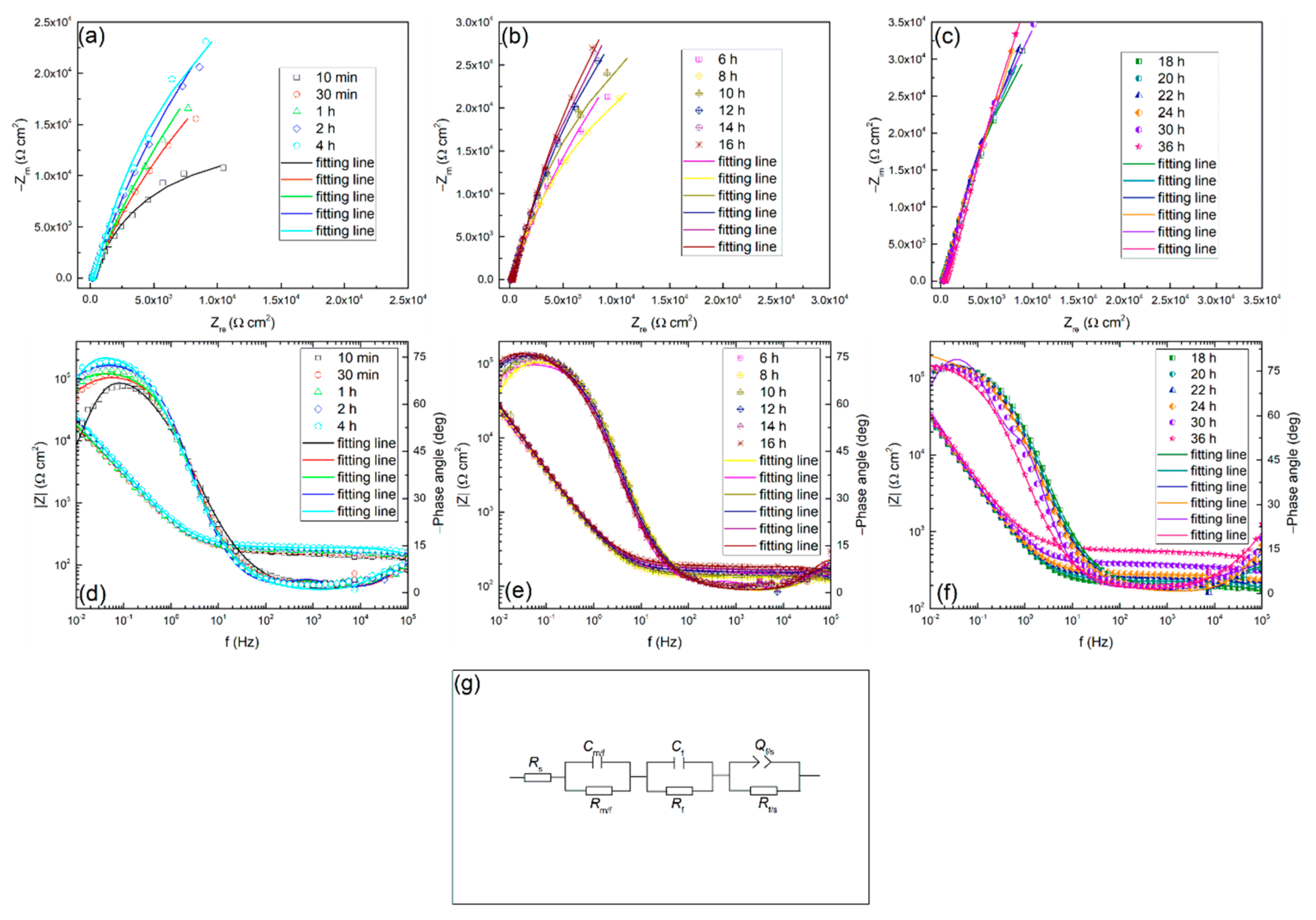
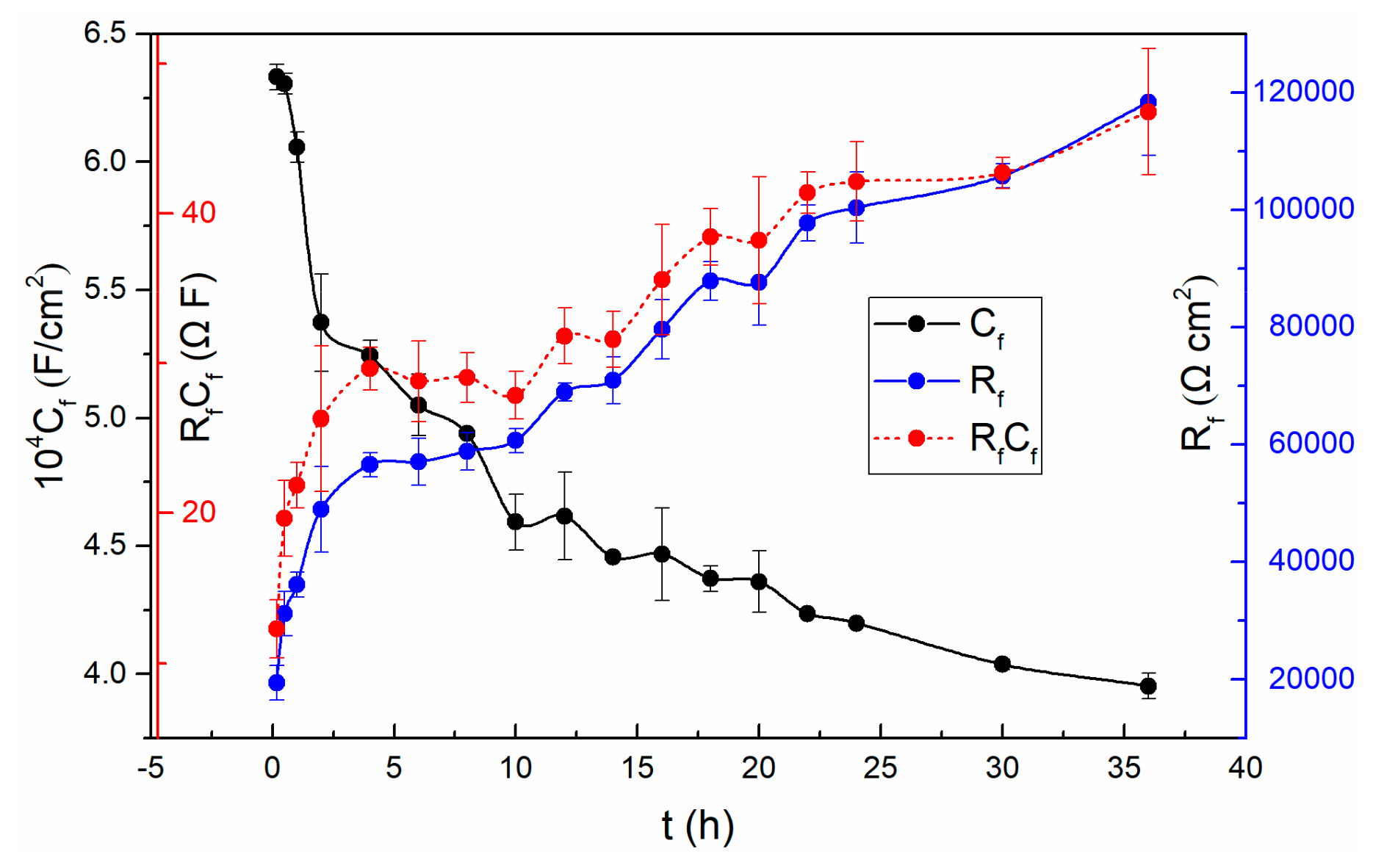

| t h | Rs Ω·cm2 | Cm/f × 104 F·cm−2 | Rm/f Ω·cm2 | Cf × 104 F·cm−2 | Rf Ω·cm2 | Y0,f/s × 104 S sn·cm−2 | n | Rf/s Ω cm2 | χ2 ×104 |
|---|---|---|---|---|---|---|---|---|---|
| 0.166 | 51.05 | 3.410 | 350.7 | 6.332 | 19,410 | 4.372 | 0.6785 | 2399 | 1.78 |
| 0.5 | 52.25 | 4.300 | 379.0 | 6.306 | 31,190 | 2.095 | 0.7971 | 2286 | 1.49 |
| 1 | 53.56 | 4.580 | 384.9 | 6.058 | 36,110 | 2.443 | 0.6995 | 2417 | 1.45 |
| 2 | 46.48 | 4.759 | 354.6 | 5.372 | 48,990 | 2.135 | 0.6973 | 4037 | 1.35 |
| 4 | 46.24 | 4.948 | 360.5 | 5.243 | 56,550 | 2.073 | 0.7854 | 2617 | 1.18 |
| 6 | 44.06 | 4.563 | 337.5 | 5.050 | 57,040 | 2.317 | 0.7783 | 3865 | 1.04 |
| 8 | 41.98 | 4.452 | 329.3 | 4.939 | 58,830 | 2.187 | 0.7454 | 3845 | 1.10 |
| 10 | 46.43 | 4.408 | 313.3 | 4.593 | 60,680 | 2.346 | 0.8434 | 4191 | 1.16 |
| 12 | 50.55 | 4.254 | 359.1 | 4.617 | 68,960 | 1.911 | 0.7064 | 3430 | 1.28 |
| 14 | 56.78 | 4.021 | 344.2 | 4.456 | 70,940 | 1.426 | 0.7716 | 4280 | 1.18 |
| 16 | 54.42 | 3.971 | 383.7 | 4.468 | 79,670 | 1.522 | 0.7868 | 4167 | 1.18 |
| 18 | 52.47 | 3.868 | 387.0 | 4.373 | 87,910 | 1.454 | 0.8450 | 4417 | 1.16 |
| 20 | 58.60 | 3.889 | 417.4 | 4.360 | 87,650 | 1.433 | 0.8270 | 3013 | 1.29 |
| 22 | 47.32 | 3.777 | 421.7 | 4.235 | 97,770 | 1.301 | 0.7990 | 4906 | 1.14 |
| 24 | 59.16 | 3.674 | 448.7 | 4.199 | 100,400 | 1.385 | 0.8071 | 3283 | 2.18 |
| 30 | 55.41 | 3.415 | 527.8 | 4.038 | 105,800 | 1.229 | 0.8180 | 4217 | 2.05 |
| 36 | 50.24 | 3.183 | 722.3 | 3.954 | 118,400 | 1.247 | 0.7490 | 3589 | 4.84 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zha, L.; Li, H.; Wang, N. In Situ Electrochemical Study of the Growth Kinetics of Passive Film on TC11 Alloy in Sulfate Solution at 300 °C/10 MPa. Materials 2020, 13, 1135. https://doi.org/10.3390/ma13051135
Zha L, Li H, Wang N. In Situ Electrochemical Study of the Growth Kinetics of Passive Film on TC11 Alloy in Sulfate Solution at 300 °C/10 MPa. Materials. 2020; 13(5):1135. https://doi.org/10.3390/ma13051135
Chicago/Turabian StyleZha, Lei, Heping Li, and Ning Wang. 2020. "In Situ Electrochemical Study of the Growth Kinetics of Passive Film on TC11 Alloy in Sulfate Solution at 300 °C/10 MPa" Materials 13, no. 5: 1135. https://doi.org/10.3390/ma13051135
APA StyleZha, L., Li, H., & Wang, N. (2020). In Situ Electrochemical Study of the Growth Kinetics of Passive Film on TC11 Alloy in Sulfate Solution at 300 °C/10 MPa. Materials, 13(5), 1135. https://doi.org/10.3390/ma13051135




