Experimental Insights into Concrete Flow-Regimes Subject to Shear-Induced Particle Migration (SIPM) during Pumping
Abstract
:1. Introduction
2. Theoretical Background
2.1. Rheology of Concrete—Single-Phase Fluid or Multiphase Suspension?
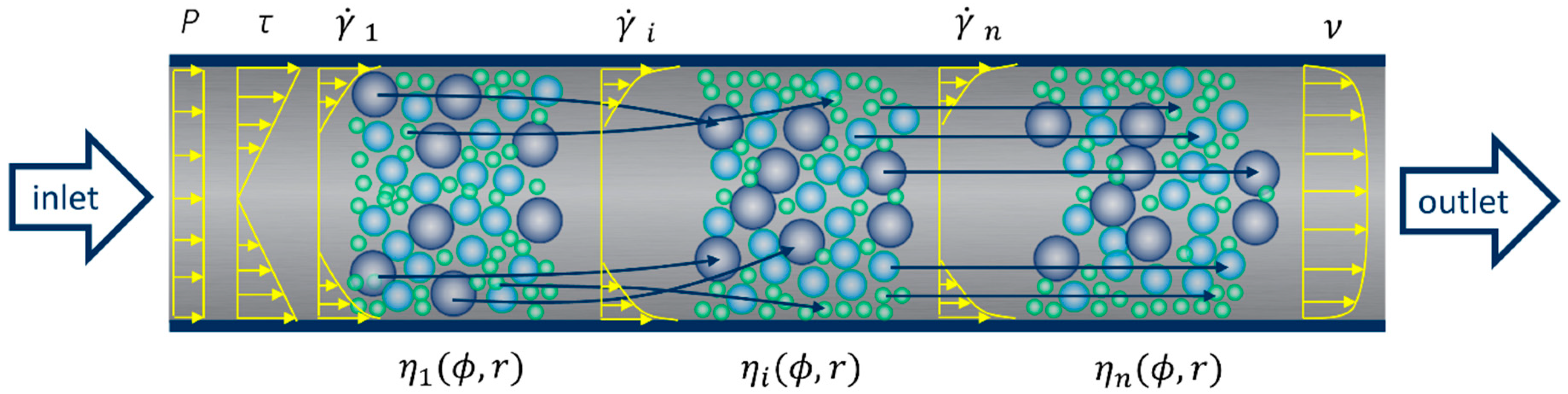
2.2. Pumping of Concrete—Modeling as Two Immiscible Single-Phase Fluids or MultiPhase Suspension with Varying Rheological Properties?
3. Materials and Methods
3.1. Materials
3.2. Rheological Measurements
3.3. Methodologies for Capturing the Lubricating Layer and Shear-Induced Particle Migration
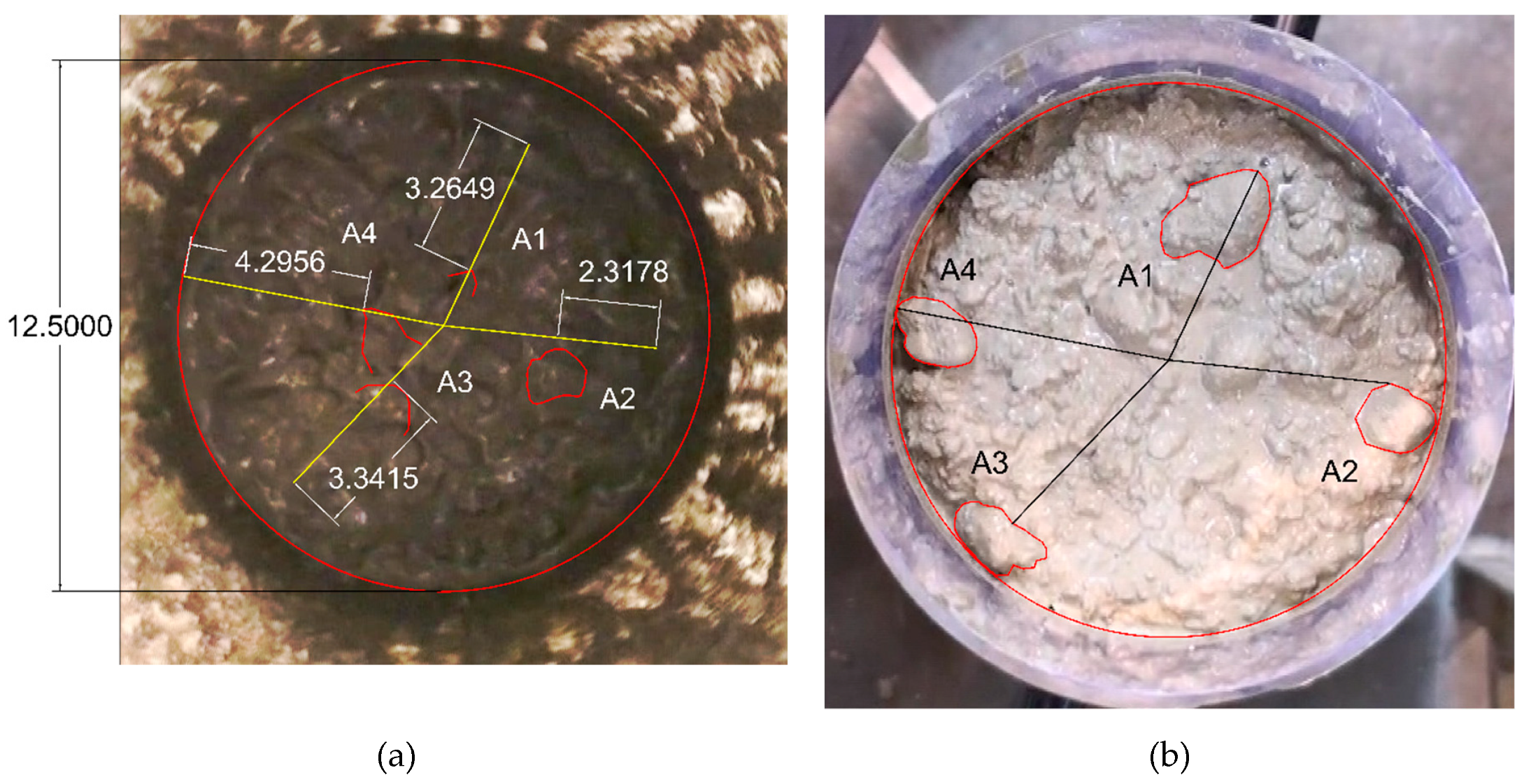
3.3.1. Quantification of Radial/Angular Displacement of Aggregates in Fresh Concrete
3.3.2. Sampling of Pumped Concrete
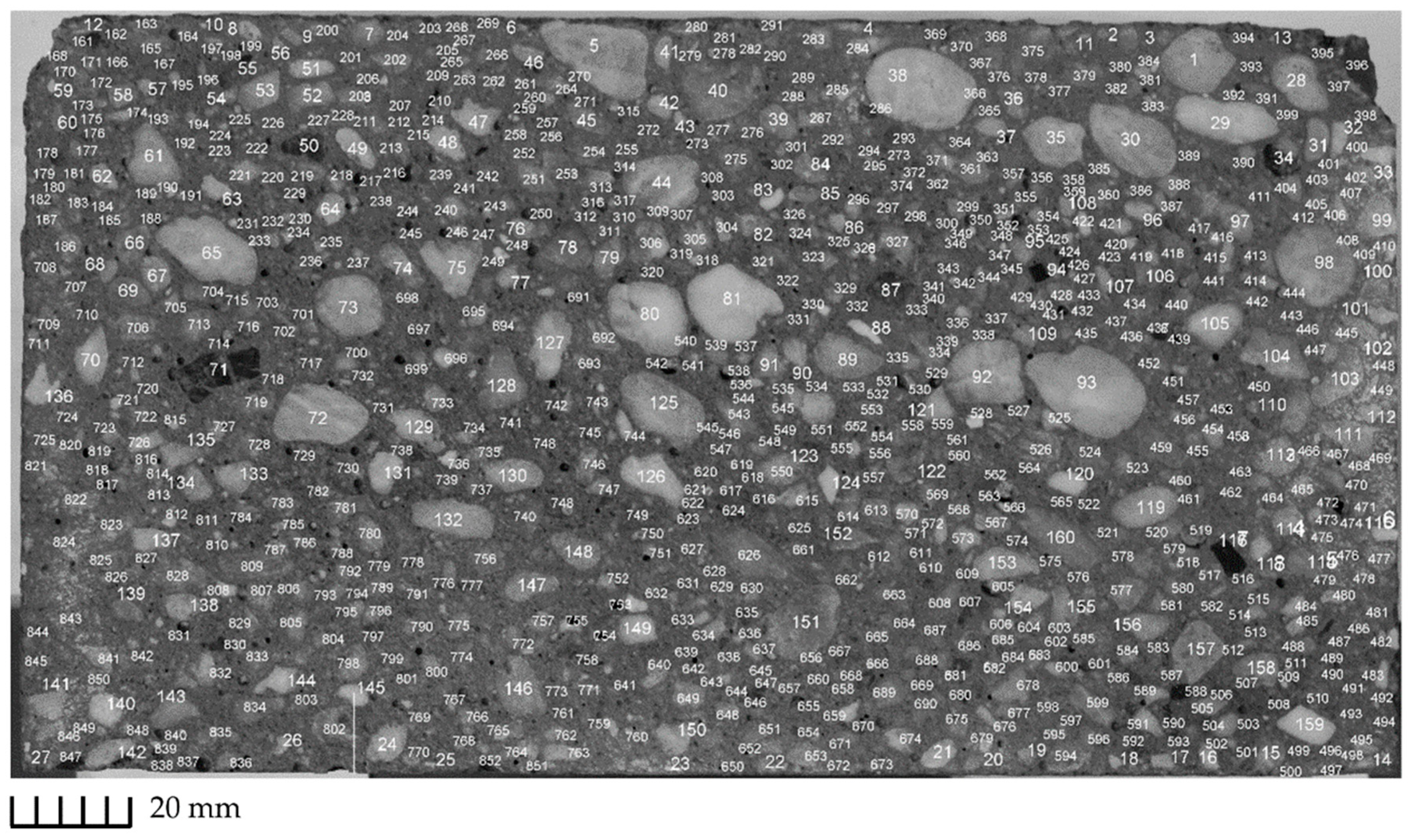
3.3.3. X-ray Microcomputed Tomography (μCT)
4. Results and Discussion
4.1. Impact of Aggregates’ Volume-Fraction on Rheological Properties of Concrete and Constitutive Mortar
4.2. Concrete Rheology during Pumping
4.3. Experimentally Determined Thickness of Lubricating Layer
- With an increasing volume-fraction of coarse particles, the thickness of the lubricating layer decreases: the migration of coarse particles is hindered when the local volume-fraction of particles in the neighboring region reaches [3,9]. With a larger , the equilibrium is reached faster and closer to the pipe wall; see Equation (8). Hence, a thinner lubricating layer develops ( and ) [3].
- With an increasing rate of discharge, the thickness of the lubricating layer increases: at higher discharge rates, shear rate, and its changes with respect to radial axis ( and , as described by Equation (7)) increase, and so does the intensity of particle migration. Since higher discharge rates were measured for CVC than for SCC (), a thicker LL could be determined for CVC ( and ), despite the fact of self-compacting concrete’s containing in general a higher volume-fraction of fine particles in comparison to conventional concrete (); see Figure 9. The total content of fine particles, including binder and fine sand, in S42 and S47 was 4.59% and 4.29% higher than that in C42 and C47, respectively.
4.4. Particle Distribution in Radial Direction
- The number of traceable particles within the range 1–2 mm was often limited to one-fourth (1/4) of the expected values (expected: , measured values: for S47 and for C47). Therefore, the tracking of particles smaller than 2 mm in this method would not provide any additional information.
- The average surface area fractions for the particle groups 2–4 mm and 4–8 mm are larger than the expected values for both mixtures, expected: and . On the contrary, for the particle group 8–16 mm, the average surface area is always less than expected. This is due to the irregular shape of the particles and their random orientation in the target cross-section.
- For particle size ranges 2–4 mm, 4–8 mm, and 8–16 mm a clear peak can be observed. It seems that the larger the particles are, the greater the distance of the peak to the pipe wall. Furthermore, the peaks are shifted from the maximum aggregate size for each group. This indicates that both wall-effect and SIPM influenced the radial displacement of the particles.
4.5. Evaluation of Surface Profile during Sliper Tests
5. Conclusions and Outlook
- The Bingham rheological parameters of concrete increase with increasing aggregates’ volume-fraction (). For CVC the changes are pronounced in the yield stress values in the first place, while in the case of SCC it is the plastic viscosity, which alters significantly.
- The thickness of the lubricating layer was measured on hardened concrete samples investigated after performing the Sliper tests. The thickness of the lubricating layer was not constant for the concrete compositions under investigation and varied under different discharge rates. The thickness of the lubricating layer increases with decreasing aggregate volume-fraction and with increasing discharge rate.
- The analytical thickness of the lubricating layer can be calculated using the rheological properties of concrete bulk and the lubricating layer and the output of Sliper. However, these values do not represent reality. The analytical thickness is suitable for eliminating the device-dependency of experimental rheological parameters for analytical or numerical modeling of the pumping process.
- Concrete plastic viscosity is the most influential parameter affecting the shape of the parabolic curve of velocity profile.
- In modern concretes with high volume-fractions of solid particles, the shear-induced particle migration can be severe enough to influence the local rheological properties of the pumping concrete and therefore, must be taken into consideration. In such cases, conventional analytical models using the rheological parameters obtained by means of concrete rheometers, are not sufficiently reliable to predict concrete pumping behavior.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Giesekus, H. Chapter 8 Viskose Flüssigkeiten. In Phänomenologische Rheologie; Springer-Verlag Berlin Heidelberg GmbH: Dortmund, Germany, 1994; pp. 155–166. [Google Scholar]
- Heirman, G.; Vandewalle, L.; Van Gemert, D.; Wallevik, Ó. Integration approach of the Couette inverse problem of powder type self-compacting concrete in a wide-gap concentric cylinder rheometer. J. Nonnewton Fluid Mech. 2008, 150, 93–103. [Google Scholar] [CrossRef] [Green Version]
- Spangenberg, J.; Roussel, N.; Hattel, J.H.; Stang, H.; Skocek, J.; Geiker, M.R. Flow induced particle migration in fresh concrete: Theoretical frame, numerical simulations and experimental results on model fluids. Cem. Concr. Res. 2012, 42, 633–641. [Google Scholar] [CrossRef]
- Browne, R.D.; Bamforth, P.B. Tests to establish concrete pumpability. ACI J Proc. 1977, 74, 93–203. [Google Scholar]
- Kaplan, D. Pompage des bétons. Ph.D. Thesis, Laboratoire Central des Ponts et Chaussées, Paris, France, 7 August 2000. [Google Scholar]
- Choi, M.; Roussel, N.; Kim, Y.; Kim, J. Lubrication layer properties during concrete pumping. Cem. Concr. Res. 2013, 45, 69–78. [Google Scholar] [CrossRef]
- Secrieru, E. Pumping behaviour of modern concretes—Characterisation and prediction. Ph.D. Thesis, TU Dresden, Dresden, Germany, 30 January 2018. [Google Scholar]
- Choi, M.S. Numerical Prediction on the Effects of the Coarse Aggregate Size to the Pipe Flow of Pumped Concrete. J Adv. Concr. Technol. 2014, 12, 239–249. [Google Scholar] [CrossRef] [Green Version]
- Phillips, R.J.; Armstrong, R.C.; Brown, R.A.; Graham, A.L.; Abbott, J.R. A constitutive equation for concentrated suspensions that accounts for shear- induced particle migration. Phys. Fluids A Fluid Dyn. 1992, 4, 30–40. [Google Scholar] [CrossRef]
- Nott, P.R.; Guazzelli, E.; Pouliquen, O. The suspension balance model revisited. Phys. Fluids 2011, 23, 43304. [Google Scholar] [CrossRef] [Green Version]
- Spangenberg, J.; Roussel, N.; Hattel, J.H.; Thorborg, J.; Geiker, M.R.; Stang, H.; Skocek, J. Prediction of flow induced heterogeneities and their consequences in Self Compacting Concrete (SCC). In Proceedings of the SCC2010, Montreal, Canada, 26–29 September 2010. [Google Scholar]
- Guazzelli, É.; Pouliquen, O. Rheology of dense granular suspensions. J. Fluid Mech. 2018, 852, P11–P173. [Google Scholar] [CrossRef] [Green Version]
- Ovarlez, G.; Mahaut, F.; Deboeuf, S.; Lenoir, N.; Hormozi, S.; Chateau, X. Flows of suspensions of particles in yield stress fluids. J. Rheol. 2015, 59, 1449–1486. [Google Scholar] [CrossRef] [Green Version]
- Ovarlez, G.; Bertrand, F.; Rodts, S. Local determination of the constitutive law of a dense suspension of noncolloidal particles through magnetic resonance imaging. J. Rheol. 2006, 50, 259. [Google Scholar] [CrossRef] [Green Version]
- Leighton, D.; Acrivos, A. The Shear-Induced Migration of Particles in Concentrated Suspensions. J. Fluid Mech. 1987, 181, 415–439. [Google Scholar] [CrossRef]
- Shauly, A.; Wachs, A.; Nir, A. Shear-induced particle migration in a polydisperse concentrated suspension. J. Rheol. 1998, 42, 1329–1348. [Google Scholar] [CrossRef]
- Kaplan, D.; de Larrard, F.; Sedran, T. Design of Concrete Pumping Circuit. ACI Mater. J. 2005, 102, 110–117. [Google Scholar]
- Feys, D.; Khayat, K.H.; Perez-schell, A.; Khatib, R. Prediction of pumping pressure by means of new tribometer for highly-workable concrete. Cem. Concr. Compos. 2015, 57, 102–115. [Google Scholar] [CrossRef]
- Secrieru, E.; Cotardo, D.; Mechtcherine, V.; Lohaus, L.; Schröfl, C.; Begemann, C. Changes in concrete properties during pumping and formation of lubricating material under pressure. Cem. Concr. Res. 2018, 108, 129–139. [Google Scholar] [CrossRef]
- Morrison, F.A. 3. Newtonian Fluid Mechanics. In Understanding Rheology; Oxford University Press: New York, NY, USA, 2001. [Google Scholar]
- De Larrard, F. Packing process used. In Concrete Mixture Proportioning: A Scientific Approach; E & FN Spon: London, UK, 1999; pp. 16–18. [Google Scholar]
- Feys, D. Interactions between rheological properties and pumping of Self-Compacting Concrete. Ph.D. Thesis, U Ghent, Ghent, Belgium, 15 May 2009. [Google Scholar]
- Kwon, H.; Park, C.K.; Jeong, J.H.; Jo, S.D.; Lee, S.H. Prediction of Concrete Pumping: Part II—Analytical Prediction and Experimental Verification. ACI Mater. J. 2013, 110, 657–668. [Google Scholar]
- Le, H.D.; Kadri, E.H.; Aggoun, S.; Vierendeels, J.; Troch, P.; De Schutter, G. Effect of lubrication layer on velocity profile of concrete in a pumping pipe. Mater. Struct. Constr. 2015, 48, 3991–4003. [Google Scholar] [CrossRef]
- Nott, R.P.; Brady, J.F. Pressure-driven flow of suspensions: Simulation and theory. J. Fluid Mech. 1994, 275, 157–199. [Google Scholar] [CrossRef] [Green Version]
- Lam, Y.C.; Chen, X.; Tan, K.W.; Chai, J.C.; Yu, S.C.M. Numerical investigation of particle migration in poiseuille flow of composite system. Compos. Sci. Technol. 2004, 64, 1001–1010. [Google Scholar] [CrossRef]
- Jo, S.D.; Park, C.K.; Jeong, J.H.; Lee, S.H.; Kwon, S.H. A computational approach to estimating a lubricating layer in concrete pumping. Comput. Mater. Contin. 2012, 27, 189–210. [Google Scholar]
- Monsorno, D.; Varsakelis, C.; Papalexandris, M.V. Poiseuille flow of dense non-colloidal suspensions: The role of intergranular and nonlocal stresses in particle migration. J. Nonnewton Fluid Mech. 2017, 247, 229–238. [Google Scholar] [CrossRef]
- Testing Fresh Concrete—Part 5: Flow Table Test, German version EN 12350-5; German Institute for Standardisation (Deutsches Institut für Normung): Berlin, Germany, 2019.
- Testing Fresh Concrete—Part 8: Self-Compacting Concrete—Slump-Flow Test, German version EN 12350-8; German Institute for Standardisation (Deutsches Institut für Normung): Berlin, Germany, 2019.
- Building Lime—Part 2: Test Methods, German version EN 459-2; German Institute for Standardisation (Deutsches Institut für Normung): Berlin, Germany, 2010.
- Secrieru, E.; Fataei, S.; Schröfl, C.; Mechtcherine, V. Study on concrete pumpability combining different laboratory tools and linkage to rheology. Constr. Build. Mater. 2017, 144, 451–461. [Google Scholar] [CrossRef]
- Haist, M. Zur Rheologie und den Ohysikalischen Wechselwirkungen bei Zementsuspensionen. Ph.D. Thesis, Universität Karlsruhe (TH), Karlsruhe, Germany, 4 February 2009. [Google Scholar]
- Feys, D.; Wallevik, J.E.; Yahia, A.; Khayat, K.H.; Wallevik, O.H. Extension of the Reiner–Riwlin equation to determine modified Bingham parameters measured in coaxial cylinders rheometers. Mater. Struct. 2012, 46, 289–311. [Google Scholar] [CrossRef]
- Heirman, G.; Van Gemert, D.; Vandewalle, L.; Toorman, E.; Wallevik, J.E.; Wallevik, O.H. Influence of Plug Flow When Testing Shear Thickening Powder Type Self-Compacting Concrete in a Wide-Gap Concentric Cylinder Rheometer. In Proceedings of the 3rd International RILEM Symposium on Rheology of Cement Suspensions such as Fresh Concrete, Reykjavik, Iceland, 19–21 August 2009. [Google Scholar]
- Mechtcherine, V.; Nerella, V.N.; Kasten, K. Testing pumpability of concrete using Sliding Pipe Rheometer. Constr. Build. Mater. 2014, 53, 312–323. [Google Scholar] [CrossRef]
- Secrieru, E.; Mohamed, W.; Fataei, S.; Mechtcherine, V. Assessment and prediction of concrete flow and pumping pressure in pipeline. Cem. Concr. Compos. 2020, 107, 103495. [Google Scholar] [CrossRef]
- Feys, D. Understanding the pumping of conventional vibrated and self-compacting concrete. In Understanding the Rheology of Concrete; Woodhead Publishing Limited: Cambridge, UK, 2011; pp. 331–353. [Google Scholar]
- Testing Hardened Concrete—Part 2: Making and Curing Specimens for Strength Tests, German version EN 12390-2; German Institute for Standardisation (Deutsches Institut für Normung): Berlin, Germany, 2019.
- Feys, D.; Verhoeven, R.; De Schutter, G. Why is fresh self-compacting concrete shear thickening? Cem. Concr. Res. 2009, 39, 510–523. [Google Scholar] [CrossRef]
- Choi, M.S.; Kim, Y.J.; Jang, K.P.; Kwon, S.H. Effect of the coarse aggregate size on pipe flow of pumped concrete. Constr. Build. Mater. 2014, 66, 723–730. [Google Scholar] [CrossRef]
- Ferraris, C.F.; Brower, L.E.; Banfill, P.; Beaupré, D.; Chapdelaine, F.; de Larrard, F.; Domone, P.; Nachbaur, L.; Sedran, T.; Wallevik, O.H.; et al. Comparison of Concrete Rheometers: International Tests at LCPC (Nantes, France) in October, 2000; US Department of Commerce, NIST: Gaithersburg, MD, USA, 2001.
- Feys, D.; Khayat, K.H.; Perez-schell, A.; Khatib, R. Relation between Rheological and Tribological Properties of Highly-Workable Concrete, in view of Estimating Pumping Pressures. In Proceedings of the 5th North American Conference on the Design and Use of Self-Consolidating Concrete, Chicago, IL, USA, 12–15 May 2013. [Google Scholar]
- Jacobsen, S.; Haugan, L.; Hammer, T.A.; Kalogiannidis, E. Flow conditions of fresh mortar and concrete in different pipes. Cem. Concr. Res. 2009, 39, 997–1006. [Google Scholar] [CrossRef]









| Materials | Density (kg/m3) | Dosage (kg) for 1 m3 of Concrete | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| C42 | C47 | C52 | C47c | S37 | S42 | S47 | S42c | |||
| Constitutive mortar | CEM III/A 42.5 N | 2990 | 425 | 388 | 351 | 388 | 392 | 361 | 330 | 361 |
| Fly ash | 2200 | - | - | - | - | 239 | 220 | 201 | 220 | |
| Quartz powder | 2680 | 57 | 52 | 47 | 52 | 52 | 48 | 44 | 48 | |
| Quartz sand 0.06–0.2 * | 2650 | 57 | 52 | 47 | 52 | 52 | 48 | 44 | 48 | |
| Quartz sand 0–1 * | 2650 | 456 | 416 | 376 | 416 | 420 | 387 | 354 | 387 | |
| Water | 1000 | 211 | 192 | 173 | 192 | 180 | 166 | 152 | 166 | |
| Superplasticizer (SP) | 1056 | 3.48 | 3.18 | 2.87 | 3.18 | 11.14 | 10.26 | 9.38 | 10.26 | |
| Aggregates | Quartz sand 1–2 * | 2650 | 210 | 235 | 260 | 260 | 185 | 210 | 235 | 210 |
| Quartz sand 2–4 * | 2650 | 210 | 235 | 260 | 260 | 185 | 210 | 235 | 210 | |
| Quartz gravel 4–8 * | 2650 | 290 | 325 | 360 | 360 | 255 | 290 | 325 | 290 | |
| Quartz gravel 8–16 * | 2650 | 403 | 451 | 500 | - | 355 | 403 | 451 | - | |
| Basalt 8–11 * (crushed) | 2900 | - | - | - | 197 | - | - | - | 176 | |
| Basalt 11–16 * (crushed) | 2900 | - | - | - | 300 | - | - | - | 268 | |
| w/b (-) | 0.50 | 0.50 | 0.50 | 0.50 | 0.30 | 0.30 | 0.30 | 0.30 | ||
| SP % bwob ** | 0.72 | 0.72 | 0.72 | 0.72 | 1.77 | 1.77 | 1.77 | 1.77 | ||
| of agg. (> 1mm) (-) | 0.42 | 0.47 | 0.52 | 0.47 | 0.37 | 0.42 | 0.47 | 0.42 | ||
| (> 1mm) (-) | 0.585 | 0.654 | 0.724 | 0.667 | 0.515 | 0.585 | 0.654 | 0.596 | ||
| FT/SF for concrete † (mm) | 640 | 545 | 450 | 530 | 720 | 680 | 600 | 650 | ||
| FT for constitutive mortar ‡ (mm) | 260 | 260 | 240 | 240 | 300 | 290 | 250 | 250 | ||
| Air content (%) | 1.7 | 2.7 | 1.3 | 1.6 | 0.7 | 0.8 | 0.4 | 1.0 | ||
| Density (kg/m3) | 2340 | 2320 | 2390 | 2370 | 2320 | 2360 | 2370 | 2410 | ||
| Compressive strength at 28 d (MPa) | 54.3 ± 1.3 | 50.8 ± 1.0 | 48.6 ± 1.1 | 53.7 ± 0.6 | 76.9 ± 1.6 | 78.6 ± 2.0 | 72.1 ± 0.7 | 81.1 ± 1.1 | ||
| Device | Material | Geometry | Testing Profile | Transformation Equation | Torque Resolution | Angular Resolution |
|---|---|---|---|---|---|---|
| ConTec 5 viscometer * | Concrete | Serrated beater = 145 mm = 100 mm = 120 mm | Hysteresis loop with rotational velocity range: 0.02 to 0.62 rps | Reiner-Riwlin [1,2]: | 0.1 Nm | 126 mrad |
| HAAKE MARS II rheometer ** | Mortar | Hollow vane = 35 mm = 26 mm = 50 mm | Hysteresis loop with rotational velocity range: 0.01 to 0.50 rps | Affine translation and calibration by reference materials [33] | 0.1 nNm | 12 nrad |
| Parameters | Mixture | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| C42 | C47 | C52 | C47c | S37 | S42 | S47 | S42c | ||
| Concretemortar | Yield stress (Pa) | 65 | 120 | 270 | 160 | 4.0 | 5.0 | 6.0 | 5.5 |
| Plastic viscosity (Pa·s) | 20 | 25 | 35 | 30 | 30 | 60 | 130 | 80 | |
| Non-linear term of MB (Pa·s2) | - | - | - | - | 5.5 | 5.0 | 1.0 | 5.5 | |
| Mortar | Yield stress (Pa) | 21 | 21 | 30 | 27 | 2.0 | 2.5 | 6.0 | 5.0 |
| Plastic viscosity (Pa·s) | 1.4 | 1.0 | 1.3 | 1.6 | 3.2 | 3.5 | 7.0 | 5.5 | |
| Non-linear term of MB (Pa·s2) | - | - | - | - | 0.3 | 0.2 | 0.5 | 0.6 | |
| Mixture | C42 | C47 | C52 | C47c | S37 | S42 | S47 | S42c |
|---|---|---|---|---|---|---|---|---|
| (mm) | 1.63 | 0.96 | 1.17 | 1.71 | 1.58 | 1.57 | 2.83 | 1.31 |
| Standard deviation (mm) | 0.18 | 0.10 | 0.14 | 0.12 | 0.11 | 0.10 | 0.44 | 0.12 |
| Mixture | C42 | C47 | C52 | C47c | S37 | S42 | S47 | S42c |
|---|---|---|---|---|---|---|---|---|
| (mm) | 1.11 | 1.04 | 0.98 | 1.25 | 1.22 | 0.95 | 0.78 | 1.06 |
| Standard deviation (mm) | 0.79 | 0.99 | 0.81 | 0.84 | 0.95 | 0.71 | 0.66 | 0.79 |
| Mixture | C42 | C47 | C52 | C47c | S37 | S42 | S47 | S42c |
|---|---|---|---|---|---|---|---|---|
| (mbar/m) | 153.89 | 184.90 | 226.20 | 194.84 | 163.72 | 229.96 | 277.14 | 185.39 |
| (cm/s) | 71.69 | 72.18 | 68.13 | 72.18 | 21.93 | 28.14 | 27.73 | 17.00 |
| (cm/s) | 7.93 | 6.53 | 4.31 | 4.24 | 3.90 | 1.85 | 0.00 | 1.85 |
| Exp. (cm) | 0 | 0 | 0 | 0 | 0 | 0 | 5.53 | 0 |
| Analyt. (cm) | 0.84 | 1.30 | 2.39 | 1.64 | 0.04 | 0.04 | 0.04 | 0.04 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fataei, S.; Secrieru, E.; Mechtcherine, V. Experimental Insights into Concrete Flow-Regimes Subject to Shear-Induced Particle Migration (SIPM) during Pumping. Materials 2020, 13, 1233. https://doi.org/10.3390/ma13051233
Fataei S, Secrieru E, Mechtcherine V. Experimental Insights into Concrete Flow-Regimes Subject to Shear-Induced Particle Migration (SIPM) during Pumping. Materials. 2020; 13(5):1233. https://doi.org/10.3390/ma13051233
Chicago/Turabian StyleFataei, Shirin, Egor Secrieru, and Viktor Mechtcherine. 2020. "Experimental Insights into Concrete Flow-Regimes Subject to Shear-Induced Particle Migration (SIPM) during Pumping" Materials 13, no. 5: 1233. https://doi.org/10.3390/ma13051233
APA StyleFataei, S., Secrieru, E., & Mechtcherine, V. (2020). Experimental Insights into Concrete Flow-Regimes Subject to Shear-Induced Particle Migration (SIPM) during Pumping. Materials, 13(5), 1233. https://doi.org/10.3390/ma13051233




