Acceleration Characteristics of Discrete Fragments Generated from Explosively-Driven Cylindrical Metal Shells
Abstract
1. Introduction
2. Theoretical Analysis
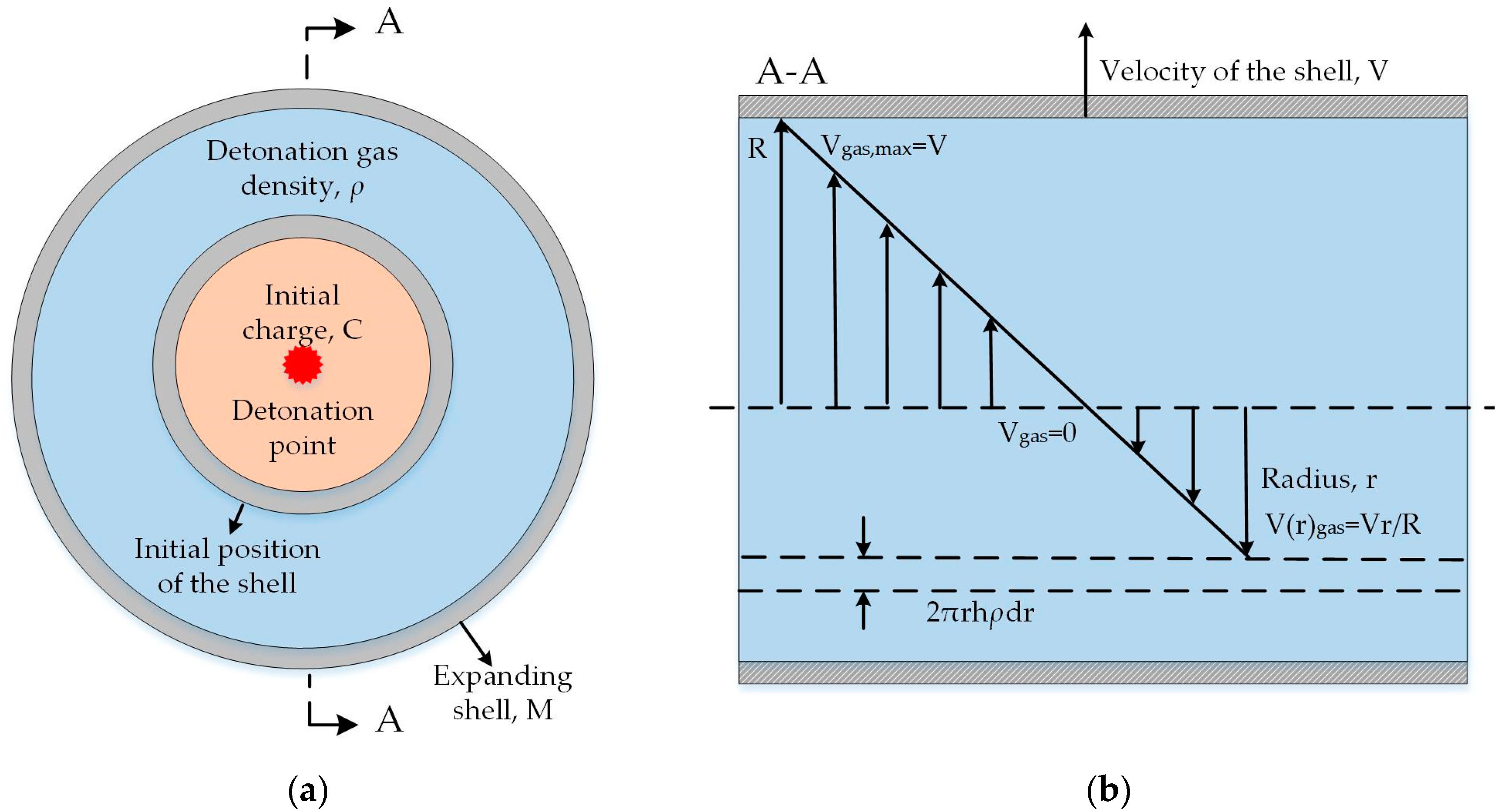
2.1. Equation of Motion
- The explosive detonates instantaneously, after which the detonation gas inside the shell has a uniform density of ρ. The velocity of the detonation gas increases linearly from the charge center to the inner surface of the shell, as shown in Figure 1b. Then, for any radial position r inside the shell, we havewhere V is the velocity of the inner surface of the shell, and R is the expanding inner radius of the shell.
- The shell fractures radially. After the shell ruptures, fragments scatter at the same radial velocity with a fixed orientation. The mass loss of the fragments during the explosion is neglected.
- The cylindrical shell is assumed to be infinite. We investigate the motion of the detonation gas and the shell cut out from the central part of the shell, where the end effect induced by the rarefaction wave can be neglected. Thus, the problem can be treated as a two-dimensional (2D) problem.
2.2. Pre-Disintegration Acceleration
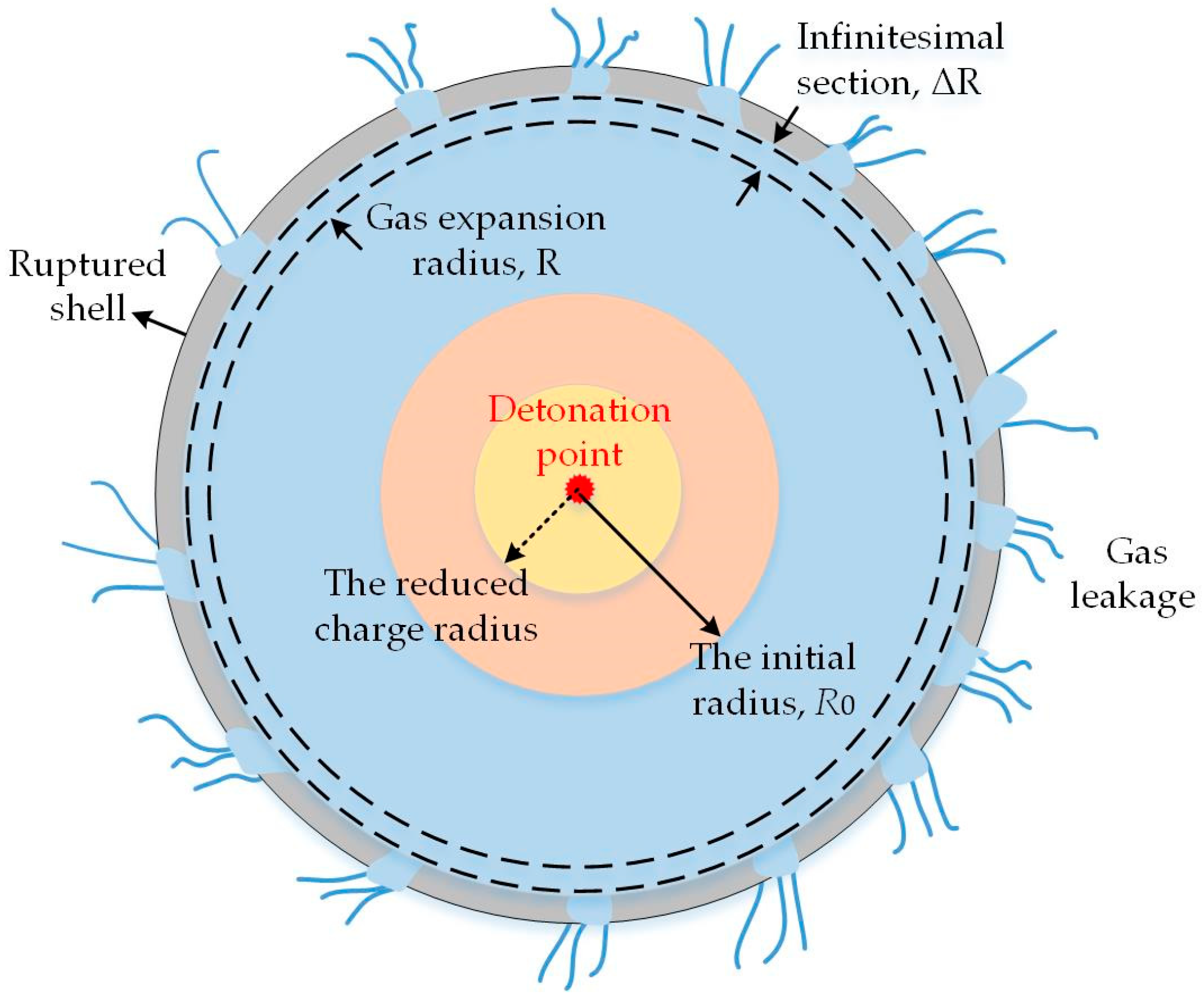
2.3. Post-Disintegration Acceleration
2.3.1. Equation for Locally Isentropic Expansion
2.3.2. Model of Gas Leakage
3. Verification of Theoretical Model
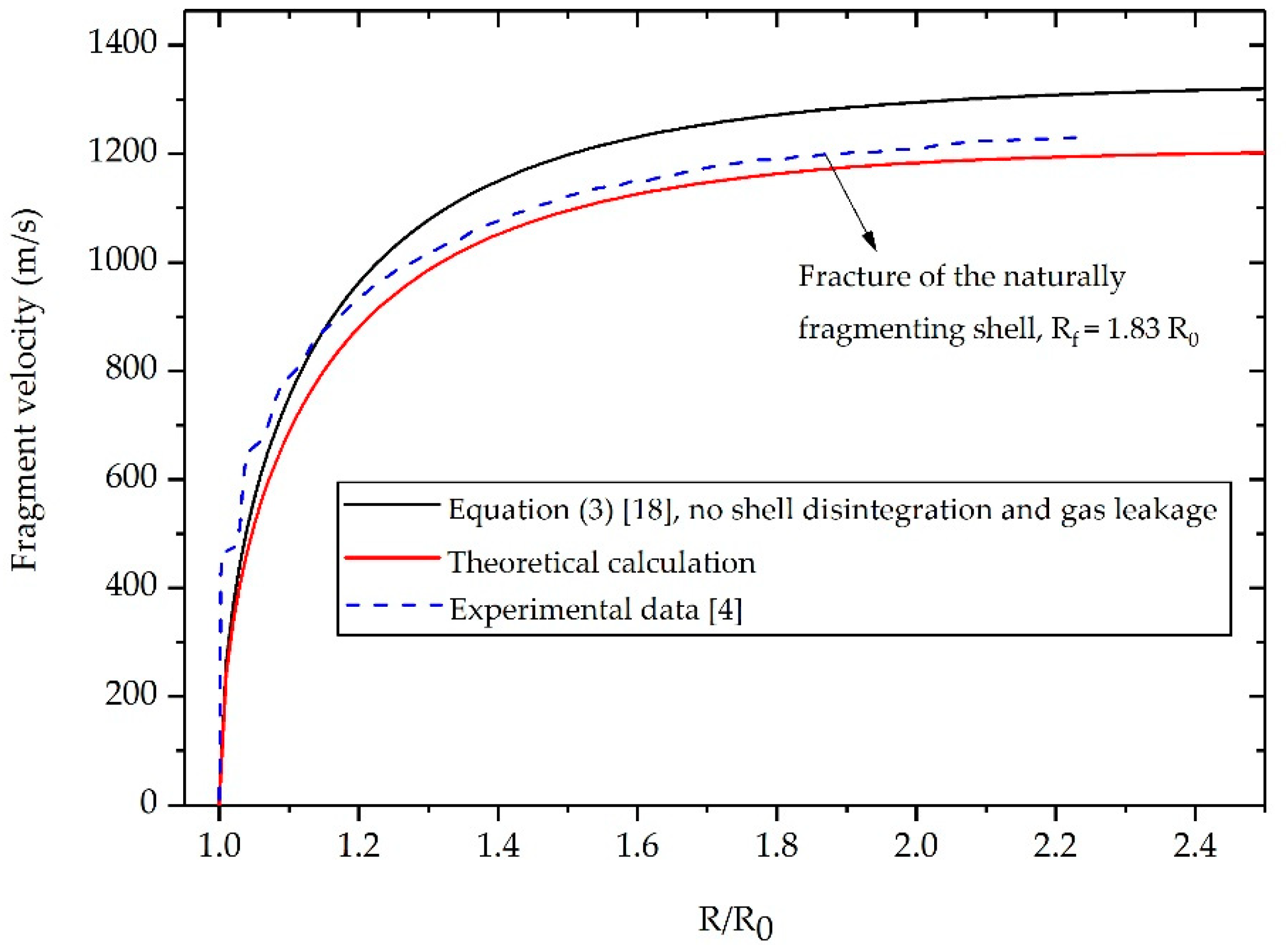
3.1. Natural Fragment Acceleration
3.2. Preformed Fragment Acceleration
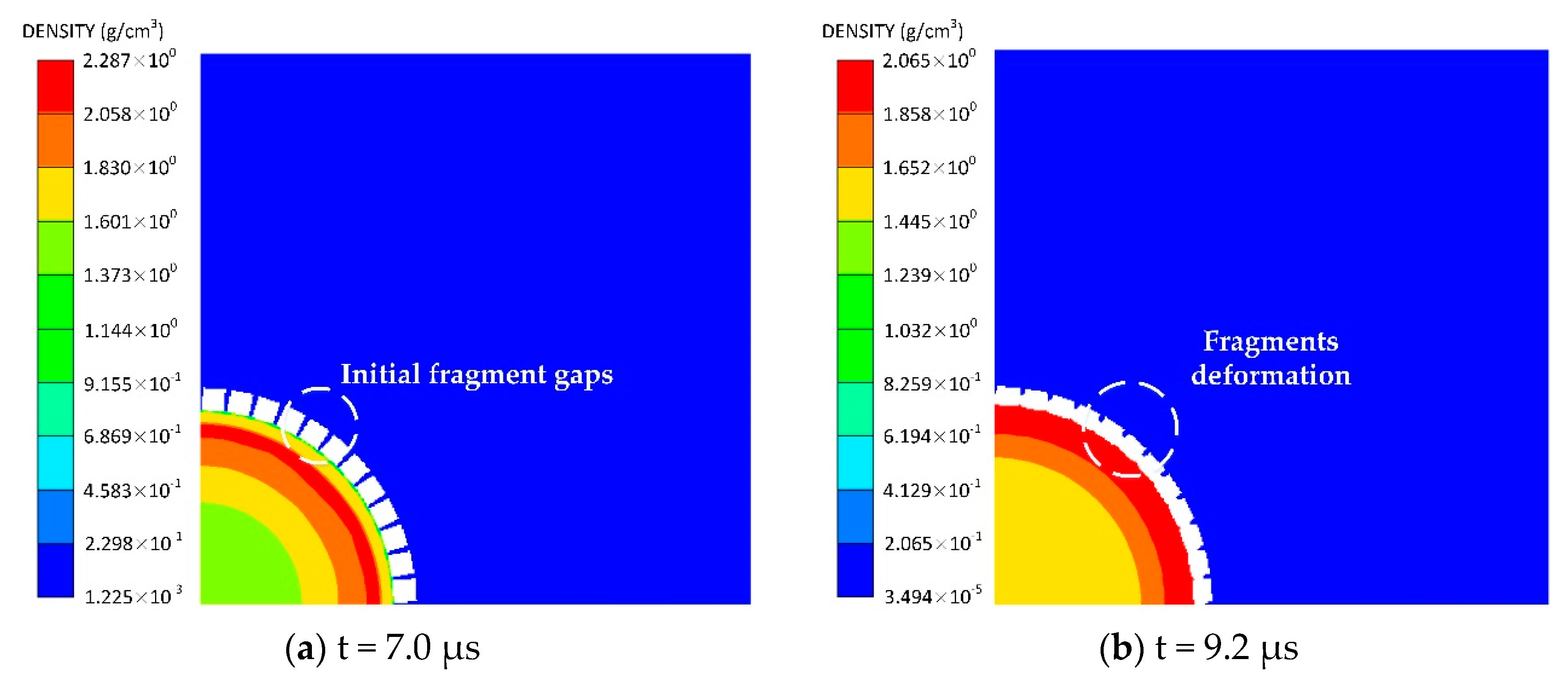
3.2.1. Numerical Simulation Model
3.2.2. Verification of Fragment Acceleration
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Karpp, R.R.; Predebon, W.W. Calculations of Fragment Velocities from Naturally Fragmenting Munitions; Ballistic Research Laboratories: Aberdeen, MD, USA, 1975. [Google Scholar] [CrossRef]
- Pearson, J. Fragmentation Model for Cylindrical Warheads; Naval Weapons Center: China Lake, CA, USA, 1990. [Google Scholar]
- Ren, G.; Guo, Z.; Fan, C.; Tang, T.; Hu, H. Dynamic shear fracture of an explosively-driven metal cylindrical shell. Int. J. Impact Eng. 2016, 95, 35–39. [Google Scholar] [CrossRef]
- Wang, X.Y.; Wang, S.S.; Ma, F. Experimental study on the expansion of metal cylinders by detonation. Int. J. Impact Eng. 2018, 114, 147–152. [Google Scholar] [CrossRef]
- Gurney, R.W. The Initial Velocities of Fragments from Bombs, Shells and Grenades; Ballistic Research Laboratories: Aberdeen, MD, USA, 1943. [Google Scholar]
- Jones, G.E. The Gurney equations for multilayered fragments. J. Appl. Phys. 1979, 50, 3746–3747. [Google Scholar] [CrossRef]
- Wang, M.; Lu, F.; Li, X.; Cao, L. A formula for calculating the velocities of fragments from velocity enhanced warhead. Propellants Explos. Pyrotech. 2013, 38, 232–237. [Google Scholar] [CrossRef]
- Li, W.; Huang, G.Y.; Feng, S.S. Effect of eccentric initiation on the fragment velocity distribution of a cylindrical casing filled with charge. Int. J. Impact Eng. 2015, 80, 107–115. [Google Scholar] [CrossRef]
- An, X.; Dong, Y.; Liu, J.; Tian, C. General formula to calculate the fragment velocity of warheads with hollow core. Int. J. Impact Eng. 2018, 113, 1–8. [Google Scholar] [CrossRef]
- Bola, M.S.; Madan, A.K.; Singh, M. Expansion of metallic cylinders under explosive loading. Def. Sci. J. 1992, 42, 157–163. [Google Scholar] [CrossRef]
- Lee, E.L.; Hornig, H.C.; Kury, J.W. Adiabatic Expansion of High Explosive Detonation Products; Univ. of California Radiation Lab: Livermore, CA, USA, 1968. [Google Scholar]
- Waggener, S. The performance of axially initiated cylindrical warheads. In Proceedings of the Fourth International Symposium on Ballistics, Monterey, CA, USA, 17–19 October 1978. [Google Scholar]
- Wang, X.; Kong, X.; Zheng, C.; Wu, W. Effect of initiation manners on the scattering characteristics of semi-preformed fragment warhead. Def. Technol. 2018, 14, 578–584. [Google Scholar] [CrossRef]
- Predebon, W.W.; Smothers, W.G.; Anderson, C.E. Missile Warhead Modeling: Computations and Experiments; Ballistic Research Laboratories: Aberdeen, MD, USA, 1977. [Google Scholar]
- Lloyd, R.M. Conventional Warhead Systems Physics and Engineering Design; American Institute of Aeronautics: Reston, VA, USA, 1998. [Google Scholar]
- Koch, A.; Arnold, N.; Estermann, M. A simple relation between the detonation velocity of an explosive and its Gurney energy. Propellants Explos. Pyrotech. 2002, 27, 365–368. [Google Scholar] [CrossRef]
- Hutchinson, M.D. Replacing the equations of fano and fisher for cased charge blast impulse—III—Yield stress method. Propellants Explos. Pyrotech. 2014, 39, 586–589. [Google Scholar] [CrossRef]
- Hutchinson, M.D.; Price, D.W. On the continued acceleration of bomb casing fragments following casing fracture. Def. Technol. 2014, 10, 211–218. [Google Scholar] [CrossRef]
- Lindsay, C.M.; Butler, G.C.; Rumchik, C.G.; Schulze, B.; Gustafson, R.; Maines, W.R. Increasing the utility of the copper cylinder expansion test. Propellants Explos. Pyrotech. 2010, 35, 433–439. [Google Scholar] [CrossRef]
- Elek, P.; Jaramaz, S.; Mickovic, D. Modeling of expansion dynamics of explosively driven metal cylinders. In Proceedings of the 27th International Symposium on Ballistics, Freiburg, Germany, 22–26 April 2013. [Google Scholar]
- Taylor, G.I. The Fragmentation of Tubular Bombs; Scientific Papers of G. I. Taylor 3; Cambridge University Press: Cambridge, UK, 1963; p. 387. [Google Scholar]
- Hoggatt, C.R.; Recht, R.F. Fracture behavior of tubular bombs. J. Appl. Phys. 1968, 39, 1856–1862. [Google Scholar] [CrossRef]
- Zhang, S.Z.; Sun, Y.B. Deformation and rupture of rigid-plastic cylinder shell due to explosion. ACTA Armamentarii 1985, 2, 59–65. [Google Scholar]
- Charron, Y.J. Estimation of Velocity Distribution of Fragmenting Warheads Using a Modified Gurney Method; Air Force Institute of Technology Wright-Pattersonafb OH School of Engineering: Wright-Patterson AFB, OH, USA, 1979. [Google Scholar]
- Kim, H.S.; Rottenkolber, E.; Hartmann, T.; Arnold, W. Modifications of the Gurney equation to account for gas leakage. In Proceedings of the 28th International Symposium of Ballistics, Atlanta, GA, USA, 22–26 September 2014. [Google Scholar]
- Martineau, R.L.; Anderson, C.A.; Smith, F.W. Expansion of cylindrical shells subjected to internal explosive detonations. Exp. Mech. 2000, 40, 219–225. [Google Scholar] [CrossRef]
- Goto, D.M.; Becker, R.; Orzechowski, T.J.; Springer, H.K.; Sunwoo, A.J.; Syn, C.K. Investigation of the fracture and fragmentation of explosively driven rings and cylinders. Int. J. Impact Eng. 2008, 35, 1547–1556. [Google Scholar] [CrossRef]
- O’Donoghue, P.E.; Predebon, W.W.; Anderson, C.E. Dynamic launch process of performed fragments. J. Appl. Phys. 1988, 63, 337–348. [Google Scholar] [CrossRef]
- Dynamics, C. Release 14.0 Documentation for ANSYS AUTODYN; ANSYS Inc.: Canonsburg, PA, USA, 2011. [Google Scholar]
- Johnson, G.R.; Cook, W.H. A constitutive model and data for metals subjected to large strain, high strain rates and high temperatures. In Proceedings of the 7th international symposium on ballistic, The Hague, The Netherlands, 19–21 April 1983. [Google Scholar]
- Buchely, M.F.; Wang, X.; Van Aken, D.C.; O’Malleya, R.J.; Lekakha, S.; Chandrashekharab, K. The use of genetic algorithms to calibrate Johnson–Cook strength and failure parameters of AISI/SAE 1018 steel. J. Eng. Technol. 2019, 141, 021012. [Google Scholar] [CrossRef]




| Numbers | Fragment Type | The Critical Parameter Values for Calculation | ||||||
| R0 (m) | Rf (m) | β | γ | D (m/s) | Vf (m/s) 1 | Step | ||
| 1-1 | Natural fragment | 0.025 | 0.04575 | 0.4 | 3 | 6700 | 1167 | 1% R/R0 |
| 1-2 | Preformed fragment | 0.06302 | 0.0818 | 0.926 | 3 | 8200 | 1610 | 1% R/R0 |
| Numbers | Fragment Type | Final Fragment Velocity (m/s) | ||||||
| Gurney Formula [1] | Theoretical Prediction | Experimental Data [4,14] | Charron Formula [24] | Kim Formula [25] | Numerical Simulation | Error | ||
| 1-1 | Natural fragment | 1330 | 1209 | 1231 [4] | — | — | — | 1.79% |
| 1-2 | Preformed fragment | 2243 | 1915 | 1970 [14] | 2073 | 1810 | 1990 2, 2000 3 | 2.79% |
| Density (g/cm3) | Detonation Velocity (D, m/s) | C-J Pressure (GPa) | E0 (kJ/m3) | C1 (GPa) | C2 (GPa) | r1 | r2 | ω |
|---|---|---|---|---|---|---|---|---|
| 1.776 | 8210 | 31.1 | 8.9 × 106 | 700 | 12.12 | 4.5 | 1.1 | 0.3 |
| Material | Density (g/cm3) | A (MPa) | B (MPa) | n | C | m | Tmelt (K) |
|---|---|---|---|---|---|---|---|
| 1018 steel | 7.9 | 735 | 309 | 0.44 | 0.0064 | 1.05 | 1793 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, M.; Wu, C.; An, F.; Liao, S.; Yuan, X.; Xue, D.; Liu, J. Acceleration Characteristics of Discrete Fragments Generated from Explosively-Driven Cylindrical Metal Shells. Materials 2020, 13, 2066. https://doi.org/10.3390/ma13092066
Zhou M, Wu C, An F, Liao S, Yuan X, Xue D, Liu J. Acceleration Characteristics of Discrete Fragments Generated from Explosively-Driven Cylindrical Metal Shells. Materials. 2020; 13(9):2066. https://doi.org/10.3390/ma13092066
Chicago/Turabian StyleZhou, Mingxue, Cheng Wu, Fengjiang An, Shasha Liao, Xiaoxia Yuan, Dongyu Xue, and Jian Liu. 2020. "Acceleration Characteristics of Discrete Fragments Generated from Explosively-Driven Cylindrical Metal Shells" Materials 13, no. 9: 2066. https://doi.org/10.3390/ma13092066
APA StyleZhou, M., Wu, C., An, F., Liao, S., Yuan, X., Xue, D., & Liu, J. (2020). Acceleration Characteristics of Discrete Fragments Generated from Explosively-Driven Cylindrical Metal Shells. Materials, 13(9), 2066. https://doi.org/10.3390/ma13092066






