Nano-Mechanical Properties and Creep Behavior of Ti6Al4V Fabricated by Powder Bed Fusion Electron Beam Additive Manufacturing
Abstract
:1. Introduction
2. Materials and Methods
2.1. Powder Preparation
2.2. EB-PBF Processing
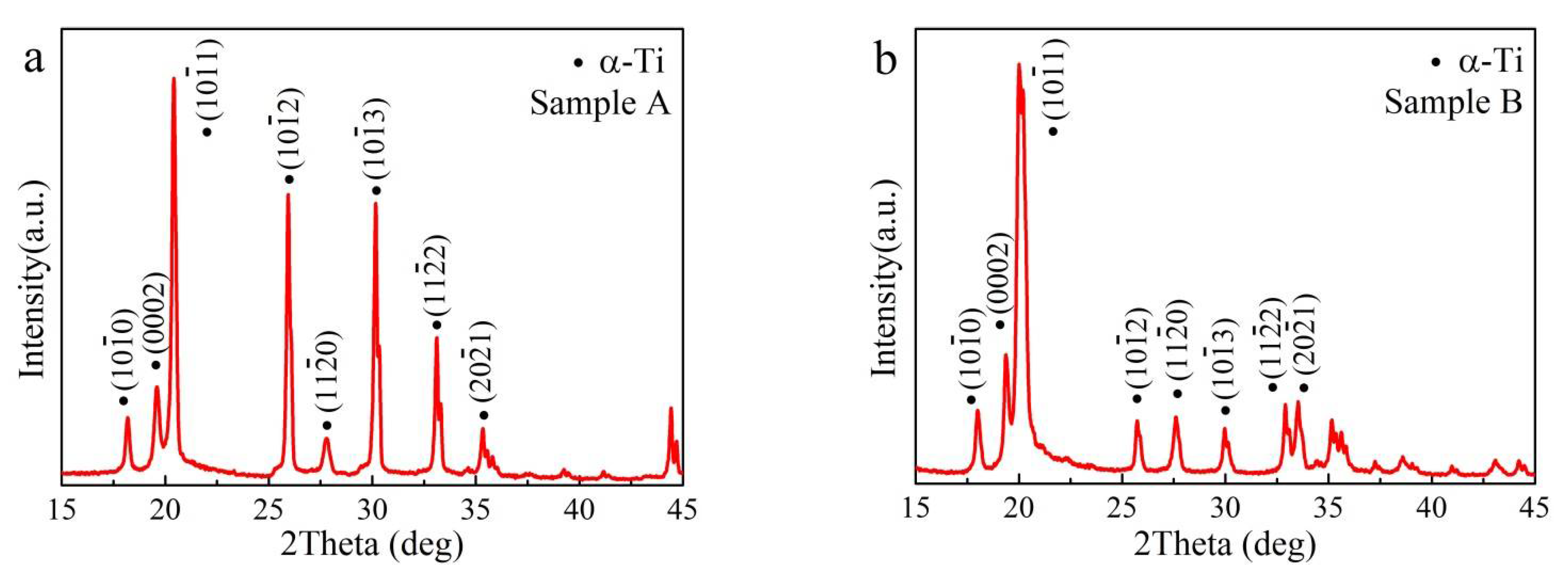
2.3. XRD Analysis
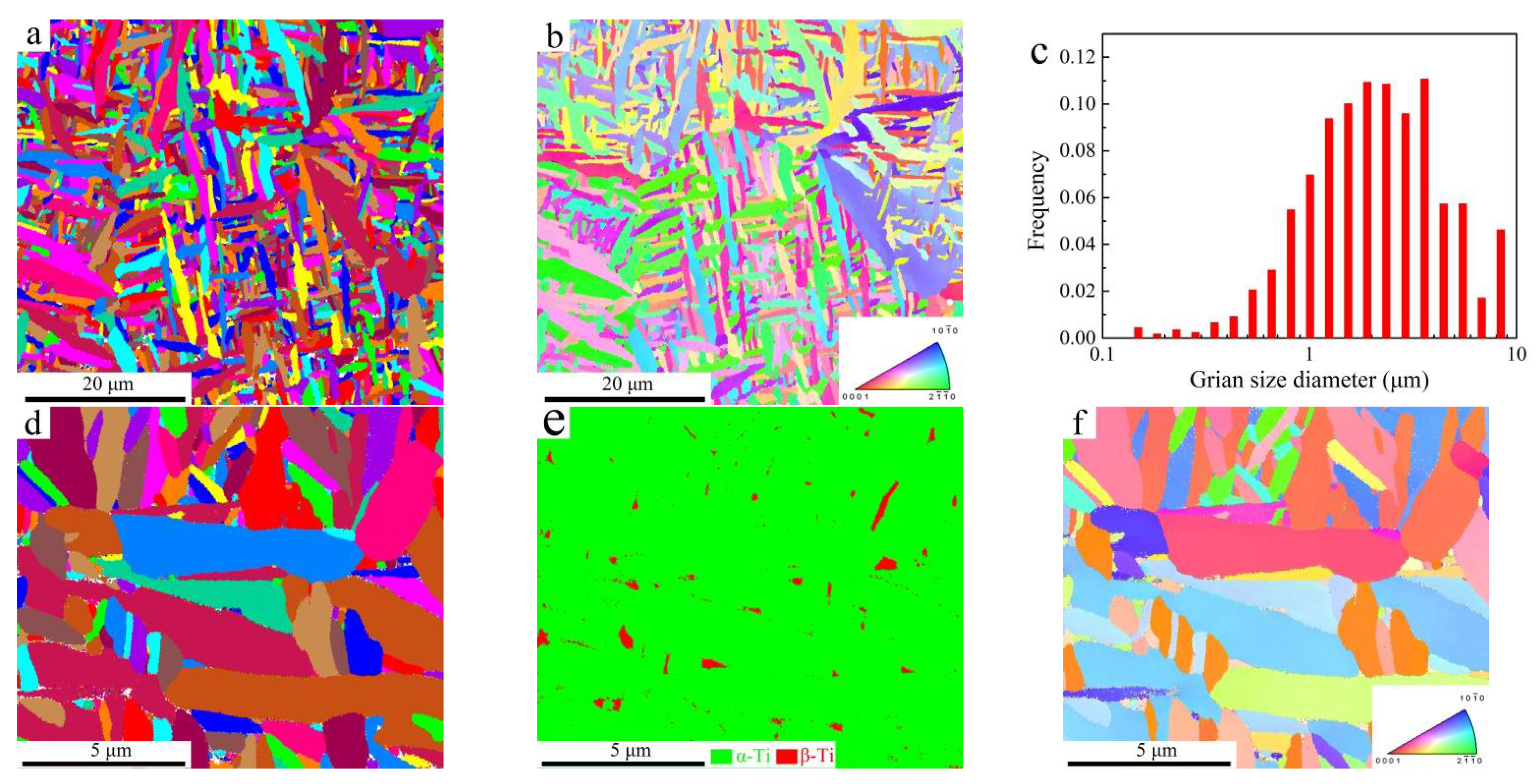
2.4. SEM Analysis
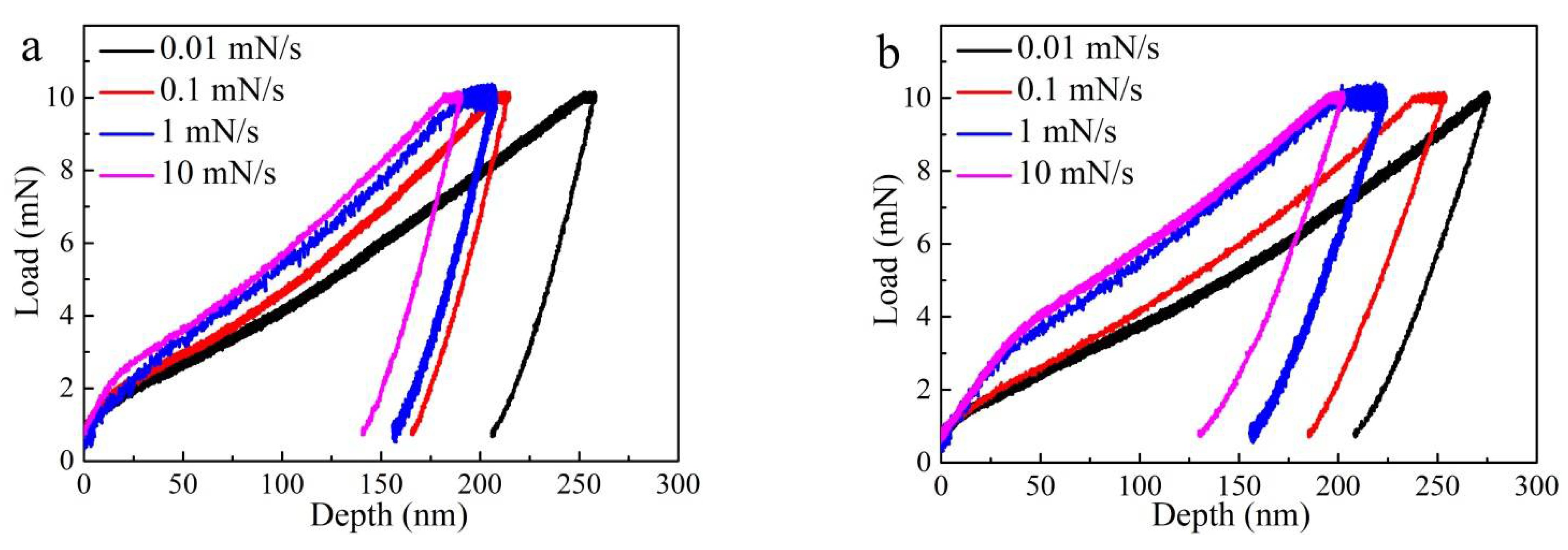
2.5. Nanoindentation Analysis
3. Results
3.1. Microstructure
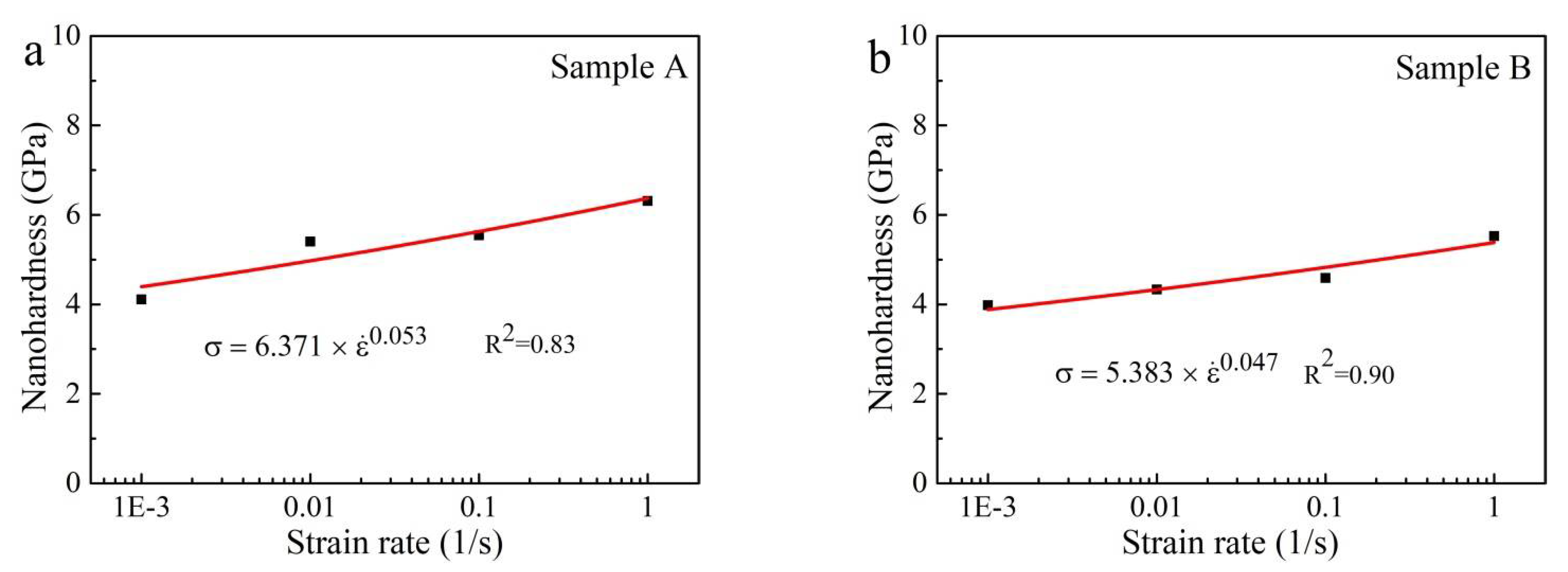
3.2. Strain-Rate Sensitivity
3.3. Creep Behavior
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, G.; Zhang, X.F.; Chen, X.L.; He, Y.H.; Cheng, L.Z.; Huo, M.K.; Yin, J.N.; Hao, F.Q.; Chen, S.Y.; Wang, P.Y.; et al. Additive manufacturing of structural materials. Mater. Sci. Eng. 2021, 9, 100596. [Google Scholar] [CrossRef]
- Cheng, B.; Price, S.; Lydon, J.; Cooper, K.; Chou, K. On Process Temperature in Powder-Bed Electron Beam Additive Manufacturing: Model Development and Validation. ASME J. Manuf. Sci. Eng. 2014, 136, 1–12. [Google Scholar] [CrossRef]
- Bourell, D.; Kruth, J.P.; Leu, M.; Levy, G.; Rosen, D.; Beese, A.M.; Clare, A. Materials for additive manufacturing. Ann. Manuf. Technol. 2017, 66, 659–681. [Google Scholar] [CrossRef]
- Zhao, Y.F.; Koizumi, Y.; Aoyagi, K.; Yamanaka, K.; Chiba, A. Isothermal γ → ε phase transformation behavior in a Co-Cr-Mo alloy depending on thermal history during electron beam powder-bed additive manufacturing. J. Mater. Sci. Technol. 2020, 50, 162–170. [Google Scholar] [CrossRef]
- Gao, B.; Peng, H.; Liang, Y.; Lin, J.; Chen, B. Electron beam melted TiC/high Nb–TiAl nanocomposite: Microstructure and mechanical property. Mater. Sci. Eng. A 2021, 811, 141059. [Google Scholar] [CrossRef]
- Kuwabara, K.; Shiratori, H.; Fujieda, T.; Yamanaka, K.; Koizumi, Y.; Chiba, A. Mechanical and corrosion properties of AlCoCrFeNi high-entropy alloy fabricated with selective electron beam melting. Addit. Manuf. 2018, 23, 264–271. [Google Scholar] [CrossRef]
- Wang, P.; Nai, M.L.S.; Sin, W.J.; Lu, S.L.; Zhang, B.C.; Bai, J.M.; Song, J.; Wei, J. Realizing a full volume component by in-situ welding during electron beam melting process. Addit. Manuf. 2018, 22, 375–380. [Google Scholar] [CrossRef]
- Klimov, A.S.; Bakeev, I.Y.; Dvilis, E.S.; Oks, E.M.; Zenin, A.A. Electron beam sintering of ceramics for additive manufacturing. Vacuum 2019, 169, 108933. [Google Scholar] [CrossRef]
- Leon, A.; Levy, G.K.; Ron, T.; Shirizly, A.; Aghion, E. The effect of hot isostatic pressure on the corrosion performance of Ti-6Al-4V produced by an electron-beam melting additive manufacturing process. Addit. Manuf. 2020, 33, 101039. [Google Scholar]
- Popov, V.V.; Muller-Kamskii, G.; Katz-Demyanetz, A.; Kovalevsky, A.; Usov, S.; Trofimcow, D.; Dzhenzhera, G.; Koptyug, A. Additive manufacturing to veterinary practice: Recovery of bony defects after the osteosarcoma resection in canines. Biomed. Eng. Lett. 2019, 9, 97–108. [Google Scholar] [CrossRef] [PubMed]
- Levy, G.K.; Kafri, A.; Ventura, Y.; Leon, A.; Vago, R.; Goldman, J.; Aghion, E. Surface stabilization treatment enhances initial cell viability and adhesion for biodegradable zinc alloys. Mater. Lett. 2019, 248, 130–133. [Google Scholar] [CrossRef]
- Popov, V.V.; Muller-Kamskii, G.; Kovalevsky, A.; Dzhenzhera, G.; Strokin, E.; Kolomiets, A.; Ramon, J. Design and 3D-printing of titanium bone implants: Brief review of approach and clinical cases. Biomed. Eng. Lett. 2018, 8, 337–344. [Google Scholar] [CrossRef]
- Rahmani, R.; Antonov, M.; Brojan, M. Lightweight 3D printed Ti6Al4V-AlSi10Mg hybrid composite for impact resistance and armor piercing shielding. J. Mater. Res. Technol. 2020, 9, 13842–13854. [Google Scholar] [CrossRef]
- Rahmani, R.; Brojan, M.; Antonov, M.; Prashanth, K.G. Perspectives of metal-diamond composites additive manufacturing using SLM-SPS and other techniques for increased wear-impact resistance. Int. J. Refract. Met. Hard Mater. 2020, 88, 105192. [Google Scholar] [CrossRef]
- Wu, Y.C.; Kuo, C.N.; Chung, Y.C.; Ng, C.H.; Huang, J.C. Effects of electropolishing on mechanical properties and bio-corrosion of Ti6Al4V fabricated by electron beam melting additive manufacturing. Materials 2019, 12, 1466. [Google Scholar] [CrossRef] [Green Version]
- Tiferet, E.; Ganor, M.; Zolotaryov, D.; Garkun, A.; Hadjadj, A.; Chonin, M.; Ganor, Y.; Noiman, D.; Halevy, I.; Tevet, O.; et al. Mapping the tray of electron beam melting of Ti-6Al-4V: Properties and microstructure. Materials 2019, 12, 1470. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Radlof, W.; Benz, C.; Heyer, H.; Sander, M. Monotonic and fatigue behavior of EBM manufactured Ti-6Al-4V solid samples: Experimental, analytical and numerical investigations. Materials 2020, 13, 4642. [Google Scholar] [CrossRef]
- Neikter, M.; Colliander, M.; Schwerz, C.d.A.; Hansson, T.; Akerfeldt, P.; Pederson, R.; Antti, M.L. Fatigue crack growth of electron beam melted Ti-6Al-4V in high-pressure hydrogen. Materials 2020, 13, 1287. [Google Scholar] [CrossRef] [Green Version]
- Tan, X.P.; Chandra, S.; Kok, Y.; Tor, S.B.; Seet, G.; Loh, N.H.; Liu, E. Revealing competitive columnar grain growth behavior and periodic microstructural banding in additively manufactured Ti-6Al-4V parts by selective electron beam melting. Materialia 2019, 7, 100365. [Google Scholar] [CrossRef]
- Wei, C.B.; Ma, X.L.; Yang, X.J.; Zhou, M.; Wang, C.M.; Zheng, Y.F.; Zhang, W.P.; Li, Z.J. Microstructural and property evolution of Ti6Al4V powders with the number of usage in additive manufacturing by electron beam melting. Mater. Lett. 2018, 221, 111–114. [Google Scholar] [CrossRef]
- Oliveira, V.M.C.A.; Silva, M.C.L.; Pinto, C.G.; Suzuki, P.A.; Machado, J.P.B.; Chad, V.M.; Barboza, M.J.R. Short-term creep properties of Ti-6Al-4V alloy subjected to surface plasma carburizing process. J. Mater. Res. Technol. 2015, 4, 359–366. [Google Scholar] [CrossRef] [Green Version]
- Kim, Y.K.; Youn, S.J.; Kim, S.W.; Hong, J.; Lee, K.A. High-temperature creep behavior of gamma Ti-48Al-2Cr-2Nb alloy additively manufactured by electron beam melting. Mater. Sci. Eng. A 2019, 763, 138138. [Google Scholar] [CrossRef]
- Yavari, P.; Langdon, T.G. An examination of the breakdown in creep by viscous glide in solid solution alloys at high stress levels. Acta Metall. 1982, 30, 2181–2196. [Google Scholar] [CrossRef]
- Gouldstone, A.; Koh, H.J.; Zeng, K.Y.; Giannakopoulos, A.E.; Suresh, S. Discrete and continuous deformation during nanoindentation of thin films. Acta Mater. 2000, 48, 2277–2295. [Google Scholar] [CrossRef]
- Xu, Z.L.; Zhang, H.; Li, W.H.; Mao, A.Q.; Wang, L.; Song, G.S.; He, Y.Z. Microstructure and nanoindentation creep behavior of CoCrFeMnNi high-entropy alloy fabricated by selective laser melting. Addit. Manuf. 2019, 28, 766–771. [Google Scholar] [CrossRef]
- Sun, S.; Gao, P.; Sun, G.X.; Cai, Z.; Hu, J.J.; Han, S.; Lian, J.S.; Liao, X.Z. Nanostructuring as a route to achieve ultra-strong high- and medium-entropy alloys with high creep resistance. J. Alloys Compd. 2020, 830, 154656. [Google Scholar] [CrossRef]
- Jun, T.S.; Armstrong, D.E.J.; Britton, T.B. A nanoindentation investigation of local strain rate sensitivity in dual-phase Ti alloys. J. Alloys Compd. 2016, 672, 282–291. [Google Scholar] [CrossRef] [Green Version]
- Li, H.; Ngan, A.H.W. Size effects of nanoindentation creep. J. Mater. Res. 2004, 19, 513–522. [Google Scholar] [CrossRef]
- Wang, C.L.; Lai, Y.H.; Huang, J.C.; Nieh, T.G. Creep of nanocrystalline nickel: A direct comparison between uniaxial and nanoindentation creep. Scr. Mater. 2010, 62, 175–178. [Google Scholar] [CrossRef]
- Goodall, R.; Clyne, T.W. A critical appraisal of the extraction of creep parameters from nanoindentation data obtained at room temperature. Acta Mater. 2006, 54, 5489–5499. [Google Scholar] [CrossRef]
- Poisl, W.H.; Oliver, W.C.; Fabes, B.D. The relationship between indentation and uniaxial creep in amorphous selenium. J. Mater. Res. 1995, 10, 2024–2032. [Google Scholar] [CrossRef]
- Choi, I.C.; Yoo, B.G.; Kim, Y.J.; Jang, J.I. Indentation creep revisited. J. Mater. Res. 2011, 27, 3–11. [Google Scholar] [CrossRef]
- Shen, L.; Cheong, W.C.D.; Foo, Y.L.; Chen, Z. Nanoindentation creep of tin and aluminium: A comparative study between constant load and constant strain rate methods. Mater. Sci. Eng. A 2012, 532, 505–510. [Google Scholar] [CrossRef]
- Song, J.; Wu, W.H.; Zhang, L.; He, B.B.; Lu, L.; Ni, X.Q.; Long, Q.L.; Zhu, G.L. Role of scanning strategy on residual stress distribution in Ti-6Al-4V alloy prepared by selective laser melting. Optik 2018, 170, 342–352. [Google Scholar] [CrossRef]
- Wang, X.; Lv, F.; Shen, L.D.; Liang, H.X.; Xie, D.Q.; Tian, Z.J. Influence of island scanning strategy on microstructures and mechanical properties of direct laser-deposited Ti–6Al–4V structures. Acta Metall. Sin. 2018, 32, 1173–1180. [Google Scholar] [CrossRef] [Green Version]
- Peng, H.L.; Hu, L.; Li, L.J.; Zhang, L.Y.; Zhang, X.L. Evolution of the microstructure and mechanical properties of powder metallurgical high-speed steel S390 after heat treatment. J. Alloys Compd. 2018, 740, 766–773. [Google Scholar] [CrossRef]
- Kenel, C.; Grolimund, D.; Li, X.; Panepucci, E.; Samson, V.A.; Sanchez, D.F.; Marone, F.; Leinenbach, C. In situ investigation of phase transformations in Ti-6Al-4V under additive manufacturing conditions combining laser melting and high-speed micro-X-ray diffraction. Sci. Rep. 2017, 7, 16358. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhao, X.L.; Li, S.J.; Zhang, M.; Liu, Y.D.; Sercombe, T.B.; Wang, S.G.; Hao, Y.L.; Yang, R.; Murr, L.E. Comparison of the microstructures and mechanical properties of Ti–6Al–4V fabricated by selective laser melting and electron beam melting. Mater. Des. 2016, 95, 21–31. [Google Scholar] [CrossRef]
- Ma, R.X.; Liu, Z.Q.; Wang, W.B.; Xu, G.J.; Wang, W. Microstructures and mechanical properties of Ti6Al4V-Ti48Al2Cr2Nb alloys fabricated by laser melting deposition of powder mixtures. Mater. Charact. 2020, 164, 110321. [Google Scholar] [CrossRef]
- Chen, G.L.; Xu, X.J.; Teng, Z.K.; Wang, Y.L.; Lin, J.P. Microsegregation in high Nb containing TiAl alloy ingots beyond laboratory scale. Intermetallics 2007, 15, 625–631. [Google Scholar] [CrossRef]
- Takashima, T.; Koizumi, Y.; Li, Y.P.; Yamanaka, K.; Saito, T.; Chiba, A. Effect of Building Position on Phase Distribution in Co-Cr-Mo Alloy Additive Manufactured by Electron-Beam Melting. Mater. Trans. 2016, 57, 2041–2047. [Google Scholar] [CrossRef] [Green Version]
- Peng, H.L.; Hu, L.; Li, L.J.; Gao, J.X.; Zhang, Q. On the correlation between L12 nanoparticles and mechanical properties of (NiCo)52+2x(AlTi)4+2xFe29-4xCr15 (x=0-4) high-entropy alloys. J. Alloys Compd. 2020, 817, 152750. [Google Scholar] [CrossRef]
- VNguyen, L.; Kim, E.A.; Yun, J.; Choe, J.; Yang, D.Y.; Lee, H.S.; Lee, C.W.; Yu, J.H. Nano-mechanical Behavior of H13 Tool Steel Fabricated by a Selective Laser Melting Method. Metall. Mater. Trans. A 2019, 50, 523–528. [Google Scholar]
- Duan, Z.; Pei, W.; Gong, X.; Chen, H. Superplasticity of Annealed H13 Steel. Materials 2017, 10, 870. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Surand, L.B.; Ruau, J.; Viguier, B. Creep behavior of Ti-6Al-4V from 450 °C to 600 °C, U.P.B. Sci. Bull. 2014, 76, 185–196. [Google Scholar]
- Barboza, M.J.R.; Perez, E.A.C.; Medeiros, M.M.; Reis, D.A.P.; Nono, M.C.A.; Neto, F.P.; Silva, C.R.M. Creep behavior of Ti–6Al–4V and a comparison with titanium matrix composites. Mater. Sci. Eng. A 2006, 428, 319–326. [Google Scholar] [CrossRef]






| Chemical Composition | Sample A | Sample B | ||
|---|---|---|---|---|
| α-Ti (wt%) | β-Ti ribs (wt %) | α-Ti (wt %) | β-Ti ribs (wt %) | |
| Al | 5.48 ± 0.64 | 5.86 ± 0.50 | 5.48 ± 0.07 | 5.32 ± 0.65 |
| V | 4.05 ± 2.55 | 2.83 ± 0.54 | 3.22 ± 0.66 | 2.93 ± 0.66 |
| Ti | 90.48 ± 1.92 | 91.32 ± 0.04 | 91.2 ± 0.59 | 90.25 ± 2.14 |
| Loading Rate (mN/s) | Strain Rate (s−1) | Hardness (GPa) | |
|---|---|---|---|
| Sample A | Sample B | ||
| 0.01 | 0.001 | 4.11 | 3.98 |
| 0.1 | 0.01 | 5.4 | 4.33 |
| 1 | 0.1 | 5.54 | 4.59 |
| 10 | 1 | 6.31 | 5.52 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peng, H.; Fang, W.; Dong, C.; Yi, Y.; Wei, X.; Luo, B.; Huang, S. Nano-Mechanical Properties and Creep Behavior of Ti6Al4V Fabricated by Powder Bed Fusion Electron Beam Additive Manufacturing. Materials 2021, 14, 3004. https://doi.org/10.3390/ma14113004
Peng H, Fang W, Dong C, Yi Y, Wei X, Luo B, Huang S. Nano-Mechanical Properties and Creep Behavior of Ti6Al4V Fabricated by Powder Bed Fusion Electron Beam Additive Manufacturing. Materials. 2021; 14(11):3004. https://doi.org/10.3390/ma14113004
Chicago/Turabian StylePeng, Hanlin, Weiping Fang, Chunlin Dong, Yaoyong Yi, Xing Wei, Bingbing Luo, and Siming Huang. 2021. "Nano-Mechanical Properties and Creep Behavior of Ti6Al4V Fabricated by Powder Bed Fusion Electron Beam Additive Manufacturing" Materials 14, no. 11: 3004. https://doi.org/10.3390/ma14113004
APA StylePeng, H., Fang, W., Dong, C., Yi, Y., Wei, X., Luo, B., & Huang, S. (2021). Nano-Mechanical Properties and Creep Behavior of Ti6Al4V Fabricated by Powder Bed Fusion Electron Beam Additive Manufacturing. Materials, 14(11), 3004. https://doi.org/10.3390/ma14113004






