Next Article in Journal
Optimized Zirconia 3D Printing Using Digital Light Processing with Continuous Film Supply and Recyclable Slurry System
Previous Article in Journal
Mechanosynthesis and Thermoelectric Properties of Fe, Zn, and Cd-Doped P-Type Tetrahedrite: Cu12-xMxSb4S13
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Materials
Volume 14
Issue 13
10.3390/ma14133449
materials-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

EPR Spectra of Sintered Cd1−xCrxTe Powdered Crystals with Various Cr Content

by
Ireneusz Stefaniuk
1,*,
Werner Obermayr
2,
Volodymyr D. Popovych
3,
Bogumił Cieniek
1 and
Iwona Rogalska
1
1
College of Natural Sciences, University of Rzeszow, Rejtana 16a, 35-310 Rzeszow, Poland
2
Institute for Electronic Engineering, FH Joanneum, Alte Poststraße 147, 8020 Graz, Austria
3
Department of Machine Science and Fundamental Technologies, Ivan Franko Drogobych State Pedagogical University, Ivan Franko Str. 24, 82100 Drogobych, Ukraine
*
Author to whom correspondence should be addressed.
Materials 2021, 14(13), 3449; https://doi.org/10.3390/ma14133449
Submission received: 23 April 2021 / Revised: 15 June 2021 / Accepted: 18 June 2021 / Published: 22 June 2021
Browse Figures
Versions Notes

Abstract

:
In this paper, we show a simple method of producing ferromagnetic materials with a Curie temperature above room temperature. The electron paramagnetic resonance (EPR) spectra of Cd1−xCrxTe (0.002 < x < 0.08) were measured with a dependence on temperature (82 K < T < 381 K). Obtained EPR lines were fitted to a Lorentz-shaped curve. The temperature dependencies of the parameters of the EPR lines, such as the peak-to-peak linewidth (Hpp), the intensity (A), as well as the resonance field (Hr), were studied. Ferromagnetism was noticed in samples at high temperatures (near room temperature). For a sample with a nominal concentration of chrome of x = 0.05, a very strong intrinsic magnetic field is observed. The value of the effective gyromagnetic factor for this sample is ge = 30 at T = 240 K. An increase of chrome concentration above x = 0.05 reduces the ferromagnetic properties considerably. Analysis of the temperature dependencies of the integral intensity of EPR spectra was carried out using the Curie–Weiss law and the paramagnetic Curie temperature was obtained.

1. Introduction

Diluted magnetic semiconductors (DMSs) are of interest, mainly due to the spin–spin exchange interaction between the localized magnetic moments and the band electrons [1,2]. This property of DMS material has potential applications in spin-dependent semiconductor electronics for information processing (spintronics) [3,4,5,6].
A substantial amount of work has been done on Mn-based DMSs. Ferromagnetism has been discovered in materials comparable with the semiconductors used in present electronics, like as (In, Mn)As [7], (Ga, Mn)As [8], Mg [9,10], and Cd1−xMnxTe [11,12,13]. Ferromagnetic properties in DMS make them basic component for spintronics. However, their Curie temperatures (TC) (e.g., 110 K for (Ga, Mn)As [14]) are not high enough for real applications.
The ferromagnetism in DMS has also been investigated theoretically using a model Hamiltonian [15,16,17]. Dietl et al. proposed Zener’s p–d exchange interaction to describe magnetism [15,16]. This model predicts room-temperature ferromagnetism (RTFM) in (Ga, Mn)N [15,16]. Liu et al. confirmed the ferromagnetic character of the p–d exchange interaction in Cr-based II-VI DMSs [18,19]. Blinowski et al. [20] pointed out very interesting properties of Cr-based DMS and predicted ferromagnetism at room temperature in CdTe:Cr. In the last few years, Cr-doped II-VI DMS compounds have been studied extensively to determine improved ferromagnetic properties and to explore the mechanism behind the half-metallic ferromagnetism (HMF) [21,22,23,24,25,26,27,28].
Some earlier experiments for semiconductor CdTe with Cr using the EPR method were performed. Ludwig and Lorentz [29] studied Cr1+ (3d5) ions substituted into the cadmium sites in CdTe using electron nuclear double resonance (ENDOR) and EPR. Chromium ions substituting for cadmium ions are expected to be in the Cr2+ valence state, i.e., in the 3d4 configuration. However, the authors of [29] determined the total spin S = 5/2, g = 1.9997 ± 0.0003, as well the parameters of the cubic zero-field splitting and the hyperfine interaction with second neighbors Cd111 and Cd113. Vallin and Watkins [30] investigated the EPR spectra of Cr2+ in CdTe. The Jahn–Teller coupling coefficients from the changes of the fine structure of the EPR spectra under uniaxial stress were determined. Stefaniuk et al. [31] studied the fine structure of Cr3+ ions in CdTe:Cr. The EPR spectra also contained a very broad line assigned to Cr2+ ions in CdTe, which are related to magnetic interactions [31,32]. Magnetic properties of Cr-based DMS were studied by Mac et al. [33] and Ko and Blamire [34,35]. Many experiments have been performed to search for DMSs with RTFM and, recently, high TC values have been reported for several systems, e.g., (Ga, Mn)N [36,37,38] (Ga, Cr)N [39], and (ZnCr)Te [40,41,42].
There is a large group of materials with ferromagnetic properties, with a Curie temperature above 500 K (see [43]). However, the goal of this work is to obtain a ferromagnetic semiconductor (DMS) meeting the needs of spintronics.
In this paper, we investigate Cd1−xCrxTe powdered bulk crystals that were doped with different concentrations of chrome (up to x = 0.08) during the synthesis process. A wide temperature range of crystals was studied using the EPR method. This study aims to determine the optimal content of Cr ions at which ferromagnetic properties are observed at room temperature.

2. Materials and Methods

The crystal preparation procedure consisted of two subsequent stages: the synthesis of polycrystalline source and a crystal growth process, described in more detail in other work [44]. High purity elemental CdTe (99.9999%) supplied by Alfa Aesar and also Cr2Te3 powder with particle sizes under 325 mesh (99.96%) from Sigma-Aldrich (Saint Louis, MO, USA) were used for (Cd,Cr)Te synthesis. The calculation of the appropriate constituent weights was done considering that chromium atoms substitute cadmium ones and a Cd1−xCrxTe solid solution was formed. We studied the pulverized Cd1−xCrxTe crystals doped with different nominal concentrations of chrome (up to x = 0.08) during the synthesis process. Growth temperatures were in the range of 1280–1320 °C. The final materials were solid solutions of the source components. The chromium concentrations in the samples were measured by X-ray energy dispersive fluorescent analysis (XFA) (Table 1). All samples for EPR studies were pulverized in an agate mortar. To investigate the crystal structure of samples X-ray diffraction (XRD) was used.
The EPR measurements were performed on an X-band (~9 GHz) spectrometer with a digital registration of the spectra. Part of the EPR measurements was performed on an EPR spectrometer (Bruker multifrequency and multiresonance FT-EPR ELEXSYS E580, Bruker Analytische Messtechnik, Rheinstetten, Germany). The temperature measurements using the digital temperature control system (Bruker ER 4131VT) in the temperature range from 82–381 K were done.

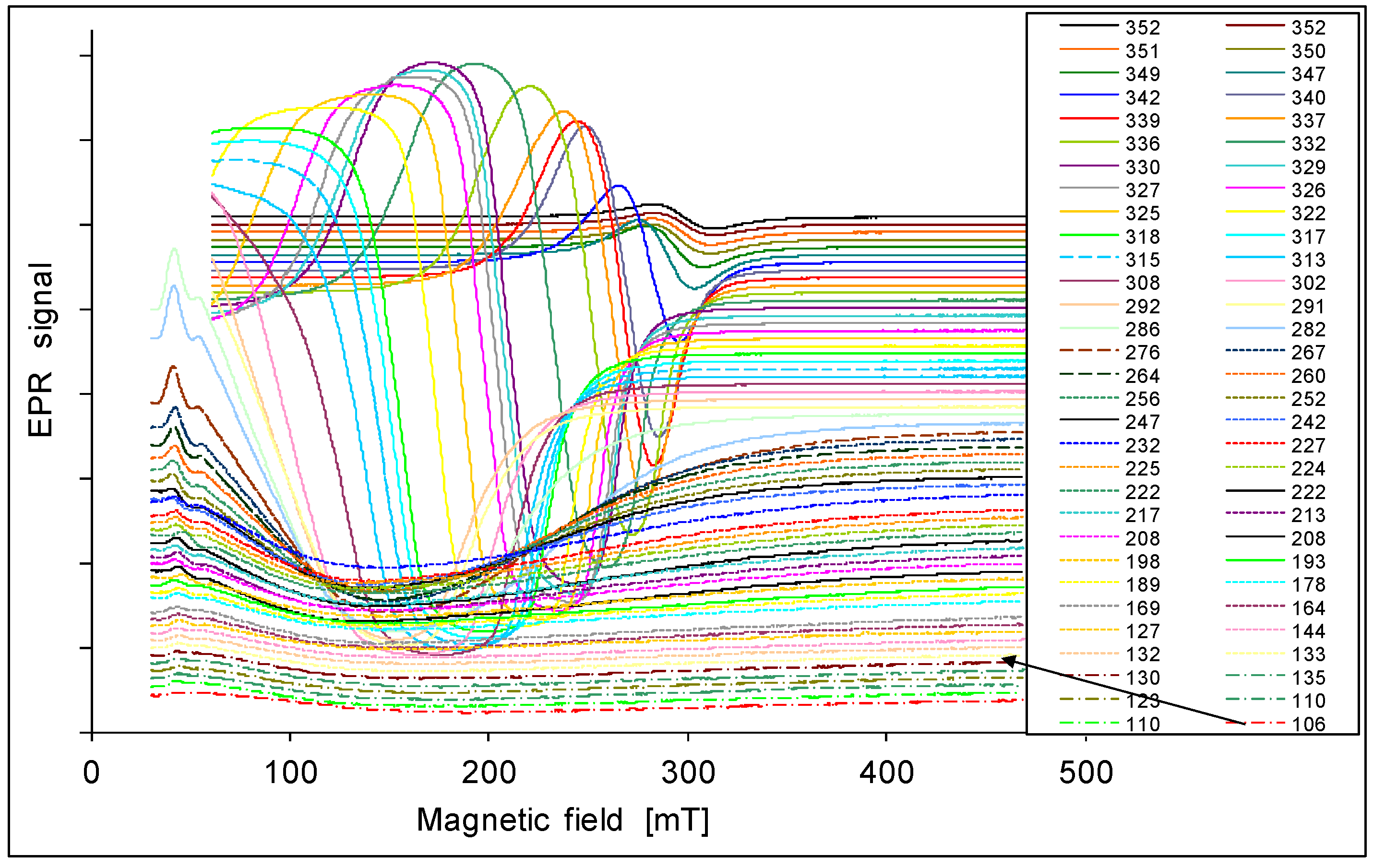
3. Results

The EPR spectra are presented in Figure 1, Figure 2, Figure 3, Figure 4 and Figure 5. In every sample, we observed a wide EPR signal which, however, is irregular for low concentrations of chrome (sample 1p and 2p). In samples 1p and 2p, as well as in sample 5p, we observed a small shift in the EPR line towards a higher magnetic field with increasing temperature. For samples 3p and 4p, very regular shapes of the EPR spectra above room temperature (Figure 3 and Figure 4) were obtained. At lower temperatures, the resonance field shifted towards lower magnetic fields, and then only a part of the EPR line was observed. The EPR lines presented in Figure 1, Figure 2, Figure 3, Figure 4 and Figure 5 are arranged such that they are shifted upwards with increasing temperature, as indicated by the legend. The amplitudes of the EPR signals for samples 1p, 2p, and 3p became smaller when the temperature was lowered from room temperature. However, for samples 4p and 5p, we first observed an increase in the amplitude of the signal with reduced temperature, but below a certain temperature a decrease was noted. Furthermore, the linewidth strongly depended on both, the temperature and the sample, i.e., the concentration of chromium.

4. Discussion

The ferromagnetic properties of semiconductors are the subject of wide investigations at present. It is known that for similar materials, the shape of an EPR line is closely related to the kind of magnetic interaction [45,46,47,48,49]. Since the exact mechanisms of ferromagnetism in the present material are still not known, the choice of the theoretical curve for the fitting of the experimental data is not straightforward. Therefore, in the initial stage of the research, we focused on the determination of the appropriate concentration of chromium, depending on strong ferromagnetic properties at high temperatures. This was realized by measurements of the temperature dependences of the peak-to-peak linewidth Hpp, the peak amplitude A, and the resonance field Hr. The latter is in simple relation to the effective gyromagnetic factor ge. Since the majority of the measured EPR spectra are not complete due to the large linewidth and their shifts towards smaller magnetic fields for lower temperatures, it is impossible to get the line parameters directly from the spectra. Thus, these parameters were determined by fitting the resonance line to the theoretical curve by using the standard computer program OriginPro 7.5, OriginLab, Northampton, MA, USA. For numerical analysis, a Lorentzian function was applied [50],
y = P − 3 1 2 × H pp × ( χ − H r ) π × ( 0.75 × H pp 2 + ( χ − H r ) 2 ) 2 ×   A
where P is a constant, Hpp is the peak-to-peak-width, Hr is the resonance field, and A is the peak amplitude. The analysis of the shape of the experimental line shows its complex structure. In this work, we used fitting with a single Lorentz shape line as the closest match to the real one. We are aware that some parameters are different (e.g., intensity of line A), but, at the same time, other parameters are obtained with good accuracy (e.g., resonance field Hr).
As an example, in Figure 6, we show the result of the fitting of an experimental spectrum to a Lorentzian curve. As it is shown in Figure 6b for the incomplete EPR lines very well-fitting results were received. However, the fitting to the whole or considerable parts of the visible lines as one can see in Figure 6a was worse. For example, the accuracy of the fitting for a small number of some very irregular lines, the EPR spectrum of the sample 5p at the temperature around 240 K, was poor. The value of the resonance field Hr is very well reproduced, whereas the linewidth Hpp shows deviations up to maximal errors of several percent. However, we are interested in the temperature dependence of Hpp. From such diagrams, we are able with good accuracy to find the temperature in which the large changes of Hpp occur.
Determining parameters of the EPR spectra such as Hr (or ge), Hpp, and A, are presented in Figure 7, Figure 8 and Figure 9. In Figure 7 the temperature dependence of the effective gyromagnetic factor ge is shown for all samples. In a paramagnetic material, the applied external Zeeman field is equal to the local field and the g-factor is obtained from the Zeeman formula. The effect is more complicated in ferromagnets. The local resonance field is a superposition of the external field H0, the demagnetizing field, and other contributions to the magnetic anisotropy energy [49,51]. We observe a paramagnetic phase at higher temperatures with ge close to 2. However, at lower temperatures, the value of ge increases due to intrinsic magnetic fields responsible for ferromagnetism. A larger value of ge is related to a larger intrinsic magnetic field. The samples 1p and 2p with small concentrations of chrome show ferromagnetic properties with ge about 8. With the increase of the concentration of chrome as in sample 3p, an increase of ge (ge = 10) was observed. In sample 4p it accepts a very large value close to ge = 30. This is a very high value in comparison with other materials. Further increase of concentration like in the sample 5p, an interesting effect is seen, that ge again accepts the value of about 2. A possible explanation may be the appearance of antiferromagnetic interactions. Similar results have been found for Mn in CaMn1−xRuxO3 [47].
In Figure 8, we show the temperature dependence of linewidth. For the samples 1p, 2p, and 3p, we find a maximum for Hpp approximately at room temperature. For sample 4p the maximum is found close to 170 K. However, for sample 5p the linewidth increases with lower temperatures. In the samples 2p and 3p, the linewidth remains almost constant below 160 K. For the samples 3p and 4p we observe a decrease of the linewidth when the temperature is raised above room temperature, and we get a minimum for Hpp in the range 340–350 K. For higher temperatures we find an increase of the linewidth again and simultaneously a reduction of the intensity; this is the typical behavior of a paramagnetic sample [49]. The largest change of the linewidth Hpp in dependence of the temperature is in the sample 4p: it changes from 25 mT at 346 K to 250 mT at 180 K.
The temperature dependence of the integral intensity is shown in Figure 9. We see that for the samples with higher concentrations of chrome the maxima of the intensities are shifted to lower temperatures. Furthermore, we observe that the intensities rise with increasing concentrations of chrome (samples 1p, 2p 3p); the maximum is obtained for the sample 4p, and then it decreases again like in sample 5p.
The presented results were completed by studies of the chemical and crystallographic homogeneity of the samples. For this aim, we performed XRD measurements. The spectra of our samples were compared with those of pure CdTe as well as with CrTe phases such as Cr7Te8, Cr3Te4, Cr5Te6, etc. which are the most likely inclusions of the second phase (Cr2Te3 was an initial component in the process of the CdCrTe synthesis). In Figure 10 spectra with those given by standard ASTM X-ray powder data were compared. The main diffraction peaks of our sample no. 4p (Figure 10f) corresponds very well with the zinc-blende structure of the CdTe crystal (Figure 10d,e). We have not detected diffraction peaks from CrTe phases which crystallize in a different structure—in the structure of NiAs [52] (see Figure 10a–c).
The lack of the second phase in our samples is also demonstrated by the EPR measurements of the Cr2Te3 powder (the initial material for CdCrTe synthesis), as well as of the Cr2Te3 solid (premelted in the conditions of CdCrTe synthesis). These spectra for the temperature of 260 K are shown in Figure 11. They also differ considerably from those obtained for CdCrTe. The resonance fields are 341 mT and 347 mT for powdered and solid Cr2Te3, respectively, whereas for CdCrTe it has a value of 95 mT. The EPR line of Cr2Te3 at room temperature (paramagnetic phase) is also of Lorentzian shape, however, with decreasing temperature the shape changes towards a Dysonian function, particularly at low temperatures. The positions of the lines (of Cr2Te3 powdered and solid) do not change with temperature contrary to the EPR line of CdCrTe.
We used the Curie–Weiss law to analyze the temperature dependences of the integral intensity, which is directly proportional to the magnetic susceptibility χ. A linear increase of χ−1 (T) at higher temperatures can be fitted to the Curie-Weiss law [53,54],
(χ − χ0)−1(T) = (T − θcw)/C,
where C is the Curie constant, θcw is the paramagnetic Curie temperature, and χ0 is a temperature-independent term to account for the diamagnetic host and any Pauli paramagnetism contribution. Figure 12 displays the temperature dependence of the quantity (χ − χ0)−1 for sample 4p. The lines are linear extrapolations illustrating the ferromagnetic (positive) Curie–Weiss temperatures. Fitting yields the following values: for sample 4p-θ(x) = 338.3 K and C(x) = 5.91 × 10−7; for sample 3p-θ(x) = 333 K and C(x) = 7.51 × 10−6; for sample 2p-θ(x) = 250 K and C(x) = 6.25 × 10−9. The Curie constant has a dimension of Km 3 kg .
Ko et al. [34,35] reported a Curie temperature for a bulk Cr-doped CdTe crystal of TC = 354 K for x = 0.06, which agrees well with our observations (Figure 7, Figure 8 and Figure 9) and Curie temperature TC = 338.3 K for sample 4pge values for the samples 3p, 4p, and 5p show small differences in the region of higher temperatures (paramagnetic region). A similar effect was observed by Son et al. [46] for Mn in Cd1−xMnxTe.
A very large ge factor (up to 30) observed for the 4p sample, shows a very strong intrinsic magnetic field, which, together with a high Curie temperature may have its origin in a superexchange interaction of Cr ions in the Cr-dissolved CdTe phase. The observed effects may indicate superparamagnetism [55,56,57].

5. Conclusions

The fine structure of the spectra only in the sample with a low concentration of Cr atoms (sample 1p, Figure 1) is visible. It is superimposed on the one intensive line positioned at a lower magnetic field.
In the samples with a concentration of Cr from x = 0.002 to 0.08, a broad line in the EPR spectrum is observed. The shape of this line is symmetric and regular for samples 3p and 4p. The position of the line strongly depends on the temperature.
At several characteristic temperatures, a change of the slope of the temperature depends on the resonance field (Hr), the linewidth (Hpp), and the total intensity (It) was observed. At about 160 K this is seen for Hpp, It, and especially for the ge factor to the different range and depending on the Cr concentration; at 240 K for the ge(T) dependence especially in sample 4p; at 280 K (room temperature) for Hpp(T) in all samples, and to a smaller extent for ge(T) and It(T); in the temperature range 340–350 K for Hpp, ge, and It in the samples 3p and 4p.
ge values for the samples 3p, 4p, and 5p show small differences in the region of higher temperatures (paramagnetic region). The value of ge is higher for larger concentrations of Mn. However, we obtained the values 2.18, 2.28, and 1.93 for the samples 3p, 4p, and 5p, respectively. Thus, ge does not shift to higher values with increasing concentration of Cr but achieves a maximum for x = 0.05 (sample 4p).
The most interesting magnetic properties for the sample 4p in the vicinity of room temperature, for all EPR line parameters, were observed. A very large ge factor (up to 30) shows a very strong intrinsic magnetic field. This intrinsic ferromagnetism together with a high Curie temperature may have its origin in a superexchange interaction of Cr ions in the Cr-dissolved CdTe phase. The observed effects indicate possible superparamagnetism. Detailed research for this sample will be conducted further, especially taking into account the precise analysis of the line shape, as well as the registration of the spectrum in the negative field. The use of a simple method of sintered powdered materials: Cd, Te, and Cr2Te3 allows for easy control of the content of chromium ions in Cd1−xCrxTe. The DMS obtained in this way shows ferromagnetic properties at room temperature, especially in the range x = 0.05–0.1.
It is found that an increase of the concentration of Cr up to x = 0.05 enlarges the ferromagnetic properties, but a further augmentation of the Cr concentration reduces ferromagnetism and may lead to the presence of antiferromagnetism.

Author Contributions

For research articles with several authors, the following statements should be used “Conceptualization, I.S.; methodology, I.S.; software, B.C.; validation, I.S., W.O. and B.C.; formal analysis, I.S.; W.O.; investigation, I.S.; I.R.; V.D.P.; resources, I.S.; data curation, I.S.; I.R.; writing—original draft preparation, I.S.; W.O.; writing—review and editing, I.S.; B.C.; visualization, I.S.; B.C.; funding acquisition, by the Center of Teaching Technical and Natural Sciences within the statutory work. All authors have read and agreed to the published version of the manuscript.

Funding

This research received no external funding.

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Data Availability Statement

Data sharing is not applicable to this article.

Conflicts of Interest

The authors declare no conflict of interest.

References

  1. Dobrowolski, W.; Kossut, J.; Story, T. II-VI and IV-VI Diluted Magnetic Semiconductors—New Bulk Materials and Low-Dimensional Quantum Structures. Handb. Magn. Mater. 2003, 15, 289–377. [Google Scholar]
  2. Kacman, P. Spin interactions in diluted magnetic semiconductors and magnetic semiconductor structures. Semicond. Sci. Technol. 2001, 16, R25–R39. [Google Scholar] [CrossRef]
  3. Awschalon, D.D.; Loss, D. Semiconductors Spintronics and Quantum Computation; Springer: Berlin/Heidelberg, Germany, 2002; ISBN 9783642075773. [Google Scholar]
  4. Ohno, H. Making nonmagnetic semiconductors ferromagnetic. Science 1998, 281, 951–956. [Google Scholar] [CrossRef] [PubMed]
  5. Wolf, S.A.; Awschalom, D.D.; Buhrman, R.A.; Daughton, J.M.; Von Molnár, S.; Roukes, M.L.; Chtchelkanova, A.Y.; Treger, D.M. Spintronics: A spin-based electronics vision for the future. Science 2001, 294, 1488–1495. [Google Scholar] [CrossRef] [Green Version]
  6. Laref, A.; AlMudlej, A.; Laref, S.; Yang, J.T.; Xiong, Y.C.; Luo, S.J. Ab-initio investigations of magnetic properties and induced half-metallicity in Ga1−xMnxP (x = 0.03, 0.25, 0.5, and 0.75) Alloys. Materials 2017, 10, 766. [Google Scholar] [CrossRef] [Green Version]
  7. Ohno, H.; Munekata, H.; Penney, T.; Von Molnar, S.; Chang, L.L. Magnetotransport properties of p-type (In,Mn)As diluted magnetic III–V semiconductors. Phys. Rev. Lett. 1992, 68, 2664–2667. [Google Scholar] [CrossRef] [PubMed]
  8. Ohno, H.; Shen, A.; Matsukura, F.; Oiwa, A.; Endo, A.; Katsumoto, S.; Iye, Y. (Ga,Mn)As: A new diluted magnetic semiconductor based on GaAs. Appl. Phys. Lett. 1996, 69, 363–365. [Google Scholar] [CrossRef]
  9. Yu, P.; Jiang, B.; Chen, Y.; Zheng, J.; Luan, L. Study on In-Doped CdMgTe Crystals Grown by a Modified Vertical Bridgman Method Using the ACRT Technique. Materials 2019, 12, 4236. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  10. Yavorskiy, D.; Szoła, M.; Karpierz, K.; Rudniewski, R.; Bozek, R.; Karczewski, G.; Wojtowicz, T.; Wróbel, J.; Łusakowski, J. Polarization of magnetoplasmons in grating metamaterials based on CdTe/CdMgTe quantum wells. Materials 2020, 13, 1811. [Google Scholar] [CrossRef] [Green Version]
  11. Furdyna, J.K.; Kossut, J. Semiconductors and Semimetals; Willardson, R.K., Beer, A.C., Eds.; Academic Press, Inc.: Cambridge, MA, USA, 1988; Volume 25, ISBN 9780080864228. [Google Scholar]
  12. Sayad, H.A.; Bhagat, S.M. Dynamic random fields in diluted magnetic semiconductors: Cd1−xMnxTe. Phys. Rev. B 1985, 31, 591–593. [Google Scholar] [CrossRef]
  13. Oseroff, S.B. Magnetic susceptibility and EPR measurements in concentrated spin-glasses: Cd1−xMnxTe and Cd1−xMnxSe. Phys. Rev. B 1982, 25, 6584–6594. [Google Scholar] [CrossRef]
  14. Matsukura, F.; Ohno, H.; Shen, A.; Sugawara, Y. Transport properties and origin of ferromagnetism in (Ga, Mn)As. Phys. Rev. B Condens. Matter Mater. Phys. 1998, 57, R2037–R2040. [Google Scholar] [CrossRef]
  15. Dietl, T.; Ohno, H.; Matsukura, F.; Cibert, J.; Ferrand, D. Zener Model Description of Ferromagnetism in Zinc-Blende Magnetic Semiconductors. Science 2000, 287, 1019–1022. [Google Scholar] [CrossRef] [Green Version]
  16. Dietl, T. Ferromagnetic semiconductors. Semicond. Sci. Technol. 2002, 17, 377–392. [Google Scholar] [CrossRef]
  17. Jungwirth, T.; König, J.; Sinova, J.; Kučera, J.; MacDonald, A.H. Curie temperature trends in (III,Mn)V ferromagnetic semiconductors. Phys. Rev. B Condens. Matter Mater. Phys. 2002, 66, 124021–124024. [Google Scholar] [CrossRef] [Green Version]
  18. Lu, X.; Tsoi, S.; Miotkowski, I.; Rodriguez, S.; Ramdas, A.K.; Alawadhi, H. Raman electron paramagnetic resonance in Zn1−xCrxTe and Cd1−xCrxTe. Phys. Rev. B Condens. Matter Mater. Phys. 2007, 75, 155206. [Google Scholar] [CrossRef]
  19. Lu, X.; Miotkowski, I.; Rodriguez, S.; Ramdas, A.K.; Alawadhi, H.; Pekarek, T.M. Magnetization and spin-flip Raman scattering in Cd1−xCrxSe and Cd1−xVxSe. Phys. Rev. B Condens. Matter Mater. Phys. 2012, 86, 115213. [Google Scholar] [CrossRef] [Green Version]
  20. Blinowski, J.; Kacman, P.; Majewski, J.A. Ferromagnetism in Cr-based diluted magnetic semiconductors. J. Cryst. Growth 1996, 159, 972–975. [Google Scholar] [CrossRef]
  21. Sajjad, M.; Zhang, H.X.; Noor, N.A.; Alay-E-Abbas, S.M.; Shaukat, A.; Mahmood, Q. Study of half-metallic ferromagnetism in V-doped CdTe alloys by using first-principles calculations. J. Magn. Magn. Mater. 2013, 343, 177–183. [Google Scholar] [CrossRef]
  22. Amari, S.; Méçabih, S.; Abbar, B.; Bouhafs, B. Half-metallic ferromagnetism in ZnCrTe and CdCrTe: Ab initio study. Comput. Mater. Sci. 2011, 50, 2785–2792. [Google Scholar] [CrossRef]
  23. Ge, X.F.; Zhang, Y.M. First-principles study of half-metallic ferromagnetism in Zn1−xCrxSe. J. Magn. Magn. Mater. 2009, 321, 198–202. [Google Scholar] [CrossRef]
  24. Saito, H.; Yamagata, S.; Ando, K. Magnetoresistance in a room temperature ferromagnetic diluted magnetic semiconductor Zn1−xCrxTe. AIP J. Appl. Phys. 2004, 95, 7175–7177. [Google Scholar] [CrossRef]
  25. Jia, X.; Qin, M.; Yang, W. Magnetism in Cr-doped ZnS: Density-functional theory studies. J. Phys. D. Appl. Phys. 2009, 42, 235001. [Google Scholar] [CrossRef] [Green Version]
  26. Soundararajan, D.; Mangalaraj, D.; Nataraj, D.; Dorosinskii, L.; Santoyo-Salazar, J.; Jeon, H.C.; Kang, T.W. Magnetic studies on ZnTe:Cr film grown on glass substrate by thermal evaporation method. Appl. Surf. Sci. 2009, 255, 7517–7523. [Google Scholar] [CrossRef]
  27. Nazir, S.; Ikram, N.; Siddiqi, S.A.; Saeed, Y.; Shaukat, A.; Reshak, A.H. First principles density functional calculations of half-metallic ferromagnetism in Zn1−xCrxS and Cd1−xCrxS. Curr. Opin. Solid State Mater. Sci. 2010, 14, 1–6. [Google Scholar] [CrossRef]
  28. Noor, N.A.; Ali, S.; Shaukat, A. First principles study of half-metallic ferromagnetism in Cr-doped CdTe. J. Phys. Chem. Solids 2011, 72, 836–841. [Google Scholar] [CrossRef]
  29. Ludwig, G.W.; Lorenz, M.R. Paramagnetic resonance of chromium in CdTe. Phys. Rev. 1963, 131, 601–604. [Google Scholar] [CrossRef]
  30. Vallin, J.T.; Watkins, G.D. EPR of Cr2+ in II–VI lattices. Phys. Rev. B 1974, 9, 2051–2072. [Google Scholar] [CrossRef]
  31. Stefaniuk, I.; Bester, M.; Virt, I.S.; Kuzma, M. EPR spectra of Cr in CdTe crystals. Acta Phys. Pol. A 2005, 108, 413–418. [Google Scholar] [CrossRef]
  32. Stefaniuk, I.; Bester, M.; Kuzma, M. Ferromagnetic resonance in CdCrTe solid solution. J. Phys. Conf. Ser. 2008, 104, 012010. [Google Scholar] [CrossRef]
  33. Mac, W.; Twardowski, A.; Eggenkamp, P.J.T.; Swagten, H.J.M.; Shapira, Y.; Demianiuk, M. Magnetic properties of Cr-based diluted magnetic semiconductors. Phys. Rev. B 1994, 50, 14144–14154. [Google Scholar] [CrossRef] [PubMed]
  34. Ko, K.Y.; Blamire, M.G. Temperature dependent magnetization in Cr-doped CdTe crystals. Appl. Phys. Lett. 2006, 88, 172101. [Google Scholar] [CrossRef]
  35. Ko, K.Y.; Blamire, M.G. Temperature-induced magnetic phase transition in bulk Cr-doped CdTe Crystals. J. Korean Phys. Soc. 2006, 49, 591–595. [Google Scholar]
  36. Sonoda, S.; Shimizu, S.; Sasaki, T.; Yamamoto, Y.; Hori, H. Molecular beam epitaxy of wurtzite (Ga, Mn)N films on sapphire(0 0 0 1) showing the ferromagnetic behaviour at room temperature. J. Cryst. Growth 2002, 237–239, 1358–1362. [Google Scholar] [CrossRef] [Green Version]
  37. Theodoropoulou, N.; Hebard, A.F.; Overberg, M.E.; Abernathy, C.R.; Pearton, S.J.; Chu, S.N.G.; Wilson, R.G. Magnetic and structural properties of Mn-implanted GaN. Appl. Phys. Lett. 2001, 78, 3475–3477. [Google Scholar] [CrossRef] [Green Version]
  38. Reed, M.L.; El-Masry, N.A.; Stadelmaier, H.H.; Ritums, M.K.; Reed, M.J.; Parker, C.A.; Roberts, J.C.; Bedair, S.M. Room temperature ferromagnetic properties of (Ga, Mn)N. Appl. Phys. Lett. 2001, 79, 3473–3475. [Google Scholar] [CrossRef]
  39. Hashimoto, M.; Zhou, Y.K.; Kanamura, M.; Asahi, H. High temperature (>400 K) ferromagnetism in III–V-based diluted magnetic semiconductor GaCrN grown by ECR molecular-beam epitaxy. Solid State Commun. 2002, 122, 37–39. [Google Scholar] [CrossRef]
  40. Pekarek, T.M.; Luning, J.E.; Miotkowski, I.; Crooker, B.C. Magnetization and heat-capacity measurements on Zn1−xCrxTe. Phys. Rev. B 1994, 50, 16914–16920. [Google Scholar] [CrossRef]
  41. Pekarek, T.M.; Arenas, D.J.; Crooker, B.C.; Miotkowski, I.; Ramdas, A.K. Magnetic measurements on ferromagnetic behavior in the bulk II–VI diluted magnetic semiconductor Zn1−xCrxTe. J. Appl. Phys. 2004, 95, 7178–7180. [Google Scholar] [CrossRef] [Green Version]
  42. Saito, H.; Zayets, V.; Yamagata, S.; Ando, K. Room-temperature ferromagnetism in highly Cr-doped II–VI diluted magnetic semiconductor Zn1−xCrxTe. AIP J. Appl. Phys. 2003, 93, 6796–6798. [Google Scholar] [CrossRef]
  43. Dahal, J.N.; Ali, K.S.; Mishra, S.R.; Alam, J. Structural, Magnetic, and Mössbauer Studies of Transition Metal-Doped Gd2Fe16Ga0.5TM0.5 Intermetallic Compounds (TM = Cr, Mn, Co, Ni, Cu, and Zn). Magnetochemistry 2018, 4, 54. [Google Scholar] [CrossRef] [Green Version]
  44. Popovych, V.D.; Virt, I.S.; Sizov, F.F.; Tetyorkin, V.V.; Tsybrii (Ivasiv), Z.F.; Darchuk, L.O.; Parfenjuk, O.A.; Ilashchuk, M.I. The effect of chlorine doping concentration on the quality of CdTe single crystals grown by the modified physical vapor transport method. J. Cryst. Growth 2007, 308, 63–70. [Google Scholar] [CrossRef]
  45. Larson, B.E.; Ehrenreich, H. Anisotropic superexchange and spin-resonance linewidth in diluted magnetic semiconductors. Phys. Rev. B 1989, 39, 1747–1759. [Google Scholar] [CrossRef] [PubMed]
  46. Son, P.K.; Heo, K.C.; Ok, C.I.; Jeen, G.S.; Kim, J.W. EPR lineshape and g-factor of Cd1−xMnxTe. J. Korean Phys. Soc. 2000, 37, 287–289. [Google Scholar]
  47. Shames, A.I.; Rozenberg, E.; Martin, C.; Maignan, A.; Raveau, B.; André, G.; Gorodetsky, G. Crystallographic structure and magnetic ordering in CaMn1−xRuxO3 (x ≤ 0.40) manganites: Neutron diffraction, ac susceptibility, and electron magnetic resonance studies. Phys. Rev. B Condens. Matter Mater. Phys. 2004, 70, 134433. [Google Scholar] [CrossRef]
  48. Joshi, J.P.; Sood, A.K.; Bhat, S.V.; Parashar, S.; Raju, A.R.; Rao, C.N.R. An electron paramagnetic resonance study of phase segregation in Nd0.5Sr0.5MnO3. J. Magn. Magn. Mater. 2004, 279, 91–102. [Google Scholar] [CrossRef] [Green Version]
  49. Abragam, A.; Bleaney, B. Electron Paramagnetic Resonance of Transition Ions; Oxford University Press: Oxford, UK, 2012; ISBN 9780199651528. [Google Scholar]
  50. Weil, J.A.; Bolton, J.R.; Wertz, J.E. Electron Paramagnetic Resonance: Elementary Theory and Practical Applications; John Wiley & Sons, Interscience: Hoboken, NJ, USA, 1994; Volume 113, ISBN 0471572349. [Google Scholar]
  51. Kittel, C. On the theory of ferromagnetic resonance absorption. Phys. Rev. 1948, 73, 155–161. [Google Scholar] [CrossRef]
  52. Dijkstra, J.; Weitering, H.H.; Van Bruggen, C.F.; Haas, C.; De Groot, R.A. Band-structure calculations, and magnetic and transport properties of ferromagnetic chromium tellurides (CrTe, Cr3Te4, Cr2Te3). J. Phys. Condens. Matter 1989, 1, 9141–9161. [Google Scholar] [CrossRef] [Green Version]
  53. Dyck, J.S.S.; Drašar, Č.; Lošt’ák, P.; Uher, C. Low-temperature ferromagnetic properties of the diluted magnetic semiconductor Sb2−xCrxTe3. Phys. Rev. B Condens. Matter Mater. Phys. 2005, 71, 115214. [Google Scholar] [CrossRef] [Green Version]
  54. Huber, D.L.; Alejandro, G.; Caneiro, A.; Causa, M.T.; Prado, F.; Tovar, M.; Oseroff, S.B. EPR linewidths in La1−xCaxMnO3 0 < x < 1. Phys. Rev. B 1999, 60, 12155–12161. [Google Scholar] [CrossRef]
  55. de Biasi, R.S.; Gondim, E.C. Use of ferromagnetic resonance to determine the size distribution of γ-Fe2O3 nanoparticles. Solid State Commun. 2006, 138, 271–274. [Google Scholar] [CrossRef]
  56. Vargas, J.M.; Zysler, R.D.; Butera, A. Order-disorder transformation in FePt nanoparticles studied by ferromagnetic resonance. Appl. Surf. Sci. 2007, 254, 274–277. [Google Scholar] [CrossRef]
  57. Yalcin, O. Ferromagnetic Resonance—Theory and Applications; InTech: Rijeka, Croatia, 2013. [Google Scholar]
Materials 14 03449 g001 550
Figure 1. EPR spectra of the sample 1p at various temperatures in K.
Figure 1. EPR spectra of the sample 1p at various temperatures in K.
Materials 14 03449 g001
Materials 14 03449 g002 550
Figure 2. EPR spectra of the sample 2p at various temperatures in K.
Figure 2. EPR spectra of the sample 2p at various temperatures in K.
Materials 14 03449 g002
Materials 14 03449 g003 550
Figure 3. EPR spectra of the sample 3p at various temperatures in K.
Figure 3. EPR spectra of the sample 3p at various temperatures in K.
Materials 14 03449 g003
Materials 14 03449 g004 550
Figure 4. EPR spectra of the sample 4p at various temperatures in K.
Figure 4. EPR spectra of the sample 4p at various temperatures in K.
Materials 14 03449 g004
Materials 14 03449 g005 550
Figure 5. EPR spectra of the sample 5p at various temperatures in K.
Figure 5. EPR spectra of the sample 5p at various temperatures in K.
Materials 14 03449 g005
Materials 14 03449 g006 550
Figure 6. Representative EPR spectra of Cd1−xCrxTe powdered crystals: (a) sample No. 3p at T = 342 K, (b) sample No 3p at T = 161 K. The arrow marks the theoretical line. The fitting parameters are given in the legend.
Figure 6. Representative EPR spectra of Cd1−xCrxTe powdered crystals: (a) sample No. 3p at T = 342 K, (b) sample No 3p at T = 161 K. The arrow marks the theoretical line. The fitting parameters are given in the legend.
Materials 14 03449 g006
Materials 14 03449 g007 550
Figure 7. The temperature dependence of the effective gyromagnetic factor ge.
Figure 7. The temperature dependence of the effective gyromagnetic factor ge.
Materials 14 03449 g007
Materials 14 03449 g008 550
Figure 8. The temperature dependence of the peak-to-peak linewidth (Hpp).
Figure 8. The temperature dependence of the peak-to-peak linewidth (Hpp).
Materials 14 03449 g008
Materials 14 03449 g009 550
Figure 9. The temperature dependence of the integral intensity It ( I t = A   ×   H pp 2 ).
Figure 9. The temperature dependence of the integral intensity It ( I t = A   ×   H pp 2 ).
Materials 14 03449 g009
Materials 14 03449 g010 550
Figure 10. XRD spectra of CrTe, CdTe, (a–d)—according to the ASTM tables, and CdTe, CdCrTe (e,f)—experimental results.
Figure 10. XRD spectra of CrTe, CdTe, (a–d)—according to the ASTM tables, and CdTe, CdCrTe (e,f)—experimental results.
Materials 14 03449 g010
Materials 14 03449 g011 550
Figure 11. EPR spectra of powered, solid Cr2Te3, and the sample 4p (T≈260 K).
Figure 11. EPR spectra of powered, solid Cr2Te3, and the sample 4p (T≈260 K).
Materials 14 03449 g011
Materials 14 03449 g012 550
Figure 12. Temperature dependence of the 1/(integral intensity) of the sample 4p.
Figure 12. Temperature dependence of the 1/(integral intensity) of the sample 4p.
Materials 14 03449 g012
Table
Table 1. Samples investigated.
Table 1. Samples investigated.
SampleGrowth Temperature, °CThe Concentration of Cr in Cd1−xCrxTe
Charge, xXFA Measurement, x
1p12800.040.002
2p13000.010.008
3p1300–13100.050.04
4p13200.050.05
5p13200.10.08
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Share and Cite

MDPI and ACS Style

Stefaniuk, I.; Obermayr, W.; Popovych, V.D.; Cieniek, B.; Rogalska, I. EPR Spectra of Sintered Cd1−xCrxTe Powdered Crystals with Various Cr Content. Materials 2021, 14, 3449. https://doi.org/10.3390/ma14133449

AMA Style

Stefaniuk I, Obermayr W, Popovych VD, Cieniek B, Rogalska I. EPR Spectra of Sintered Cd1−xCrxTe Powdered Crystals with Various Cr Content. Materials. 2021; 14(13):3449. https://doi.org/10.3390/ma14133449

Chicago/Turabian Style

Stefaniuk, Ireneusz, Werner Obermayr, Volodymyr D. Popovych, Bogumił Cieniek, and Iwona Rogalska. 2021. "EPR Spectra of Sintered Cd1−xCrxTe Powdered Crystals with Various Cr Content" Materials 14, no. 13: 3449. https://doi.org/10.3390/ma14133449

APA Style

Stefaniuk, I., Obermayr, W., Popovych, V. D., Cieniek, B., & Rogalska, I. (2021). EPR Spectra of Sintered Cd1−xCrxTe Powdered Crystals with Various Cr Content. Materials, 14(13), 3449. https://doi.org/10.3390/ma14133449

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop