Cyclic Mechanical Fatigue Lifetime of Bi0.5Na0.5TiO3-Based Eco-Piezoceramics
Abstract
:1. Introduction
2. Materials and Methods
2.1. Material
2.2. X-ray Diffraction (XRD) and Microstructural Analysis
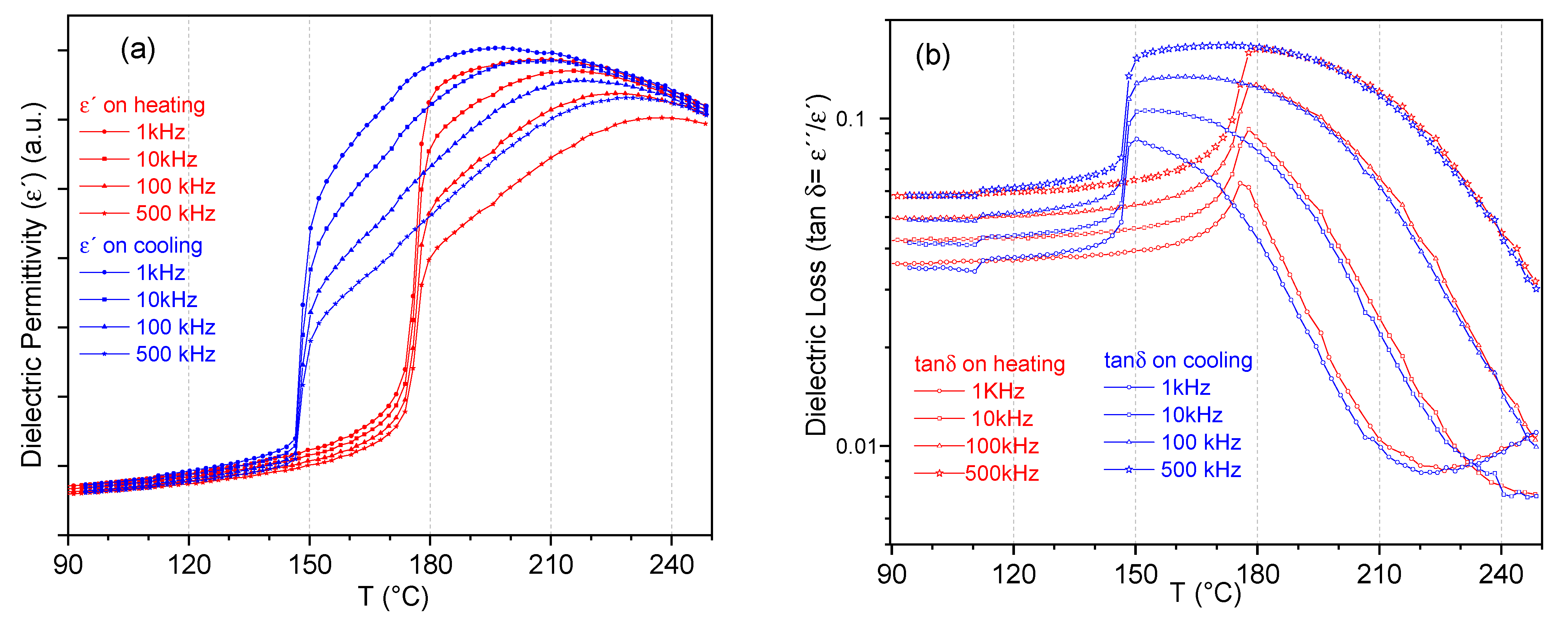
2.3. Electrical Characterization
2.4. Mechanical Characterization
2.4.1. Biaxial Flexural Strength
2.4.2. Fatigue Behavior
3. Results
4. Discussion
5. Conclusions
- −
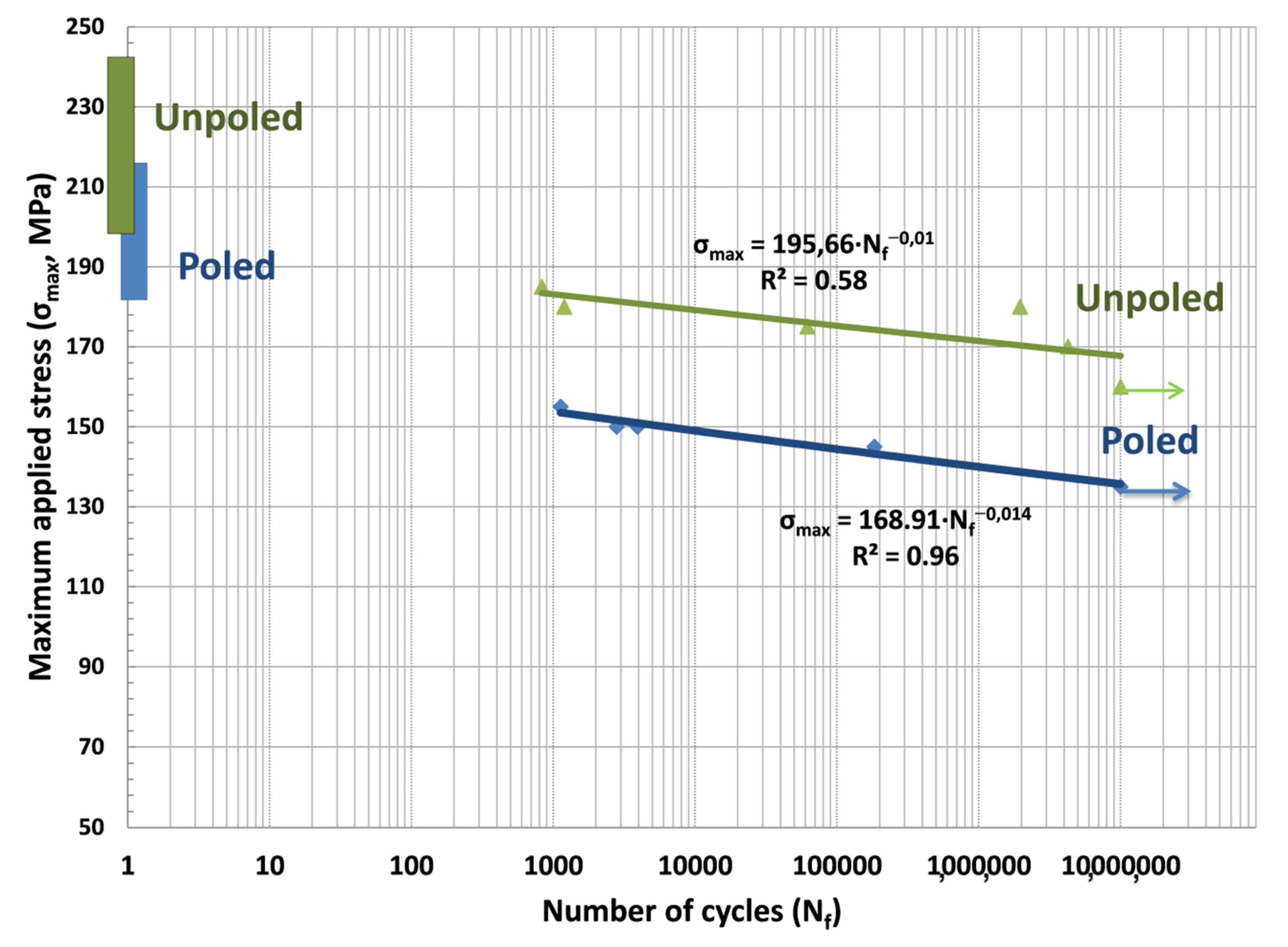
- The biaxial bending strength and fatigue strength for the unpoled samples are about 10% and 15% higher than for the poled samples, respectively.
- −
- The fatigue lifetime of the poled samples is much shorter than that of unpoled ones when subjected to the same external stress, which indicates a lower cyclic fatigue resistance.
- −
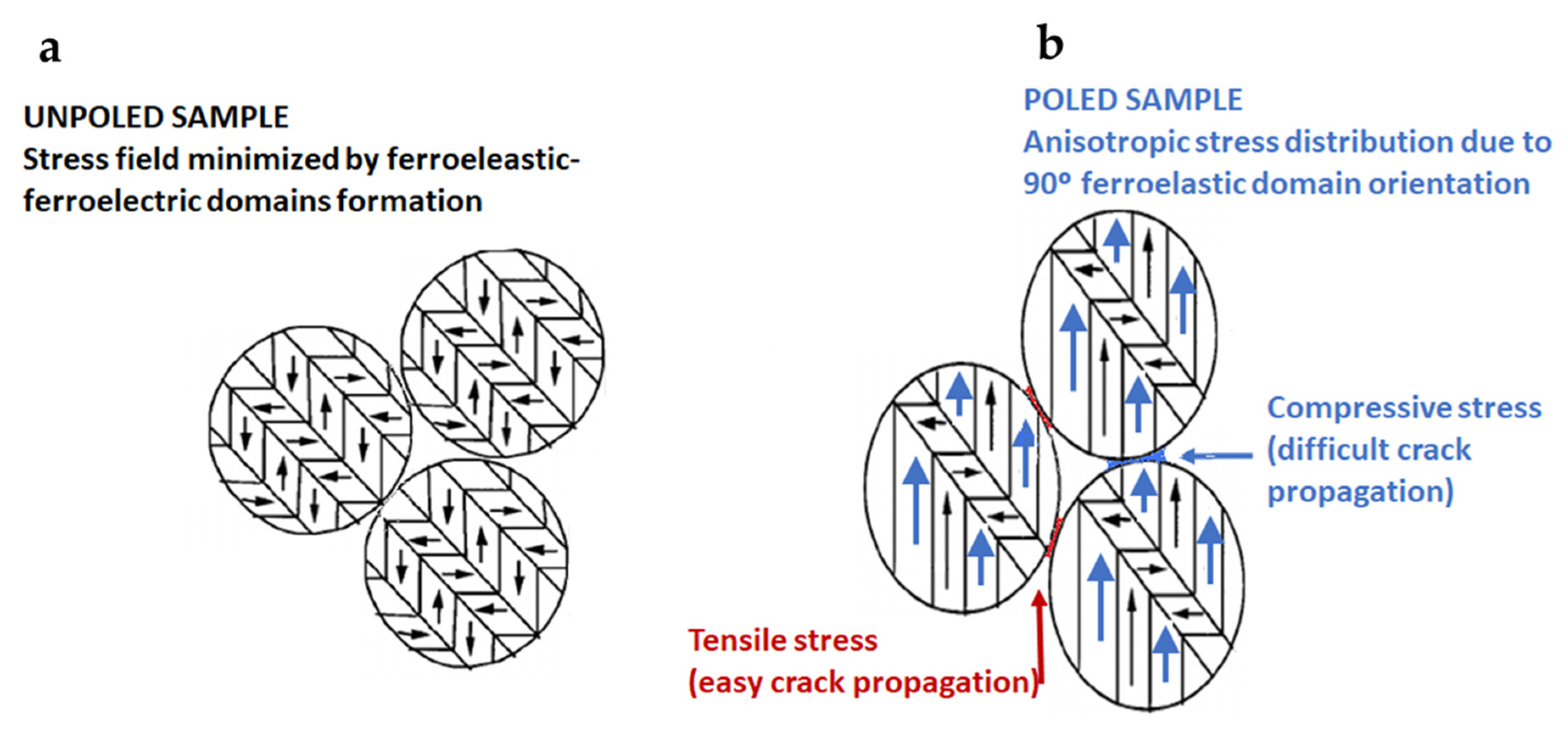
- The different fatigue crack growth behavior produces a different fracture pattern, with flat transgranular-based fractures in the unpoled samples and a mixture of transgranular and intergranular wavy fractures in the poled ones.
- −
- The poling process decreases the mechanical strength and further deteriorates the cyclic fatigue properties due to the domain orientation that generates an anisotropic residual stress field; crack propagation occurs mainly along the domain walls and the grain boundaries.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Harne, R.L.; Wang, K.W. A review of the recent research on vibration energy harvesting via bistable systems. Smart Mater. Struct. 2013, 22, 023001. [Google Scholar] [CrossRef]
- Lupascu, D.; Rödel, J. Fatigue in Bulk Lead Zirconate Titanate. Actuator Mater. 2005, 7, 882–898. [Google Scholar] [CrossRef]
- Okayasu, M.; Ogawa, T. Failure Characteristics of PZT Ceramic During Cyclic Loading. J. Electron. Mater. 2020, 49, 5534–5541. [Google Scholar] [CrossRef]
- EU-Directive 2011/65/EU: Restriction of the use of certain hazardous substances in electrical and electronic equipment (RoHS). Off. J. Eur. Communities 2013, 46, 19–23.
- Sezer, N.; Muammer, K. A comprehensive review on the state-of-the-art of piezoelectric energy harvesting. Nano Energy 2021, 80, 105567. [Google Scholar] [CrossRef]
- Schneider, G. A Influence of Electric Field and Mechanical Stresses on the Fracture of Ferroelectrics. Annu. Rev. Mater. Res. 2007, 37, 491–538. [Google Scholar] [CrossRef]
- Okayasu, M.; Bamba, K. Domain switching behavior in lead zirconate titanate piezoelectric ceramics. Scr. Mater. 2018, 146, 272–275. [Google Scholar] [CrossRef]
- Pojprapai, S.I.; Jones, J.L.; Studer, A.J.; Russell, J.; Valanoor, N.; Hoffman, M. Ferroelastic domain switching fatigue in lead zirconate titanate ceramics. Acta Mater. 2008, 56, 1577–1587. [Google Scholar] [CrossRef]
- Koruza, J.; Bell, A.J.; Frömling, T.; Webber, K.G.; Wang, K.; Rödel, J. Requirements for the transfer of lead-free piezoceramics into application. J. Materiomics 2018, 4, 13–26. [Google Scholar] [CrossRef]
- Shibata, K.; Wang, R.; Tou, T.; Koruza, J. Applications of lead-free piezoelectric materials. MRS Bull. 2018, 43, 612–616. [Google Scholar] [CrossRef]
- Webber, K.G.; Vögler, M.; Khansur, N.H.; Kaeswurm, B.; Daniels, J.E.; Schader, F.H. Review of the mechanical and fracture behavior of perovskite lead-free ferroelectrics for actuator applications. Smart Mater Struct. 2017, 26, 063001. [Google Scholar] [CrossRef]
- Denkhaus, S.M.; Vögler, M.; Novak, N.; Rödel, J. Short crack fracture toughness in (1 − x)(Na1/2Bi1/2)TiO3-xBaTiO3 relaxor ferroelectrics. J. Am. Ceram. Soc. 2017, 100, 4760–4769. [Google Scholar] [CrossRef]
- Vögler, M.; Daniels, J.E.; Webber, K.G.; Rödel, J. Absence of toughening behavior in 0.94(Na1/2Bi1/2)TiO3-0.06BaTiO3 relaxor ceramic. Scr Mater. 2017, 136, 115–119. [Google Scholar] [CrossRef]
- Takahashi, H.; Nagata, H.; Takenaka, T. Mechanical bending strength of Bi0.5Na0.5)TiO3-based lead-Free piezoelectric ceramics. J. Asian Ceram. Soc. 2017, 5, 242–246. [Google Scholar] [CrossRef]
- Zhang, T.Y.; Gao, C.F. Fracture behaviors of piezoelectric materials. Theor. Appl. Frac. Mech. 2004, 41, 339–379. [Google Scholar] [CrossRef]
- Wang, H.; Lin, H.T.; Wereszczak, A.A. Strength Properties of poled lead zirconate titanate subjected to biaxial flexural loading in high electric field. J. Am. Ceram. Soc. 2010, 93, 2843–2849. [Google Scholar] [CrossRef]
- Zhang, K.; Zeng, F.W.; Wang, H.; Lin, H.-T. Biaxial flexural strength of poled lead zirconate titanate under high electric field with extended field range. Ceram. Int. 2013, 39, 2023–2030. [Google Scholar] [CrossRef]
- Quintana, C.; Rodríguez, C.; Belzunce, F.J.; Caballero, A.C.; Baudín, C. Ceramic materials characterization using miniature mechanical tests:comparison between B3B and SPT tests. J. Eur. Ceram. Soc. 2019, 39, 4113–4121. [Google Scholar] [CrossRef]
- Wöhler, A. Bericht über die versuche, welche auf der Königl. Niederschlesisch-Markischen Eisenbahn mit Apparaten zum Messen der Biegung und Verdrehung von Eisenbahn-wagen-Achsen wahrend der Fahrt, angestellt wurden. Z. Bauwese. 1858, 8, 642–652. [Google Scholar]
- Gall, M.; Thielicke, B.; Schmidt, I. Integrity of piezoceramic patch transducers under cyclic loading at different temperatures. Smart Mater. Struct. 2009, 18, 104009. [Google Scholar] [CrossRef]
- Tai, W.; Kim, S.-H. Relationship between cyclic loading and degradation of piezoelectric properties in Pb(Zr,Ti)03 ceramics. Mater. Sci. Eng. B 1996, 38, 182–185. [Google Scholar] [CrossRef]
- Kaduichi, K.; Yamamoto, T.; Kitagawa, K. Cyclic Fatigue Behavior and Mechanical Properties of PZT Piezoelectric Ceramics. J. Jpn. Soc. Powder Metall. 2005, 52, 16–21. [Google Scholar] [CrossRef] [Green Version]
- Okayasu, M.; Ozeki, G.; Mizuno, M. Fatigue failure characteristics of lead zirconate titanate piezoelectric ceramics. J. Eur. Ceram. Soc. 2010, 30, 713–725. [Google Scholar] [CrossRef]
- Makino, H.; Kamiya, N. Effects of dc Electric Field on Mechanical Properties of Piezoelectric Ceramics. Jpn. J. Appl. Phys. 1994, 33, 5323–5327. [Google Scholar] [CrossRef]
- PI Piezo Technology. Available online: https://www.piceramic.com/en/products/piezoelectric-materials/ (accessed on 18 June 2021).
- Ditas, P.; Hennig, E.; Kynast, A. Lead-Free Piezoceramic Materials for Ultrasonic Applications. In Proceedings of the Sensors and Measuring Systems ITG/GMA Symposium, Nuremberg, Germany, 3–4 June 2014; Volume 17, pp. 1–4. [Google Scholar]
- Fuentes-Cobas, L.; Montero-Cabrera, M.; Pardo, L.; Fuentes-Montero, L. Ferroelectrics under the Synchrotron Light: A Review. Materials 2016, 9, 34. [Google Scholar] [CrossRef] [Green Version]
- Montero-Cabrera, M.E.; Pardo, L.; García, A.; Fuentes-Montero, M.E.; Ballinas-Casarrubias, M.L.; Fuentes-Cobas, L.E. The Global and Local Symmetries of Nanostructured Ferroelectric Relaxor 0. 94(Bi0.5Na0.5)TiO3-0.06BaTiO3 Ferroelectrics 2014, 469, 50–60. [Google Scholar] [CrossRef]
- Rodriguez-Carvajal, J. FULLPROF: A program for Rietveld refinement and pattern matching analysis. In Proceedings of the Satellite Meeting on Powder Diffraction of the XV Congress of the International Union of Crystallography, Touluse, France, 16–19 July 1990. [Google Scholar]
- Fuentes-Cobas, L.E. Synchrotron Radiation Diffraction and Scattering in Ferroelectrics. In Multifunctional Polycrystalline Ferroelectric Materials: Processing and Properties; Springer: Dordrecht, The Netherlands, 2011; pp. 217–280. [Google Scholar]
- Schönau, K.A.; Knapp, M.; Kungl, H.; Hoffmann, M.J.; Fuess, H. In situ synchrotron diffraction investigation of morphotropic PbZr1-xTixO3 under an applied electric field. PRB 2007, 76, 144112. [Google Scholar] [CrossRef] [Green Version]
- Jo, W.; Daniels, J.E.; Jones, J.L.; Tan, X.; Thomas, P.A.; Damjanovic, D.; Rödel, J. Evolving morphotropic phase boundary in lead-free (Bi1/2Na1/2)TiO3-BaTiO3 piezoceramics. J. Appl. Phys. 2011, 109, 014110. [Google Scholar] [CrossRef] [Green Version]
- Pardo, L.; García, A.; Brebol, K.; Mercadelli, E.; Galassi, C. Enhanced properties for ultrasonic transduction, phase transitions and thermal depoling in 0.96 (Bi0.5Na0.5) TiO3-0.04BaTiO3 submicrometer-structured ceramics. J. Phys. D. Appl. Phys. 2011, 44, 335404. [Google Scholar] [CrossRef] [Green Version]
- Subbarao, E.C.; Srikanth, V.; Cao, W.; Cross, L.E. Domain switching and microcracking during poling of lead zirconate titanate ceramics. Ferroelectrics 1993, 145, 271–281. [Google Scholar] [CrossRef]
- International Organization for Standardization. Dynamic Loading Test for Endosseous Dental Implants; ISO 14801; International Organization for Standardization: Geneva, Switzerland, 2016. [Google Scholar]
- American Society for Testing and Materials. ASTM F 394–78: Standard Test Method for Biaxial Flexure Strength (Modulus of Rupture) of Ceramic Substrates; American Society for Testing and Materials: West Conshohocken, PA, USA, 2001. [Google Scholar]
- Smirnov, A.; Bartolomé, J.F. Mechanical properties and fatigue life of ZrO2-Ta composites prepared by hot pressing. J. Eur. Ceram. Soc. 2012, 32, 3899–3904. [Google Scholar] [CrossRef]
- Smirnov, A.; Bartolomé, J.F. Microstructure and mechanical properties of ZrO2 ceramics toughened by 5–20 vol % Ta metallic particles fabricated by pressureless sintering. Ceram. Int. 2014, 40, 1829–1834. [Google Scholar] [CrossRef]
- Bartolomé, J.F.; Requena, J.; Moya, J.S.; Li, M.; Guiu, F. Cyclic fatigue of Al2O3 and Al2O3—Al2TiO5 composites in direct push-pull. Fatigue Fract. Eng. Mater. Struct. 1997, 20, 789–798. [Google Scholar] [CrossRef]
- Smirnov, A.; Gutierrez-Gonzalez, C.F.; Bartolomé, J.F. Cyclic Fatigue Life- and Crack-Growth Behavior of Zirconia-Niobium Composites. J. Am. Ceram. Soc. 2013, 96, 1709–1712. [Google Scholar] [CrossRef]
- Bartolomé, J.F.; Moya, J.S.; Requena, J.; Llorca, J.; Anglada, M. Fatigue Crack Growth Behavior in Mullite/Alumina Functionally Graded Ceramics. J. Am. Ceram. Soc. 1998, 81, 1502–1508. [Google Scholar] [CrossRef]
- Smirnov, A.; Beltrán, J.-I.; Rodriguez-Suarez, T.; Pecharromán, C.; Muñoz, M.C.; Moya, J.S.; Bartolomé, J.F. Unprecedented simultaneous enhancement in damage tolerance and fatigue resistance of zirconia/Ta composites. Sci. Rep. 2017, 7, 44922. [Google Scholar] [CrossRef] [Green Version]
- Buch, A. Fatigue Strength Calculation; Transtech Publications: Aedermannsdorf, Switzerland, 1998. [Google Scholar]
- Siváka, P.; Ostertagová, E. Evaluation of fatigue tests by means of mathematical statistics. Procedia Eng. 2012, 48, 636–642. [Google Scholar] [CrossRef] [Green Version]
- Berlincourt, D.; Krueger, H.A. Domain Processes in Lead Titanate Zirconate and Barium Titanate Ceramics. J. Appl. Phys. 1959, 30, 1804. [Google Scholar] [CrossRef]
- Chu, B.; Chen, D.-R.; Li, G.-R.; Yin, Q.-R. Electrical properties of (Na1/2Bi1/2)TiO3-BaTiO3 ceramics. J. Eur. Ceram. Soc. 2002, 22, 2115–2121. [Google Scholar] [CrossRef]
- Craciun, F.; Galassi, C.; Birjega, R. Electric-field-induced and spontaneous relaxor-ferroelectric phase transitions in (Na1/2Bi1/2)1−xBaxTiO3. J. Appl. Phys. 2012, 112, 124106. [Google Scholar] [CrossRef] [Green Version]
- Fu, R.; Zhang, T.-Y. Influences of temperature and electric field on the bending strength of lead zirconate titanate ceramics. Acta Mater. 2000, 48, 1729–1740. [Google Scholar] [CrossRef]


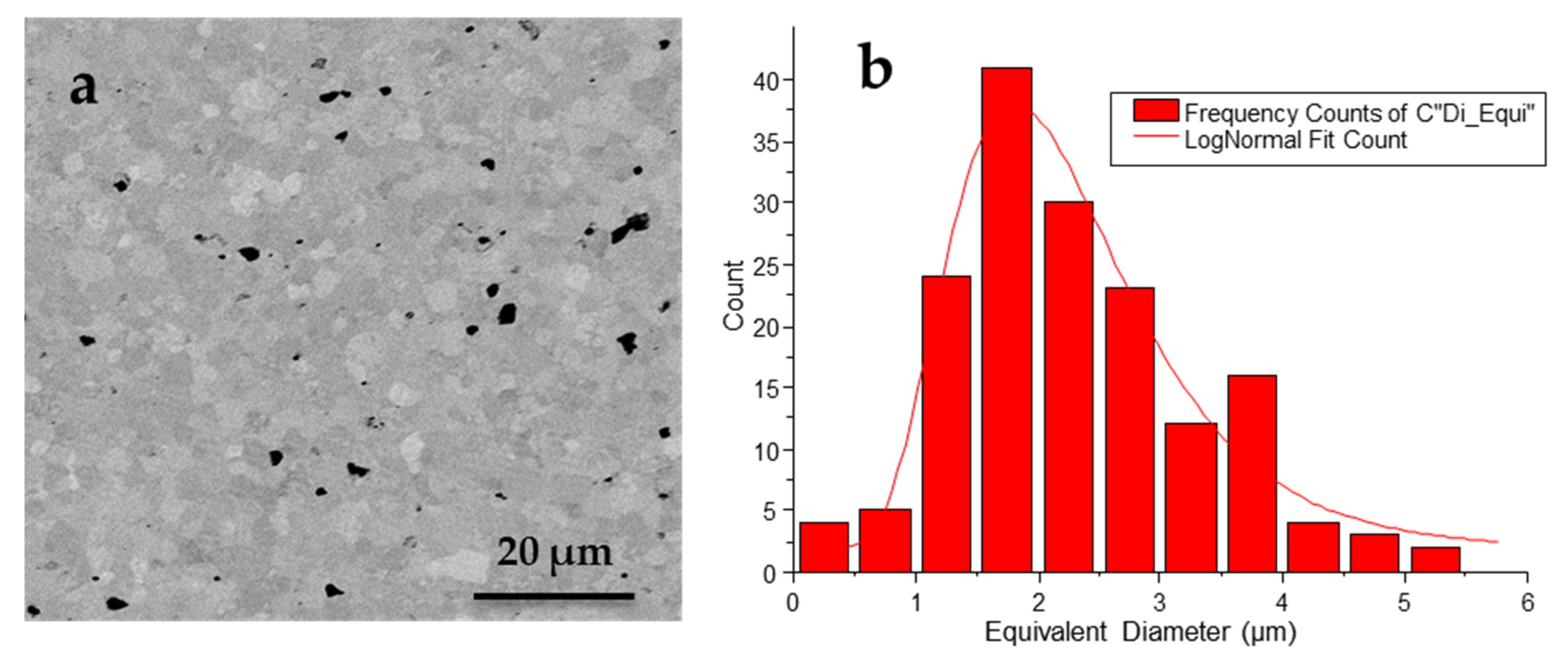

| PIC700 Perovskite-Type Structure | Density ρ (g.cm−3) | Mean Grain Size <G> (μm) | <G>’s Standard Deviation σ<G> (μm) | Porosity p (%) |
|---|---|---|---|---|
| 5.76 | 2.12 ± 0.09 | 0.20 ± 0.03 | 3.2 ± 0.3 | |
| Symmetry | S.G. | a (Å) | c (Å) | c/a |
| Tetragonal | P4 mm | 3.9024 (1) | 3.9747 (2) | 1.019 (1) |
| Material | Bending Strength (MPa) | Fatigue Limit (MPa) | Fatigue Exponent (B) | Fatigue Coefficient (A) (MPa) |
|---|---|---|---|---|
| Unpoled | 220 ± 20 | 160 | −0.010 | 196 |
| Poled | 200 ± 13 | 135 | −0.014 | 169 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bartolomé, J.F.; Fuentes-Cobas, L.E.; García, Á.; Jacas, A.; Pardo, L. Cyclic Mechanical Fatigue Lifetime of Bi0.5Na0.5TiO3-Based Eco-Piezoceramics. Materials 2021, 14, 4113. https://doi.org/10.3390/ma14154113
Bartolomé JF, Fuentes-Cobas LE, García Á, Jacas A, Pardo L. Cyclic Mechanical Fatigue Lifetime of Bi0.5Na0.5TiO3-Based Eco-Piezoceramics. Materials. 2021; 14(15):4113. https://doi.org/10.3390/ma14154113
Chicago/Turabian StyleBartolomé, José F., Luis E. Fuentes-Cobas, Álvaro García, Alfredo Jacas, and Lorena Pardo. 2021. "Cyclic Mechanical Fatigue Lifetime of Bi0.5Na0.5TiO3-Based Eco-Piezoceramics" Materials 14, no. 15: 4113. https://doi.org/10.3390/ma14154113
APA StyleBartolomé, J. F., Fuentes-Cobas, L. E., García, Á., Jacas, A., & Pardo, L. (2021). Cyclic Mechanical Fatigue Lifetime of Bi0.5Na0.5TiO3-Based Eco-Piezoceramics. Materials, 14(15), 4113. https://doi.org/10.3390/ma14154113








