Effect of Partial Ba Substitutions on the Crystallization of Sr2TiSi2O8 (STS) Glass–Ceramics and on the Generation of a SAW Signal at High Temperatures
Abstract
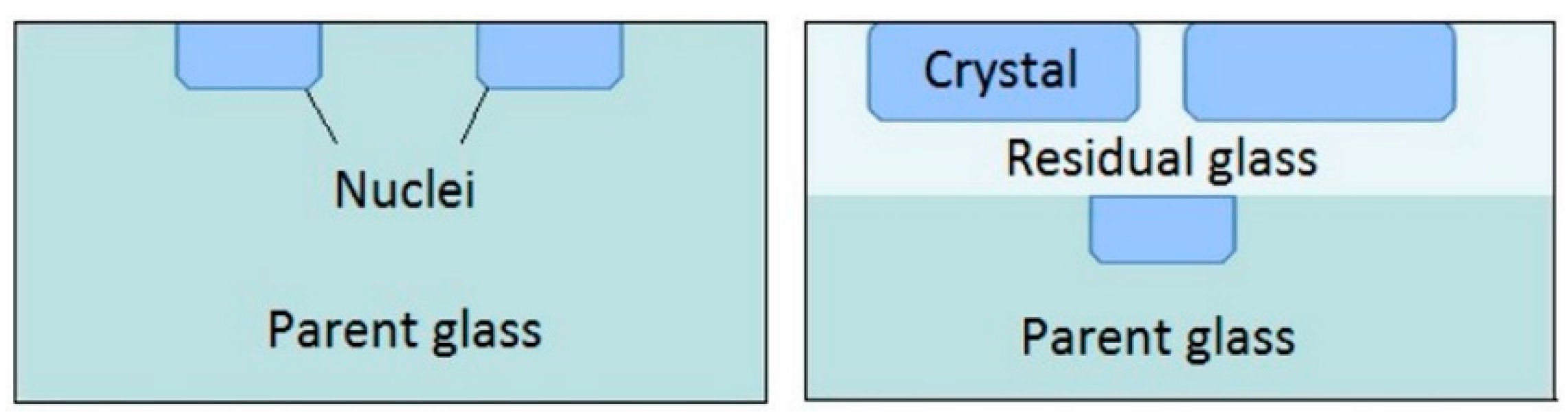
:1. Introduction
2. Materials and Methods
2.1. Synthesis of the Glass–Ceramics
- -
- 300 °C/h from room temperature to 900 °C;
- -
- Dwell time of 20 h at 900 °C;
- -
- Slow cooling in switch-off furnace;
- -
- When needed, shorter dwell times are applied.
2.2. Investigation of the Crystallization
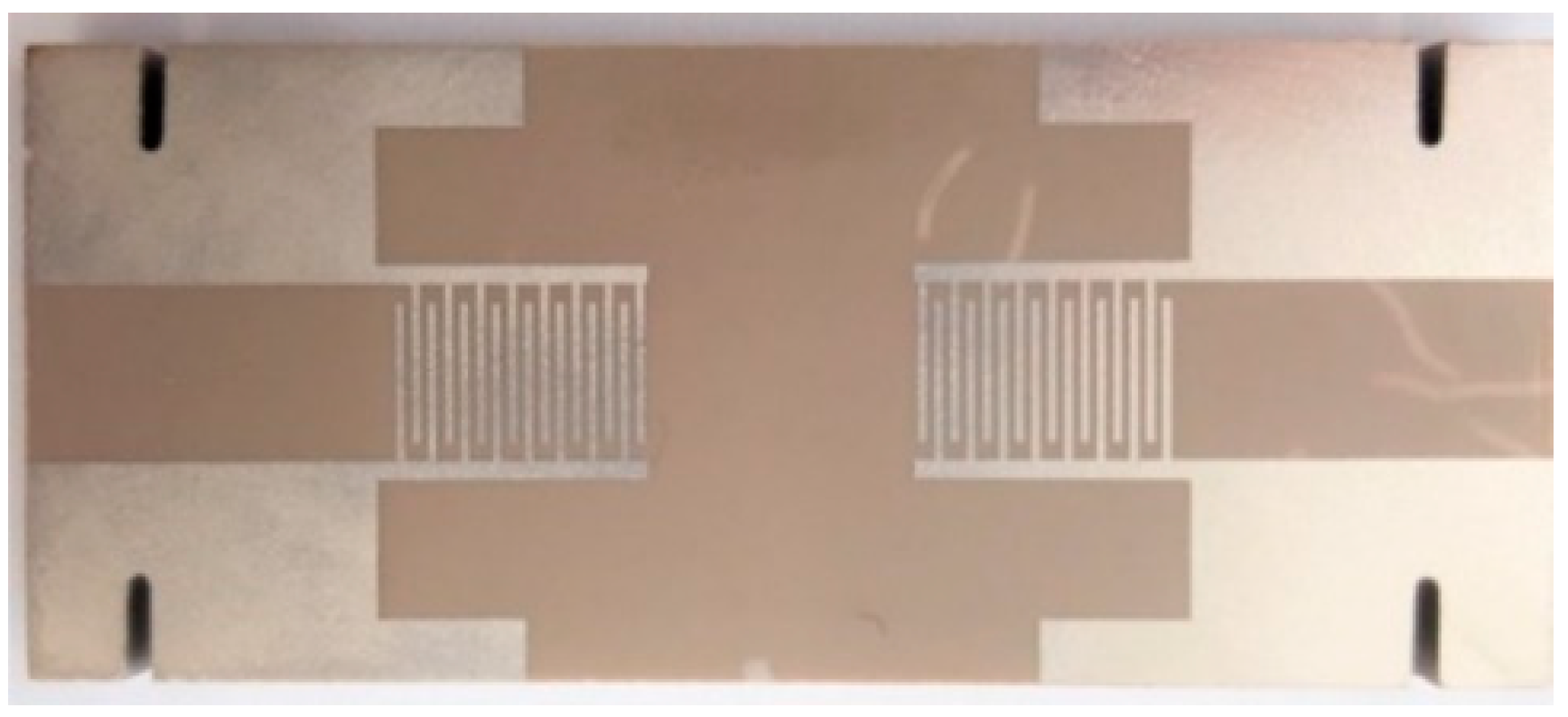
2.3. SAW Testing Devices
3. Results
3.1. Densities and Glass Transition Temperatures
3.2. Crystallization
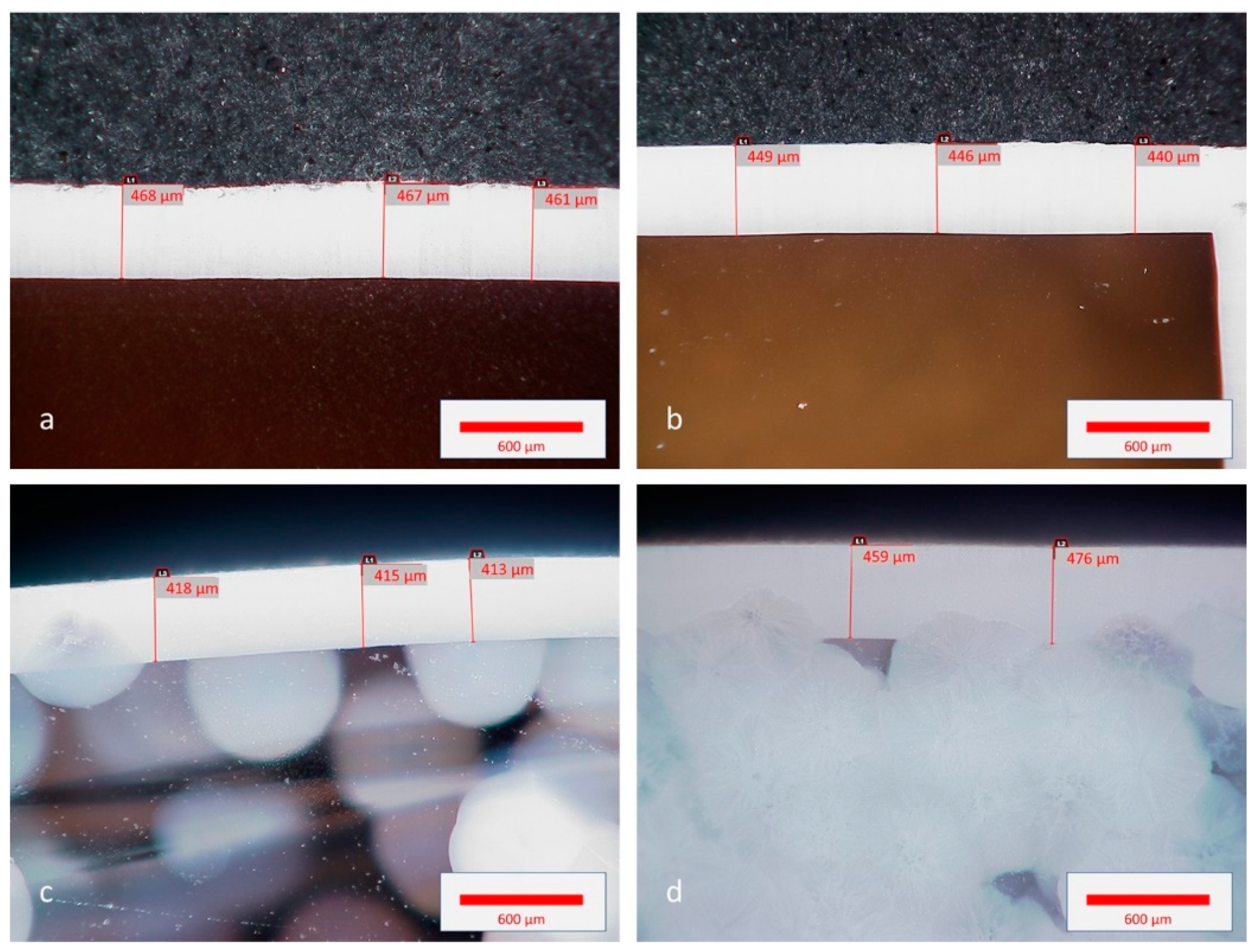
3.2.1. Effect of the Ba Substitution on the Characteristics of the Fresnoite Crystals in the Glass–Ceramic
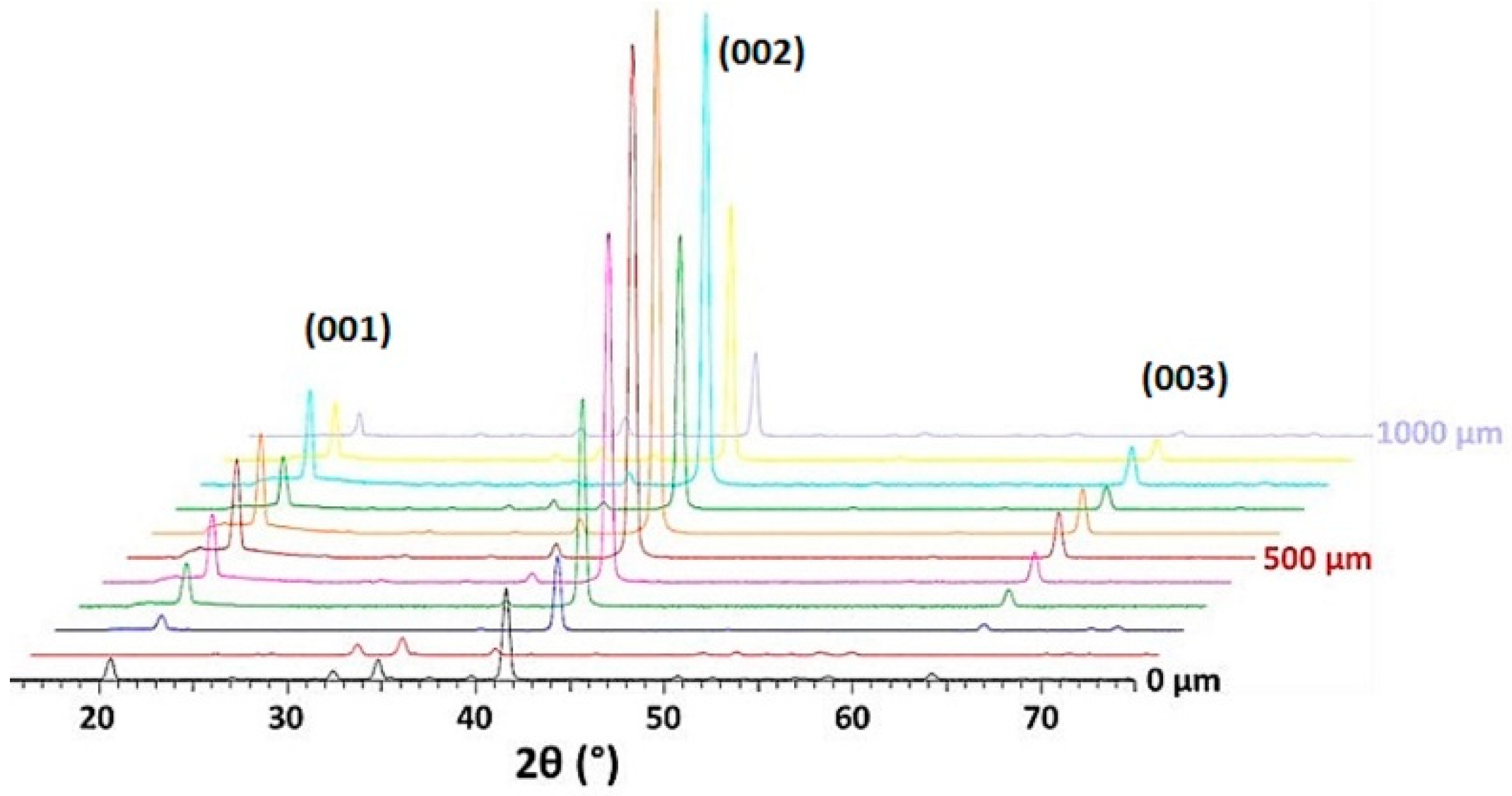
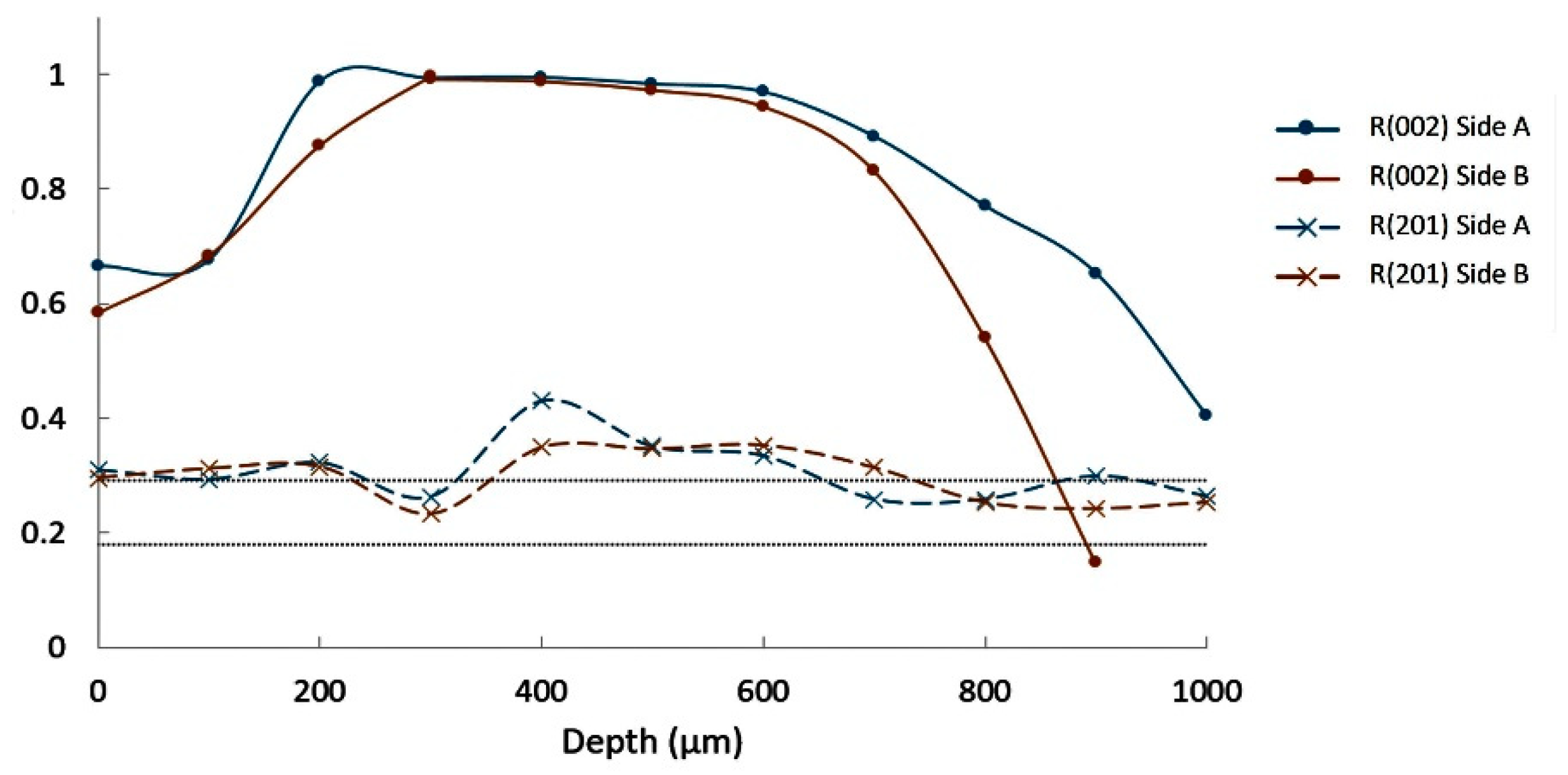
3.2.2. Effect of the Substitution on the Orientation of the Fresnoite Crystals
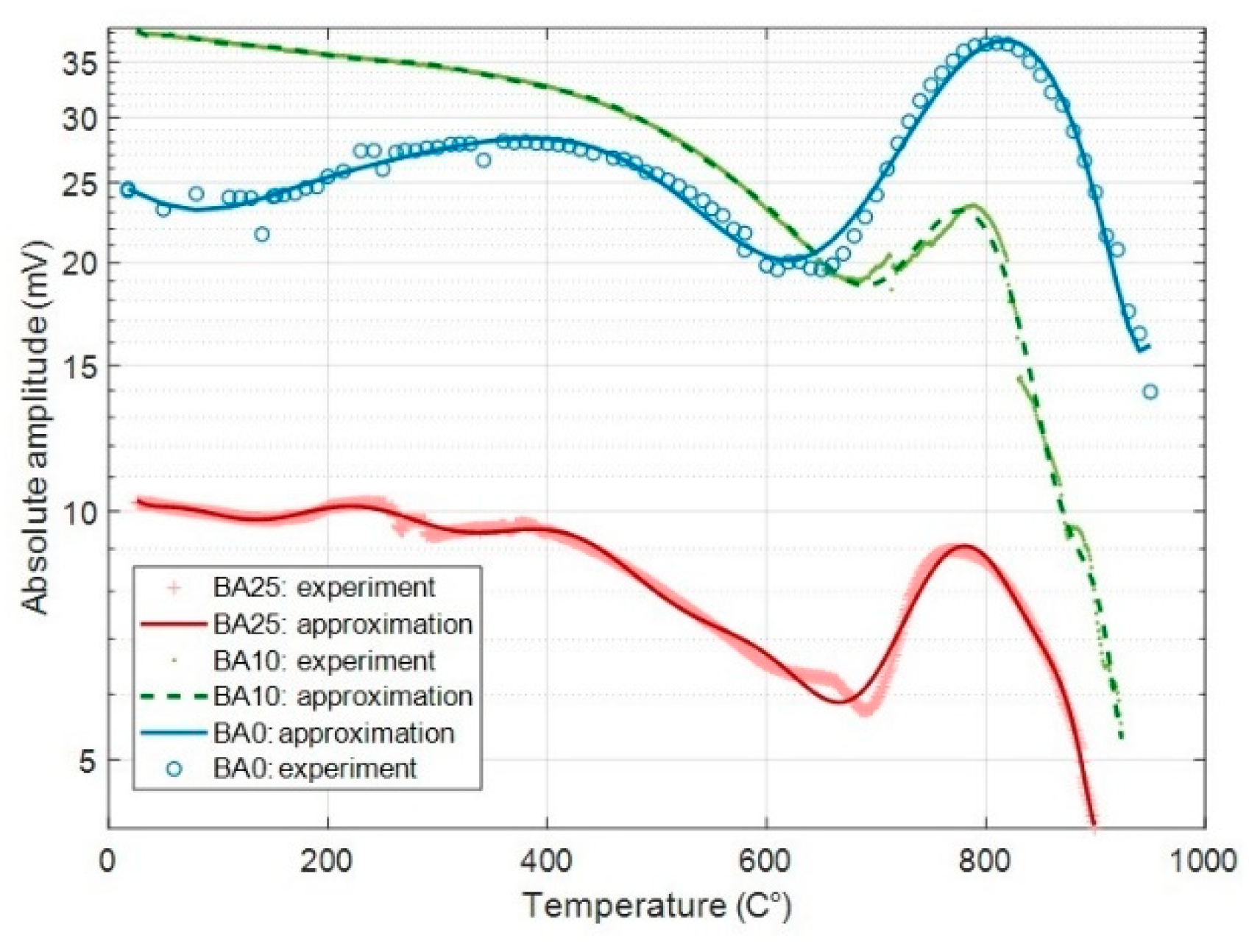
3.3. Testing of the SAW Devices
4. Discussion
4.1. Effect of the Substitution on the Characteristics of Glass–Ceramics
4.2. Test of the SAW Devices
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Heywang, W.; Lubitz, K.; Wersing, W. (Eds.) Piezoelectricity Evolution and Future of a Technology; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Uchino, K. (Ed.) Advanced Piezoelectric Materials: Science and Technology; Woodhead Publishing Limited: Cambridge, UK, 2010. [Google Scholar]
- Hemangi, K.; Deore, H.A.; Pranita, P. Review on Advanced Piezoelectric Materials (BaTiO3, PZT). JETIR 2008, 6, 950–957. [Google Scholar]
- Lupascu, D.C.; Genenko, Y.A.; Balke, N. Aging in ferroelectrics. J. Am. Ceram 2006, 89, 224–229. [Google Scholar] [CrossRef]
- Turner, R.C.; Fuierer, P.A.; Newnham, R.E.; Shrout, T.R. Materials for high-temperature acoustic and vibration sensors—A review. Appl. Acoust. 1994, 41, 299–324. [Google Scholar] [CrossRef]
- Alfors, J.T.; Stinson, M.C.; Matthews, R.A.; Pabst, A. Seven new barium minerals from Eastern Fresno County. Am. Min. 1965, 50, 314–340. [Google Scholar]
- Moore, P.B.; Louisnathan, J. Fresnoite: Unusal titanium coordination. Science 1967, 156, 1361–1362. [Google Scholar] [CrossRef]
- Kimura, M.; Doi, K.; Nanamatsu, S.; Kawamura, T. A new piezoelectric crystal: Ba2Ge2TiO8. Appl. Phys. Lett. 1973, 23, 531–532. [Google Scholar] [CrossRef]
- Höche, T.; Rüssel, C.; Neumann, W. Incommensurate modulations in Ba2TiSi2O8, Sr2TiSi2O8, and Ba2TiGe2O8. Solid State Commun. 1999, 110, 651–656. [Google Scholar] [CrossRef]
- Moore, P.B.; Louisnathan, J. The crystal structure of fresnoite, Ba(TiO)Si2O7. Z. Krist. 1969, 130, 438–448. [Google Scholar] [CrossRef]
- Höche, T.; Neumann, W.; Esmaeilzadeh, S.; Uecker, R.; Lentzen, M.; Rüssel, C. The crystal structure of Sr2TiSi2O8. J. Solid State Chem. 2002, 166, 15–23. [Google Scholar] [CrossRef]
- Markgraf, S.A.; Bhalla, A.S. Low-temperature phase transition in Ba2TiGe2O8. Phase Transit. 1989, 18, 55–76. [Google Scholar] [CrossRef]
- Müller, R.; Zanotto, E.D.; Fokin, W.M. Surface crystallization of silicates glasses: Nucleation and kinetics. J. Non. Cryt. Solids 2000, 274, 208–231. [Google Scholar] [CrossRef]
- Schmelzer, J.; Pascova, R.; Möller, J.; Gutzow, I. Surface-induced devitrification of glasses: The influence of elastic strains. J. Non. Cryt. Solids 1993, 162, 26–39. [Google Scholar] [CrossRef]
- Halliyal, A.; Bhalla, A.S.; Newnham, R.E. Polar glass ceramics—A new family of electroceramic materials: Tailoring the piezoelectric and pyroelectric properties. Mater. Res. Bull. 1983, 18, 1007–1019. [Google Scholar] [CrossRef]
- Halliyal, A.; Safari, A.; Bhalla, A.S.; Newnham, R.E.; Cross, L.E. Grain-oriented glass-ceramics for piezoelectric devices. J. Am. Ceram 1984, 67, 331–335. [Google Scholar] [CrossRef]
- Halliyal, A.; Bhalla, S.A.; Cross, L.E.; Newnham, R.E. Dielectric, piezoelectric and pyroelectric properties of Sr2TiSi2O8 polar glass-ceramic: A new polar material. J. Mater. Sci. 1985, 20, 3745–3749. [Google Scholar] [CrossRef]
- Halliyal, A.; Bhalla, S.A.; Newnham, R.E.; Cross, E. Glass-ceramics for piezoelectric and pyroelectric devices. In Glass and Glass-Ceramics; Lewis, M.H., Ed.; Springer: Dordrecht, The Netherlands, 1989; pp. 272–315. [Google Scholar]
- Wisniewski, W.; Thieme, K.; Rüssel, C. Fresnoite glass-ceramics—A review. Prog. Mater. Sci. 2018, 98, 68–107. [Google Scholar] [CrossRef]
- Bechmann, R. Elastic and piezoelectric constants of alpha-quartz. Phys. Rev. 1958, 110, 1060–1061. [Google Scholar] [CrossRef]
- Feifei, C.; Lingfeng, K.; Wei, S.; Chao, J.; Shiwei, T.; Fapeng, Y.; Lifeng, Q.; Chunlei, W.; Xian, Z. The electromechanical features of LiNbO3 crystal for potential high temperature piezoelectric applications. J. Mater. 2019, 5, 73–80. [Google Scholar]
- Hauser, R.; Reindl, L.; Biniasch, J. High-Temperature Stability of LiNbO3 Based SAW Devices. In Proceedings of the IEEE Symposium on Ultrasonics, Honolulu, HI, USA, 5–8 October 2003; pp. 192–195. [Google Scholar]
- Fachberger, R.; Bruckner, G.; Knoll, G.; Hauser, R.; Biniasch, J.; Reindl, L. Applicability of LiNbO3, Langasite and GaPO4 in high temperature SAW sensors operating at radio frequencies. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2004, 51, 1427–1431. [Google Scholar] [CrossRef]
- Nosek, J.; Pustka, M. Determination of the electromechanical coupling factor of gallium orthophosphate (GaPO4) and its influence on resonance-frequency temperature dependencies. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2006, 53, 10–14. [Google Scholar] [CrossRef] [PubMed]
- Reiter, C.; Krempl, M.W.; Thanner, H.; Wallnöfer, W.; Worsch, P. Material properties of GaPO4 and their relevance for applications. Ann. Chim. Sci. Matériaux 2001, 26, 91–94. [Google Scholar] [CrossRef]
- Takeda, H.; Tanaka, S.; Izukawa, S.; Shimizu, H.; Nishida, T.; Shiosaki, T. Effective Substitution of Aluminum for Gallium in Langasite-Type Crystals for A Pressure Sensor Use at High Temperature. In Proceedings of the IEEE Ultrasonics Symposium, Rotterdam, The Netherlands, 18–24 September 2005; pp. 560–563. [Google Scholar]
- Zhihang, P.; Dongxu, Y.; Qiang, C.; Deqiong, X.; Dan, L.; Dingquan, X.; Jianguo, Z. Crystal structure, dielectric and piezoelectric properties of Ta/W codoped Bi3TiNbO9 Aurivillius phase ceramics. Curr. Appl. Phys. 2014, 14, 1861–1866. [Google Scholar]
- Wang, Q.; Wang, C.; Wang, J.; Zhang, S. High performance aurivillius-type bismuth titanate niobate (Bi3TiNbO9) piezoelectric ceramics for high temperature applications. Ceram. Int. 2016, 42. [Google Scholar] [CrossRef]
- Bekhtin, M.A.; Bush, A.A.; Kamentsev, K.E.; Segalla, A.G. Preparation and dielectric and piezoelectric properties of Bi3TiNbO9, Bi2CaNb2O9, and Bi2.5Na0.5Nb2O9 ceramics doped with various elements. Inorg. Mater. 2016, 52, 510–516. [Google Scholar] [CrossRef]
- Davis, M.J.; Vullo, P.; Kocher, M.; Hovhannisyan, M.; Letz, M. Piezoelectric glass-ceramic for high-temperature applications. J. Non. Cryst. Solids 2018, 501, 159–166. [Google Scholar] [CrossRef]
- Dupla, F.; Renoirt, M.-S.; Gonon, M.; Smagin, N.; Duquennoy, M.; Martic, G.; Erauw, J.-P. A lead-free non-ferroelectric piezoelectric glass-ceramic for high temperature surface acoustic wave devices. J. Eur. Ceram. Soc. 2020, 40, 3759–3765. [Google Scholar] [CrossRef]
- Gerace, K.S.; Mauro, J.C.; Randall, C.A. Piezoelectric glass-ceramics: Crystal chemistry, orientation mechanisms, and emerging applications. J. Am. Ceram. Soc. 2021, 104, 1915–1944. [Google Scholar] [CrossRef]
- Masai, H.; Tsuji, S.; Fujiwara, T.; Benino, Y.; Komatsu, T. Structure and non-linear optical properties of BaO-TiO2-SiO2 glass containing Ba2TiSi2O8 crystal. J. Non Cryst. Solids 2007, 353, 2258–2262. [Google Scholar] [CrossRef]
- Ochi, Y.; Meguro, T.; Kakegawa, K. Orientated crystallization of fresnoite glass-ceramics by using a thermal gradient. J. Eur. Ceram. Soc. 2006, 26, 627–630. [Google Scholar] [CrossRef]
- Keding, R.; Rüssel, C. Oriented glass-ceramic containing fresnoite prepared by electrochemical nucleation of a BaO-TiO2-SiO2-B2O3 melt. J. Non Cryst. Solids 2000, 278, 7–12. [Google Scholar] [CrossRef]
- Höche, T.; Keding, R.; Rüssel, C. Microstructural characterization of grain-oriented glass ceramics in the system Ba2TiSi2O8. J. Mater. Sci. 1999, 34, 195–208. [Google Scholar] [CrossRef]
- Ding, Y.; Masuda, N.; Miura, Y.; Osaka, A. Preparation of polar oriented Sr2TiSi2O8 films by surface crystallization of glass and second harmonic generation. J. Non Cryst. Solids 1996, 203, 88–95. [Google Scholar] [CrossRef]
- Patschger, M.; Wisniewski, W.; Rüssel, C. Piezoelectric glass-ceramics produced via oriented growth of Sr2TiSi2O8 fresnoite: Thermal annealing of surface modified quenched glasses. CrystEngComm 2012, 14, 7368–7373. [Google Scholar] [CrossRef]
- Maury, N.; Cambier, F.; Gonon, M. Bulk crystallisation of (00l) oriented fresnoite Sr2TiSi2O8 in glass-ceramics of the Sr–Ti–Si–K–B–O system. J. Non Cryst. Solids 2011, 357, 1079–1084. [Google Scholar] [CrossRef]
- Renoirt, M.-S.; Maury, N.; Dupla, F.; Gonon, M. Structure and Properties of Piezoelectric Strontium Fresnoite Glass-Ceramics Belonging to the Sr–Ti–Si–Al–K–O System. Ceramics 2019, 2, 86–97. [Google Scholar] [CrossRef] [Green Version]
- Tyagi, S.; Mahesh, V.G. SAW and interdigital transducers. IJSER 2012, 3, 1–4. [Google Scholar]
- Anghelescu, A.; Nedelcu, M. New Piezoelectric Materials for SAW Filters. In Proceedings of the SPIE, Advanced Topics in Optoelectronics, Microelectronics, and Nanotechnologies V, Constanta, Romania, 26–29 August 2010. [Google Scholar]
- Schiopu, P.; Cristea, I.; Grosu, N.; Craciun, A. Recent Developments in Surface Acoustic Wave Sensors. In Proceedings of the SPIE, Advanced Topics in Optoelectronics, Microelectronics, and Nanotechnologies IV, Constanta, Romania, 28–31 August 2008. [Google Scholar]
- Wisniewski, W.; Dimitrijevic, J.; Rüssel, C. Oriented nucleation and crystal growth of Sr-fresnoite (Sr2TiSi2O8) in 2SrO·TiO2·2SiO2 glasses with additional SiO2. Cryst. Eng. Comm. 2018, 20, 3234–3245. [Google Scholar] [CrossRef]
- Wisniewski, W.; Rüssel, C. Oriented surface nucleation in inorganic glasses—A review. Prog. Mater. Sci. 2021, 118, 100758. [Google Scholar] [CrossRef]
- Sagnard, M.; Laroche, T.; Ballandras, S. Surface Acoustic Waves Properties on Ba2TiSi2O8 for High Temperature Sensors. In Proceedings of the International Ultrasonic Symposium (IUS), Tours, France, 18–21 September 2016; pp. 3–6. [Google Scholar]
- Ito, I.; Nagatsuma, K.; Ashida, S. Surface acoustic wave characteristics of (Ba2−x Srx)TiSi2O8 crystals. Appl. Phys. Lett. 1980, 36, 894–895. [Google Scholar] [CrossRef]
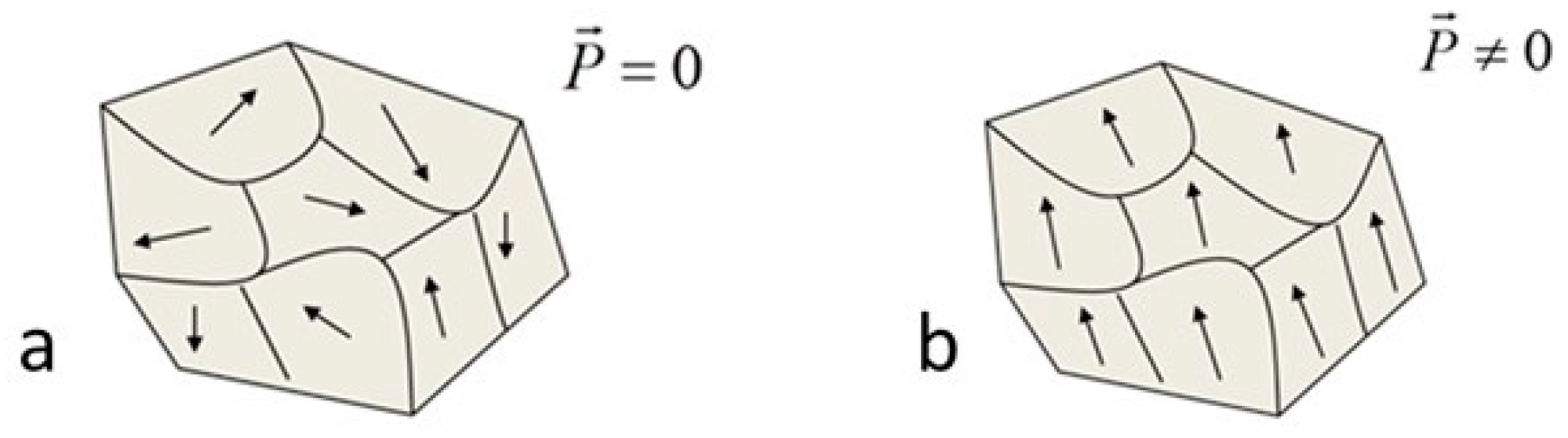









| Reference | SrO | BaO | TiO2 | SiO2 | K2O | Al2O3 |
|---|---|---|---|---|---|---|
| Ba(0) | 2.0 | 0.0 | 1 | 3.3 | 0.2 | 0.1 |
| Ba(10) | 1.8 | 0.2 | 1 | 3.3 | 0.2 | 0.1 |
| Ba(25) | 1.5 | 0.5 | 1 | 3.3 | 0.2 | 0.1 |
| Ba(50) | 1.0 | 1.0 | 1 | 3.3 | 0.2 | 0.1 |
| Composition | Parent Glass Density ±0.01 g/cm3 | Tg of Parent Glass ±2 °C | Glass–Ceramic Density ±0.01 g/cm3 | Tg of Glass–Ceramic ±2 °C |
|---|---|---|---|---|
| Ba(0) | 3.40 | 712 | 3.40 | 634 |
| Ba(10) | 3.47 | 702 | 3.48 | 632 |
| Ba(25) | 3.51 | 681 | 3.53 | 630 |
| Ba(50) | 3.62 | 640 | 3.64 | 632 |
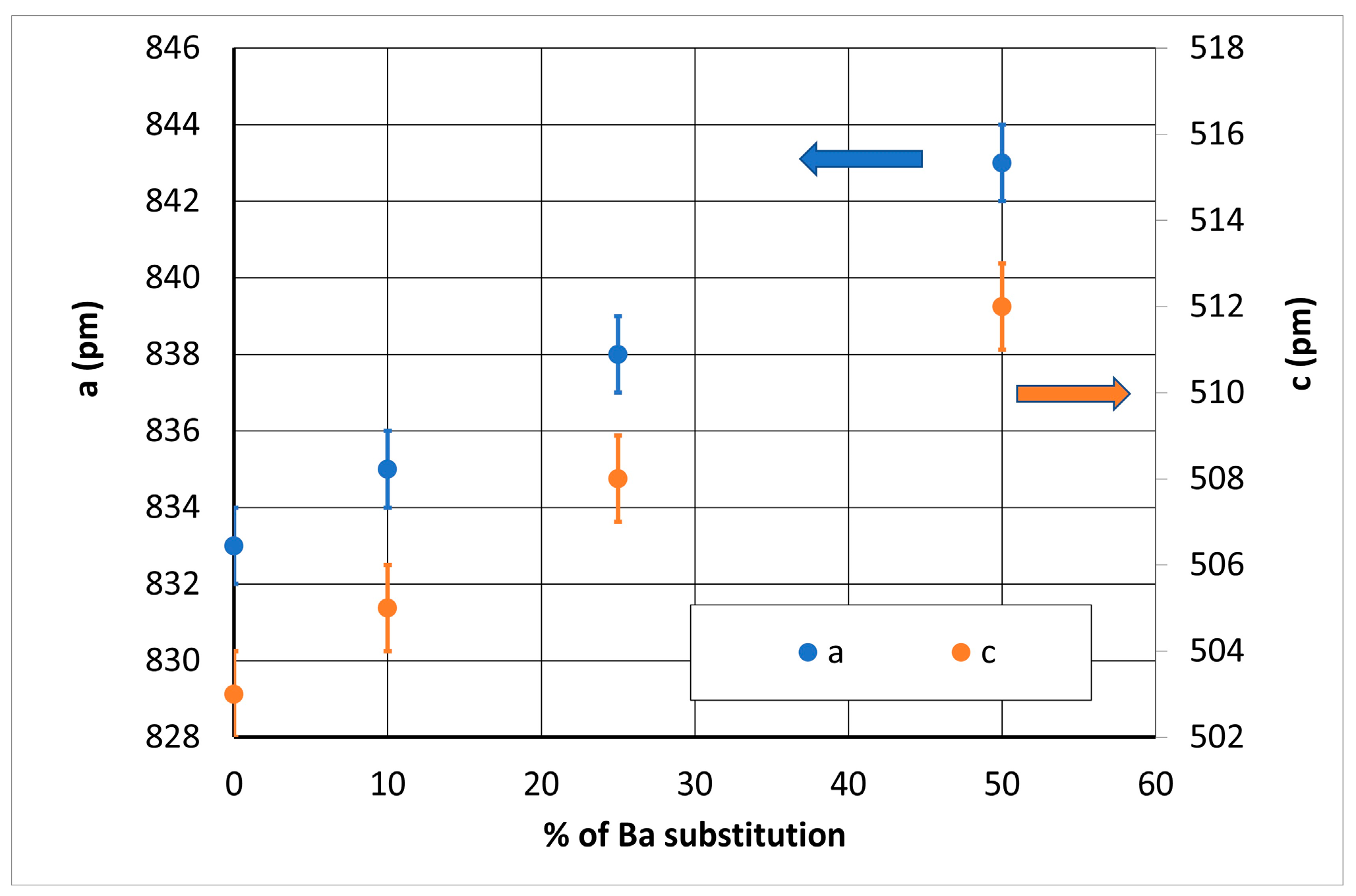
| Composition | A (pm) ± 1 | C (pm) ± 1 |
|---|---|---|
| Ba(0) | 833 | 503 |
| Ba(10) | 835 | 505 |
| Ba(25) | 838 | 508 |
| Ba(50) | 843 | 512 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gonon, M.; Dupla, F.; Alhousseini, H.; Duquennoy, M.; Smagin, N.; Martic, G. Effect of Partial Ba Substitutions on the Crystallization of Sr2TiSi2O8 (STS) Glass–Ceramics and on the Generation of a SAW Signal at High Temperatures. Materials 2021, 14, 4648. https://doi.org/10.3390/ma14164648
Gonon M, Dupla F, Alhousseini H, Duquennoy M, Smagin N, Martic G. Effect of Partial Ba Substitutions on the Crystallization of Sr2TiSi2O8 (STS) Glass–Ceramics and on the Generation of a SAW Signal at High Temperatures. Materials. 2021; 14(16):4648. https://doi.org/10.3390/ma14164648
Chicago/Turabian StyleGonon, Maurice, Florian Dupla, Hassan Alhousseini, Marc Duquennoy, Nikolay Smagin, and Grégory Martic. 2021. "Effect of Partial Ba Substitutions on the Crystallization of Sr2TiSi2O8 (STS) Glass–Ceramics and on the Generation of a SAW Signal at High Temperatures" Materials 14, no. 16: 4648. https://doi.org/10.3390/ma14164648
APA StyleGonon, M., Dupla, F., Alhousseini, H., Duquennoy, M., Smagin, N., & Martic, G. (2021). Effect of Partial Ba Substitutions on the Crystallization of Sr2TiSi2O8 (STS) Glass–Ceramics and on the Generation of a SAW Signal at High Temperatures. Materials, 14(16), 4648. https://doi.org/10.3390/ma14164648








