Lateral Formwork Pressure for Self-Compacting Concrete—A Review of Prediction Models and Monitoring Technologies
Abstract
:1. Introduction
2. Parameters Affecting the Lateral Formwork Pressure
2.1. Concrete Mix Design
2.2. Fresh Concrete Properties
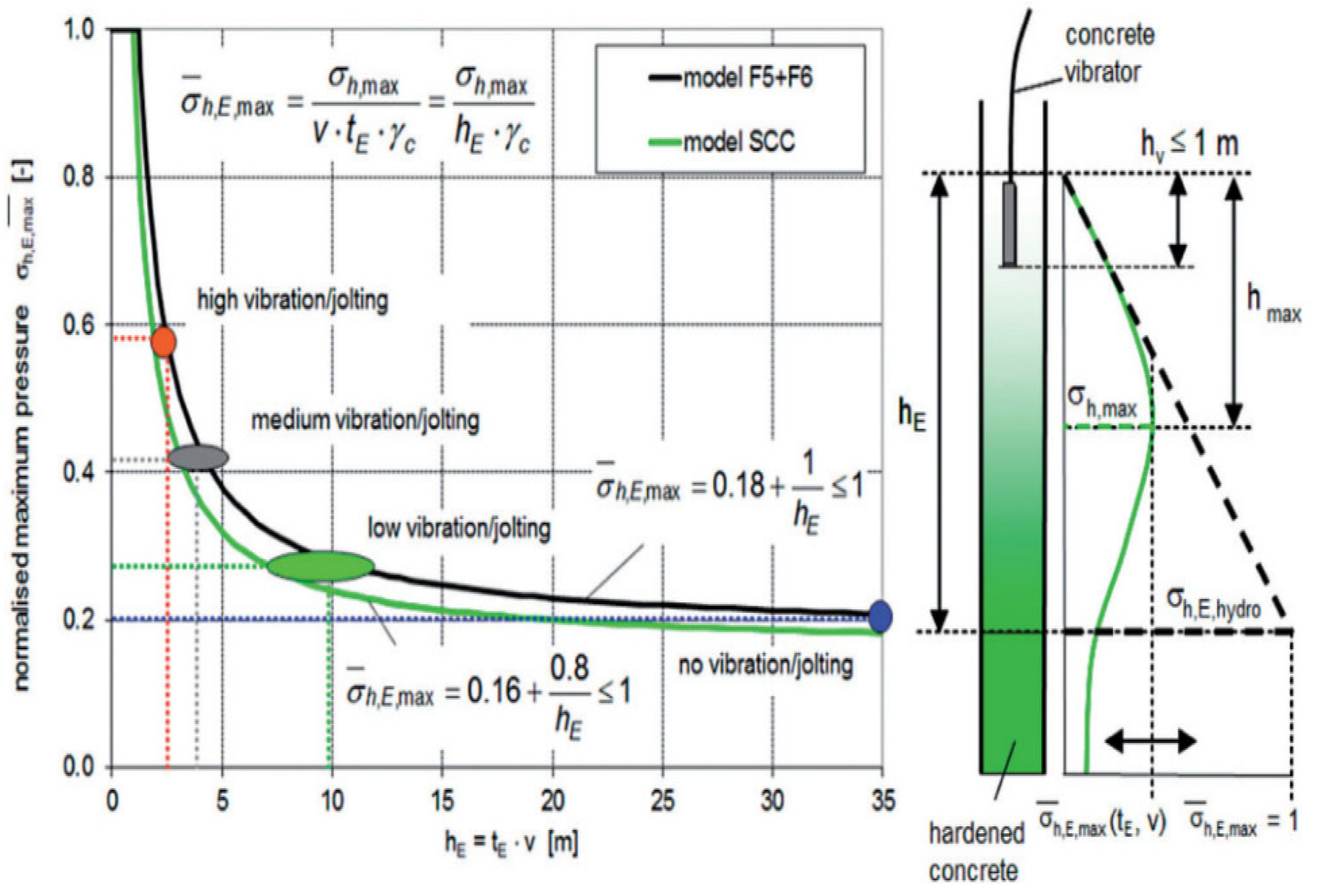
2.3. Placement Technology
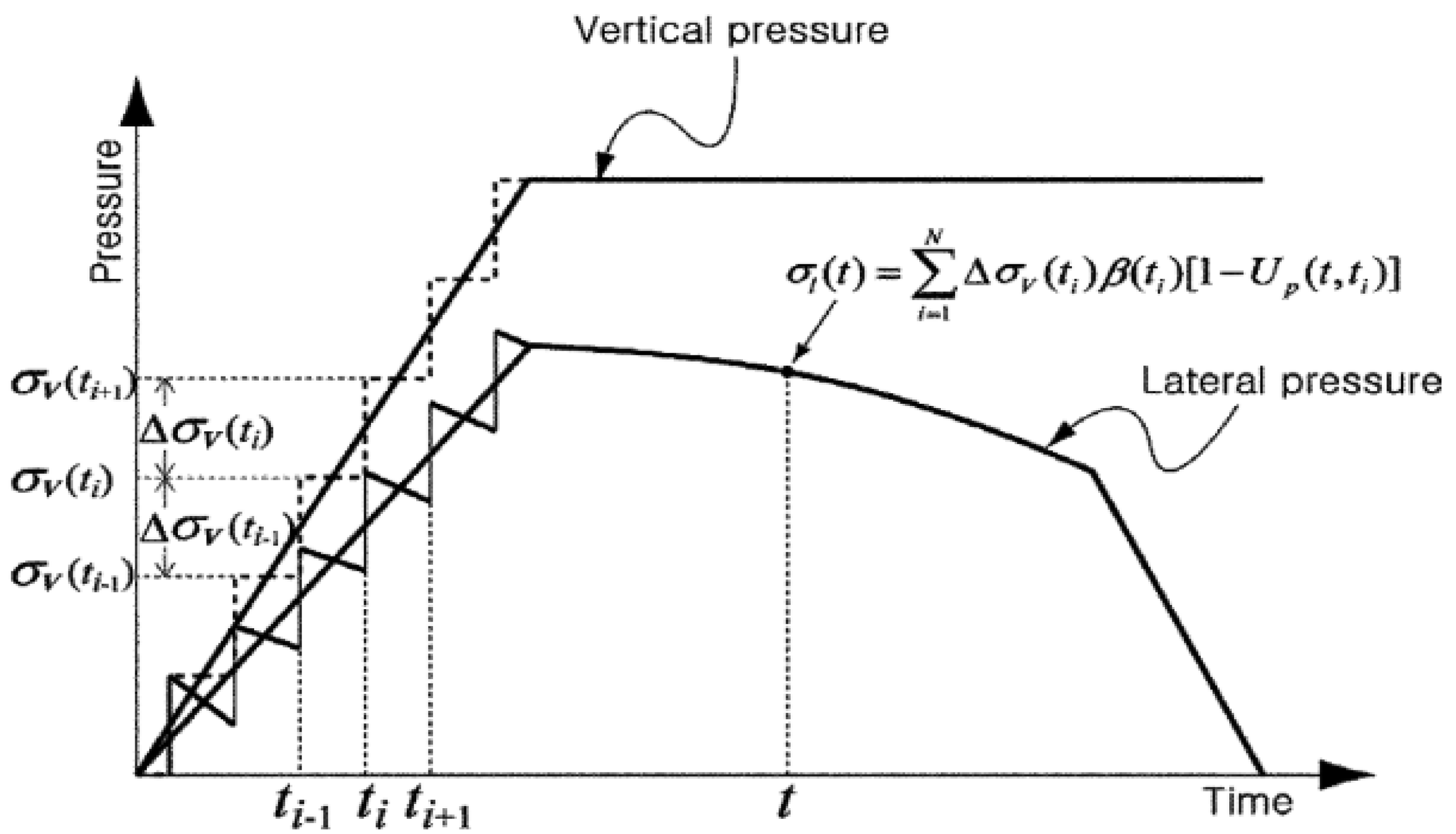
3. Modelling
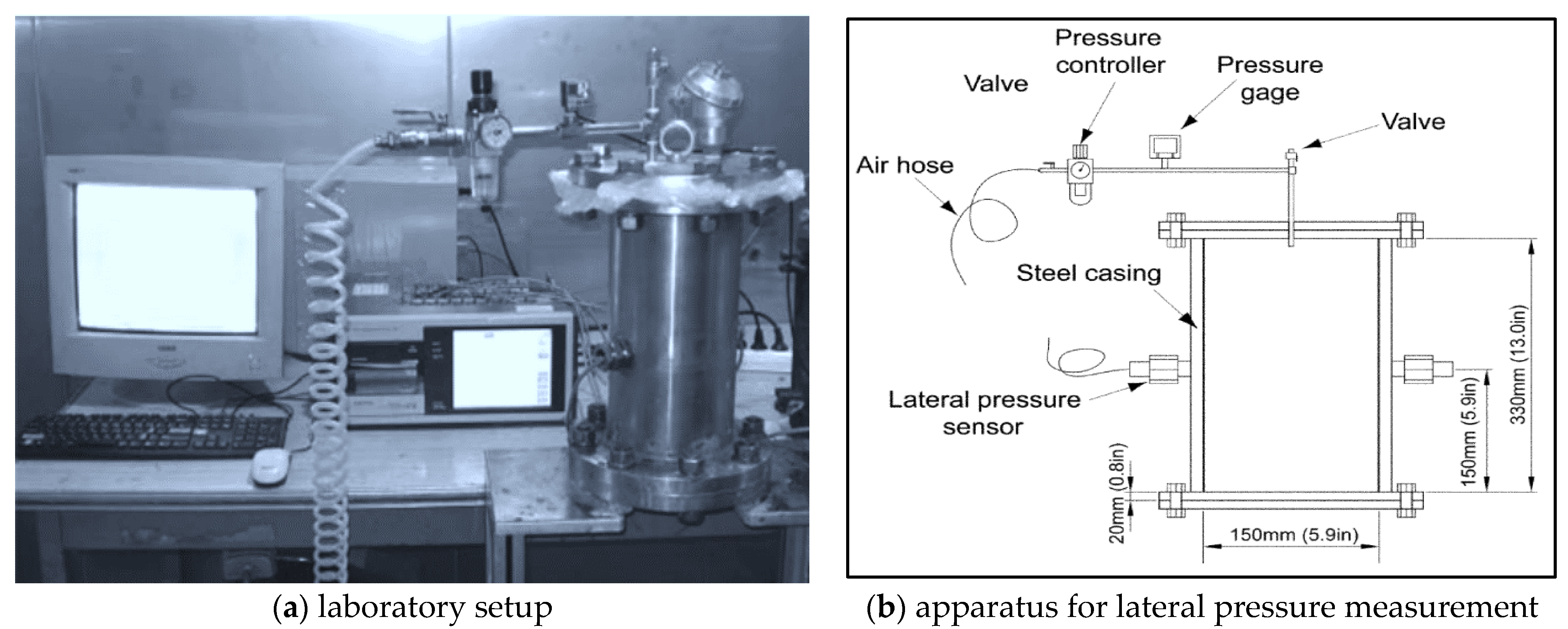
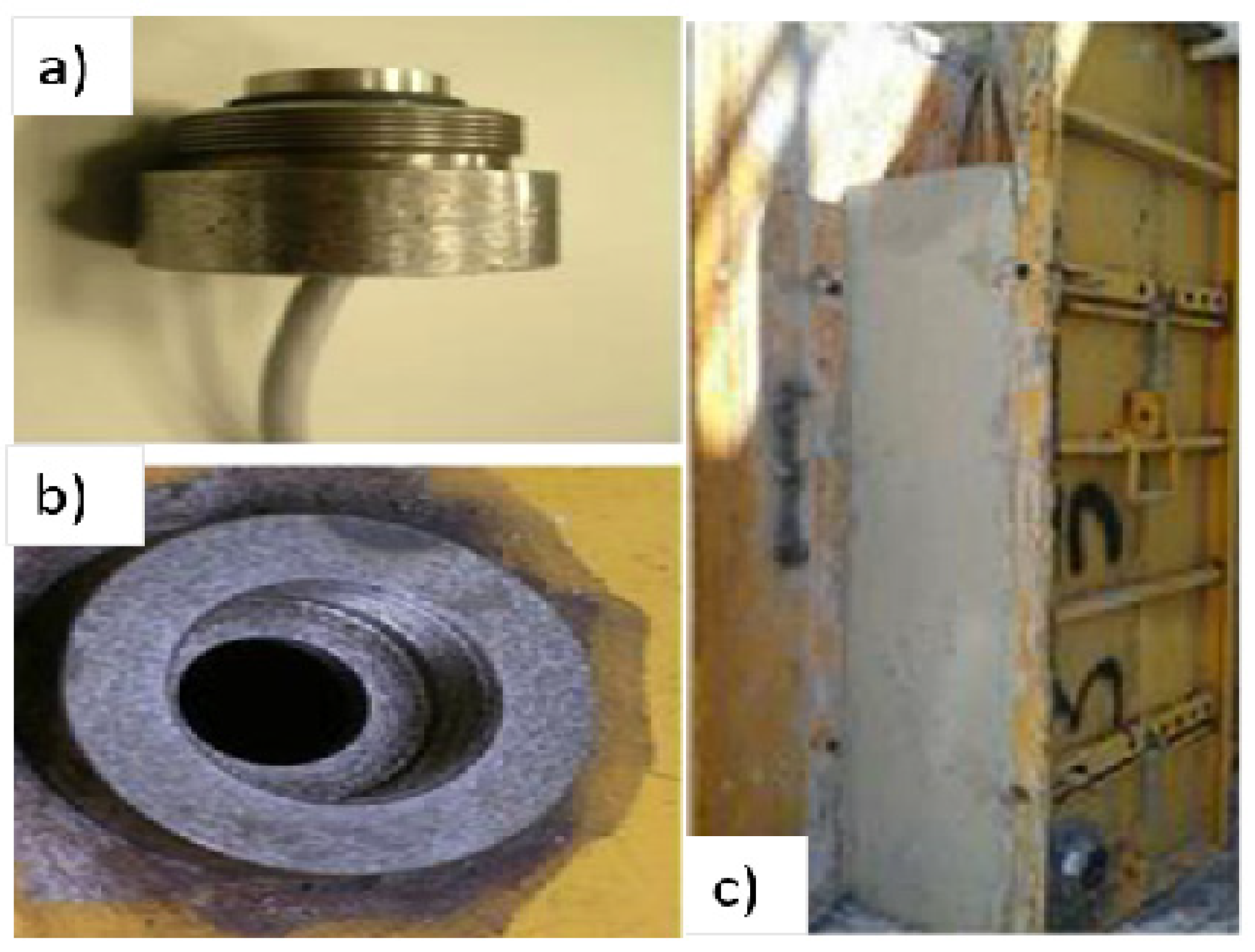
4. Formwork Pressure-Monitoring and Measurements
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Billberg, P. Understanding formwork pressure generated by fresh concrete. In Understanding the Rheology of Concrete; Woodhead Publishing: Sawston, UK, 2012; pp. 296–330. [Google Scholar]
- Khayat, K.H.; De Schutter, G. Mechanical Properties of Self-Compacting Concrete State-of-the-Art Report of the RILEM Technical Committee 228-MPS on Mechanical Properties of Self-Compacting Concrete; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Okamura, H.; Ouchi, M. Self-Compacting Concrete. J. Adv. Concr. Technol. 2003, 1, 5–15. [Google Scholar] [CrossRef]
- Ovarlez, G.; Roussel, N. A physical model for the prediction of lateral stress exerted by self-compacting concrete on formwork. Mater. Struct. Constr. 2006, 39, 269–279. [Google Scholar] [CrossRef]
- Billberg, P. Form Pressure Generated by Self-Compacting Concrete: Influence of Thixotropy and Structural Behavior at Rest. Ph.D. Thesis, Royal Institute of Technology, Stockholm, Sweden, 2006. [Google Scholar]
- Roussel, N. Rheology of fresh concrete: From measurements to predictions of casting processes. Mater. Struct. Constr. 2007, 40, 1001–1012. [Google Scholar] [CrossRef]
- Kim, J.H.; Beacraft, M.; Shah, S.P. Effect of mineral admixtures on formwork pressure of self-consolidating concrete. Cem. Concr. Compos. 2010, 32, 665–671. [Google Scholar] [CrossRef]
- Emanuelsson. Calculation Models for Calculating Lateral Pressures on Formwork when Using Self-Compacting Concrete. Master’s Thesis, Luleå Tekniska Universitet, Luleå, Sweden, 2018. [Google Scholar]
- Hurd, M.K. Lateral Pressures for Formwork Design. Concr. Int. 2007, 29, 31–33. [Google Scholar]
- Sagadevan, R.; Rao, B.N. Experimental and analytical investigation of structural performance of vertical concrete formworks. Asian J. Civ. Eng. 2020, 21, 2. [Google Scholar] [CrossRef]
- Benaicha, M.; Belcaid, A.; Alaoui, A.H.; Jalbaud, O.; Burtschell, Y. Evolution of pressure generated by self-compacting concrete on a vertical channel. Eng. Struct. 2019, 191, 432–438. [Google Scholar] [CrossRef]
- Khayat, K.H.; Omran, A.F. State-of-The Art Review of Form Pressure Exerted by Self-Consolidating Concrete; Université de Sherbrooke: Sherbrooke, QC, Canada, 2009. [Google Scholar]
- Khayat, K.H.; Assaad, J. Measurement systems for determining formwork pressure of highly-flowable concrete. Mater. Struct. Constr. 2008, 41, 37–46. [Google Scholar] [CrossRef]
- Omran, F.; Khayat, K.H. Choice of thixotropic index to evaluate formwork pressure characteristics of self-consolidating concrete. Cem. Concr. Res. 2014, 63, 89–97. [Google Scholar] [CrossRef]
- Gardner, N.J. Pressure of concrete on formwork A review. ACI J. 1986, 82, 744–753. [Google Scholar]
- Puente, I.; Santilli, A.; Lopez, A. Lateral pressure over formwork on large dimension concrete blocks. Eng. Struct. 2010, 32, 195–206. [Google Scholar] [CrossRef]
- Drewniok, M.P.; Cygan, G.; Gołaszewski, J. Influence of the Rheological Properties of SCC on the Formwork Pressure. Procedia Eng. 2017, 192, 124–129. [Google Scholar] [CrossRef]
- Kurakova, O. Use of formwork systems in high-rise construction. E3S Web Conf. 2018, 33, 7. [Google Scholar] [CrossRef] [Green Version]
- Perrot, A.; Pierre, S.; Picandet, V. Prediction of lateral form pressure exerted by concrete at low casting rates. Mater. Struct. Constr. 2015, 48, 2315–2322. [Google Scholar] [CrossRef]
- EFNARC. The European Guidelines for Self-Compacting Concrete: Specification. The European Guidelines for Self-Compacting Concrete: Specification, Production and Use; EFNARC: Farnham, UK, 2005. [Google Scholar]
- Gregori, A.; Ferron, R.P.; Sun, Z.; Shah, S.P. Experimental simulation of self-consolidating concrete formwork pressure. ACI Mater. J. 2008, 105, 97–105. [Google Scholar]
- Proske, T.; Khayat, K.H.; Omran, A.; Leitzbach, O. Form pressure generated by fresh concrete: A review about practice in formwork design. Mater. Struct. Constr. 2014, 47, 1099–1113. [Google Scholar] [CrossRef]
- Gardner, N.J.; Keller, J.; Quattrociocchi, R.; Charitou, G. Field Investigation of Formwork Pressures Using Self-Consolidating Concrete. Concr. Int. 2020, 34, 41–47. [Google Scholar]
- Assaad, J.J.; Khayat, K.H. Effect of casting rate and concrete temperature on formwork pressure of self-consolidating concrete. Mater. Struct. 2006, 39, 333–341. [Google Scholar] [CrossRef]
- Assaad, J.J.; Harb, J. Formwork pressure of self-consolidating concrete containing recycled coarse aggregates. ACI Struct. J. 2017, 114, 491–500. [Google Scholar] [CrossRef]
- Khayat, K.H.; Omran, A.F.; Elaguab, Y.M. Effect of SCC mixture composition on thixotropy and formwork pressure. J. Mater. Civ. Eng. 2012, 24, 876–888. [Google Scholar]
- Ghoddousi, P.; Shirzadi Javid, A.A.; Ghodrati Amiri, G.; Donyadideh, K. Predicting the Formwork Lateral Pressure of Self-consolidating Concrete Based on Experimental Thixotropy Values. Int. J. Civ. Eng. 2019, 17, 1131–1144. [Google Scholar] [CrossRef]
- Tuyan, M.; Saleh Ahari, R.; Erdem, T.K.; Andiç Çakır, Ö.; Ramyar, K. Influence of thixotropy determined by different test methods on formwork pressure of self-consolidating concrete. Constr. Build. Mater. 2018, 173, 189–200. [Google Scholar] [CrossRef]
- Khayat, K.H.; Assaad, J.J. Effect of w/cm and high-range water-reducing admixture on formwork pressure and thixotropy of self-consolidating concrete. ACI Mater. J. 2006, 103, 186. [Google Scholar]
- Assaad, J.; Khayat, K.H.; Mesbah, H. Variation of formwork pressure with thixotropy of self-consolidating concrete. ACI Mater. J. 2003, 100, 29–37. [Google Scholar]
- Jiří Němeček, P.T.; Vojtěch, Z. Reduction of Formwork Pressures in Self-Compacting Concrete. In Proceedings of the IRF2020: 7th International Conference Integrity-Reliability-Failure, Funchal, Portugal, 6–10 September 2020; pp. 545–546. [Google Scholar]
- Kim, J.J.H.; Noemi, N.; Shah, S.P. Effect of powder materials on the rheology and formwork pressure of self-consolidating concrete. Cem. Concr. Compos. J. 2012, 34, 746–753. [Google Scholar] [CrossRef]
- Saleem, N.S.; Baluch, M.H.; Rahman, M.K.; Al-Osta, M. Experimental Investigations and a New Numerical Model for Evolution of Formwork Pressure in SCC. Arab. J. Sci. Eng. 2017, 42, 3907–3921. [Google Scholar] [CrossRef]
- Kwon, S.H.; Phung, Q.T.; Park, H.Y.; Kim, J.H.; Shah, S.P. Experimental study on effect of wall friction on formwork pressure of self-consolidating concrete. In Proceedings of the 6th International RILEM Symposium on Self-Compacting Concrete and 4th North American Conference on the Design and Use of SCC, Washington DC, USA, 26 September 2010; pp. 26–29. [Google Scholar]
- Wu, W.; Li, X. Lateral Stress Characteristics of Steel Structure Wall Module Exerted by Self-Compacting Concrete. Iran. J. Sci. Technol. Trans. Civ. Eng. 2020, 44, 79–89. [Google Scholar] [CrossRef]
- Cygan, G.; Golaszewski, J.; Drewniok, M.P. Influence on Type of Cement on the SCC Formwork Pressure during and after Casting. IOP Conf. Ser. Mater. Sci. Eng. 2019, 471, 112025. [Google Scholar] [CrossRef]
- Feys, D.; De Schutter, G.; Verhoeven, R. Parameters influencing pressure during pumping of self-compacting concrete. Mater. Struct. Constr. 2013, 46, 533–555. [Google Scholar] [CrossRef]
- Leemann, A.; Winnefeld, F. Pressure of Self-Consolidating Concrete on Formwork. Concr. Int. 2006, 28, 27–31. [Google Scholar]
- Santilli, A.; Puente, I.; Tanco, M. A factorial design study to determine the significant parameters of fresh concrete lateral pressure and initial rate of pressure decay. Constr. Build. Mater. 2011, 25, 1946–1955. [Google Scholar] [CrossRef]
- Santilli, A.; Puente, I. An empirical model to predict fresh concrete lateral pressure. Constr. Build. Mater. 2013, 47, 379–388. [Google Scholar] [CrossRef]
- Glinicki, M.A.; Gołaszewski, J.; Cygan, G. Formwork Pressure of a Heavyweight Self-Compacting Concrete Mix. Materials 2021, 14, 6. [Google Scholar] [CrossRef]
- Graubner, C.A.; Boska, E.; Motzko, C.; Proske, T.; Dehn, F. Formwork pressure induced by highly flowable concretes—Design approach and transfer into practice. Struct. Concr. 2012, 13, 51–60. [Google Scholar] [CrossRef]
- Esmaeilkhanian, B.; Khayat, K.H.; Yahia, A.; Feys, D. Effects of mix design parameters and rheological properties on dynamic stability of self-consolidating concrete. Cem. Concr. Compos. 2014, 54, 21–28. [Google Scholar] [CrossRef]
- Assaad, J.J.; Khayat, K.H. Effect of viscosity-enhancing admixtures on formwork pressure and thixotropy of self-consolidating concrete. ACI Mater. J. 2006, 103, 280. [Google Scholar]
- Santilli, A.; Teixeira, S.; Puente, I. Influence of temperature and concrete reinforcement on vertical formwork design. Constr. Build. Mater. 2015, 88, 188–195. [Google Scholar] [CrossRef]
- Kwon, S.H.; Shah, S.P.; Phung, T.Q.; Kim, J.H.; Lee, Y. Intrinsic model to predict formwork pressure. ACI Mater. J. 2010, 1, 20–26. [Google Scholar]
- Assaad, J.J. Correlating Thixotropy of Self-Consolidating Concrete to Stability, Formwork Pressure, and Multilayer Casting. J. Mater. Civ. Eng. 2016, 28, 04016107. [Google Scholar] [CrossRef]
- Song, H.; Cui, W.; Wei-Shuo, Y. Analysis of tangential stress between fresh self-compacting concrete and wall based on thixotropy theory. Constr. Build. Mater. 2019, 196, 126–133. [Google Scholar] [CrossRef]
- Lomboy, G.R.; Wang, X.; Wang, K. Rheological behavior and formwork pressure of SCC, SFSCC, and NC mixtures. Cem. Concr. Compos. 2014, 54, 110–116. [Google Scholar] [CrossRef]
- Tichko, S.; De Schutter, G.; Troch, P.; Vierendeels, J.; Verhoeven, R.; Lesage, K.; Cauberg, N. Influence of the viscosity of self-compacting concrete and the presence of rebars on the formwork pressure while filling bottom-up. Eng. Struct. 2015, 101, 698–714. [Google Scholar] [CrossRef]
- Lange, B.; Birch, J.; Henchen, Y.-S.; Liu, F.; Struble, L. Modeling formwork pressure of SCC. In Proceedings of the 3rd North America Conference Design Use Self-Consolidating Concrete, Chicago, IL, USA, 10–12 November 2008. [Google Scholar]
- Teixeira, S.; Santilli, A.; Puente, I. Analysis of casting rate for the validation of models developed to predict the maximum lateral pressure exerted by self-compacting concrete on vertical formwork. J. Build. Eng. 2016, 6, 215–224. [Google Scholar] [CrossRef]
- Tejeda-Dominguez, F. Laboratory and Field Study of Self-Consolidating Concrete (SCC) Formwork Pressure; University of Illinois at Urbana-Champaign: Champaign, IL, USA, 2005. [Google Scholar]
- Henschen, L. Formwork Pressure Model for Self-Consolidating Concrete Using Pressure Decay Signature. ACI Mater. J. 2018, 115, 339–348. [Google Scholar] [CrossRef]
- Shakor, P.; Gowripalan, N. Pressure exerted on formwork and early age shrinkage of self- technical papers pressure exerted on formwork and early age shrinkage of self-compacting concrete. Concr. Aust. 2020, 46, 30–34. [Google Scholar]
- Matar, P.; Assaad, J.J. Effect of vertical reinforcing bars on formwork pressure of SCC containing recycled aggregates. J. Build. Eng. 2017, 13, 159–168. [Google Scholar] [CrossRef]
- Perrot, S.; Amziane, G.; Ovarlez, G.; Roussel, N. SCC formwork pressure: Influence of steel rebars. Cem. Concr. Res. 2009, 39, 524–528. [Google Scholar] [CrossRef]
- Labuschagne, J. Formwork Pressures by Self-Compacting Concrete: A Practical Perspective. Master’s Thesis, Stellenbosch University, Stellenbosch Central, South Africa, 2018. [Google Scholar]
- McCarthy, M.J.; Dhir, R.K.; Caliskan, S.; Kashif, A. Influence of self-compacting concrete on the lateral pressure on formwork. Proc. Inst. Civ. Eng. Struct. Build. 2012, 165, 127–138. [Google Scholar] [CrossRef]
- Kwon, S.H.; Phung, Q.T.; Park, H.Y.; Kim, J.H.; Shah, S.P. Effect of wall friction on variation of formwork pressure over time in self-consolidating concrete. Cem. Concr. Res. 2011, 41, 90–101. [Google Scholar] [CrossRef]
- Tichko, S.; Van De Maele, J.; Vanmassenhove, N.; De Schutter, G.; Vierendeels, J.; Verhoeven, R.; Troch, P. Numerical simulation of formwork pressure while pumping self-compacting concrete bottom-up. Eng. Struct. 2014, 70, 218–233. [Google Scholar] [CrossRef]
- Omran, Y.M.; Elaguab, Y.M.; Khayat, K.H. Effect of placement characteristics on SCC lateral pressure variations. Constr. Build. Mater. 2014, 66, 507–514. [Google Scholar] [CrossRef]
- Arslan, M.; Şimşek, O.; Subaşi, S. Effects of formwork surface materials on concrete lateral pressure. Constr. Build. Mater. 2005, 19, 319–325. [Google Scholar] [CrossRef]
- Brameshuber, W.; Beitzel, H.; Beitzel, M.; Bohnemann, C.; Boska, E.; Dehn, F.; Graubner, C.A.; König, A.; Motzko, C.; Müller, H.S.; et al. Formwork pressure induced by highly flowable concretes-material investigations and large-scale tests. Struct. Concr. 2011, 12, 270–280. [Google Scholar] [CrossRef]
- Nemati, S.; Samali, B.; Sanati, F.; Aliabadizadeh, Y.; Yaghmaei, F. A creative validation method for Self-Compacting Concrete (SCC) lateral pressure model using Archimedes’ Law. Int. J. Geomate 2019, 17, 41–48. [Google Scholar] [CrossRef]
- Omran, F.; Khayat, K.H. Effect of Formwork Characteristics on SCC Lateral Pressure. J. Mater. Civ. Eng. 2017, 29, 04016293. [Google Scholar] [CrossRef]
- Tchamba, J.C.; Amziane, S.; Ovarlez, G.; Roussel, N. Lateral stress exerted by fresh cement paste on formwork: Laboratory experiments. Cem. Concr. Res. 2008, 38, 459–466. [Google Scholar] [CrossRef]
- Khayat, K.H.; Omran, A.F. Evaluation of SCC formwork pressure. Concr. Int. 2010, 32, 30–34. [Google Scholar]
- Gardner, N.J. Pressure of Concrete Against Formwork. J. Am. Concr. Inst. 1980, 77, 279–286. [Google Scholar]
- Billberg, P.H.; Roussel, N.; Amziane, S.; Beitzel, M.; Charitou, G.; Freund, B.; Gardner, J.N.; Grampeix, G.; Graubner, C.A.; Keller, L.; et al. Field validation of models for predicting lateral form pressure exerted by SCC. Cem. Concr. Compos. 2014, 54, 70–79. [Google Scholar] [CrossRef]
- Park, K.; Kim, J.H.; Han, S.H. A pore water pressure diffusion model to predict formwork pressure exerted by freshly mixed concrete. Cem. Concr. Compos. 2017, 75, 1–9. [Google Scholar] [CrossRef]
- ACI (American Concrete Institute). ACI 347R-14. In Guide to Formwork for Concrete; ACI: Farmington Hill, MI, USA, 2014. [Google Scholar]
- Proske, T.; Graubner, C.A. Formwork Pressure of Highly Workable Concrete—Experiments Focused on Setting, Vibration and Design Approach. In Design, Production and Placement of Self-Consolidating Concrete, 1; Springer: Dordrecht, The Netherland, 2010; pp. 255–267. [Google Scholar]
- Kwon, S.H.; Kim, J.H.; Shah, S.P. Development, and applications of the intrinsic model for formwork pressure of self-consolidating concrete. Int. J. Concr. Struct. Mater. 2012, 6, 31–40. [Google Scholar] [CrossRef] [Green Version]
- DIN 18218. DIN Standard on Formwork Pressures Updated; American Concrete Institute: Farmington Hills, MI, USA, 2010; pp. 27–29. [Google Scholar]
- Teixeira, S.; Puente, I.; Santilli, A. Statistical model for predicting the maximum lateral pressure exerted by self-consolidating concrete on vertical formwork. J. Build. Eng. 2017, 12, 77–86. [Google Scholar] [CrossRef]
- Assaad, J.J.; Khayat, K.H. Kinetics of formwork pressure drop of self-consolidating concrete containing various types and contents of binder. Cem. Concr. Res. 2005, 35, 1522–1530. [Google Scholar] [CrossRef]
- Tejeda-Dominguez, F.; Lange, D.A.; D’Ambrosia, M.D. Formwork pressure of self-consolidating concrete in tall wall field applications. Transp. Res. Rec. 2005, 1914, 1–7. [Google Scholar] [CrossRef]
- Assaad, J.J. Matar: Regression models to predict SCC pressure exerted on formworks containing vertical and transverse reinforcing bars. Mater. Struct. Constr. 2018, 51, 3. [Google Scholar] [CrossRef]
- McCarthy, R.; Silfwerbrand, J. Comparison of Three Methods to Measure Formwork Pressure When Using SCC. Concr. Int. 2011, 33, 27–32. [Google Scholar]
- Omran, F.; Khayat, K.H. Portable pressure device to evaluate lateral formwork pressure exerted by fresh concrete. J. Mater. Civ. Eng. 2013, 25, 731–740. [Google Scholar] [CrossRef]




| Category | Parameters | References |
|---|---|---|
| Concrete Mix design | Gradation, shape, texture, and amount of fine and coarse aggregate | [1,12,24,25,26] |
| Water to cement ratio | [21,24,27,28,29] | |
| Amount and type of SCMs, Amount and type of chemical admixtures | [1,12,24,30,31,32,33] | |
| Cement type and amount | [12,27,32,34,35,36,37,38,39] | |
| Fresh concrete properties | Concrete temperature | [14,23,24,38,39,40] |
| Setting time (rate of hardening) | [12,32,41] | |
| Concrete density | [1,41] | |
| The initial low shear stress | [17,24,36] | |
| Slump flow and T50 (consistency class) | [17,37,42,43,44] | |
| Thixotropy and viscosity | [12,36,42,43,44,45,46,47,48] | |
| Placement technology | Casting rate and casting method | [13,23,24,36,48,49,50,51] |
| Humidity and ambient temperature | [52,53] | |
| Reinforcement | [12,39,47,54,55] | |
| Pumping location | [37,47,55,56,57,58] | |
| Size of the structure, casting height | [12,36,59] | |
| Type of formwork and its geometry (including stiffness, surface friction, surface roughness, use of demoulding agents, weight) | [12,32,46,60,61,62,63,64,65] | |
| External stresses imposed by workers, equipment and materials, possible external loads created, e.g., by wind, pressure sensor location and mounting direction of the sensors | [12,60] |
| Casting Rate m/h | Approximate Max Recorded Pressure (kPa) | Associated Hydrostatic Pressure (kPa) | Height (m) | Reference |
|---|---|---|---|---|
| 19 | 97 | 93 | 0.8 | [37] |
| 10 | 180 | 290 | 12.5 | [67] |
| 7 | 24.01 | 28.5 | 1.2 | [17] |
| 6 | 101 | 155.3 | 6.6 | [68] |
| 3.5 | 23 | 25 | 2.6 | [70] |
| 2.74 | 33.78 | 24.54 | 1.10 | [51] |
| Model Reference | Variables Included | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Casting Rate | Concrete Temperature | Density | Formwork Geometry | Setting Time | Casting Height | Yield Stress | Maximum Size Aggregate | Slump Flow | Reinforcing Bar | |
| Proske and Graubner [73] | X | X | X | |||||||
| Kwon et al. [46] | X | X | ||||||||
| DIN 18,218 [75] | X | X | X | X | X | |||||
| Khayat and Omran [68] | X | X | X | X | X | X | X | |||
| Graubner et al. [42] | X | X | X | X | ||||||
| Gardner et al. [23] | X | X | X | |||||||
| Teixeira et al. [76] | X | X | X | X | X | X | ||||
| Assaad and Matar [56] | X | X | ||||||||
| Ovarlez and Roussel [4] | X | X | X | X | X | |||||
| Main Study Parameters | Key Findings | Reference(s) |
|---|---|---|
| Casting method, workability, and mix proportions. | 1. Casting method influences the pressure amount and casting from the bottom generates high pressure at the bottom than casting from the top. 2. High slump flow affects lateral pressure. 3. Mix design influence the setting time having a subsequent influence on the pressure. | [37] |
| Formwork pressure, mixture proportions, height, casting rate. | A high casting rate induces high lateral pressure. | [34,46,60,74] |
| Pore water pressure, lateral pressure, time. | Form pressure diverges from hydrostatic pressure due to the thixotropic property of concrete. | [13] |
| Addition of mineral admixture and monitor the pressure change. | Mineral admixture such as processed clays lessens the lateral pressure. | [7] |
| Casting height, casting rate, the temperature of concrete and static and dynamic yield stress. | Lateral pressure exerted by SCC is less than the hydrostatic pressure. | [77] |
| Mix proportions, strain, formwork pressure, tie tension force. | Good correlation between lateral pressure and form deformation (strain) and a good correlation between the tie tension force and the pressure. | [70] |
| Slump flow and method of placement. | Pressure varies depending on the class consistency (slump flow) and method of placement. | [41] |
| Casting rate and slump flow. | Lateral form pressure depends on the performance of the admixture and placement rate. | [23] |
| Casting rate, slump loss, pressure, Wall geometries. | A notable correlation between casting rate, slump flow and the pressure were found in the study. | [68] |
| Casting rate. | A high casting rate leads to high pressure. | [19] |
| Viscosity, reinforcing rebar, casting location. | Pumping concrete from the bottom generates higher lateral pressure than from the top. | [46] |
| Recycled aggregate, Vertical reinforcement bars. | The finding indicated that using recycled aggregate reduces the initial pressure due to high surface roughness which increases the internal friction. | [52] |
| Pressure Measurement Tools | Formwork Type | Type of Structure\Dimensions | Reference |
|---|---|---|---|
| Mounted pressure sensor | Steel | Wall structure of dimensions 0.2 m × 0.75 m × 2.7 m & 0.25 m × 4.9 m × 4.7 m & 0.20 × 0.20 × 0.975 m | [37] |
| Strain gauge- based pressure sensors | Steel | Three walls and one column | [46,74] |
| Flush Diaphragm Millivolt Output Type pressure sensors | Steel | Retaining walls | [13] |
| Pressure transducers | Steel | Lab setup | [49] |
| Pressure sensors with 19 mm diameter and electronic transducers with 0–1380 kPa range and 0.25% accuracy | PVC | Lab setup using PVC with a diameter of 200 mm and a height of 700 mm. | [67] |
| Honeywell ABH100PSC1B pressure sensors rated for 0 to 689 kPa. | Thick plywood panels mounted on steel frames | Security Wall 0.27 m thick, 6 m tall, and 400 m long wall | [70] |
| Pressure transducers Omega PX43E0-100GI and load cells attached to tie-bars and pressure transducers installed in the inner surface of fresh concrete | Steel | Used 8 different walls dimensions Walls Nos. 1, 3, 5 and 7 are 6.6 m in height, 2.4 m in length, and 0.2 m in thickness. Walls Nos. 2, 4 and 6 differ only by height = 4.2 m. Wall no 8 is 4.2 m in height but had a thickness of 0.4 m | [68] |
| Pressure transducer | Steel | Column 2 m height | [19] |
| Pressure sensors with a diameter of 87 mm placed at 0.135 m, 0.375 m and 0.75 m from the bottom | N\A | Column (0.2 × 0.2 × 1.2) m | [17] |
| Linear variable differential transformers and high-precision digital micrometre strain gages | A Plexiglas acrylic | Lab setup rectangular sample 1600 mm height, 400 mm length, and 200 mm width | [79] |
| Flush diaphragm pressure sensors | Transparent plastic | Lab setup with square column dimension 16 × 16 × 70 cm | [11] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gamil, Y.; Nilimaa, J.; Emborg, M.; Cwirzen, A. Lateral Formwork Pressure for Self-Compacting Concrete—A Review of Prediction Models and Monitoring Technologies. Materials 2021, 14, 4767. https://doi.org/10.3390/ma14164767
Gamil Y, Nilimaa J, Emborg M, Cwirzen A. Lateral Formwork Pressure for Self-Compacting Concrete—A Review of Prediction Models and Monitoring Technologies. Materials. 2021; 14(16):4767. https://doi.org/10.3390/ma14164767
Chicago/Turabian StyleGamil, Yaser, Jonny Nilimaa, Mats Emborg, and Andrzej Cwirzen. 2021. "Lateral Formwork Pressure for Self-Compacting Concrete—A Review of Prediction Models and Monitoring Technologies" Materials 14, no. 16: 4767. https://doi.org/10.3390/ma14164767
APA StyleGamil, Y., Nilimaa, J., Emborg, M., & Cwirzen, A. (2021). Lateral Formwork Pressure for Self-Compacting Concrete—A Review of Prediction Models and Monitoring Technologies. Materials, 14(16), 4767. https://doi.org/10.3390/ma14164767








