Porosity of Calcium Silicate Hydrates Synthesized from Natural Rocks
Abstract
:1. Introduction
2. Materials and Methods
2.1. Characterization of the Raw Materials
- Silica-calcite sedimentary rock opoka (Stoniskis-Zemaitkiemis quarry, Silute, Lithuania). The chemical and mineralogical composition of opoka is given in Table 1 and Table 2, respectively. It was obtained that opoka mainly consists of four different modifications of SiO2: quartz, cristobalite, and tridymite (in total 35 wt%) and amorphous SiO2 (19.6 wt%). Since opoka contains calcite, it was calcined at 775 °C for 1 h and later milled in a ball mill to reach Sa ≈ 970 m2/kg [34]. The amount of CaOfree from total CaO was equal to 50.67%. It was obtained that crystalline modifications of SiO2 remained stable during calcination, while the amorphous part of SiO2 reacted with calcium oxide and as a result wollastonite and larnite were formed.
- Limestone (Karpenai quarry, Naujoji Akmene, Lithuania) consists of 93.42% calcite, 3.23% dolomite, 2.61% quartz and 0.74% other impurities. It was additionally calcined at 900 °C for 1 h in a laboratory kiln Nabertherm LV 15/11/P330 (Lilienthal, Germany) and later milled until Sa ≈ 650 m2/kg. CaOfree = 91.2%.
2.2. Experimental Methods
2.2.1. Hydrothermal Synthesis
2.2.2. Instrumental Analysis
2.2.3. Determination of Pore Characteristics
3. Results
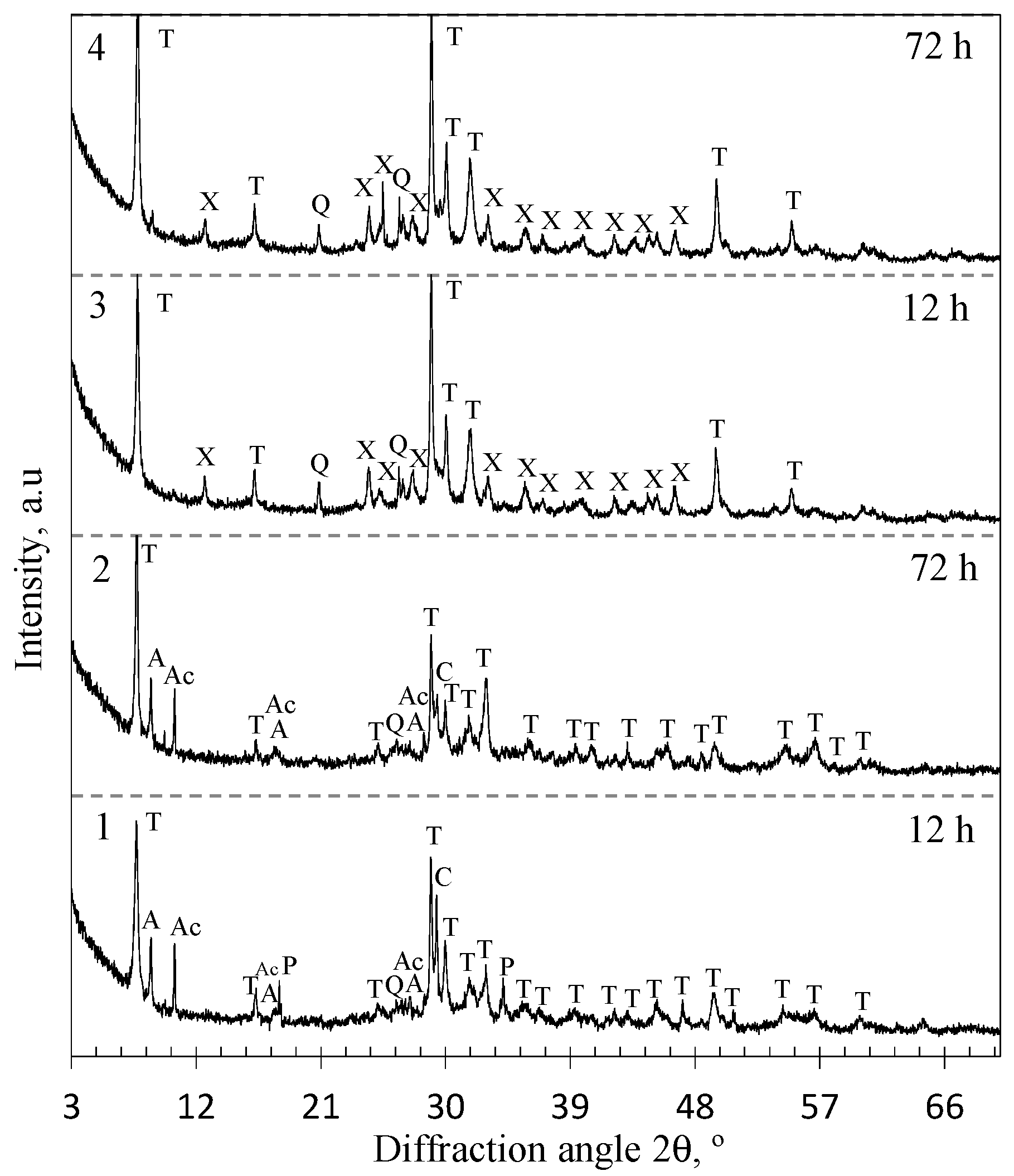
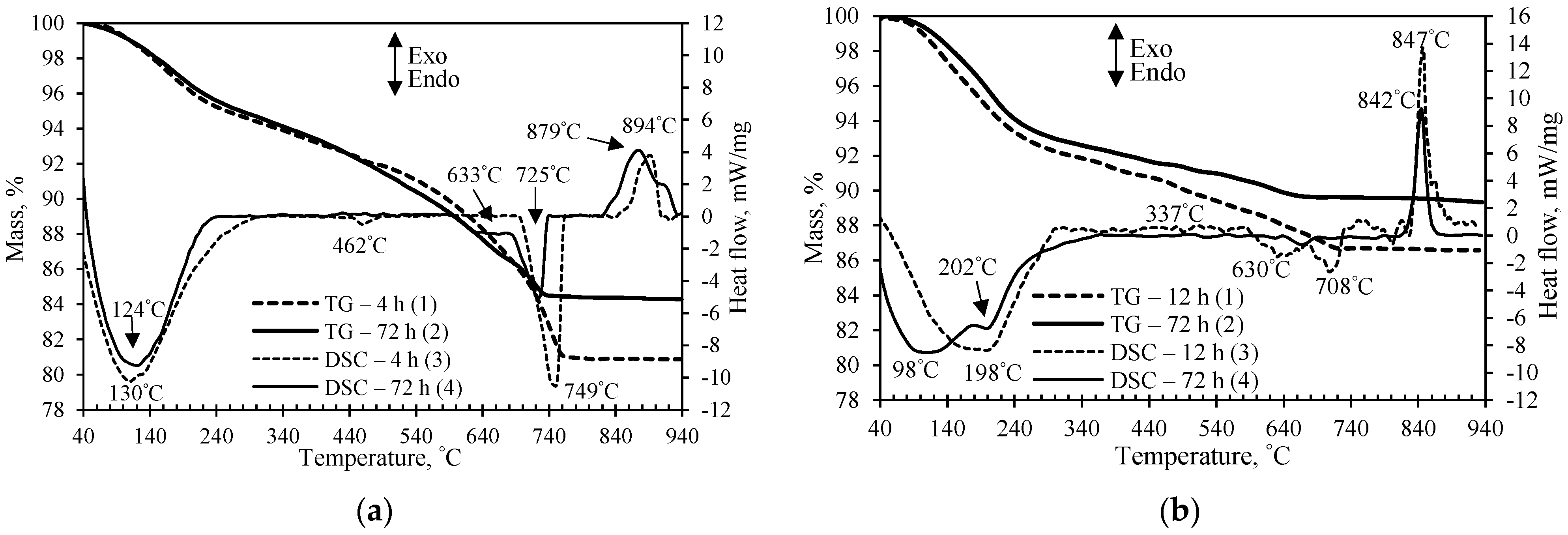
3.1. Characterization of Synthesis Products
3.2. Specific Surface Area and Porosity of Synthesis Products
4. Conclusions
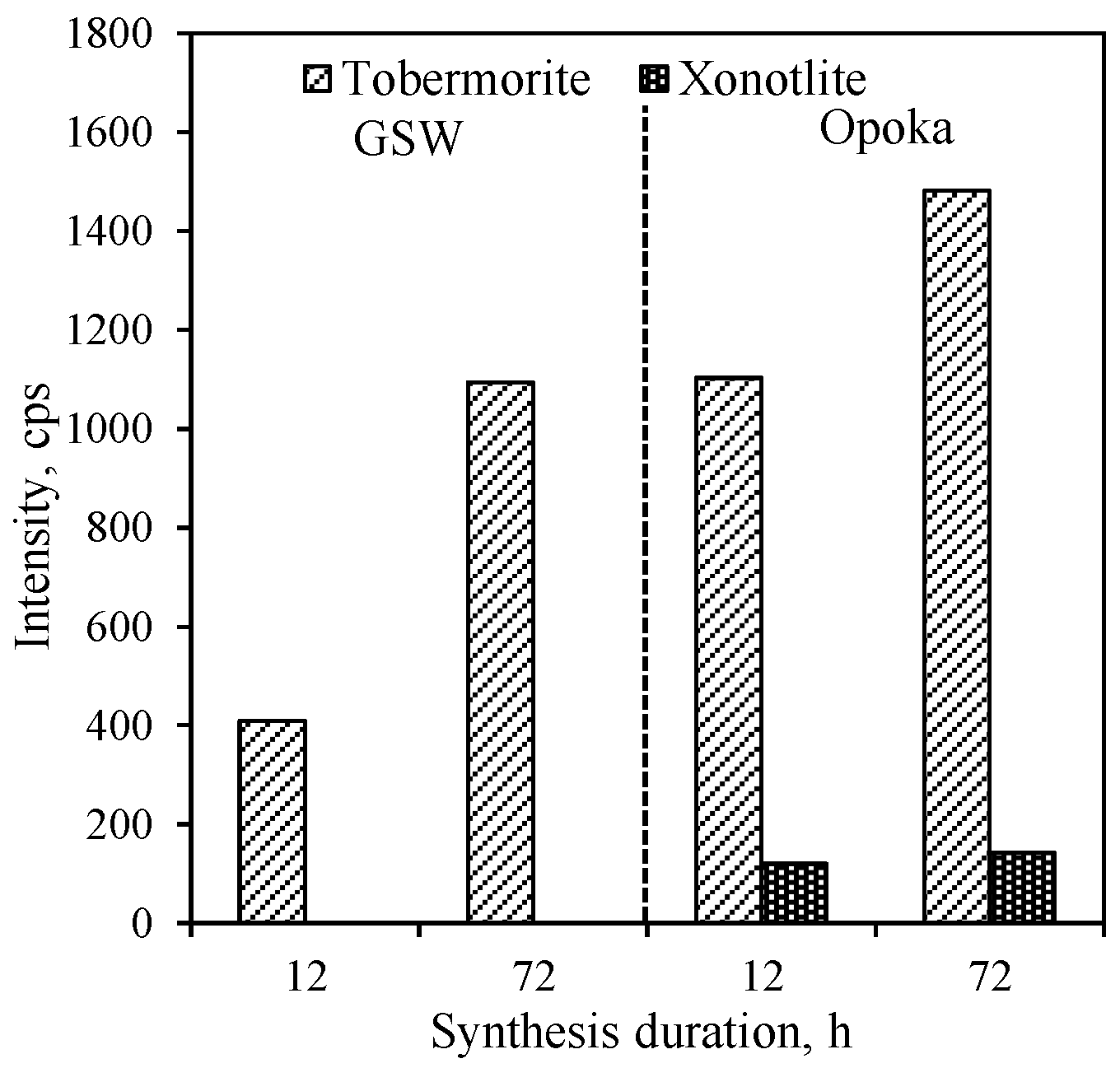
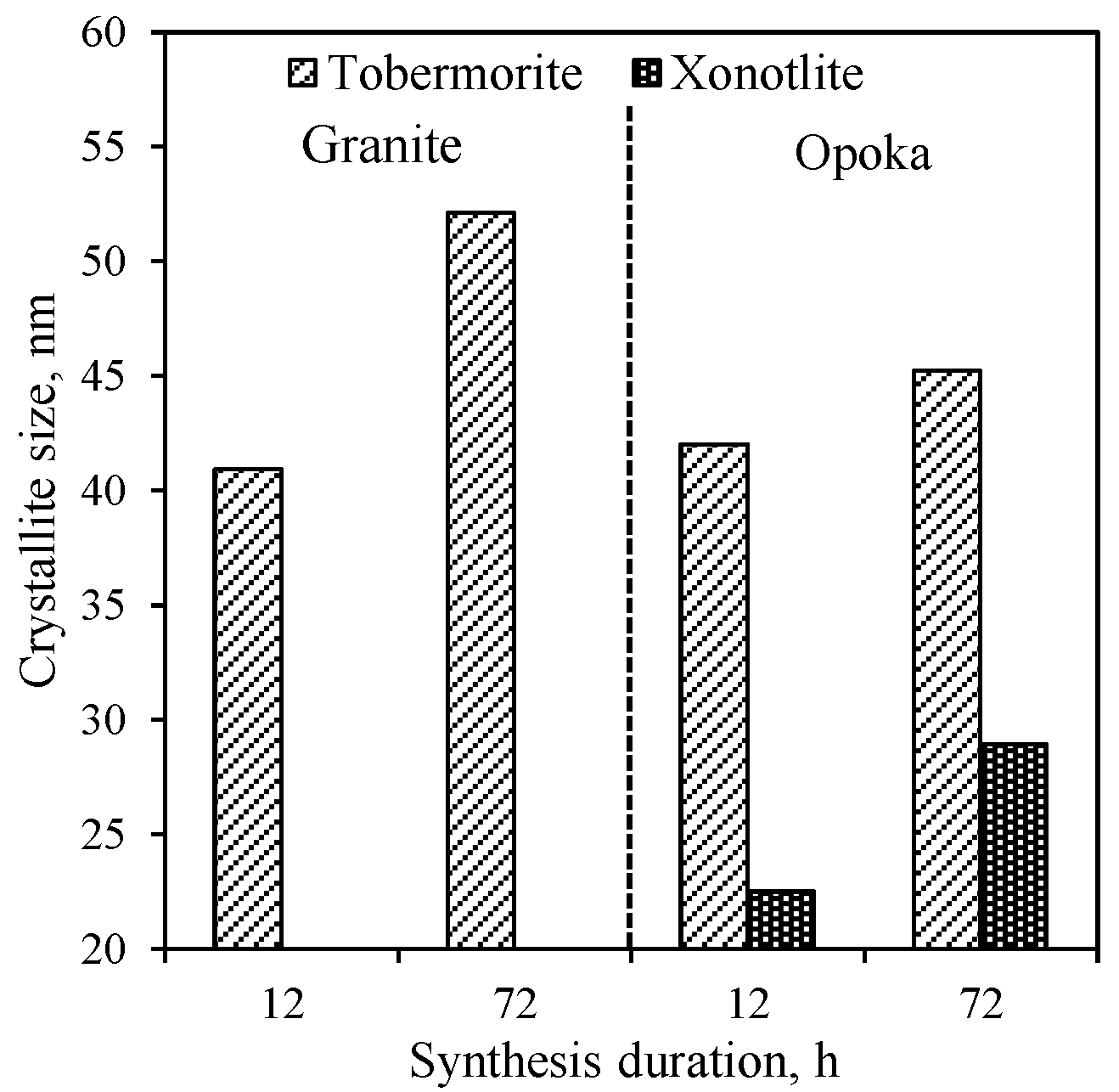
- It has been found that the granite sawing waste is a suitable material for the synthesis of 1.13 nm tobermorite—it begins to dominate in the product already after 12 h of hydrothermal curing at 200 °C. The size of the crystallites of this compound increases gradually but constantly by prolonging the duration of isothermal curing at 200 °C from 12 h to 72 h. Synthesis of xonotlite from this raw material is not recommended.
- In lime–calcined opoka suspensions, the formation of crystalline calcium silicate hydrates takes place much faster than in the lime–granite sawing waste mixture. The high reactivity of amorphous SiO2 results in the rapid formation of 1.13 nm tobermorite and xonotlite (12 h). After extending the curing duration to 72 h, the size of the crystallites of the formed compounds changes differently. In the case of 1.13 nm tobermorite this value increases only slightly (from 42 to 45.2 nm), while in the case of xonotlite increases by almost 30% (from 22.5 to 28.9 nm).
- The high reactivity of calcined opoka under hydrothermal conditions is due to its chemical composition, especially the presence of 2.53% Al2O3 and 0.83% K2O. Compounds containing aluminum and potassium ions are evenly distributed throughout the raw material. Al3+ ions stimulate the reactions of amorphous SiO2 and CaO, which results in the faster formation of 1.13 nm tobermorite in the early stages of hydrothermal synthesis. K+ ions accelerate the dissolution of SiO2 crystalline modifications (quartz, tridymite, and cristobalite) by destroying the surface of particles and promoting xonotlite formation processes.
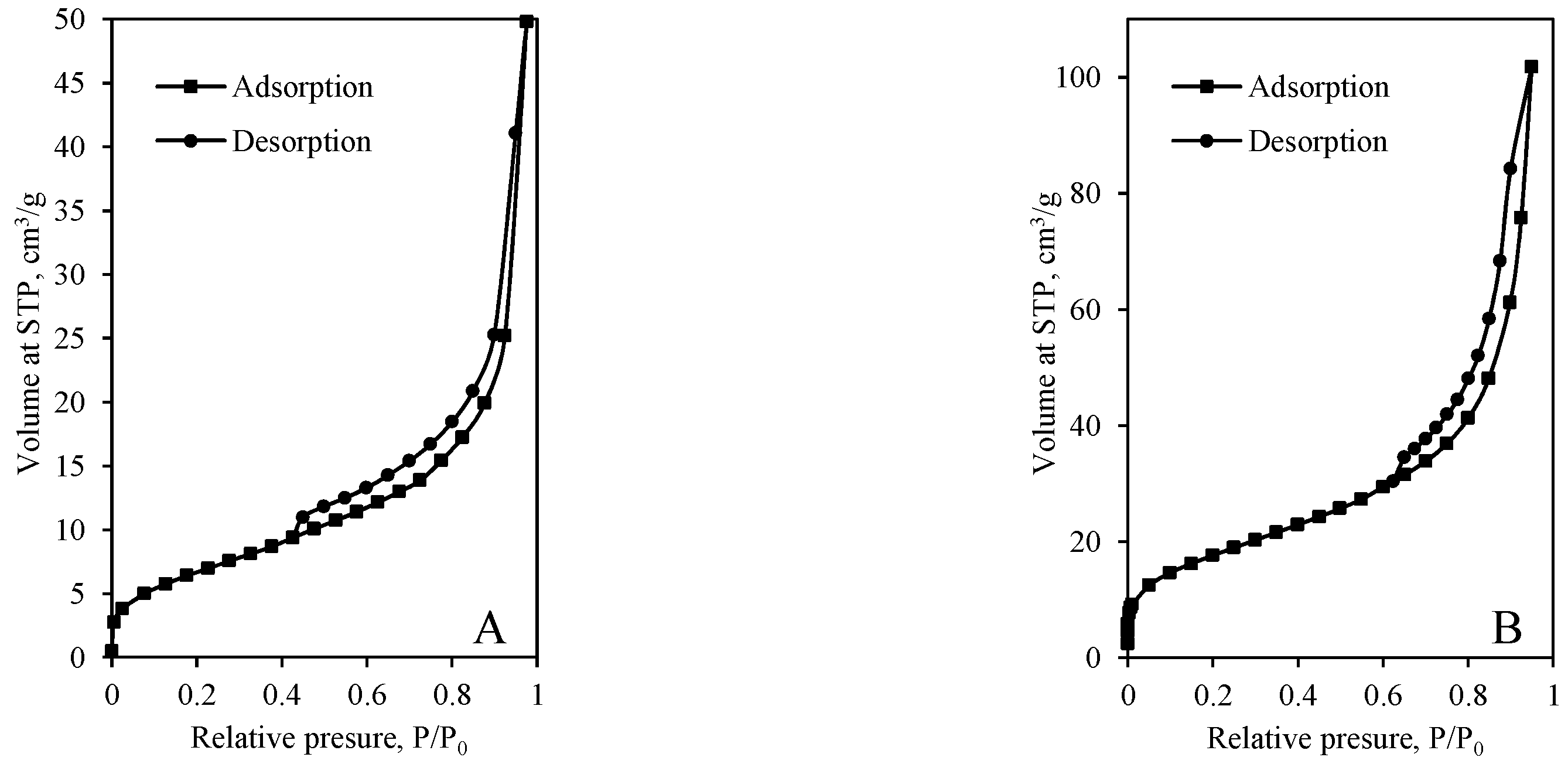
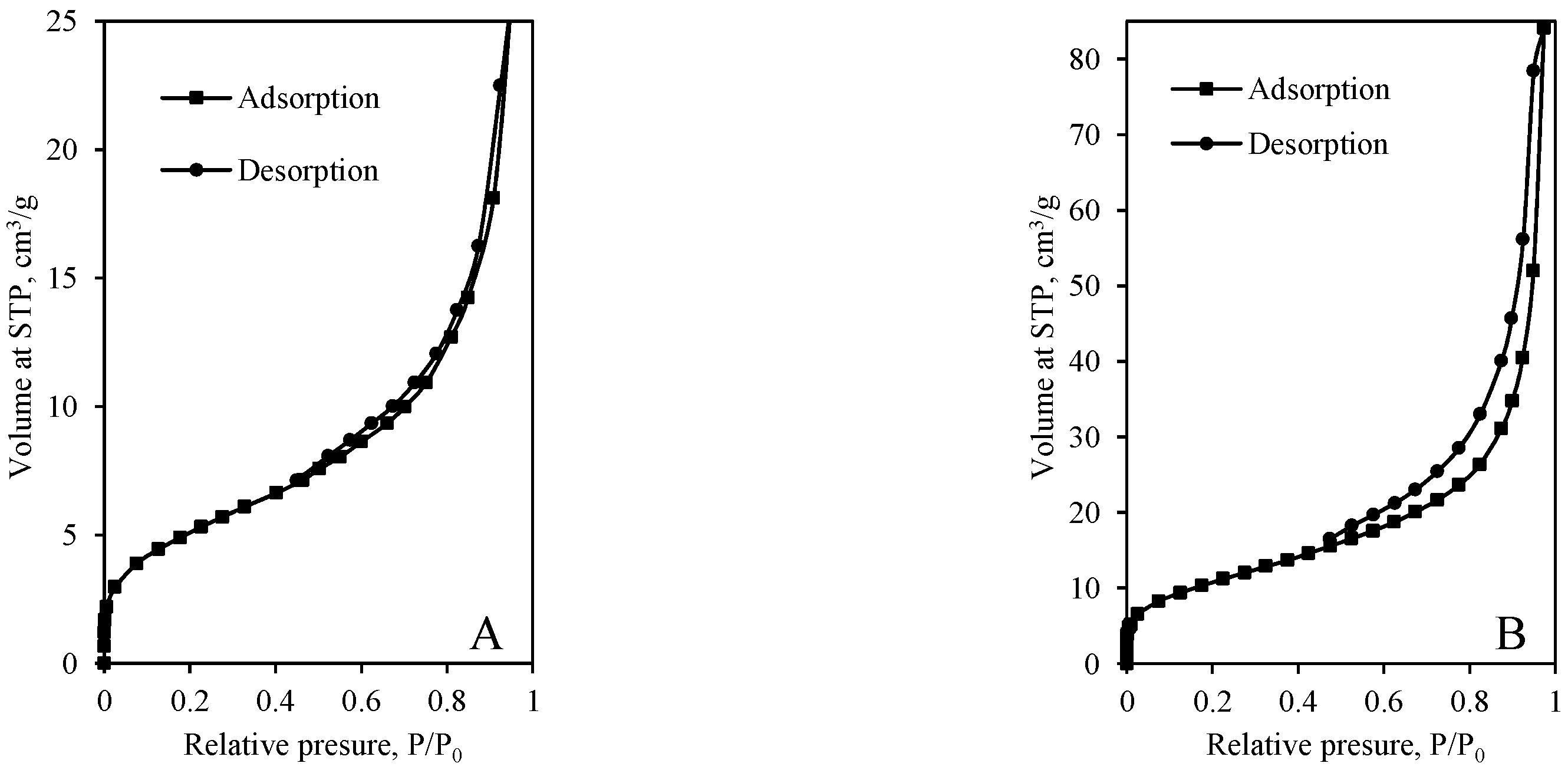
- It was determined that the shape of pores and specific surface area depend on both the chemical composition of the primary mixture and the duration of synthesis. The specific surface area and total pore volume of sample synthesized in granite sawing waste–CaO mixture for 12 h are equal to 25 m2/g and 80 mm3/g, respectively. The calculations revealed that cylindrical-shape and slit-shape pores are presented in the sample. It was obtained that by extending the duration of hydrothermal synthesis to 72 h, the sample becomes nonporous whose SBET is equal to ~19 m2/g.
- It was obtained that the cylindrical-shape pores were formed in synthesis products obtained in opoka–CaO mixture. Since a high amount of tobermorite and xonotlite is presented in the samples synthesized for 12 h and 72 h, their specific surface area is quite high and equal to 64 m2/g and 40 m2/g, respectively. It was calculated that the total pore volume decreased from 245 mm3/g to 137 mm3/g by prolonging the duration of hydrothermal synthesis. In both samples pores with 1–2.5 nm and 5–20 nm diameter are dominant. Such parameters of synthesis products should provide good thermal insulation properties for the products made from this material as no air convection occurs in the fine pores.
- The results obtained in the work form the basis in the development of xonotlite-type heat-resistant (up to 1000 °C), low-density (up to 200 kg/m3) thermal insulation products from the lime–calcined opoka mixture.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Somarathna, H.M.; Raman, S.N.; Mohotti, D.; Mutalib, A.A.; Badri, K.H. The use of polyurethane for structural and infrastructural engineering applications: A state-of-the-art review. Constr. Build. Mater. 2018, 190, 995–1014. [Google Scholar] [CrossRef]
- Sudol, E.; Kozikowska, E. Mechanical properties of polyurethane adhesive bonds in a mineral wool-based external thermal insulation composite system for timber frame buildings. Materials 2021, 14, 2527. [Google Scholar] [CrossRef]
- Choi, H.J.; Kang, J.S.; Huh, J.H. A study of variation of thermal characteristics of insulation materials for buildings according to actual long-term annual aging variation. Int. J. Thermophys. 2018, 39, 2. [Google Scholar] [CrossRef] [Green Version]
- Thermodynamics, Heat Transfer and Fluid Flow. In DOE Fundamentals Handbook; U.S. Department of Energy: Washington, WA, USA, 3 May 2016; Volume 2.
- Yang, L.; Mukhopadhyay, A.; Jiao, Y.; Yong, Q.; Chen, L.; Xing, Y.; Hamel, J.; Zhu, H. Ultralight, highly thermally insulating and fire resistant aerogel by encapsulating cellulose nanofibers with two-dimensional MoS2. Nanoscale 2017, 9, 11452. [Google Scholar] [CrossRef] [PubMed]
- Popov, T.A.; Emberlin, J.; Church, M.K.; Aberg, N.; Josling, P. Powder cellulose in allergic rhinitis management: Relevance of vitro findings to real-life safety. Int. Arch. Allergy Immunol. 2019, 179, 17–18. [Google Scholar] [CrossRef]
- Engineering ToolBox, Insulation Materials—Temperature Ranges. 2005. Available online: https://www.engineeringtoolbox.com/insulation-temperatures-d_922.html (accessed on 7 August 2021).
- Mahltig, B.; Kyosev, Y. Inorganic and Composite Fibers: Production, Properties, and Applications; Chapter 7—Glass Fibers; Martynova, E., Cebull, H., Eds.; Woodhead Publishing: Sawston, UK, 2019; pp. 131–163. [Google Scholar]
- Eske, J. How to remove fiberglass in skin. Medical News Today. 2020. Available online: https://www.medicalnewstoday.com/articles/fiberglass-in-skin (accessed on 23 July 2021).
- Tingley, D.D.; Hathway, A.; Davison, B.; Allwood, D. The environmental impact of phenolic foam insulation boards. Proc. Inst. Civ. Eng. Constr. Mater. 2017, 170, 91–103. [Google Scholar] [CrossRef] [Green Version]
- Ko, H.; Lee, H.S.; Lim, H.M. Effects of additives in colloidal silica based inorganic-hybrid binder for mineral wool insulation boards. J. Asian Ceram. Soc. 2020, 8, 1285–1295. [Google Scholar] [CrossRef]
- Maleki, H.; Duraes, L.; Portugal, A. An overview on silica aerogels synthesis and different mechanical reinforcing strategies. J. Non-Cryst. Solids 2014, 365, 55–74. [Google Scholar] [CrossRef] [Green Version]
- Lin, J.; Li, G.; Liu, W.; Qiu, R.; Wei, H.; Zong, K.; Cai, X. A review of recent progress on the silica aerogel monoliths: Synthesis, reinforcement, and applications. J. Mater. Sci. 2021, 56, 10812–10833. [Google Scholar] [CrossRef]
- Wei, G.; Liu, Y.; Zhang, Y.; Yu, F.; Du, X. Thermal conductivities study on silica aerogel and its composite insulation materials. Int. J. Heat Mass Transf. 2011, 54, 2355–2366. [Google Scholar] [CrossRef]
- Liu, F.; Zeng, L.; Cao, J.; Li, J. Preparation of ultra-light xonotlite thermal insulation material using carbide slag. J. Wuhan Univ. Technol.-Mater. Sci. Ed. 2010, 25, 295–297. [Google Scholar] [CrossRef]
- Wei, G.; Zhang, X.; Yu, F. Thermal conductivity of xonotlite insulation material. Int. J. Thermophys. 2007, 28, 1718–1729. [Google Scholar] [CrossRef]
- Zheng, Q.; Wang, W. Calcium silicate based high efficiency thermal insulation. Br. Ceram. Trans. 2000, 99, 187–190. [Google Scholar] [CrossRef]
- Standard LST EN 16977: 2021. Thermal Insulation Products for Buildings—Factory Made Calcium Silicate (CS) Products—Specification; Lithuanian Standards Board: Vilnius, Lithuania, 2020; pp. 1–34. [Google Scholar]
- Galvankova, L.; Masilko, J.; Solny, T.; Stepankova, E. Tobermorite synthesis under hydrothermal conditions. Procedia Eng. 2016, 151, 100–107. [Google Scholar] [CrossRef]
- Jimenez, I.; Perez, G.; Guerrero, A.; Ruiz, B. Mineral phases synthesized by hydrothermal treatment from biomass ashes. Int. J. Miner. Process. 2017, 158, 8–12. [Google Scholar] [CrossRef]
- Smalakys, G.; Siauciunas, R. The synthesis of 1.13 nm tobermorite from carbonated opoka. J. Therm. Anal. Calorim. 2018, 134, 493–502. [Google Scholar] [CrossRef]
- Alizadeh, R.; Beaudoin, J.J.; Raki, L. Mechanical properties of calcium silicate hydrates. Mater. Struct. 2011, 44, 13–28. [Google Scholar] [CrossRef] [Green Version]
- Pugovkina, Y.; Kutugin, V.; Ostroumova, A.; Rymanova, I. High temperature and heat insulated calcium silicate materials. Key Eng. Mater. Trans. Tech. Publ. 2016, 683, 209–214. [Google Scholar] [CrossRef] [Green Version]
- Akbayrak, S.; Ozkar, S. Inverse relation between the catalytic activity and catalyst concentration for the ruthenium (0) nanoparticles supported on xonotlite nanowire in hydrogen generation from the hydrolysis of sodium borohydride. J. Mol. Catal. A Chem. 2016, 424, 254–260. [Google Scholar] [CrossRef]
- Shaw, S.; Henderson, C.M.B.; Komanschek, B.U. Dehydration/recrystallization mechanisms, energetics, and kinetics of hydrated calcium silicate minerals: An in situ TGA/DSC and synchrotron radiation SAXS/WAXS study. Chem. Geol. 2000, 167, 141–159. [Google Scholar] [CrossRef]
- Biagoni, C.; Bonaccorsi, E.; Lezzerini, M.; Merlino, S. Thermal behaviour of Al-rich tobermorite. Eur. J. Miner. 2016, 28, 23–32. [Google Scholar] [CrossRef]
- Rodriguez, E.T.; Garbev, K.; Merz, D.; Black, L.; Richardson, I. Thermal stability of C-S-H phases and applicability of Richardson and Groves’ and Richardson C-(A)-S-H(I) models to synthetic C-S-H. Cem. Concr. Res. 2017, 93, 45–56. [Google Scholar] [CrossRef]
- Akbayrak, S.; Ozkar, S. Ruthenium (0) nanoparticles supported on xonotlite nanowire: A long-lived catalyst for hydrolytic dehydrogenation of ammonia-borane. Dalton Trans. 2014, 43, 1797–1805. [Google Scholar] [CrossRef]
- Li, S.; Qin, H.; Zhang, T.; Cong, H.-P.; Yu, S.-H. Bioinspired ternary hydrogels synergistically reinforced by graphene/xonotlite network. Small 2018, 14, 1800673. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.Y.; Chang, F.J.; Gao, H.L.; Chen, S.; Gao, M.-R.; Zheng, Y.; Cölfen, H.; He, C.; Yu, S.-H. Bio-inspired synthesis of hematite mesocrystals by using xonotlite nanowires as growth modifiers and their improved oxygen evolution activity. ChemSusChem 2019, 12, 3747–3752. [Google Scholar] [CrossRef] [PubMed]
- Liu, F.; Chen, S.; Lin, Q.; Wang, X.D.; Cao, J.X. Study on hydrothermal synthesis dynamics of nanoscale xonotlite fibers. IOP Conf. Ser. Mater. Sci. Eng. 2018, 284, 12021. [Google Scholar] [CrossRef] [Green Version]
- Paradiso, P.; Santos, R.L.; Horta, R.B.; Lopes, J.; Ferreira, P.; Colaço, R. Formation of nanocrystalline tobermorite in calcium silicate binders with low C/S ratio. Acta Mater. 2018, 152, 7–15. [Google Scholar] [CrossRef]
- Grangeon, S.; Claret, F.; Roosz, C.; Sato, T.; Gaboreau, S.; Linard, Y. Structure of nanocrystalline calcium silicate hydrates: Insights from X-ray diffraction, synchrotron X-ray absorption and nuclear magnetic resonance. J. Appl. Crystallogr. 2016, 49, 771–783. [Google Scholar] [CrossRef]
- Siauciunas, R.; Mikaliunaite, J.; Urbonas, L.; Baltakys, K.K. Tribochemical and thermal activation of α-C2S hydrate as precursor for cementitious binders. J. Therm. Anal. Calorim. 2014, 118, 817–823. [Google Scholar] [CrossRef]
- Smalakys, G.; Siauciunas, R. Peculiarities of xonotlite synthesis from the raw materials with different SiO2 activities. J. Therm. Anal. Calorim. 2020, 142, 1671–1679. [Google Scholar] [CrossRef]
- Rouquerol, L.; Rouquerol, J.; Sing, K.S.W.; Llewellyn, P.; Maurin, G. Adsorption by Powders and Porous Solids, Principles, Methodology and Applications, 2nd ed.; Academic Press: Cambridge, MA, USA, 2014. [Google Scholar]
- Baltakys, K.; Eisinas, A.; Dizhbite, T.; Jasina, L.; Šiaučiūnas, R.; Kitrys, S. The influence of hydrothermal synthesis conditions on gyrolite texture and specific surface area. Mater. Struct. 2011, 44, 1687–1701. [Google Scholar] [CrossRef]
- Baltakys, K.; Siauciunas, R. The influence of gamma-Al2O3 and Na2O on the formation of gyrolite in the stirring suspension. J. Mater. Sci. 2006, 41, 4799–4805. [Google Scholar] [CrossRef]
- Jackson, M.D.; Moon, J.; Gotti, E.; Taylor, R.; Chae, S.R.; Kunz, M.; Emwas, A.-H.; Meral, C.; Guttmann, P.; Levitz, P.; et al. Material and elastic properties of Al-tobermorite in ancient Roman seawater concrete. J. Am. Ceram. Soc. 2013, 96, 2598–2606. [Google Scholar] [CrossRef]
- Suzuki, K.; Nishkawa, T.; Ito, S. Formation and carbonation of CSH in water. Cem. Concr. Res. 1985, 15, 213–224. [Google Scholar] [CrossRef]
- Matsushita, F.; Aono, Y.; Shibata, S. Calcium silicate structure and carbonation shrinkage of a tobermorite-based material. Cem. Concr. Res. 2004, 34, 1251–1257. [Google Scholar] [CrossRef]
- Saito, T.; Sakai, E.; Morioka, M.; Otsuki, N. Carbonation of γ-Ca2SiO4 and the Mechanism of Vaterite Formation. J. Adv. Concr. Technol. 2010, 8, 273–280. [Google Scholar] [CrossRef] [Green Version]
- Youssef, H.; Ibrahim, D.; Komarneni, S.; Mackenzie, K.J.D. Synthesis of 11 Å Al-substituted tobermorite from trachyte rock by hydrothermal treatment. Ceram. Int. 2010, 36, 203–209. [Google Scholar] [CrossRef]




| Material | Oxides, wt% | Other | Loss of Ignition, wt% | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| SiO2 | CaO | Al2O3 | K2O | Na2O | MgO | Fe2O3 | SO3 | |||
| Opoka | 54.60 | 22.10 | 2.53 | 0.83 | 0.09 | 0.55 | 1.66 | 0.58 | 0.74 | 16.41 |
| Granite | 58.41 | 3.95 | 15.41 | 3.86 | 3.45 | 2.87 | 7.17 | 0.19 | 0.48 | 4.31 |
| Limestone | 4.38 | 50.88 | 0.22 | 0.23 | – | 1.67 | 0.70 | 0.53 | – | 41.39 |
| Material | Minerals | ||||||
|---|---|---|---|---|---|---|---|
| Opoka | Quartz | Cristobalite | Tridymite | Amorphous Part | Muscovite | Calcite | Dolomite |
| wt% | 8.7 | 21.1 | 5.2 | 19.6 | 3.9 | 38.8 | 2.7 |
| Granite | Quartz | Albite | Anorthite | Labradorite | Microcline | Annite | Actinolite |
| wt% | 23.4 | 17.8 | 11.4 | 13.4 | 19.2 | 6.7 | 7.9 |
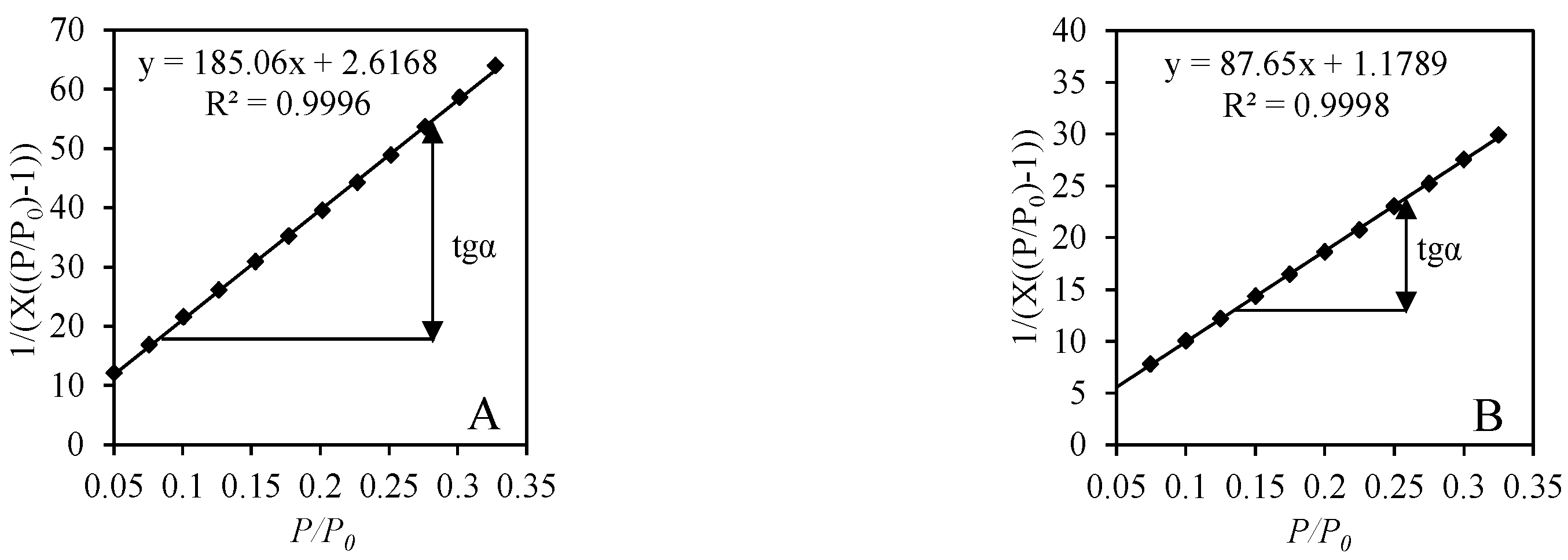
| Raw Materials | Duration, h | BET Equation Constants | CBET Constant | Capacity of Monolayer Xm, g | SBET, m2/g | |
|---|---|---|---|---|---|---|
| Slope S | Intercept I | |||||
| Lime–GSW | 12 | 137.26 | 2.652 × 10−1 | 52.74 | 0.0072 | 24.91 |
| Lime–GSW | 72 | 183.09 | 2.816 × 10−1 | 66.02 | 0.0054 | 18.73 |
| Lime–calcined opoka | 12 | 53.81 | 6.230 × 10−2 | 87.38 | 0.0182 | 63.98 |
| Lime–calcined opoka | 72 | 86.76 | 1.303 × 10−1 | 67.58 | 0.0114 | 39.55 |
| Mixture | Duration | Calculation Results Using the Cylindrical Pore Model | Calculation Results Using the Parallel Plate Pore Model | ||
|---|---|---|---|---|---|
| ΣA, m2/g | |SBET − ΣA|, % | ΣA, m2/g | |SBET − ΣA|, % | ||
| Lime–GSW | 12 h | 31.07 | 24.72 | 16.73 | 32.83 |
| Lime–GCW | 72 h | - | - | - | - |
| Lime–calcined opoka | 12 h | 68.93 | 7.73 | 37.82 | 40.89 |
| Lime–calcined opoka | 72 h | 50.63 | 28.01 | 27.18 | 31.22 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Siauciunas, R.; Smalakys, G.; Dambrauskas, T. Porosity of Calcium Silicate Hydrates Synthesized from Natural Rocks. Materials 2021, 14, 5592. https://doi.org/10.3390/ma14195592
Siauciunas R, Smalakys G, Dambrauskas T. Porosity of Calcium Silicate Hydrates Synthesized from Natural Rocks. Materials. 2021; 14(19):5592. https://doi.org/10.3390/ma14195592
Chicago/Turabian StyleSiauciunas, Raimundas, Giedrius Smalakys, and Tadas Dambrauskas. 2021. "Porosity of Calcium Silicate Hydrates Synthesized from Natural Rocks" Materials 14, no. 19: 5592. https://doi.org/10.3390/ma14195592
APA StyleSiauciunas, R., Smalakys, G., & Dambrauskas, T. (2021). Porosity of Calcium Silicate Hydrates Synthesized from Natural Rocks. Materials, 14(19), 5592. https://doi.org/10.3390/ma14195592







