A Calculation Model for Cooling Rate of Aluminum Alloy Melts during Continuous Rheo-Extrusion
Abstract
:1. Introduction
2. Calculation Model and Governing Equations
- The process is a continuous and stable process. The impact, leakage and flow undulation of melt at the initial and finishing stages are neglected.
- The melt is incompressible viscoplastic material, and the volume constancy is satisfied.
- The temperature of the roll and shoe surfaces contacting to melt is considered as the constant.
- The heat transfer by the water in the inlet and outlet pipes is ignored.
- The temperature of the sections where the cooling water pipe contacting with the roll is considered to be identical.
- The flow velocity in the cooling water pipes is uniform, and the velocity of water is regarded as a constant value at the same geometric position.
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Thermal conductivity, W/(m·K) | |
| thermal conductivity of low-carbon steel roll | |
| thermal conductivity of cooling water | |
| thermal conductivity of melt | |
| Heat, J | |
| released heat due to the decrease of melt temperature | |
| heat taked away by the cooling water | |
| convective heat at the cooling water/roll interface | |
| heat conducted inside the roll | |
| convective heat at the melt/roll interface | |
| heat released by latent heat of melt solidification | |
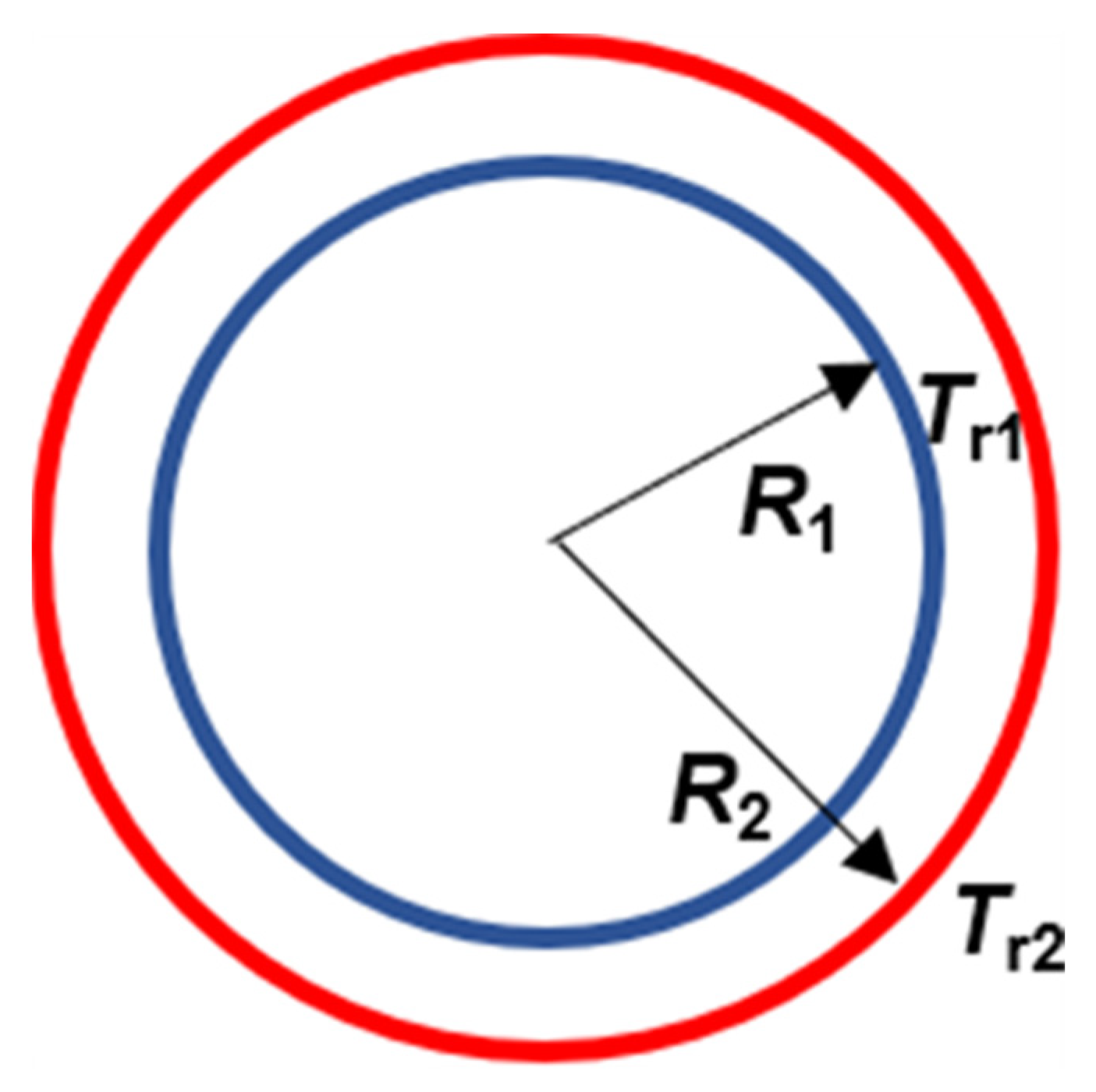
| Radius, m | |
| radius of the roll at the cooling water pipes position | |
| outside radius of roll | |
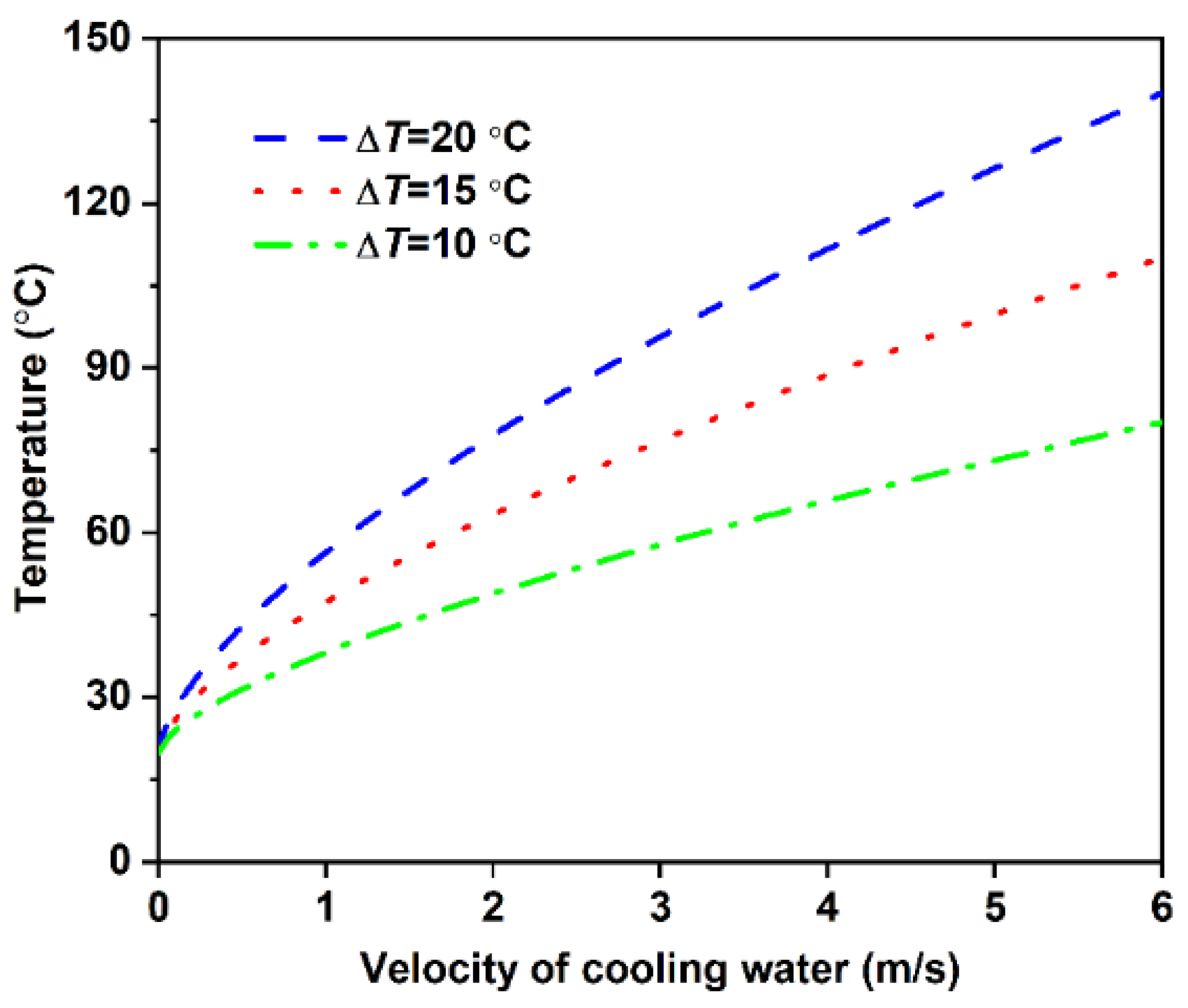
| Temperature, °C | |
| initial temperature of melt that is equal to the pouring temperature | |
| temperature of melt in roll-shoe gap | |
| temperature of cooling water in inlet pipe | |
| temperature of cooling water in outlet pipe | |
| temperature of cooling water at the cooling water/roll interface | |
| temperature of roll at the cooling water/roll interface | |
| temperature of roll at the melt/roll interface | |
| Diameter, m | |
| diameter of cooling water inlet pipe | |
| diameter of cooling water pipes parallel to the axis of roll | |
| equivalent diameter of cooling water pipe parallel to the axis of roll | |
| equivalent diameter of cooling water pipe vertical to the axis of roll | |
| Feature length, m | |
| length of cross section of roll-shoe gap | |
| lengths are shown in Figure 3 | |
| Area, m2 | |
| area of contact surface at the cooling water/roll interface | |
| area of contact surface at the cooling melt/roll interface | |
| Specific pressure heat capacity, J/(kg·K) | |
| specific heat capacity of low-carbon steel roll | |
| specific heat capacity of melt | |
| specific heat capacity of cooling water | |
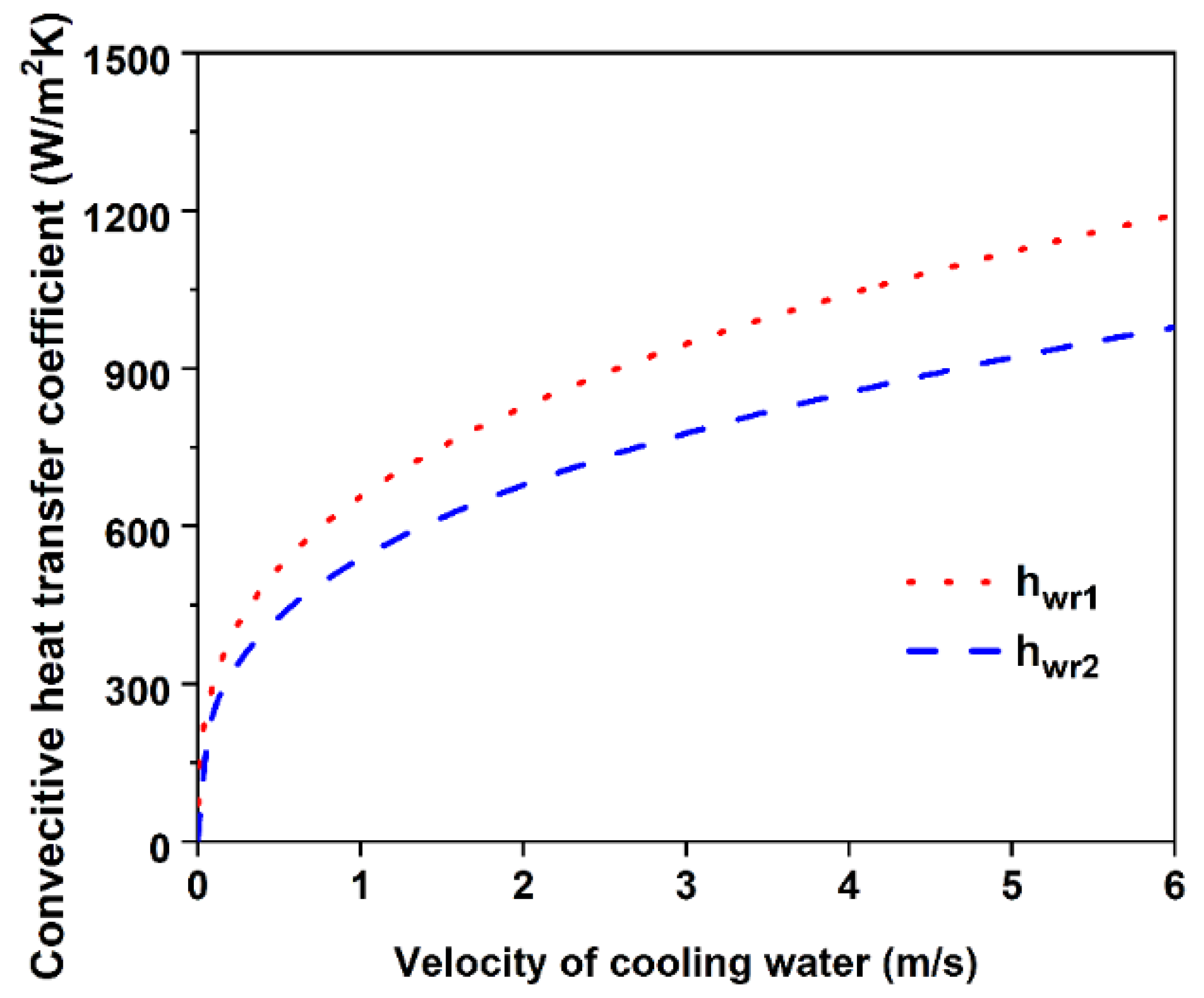
| Convective heat transfer coefficient, W/(m2·K) | |
| heat transfer coefficient acting at the cooling water/roll interface | |
| heat transfer coefficient acting at the melt/roll interface | |
| Velocity, m/s | |
| velocity of cooling water in the inlet pipe | |
| velocity of cooling water in the pipes parallel to the axis of roll | |
| velocity of cooling water in the pipes vertical to the axis of roll | |
| Motion viscosity, m2/s | |
| Density, kg/m3 | |
| density of melt | |
| density of cooling water | |
| Viscosity, Pa·s | |
| viscosity of water corresponding to the average temperature of cooling water | |
| viscosity of water corresponding to the temperature of roll at the cooling water/roll interface | |
| Time, s | |
| Volume expansion coefficient | |
| Diffusion coefficient, m2/s | |
| Reynolds number | |
| Prandlt number |
References
- Hanizam, H.; Salleh, M.S.; Omar, M.Z.; Sulong, A.B.; Arif, M.A.M. Effects of hybrid processing on microstructural and mechanical properties of thixoformed aluminum matrix composite. J. Alloy. Compd. 2020, 836, 155378. [Google Scholar] [CrossRef]
- Duan, Y.L.; Xu, G.F.; Tang, L.; Liu, Y.; Xu, J.W.; Deng, Y.; Yin, Z.M. Excellent high strain rate superplasticity of Al–Mg–Sc–Zr alloy sheet produced by an improved asymmetrical rolling process. J. Alloy. Compd. 2017, 715, 311–321. [Google Scholar] [CrossRef]
- Zang, Q.H.; Feng, D.; Lee, Y.S.; Chen, H.M.; Kim, M.S.; Kim, H.W. Microstructure and mechanical properties of Al–7.9Zn–2.7Mg–2.0Cu (wt%) alloy strip fabricated by twin roll casting and hot rolling. J. Alloy. Compd. 2020, 847, 156481. [Google Scholar] [CrossRef]
- Guan, R.G.; Zhao, Z.Y.; Chao, R.Z.; Lian, C.; Wen, J.L. Simulation of temperature field and metal flow during continuous semisolid extending extrusion process of 6201 alloy tube. T. Nonferr. Metal. Soc. 2012, 22, 1182–1189. [Google Scholar] [CrossRef]
- Chen, Z.W.; Fan, Q.Y.; Kai, Z. Microstructure and microhardness of nanostructured Al–4.6Cu–Mn alloy ribbons. Int. J. Min. Met. Mater. 2015, 22, 860–867. [Google Scholar] [CrossRef]
- Tang, Y.; Wu, Y.; Zhang, Y.; Dai, Y.; Dong, Q.; Han, Y.; Zhu, G.; Zhang, J.; Fu, Y.; Sun, B. Intermittent nucleation and periodic growth of grains under thermo-solutal convection during directional solidification of Al–Cu alloy. Acta Mater. 2021, 212, 116861. [Google Scholar] [CrossRef]
- Tang, H.P.; Wang, Q.D.; Lei, C.; Wang, K.; Ye, B.; Jiang, H.Y.; Ding, W.J. Effect of cooling rate on microstructure and mechanical properties of sand-casted Al–5.0Mg–0.6Mn–0.25Ce alloy. Acta Metall. Sin. 2019, 32, 1549–1564. [Google Scholar] [CrossRef] [Green Version]
- Tang, H.P.; Wang, Q.D.; Lei, C.; Ye, B.; Wang, K.; Jiang, H.Y.; Ding, W.J.; Zhang, X.F.; Lin, Z.; Zhang, J.B. Effect of cooling rate on microstructure and mechanical properties of an Al–5.0Mg–3.0Zn–1.0Cu cast alloy. J. Alloy. Compd. 2019, 801, 596–608. [Google Scholar] [CrossRef]
- Pang, S.; Wu, G.H.; Liu, W.C.; Sun, M.; Zhang, Y.; Liu, Z.J.; Ding, W.J. Effect of cooling rate on the microstructure and mechanical properties of sand-casting Mg–10Gd–3Y–0.5Zr magnesium alloy. Mater. Sci. Eng. A 2013, 562, 152–160. [Google Scholar] [CrossRef]
- Paliwal, M.; Jung, I.H. The evolution of the growth morphology in Mg–Al alloys depending on the cooling rate during solidification. Acta Mater. 2013, 61, 4848–4860. [Google Scholar] [CrossRef]
- Souza, E.N.; Cheung, N.; Santos, C.A.; Garcia, A. The variation of the metal/mold heat transfer coefficient along the cross section of cylindrical shaped castings. Inverse Probl. Sci. En. 2006, 14, 467–481. [Google Scholar] [CrossRef]
- Liu, Y.L.; Liu, M.; Luo, L.; Wang, J.J.; Liu, C.Z. The solidification behavior of AA2618 aluminum alloy and the influence of cooling rate. Materials 2014, 7, 7875–7890. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Liu, Z.W.; Wang, G.; Yi, J. Study on heat transfer behaviors between Al–Mg–Si alloy and die material at different contact conditions based on inverse heat conduction algorithm. J. Mater. Res. Technol. 2020, 9, 1918–1928. [Google Scholar] [CrossRef]
- Ramírez-López, A.; Aguilar-López, R.; Palomar-Pardavé, M.; Romero-Romo, M.A.; Muñoz-Negrón, D. Simulation of heat transfer in steel billets during continuous casting. Int. J. Min. Met. Mater. 2010, 17, 403–416. [Google Scholar] [CrossRef]
- Cheung, N.; Santos, N.S.; Quaresma, J.M.V.; Dulikravich, G.S.; Garcia, A. Interfacial heat transfer coefficients and solidification of an aluminum alloy in a rotary continuous caster. Int. J. Heat Mass. Tran. 2009, 52, 451–459. [Google Scholar] [CrossRef]
- Dutra, J.C.; Silva, R.E.; Savi, B.M.; Marques, C.; Alarcon, O.E. Metallurgical characterization of the 5083H116 aluminum alloy welded with the cold metal transfer process and two different wire-electrodes (5183 and 5087). Weld. World 2015, 59, 797–807. [Google Scholar] [CrossRef]
- Reza, T.; Reza, E.A.; Mehdi, A.S.; Reza, Y.A. The effect of harmonic vibration with a frequency below the resonant range on the mechanical properties of AA-5083-H321 aluminum alloy GMAW welded parts. Mater. Sci. Eng. A 2018, 736, 248–257. [Google Scholar] [CrossRef]
- Bergman, T.L.; Lavine, A.S.; Incropera, F.P.; DeWitt, D.P. Fundamentals of Heat and Mass Transfer, 7th ed.; John Wiley and Sons: Hoboken, NJ, USA, 2011; ISBN 9780470501979. [Google Scholar]
- Yang, S.M.; Tao, W.S. Heat Transfer, 4th ed.; Higer Education Press: Beijing, China, 2006; ISBN 9787040189186. [Google Scholar]
- Chapman, B. Heat transfer. Magn. Reson. Mater. Phy. 1974, 9, 146–151. [Google Scholar] [CrossRef] [PubMed]





| 9.982 × 102 | |
|---|---|
| Constant pressure specific heat capacity, , J/(kg·K) | 4.183 × 103 |
| Motion viscosity, , m2/s | 1.006 × 10−6 |
| Thermal conductivity, , W/(m·K) | 0.599 |
| Viscosity, , Pa·s | 1.004 × 10−9 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Gao, M.; Yang, B.; Bai, J.; Guan, R. A Calculation Model for Cooling Rate of Aluminum Alloy Melts during Continuous Rheo-Extrusion. Materials 2021, 14, 5684. https://doi.org/10.3390/ma14195684
Wang Y, Gao M, Yang B, Bai J, Guan R. A Calculation Model for Cooling Rate of Aluminum Alloy Melts during Continuous Rheo-Extrusion. Materials. 2021; 14(19):5684. https://doi.org/10.3390/ma14195684
Chicago/Turabian StyleWang, Yu, Minqiang Gao, Bowei Yang, Jingyuan Bai, and Renguo Guan. 2021. "A Calculation Model for Cooling Rate of Aluminum Alloy Melts during Continuous Rheo-Extrusion" Materials 14, no. 19: 5684. https://doi.org/10.3390/ma14195684
APA StyleWang, Y., Gao, M., Yang, B., Bai, J., & Guan, R. (2021). A Calculation Model for Cooling Rate of Aluminum Alloy Melts during Continuous Rheo-Extrusion. Materials, 14(19), 5684. https://doi.org/10.3390/ma14195684






