New Edge Crush Test Configuration Enhanced with Full-Field Strain Measurements
Abstract
1. Introduction
2. Materials and Methods
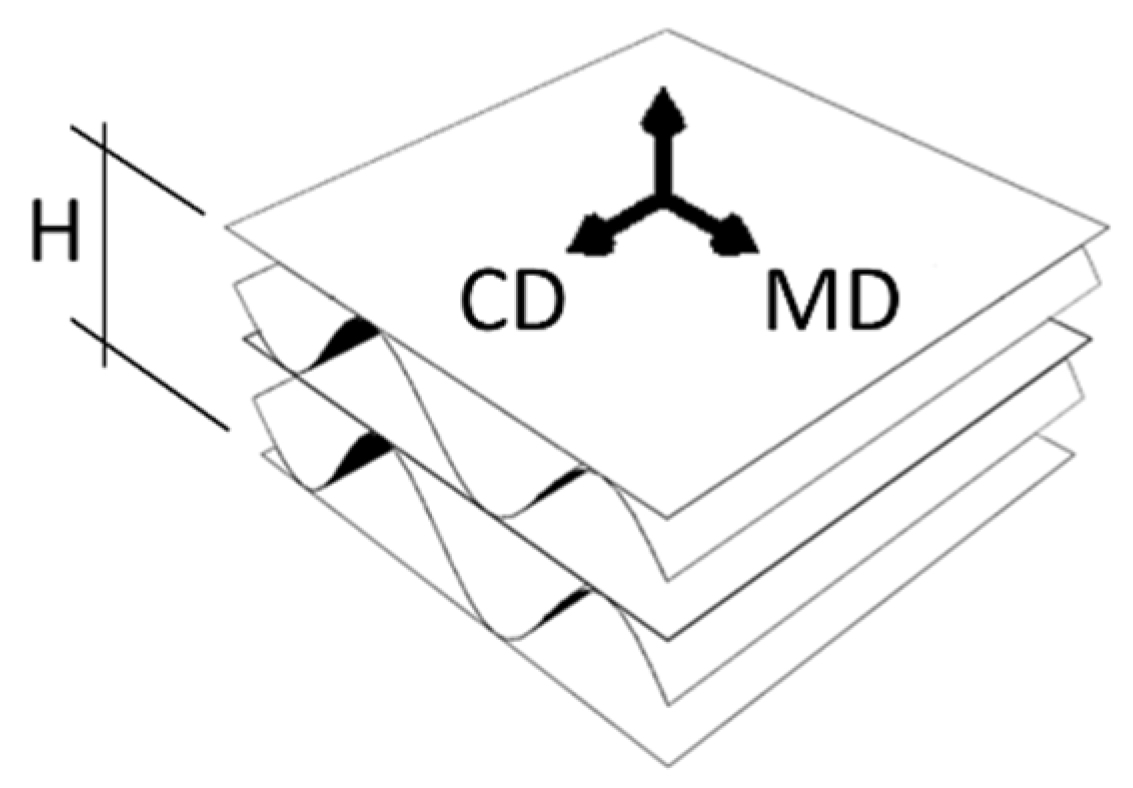
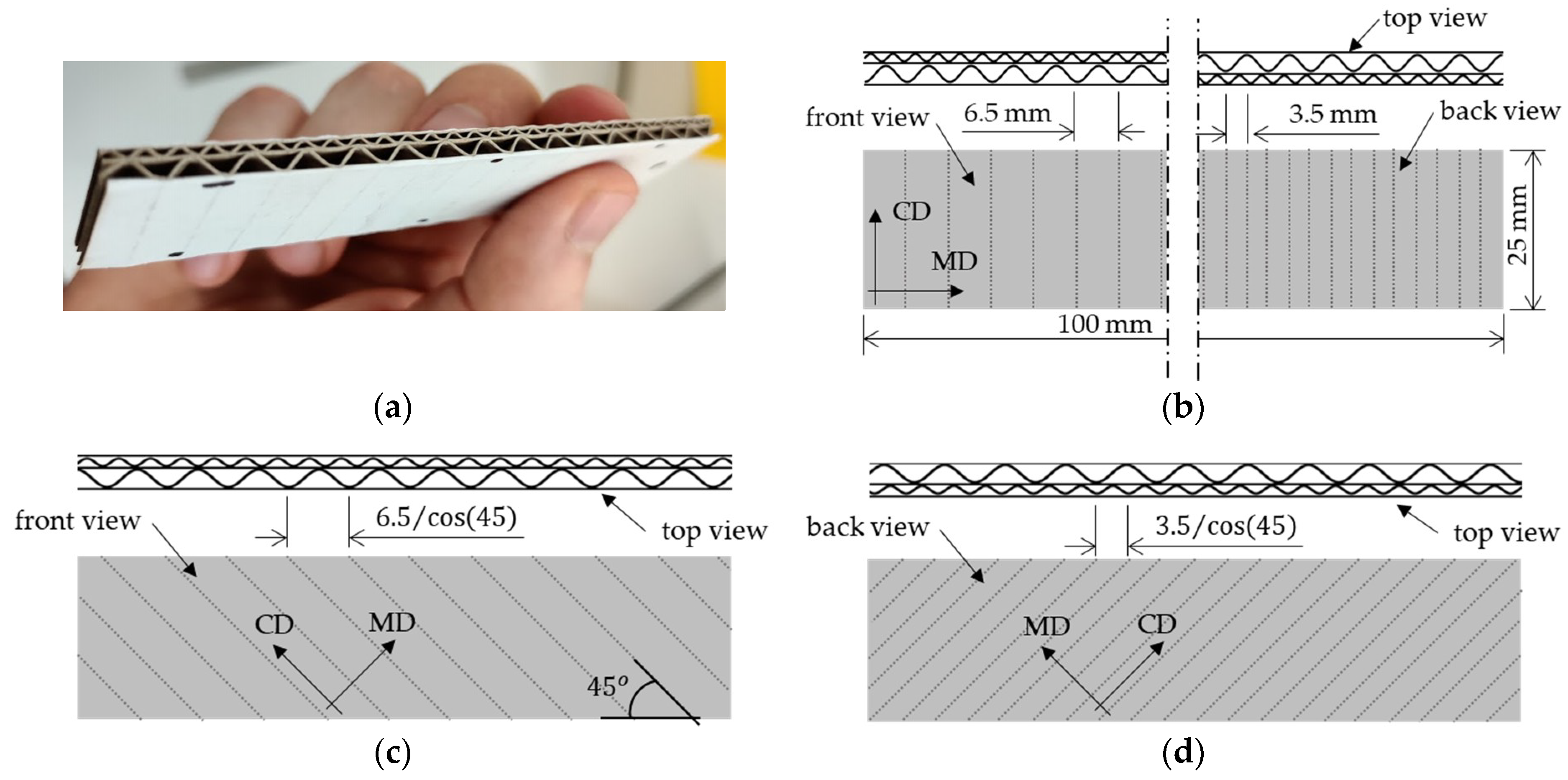
2.1. Corrugated Cardboard
2.2. The Edge Crush Test
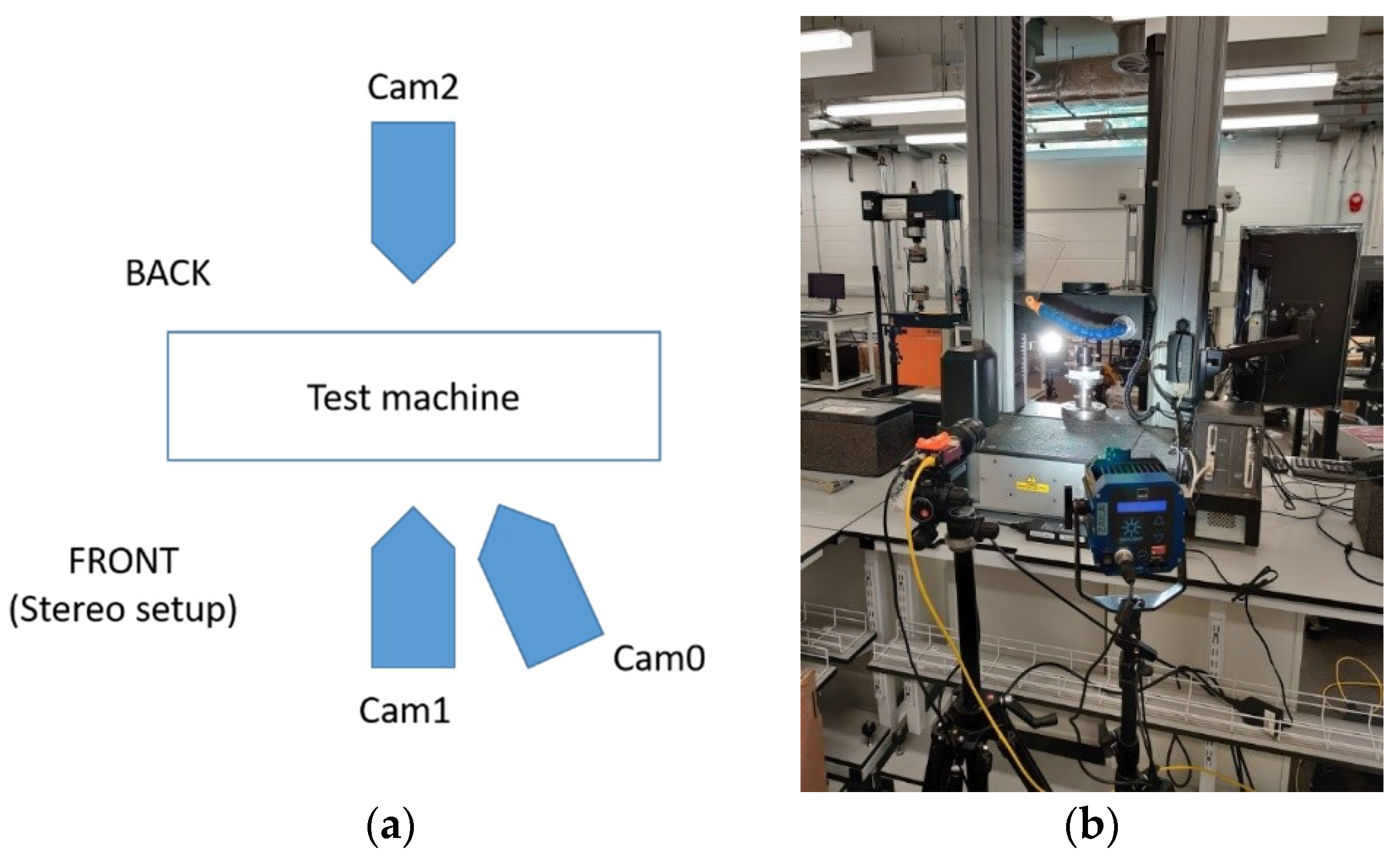
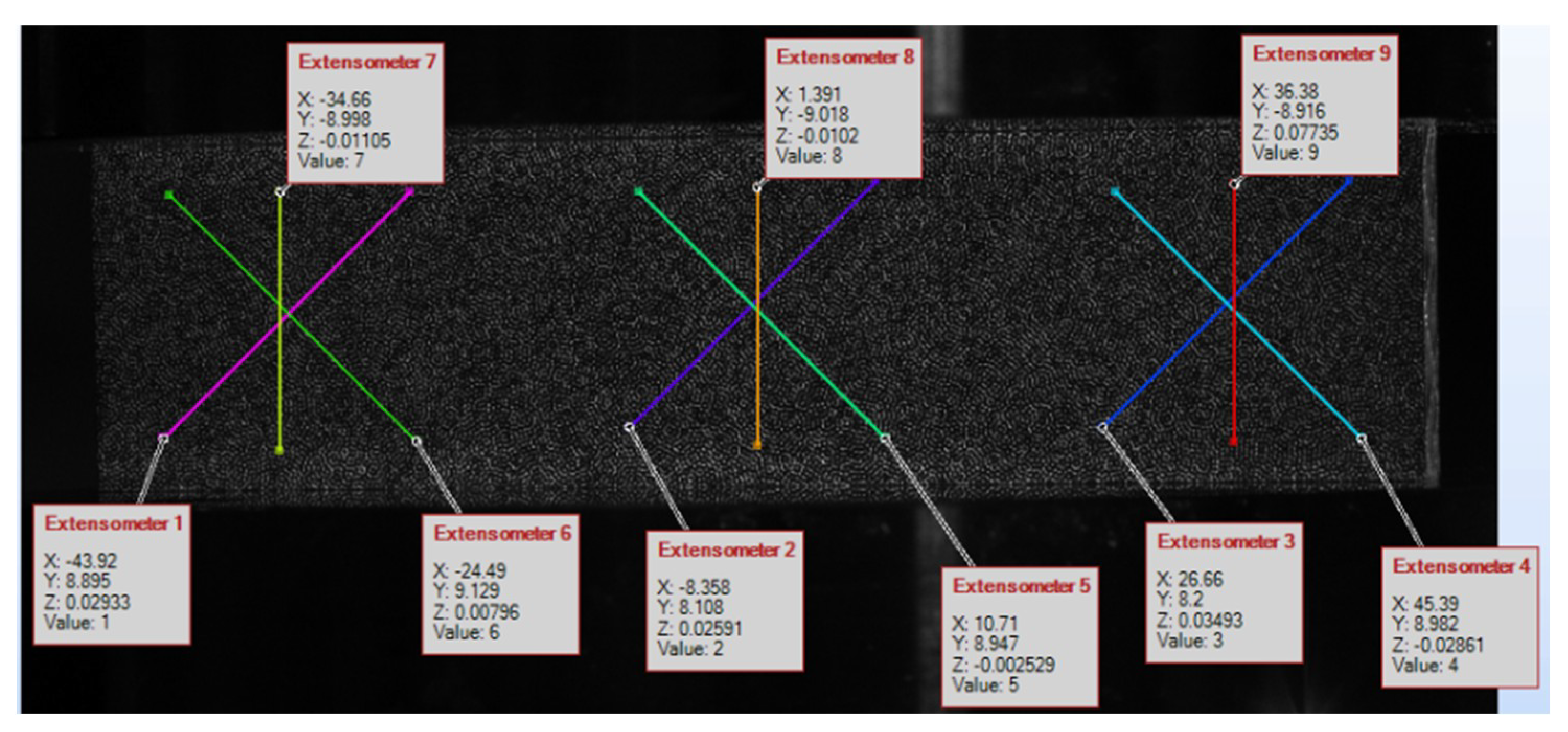
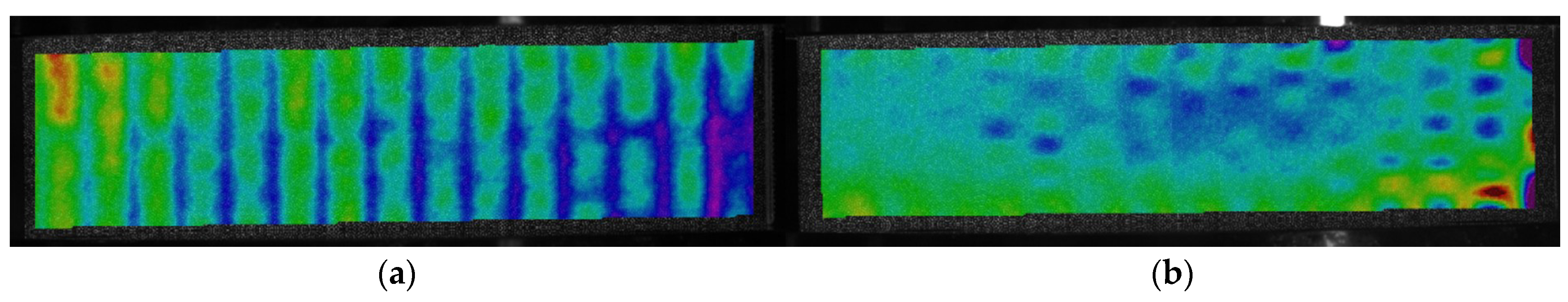
2.3. Optical Measurements of Sample Deformation
- Crosshead from the machine.
- Stereo (2.5D) DIC on the front (see Figure 5b) plus extensometry on the back.
- Extensometry on the front and back.
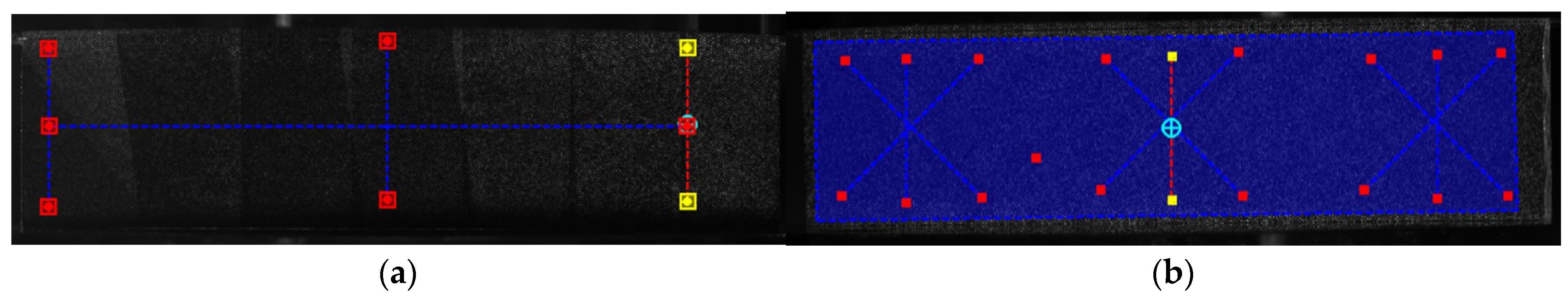
- Perform DIC on the sample’s face while using images from Cam1 and Cam0; region of interest (ROI) visible in Figure 6b.
- Align the data coordinate system with the specimen material direction, i.e., , , vertical (see Figure 6a).
- Calculate strain from the displacements.
- Select a subregion and extract the data; all data in the subregion is averaged giving one value of desired quantities per image, namely: .
- Shear strains reported as tensor shear strain component , need to be doubled for the engineering component.
- Use a speckle pattern compatible with DIC (pen marks would work equally well, per [15]).
- Only perpendicular cameras were used (front = Cam1, back = Cam2).
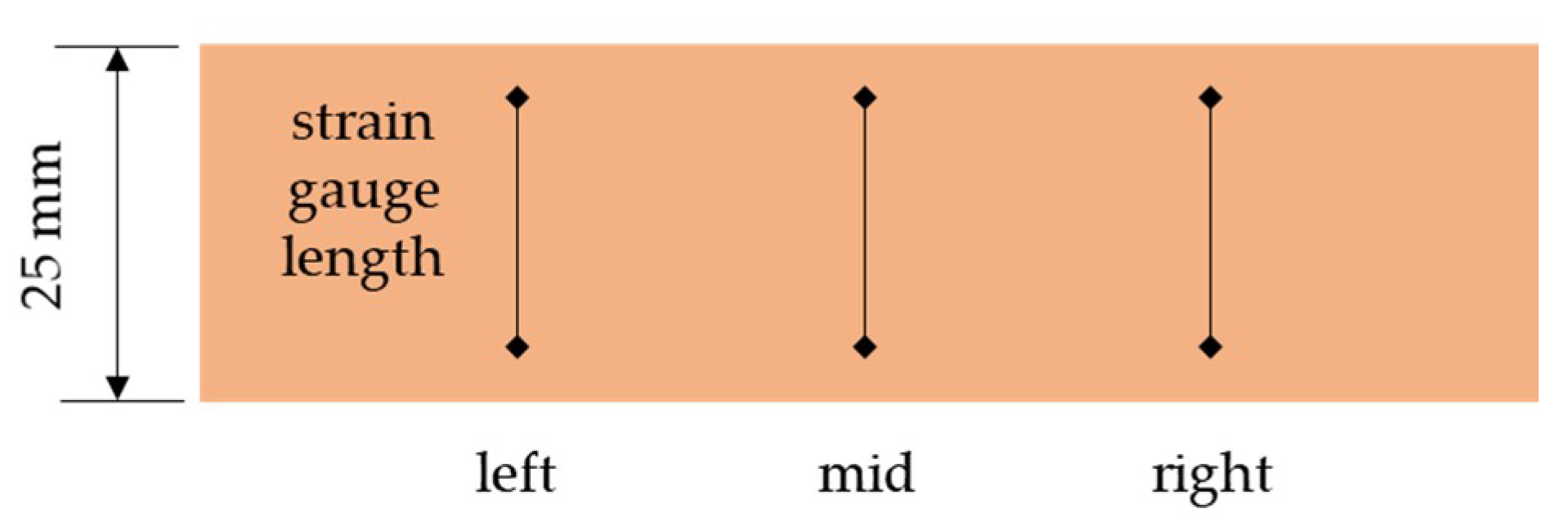
- The three gauges in their respective directions were averaged to produce a single value of strain, i.e., , and in the 45° direction tests, or and in the CD tests.
- All membrane strain is the average of the front and back strains. Ideally, it should be obtained from the trapezoidal distribution of the paperboard cross-section under combined compression/bending. Here, it was simply averaged.
- The shear strain can be calculated from the strain gauge rosette (see Figure 7b): .
2.4. Proposed Method to Identify Matrix A
3. Results
3.1. The ECT Enhanced with Optical Measurement Techniques
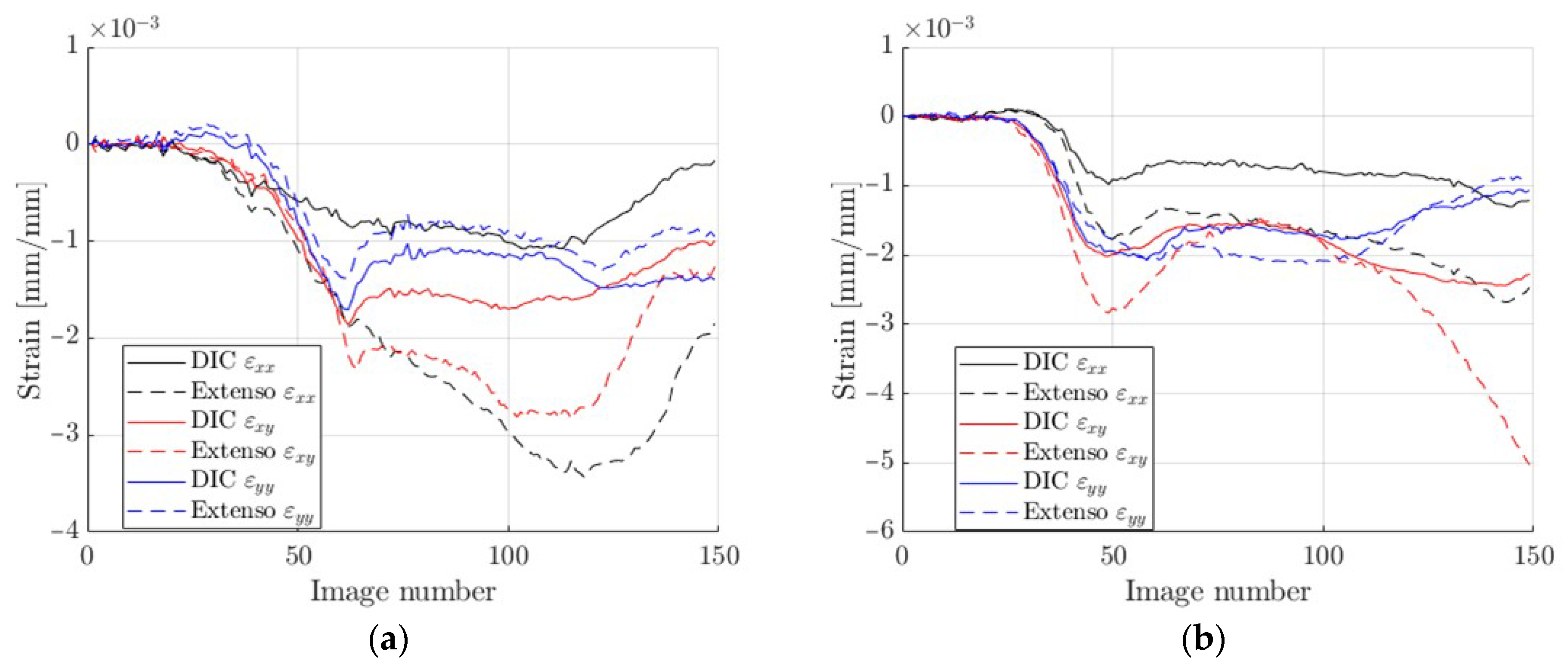
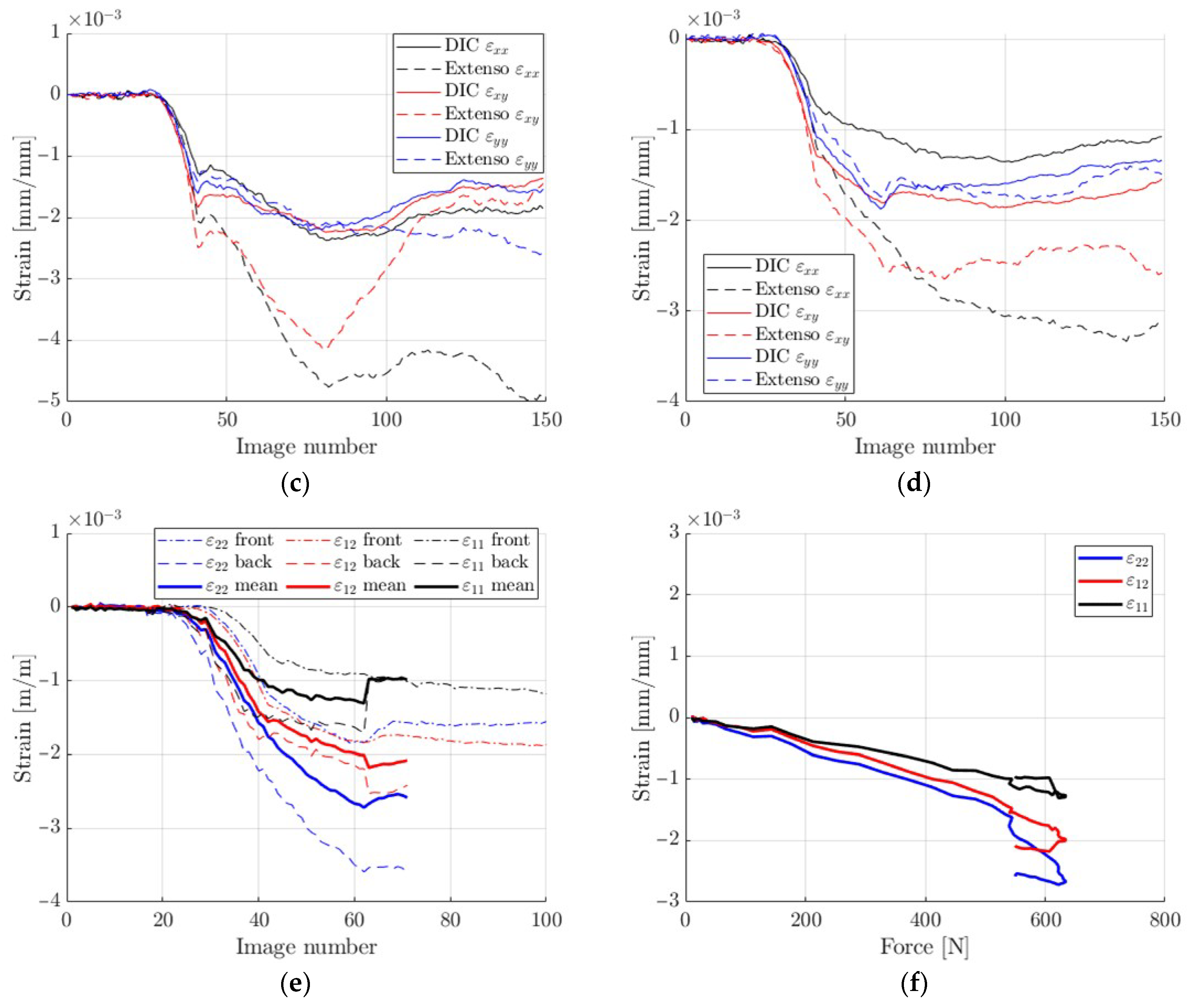
3.2. DIC vs. Extensometry
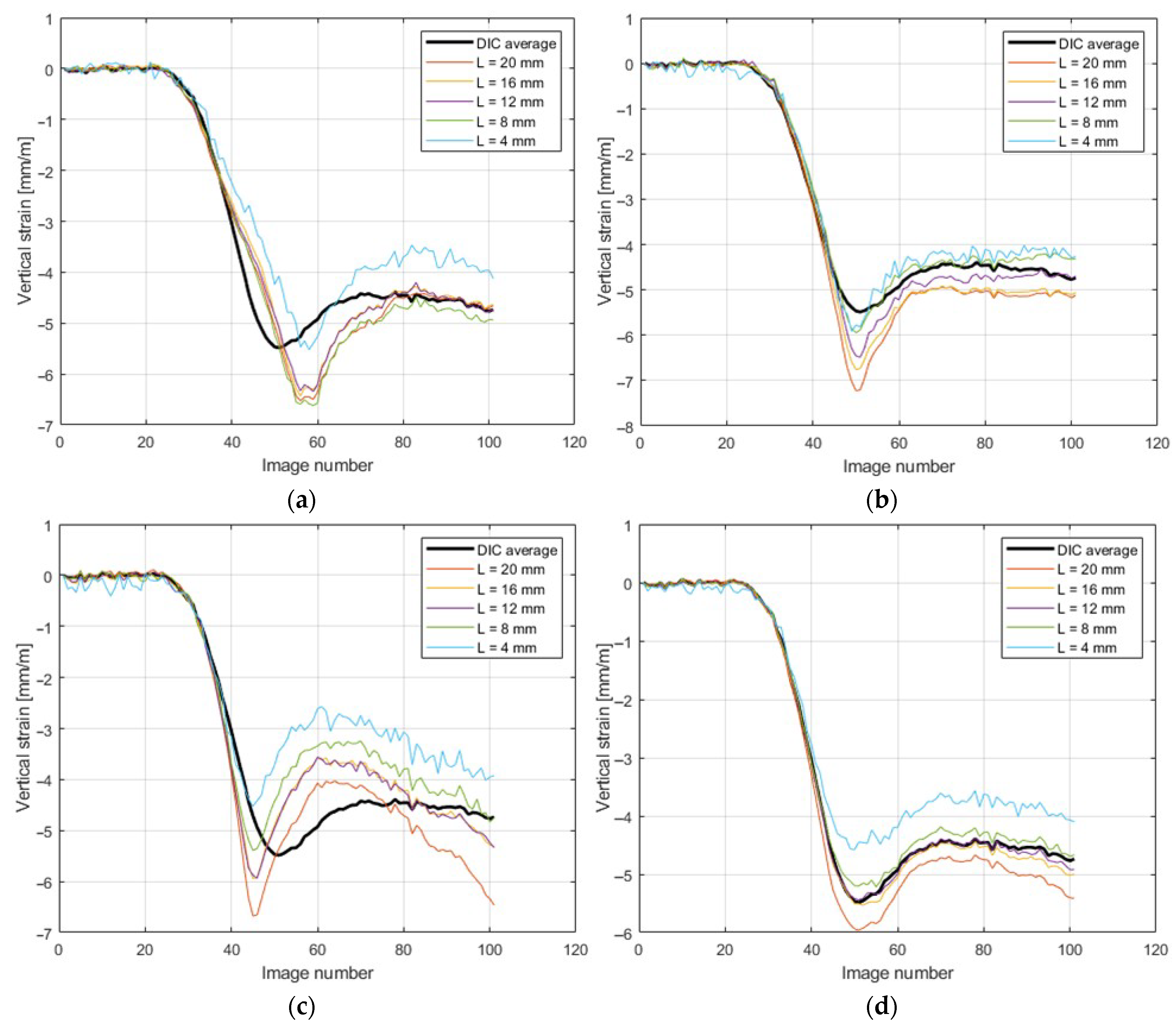
3.3. Length of Virtual Extensometry
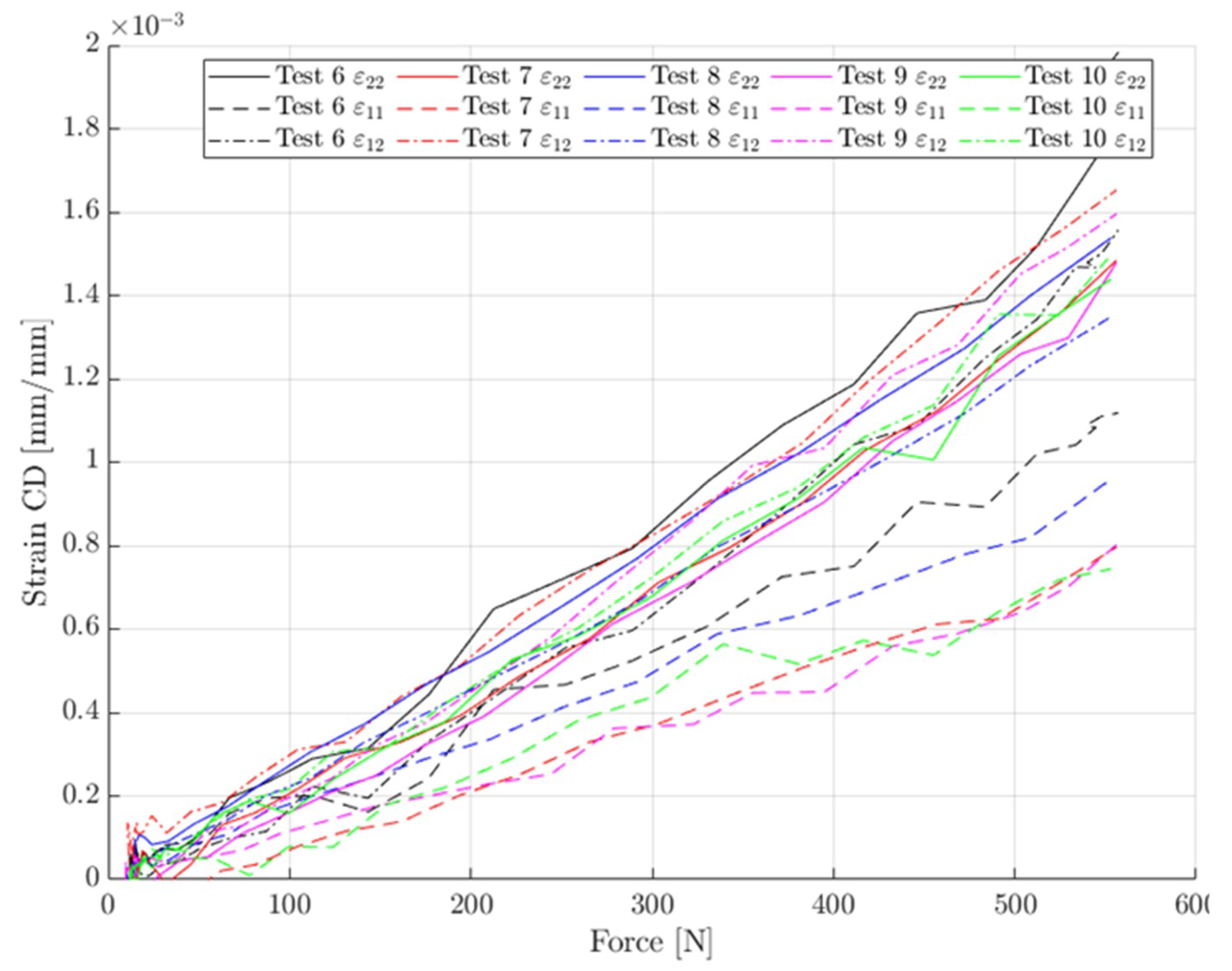
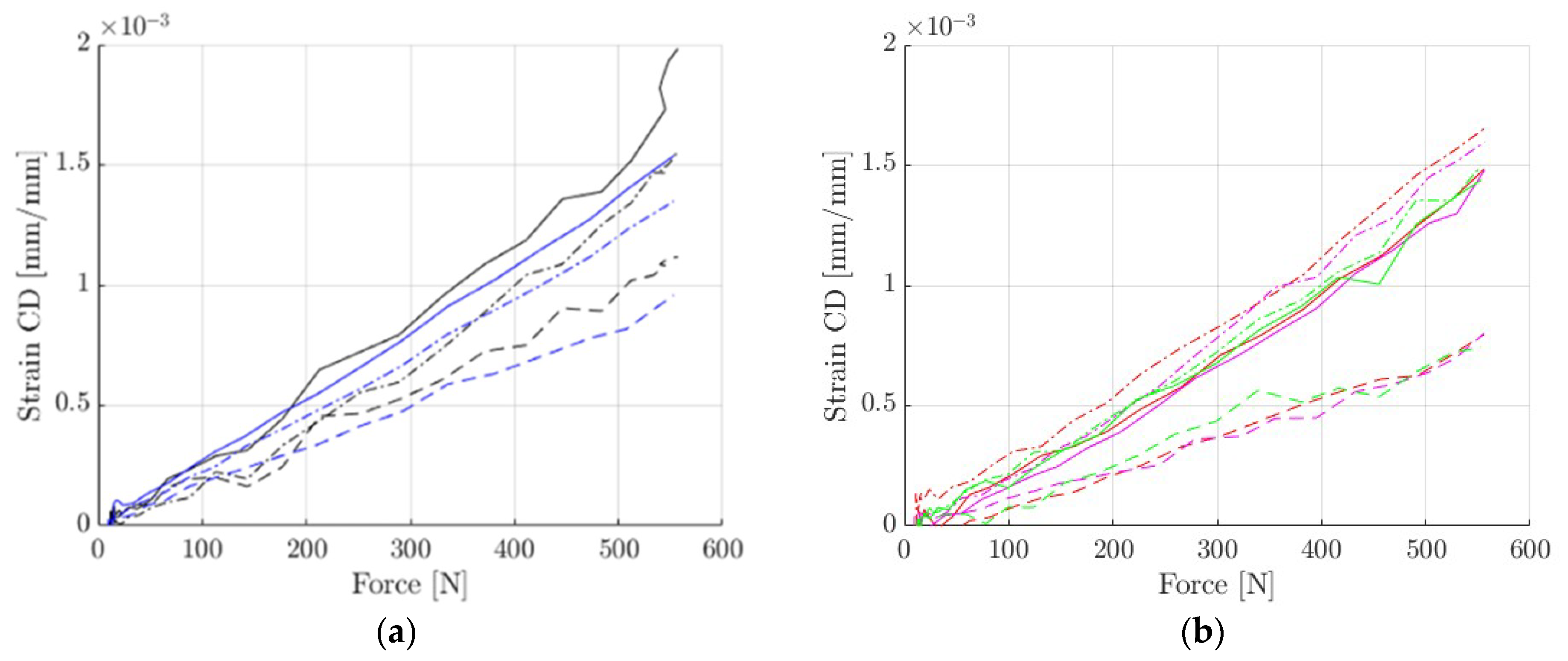
3.4. Consistency of Tests in 45 Deg Direction
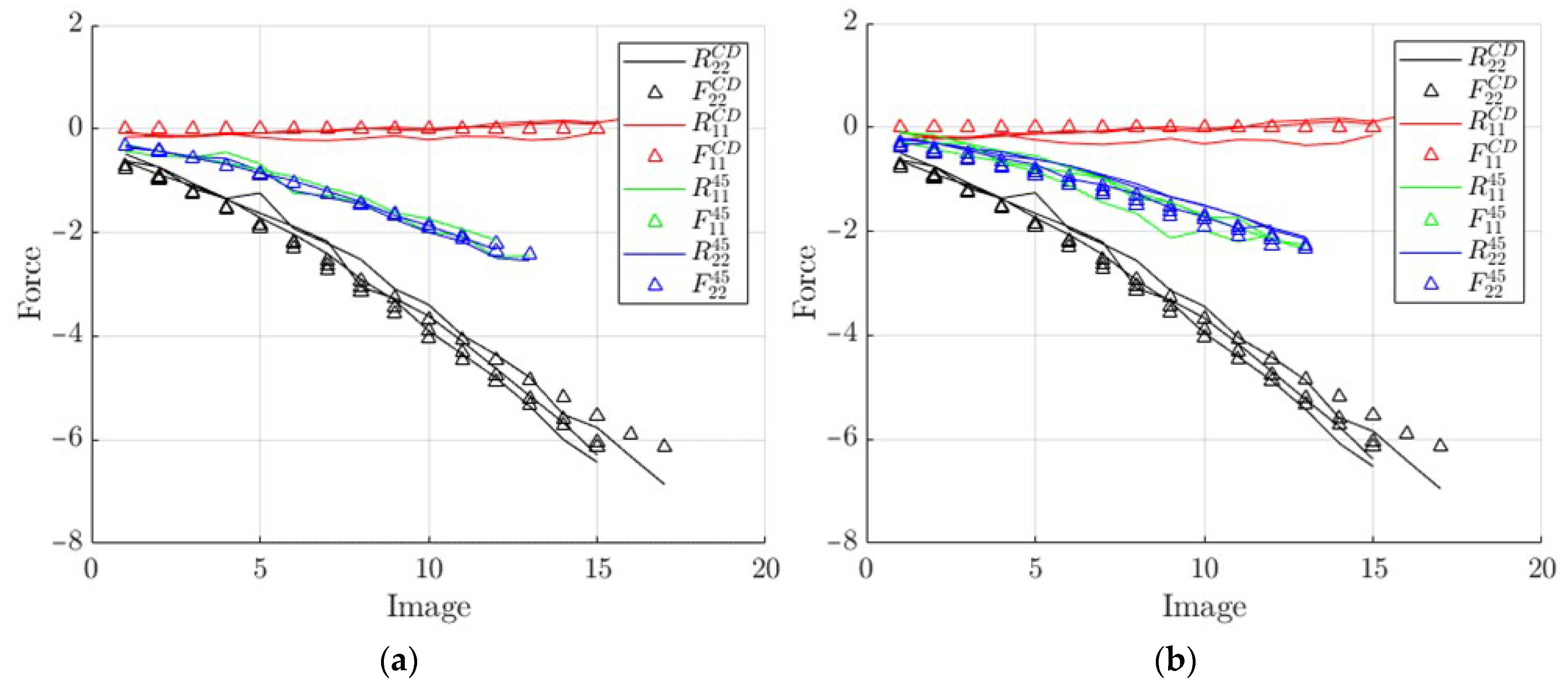
3.5. Full Matrix A Identification
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kellicutt, K.; Landt, E. Development of design data for corrugated fibreboard shipping containers. Tappi J. 1952, 35, 398–402. [Google Scholar]
- Maltenfort, G. Compression strength of corrugated containers. Fibre Contain. 1956, 41, 106–121. [Google Scholar]
- McKee, R.C.; Gander, J.W.; Wachuta, J.R. Compression strength formula for corrugated boxes. Paperboard Packag. 1963, 48, 149–159. [Google Scholar]
- Magnucka-Blandzi, E.; Magnucki, K.; Wittenbeck, L. Mathematical modeling of shearing effect for sandwich beams with sinusoidal corrugated cores. Appl. Math. Model. 2015, 39, 1796–2808. [Google Scholar] [CrossRef]
- Magnucka-Blandzi, E.; Magnucki, K. Transverse shear modulus of elasticity for thin-walled corrugated cores of sandwich beams. Theoretical study. J. Theor. Appl. Mech. 2014, 52, 971–980. [Google Scholar] [CrossRef][Green Version]
- Nordstrand, T.M.; Carlsson, L.A. Evaluation of transverse shear stiffness of structural core sandwich plates. Comp. Struct. 1997, 37, 145–153. [Google Scholar] [CrossRef]
- Garbowski, T.; Gajewski, T.; Grabski, J.K. Role of transverse shear modulus in the performance of corrugated materials. Materials 2020, 13, 3791. [Google Scholar] [CrossRef] [PubMed]
- Garbowski, T.; Gajewski, T.; Grabski, J.K. Torsional and transversal stiffness of orthotropic sandwich panels. Materials 2020, 13, 5016. [Google Scholar] [CrossRef] [PubMed]
- Garbowski, T.; Gajewski, T. Determination of transverse shear stiffness of sandwich panels with a corrugated core by numerical homogenization. Materials 2021, 14, 1976. [Google Scholar] [CrossRef] [PubMed]
- Garbowski, T.; Knitter-Piątkowska, A.; Mrówczyński, D. Numerical homogenization of multi-layered corrugated cardboard with creasing or perforation. Materials 2021, 14, 3786. [Google Scholar] [CrossRef]
- Domaneschi, M.; Perego, U.; Borgqvist, E.; Borsari, R. An industry-oriented strategy for the finite element simulation of paperboard creasing and folding. Packag. Tech. Sci. 2017, 30, 269–294. [Google Scholar] [CrossRef]
- Garbowski, T.; Gajewski, T.; Grabski, J.K. The role of buckling in the estimation of compressive strength of corrugated cardboard boxes. Materials 2020, 13, 4578. [Google Scholar] [CrossRef]
- Garbowski, T.; Gajewski, T.; Grabski, J.K. Estimation of the compressive strength of corrugated cardboard boxes with various openings. Energies 2021, 14, 155. [Google Scholar] [CrossRef]
- Garbowski, T.; Gajewski, T.; Grabski, J.K. Estimation of the compressive strength of corrugated cardboard boxes with various perforations. Energies 2021, 14, 1095. [Google Scholar] [CrossRef]
- Garbowski, T.; Grabski, J.K.; Marek, A. Full-field measurements in the edge crush test of a corrugated board—Analytical and numerical predictive models. Materials 2021, 14, 2840. [Google Scholar] [CrossRef] [PubMed]
- Gajewski, T.; Garbowski, T.; Staszak, N.; Kuca, M. Crushing of double-walled corrugated board and its influence on the load capacity of various boxes. Energies 2021, 14, 4321. [Google Scholar] [CrossRef]
- Kmita-Fudalej, G.; Szewczyk, W.; Kołakowski, Z. Calculation of honeycomb paperboard resistance to edge crush test. Materials 2020, 13, 1706. [Google Scholar] [CrossRef] [PubMed]
- Park, J.; Park, M.; Choi, D.S.; Jung, H.M.; Hwang, S.W. Finite element-based simulation for edgewise compression behavior of corrugated paperboard for packaging of agricultural products. Appl. Sci. 2020, 10, 6716. [Google Scholar] [CrossRef]
- Wong, J.E.; Mustapha, K.B.; Shimizu, Y.; Kamiya, A.; Arumugasamy, S.K. Development of surrogate predictive models for the nonlinear elasto-plastic response of medium density fiberboard-based sandwich structures. Int. J. Lightweight Mater. Manuf. 2021, 4, 302–314. [Google Scholar]
- Marek, A.; Garbowski, T. Homogenization of sandwich panels. Comput. Assist. Methods Eng. Sci. 2015, 22, 39–50. [Google Scholar]
- Hohe, J. A direct homogenization approach for determination of the stiffness matrix for microheterogeneous plates with application to sandwich panels. Compos. Part. B 2003, 34, 615–626. [Google Scholar] [CrossRef]
- FEMat Systems. Available online: http://fematsystems.pl/home_en (accessed on 25 April 2021).
- TAPPI T 839 om-12. Edge Compression Test for Strength of Corrugated Fiberboard Using the Clamp Method (Short Column Test); TAPPI: Peachtree Corners, GA, USA, 2009. [Google Scholar]
- TAPPI T 838 cm-12. Edge Crush Test Using Neckdown; TAPPI: Peachtree Corners, GA, USA, 2009. [Google Scholar]
- FEFCO NO.8. Edgewise Crush Resistance of Corrugated Fiberboard; FEFCO: Brussel, Belgium, 1997. [Google Scholar]
- ISO 3037:2013. Corrugated Fibreboard—Determination of Edgewise Crush Resistance (Unwaxed Edge Method); ISO: Geneva, Switzerland, 2013. [Google Scholar]
- TAPPI T 811 om-11. Edgewise Compressive Strength of Corrugated Fibreboard (Short Column Test); TAPPI: Peachtree Corners, GA, USA, 2009. [Google Scholar]
- ISO 13821:2002. Corrugated Fibreboard—Determination of Edgewise Crush Resistance—Waxed Edge Method; ISO: Geneva, Switzerland, 2002. [Google Scholar]
- Hägglund, R.; Åslund, P.E.; Carlsson, L.A.; Isaksson, P. Measuring thickness changes of edgewise compression loaded corrugated board panels using digital image correlation. J. Sandw. Struct. Mater. 2010, 14, 75–94. [Google Scholar] [CrossRef]
- Viguié, J.; Dumont, P.J.J.; Vacher, P.; Orgéas, L.; Desloges, I.; Mauret, E. Analysis of the strain and stress field of cardboard box during compression by 3D Digital Image Correlation. Appl. Mech. Mater. 2010, 24–25, 103–108. [Google Scholar] [CrossRef]
- Viguié, J.; Dumont, P.J.J.; Orgéas, L.; Vacher, P.; Desloges, I.; Mauret, E. Surface stress and strain fields on compressed panels of corrugated board boxes. An experimental analysis by using Digital Image Stereocorrelation. Comp. Struct. 2011, 93, 2861–2873. [Google Scholar] [CrossRef]
- Viguié, J.; Dumont, P.J.J. Analytical post-buckling model of corrugated board panels using digital image correlation measurements. Comp. Struct. 2013, 101, 243–254. [Google Scholar] [CrossRef]
- Borgqvist, E.; Lindström, T.; Tryding, J.; Wallin, M.; Ristinmaa, M. Distortional hardening plasticity model for paperboard. Int. J. Solids Struct. 2014, 51, 2411–2423. [Google Scholar] [CrossRef]
- Cocchetti, G.; Mahini, M.R.; Maier, G. Mechanical characterization of foils with compression in their planes. Mech. Adv. Mater. Struct. 2014, 21, 853–870. [Google Scholar] [CrossRef]
- Garbowski, T.; Maier, G.; Novati, G. On calibration of orthotropic elastic-plastic constitutive models for paper foils by biaxial tests and inverse analyses. Struct. Multidisc. Optim. 2012, 46, 111–128. [Google Scholar] [CrossRef]
- Considine, J.M.; Pierron, F.; Turner, K.T.; Vahey, D.W. General anisotropy identification of paperboard with virtual fields method. Exp. Mech. 2014, 54, 1395–1410. [Google Scholar] [CrossRef]
- Åslund, P.E.; Hägglund, R.; Carlsson, L.A.; Isaksson, P. An analysis of strain localization and formation of face wrinkles in edge-wise loaded corrugated sandwich panels using a continuum damage model. Int. J. Solids Struct. 2015, 56–57, 248–257. [Google Scholar] [CrossRef]
- Zappa, E.; Liu, R.; Bolzon, G.; Shahmardani, M. High resolution non-contact measurement techniques for three-dimensional deformation processes of paperboard laminates. Mater. Today Proc. 2017, 4, 5872–5876. [Google Scholar] [CrossRef]
- Fadiji, T.; Coetzee, C.J.; Opara, U.L. Evaluating the displacement field of paperboard packages subjected to compression loading using digital image correlation (DIC). Food Bioprod. Process. 2020, 123, 60–71. [Google Scholar] [CrossRef]
- Maier, G.; Bolzon, G.; Buljak, V.; Garbowski, T.; Miller, B. Synergic Combinations of Computational Methods and Experiments for Structural Diagnoses. In Computer Methods in Mechanics; Advanced Structured Materials; Kuczma, M., Wilmanski, K., Eds.; Springer: Berlin/Heidelberg, Germany, 2010; Volume 1, pp. 453–476. [Google Scholar]






| Wave (Flute) | Pitch (mm) | Height (mm) | Take-Up Ratio (–) |
|---|---|---|---|
| E | 3.50 | 1.18 | 1.242 |
| B | 6.48 | 2.5 | 1.315 |
| Layer Name | Thickness () | |||
|---|---|---|---|---|
| TLWC 140 | 180 | 725 | 323 | 2.32 |
| W 100 | 160 | 886 | 328 | 1.76 |
| TLW 120 | 170 | 907 | 313 | 1.81 |
| Test ID | E—Optical (N/mm) | E—Crosshead (N/mm) | ECT (N/mm) |
|---|---|---|---|
| 1447.45 | 441.82 | −7.548 | |
| 1380.25 | 536.82 | −7.151 | |
| 1531.96 | 450.66 | −7.609 | |
| 1615.12 | 611.39 | −7.640 | |
| Mean (N/mm) | 1493.70 | 510.17 | −7.487 |
| Std (N/mm) | 102.01 | 79.93 | 0.227 |
| Cov (%) | 6.829 | 15.668 | −3.038 |
| Parameter: | Test 2 and 6 | Group 1 | Group 2 |
|---|---|---|---|
| (N/mm) | 2581 | 2583.0 | 3554.0 |
| (N/mm) | 158 | 103.5 | 158.1 |
| (N/mm) | 1674 (1500 1) | 1765.0 | 1792.0 |
| (N/mm) | 1078 | 1061.0 | 946.0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Garbowski, T.; Knitter-Piątkowska, A.; Marek, A. New Edge Crush Test Configuration Enhanced with Full-Field Strain Measurements. Materials 2021, 14, 5768. https://doi.org/10.3390/ma14195768
Garbowski T, Knitter-Piątkowska A, Marek A. New Edge Crush Test Configuration Enhanced with Full-Field Strain Measurements. Materials. 2021; 14(19):5768. https://doi.org/10.3390/ma14195768
Chicago/Turabian StyleGarbowski, Tomasz, Anna Knitter-Piątkowska, and Aleksander Marek. 2021. "New Edge Crush Test Configuration Enhanced with Full-Field Strain Measurements" Materials 14, no. 19: 5768. https://doi.org/10.3390/ma14195768
APA StyleGarbowski, T., Knitter-Piątkowska, A., & Marek, A. (2021). New Edge Crush Test Configuration Enhanced with Full-Field Strain Measurements. Materials, 14(19), 5768. https://doi.org/10.3390/ma14195768








