In Situ Observation of the Tensile Deformation and Fracture Behavior of Ti–5Al–5Mo–5V–1Cr–1Fe Alloy with Different Microstructures
Abstract
:1. Introduction
2. Materials and Methods
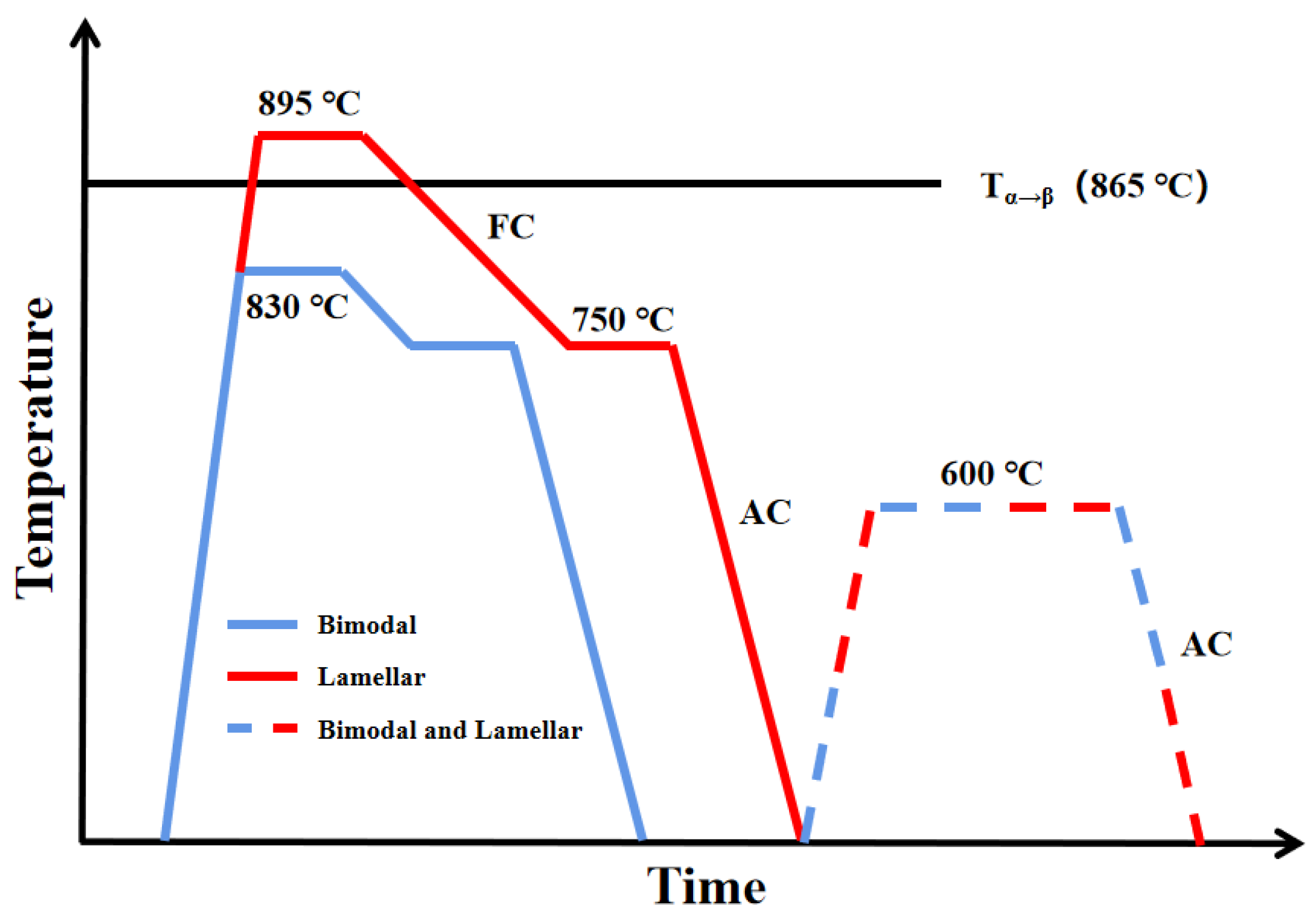
2.1. Materials
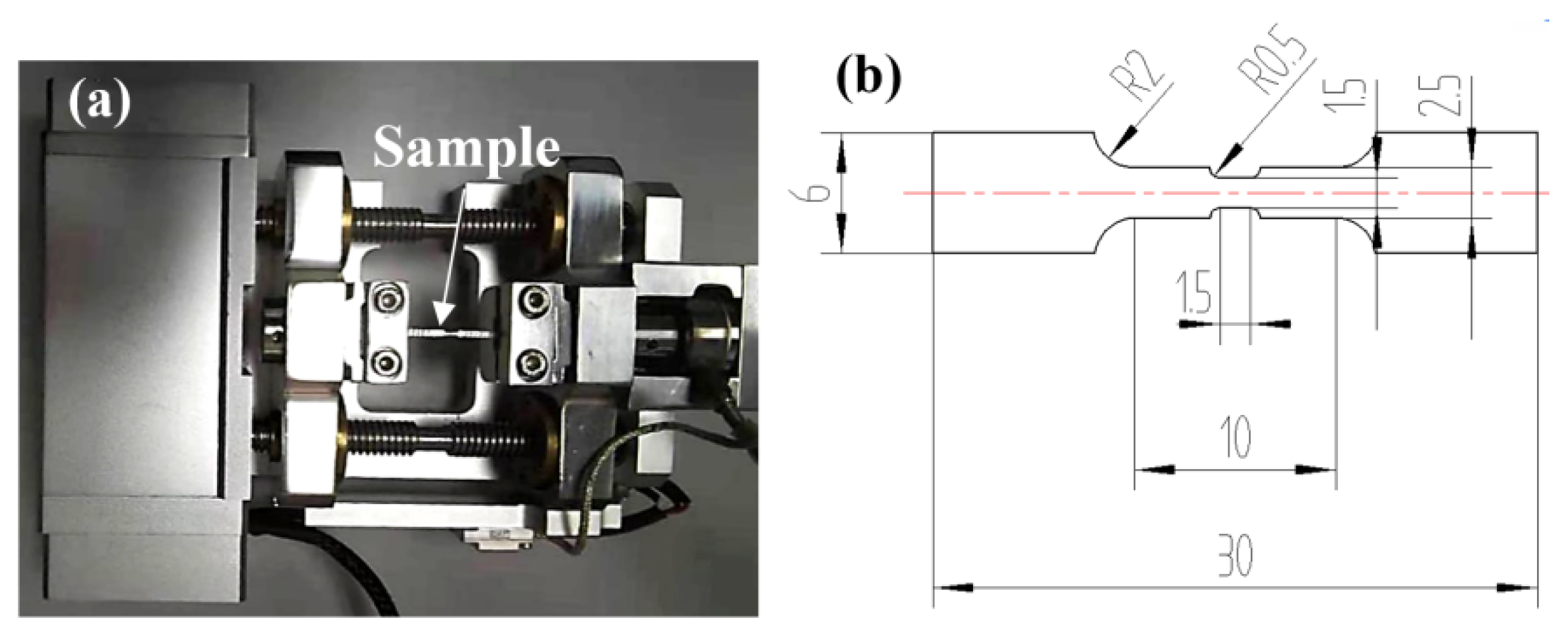
2.2. In Situ Tensile Test
3. Results
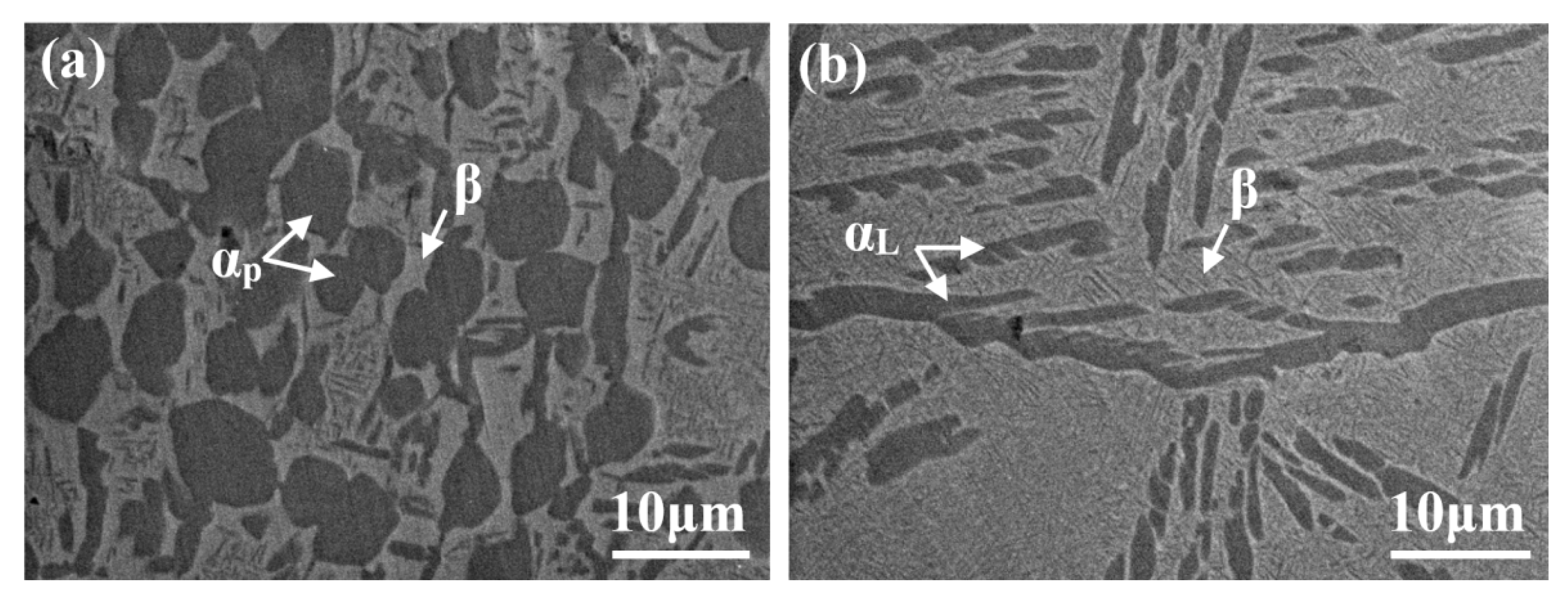
3.1. Microstructure of As-Heat-Treated Samples
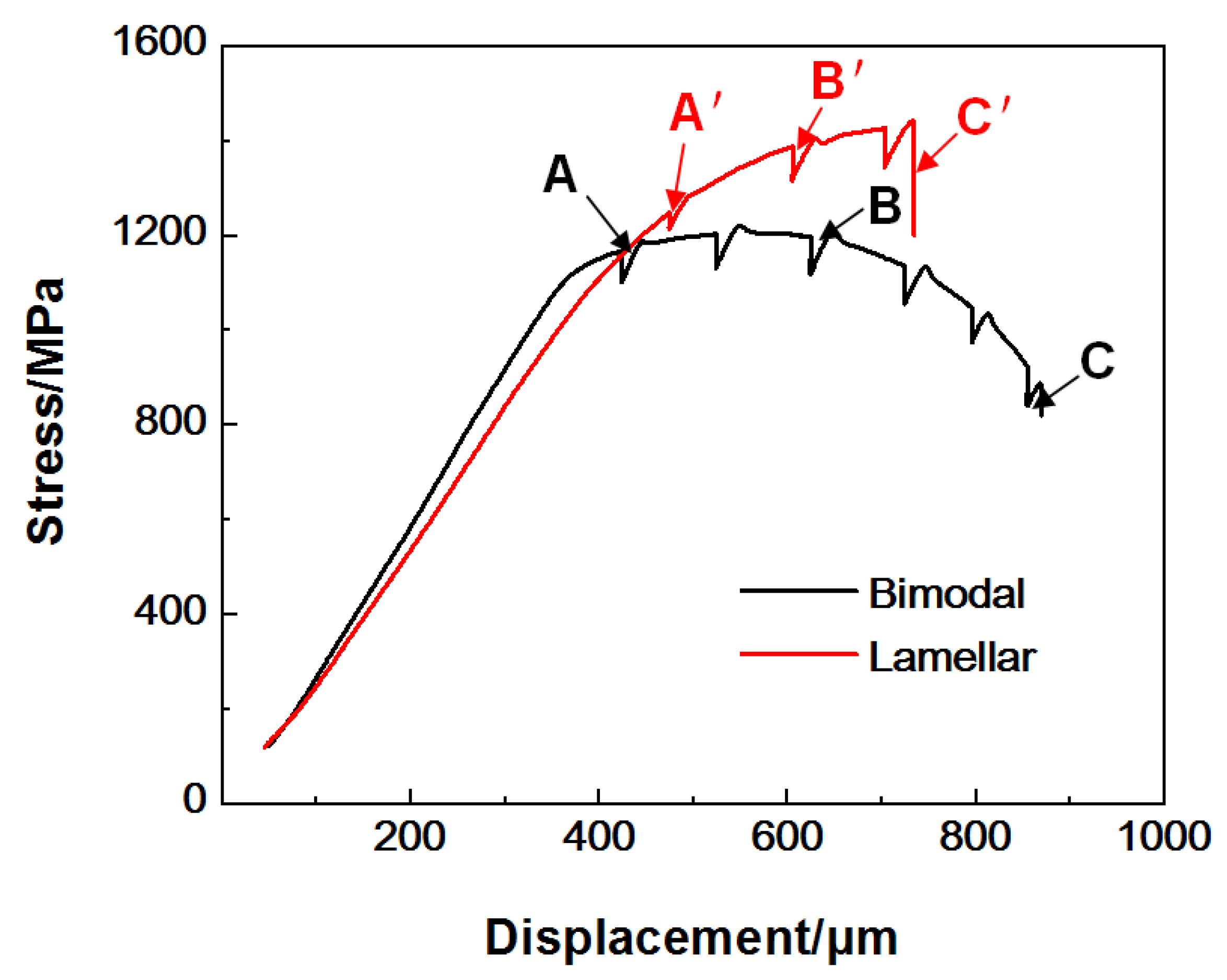
3.2. The Stress–Displacement Curves during In Situ Testing
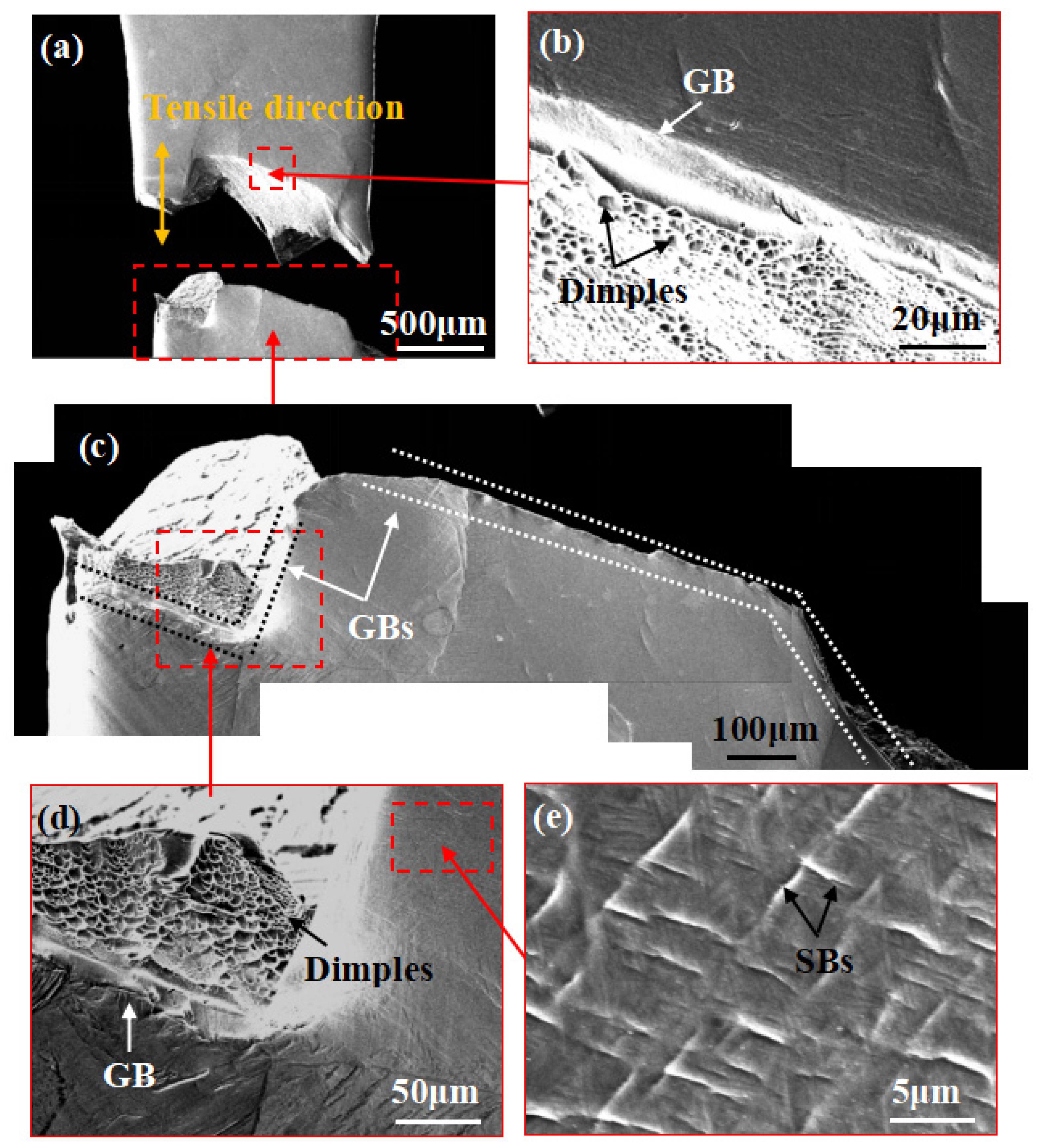
3.3. Microstructure Evolution during In Situ Stretching
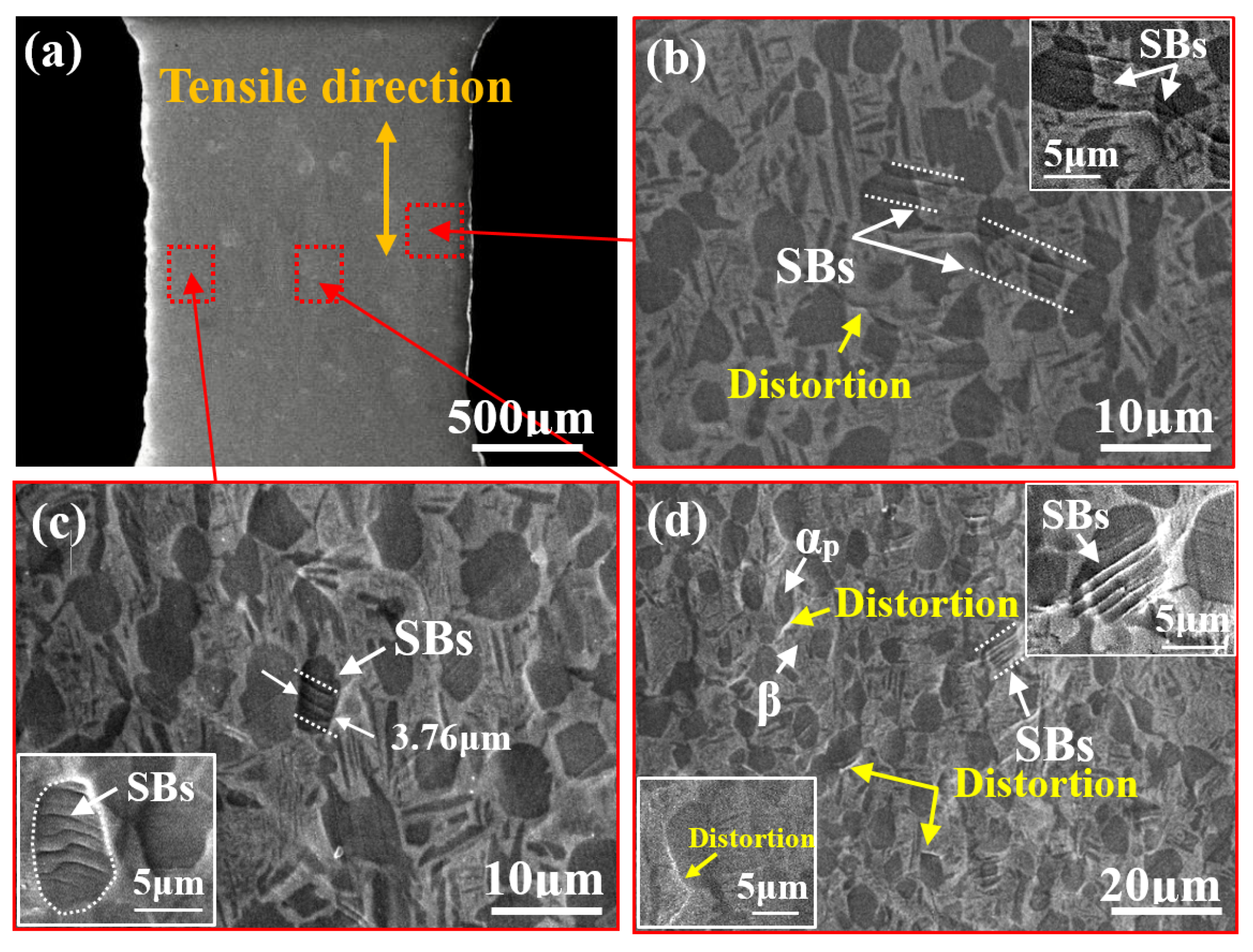
3.3.1. Microstructure Evolution of Bimodal Microstructure
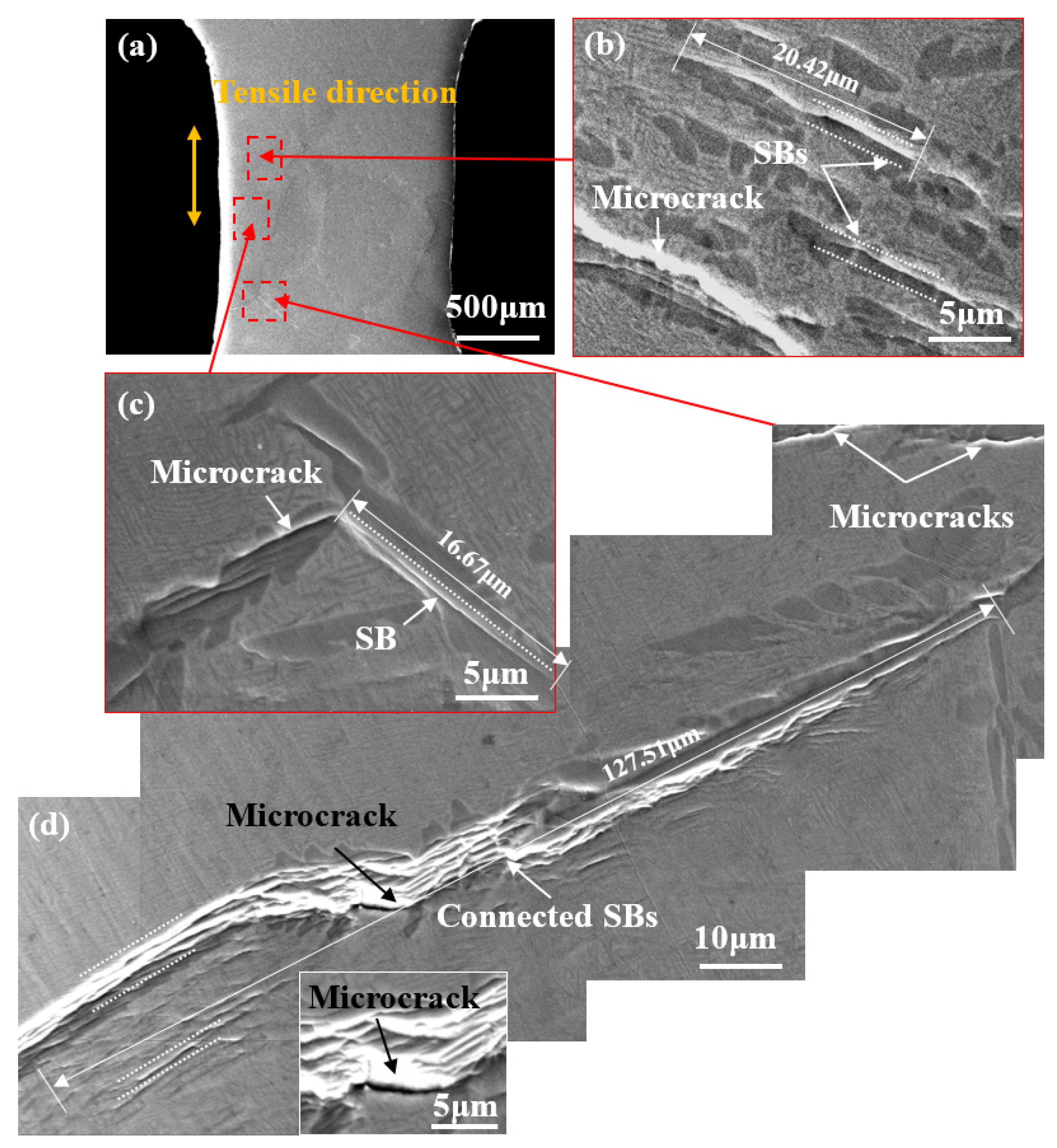
3.3.2. Evolution of the Lamellar Microstructure
4. Discussion
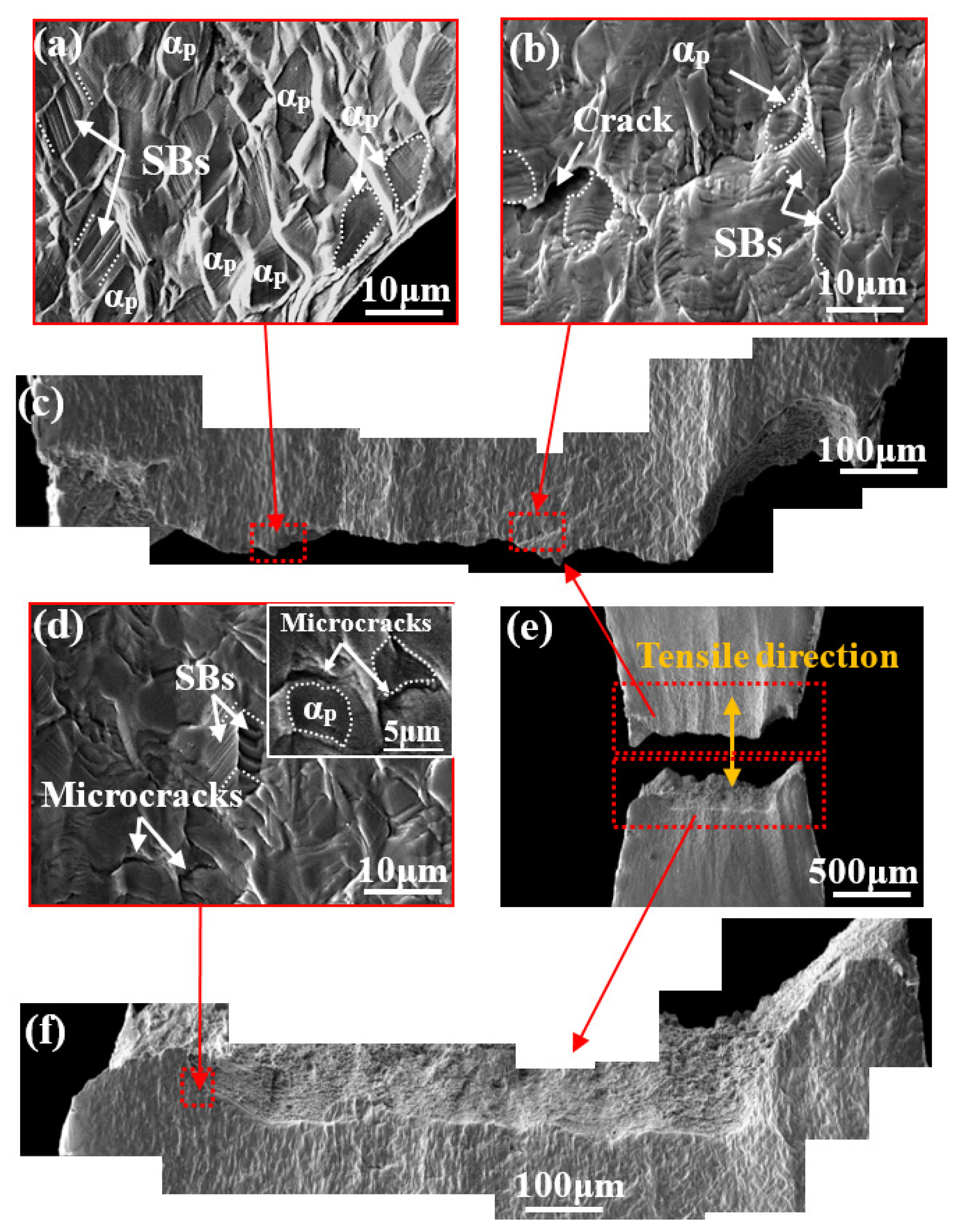
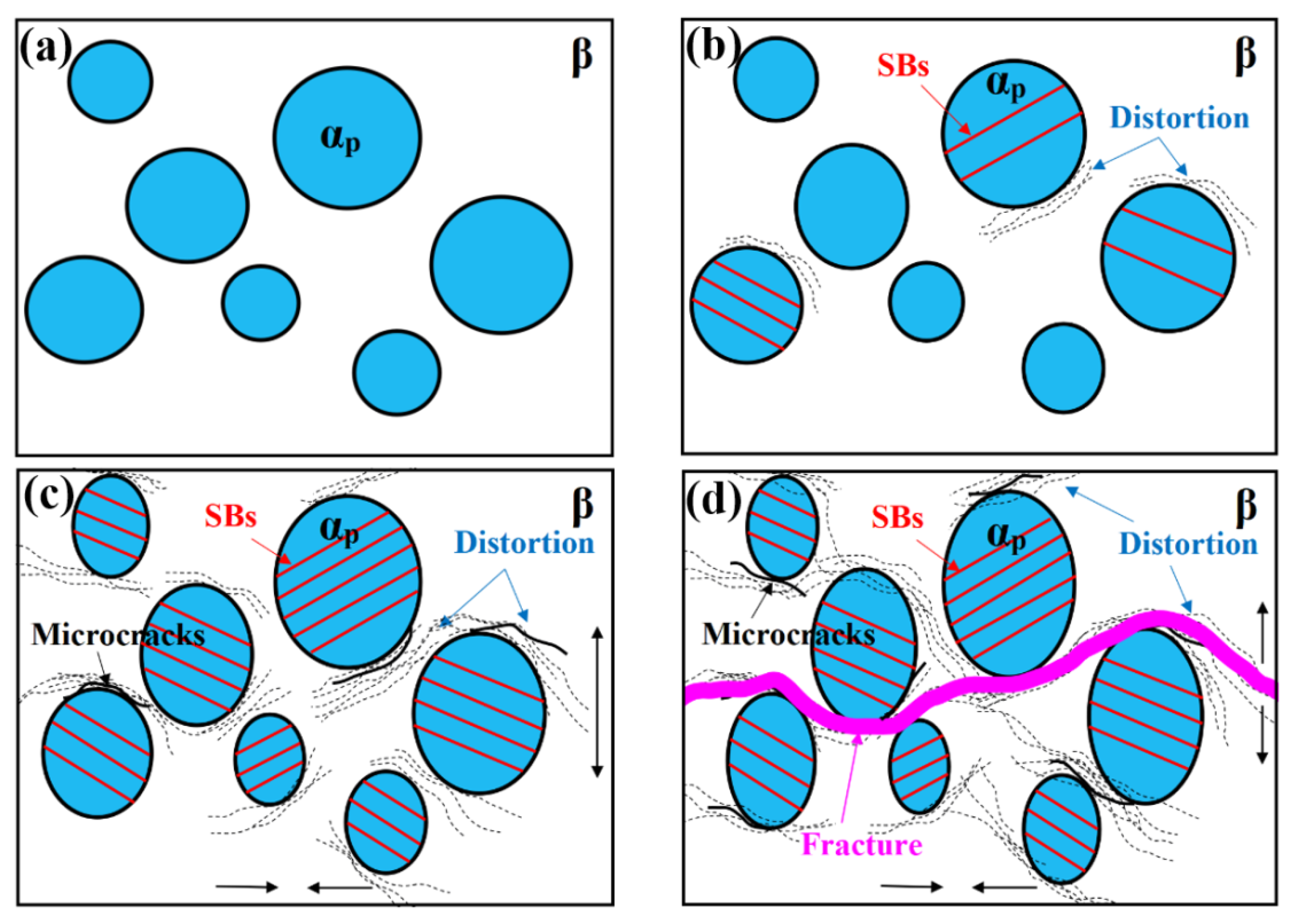
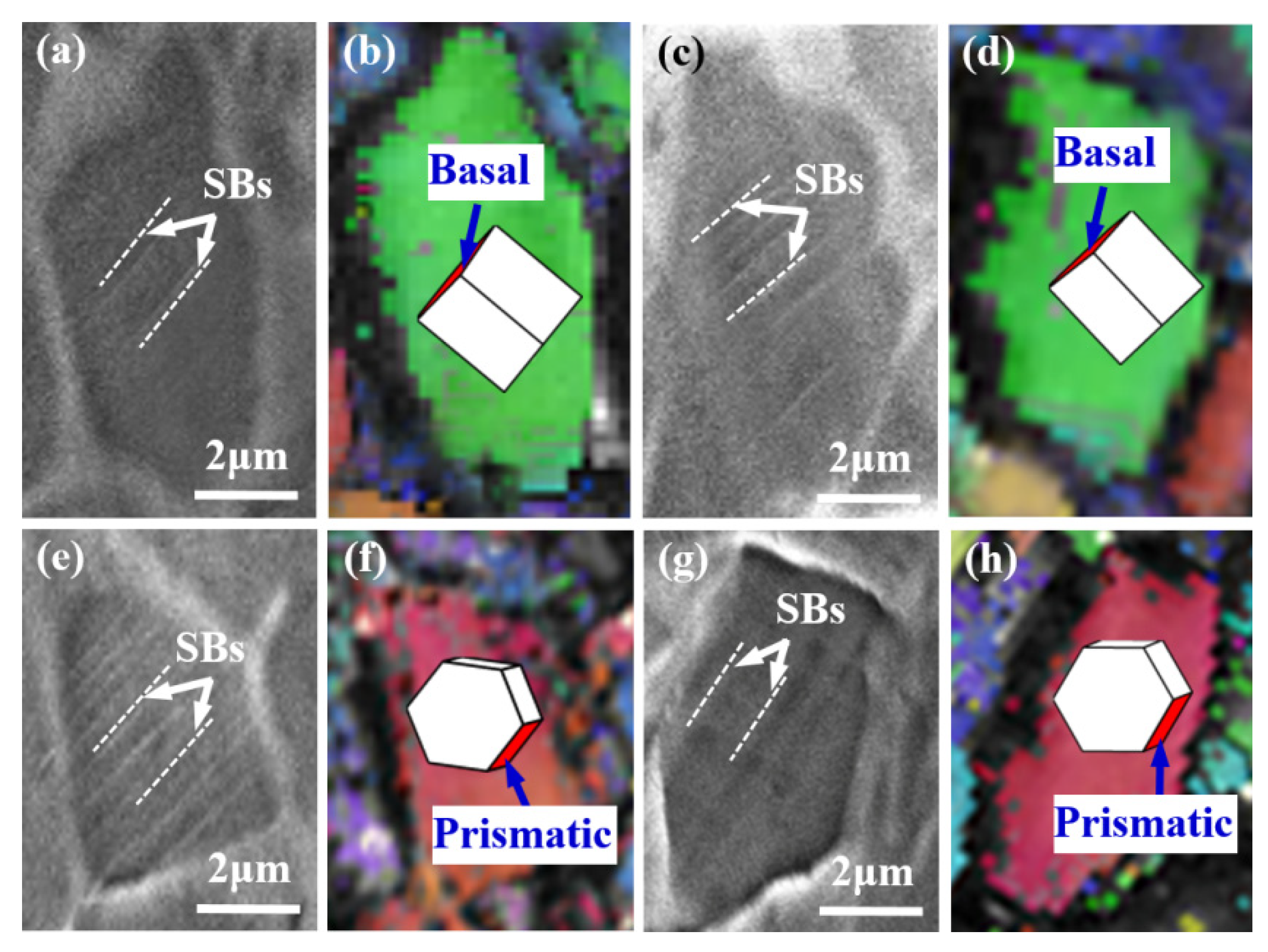
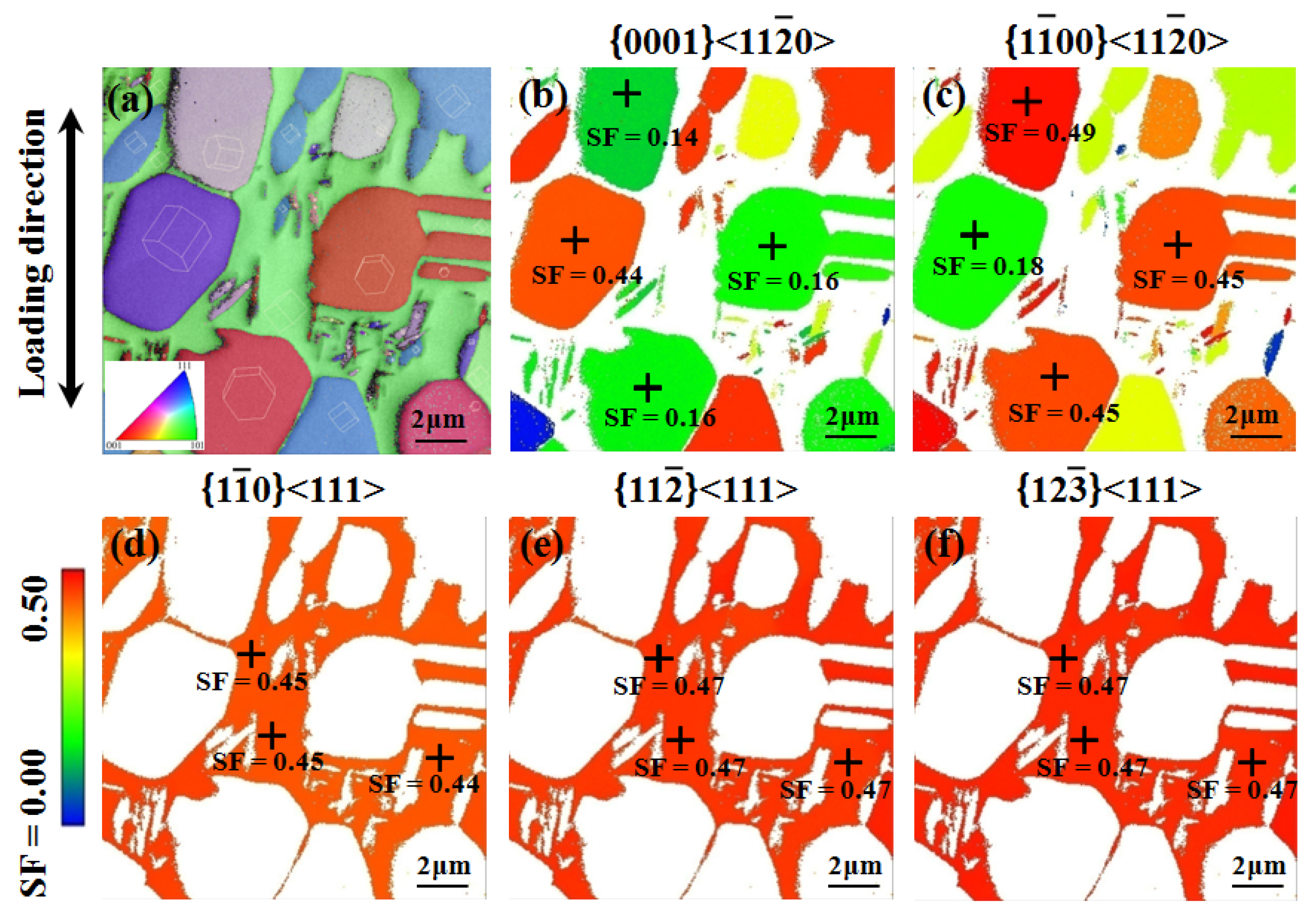
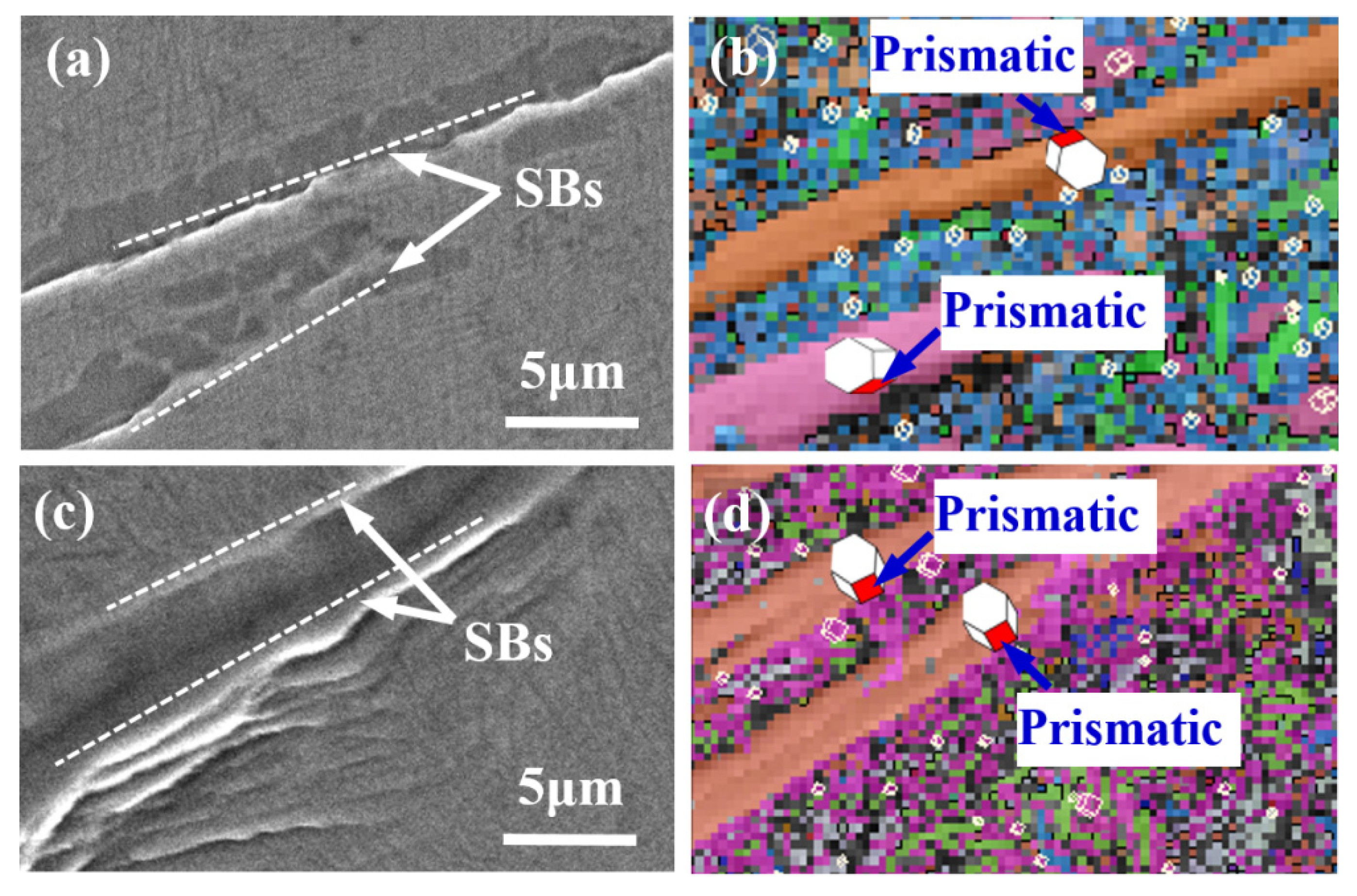
4.1. Deformation Mechanisms and Microstructural Evolution of the Bimodal Microstructure
4.2. Deformation Mechanisms and Microstructural Evolution of the Lamellar Microstructure
5. Conclusions
- For the bimodal microstructure, parallel and deep SBs, at around 45° to the tensile direction, are first observed in the αp phase due to the limited slip systems therein and the stress concentration caused by its large size. These SBs mainly correspond to prismatic or basal slip systems. With increasing strain, distortion at the αp/β interface arises, leading to the evolution of microcracks: the interconnection of the microcracks at the αp/β interface finally leads to the fracturing of the bimodal microstructure.
- For the lamellar microstructure, parallel and deep SBs are first observed in coarse αL phase grains or its interfaces at GBs, making an angle of around 45° to the tensile direction. With increasing strain, these SBs grow along the length of the αL phase and gradually interconnect, thus forming microcracks. The lamellar microstructure finally fractures along the αL phase at GBs through the interconnection of those microcracks in the αL phase.
- Due to the connected distribution of β and isolated distribution of the αp phase in the bimodal microstructure, the localized deformation readily propagates into the surrounding area through β, which is softer and has a better ability to undergo plastic deformation. This results in the more uniform deformation and higher ductility of specimens with a bimodal microstructure.
- The coarse αL phase at the GBs greatly restricts the deformation in the β matrix during tensile loading, which in turn produces a significant stress concentration and local deformation in the coarse αL phase. This finally leads to intergranular fracture and contributes to the higher strength and lower ductility of those specimens with a lamellar microstructure.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gao, F.Y.; Guo, Y.F.; Yang, S.L.; Yu, Y.; Yu, W. Fatigue properties of friction stir welded joint of titanium alloy. Mater. Sci. Eng. A 2020, 793, 139819. [Google Scholar] [CrossRef]
- Vitus, M.T.; Li, C.; Wang, S.F.; Li, J.W.; Xu, X.J. Mechanical properties of near alpha titanium alloys for high–temperature applications—A review. Aircr. Eng. Aerosp. Technol. 2020, 92, 521–540. [Google Scholar]
- Chen, W.; Li, C.; Zhang, X.Y.; Chen, C.; Lin, Y.C.; Zhou, K.C. Deformation−induced variations in microstructure evolution and mechanical properties of bi−modal Ti−55511 titanium alloy. J. Alloys Compd. 2019, 783, 709–717. [Google Scholar] [CrossRef]
- Zhang, D.D.; Liu, N.; Chen, Y.Y.; Zhang, G.Q.; Tian, J.; Kong, F.T.; Xiao, S.L.; Sun, J.F. Microstructure Evolution and Mechanical Properties of PM-Ti43Al9V0.3Y Alloy. Materials 2020, 13, 198. [Google Scholar] [CrossRef] [Green Version]
- Di, W.; Tian, Y.Y.; Zhang, L.G.; Wang, Z.Y.; Sheng, J.W.; Wang, W.L.; Zhou, K.C.; Liu, L.B. Optimal Design of High–Strength Ti–Al–V–Zr Alloys through a Combinatorial Approach. Materials 2018, 11, 1603. [Google Scholar]
- Shao, H.; Shan, D.; Wang, K.X.; Zhang, G.J.; Zhao, Y.Q. Massive α precipitation selectivity and tensile fracture behavior of TC18 alloy. J. Alloys Compd. 2019, 797, 10–17. [Google Scholar] [CrossRef]
- Li, C.; Chen, J.; Li, W.; He, J.J.; Qin, W.; Ren, Y.J.; Chen, J.L.; Chen, J.H. Study on the relationship between microstructure and mechanical property in a metastable β titanium alloy. J. Alloys Compd. 2015, 627, 222–230. [Google Scholar] [CrossRef]
- Cui, N.; Wu, Q.Q.; Bi, K.X.; Xu, T.W.; Kong, F.T. Effect of Heat Treatment on Microstructures and Mechanical Properties of a Novel β-Solidifying TiAl Alloy. Materials 2019, 12, 1672. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yadav, P.; Saxena, K.K. Effect of heat−treatment on microstructure and mechanical properties of Ti alloys: An overview. Mater. Today Proc. 2020, 26, 2546–2557. [Google Scholar] [CrossRef]
- Zhu, W.G.; Sun, Q.Y.; Tan, C.S.; Li, P.; Xiao, L.; Sun, J. Tensile brittleness and ductility improvement in a novel metastable β titanium alloy with lamella structure. J. Alloys Compd. 2020, 827, 154311. [Google Scholar] [CrossRef]
- Srinivasu, G.; Natraj, Y.; Bhattacharjee, A.; Nandy, T.K.; Rao, G.V.S.N. Tensile and fracture toughness of high strength β Titanium alloy, Ti–10V–2Fe–3Al, as a function of rolling and solution treatment temperatures–ScienceDirect. Mater. Des. 2013, 47, 323–330. [Google Scholar] [CrossRef]
- Kar, S.K.; Suman, S.; Shivaprasad, S.; Chaudhuri, A.; Bhattacharjee, A. Processing–microstructure–yield strength correlation in a near β Ti alloy, Ti–5Al–5Mo–5V–3Cr. Mater. Sci. Eng. A 2014, 610, 171–180. [Google Scholar] [CrossRef]
- Wu, G.Q.; Shi, C.L.; Sha, W.; Sha, A.X.; Jiang, H.R. Microstructure and high cycle fatigue fracture surface of a Ti–5Al–5Mo–5V–1Cr–1Fe titanium alloy. Mater. Sci. Eng. A 2013, 575, 111–118. [Google Scholar] [CrossRef] [Green Version]
- Qin, D.Y.; Li, Y.L. The role of microstructure and stress state in dynamic mechanical behavior of Ti−5Al−5V−5Mo−3Cr alloy. Mater. Charact. 2018, 147, 421–433. [Google Scholar] [CrossRef]
- Zheng, C.; Wang, F.C.; Cheng, X.W.; Fu, K.Q.; Liu, J.X.; Wang, Y.F.; Liu, T.T.; Zhu, Z.X. Effect of microstructures on ballistic impact property of Ti-6Al-4V targets. Mater. Sci. Eng. A 2014, 608, 53–62. [Google Scholar] [CrossRef]
- Wu, D.; Liu, L.B.; Zhang, L.G.; Wang, W.L.; Zhou, K.C. Tensile deformation mechanism and micro−void nucleation of Ti−55531 alloy with bimodal microstructure. J. Mater. Res. Technol. 2020, 9, 15442–15453. [Google Scholar] [CrossRef]
- Qin, D.Y.; Li, Y.L.; Zhang, S.Y.; Zhou, L. On the tensile embrittlement of lamellar Ti–5Al–5V–5Mo–3Cr alloy. J. Alloys Compd. 2016, 663, 581–593. [Google Scholar] [CrossRef]
- Zhao, Q.Y.; Yang, F.; Torrens, R.; Bolzoni, L. In-situ observation of the tensile deformation and fracture behaviour of powder–consolidated and as–cast metastable beta titanium alloys. Mater. Sci. Eng. A 2019, 750, 45–59. [Google Scholar] [CrossRef]
- Wan, X.; Zhu, K.; Xu, Y.J.; Han, B.S.; Jing, T. In-Situ Observation of Fracture Behavior of Ti–Aluminide Multi–Layered Composites Produced by a Hybrid Sintering Process. Materials 2019, 12, 1568. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Huang, S.X.; Zhao, Q.Y.; Lin, C.; Wu, C.; Zhao, Y.Q.; Jia, W.J.; Mao, C.L. In-situ investigation of tensile behaviors of Ti–6Al alloy with extra low interstitial. Mater. Sci. Eng. A 2021, 809, 140958. [Google Scholar] [CrossRef]
- Zhang, S.F.; Zeng, W.D.; Zhao, Q.Y.; Ge, L.L.; Zhang, M. In situ SEM study of tensile deformation of a near–β titanium alloy. Mater. Sci. Eng. A 2017, 708, 574–581. [Google Scholar] [CrossRef]
- Shao, H.; Zhao, Y.Q.; Ge, P.; Zeng, W.D. In-situ SEM observations of tensile deformation of the lamellar microstructure in TC21 titanium alloy. Mater. Sci. Eng. A 2013, 559, 515–519. [Google Scholar] [CrossRef]
- Huang, J.; Wang, Z.R.; Xue, K.M. Cyclic deformation response and micromechanisms of Ti alloy Ti–5Al–5V–5Mo–3Cr–0.5Fe. Mater. Sci. Eng. A 2011, 528, 8723–8732. [Google Scholar] [CrossRef]
- Tan, C.S.; Sun, Q.Y.; Xiao, L.; Zhao, Y.Q.; Sun, J. Characterization of deformation in primary α phase and crack initiation and propagation of TC21 alloy using in-situ SEM experiments. Mater. Sci. Eng. A 2018, 725, 33–42. [Google Scholar] [CrossRef]
- Liu, R.; Hui, S.X.; Ye, W.J.; Xiong, B.Q.; Yu, Y.; Fu, Y.Y. Dynamic fracture toughness of TA15ELI alloy studied by instrumented impact test. Rare Metals 2010, 29, 608–612. [Google Scholar] [CrossRef]
- Liu, R.; Hui, S.X.; Ye, W.J.; Yu, Y.; Fu, Y.Y.; Song, X.Y.; Deng, X.G. Tensile and fracture properties of Ti–62A alloy plate with different microstructures. Rare Metals 2012, 31, 420–423. [Google Scholar] [CrossRef]
- Qin, D.Y.; Lu, Y.F.; Liu, Q.; Zheng, L.; Zhou, L. Transgranular shearing introduced brittlement of Ti–5Al–5V–5Mo–3Cr alloy with full lamellar structure at room temperature. Mater. Sci. Eng. A 2013, 572, 19–24. [Google Scholar] [CrossRef]
- Semiatin, S.L.; Bieler, T.R. Effect of texture and slip mode on the anisotropy of plastic flow and flow softening during hot working of Ti–6Al–4V. Metall. Mater. Trans. A 2001, 32, 1787–1799. [Google Scholar] [CrossRef]
- Banerjee, D.; Williams, J.C. Microstructure and slip character in titanium alloys. Def. Sci. J. 2014, 36, 191–206. [Google Scholar] [CrossRef]
- Chong, Y.; Bhattacharjee, T.; Park, M.H.; Shibata, A.; Tsuji, N. Factors determining room temperature mechanical properties of bimodal microstructures in Ti–6Al–4V alloy. Mater. Sci. Eng. A 2018, 730, 217–222. [Google Scholar] [CrossRef]
- Chong, Y.; Deng, G.Y.; Yi, J.H.; Shibata, A.; Tsuji, N. On the strain hardening abilities of α+β titanium alloys: The roles of strain partitioning and interface length density. J. Alloys Compd. 2019, 811, 152040. [Google Scholar] [CrossRef]

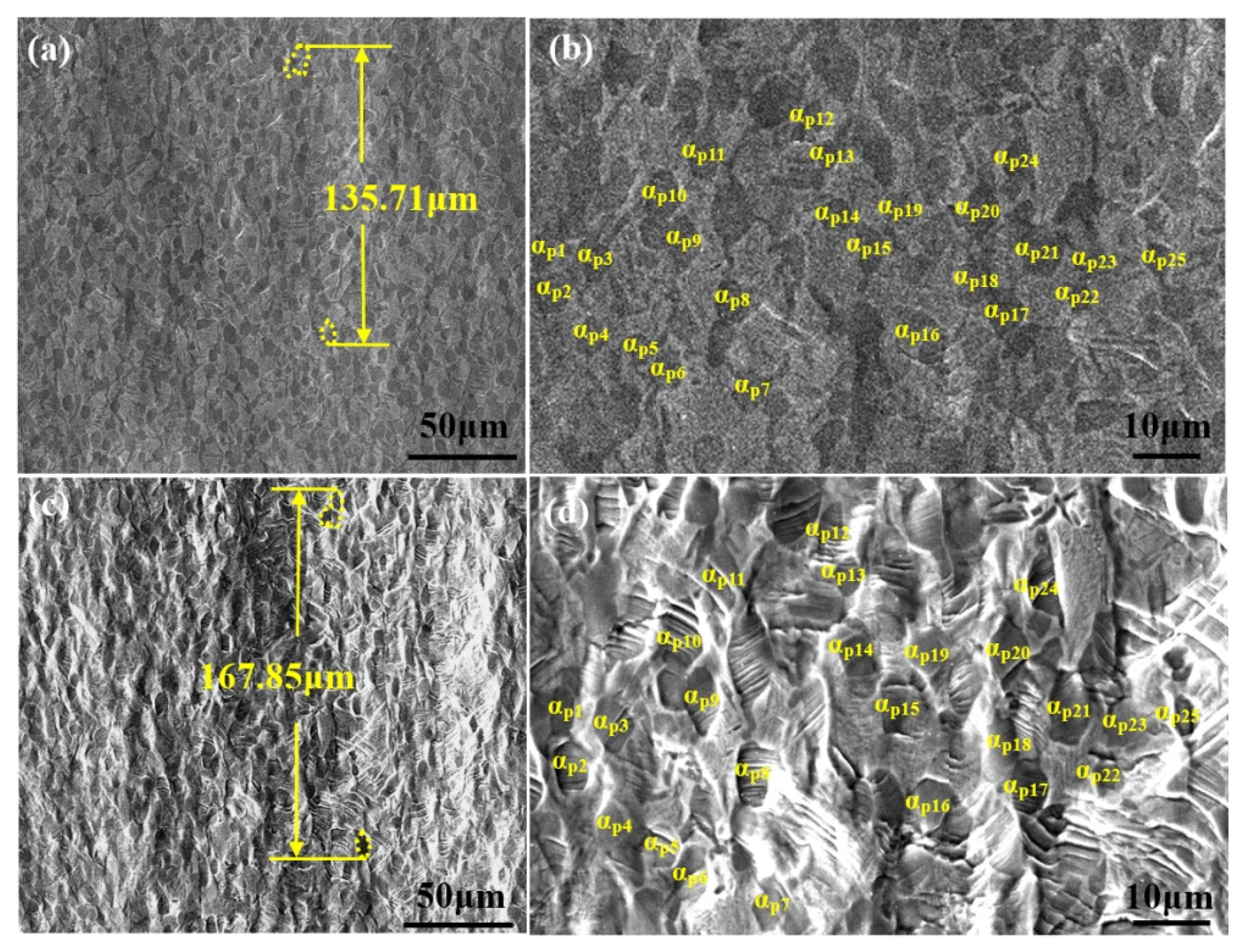



| No. | Position A (μm) | Position B (μm) | Δ (Position A→B) (μm) | εL (%) |
|---|---|---|---|---|
| αp1 | 6.54 | 7.15 | 0.61 | 9.33 |
| αp2 | 6.78 | 7.30 | 0.52 | 7.67 |
| αp3 | 6.84 | 7.52 | 0.68 | 9.94 |
| αp4 | 5.67 | 6.13 | 0.46 | 8.11 |
| αp5 | 5.34 | 5.77 | 0.43 | 8.05 |
| αp6 | 6.78 | 7.44 | 0.66 | 9.73 |
| αp7 | 7.12 | 7.73 | 0.61 | 8.57 |
| αp8 | 7.46 | 8.32 | 0.86 | 11.53 |
| αp9 | 8.64 | 9.43 | 0.79 | 9.14 |
| αp10 | 7.54 | 8.61 | 1.07 | 14.19 |
| αp11 | 8.13 | 9.27 | 1.14 | 14.02 |
| αp12 | 5.49 | 5.98 | 0.49 | 8.93 |
| αp13 | 4.91 | 5.39 | 0.48 | 9.78 |
| αp14 | 5.17 | 5.62 | 0.45 | 8.70 |
| αp15 | 7.80 | 8.25 | 0.45 | 5.77 |
| αp16 | 6.19 | 6.86 | 0.67 | 10.82 |
| αp17 | 6.89 | 7.29 | 0.40 | 5.81 |
| αp18 | 8.22 | 9.12 | 0.90 | 10.95 |
| αp19 | 5.94 | 6.43 | 0.49 | 8.25 |
| αp20 | 9.83 | 10.57 | 0.74 | 7.53 |
| αp21 | 8.14 | 9.36 | 1.22 | 14.99 |
| αp22 | 4.87 | 5.21 | 0.34 | 6.98 |
| αp23 | 5.59 | 6.28 | 0.69 | 12.34 |
| αp24 | 8.64 | 9.78 | 1.14 | 13.19 |
| αp25 | 6.19 | 6.72 | 0.53 | 8.56 |
| Average | 9.72 |
| No. | Position A’ (μm) | Position B’ (μm) | Δ (Position A’→B’) (μm) | εL (%) |
|---|---|---|---|---|
| αL1 | 3.441 | 3.459 | 0.018 | 0.52 |
| αL2 | 2.957 | 2.973 | 0.016 | 0.54 |
| αL3 | 6.068 | 6.108 | 0.040 | 0.66 |
| αL4 | 3.925 | 3.934 | 0.009 | 0.23 |
| αL5 | 4.084 | 4.108 | 0.024 | 0.59 |
| αL6 | 7.189 | 7.257 | 0.068 | 0.95 |
| αL7 | 2.996 | 3.012 | 0.016 | 0.53 |
| αL8 | 5.839 | 5.874 | 0.035 | 0.60 |
| αL9 | 6.226 | 6.283 | 0.057 | 0.92 |
| αL10 | 5.144 | 5.181 | 0.037 | 0.72 |
| αL11 | 2.527 | 2.545 | 0.018 | 0.71 |
| αL12 | 26.774 | 28.324 | 1.550 | 5.79 |
| - | - | - | Average | 1.06 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pan, S.; Fu, M.; Liu, H.; Chen, Y.; Yi, D. In Situ Observation of the Tensile Deformation and Fracture Behavior of Ti–5Al–5Mo–5V–1Cr–1Fe Alloy with Different Microstructures. Materials 2021, 14, 5794. https://doi.org/10.3390/ma14195794
Pan S, Fu M, Liu H, Chen Y, Yi D. In Situ Observation of the Tensile Deformation and Fracture Behavior of Ti–5Al–5Mo–5V–1Cr–1Fe Alloy with Different Microstructures. Materials. 2021; 14(19):5794. https://doi.org/10.3390/ma14195794
Chicago/Turabian StylePan, Suping, Mingzhu Fu, Huiqun Liu, Yuqiang Chen, and Danqing Yi. 2021. "In Situ Observation of the Tensile Deformation and Fracture Behavior of Ti–5Al–5Mo–5V–1Cr–1Fe Alloy with Different Microstructures" Materials 14, no. 19: 5794. https://doi.org/10.3390/ma14195794
APA StylePan, S., Fu, M., Liu, H., Chen, Y., & Yi, D. (2021). In Situ Observation of the Tensile Deformation and Fracture Behavior of Ti–5Al–5Mo–5V–1Cr–1Fe Alloy with Different Microstructures. Materials, 14(19), 5794. https://doi.org/10.3390/ma14195794








