Impact Resistance Analysis and Optimization of Variant Truss Beam Structure Based on Material Properties
Abstract
:1. Introduction
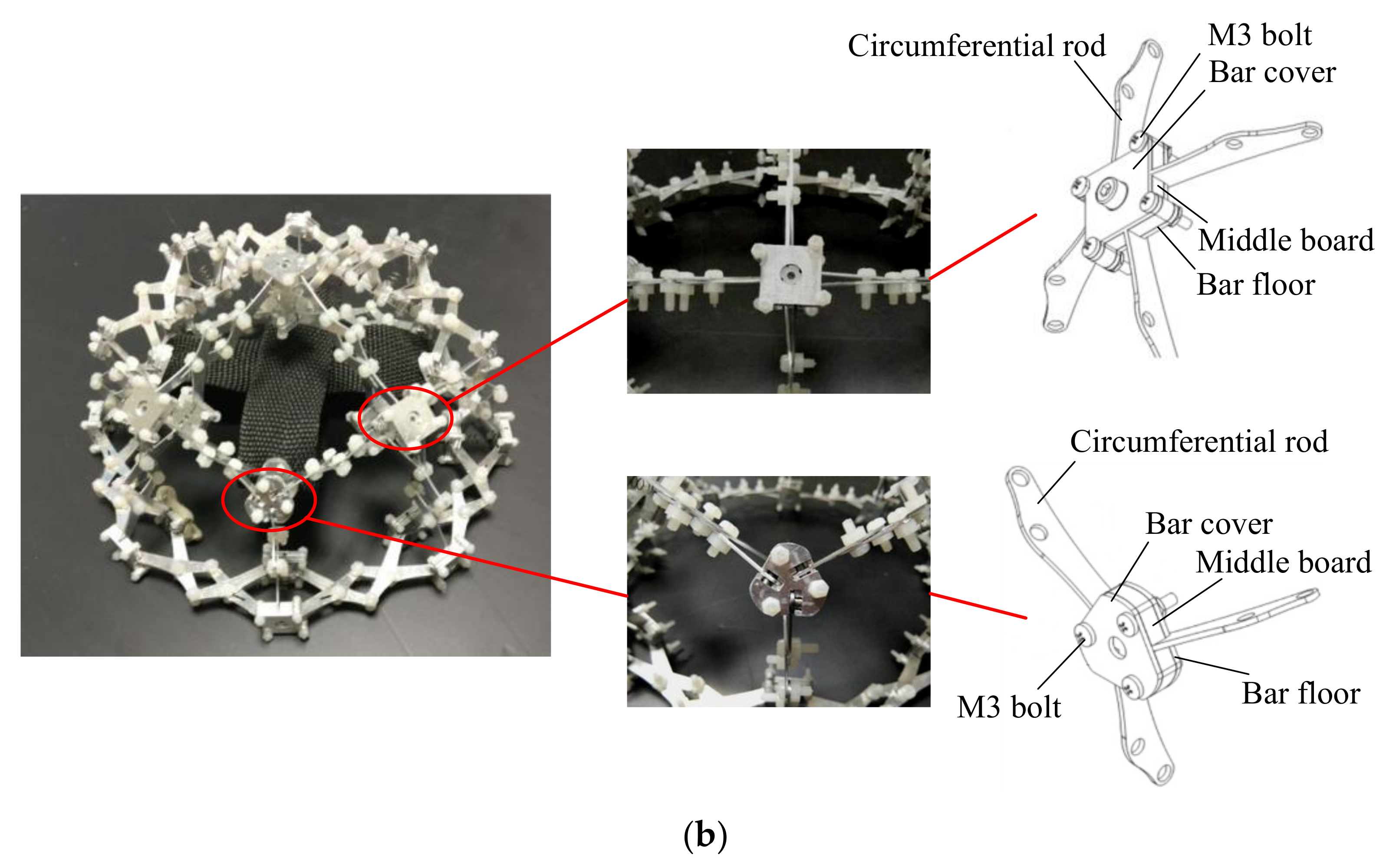
2. Design of Variant Truss Beam Structure
3. Analysis of the Anti-Impact Dynamics Mechanism of Variant Truss Beam Structure
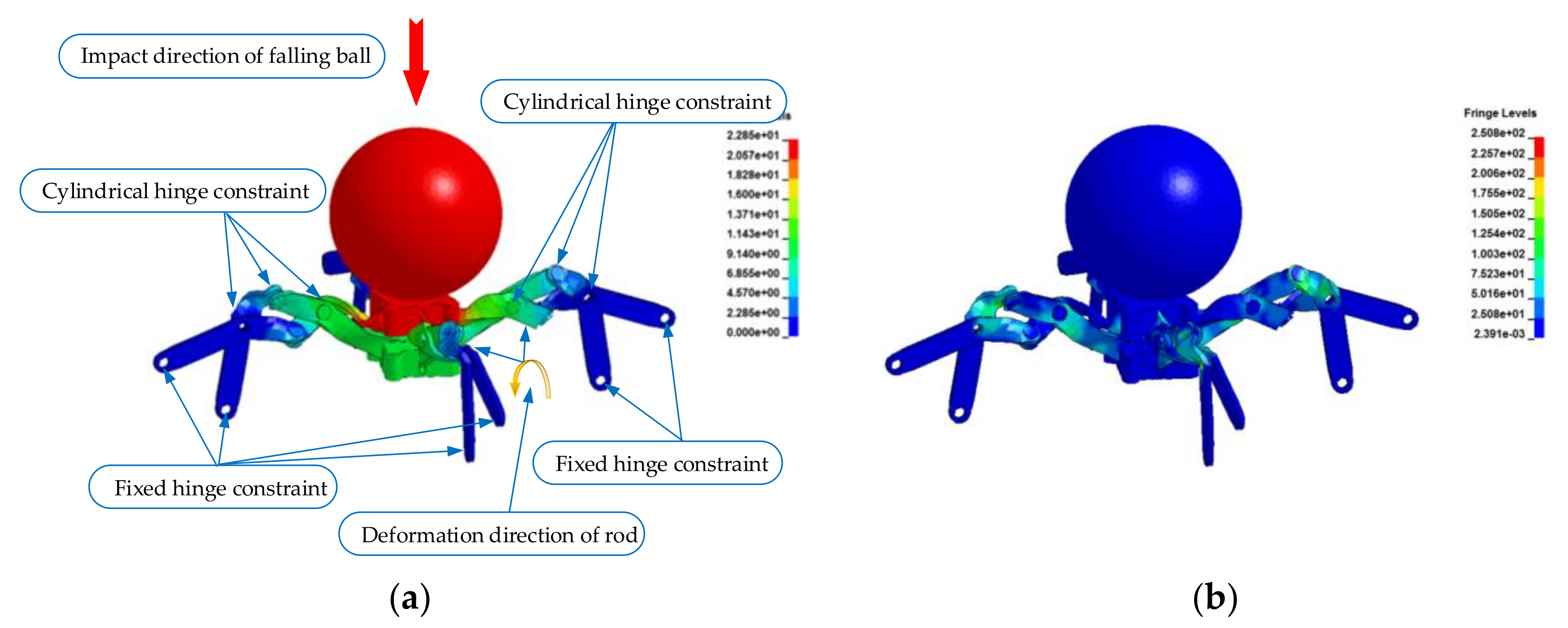
3.1. Finite Element Simulation Analysis of Impact Load of Truss Beam Structural Element
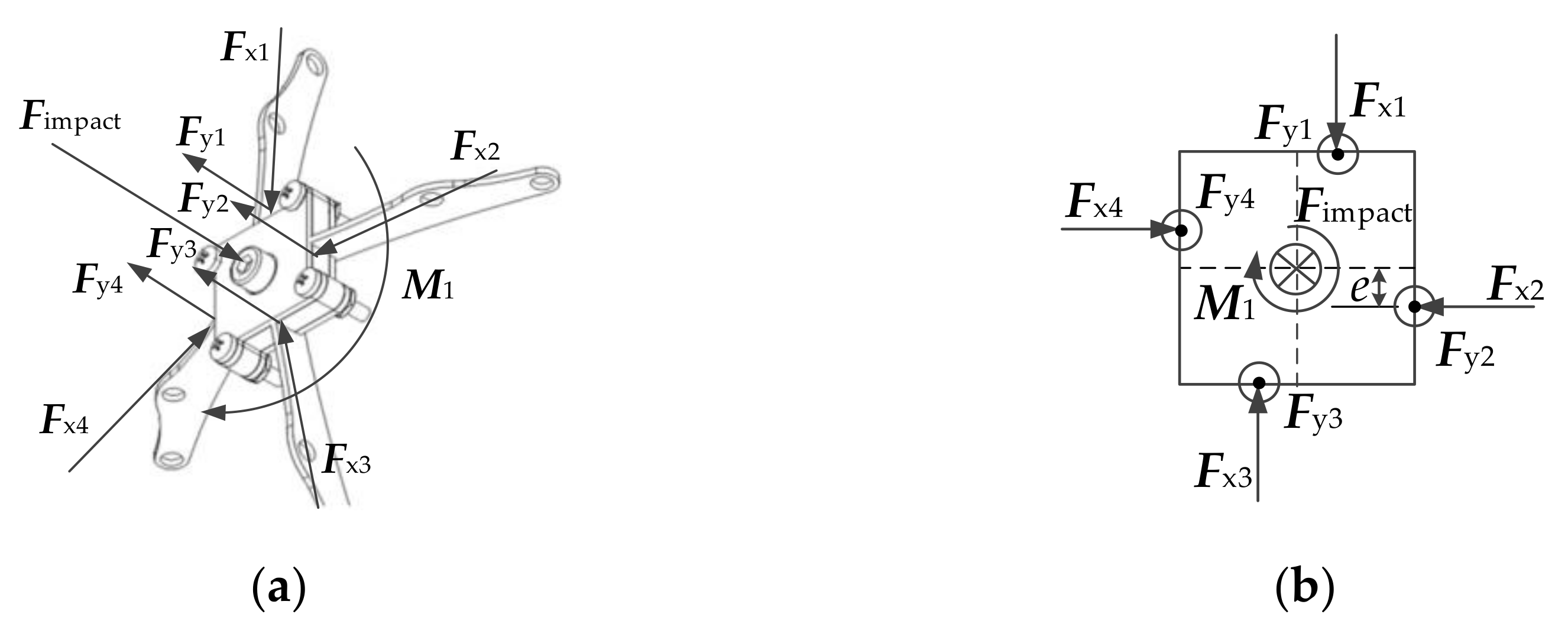
3.2. Theoretical Analysis of Impact Load of Truss Structure Elements
- Initial phase
- 2.
- Intermediate transition state
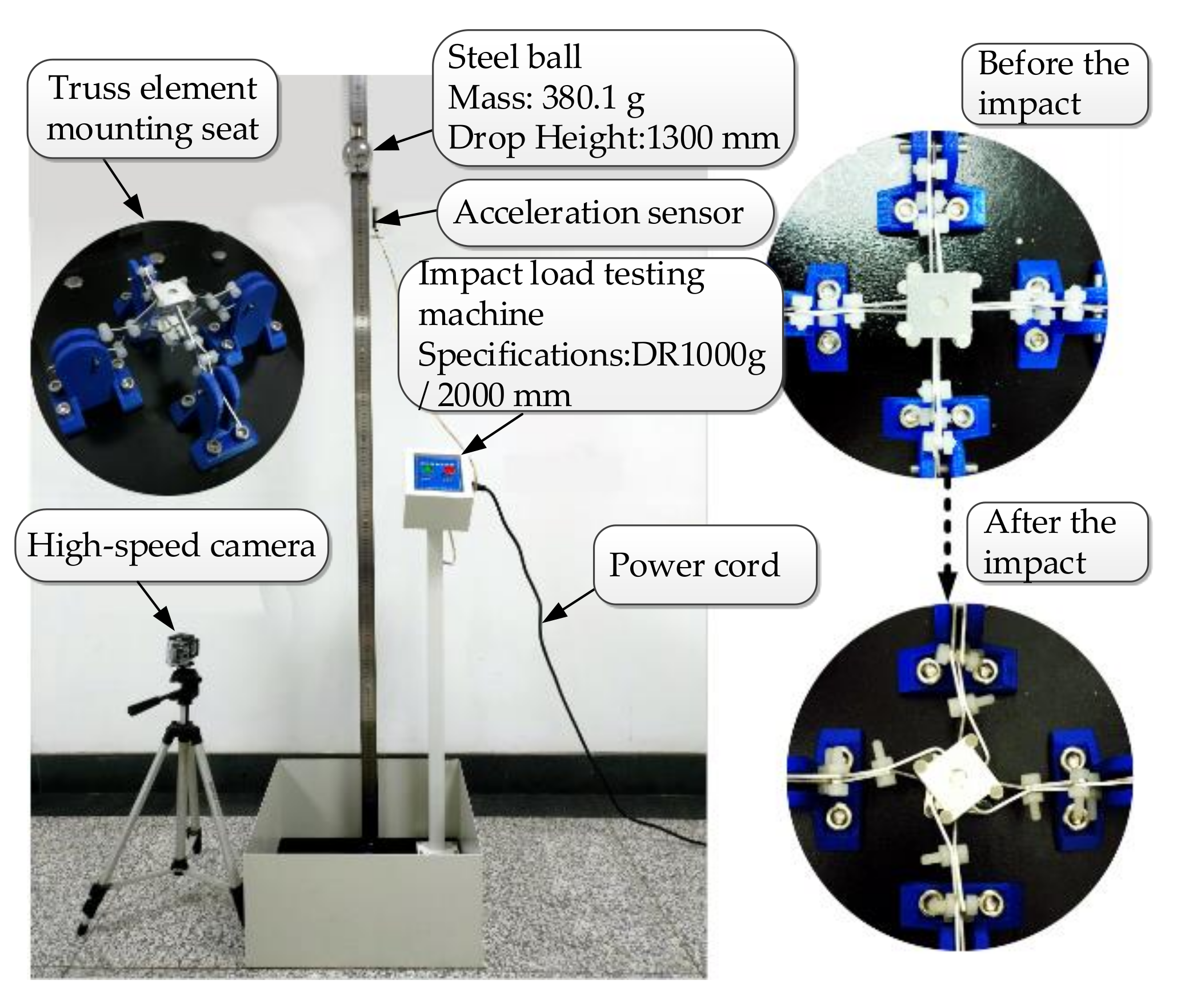
3.3. Physical Experiment of Impact Load of Truss Beam Structure
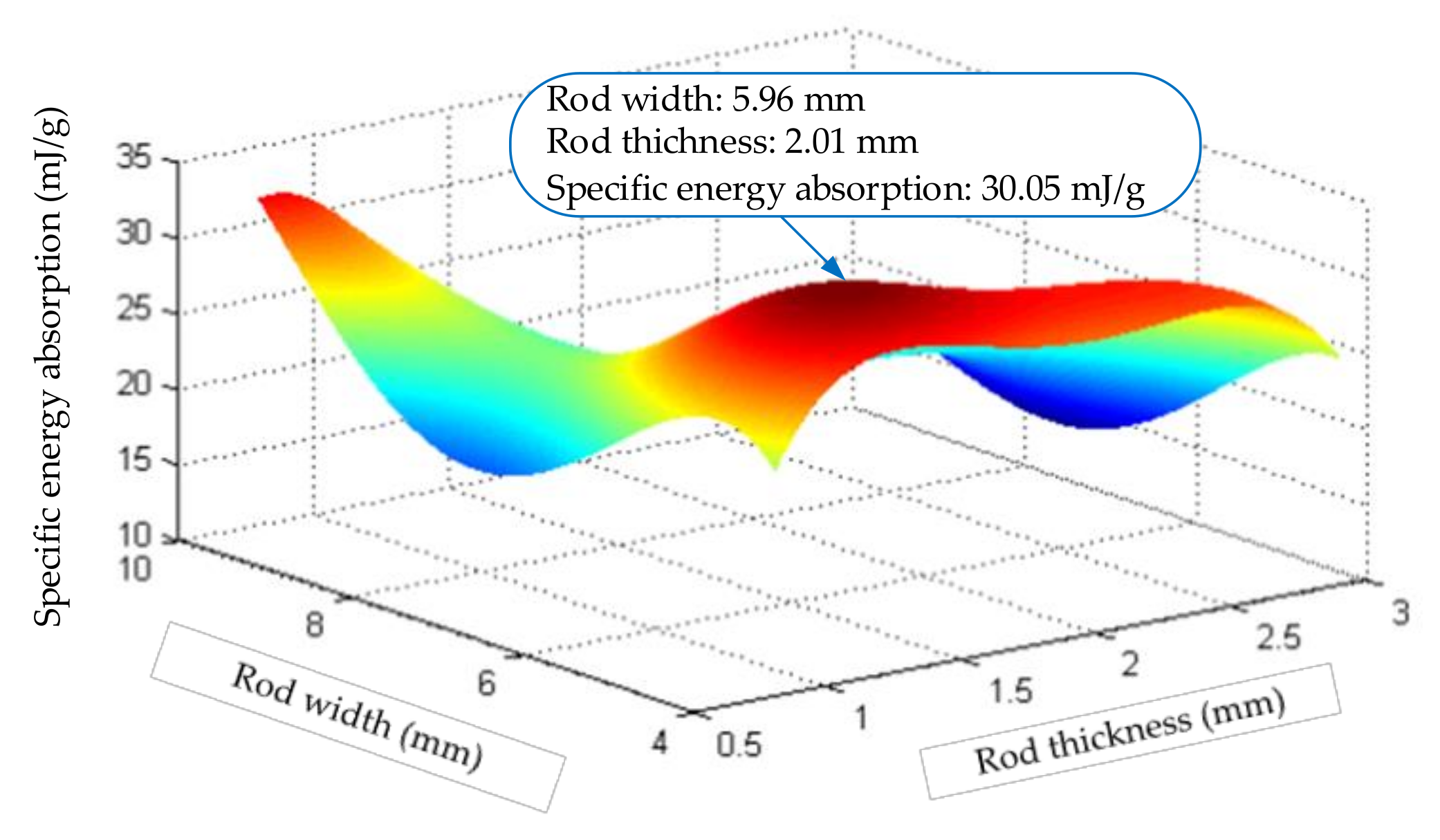
4. Optimization of the Rod Parameters of Variant Truss Beam Structure
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yao, J.M.; Wang, L.; Yan, Y.Y.; He, F.L.; Jian, L. The supporting principle of truss cable in the rockburst roadway and its application. J. Min. Saf. Eng. 2017, 34, 535–541. [Google Scholar] [CrossRef]
- Cao, D.F.; Zhang, X.; Lu, Y.H.; Wang, K.; Li, C.Q.; Liu, Q.; Han, Z.X. Seismic performance of steel-truss reinforced concrete portal frame structures. J. Build. Struct. 2021, 42, 36–48. [Google Scholar] [CrossRef]
- Yang, J.J.; Yao, J.; Peng, H.K.; Gu, Z.Y.; Wang, Z.G. Research on rapid design method of spacecraft truss structure. Electro-Mech. Eng. 2015, 31, 25–28. [Google Scholar] [CrossRef]
- Hu, H.Y.; Tian, Q.; Zhang, W.; Jin, D.P.; Hu, G.K.; Song, Y.P. Nonlinear dynamics and control of large deployable space structures composed of trusses and meshes. Adv. Mech. 2013, 43, 390–414. [Google Scholar] [CrossRef]
- Qiao, D.; Zhang, L.; Long, P.P. Rapid splicing portable truss bridge technology research and application. Mech. Manag. Dev. 2014, 29, 90–92. [Google Scholar] [CrossRef]
- Luo, Y.X.; Che, X.Y.; Liu, Q.Y.; Wang, C. The research of hyper-chaotic neural network method to displacement analysis of variable geometry truss manipulator. J. Hunan Univ. Sci. Tech. (Nat. Sci. Edit.) 2008, 23, 37–40. [Google Scholar] [CrossRef]
- Guan, K.; Pan, C.Y.; Zhang, X.; Deng, H. Establishment and analysis of general kinematic model of plane scissor mechanism. J. Mech. Des. Res. 2010, 6, 27–30. [Google Scholar] [CrossRef]
- Wu, G.R. Study on Design Theory of Curve Trajectory Scissors. Master’s Thesis, Changsha University of Science and Technology, Changsha, China, 2013. [Google Scholar]
- Li, J.L.; Liu, J.X.; Wang, Y.F. Development and finite element analysis of a scissor-type telescopic stent. Mach. Manuf. 2016, 54, 4–7. [Google Scholar] [CrossRef]
- Han, B.; Xu, Y.D.; Yao, J.T.; Zhang, S.; Zhen, D.; Zhao, Y.S. Design and analysis of an overconstrained scissors double-hoop truss deployable antenna mechanism. J. Yanshan Univ. 2019, 43, 298–305. [Google Scholar] [CrossRef]
- Wu, N.; Liu, R.Q.; Guo, H.W. The Design and Kinematics Analysis of an Underactuated Cable-Truss Mechanism. Adv. Mater. Res. 2014, 915, 256–263. [Google Scholar] [CrossRef]
- Liu, G.; Chen, W.; Wang, W.; Chen, Y. Design and analysis of a novel space deployable mechanism of ring and frustum type. Int. J. Adv. Manuf. Technol. 2018, 94, 3251–3264. [Google Scholar] [CrossRef]
- Dai, J.S.; Li, D.; Zhang, Q.; Jin, G. Mobility analysis of a complex structured ball based on mechanism decomposition and equivalent screw system analysis. Mech. Mach. Theory 2004, 39, 445–458. [Google Scholar] [CrossRef]
- Huang, W.L. Research of Static Behavior of Tubular Gap KK-Joints Made of Square Chord and Circular Braces. Master’s Thesis, Beijing Jiaotong University, Beijing, China, 2011. [Google Scholar]
- Li, B.; Cui, Q.F. Topological characteristics of a spatial truss deployable mechanism. Mech. Sci. Tech. Aeros. Eng. 2011, 30, 609–612. [Google Scholar] [CrossRef]
- Huang, Z.B.; Liu, J.Y.; Yuan, T.T.; Hou, P. Dynamic modeling and analysis on a deployable space truss multi-body system. J. Vib. Shock 2021, 40, 170–179. [Google Scholar] [CrossRef]
- Yan, Z.T.; Cao, J.Y.; Zhu, C.H.; Zhai, Y.Q. A new topology optimization structure of aluminum alloy truss. Sichuan Build. Sci. 2020, 46, 43–49. [Google Scholar] [CrossRef]
- Chen, J.J.; Cao, Y.B.; Sun, H.A. Topology optimization of truss structures with systematic reliability constraints under multiple load cases. Acta Mech. Solida Sin. 2000, 21, 11–18. [Google Scholar] [CrossRef]
- Zhang, L.; Bhatti, M.M.; Marin, M.; Mekheimer, K.S. Entropy Analysis on the Blood Flow through Anisotropically Tapered Arteries Filled with Magnetic Zinc-Oxide (ZnO) Nanoparticles. Entropy 2020, 22, 1070. [Google Scholar] [CrossRef]
- Sun, X.; Jing, X.; Xu, J.; Cheng, L. Vibration isolation via a scissor-like structured platform. J. Sound Vib. 2014, 333, 2404–2420. [Google Scholar] [CrossRef]
- Chen, C.Z.; Wei, J.; Chen, J.B.; Nie, H.; Zheng, G.; Yuan, Y.N. Characteristics analysis of landing buffer in the truss type mars lander. J. Vib. Meas. Diagn. 2018, 38, 943–947. [Google Scholar] [CrossRef]
- Li, Y.N.; Zhang, W.; Cao, D.X.; Cui, Y.T. Modal experiment analysis and FEM simulation for a ring truss structure. Chin. J. Appl. Mech. 2017, 34, 119–124. [Google Scholar] [CrossRef]
- Marin, M.; Othman, M.I.A.; Seadawy, A.R.; Carstea, C. A domain of influence in the Moore–Gibson–Thompson theory of dipolar bodies. J. Taibah Univ. Sci. 2020, 14, 653–660. [Google Scholar] [CrossRef]
- Jamal-Omidi, M.; Benis, A.C. A numerical study on energy absorption capability of lateral corrugated composite tube under axial crushing. Int. J. Crashworthiness 2021, 26, 147–158. [Google Scholar] [CrossRef]
- Li, J.; Tian, C.; Hong, W.; Duan, S.; Zhang, Y.; Wu, W.; Hu, G.; Xia, R. Shock responses of nanoporous gold subjected to dynamic loadings: Energy absorption. Int. J. Mech. Sci. 2021, 192, 106191. [Google Scholar] [CrossRef]
- Yuan, Z.Z.; Dai, Q.X. Metallic Materials, 3rd ed.; Chemical Industry Press: Beijing, China, 2019; pp. 27–91. [Google Scholar]
- Dewey, H.H.; Pierce, G.A. Introduction to Structural Dynamics and Aeroelasticity, 2nd ed.; Cambridge University Press: New York, NY, USA, 2011; pp. 62–185. [Google Scholar]
- Ministry of Housing and Urban-Rural Development of the People’s Republic of China. Quality Standard of Steel Trusses (JG 8-1999); Standards Press of China: Beijing, China, 2001; pp. 2–7. [Google Scholar]
- Zheng, Y. Optimization Design of a Parallel Mechanism Driven by Nine Cables Based on Experimental Design Methods and Response Surface Methods. Chin. J. Mech. Eng. 2017, 53, 92–102. [Google Scholar] [CrossRef]
- Besharatlou, S.; Anbia, M.; Salehi, S. Optimization of sulfate removal from aqueous media by surfactant-modified layered double hydroxide using response surface methodology. Mater. Chem. Phys. 2021, 262, 124322. [Google Scholar] [CrossRef]
- Chen, X.Y. Study on Crashworthiness Optimization of Thin-Walled Structure and Truss Structure. Master’s Thesis, Hunan University, Changsha, China, 2007. [Google Scholar]







| Material Properties | Steel | AL |
|---|---|---|
| Density/kg · m−3 | 7850 | 2700 |
| Young’s modulus/MPa | 200,000 | 6900 |
| Poisson’s ratio | 0.30 | 0.33 |
| Yield strength/MPa | 620.42 | 240 |
| Tangent modulus/MPa | 79,000 | 2700 |
| AL: Width(mm)/Thickness(mm) | 0.8 | 1.1 | 1.4 | 1.7 | 2 | 2.3 | 2.6 | 2.9 |
|---|---|---|---|---|---|---|---|---|
| 3 | 39.411 | - | 32.720 | - | 135.055 | - | 119.739 | - |
| 4 | - | 62.618 | - | 136.751 | - | 138.018 | - | 99.657 |
| 5 | 27.403 | - | 74.369 | - | - | - | - | - |
| 6 | - | 50.105 | - | 121.490 | - | 91.766 | - | 67.357 |
| 7 | 26.966 | - | - | - | 97.478 | - | - | - |
| 8 | - | 62.227 | - | 97.189 | - | 73.638 | - | 51.704 |
| 9 | 29.448 | - | - | - | - | - | 59.171 | - |
| 10 | - | 124.812 | - | 79.658 | - | 56.362 | - | 47.102 |
| Steel: Width(mm)/Thickness(mm) | 0.8 | 1.1 | 1.4 | 1.7 | 2 | 2.3 | 2.6 | 2.9 |
|---|---|---|---|---|---|---|---|---|
| 3 | 25.994 | - | 26.007 | - | 33.897 | - | 27.849 | - |
| 4 | - | 34.443 | - | 32.708 | - | 28.695 | - | 22.720 |
| 5 | 17.788 | - | 25.626 | - | 35.051 | - | - | - |
| 6 | - | 31.497 | - | 26.851 | - | 21.006 | - | 16.773 |
| 7 | 20.435 | - | - | - | 24.597 | - | - | - |
| 8 | - | 22.510 | - | - | - | 18.635 | - | 14.258 |
| 9 | 23.686 | - | - | - | - | - | 20.182 | - |
| 10 | - | 29.842 | - | 16.519 | - | 16.268 | - | 16.280 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, X.; Pan, J.; Zhou, X. Impact Resistance Analysis and Optimization of Variant Truss Beam Structure Based on Material Properties. Materials 2021, 14, 5847. https://doi.org/10.3390/ma14195847
Li X, Pan J, Zhou X. Impact Resistance Analysis and Optimization of Variant Truss Beam Structure Based on Material Properties. Materials. 2021; 14(19):5847. https://doi.org/10.3390/ma14195847
Chicago/Turabian StyleLi, Xiaohao, Junqi Pan, and Xingchen Zhou. 2021. "Impact Resistance Analysis and Optimization of Variant Truss Beam Structure Based on Material Properties" Materials 14, no. 19: 5847. https://doi.org/10.3390/ma14195847
APA StyleLi, X., Pan, J., & Zhou, X. (2021). Impact Resistance Analysis and Optimization of Variant Truss Beam Structure Based on Material Properties. Materials, 14(19), 5847. https://doi.org/10.3390/ma14195847





