Porous Phantoms Mimicking Tissues—Investigation of Optical Parameters Stability Over Time
Abstract
:1. Introduction
- -
- -
- calibration of optical devices
- -
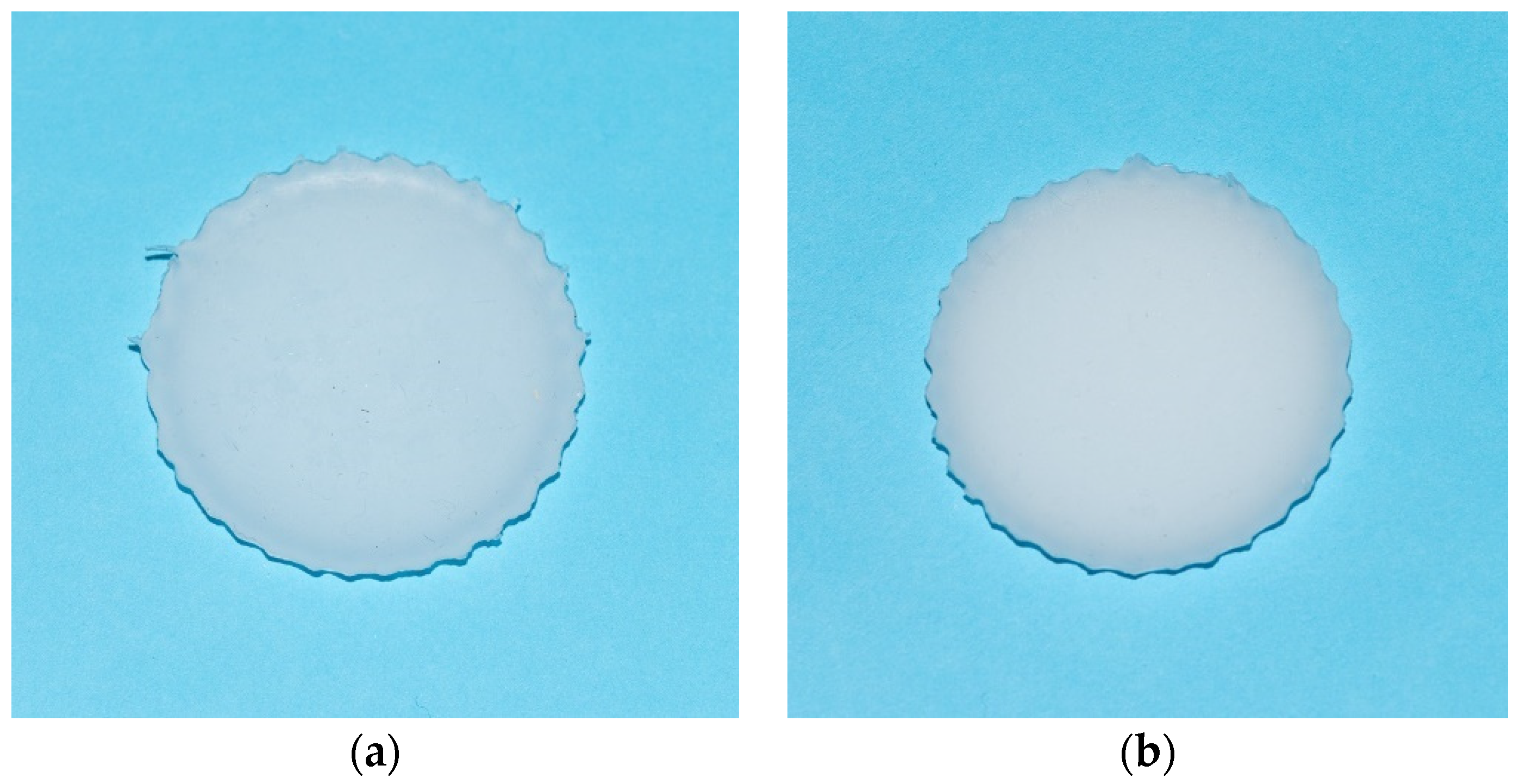
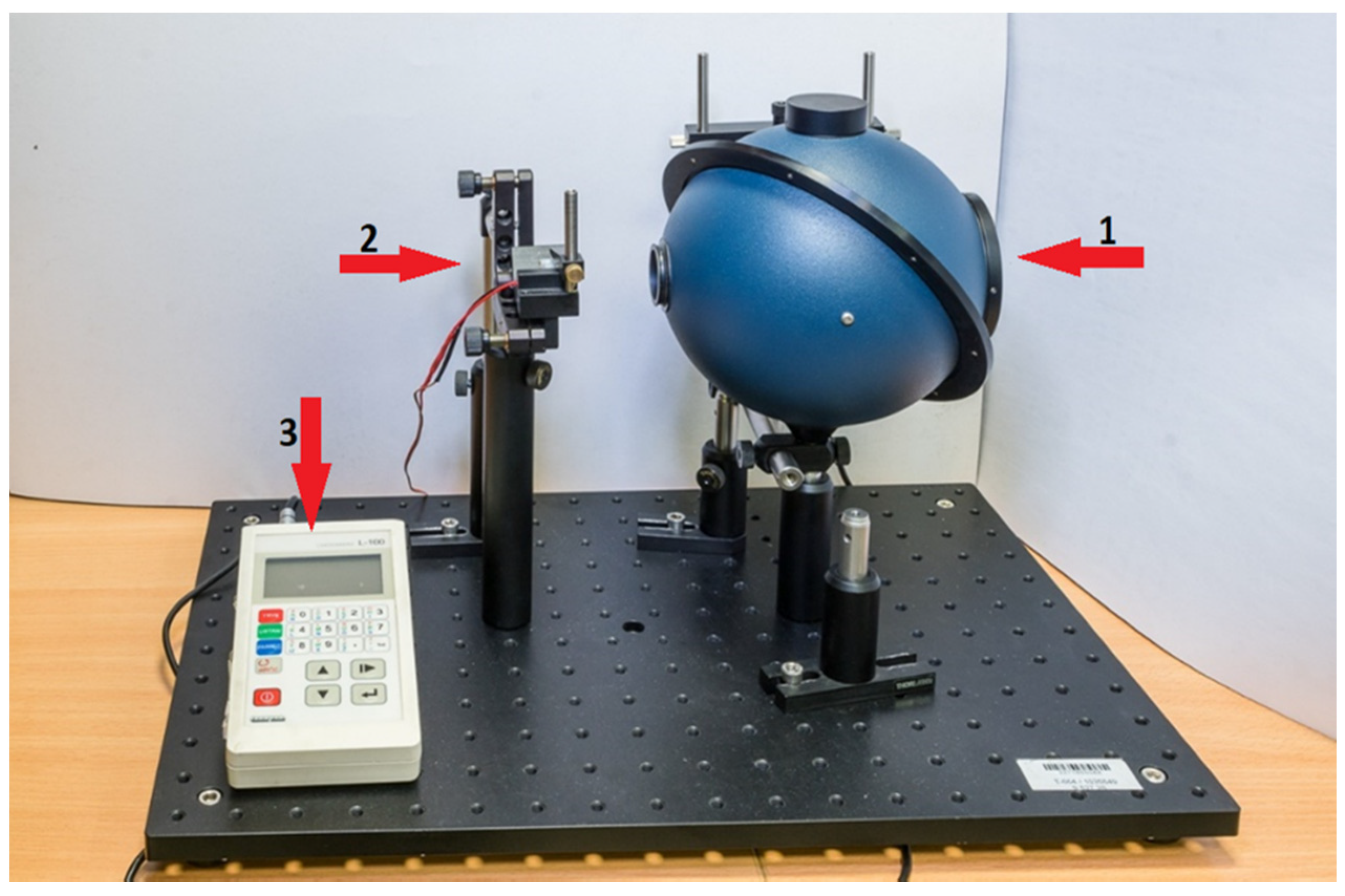
2. Materials and Methods
3. Results
3.1. Optical Parameters Measurements
3.2. Optical Parameters Stability Measurements
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Feder, I.; Duadi, H.; Fixler, D. Experimental System for Measuring the Full Scattering Profile of Circular Phantoms. Biomed. Opt. Express 2015, 6, 2877. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Duadi, H.; Feder, I.; Fixler, D. Linear Dependency of Full Scattering Profile Isobaric Point on Tissue Diameter. J. Biomed. Opt. 2014, 19, 026007. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jacques, S.L. Optical Properties of Biological Tissues: A Review. Phys. Med. Biol. 2013, 58, R37–R61. [Google Scholar] [CrossRef] [PubMed]
- Lu, G.; Fei, B. Medical Hyperspectral Imaging: A Review. J. Biomed. Opt. 2014, 19, 010901. [Google Scholar] [CrossRef] [PubMed]
- Feder, I.; Wróbel, M.; Duadi, H.; Jędrzejewska-Szczerska, M.; Fixler, D. Experimental Results of Full Scattering Profile from Finger Tissue-like Phantom. Biomed. Opt. Express 2016, 7, 4695. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wróbel, M.S.; Popov, A.P.; Bykov, A.V.; Tuchin, V.V.; Jędrzejewska-Szczerska, M. Nanoparticle-Free Tissue-Mimicking Phantoms with Intrinsic Scattering. Biomed. Opt. Express 2016, 7, 2088. [Google Scholar] [CrossRef] [Green Version]
- Karpienko, K.; Gnyba, M.; Milewska, D.; Wróbel, M.S.; Jędrzejewska-Szczerska, M. Blood Equivalent Phantom vs Whole Human Blood, a Comparative Study. J. Innov. Opt. Health Sci. 2016, 9, 1650012. [Google Scholar] [CrossRef] [Green Version]
- Ziemczonok, M.; Kuś, A.; Wasylczyk, P.; Kujawińska, M. 3D-Printed Biological Cell Phantom for Testing 3D Quantitative Phase Imaging Systems. Sci. Rep. 2019, 9, 18872. [Google Scholar] [CrossRef] [Green Version]
- Iliescu, C.; Taylor, H.; Avram, M.; Miao, J.; Franssila, S. A Practical Guide for the Fabrication of Microfluidic Devices Using Glass and Silicon. Biomicrofluidics 2012, 6, 016505. [Google Scholar] [CrossRef] [Green Version]
- Delfino, I.; Lepore, M.; Esposito, R. Optical Characterization of Homogeneous and Heterogeneous Intralipid-Based Samples. Appl. Sci. 2020, 10, 6234. [Google Scholar] [CrossRef]
- Ruiz, C.C.; Molina-Bolívar, J.A.; Aguiar, J.; MacIsaac, G.; Moroze, S.; Palepu, R. Thermodynamic and Structural Studies of Triton X-100 Micelles in Ethylene Glycol–Water Mixed Solvents. Langmuir 2001, 17, 6831–6840. [Google Scholar] [CrossRef]
- Pogue, B.W.; Patterson, M.S. Review of Tissue Simulating Phantoms for Optical Spectroscopy, Imaging and Dosimetry. J. Biomed. Opt. 2006, 11, 041102. [Google Scholar] [CrossRef] [PubMed]
- Chang, R.C.; Johnson, P.; Stafford, C.M.; Hwang, J. Fabrication and Characterization of a Multilayered Optical Tissue Model with Embedded Scattering Microspheres in Polymeric Materials. Biomed. Opt. Express BOE 2012, 3, 1326–1339. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kennedy, B.F.; Curatolo, A.; Hillman, T.R.; Saunders, C.M.; Sampson, D.D. Speckle Reduction in Optical Coherence Tomography Images Using Tissue Viscoelasticity. J. Biomed. Opt. 2011, 16, 020506. [Google Scholar] [CrossRef] [Green Version]
- de Bruin, D.M.; Bremmer, R.H.; Kodach, V.M.; de Kinkelder, R.; van Marle, J.; van Leeuwen, T.G.; Faber, D.J. Optical Phantoms of Varying Geometry Based on Thin Building Blocks with Controlled Optical Properties. J. Biomed. Opt. 2010, 15, 025001. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Liang, X.; Oldenburg, A.L.; Crecea, V.; Chaney, E.J.; Boppart, S.A. Optical Micro-Scale Mapping of Dynamic Biomechanical Tissue Properties. Opt. Express 2008, 16, 11052. [Google Scholar] [CrossRef] [Green Version]
- Grimwood, A.; Garcia, L.; Bamber, J.; Holmes, J.; Woolliams, P.; Tomlins, P.; Pankhurst, Q.A. Elastographic Contrast Generation in Optical Coherence Tomography from a Localized Shear Stress. Phys. Med. Biol. 2010, 55, 5515–5528. [Google Scholar] [CrossRef]
- Friebel, M.; Roggan, A.; Müller, G.; Meinke, M. Determination of Optical Properties of Human Blood in the Spectral Range 250 to 1100 Nm Using Monte Carlo Simulations with Hematocrit-Dependent Effective Scattering Phase Functions. J. Biomed. Opt. 2006, 11, 034021. [Google Scholar] [CrossRef]
- Bohndiek, S.E.; Bodapati, S.; Van De Sompel, D.; Kothapalli, S.-R.; Gambhir, S.S. Development and Application of Stable Phantoms for the Evaluation of Photoacoustic Imaging Instruments. PLoS ONE 2013, 8, e75533. [Google Scholar] [CrossRef] [Green Version]
- Kennedy, B.F.; Loitsch, S.; McLaughlin, R.A.; Scolaro, L.; Rigby, P.; Sampson, D.D. Fibrin Phantom for Use in Optical Coherence Tomography. J. Biomed. Opt. 2010, 15, 030507. [Google Scholar] [CrossRef] [Green Version]
- Fixler, D.; Nayhoz, T.; Ray, K. Diffusion Reflection and Fluorescence Lifetime Imaging Microscopy Study of Fluorophore-Conjugated Gold Nanoparticles or Nanorods in Solid Phantoms. ACS Photonics 2014, 1, 900–905. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ankri, R.; Taitelbaum, H.; Fixler, D. Reflected Light Intensity Profile of Two-Layer Tissues: Phantom Experiments. J. Biomed. Opt. 2011, 16, 085001. [Google Scholar] [CrossRef] [PubMed]
- Quirk, B.C.; McLaughlin, R.A.; Pagnozzi, A.M.; Kennedy, B.F.; Noble, P.B.; Sampson, D.D. Optofluidic Needle Probe Integrating Targeted Delivery of Fluid with Optical Coherence Tomography Imaging. Opt. Lett. 2014, 39, 2888. [Google Scholar] [CrossRef] [PubMed]
- Ochs, M.; Nyengaard, J.R.; Jung, A.; Knudsen, L.; Voigt, M.; Wahlers, T.; Richter, J.; Gundersen, H.J.G. The Number of Alveoli in the Human Lung. Am. J. Respir. Crit. Care Med. 2004, 169, 120–124. [Google Scholar] [CrossRef]
- Ray, L.A.; Heys, J.J. Fluid Flow and Mass Transport in Brain Tissue. Fluids 2019, 4, 196. [Google Scholar] [CrossRef] [Green Version]
- Graczyk, K.M.; Matyka, M. Predicting Porosity, Permeability, and Tortuosity of Porous Media from Images by Deep Learning. Sci. Rep. 2020, 10, 21488. [Google Scholar] [CrossRef]
- Listewnik, P.; Wąsowicz, M.; Kosowska, M.; Mazikowski, A. A Measurement System for Quasi-Spectral Determination of Absorption and Scattering Parameters of Veterinary Tissue Phantoms. Appl. Sci. 2019, 9, 1632. [Google Scholar] [CrossRef] [Green Version]
- Tuchin, V.V. Tissue Optics: Light Scattering Methods and Instruments for Medical Diagnosis; Society of Photo-Optical Instrumentation Engineers (SPIE): Bellingham, WA, USA, 2015; ISBN 978-1-62841-516-2. [Google Scholar]
- Hagberg, G.E.; Mamedov, I.; Power, A.; Beyerlein, M.; Merkle, H.; Kiselev, V.G.; Dhingra, K.; Kubìček, V.; Angelovski, G.; Logothetis, N.K. Diffusion Properties of Conventional and Calcium-Sensitive MRI Contrast Agents in the Rat Cerebral Cortex: Effective Diffusion of MRI Contrast Agents. Contrast Media Mol. Imaging 2014, 9, 71–82. [Google Scholar] [CrossRef]
- Mériaux, S.; Conti, A.; Larrat, B. Assessing Diffusion in the Extra-Cellular Space of Brain Tissue by Dynamic MRI Mapping of Contrast Agent Concentrations. Front. Phys. 2018, 6, 38. [Google Scholar] [CrossRef] [Green Version]
- Conti, A.; Magnin, R.; Gerstenmayer, M.; Tsapis, N.; Dumont, E.; Tillement, O.; Lux, F.; Le Bihan, D.; Mériaux, S.; Della Penna, S.; et al. Empirical and Theoretical Characterization of the Diffusion Process of Different Gadolinium-Based Nanoparticles within the Brain Tissue after Ultrasound-Induced Permeabilization of the Blood-Brain Barrier. Contrast Media Mol. Imaging 2019, 2019, 1–13. [Google Scholar] [CrossRef]
- Jacques, S.L.; Pogue, B.W. Tutorial on Diffuse Light Transport. J. Biomed. Opt. 2008, 13, 041302. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Graaff, R.; Aarnoudse, J.G.; Zijp, J.R.; Sloot, P.M.A.; de Mul, F.F.M.; Greve, J.; Koelink, M.H. Reduced Light-Scattering Properties for Mixtures of Spherical Particles: A Simple Approximation Derived from Mie Calculations. Appl. Opt. 1992, 31, 1370. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Krainov, A.D.; Mokeeva, A.M.; Sergeeva, E.A.; Agrba, P.D.; Kirillin, M.Y. Optical Properties of Mouse Biotissues and Their Optical Phantoms. Opt. Spectrosc. 2013, 115, 193–200. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Listewnik, P.; Ronowska, M.; Wąsowicz, M.; Tuchin, V.V.; Szczerska, M. Porous Phantoms Mimicking Tissues—Investigation of Optical Parameters Stability Over Time. Materials 2021, 14, 423. https://doi.org/10.3390/ma14020423
Listewnik P, Ronowska M, Wąsowicz M, Tuchin VV, Szczerska M. Porous Phantoms Mimicking Tissues—Investigation of Optical Parameters Stability Over Time. Materials. 2021; 14(2):423. https://doi.org/10.3390/ma14020423
Chicago/Turabian StyleListewnik, Paulina, Monika Ronowska, Michał Wąsowicz, Valery V. Tuchin, and Małgorzata Szczerska. 2021. "Porous Phantoms Mimicking Tissues—Investigation of Optical Parameters Stability Over Time" Materials 14, no. 2: 423. https://doi.org/10.3390/ma14020423
APA StyleListewnik, P., Ronowska, M., Wąsowicz, M., Tuchin, V. V., & Szczerska, M. (2021). Porous Phantoms Mimicking Tissues—Investigation of Optical Parameters Stability Over Time. Materials, 14(2), 423. https://doi.org/10.3390/ma14020423






