Cement Paste Mixture Proportioning with Particle Packing Theory: An Ambiguous Effect of Microsilica
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
- Portland cement CEM I 42.5 R, commercially available and manufactured by Lafarge Cement SA (28-366 Małogoszcz, Poland), that meets the standard requirements of PN-EN 197-1 [36], with a density of 3.09 g/cm3, the specific surface area of 3360 cm2/g, and the chemical and phase composition, that was delivered by the manufacturer, shown in Table 1;
- microsilica 920 EN D, commercially available and manufactured by Elkem Silicones France (69486 Lyon, France), which meets the requirements of PN-EN 13263 [37], with a density of 1.76 g/cm3, the specific surface area of 15 ÷ 35 m2/g, pozzolanic activity index of minimum 100%, and has the chemical composition, that was delivered by the manufacturer, shown in Table 2;
- nanoparticles of SiO2 in the form of powder, delivered by Sigma-Aldrich company (61-626 Poznań, Poland), with a particle size of 10 ÷ 20 nm, a density at 25 °C of 2.4 g/cm3, a specific surface area of 450 m2/g and a purity of 99.5% (the SEM image of the used nanoparticles is shown in Figure 2);

- universal superplasticizer (SP) Sikament FM6 produced by Sika AG (CH-6341 Baar, Switzerland), based on sulfonated naphthalene and melamine polycondensates, with a density of 1.15 g/cm3.
2.2. Mix Design
2.3. Mixing Process and Samples Preparations
2.4. Physical Properties
2.5. Mechanical Properties
2.6. Micromechanical Properties
2.7. Microstructural Analysis
2.8. ASR
3. Results, Analysis and Discussion
3.1. Mix Design
3.2. Physical Properties
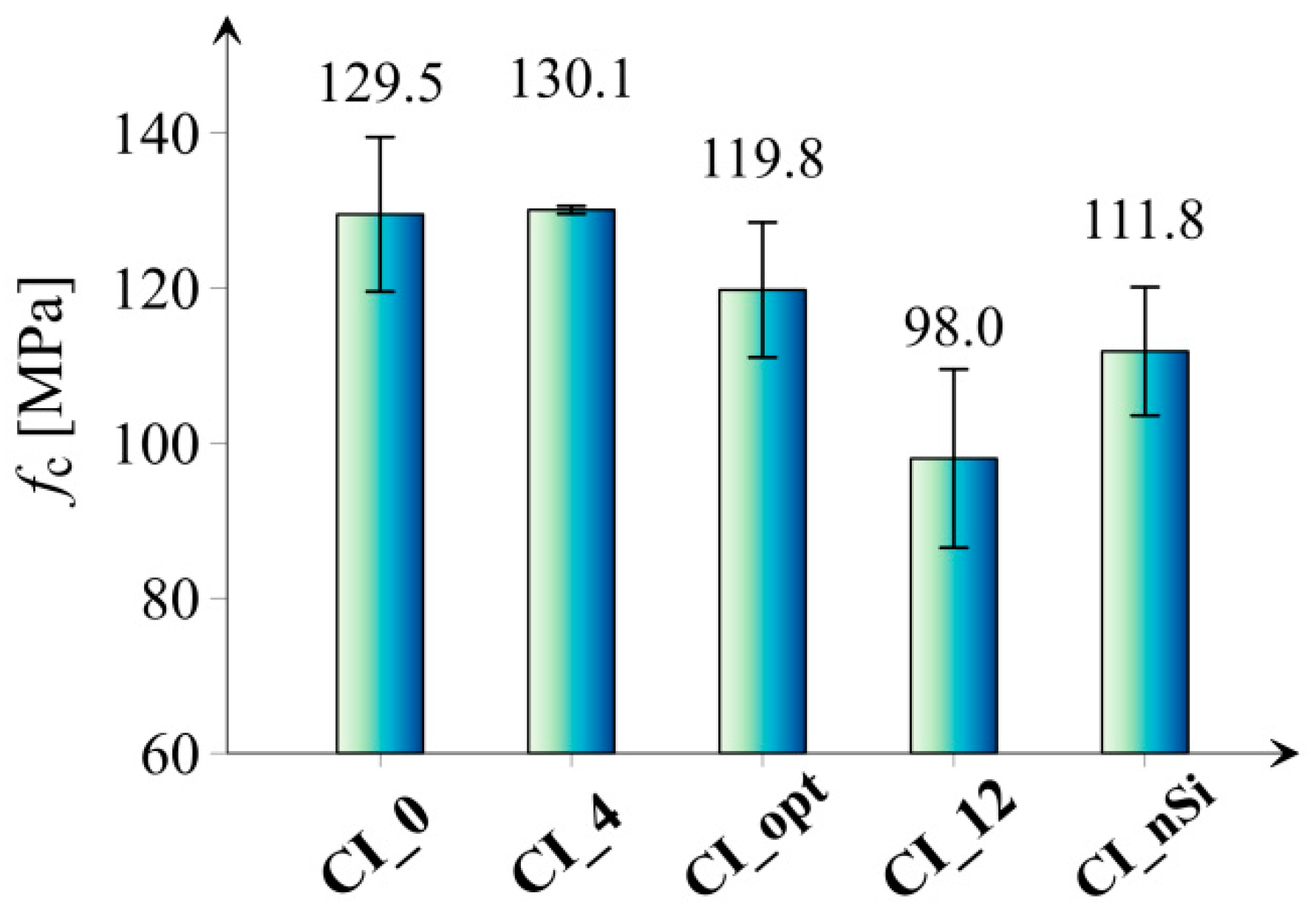
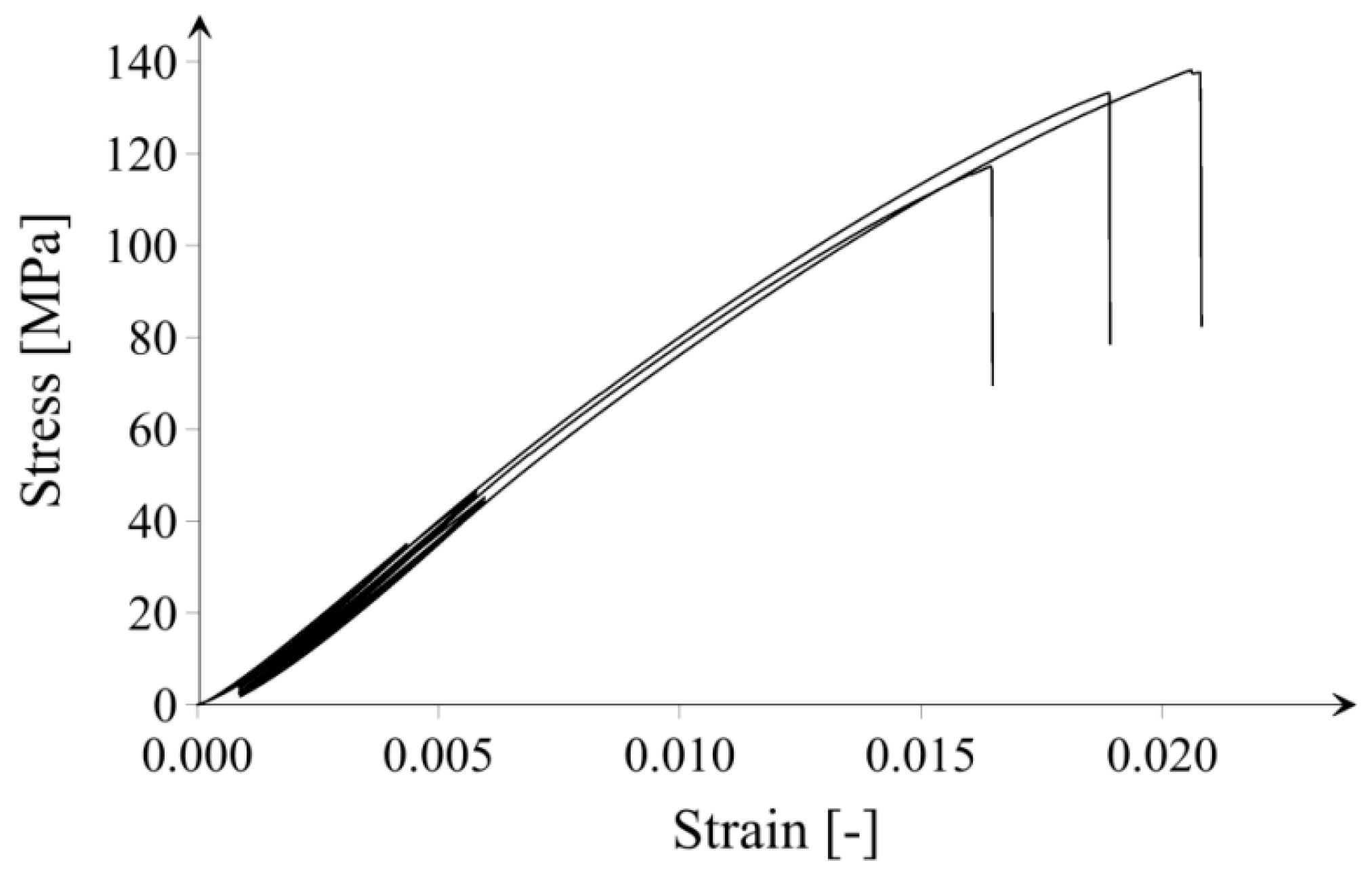
3.3. Mechanical Properties
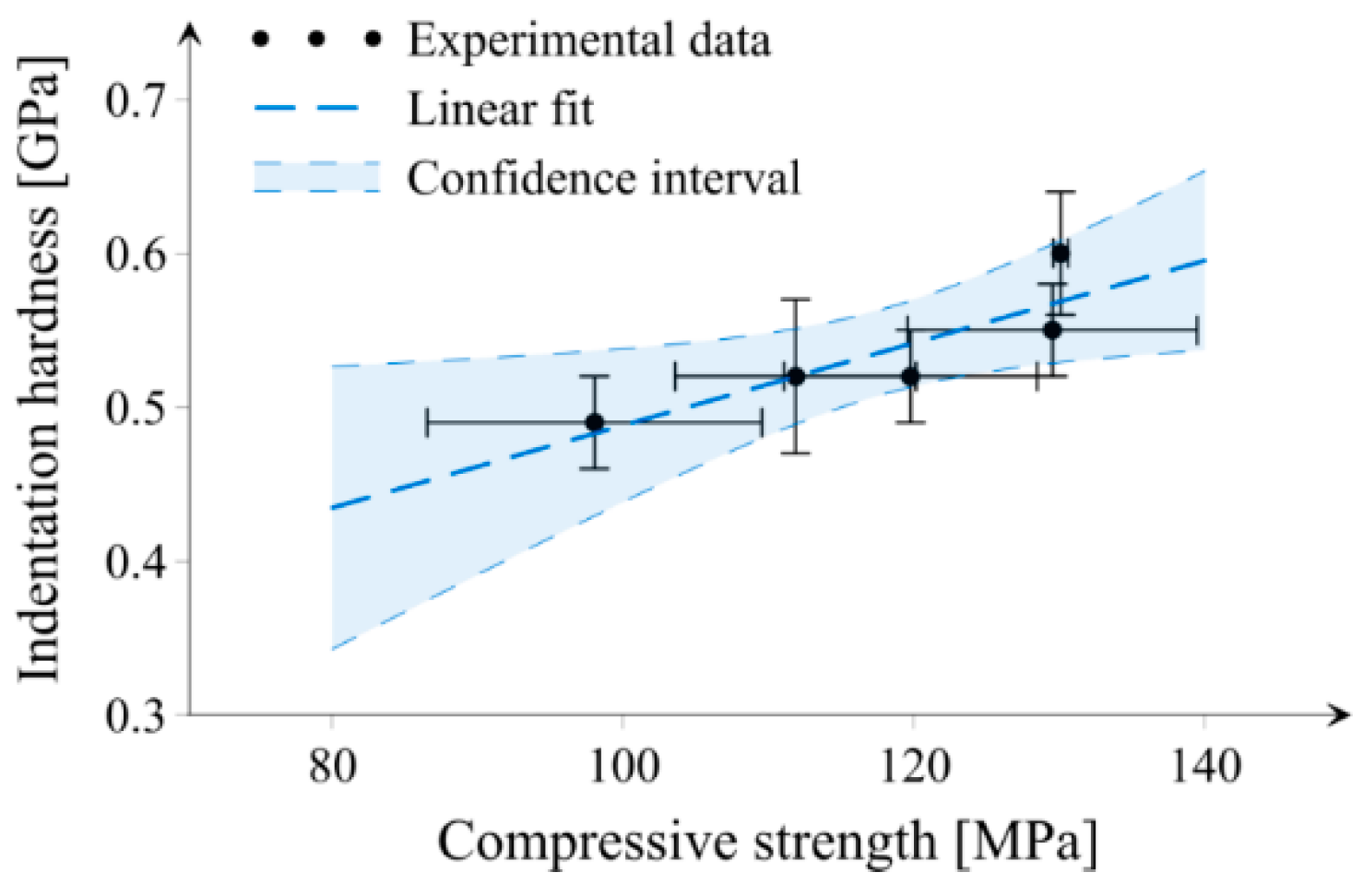
3.4. Micromechanical Properties
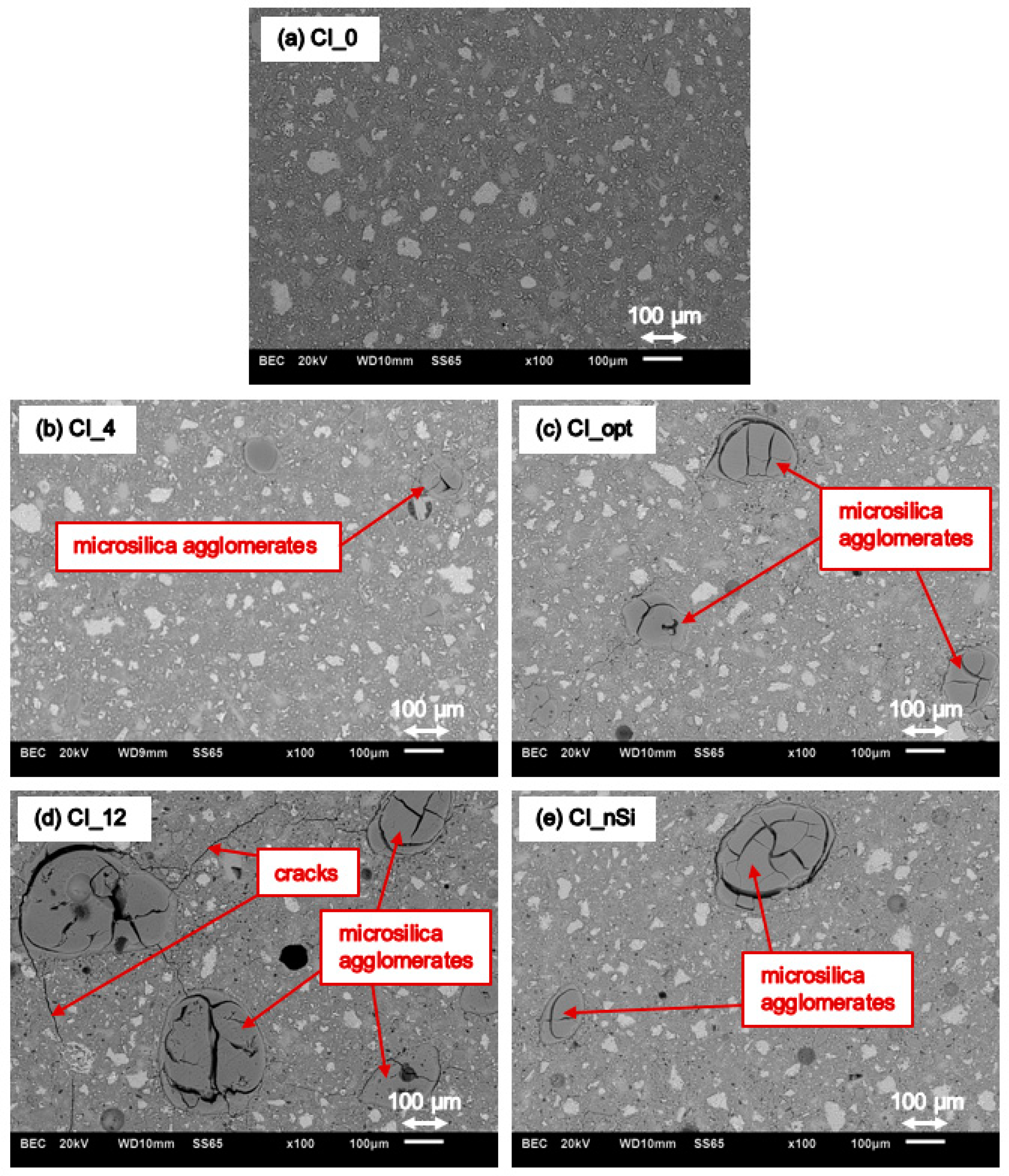
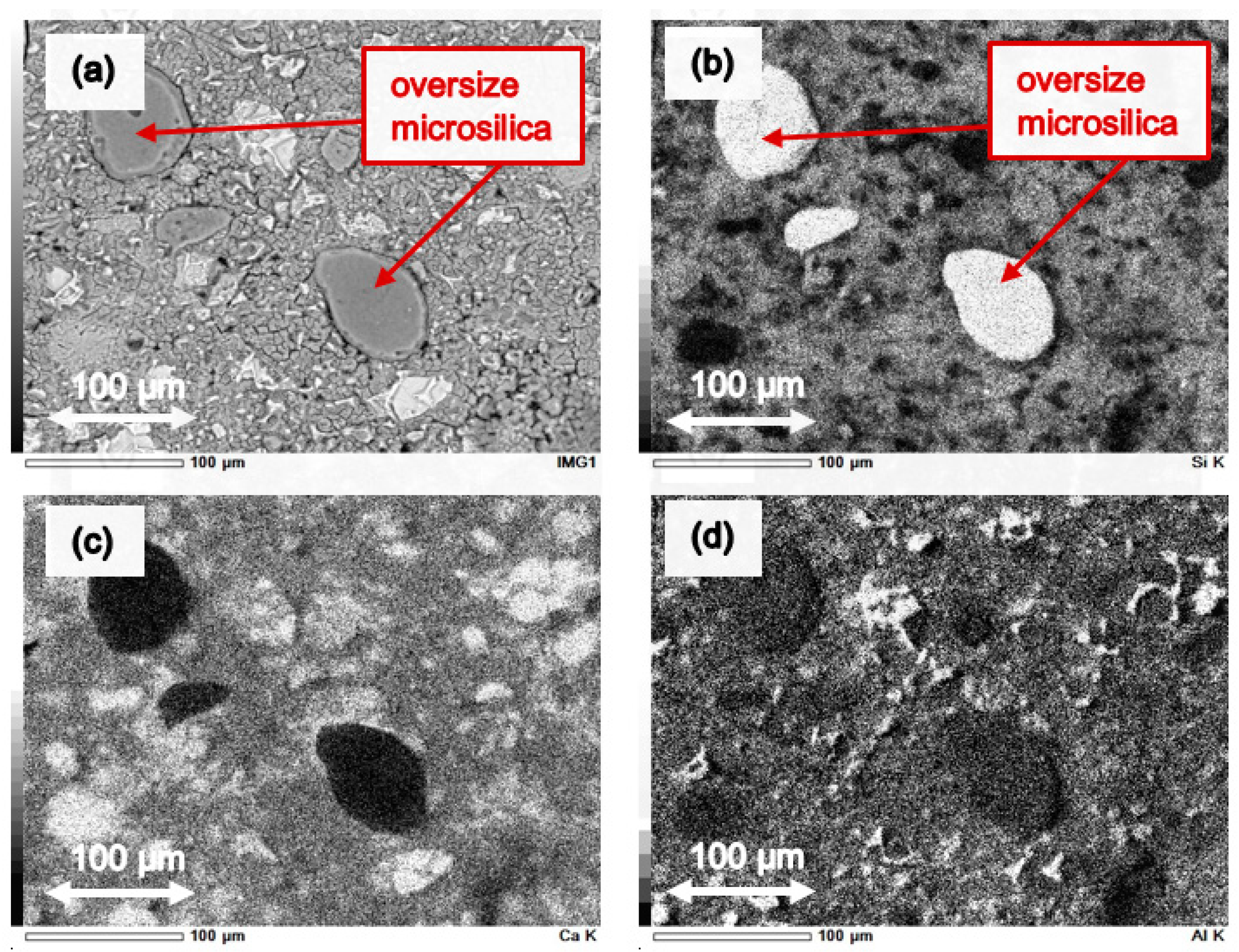
3.5. Microstructural Analysis
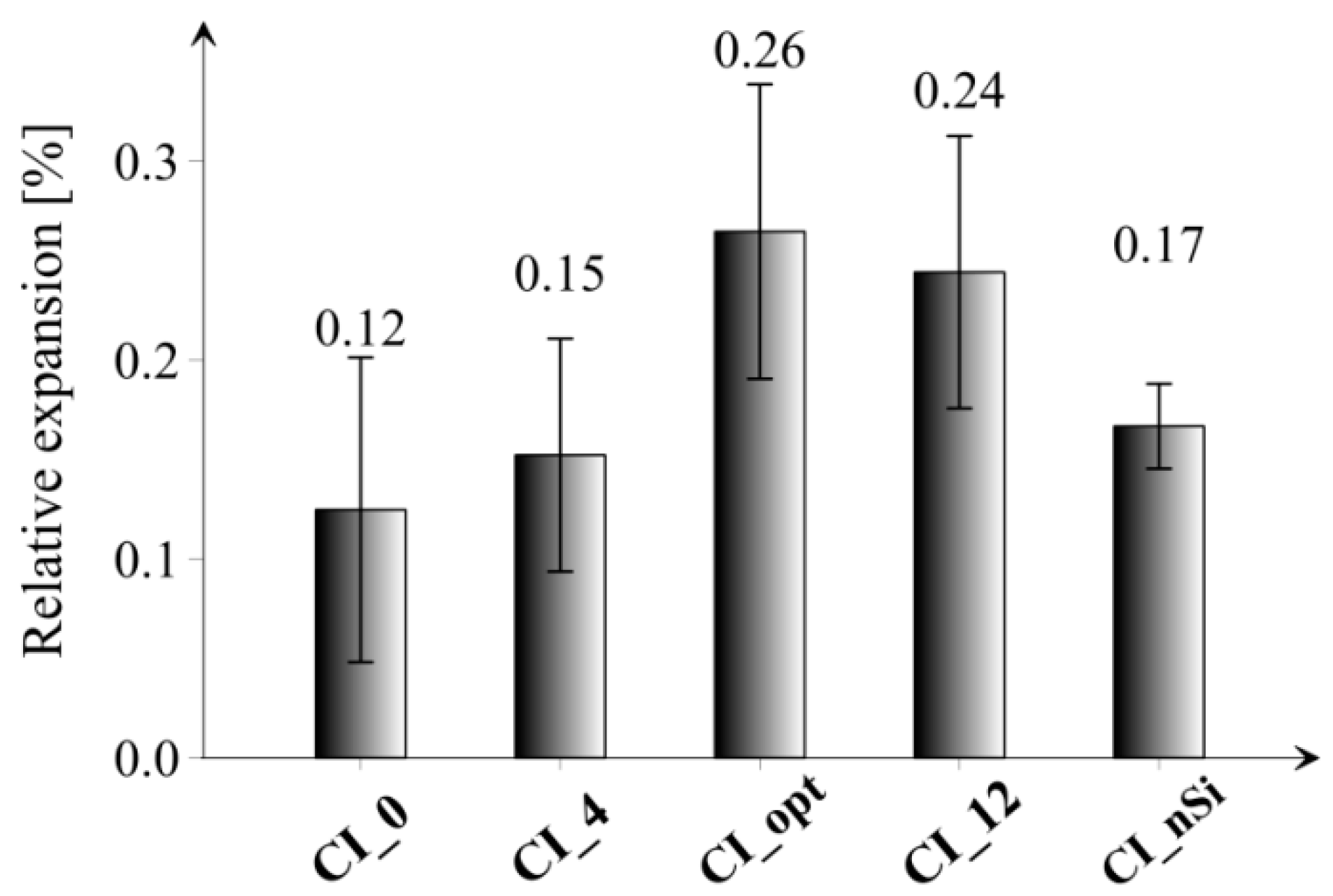
3.6. ASR
3.7. Discussion
4. Conclusions
- The packing density model is an effective tool in the case of designing the cement paste with the addition of the optimal amount of microsilica regarding the lowest porosity. In this case, the use of about 7.5 wt. % of microsilica is optimum. Note that this value depends on the type of microsilica and cement characterized by different particle size distributions.
- On the other hand, if cement paste is designed, mainly in regard to mechanical properties, such as compressive strength and hardness, then the use of the packing density model does not bring satisfactory results. To improve the mechanical properties of cement paste, the use of 4 wt. % of microsilica appeared to be optimal, which value is not a result of applied AA packing density model.
- In the case of using microsilica as an additive to cement paste, the mixing process is an important issue. It may be related with inhomogeneous dispersion of microsilica and creation of agglomerates acting as reactive aggregates that in consequence cause ASR reaction, crack occurrence, and lowered values of mechanical properties.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lothenbach, B.; Scrivener, K.; Hooton, R.D. Supplementary cementitious materials. Cem. Concr. Res. 2011, 41, 1244–1256. [Google Scholar] [CrossRef]
- Siddique, R.; Chahal, N. Use of silicon and ferrosilicon industry by-products (silica fume) in cement paste and mortar. Resour. Conserve. Recycl. 2011, 55, 739–744. [Google Scholar] [CrossRef]
- Behnood, A.; Golafshani, E.M. Predicting the compressive strength of silica fume concrete using hybrid artificial neural network with multi-objective grey wolves. J. Clean. Prod. 2018, 202, 54–64. [Google Scholar] [CrossRef]
- Siddique, R. Utilization of silica fume in concrete: Review of hardened properties. Resour. Conserv. Recy. 2011, 55, 923–932. [Google Scholar] [CrossRef]
- Udgata, G.; Bhowmik, P.; Das, S.P. Effect of Lime on Mechanical Properties of Silica Fume Modified Concrete. In Recent Developments in Sustainable Infrastructure; Springer: Singapore, 2021; pp. 649–657. [Google Scholar]
- Ajileye, F.V. Investigations on microsilica (silica fume) as partial cement replacement in concrete. Glob. J. Res. Eng. 2012, 12, 16–23. [Google Scholar]
- Sobolev, K.; Gutiérrez, M.F. How nanotechnology can change the concrete world. Am. Ceram. Soc. Bull. 2005, 84, 14–18. [Google Scholar] [CrossRef]
- Li, H.; Xiao, H.G.; Yuan, J.; Ou, J. Microstructure of cement mortar with nano-particles. Compos. B Eng. 2004, 35, 185–189. [Google Scholar] [CrossRef]
- Niewiadomski, P.; Hoła, J.; Ćwirzeń, A. Study on properties of self-compacting concrete modified with nanoparticles. Arch. Civ. Mech. Eng. 2018, 18, 877–886. [Google Scholar] [CrossRef]
- Ji, T. Preliminary study on the water permeability and microstructure of concrete incorporating nano-SiO2. Cem. Concr. Res. 2005, 35, 1943–1947. [Google Scholar] [CrossRef]
- Szymanowski, J.; Sadowski, Ł. Functional and adhesive properties of cement-based overlays modified with amorphous silica nanospheres. J. Adhes. 2020, 96, 207–228. [Google Scholar] [CrossRef]
- Beigi, M.H.; Berenjian, J.; Omran, O.L.; Nik, A.S.; Nikbin, I.M. An experimental survey on combined effects of fibers and nanosilica on the mechanical, rheological, and durability properties of self-compacting concrete. Mater. Design. 2013, 34, 1019–1029. [Google Scholar] [CrossRef]
- De Rousseau, M.A.; Kasprzyk, J.R.; Srubar, W.V., III. Computational design optimization of concrete mixtures: A review. Cem. Concr. Res. 2018, 109, 42–53. [Google Scholar] [CrossRef]
- Stefaniuk, D.; Niewiadomski, P.; Musiał, M.; Łydzba, D. Elastic properties of self-compacting concrete modified with nanoparticles: Multiscale approach. Arch. Civ. Mech. Eng. 2020, 19, 1150–1162. [Google Scholar] [CrossRef]
- Nikoo, M.; Torabian, M.F.; Sadowski, Ł. Prediction of concrete compressive strength by evolutionary artificial neural networks. Adv. Mater. Sci. Eng. 2015, 2015, 1–8. [Google Scholar] [CrossRef]
- Golafshani, E.M.; Behnood, A. Estimating the optimal mix design of silica fume concrete using biogeography-based programming. Cem. Concr. Compos. 2019, 96, 95–105. [Google Scholar] [CrossRef]
- Mehta, A.; Ashish, D.K. Silica fume and waste glass in cement concrete production: A review. J. Build. Eng. 2020, 29, 100888. [Google Scholar] [CrossRef]
- Niewiadomski, P. Short Overview of the Effects of Nanoparticles on Mechanical Properties of Concrete. In Key Engineering Materials; Trans Tech Publications, Ltd.: Baech, Switzerland, 2015; Volume 662, pp. 257–260. [Google Scholar] [CrossRef]
- De Larrard, F.; Sedran, T. Optimization of ultra-high-performance concrete by the use of a packing model. Cem. Concr. Res. 1994, 24, 997–1009. [Google Scholar] [CrossRef]
- Hüsken, G. A Multifunctional Design Approach for Sustainable Concrete: With Application to Concrete Mass Products. Ph.D. Thesis, Eindhoven University of Technology, Eindhoven, The Netherlands, 2010. [Google Scholar]
- Snehal, K.; Das, B.B. Application of Andreassen and Modified Andreassen Model on Cementitious Mixture Design: A Review. Recent Dev. Sustain. Infrastruct. 2021, 75, 729–750. [Google Scholar] [CrossRef]
- Mehdipour, I.; Khayat, K.H. Effect of particle-size distribution and specific surface area of different binder systems on packing density and flow characteristics of cement paste. Cem. Concr. Compos. 2017, 78, 120–131. [Google Scholar] [CrossRef]
- Yu, Q.L.; Spiesz, P.; Brouwers, H.J.H. Development of cement-based lightweight composites–Part 1: Mix design methodology and hardened properties. Cem. Concr. Compos. 2013, 44, 17–29. [Google Scholar] [CrossRef] [Green Version]
- Yu, Q.L.; Spiesz, P.; Brouwers, H.J.H. Ultra-lightweight concrete: Conceptual design and performance evaluation. Cem. Concr. Compos. 2015, 61, 18–28. [Google Scholar] [CrossRef]
- Hunger, M. An Integral Design Concept for Ecological Self-Compacting Concrete. Ph.D Thesis, Eindhoven University of Technology, Eindhoven, The Netherlands, 2010. [Google Scholar]
- Yu, Q.L. Design of Environmentally Friendly Calcium Sulfate-Based Building Materials. Towards and Improved Indoor Air Quality. Ph.D Thesis, Eindhoven University of Technology, Eindhoven, The Netherlands, 2012. [Google Scholar]
- Yu, R.; Spiesz, P.; Brouwers, H.J.H. Development of ultra-high performance fibre reinforced concrete (UHPFRC): Towards an efficient utilization of binders and fibres. Constr. Build. Mater. 2015, 79, 273–282. [Google Scholar] [CrossRef] [Green Version]
- Leemann, A.; Le Saout, G.; Winnefeld, F.; Rentsch, D.; Lothenbach, B. Alkali–silica reaction: The influence of calcium on silica dissolution and the formation of reaction products. J. Am. Ceram. Soc. 2011, 94, 1243–1249. [Google Scholar] [CrossRef]
- Mohammadi, A.; Ghiasvand, E.; Nili, M. Relation between mechanical properties of concrete and alkali-silica reaction (ASR); A review. Constr. Build. Mater. 2020, 258, 119567. [Google Scholar] [CrossRef]
- Lindgård, J.; Andiç-Çakır, Ö.; Fernandes, I.; Rønning, T.F.; Thomas, M.D.A. Alkali–silica reactions (ASR): Literature review on parameters influencing laboratory performance testing. Cem. Concr. Res. 2012, 42, 223–243. [Google Scholar] [CrossRef] [Green Version]
- Duchesne, J.; Bérubé, M.A. Long-term effectiveness of supplementary cementing materials against alkali–silica reaction. Cem. Concr. Res. 2001, 31, 1057–1063. [Google Scholar] [CrossRef]
- Leemann, A.; Bernard, L.; Alahrache, S.; Winnefeld, F. ASR prevention—Effect of aluminum and lithium ions on the reaction products. Cem. Concr. Res. 2015, 76, 192–201. [Google Scholar] [CrossRef]
- Hussain, S.E. Effect of microsilica and blast furnace slag on pore solution composition and alkali-silica reaction. Cem. Concr. Compos. 1991, 13, 219–225. [Google Scholar]
- Pettersson, K. Effects of silica fume on alkali-silica expansion in mortar specimens. Cem. Concr. Res. 1992, 22, 15–22. [Google Scholar] [CrossRef]
- Diamond, S. Alkali silica reactions—some paradoxes. Cem. Concr. Composites 1997, 19, 391–401. [Google Scholar] [CrossRef]
- PN-EN 197-1:2012—(Polish Version) Cement—Part 1: Composition, Specifications and Conformity Criteria for Common Cements; Polish Committee for Standarization: Warsaw, Poland, 2012.
- PN-EN 13263-1+A1:2010—(Polish Version) Silica Fume for Concrete—Part 1: Definitions, Requirements and Conformity Criteria; Polish Committee for Standarization: Warsaw, Poland, 2010.
- De Larrard, F.; Sedran, T. Mixture-proportioning of high-performance concrete. Cem. Concr. Res. 2002, 32, 1699e1704. [Google Scholar] [CrossRef]
- Andreasen, A.H.M.; Andersen, J. Ueber die Beziehung zwischen Kornabstufung und Zwischenraum in Produkten aus losen Körnern (mit einigen Experimenten). Kolloid-Zeitschrift 1930, 50, 217–228. (In German) [Google Scholar] [CrossRef]
- PN-EN 196-1:2016—(Polish Version) Methods of Testing Cement—Part 1: Determination of Strength; Polish Committee for Standarization: Warsaw, Poland, 2016.
- PN-EN 196-6:2019-01—(Polish Version) Methods of Testing Cement—Part 6: Determination of Fineness; Polish Committee for Standarization: Warsaw, Poland, 2019.
- PN-EN 12390-7:2019-8—(Polish Version) Testing Hardened Concrete—Part 7: Density of Hardened Concrete; Polish Committee for Standarization: Warsaw, Poland, 2019.
- Sneddon, I.N. The relation between load and penetration in the axisymmetric Boussinesq problem for a punch of arbitrary profile. Int. J. Eng. Sci. 1965, 3, 47–57. [Google Scholar] [CrossRef]
- Oliver, W.C.; Pharr, G.M. Measurement of hardness and elastic modulus by instrumented indentation: Advances in understanding and refinements to methodology. J. Mater. Res. 2004, 19, 3–20. [Google Scholar] [CrossRef]
- Meng, W.; Kumar, A.; Khayat, K.H. Effect of silica fume and slump-retaining polycarboxylate-based dispersant on the development of properties of portland cement paste. Cem Concr Compos. 2019, 99, 181–190. [Google Scholar] [CrossRef]
- Niewiadomski, P.; Stefaniuk, D. Creep Assessment of the Cement Matrix of Self-Compacting Concrete Modified with the Addition of Nanoparticles Using the Indentation Method. Appl. Sci. 2020, 10, 2442. [Google Scholar] [CrossRef] [Green Version]
- Bumanis, G.; Bajare, D.; Locs, J.; Korjakins, A. Alkali-silica reactivity of foam glass granules in structure of lightweight concrete. Constr. Build. Mater. 2013, 47, 274–281. [Google Scholar] [CrossRef]
- Chen, J.J.; Ng, P.L.; Chu, S.H.; Guan, G.X.; Kwan, A.K.H. Ternary blending with metakaolin and silica fume to improve packing density and performance of binder paste. Constr. Build. Mater. 2020, 252, 119031. [Google Scholar] [CrossRef]








| Component | [wt.%] | Phase | [wt.%] |
|---|---|---|---|
| CaO | 66.00 | Tricalcium silicate (C3S) | 56.46 |
| SiO2 | 21.50 | Dicalcium silicate (C2S) | 19.29 |
| Al2O3 | 5.60 | Tricalcium aluminate (C3A) | 10.11 |
| Fe2O3 | 2.80 | Tetracalcium aluminoferrite (C4AF) | 8.51 |
| MgO | 1.50 | Gypsum (CSH2) | 5.38 |
| SO3 | 2.50 | ||
| Cl− | 0.08 | ||
| Loss of ignition | 3.30 |
| Component | [wt.%] |
|---|---|
| SiO2 | ≥85.00 |
| SO3 | <2.00 |
| Cl− | <0.30 |
| Free CaO | <1.00 |
| Free Si | <0.40 |
| Loss of ignition | <4.00 |
| Cement Paste | Microsilica [kg/m3] (wt.%) | Nanoparticles [kg/m3] (wt.%) | Cement [kg/m3] | Water [kg/m3] | SP [kg/m3] |
|---|---|---|---|---|---|
| CI_0 | – | – | 1463 | 527 | 15 |
| CI_4 | 55 (4.00%) | – | 1387 | 519 | 14 |
| CI_opt | 101 (7.63%) | – | 1325 | 514 | 13 |
| CI_12 | 151 (12.00%) | – | 1258 | 507 | 13 |
| CI_nSi | 120 (9.34%) | 12 (0.93%) | 1286 | 511 | 13 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Niewiadomski, P.; Karolak, A.; Stefaniuk, D.; Królicka, A.; Szymanowski, J.; Sadowski, Ł. Cement Paste Mixture Proportioning with Particle Packing Theory: An Ambiguous Effect of Microsilica. Materials 2021, 14, 6970. https://doi.org/10.3390/ma14226970
Niewiadomski P, Karolak A, Stefaniuk D, Królicka A, Szymanowski J, Sadowski Ł. Cement Paste Mixture Proportioning with Particle Packing Theory: An Ambiguous Effect of Microsilica. Materials. 2021; 14(22):6970. https://doi.org/10.3390/ma14226970
Chicago/Turabian StyleNiewiadomski, Paweł, Anna Karolak, Damian Stefaniuk, Aleksandra Królicka, Jacek Szymanowski, and Łukasz Sadowski. 2021. "Cement Paste Mixture Proportioning with Particle Packing Theory: An Ambiguous Effect of Microsilica" Materials 14, no. 22: 6970. https://doi.org/10.3390/ma14226970
APA StyleNiewiadomski, P., Karolak, A., Stefaniuk, D., Królicka, A., Szymanowski, J., & Sadowski, Ł. (2021). Cement Paste Mixture Proportioning with Particle Packing Theory: An Ambiguous Effect of Microsilica. Materials, 14(22), 6970. https://doi.org/10.3390/ma14226970









