Comparison of Calculation Methods of Elastic Bonding: Limits of the Gamma Method Using an Example of a Wood–Concrete Composite Floor with Single Loads
Abstract
:1. Introduction
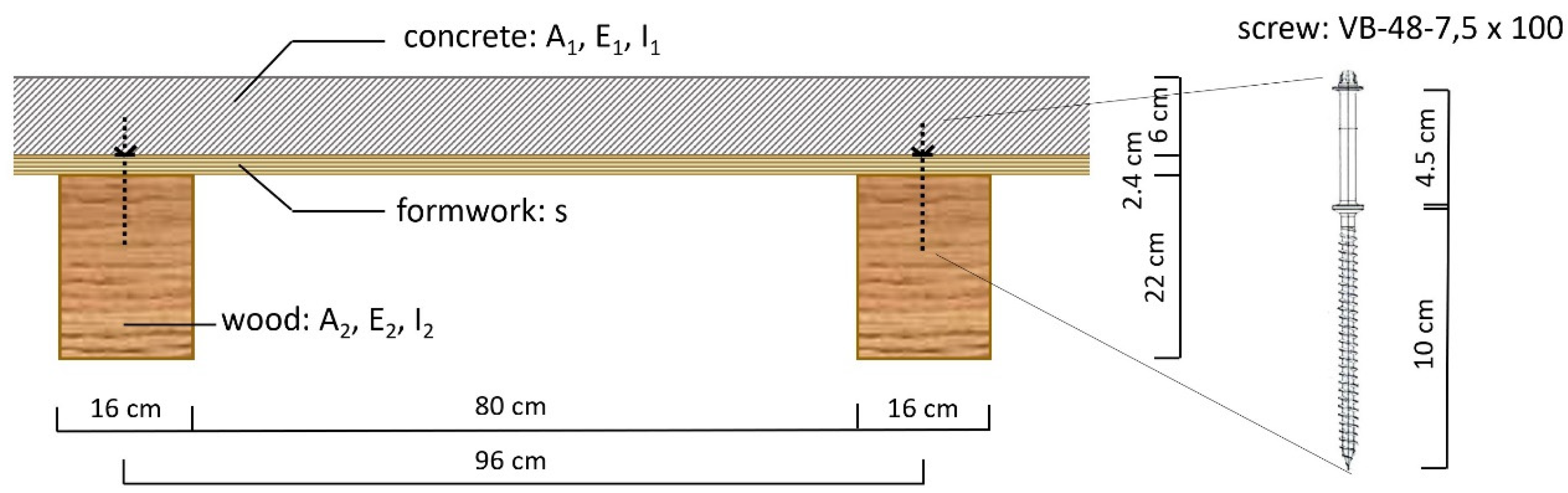
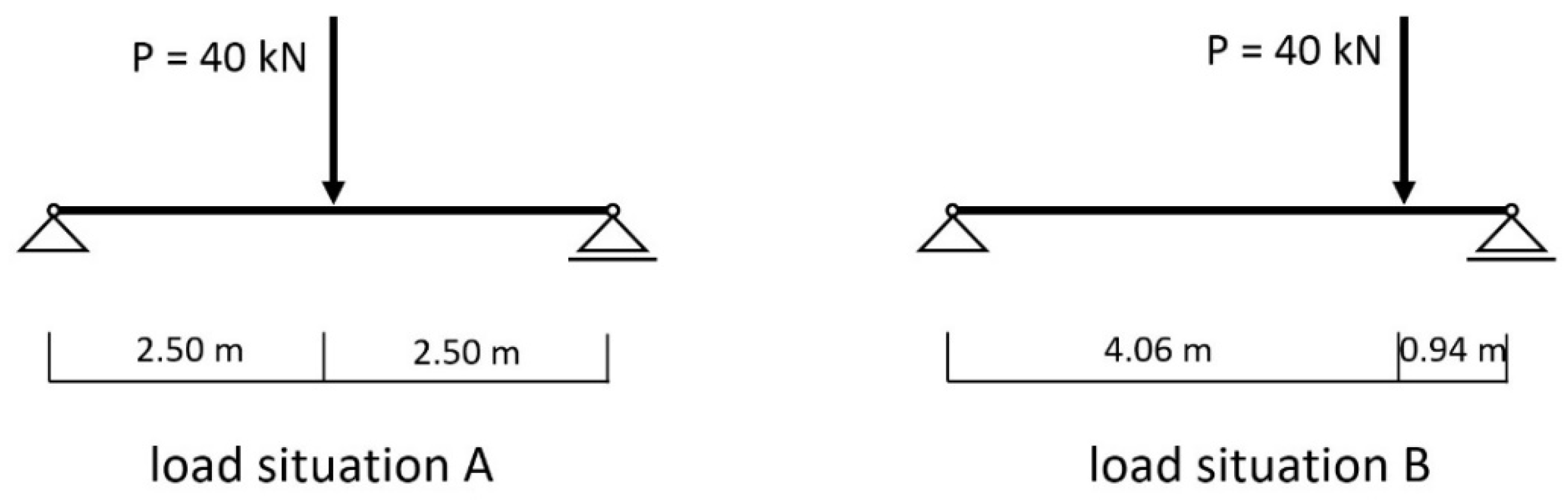
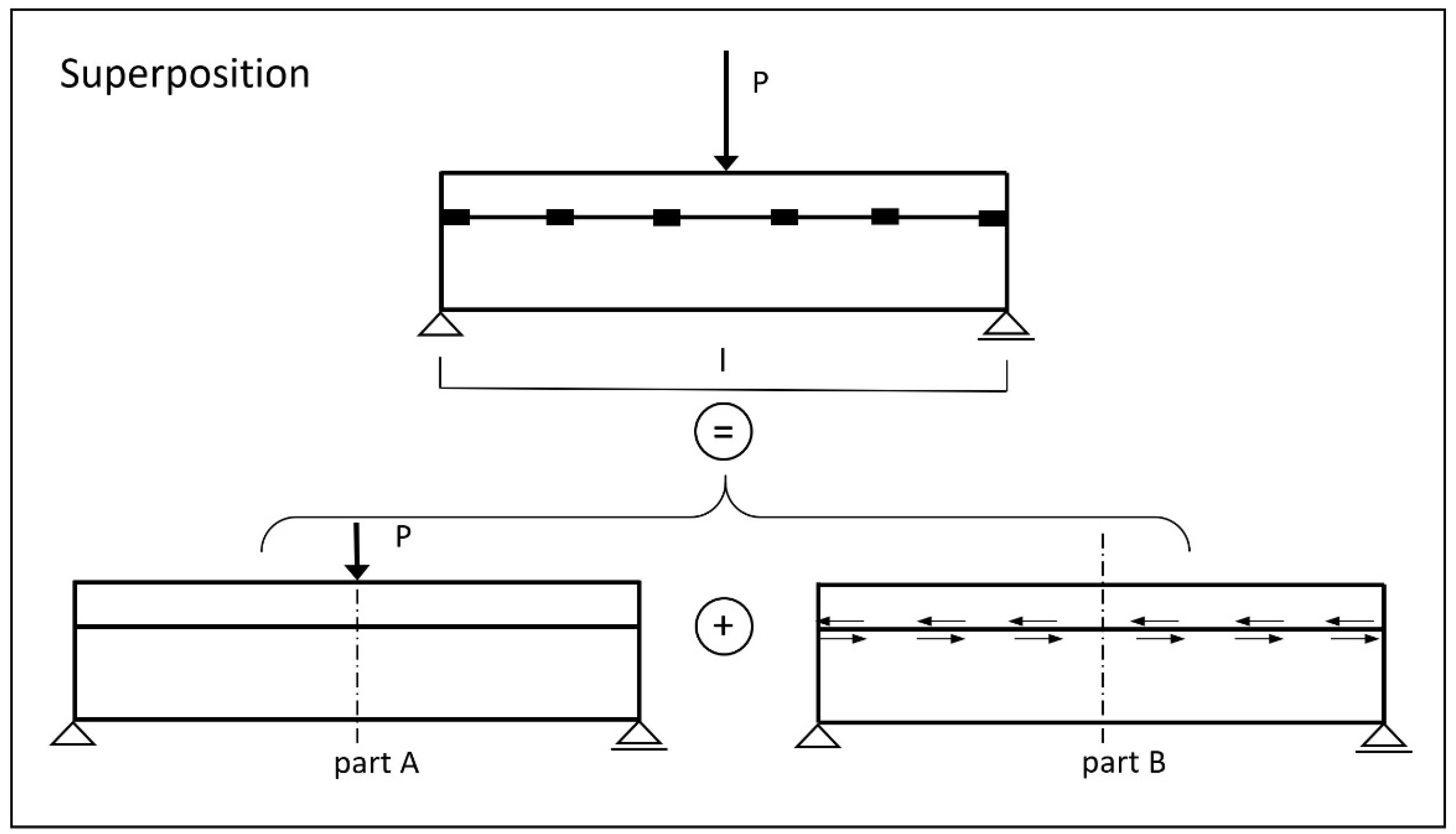
2. Static System
3. Theory and Calculation
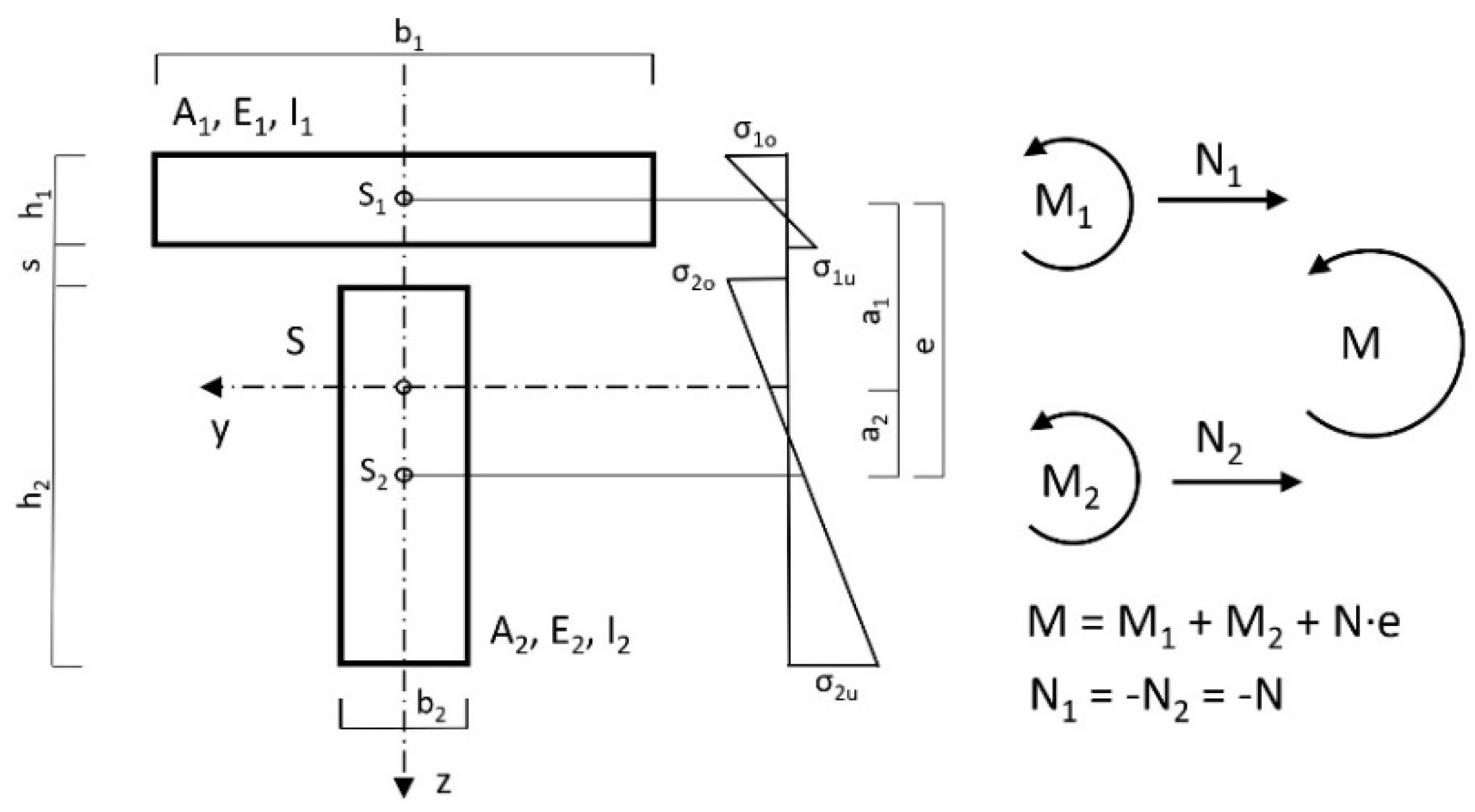
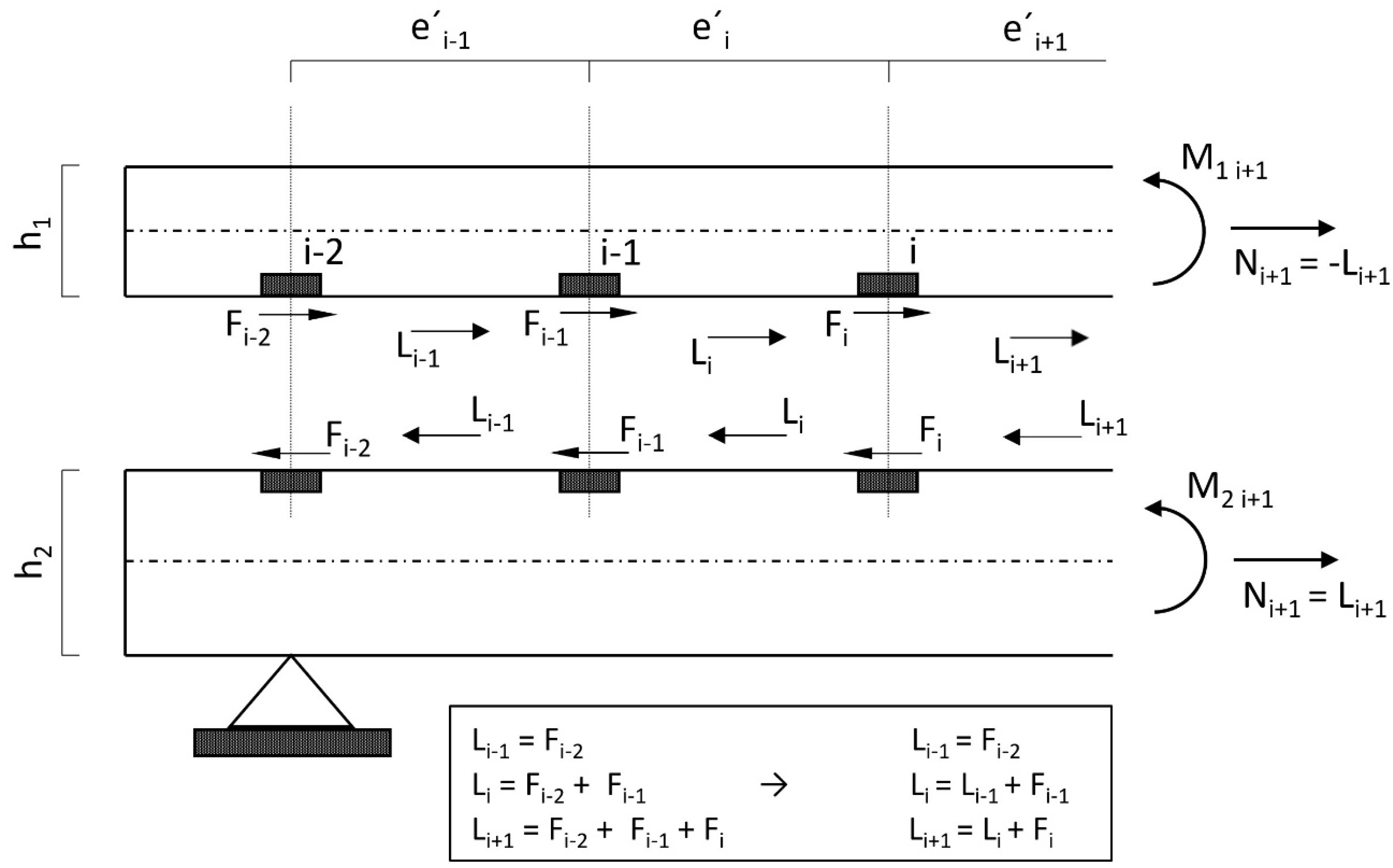
3.1. Calculation of Force Quantities with the Discrete Method
3.1.1. Partial Moments Due to the External Load
3.1.2. Partial Moments According to Dowel Forces
3.1.3. Compatibility Condition
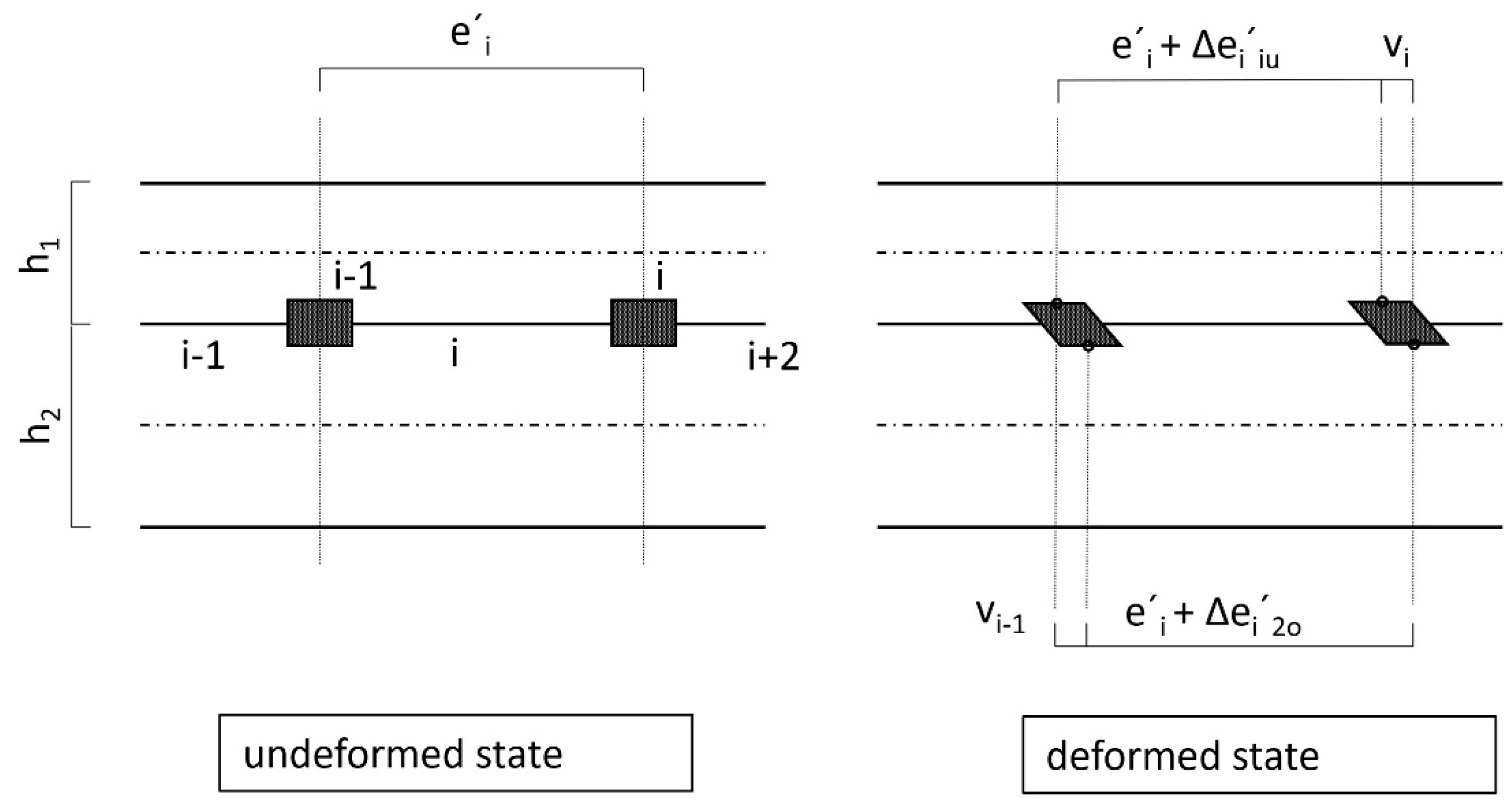
3.2. Derivation of Deformations for the Discrete Method
3.3. Calculation of Force Quantities and Deformations with the Differential Equation Method
3.4. Gamma Method
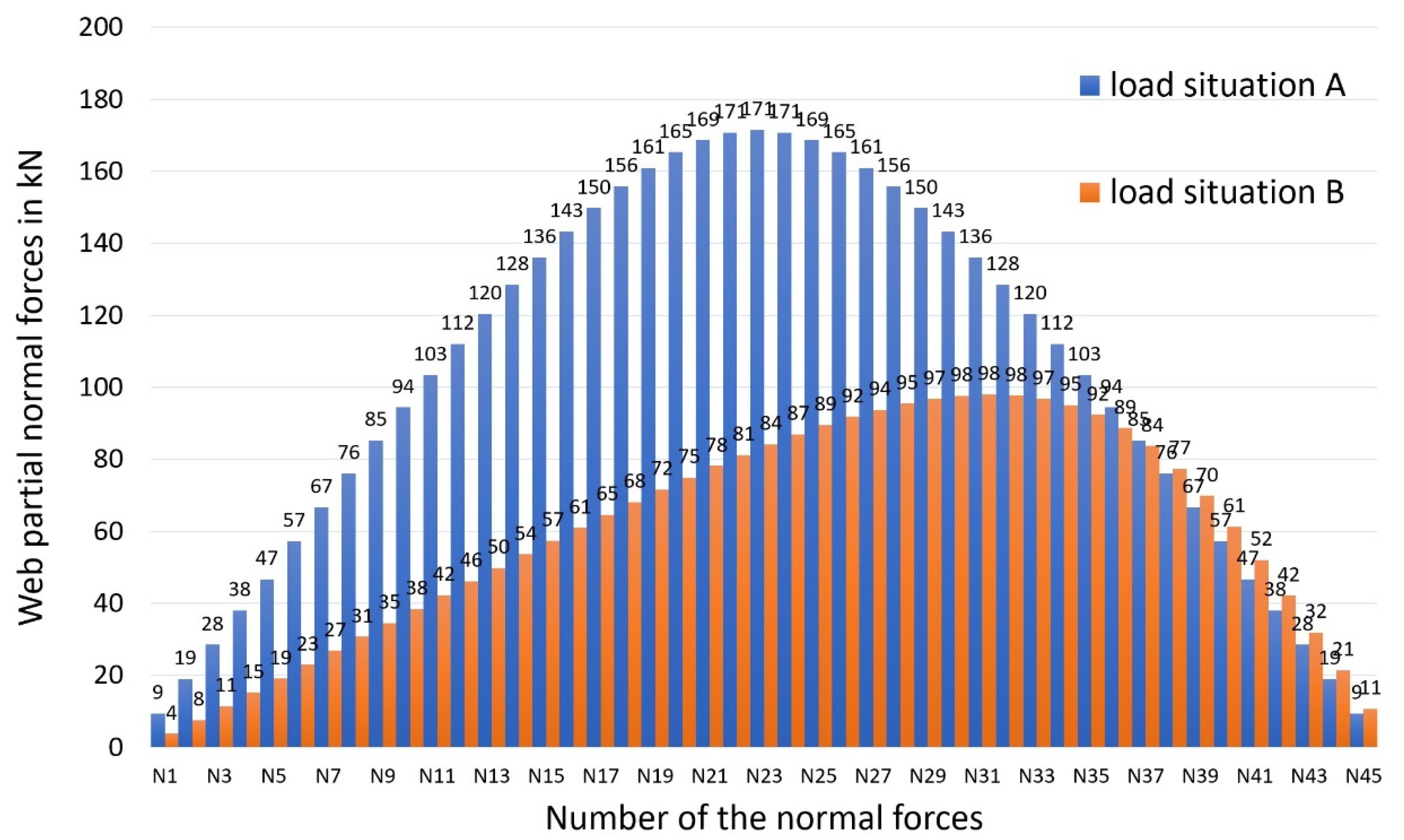
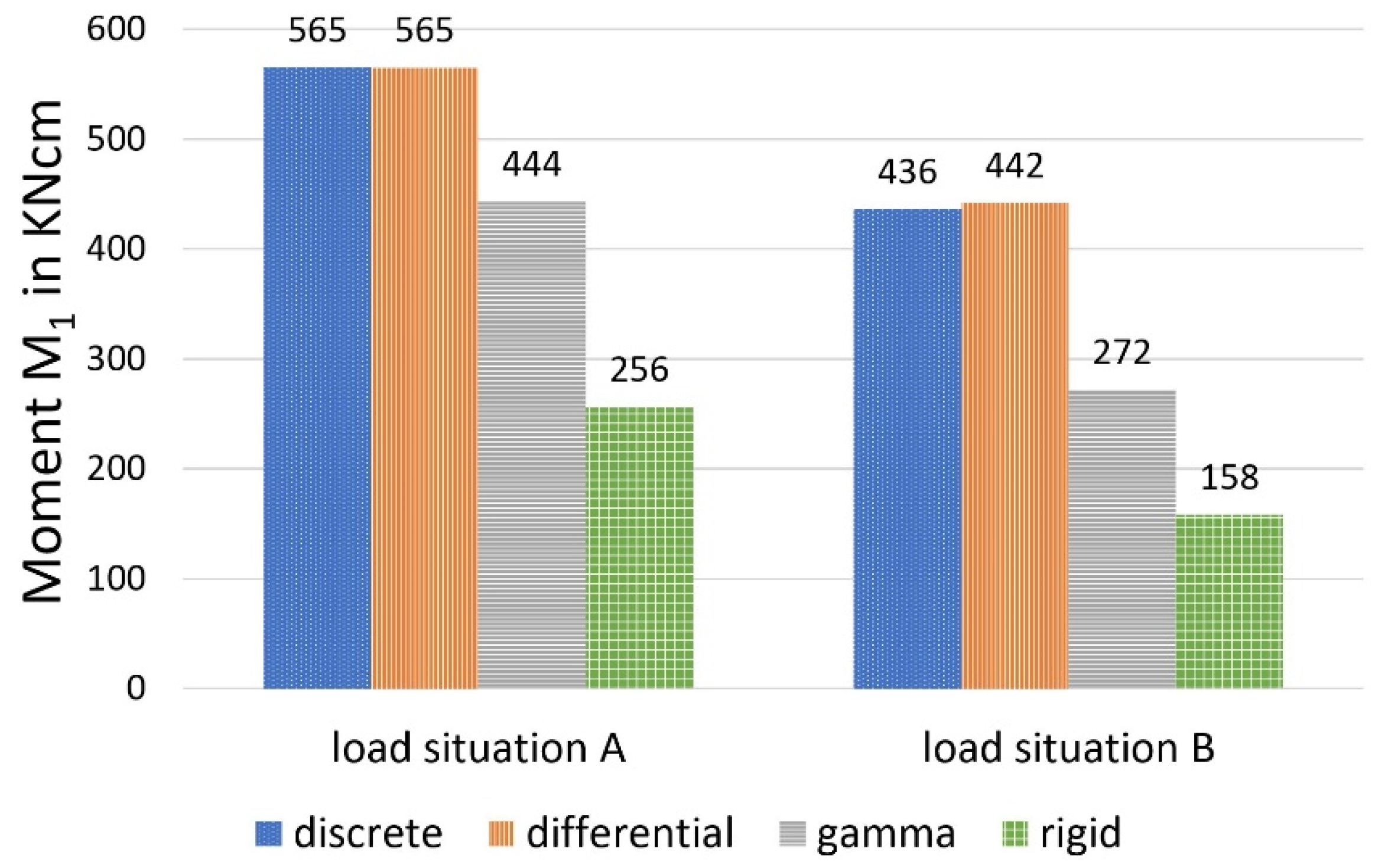
4. Comparison of the Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Holschemacher, K.; Selle, R.; Schmidt, J.; Kieslich, H. Wood-Concrete-Composite; Betonkalender: Berlin, Germany, 2013; pp. 241–287. (In German) [Google Scholar]
- Yeoh, D.; Fragiacomo, M.; de Franceschi, M.; Boon, K.H. State of the Art on Timber-Concrete Composite Structures: Literature Review. J. Struct. Eng. 2011, 137, 1085–1095. [Google Scholar] [CrossRef]
- Grosse, M.; Rautenstrauch, K. Numerical modelling of timber and connection elements used in timber-concrete-composite constructions. Edinburgh 2004, 17, CIB-W18/37-7-15. [Google Scholar]
- Kolbitsch, A.; Pauser, A.; Bölcskey, E.; Zajicek, P. Reinforcement of Existing Wooden Ceilings with Consideration of Subsequently Manufactured Wood-Concrete Composite Structures, Research Project F 1021—Part Wooden Ceiling Structures; Österreichische Gesellschaft zur Erhaltung von Bauten: Wien, Austria, 1992. (In German) [Google Scholar]
- Grosse, M.; Hartnack, R.; Lehmann, S.; Rautenstrauch, K. Modeling of discontinuously connected wood-concrete composite slabs/Part 1: Short-term behavior. Bautechnik 2003, 80, 534–541. (In German) [Google Scholar] [CrossRef]
- Kuhlmann, U.; Schänzlin, J.; Michelfelder, B. Calculation of wood-concrete composite floors. Beton-Und Stahlbetonbau 2004, 99, 262–270. (In German) [Google Scholar] [CrossRef]
- Grosse, M.; Hartnack, R.; Rautenstrauch, K. Modeling of discontinuously connected wood-concrete composite slabs/Part 2: Long-term behavior. Bautechnik 2003, 80, 693–701. (In German) [Google Scholar] [CrossRef]
- Avak, R.; Glaser, R. Simplified method for predicting the long-term behavior of wood-concrete composite structures. Bautechnik 2005, 82, 200–211. (In German) [Google Scholar] [CrossRef]
- Gerold, M.; Schänzlin, J.; Kuhlmann, U. Wood as an ideal composite partner for concrete as a building material. Bautechnik 2003, 80, 840–845. (In German) [Google Scholar] [CrossRef]
- Schmidt, J.; Schneider, W.; Thiel, R. To design wood/concrete composite beams. Bautechnik 2003, 80, 302–309. (In German) [Google Scholar] [CrossRef]
- Schmidt, J.; Kaliske, M.; Schneider, W.; Thiele, R. Design proposal for wood/concrete composite beams considering graded fastener spacings. Bautechnik 2004, 81, 172–179. [Google Scholar] [CrossRef]
- Rautenstrauch, K.; Grosse, M.; Lehmann, S.; Hartnack, R. Practical dimensioning of wood-concrete composite floors, 6. Informationstag des IKI; Bauhaus Universität Weimar: Weimar, Germany, 2003. (In German) [Google Scholar]
- Forsberg, A.; Farbäck, F. Timber Concrete Composite Floors with Cross Laminated Timber—Structural Behaviour & Design, Division of Structural Engineering, Faculty of Engineering; Report TVBK-20/5279; Lund University: Lund, Sweden, 2020. [Google Scholar]
- Wallner-Novak, M.; Koppelhuber, J.; Pock, K. Cross-Laminated Timber Structural Design|Basic Design and Engineering Principles According to Eurocode; Proholz: Vienna, Austria, 2014. (In German) [Google Scholar]
- Heller, H. Wood-concrete composite floors (HBV). Bautechnik 2008, 85, 667–677. (In German) [Google Scholar] [CrossRef]
- Schröter, H.; Simon, A.; Timmler, H.-G.; Rautenstrauch, K.; Raue, E. For the calculation of wood-concrete composite beams with methods of mathematical optimization. Bautechnik 2010, 87, 474–481. (In German) [Google Scholar]
- Schäfers, M.; Seim, W. Bonded composite components made of wood and high-strength and ultra-high-performance. Bautechnik 2011, 88. (In German) [Google Scholar]
- Grosse, M.; Rautenstrauch, K.; Schlegel, R. Numerical modeling of wood and fasteners in wood-concrete composite structures. Bautechnik 2005, 82, 355–364. (In German) [Google Scholar] [CrossRef]
- Schönborn, F.; Flach, M.; Feix, J. Design rules and design information for shear collars in wood-concrete composite structures. Beton-Und Stahlbetonbau 2011, 106, 385–393. (In German) [Google Scholar] [CrossRef]
- Woschitz, R.; Deix, K.; Huber, C.; Kampitsch, T. Development of innovative wood-concrete composite slabs in prefabricated construction method. Bautechnik 2021, 98, 12–22. (In German) [Google Scholar] [CrossRef]
- Dias, A.; Schänzlin, J.; Dietsch, P. Design of Timber-Concrete Composite Structures; Shaker Verlag GmbH: Aachen, Germany, 2018. [Google Scholar] [CrossRef]
- Möhler, K. On the Load-Carrying Behavior of Flexural Beams and Compression Members with Composite Cross-Sections and Yielding Connectors; TH Karlsruhe: Karlsruhe, Germany, 1956. (In German) [Google Scholar]
- Heimeshoff, B. To calculate flexural beams made of yieldingly connected cross-section parts in engineered timber construction. Holz Als Roh-Und Werkst. 1987, 45, 237–241. (In German) [Google Scholar] [CrossRef]
- ÖNORM EN 1995-1-1, Eurocode 5. Designs of Timber Strutures, Part 1-1: General—Common Rules and Rules for Buildings; Austrian Standards: Vienna, Austria, 2019. [Google Scholar]
- Natterer, J.; Hoeft, M. The Load-Bearing Behavior of Wood-Concrete Composite Structures; Forschungsbericht CERS Nr. 1345; EPFL/IBOIS: Lausanne, Switzerland, 1987. (In German) [Google Scholar]
- Stüssi, F. About the doweled beam. Schweiz. Bauz. 1943, 122, 271–274. (In German) [Google Scholar]
- Stüssi, F. Composite Solid Wall Beams; Abhandlung der Int. Ver. für Brückenbau und Hochbau: Zürich, Switzerland, 1947; pp. 249–268. [Google Scholar]
- Huber, C. Document on the Design of Wood-Concrete Composite Floors with Selected Fasteners under Fire Exposure; Institut für Hochbau und Technologie TU Wien: Vienna, Austria, 2010. (In German) [Google Scholar]
- Zulassungsnummer Z-9.1-342. SFS-Compound Screws, VB-48-7,5x100 as a Fastener for the SFS Wood-Concrete Composite System; Allgemeine Bauaufsichtliche Zulassung: Berlin, Germany, 2003. (In German) [Google Scholar]

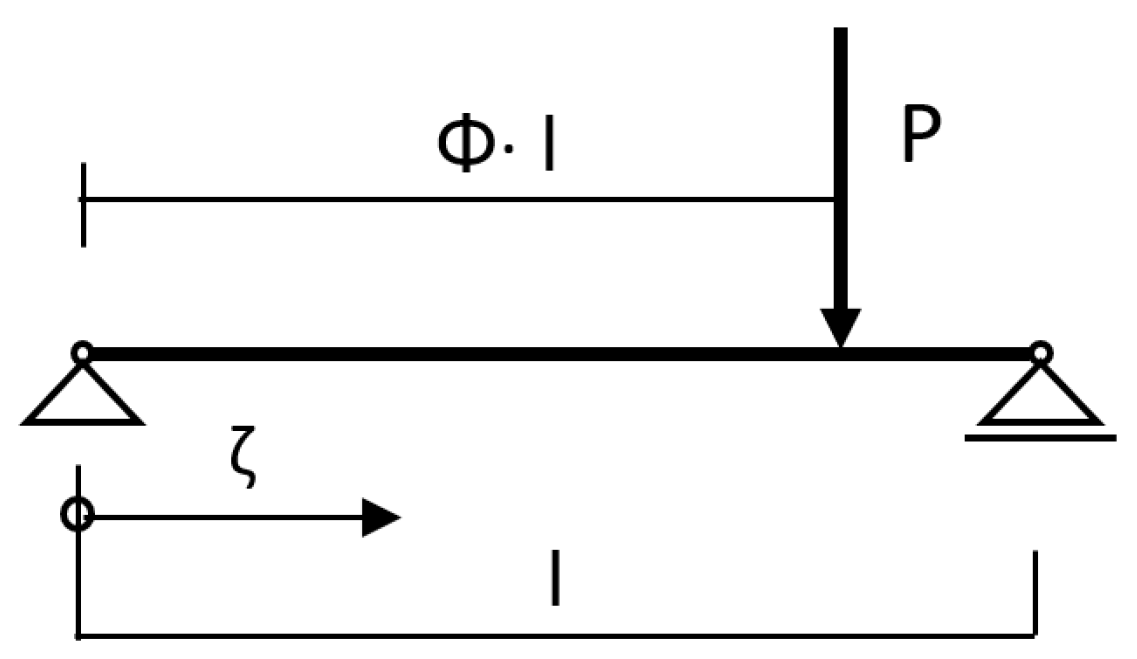




| Plate | Beam | ||
|---|---|---|---|
| Concrete | C20/25 | Wood | C22 |
| Elastic modulus E1 | 3000 kN/cm2 | Elastic modulus E2 | 1000 kN/cm2 |
| Thickness h1 | 6 cm | Beam high h2 | 22 cm |
| Contributing plate width b1 | 91 cm | Beam width b2 | 16 cm |
| Area A1 | 546 cm2 | Area A2 | 352 cm2 |
| Moment of Inertia I1 | 1638 cm4 | Moment of Inertia I2 | 14,197 cm4 |
| Centre of mass distance e | 16.4 cm | Thickness of the formwork s | 2.4 cm |
| Load Situation | M1 | M2 | N | σ1o | σ2u |
|---|---|---|---|---|---|
| A | 565 kNcm | 1622 kNcm | 171.5 kN | −1.35 kN/cm2 | 1.74 kN/cm2 |
| B | 436 kNcm | 1255 kNcm | 83.7 kN | −0.95 kN/cm2 | 1.21 kN/cm2 |
| Load Situation | M1 | M2 | N | σ1o | σ2u |
|---|---|---|---|---|---|
| A | 565 kNcm | 1632 kNcm | 171.0 kN | −1.35 kN/cm2 | 1.75 kN/cm2 |
| B | 442 kNcm | 1277 kNcm | 82.0 kN | −0.96 kN/cm2 | 1.22 kN/cm2 |
| Load Situation | M1 | M2 | N | σ1o | σ2u |
|---|---|---|---|---|---|
| A | 256 kNcm | 741 kNcm | 244.1 kN | −0.92 kN/cm2 | 1.27 kN/cm2 |
| B | 158 kNcm | 458 kNcm | 149.2 kN | −0.56 kN/cm2 | 0.78 kN/cm2 |
| Load Situation | M1 | M2 | N | σ1o | σ2u |
|---|---|---|---|---|---|
| A | 444 kNcm | 1282 kNcm | 199.7 kN | −1.18 kN/cm2 | 1.56 kN/cm2 |
| B | 272 kNcm | 785 kNcm | 122.3 kN | −0.72 kN/cm2 | 0.96 kN/cm2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huber, C.; Deix, K. Comparison of Calculation Methods of Elastic Bonding: Limits of the Gamma Method Using an Example of a Wood–Concrete Composite Floor with Single Loads. Materials 2021, 14, 7211. https://doi.org/10.3390/ma14237211
Huber C, Deix K. Comparison of Calculation Methods of Elastic Bonding: Limits of the Gamma Method Using an Example of a Wood–Concrete Composite Floor with Single Loads. Materials. 2021; 14(23):7211. https://doi.org/10.3390/ma14237211
Chicago/Turabian StyleHuber, Christian, and Karl Deix. 2021. "Comparison of Calculation Methods of Elastic Bonding: Limits of the Gamma Method Using an Example of a Wood–Concrete Composite Floor with Single Loads" Materials 14, no. 23: 7211. https://doi.org/10.3390/ma14237211
APA StyleHuber, C., & Deix, K. (2021). Comparison of Calculation Methods of Elastic Bonding: Limits of the Gamma Method Using an Example of a Wood–Concrete Composite Floor with Single Loads. Materials, 14(23), 7211. https://doi.org/10.3390/ma14237211





