Backward Flux Re-Deposition Patterns during Multi-Spot Laser Ablation of Stainless Steel with Picosecond and Femtosecond Pulses in Air
Abstract
:1. Introduction
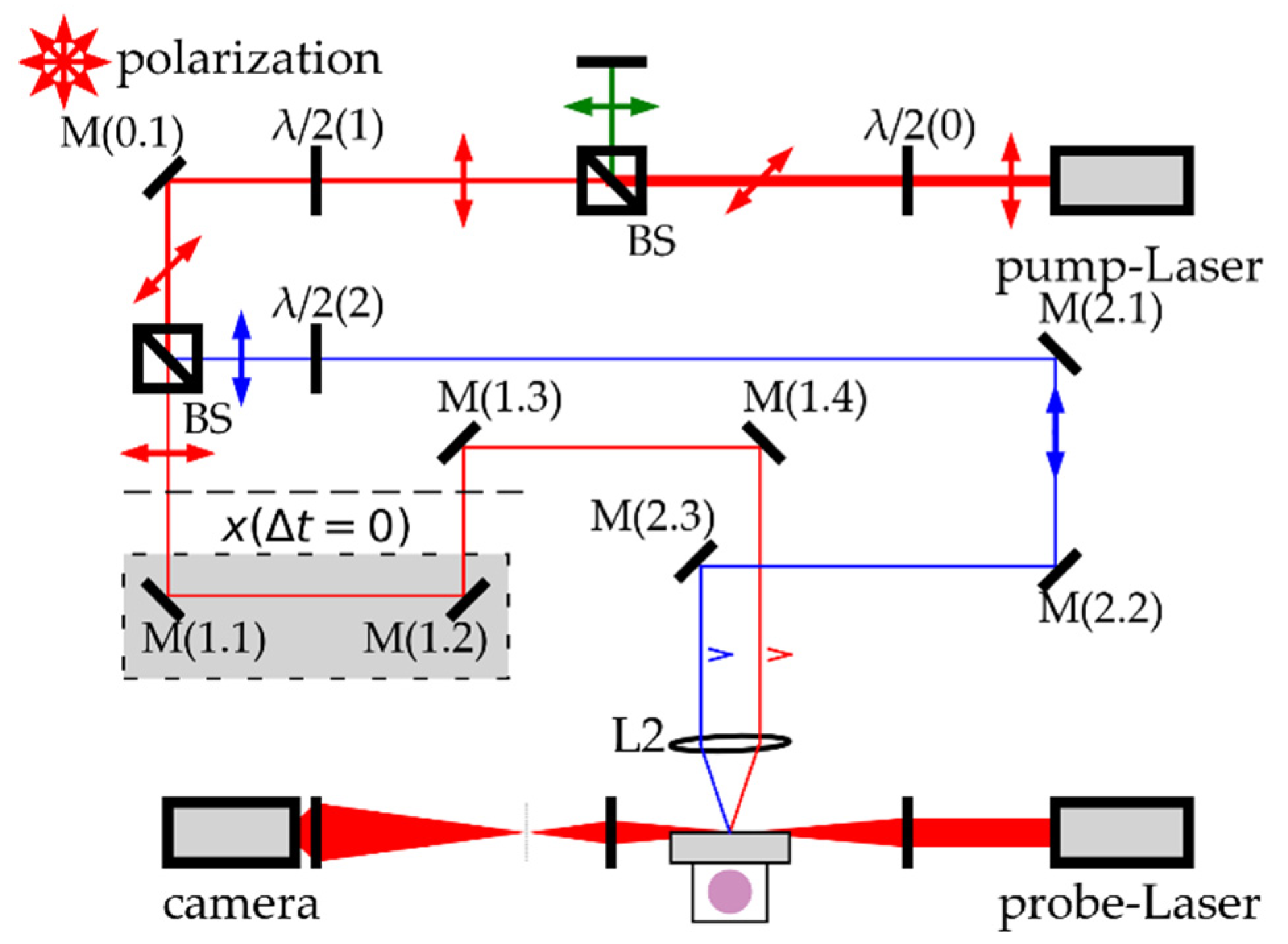
2. Materials and Methods
3. Results
3.1. 2-Spot Ablation with 10 ps Pulses at 1064 nm/5 kHz Repetition Rate
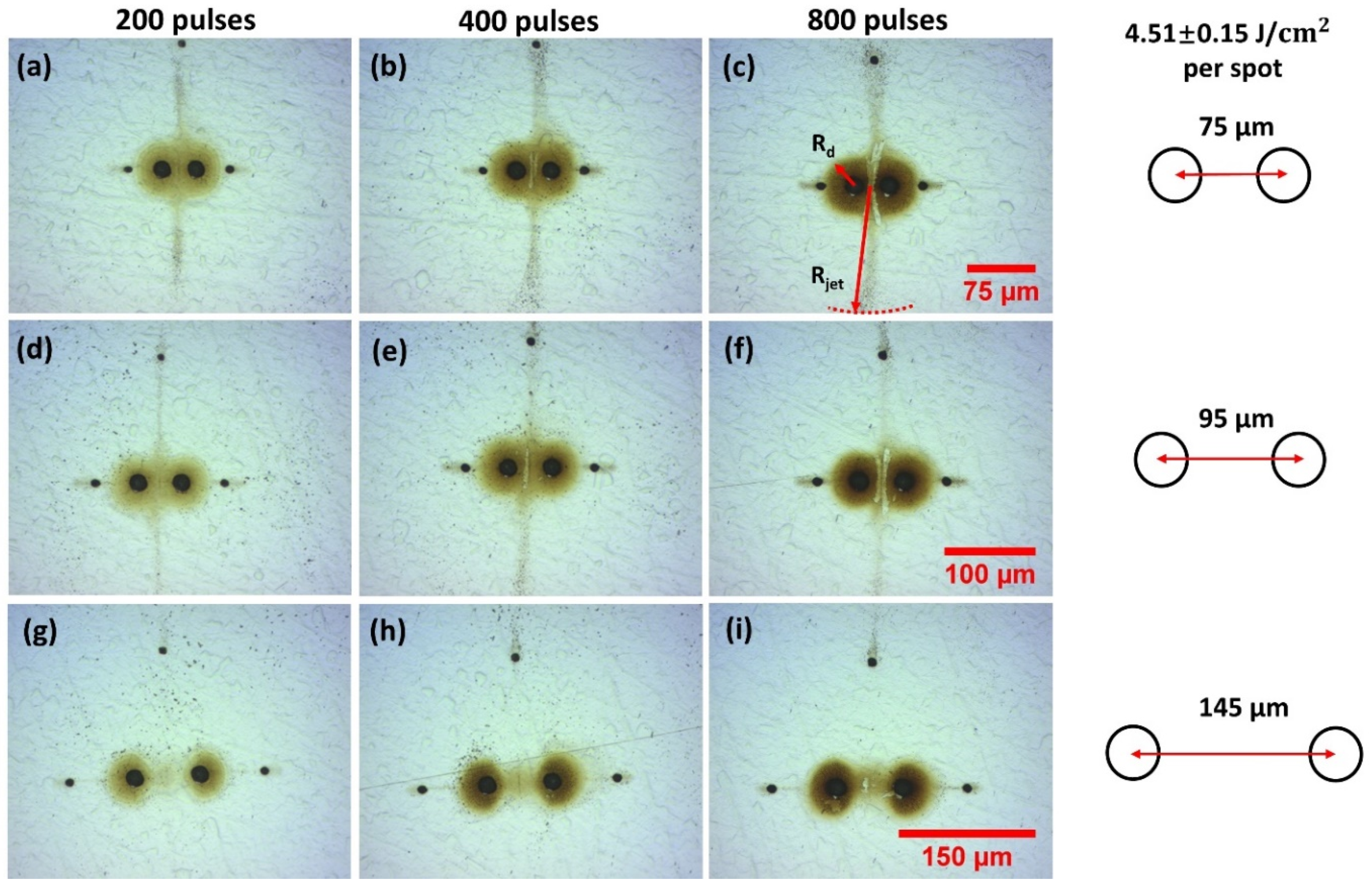
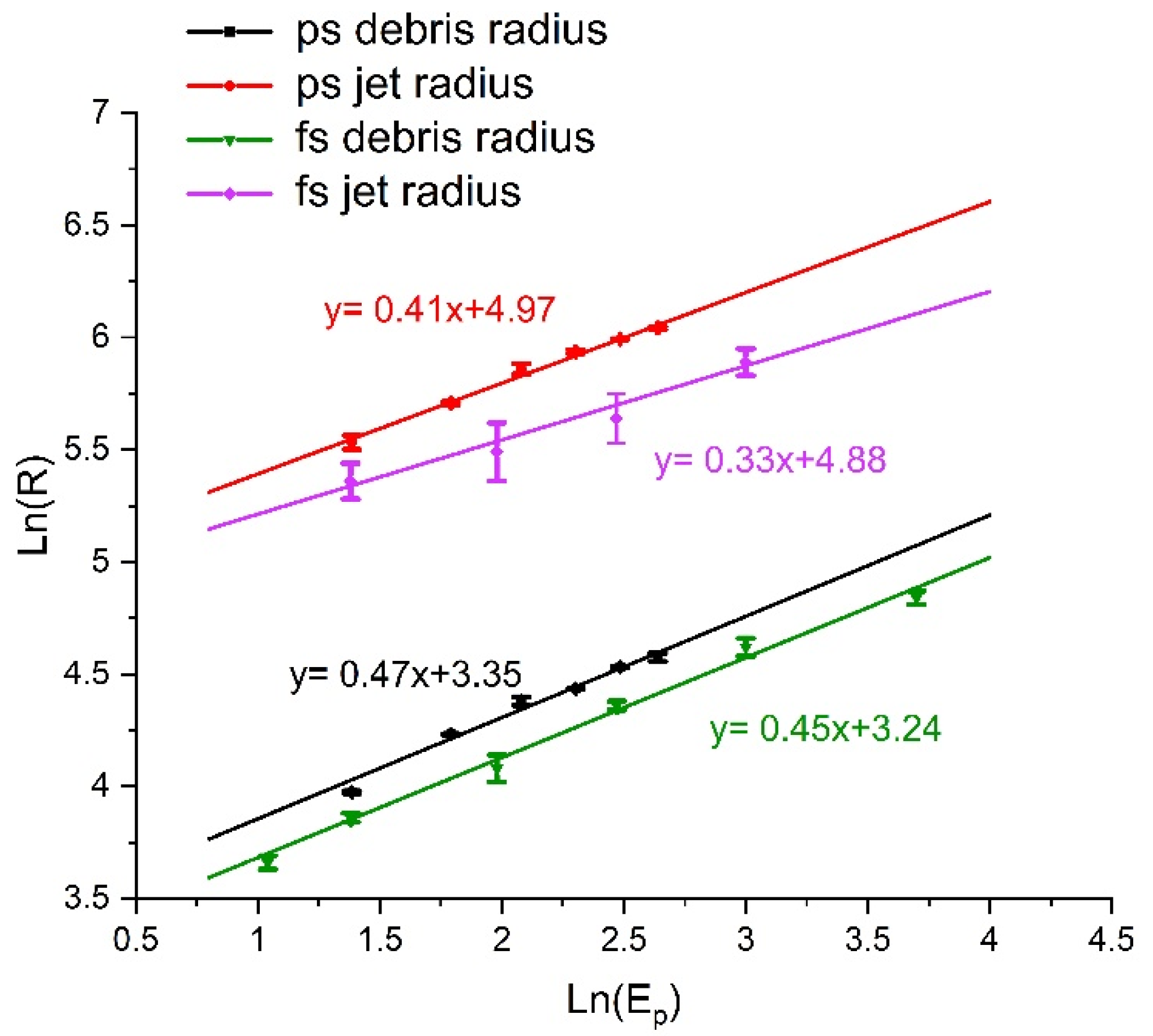
3.2. Ablation Rates and Debris Radii (10 ps Pulses)
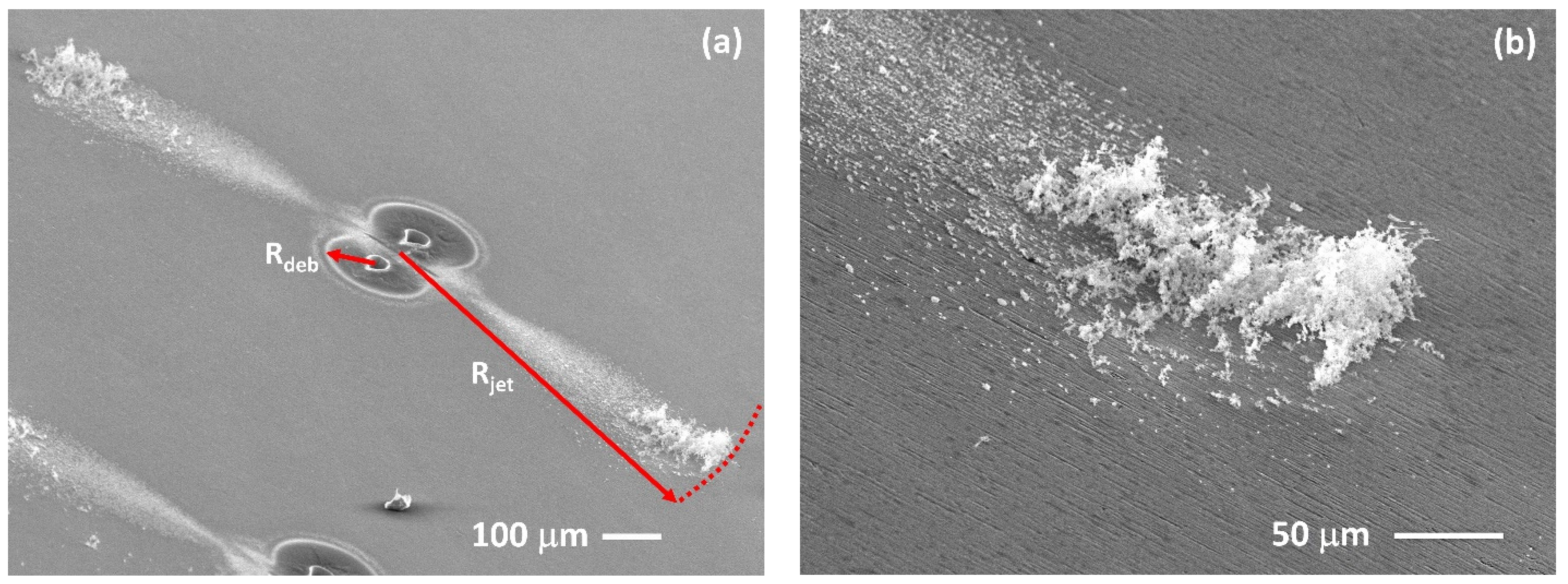
3.3. SEM Imaging of the Debris Fields, Two Spot Patterns
3.4. Time-Resolved Plasma Emission Spectra
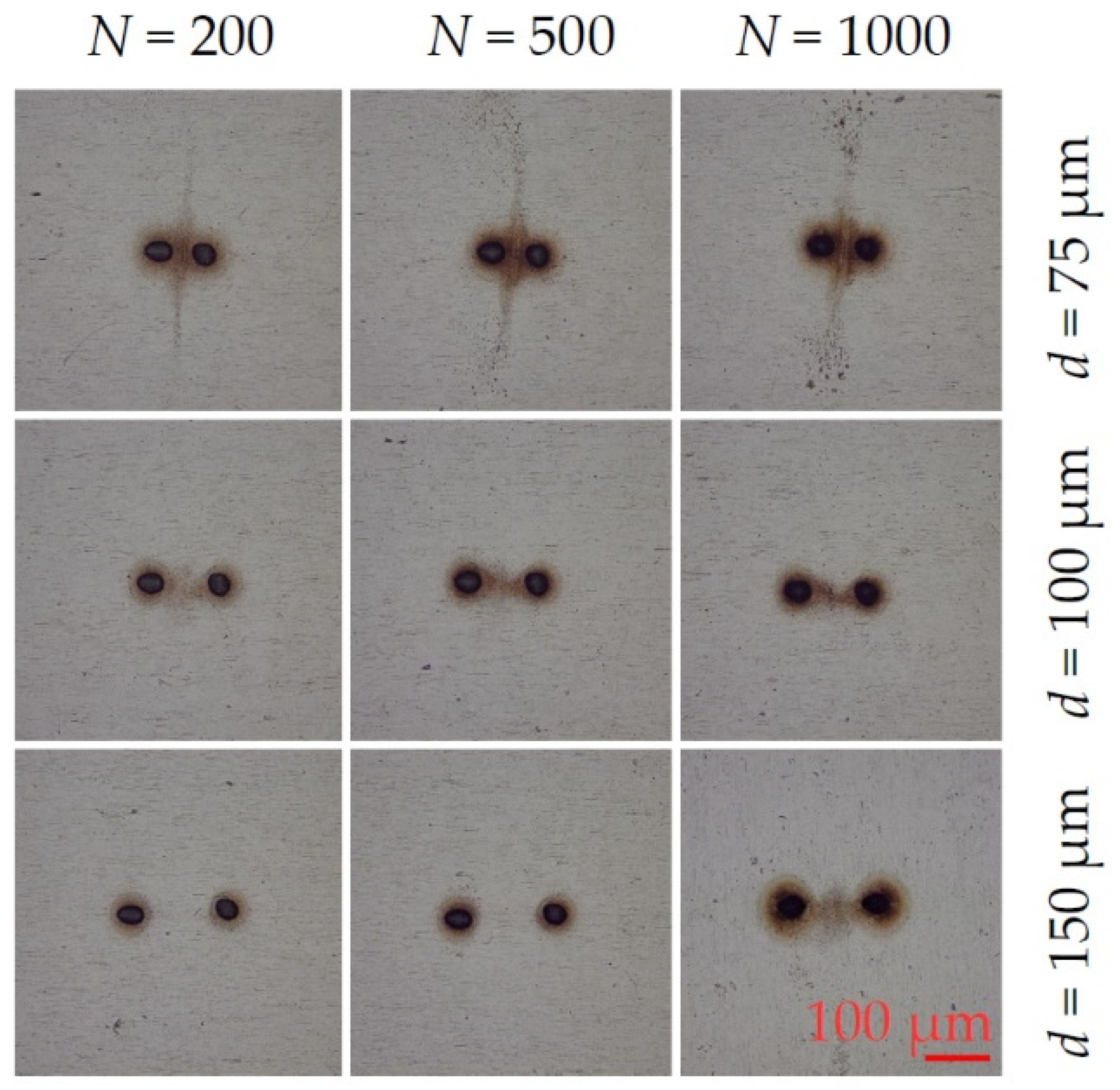
3.5. Two Spot Ablation with 600 fs/5 kHz Temporal Pulses at 1030 nm
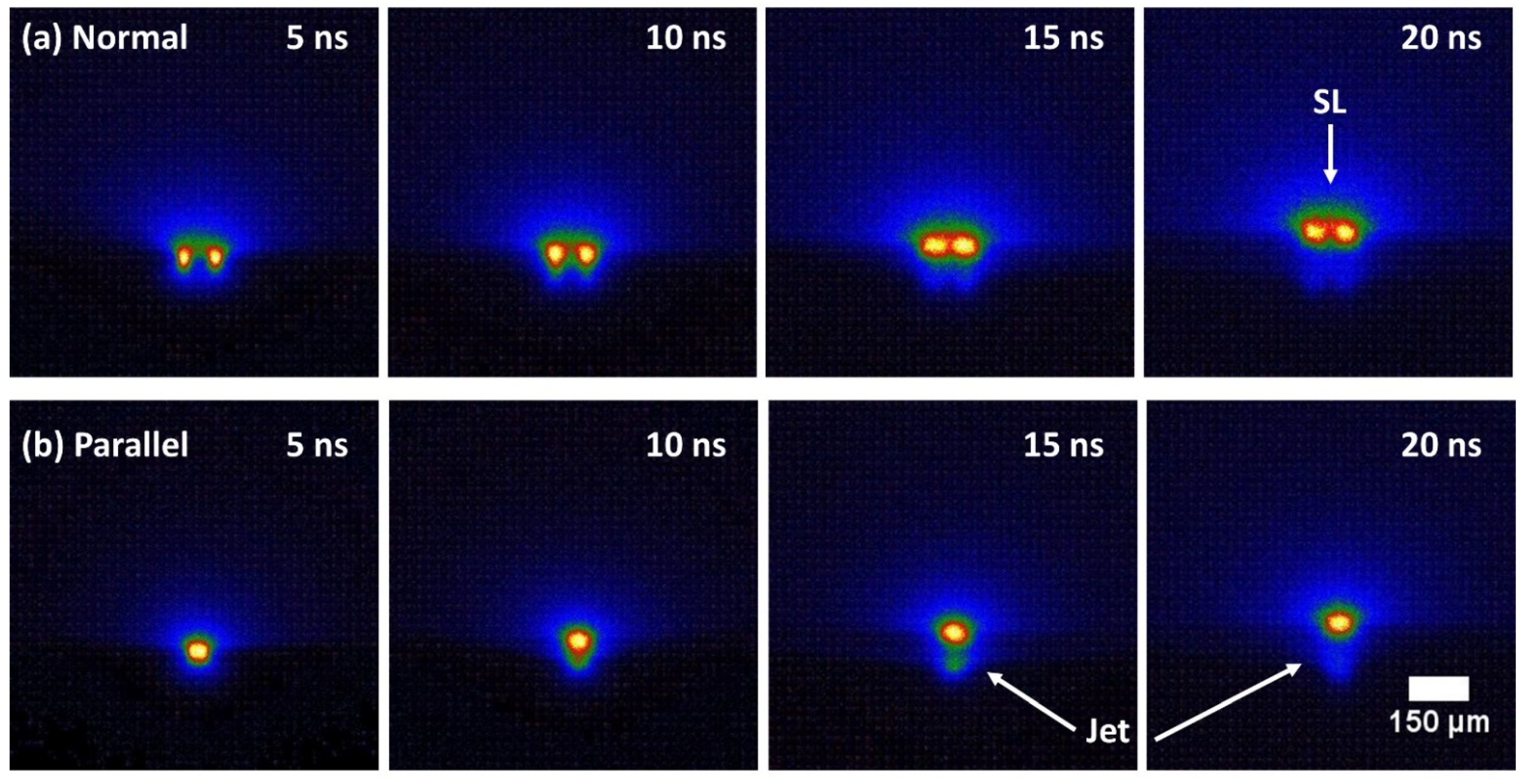
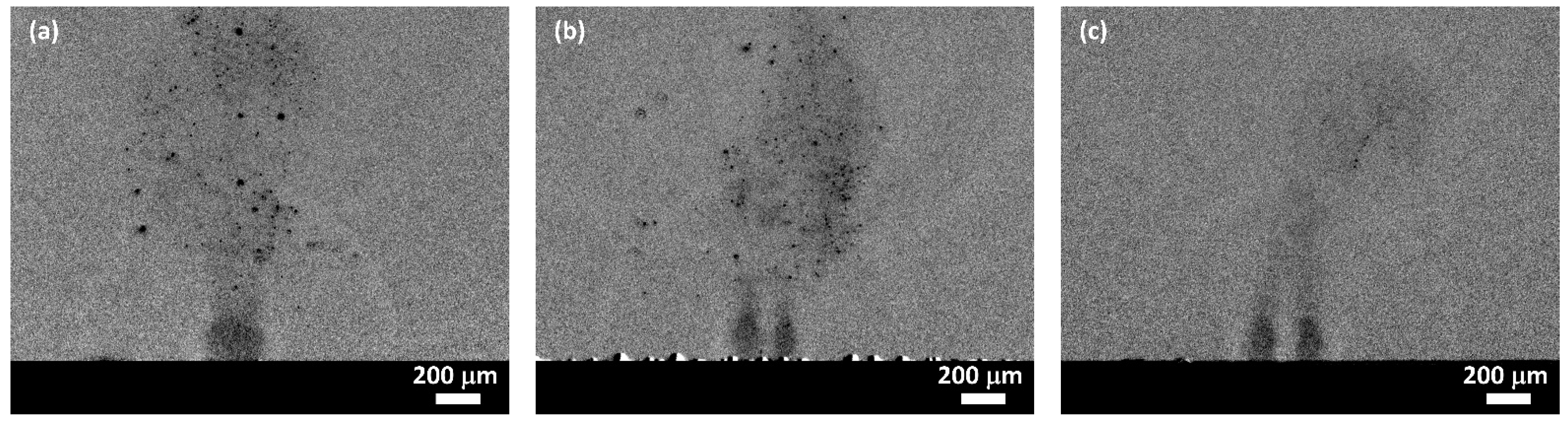
3.6. Time-Resolved Shadowgraphy (600 fs/5 kHz).
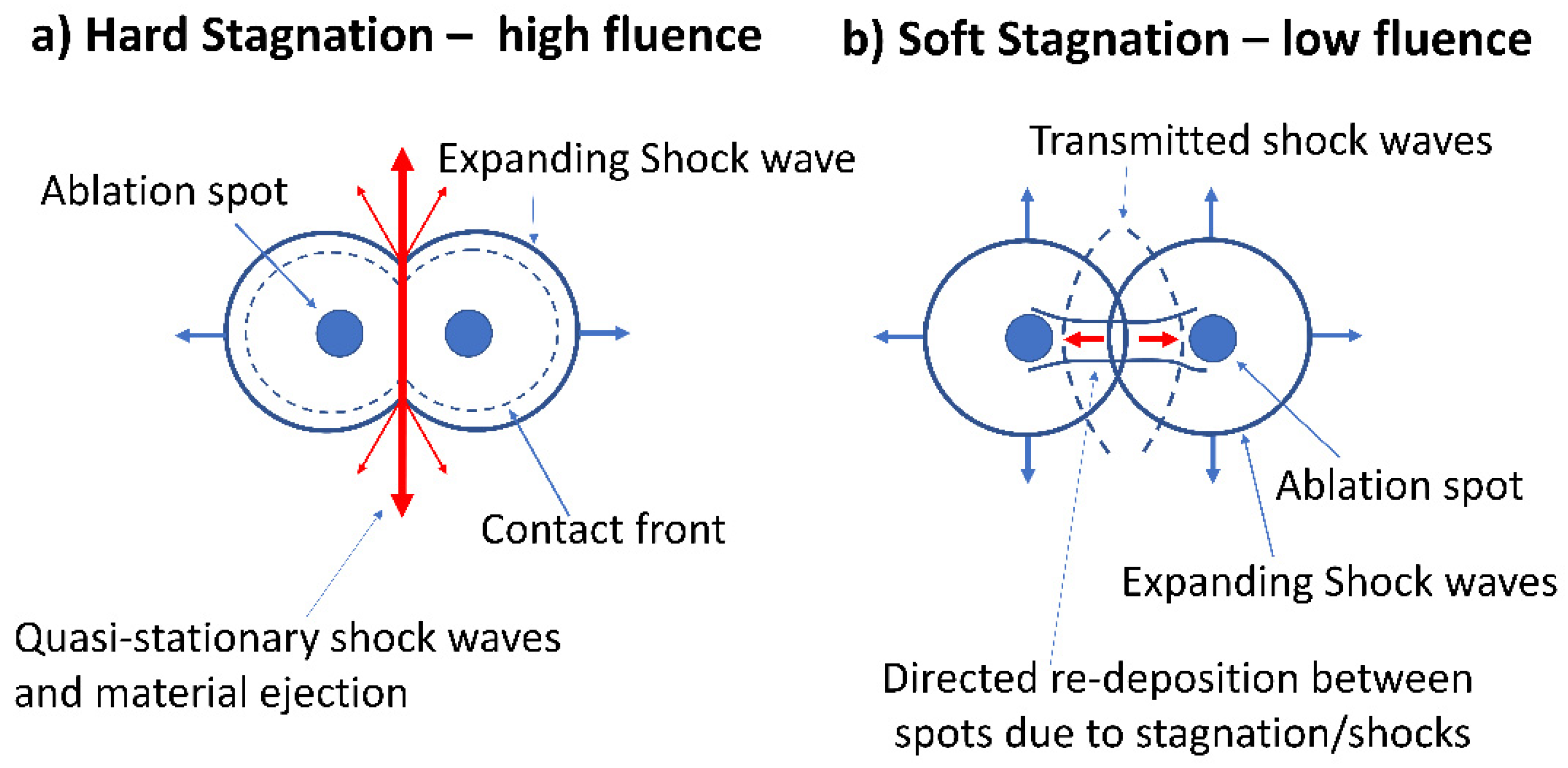
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Krausz, F.; Stockman, M.I. Attosecond metrology: From electron capture to future signal processing. Nat. Photonics 2014, 8, 205–213. [Google Scholar] [CrossRef]
- Austin, D.R.; Johnson, A.S.; McGrath, F.; Wood, D.; Miseikis, L.; Siegel, T.; Hawkins, P.; Harvey, A.; Mašín, Z.; Patchkovskii, S. Extracting sub-cycle electronic and nuclear dynamics from high harmonic spectra. Sci. Rep. 2021, 11, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Wille, H.; Rodríguez, M.; Kasparian, J.; Mondelain, D.; Yu, J.; Mysyrowicz, A.; Sauerbrey, R.; Wolf, J.-P.; Woeste, L. Teramobile: A mobile femtosecond-terawatt laser and detection system. EPJ Appl. Phys. 2002, 20, 183–190. [Google Scholar] [CrossRef]
- Tochitsky, S.; Welch, E.; Panagiotopoulos, P.; Polyanskiy, M.; Pogorelsky, I.; Kolesik, M.; Koch, S.W.; Moloney, J.V.; Joshi, C. Filamentation of long-wave infrared pulses in the atmosphere. JOSA B 2019, 36, G40–G51. [Google Scholar] [CrossRef]
- So, P.T.; Dong, C.Y.; Masters, B.R.; Berland, K.M. Two-photon excitation fluorescence microscopy. Annu. Rev. Biomed. Eng. 2000, 2, 399–429. [Google Scholar] [CrossRef] [Green Version]
- Varnavski, O.; Goodson, T., III. Two-photon fluorescence microscopy at extremely low excitation intensity: The power of quantum correlations. J. Am. Chem. Soc. 2020, 142, 12966–12975. [Google Scholar] [CrossRef]
- Abouzeid, H.; Ferrini, W. Femtosecond-laser assisted cataract surgery: A review. Acta Ophthalmol. 2014, 92, 597–603. [Google Scholar] [CrossRef]
- Russo, R.E.; Mao, X.; Gonzalez, J.J.; Mao, S.S. Femtosecond laser ablation ICP-MS. J. Anal. At. Spectrom. 2002, 17, 1072–1075. [Google Scholar] [CrossRef]
- Diwakar, P.K.; Harilal, S.S.; LaHaye, N.L.; Hassanein, A.; Kulkarni, P. The influence of laser pulse duration and energy on ICP-MS signal intensity, elemental fractionation, and particle size distribution in NIR fs-LA-ICP-MS. J. Anal. At. Spectrom. 2013, 28, 1420–1429. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Pozebon, D.; Scheffler, G.; Dressler, V. Recent applications of laser ablation inductively coupled plasma mass spectrometry (LA-ICP-MS) for biological sample analysis: A follow-up review. J. Anal. At. Spectrom. 2017, 32, 890–919. [Google Scholar] [CrossRef]
- Le Drogoff, B.; Margot, J.; Chaker, M.; Sabsabi, M.; Barthelemy, O.; Johnston, T.; Laville, S.; Vidal, F.; Von Kaenel, Y. Temporal characterization of femtosecond laser pulses induced plasma for spectrochemical analysis of aluminum alloys. Spectrochim. Acta B 2001, 56, 987–1002. [Google Scholar] [CrossRef]
- Zhu, Y.; Zhou, P.-W.; Zhong, S.-C.; Peng, Q.-X.; Zhu, L.-G. A multi-spot laser induced breakdown spectroscopy system based on diffraction beam splitter. Rev. Sci. Instrum. 2019, 90, 123105. [Google Scholar] [CrossRef] [PubMed]
- Ihlemann, J.; Wolff, B.; Simon, P. Nanosecond and femtosecond excimer laser ablation of fused silica. Appl. Phys. A 1992, 54, 363–368. [Google Scholar] [CrossRef]
- Allahyari, E.; Nivas, J.J.; Avallone, G.; Valadan, M.; Singh, M.; Granata, V.; Cirillo, C.; Vecchione, A.; Bruzzese, R.; Altucci, C. Femtosecond laser surface irradiation of silicon in air: Pulse repetition rate influence on crater features and surface texture. Opt. Laser Technol. 2020, 126, 106073. [Google Scholar] [CrossRef]
- Rajab, F.H.; Liauw, C.M.; Benson, P.S.; Li, L.; Whitehead, K.A. Picosecond laser treatment production of hierarchical structured stainless steel to reduce bacterial fouling. Food Bioprod. Process. 2018, 109, 29–40. [Google Scholar] [CrossRef]
- Xu, S.; Ding, R.; Yao, C.; Liu, H.; Wan, Y.; Wang, J.; Ye, Y.; Yuan, X. Effects of pulse durations and environments on femtosecond laser ablation of stainless steel. Appl. Phys. A 2018, 124, 1–8. [Google Scholar] [CrossRef]
- Hauschwitz, P.; Jochcová, D.; Jagdheesh, R.; Cimrman, M.; Brajer, J.; Rostohar, D.; Mocek, T.; Kopeček, J.; Lucianetti, A.; Smrž, M. Large-beam picosecond interference patterning of metallic substrates. Materials 2020, 13, 4676. [Google Scholar] [CrossRef] [PubMed]
- Kautek, W.; Krüger, J. Femtosecond Pulse Laser Ablation of Metallic, Semiconducting, Ceramic, and Biological Materials. In Laser Materials Processing: Industrial and Microelectronics Applications; Springer: Berlin/Heidelberg, Germany, 1994; pp. 600–611. [Google Scholar]
- Schille, J.; Schneider, L.; Kraft, S.; Hartwig, L.; Loeschner, U. Experimental study on double-pulse laser ablation of steel upon multiple parallel-polarized ultrashort-pulse irradiations. Appl. Phys. A 2016, 122, 1–11. [Google Scholar] [CrossRef]
- Dyer, P.; Sidhu, J. Spectroscopic and fast photographic studies of excimer laser polymer ablation. Int. J. Appl. Phys. 1988, 64, 4657–4663. [Google Scholar] [CrossRef]
- Yalçın, Ş.; Tsui, Y.Y.; Fedosejevs, R. Pressure dependence of emission intensity in femtosecond laser-induced breakdown spectroscopy. J. Anal. At. Spectrom. 2004, 19, 1295–1301. [Google Scholar] [CrossRef]
- Yalçin, S.; Tsui, Y.Y.; Fedosejevs, R. Images of femtosecond laser plasma plume expansion into background air. IEEE Trans. Plasma Sci. 2005, 33, 482–483. [Google Scholar] [CrossRef]
- Farid, N.; Harilal, S.; Ding, H.; Hassanein, A. Dynamics of ultrafast laser plasma expansion in the presence of an ambient. Appl. Phys. Lett. 2013, 103, 191112. [Google Scholar] [CrossRef]
- Lichtman, D.; Ready, J. Laser beam induced electron emission. Phys. Rev. Lett. 1963, 10, 342. [Google Scholar] [CrossRef]
- Bechtel, J.; Smith, W.L.; Bloembergen, N. Two-photon photoemission from metals induced by picosecond laser pulses. Phys. Rev. B 1977, 15, 4557. [Google Scholar] [CrossRef]
- Mao, S.S.; Mao, X.-L.; Greif, R.; Russo, R.E. Simulation of infrared picosecond laser-induced electron emission from semiconductors. Appl. Surf. Sci. 1998, 127, 206–211. [Google Scholar] [CrossRef]
- Russo, R.; Mao, X.; Liu, H.; Yoo, J.; Mao, S. Time-resolved plasma diagnostics and mass removal during single-pulse laser ablation. Appl. Phys. A 1999, 69, S887–S894. [Google Scholar] [CrossRef]
- Mao, S.S.; Mao, X.; Greif, R.; Russo, R.E. Initiation of an early-stage plasma during picosecond laser ablation of solids. Appl. Phys. Lett. 2000, 77, 2464–2466. [Google Scholar] [CrossRef]
- Mao, S.S.; Mao, X.; Greif, R.; Russo, R.E. Simulation of a picosecond laser ablation plasma. Appl. Phys. Lett. 2000, 76, 3370–3372. [Google Scholar] [CrossRef]
- Zeng, X.; Mao, X.; Greif, R.; Russo, R. Experimental investigation of ablation efficiency and plasma expansion during femtosecond and nanosecond laser ablation of silicon. Appl. Phys. A 2005, 80, 237–241. [Google Scholar] [CrossRef]
- Nica, P.; Gurlui, S.; Osiac, M.; Agop, M.; Ziskind, M.; Focsa, C. Investigation of femtosecond laser-produced plasma from various metallic targets using the Langmuir probe characteristic. Phys. Plasmas 2017, 24, 103119. [Google Scholar] [CrossRef]
- Wen, S.-B.; Mao, X.; Greif, R.; Russo, R.E. Expansion of the laser ablation vapor plume into a background gas. I. analysis. Int. J. Appl. Phys. 2007, 101, 023114. [Google Scholar] [CrossRef]
- Wu, J.; Wei, W.; Li, X.; Jia, S.; Qiu, A. Infrared nanosecond laser-metal ablation in atmosphere: Initial plasma during laser pulse and further expansion. Appl. Phys. Lett. 2013, 102, 164104. [Google Scholar] [CrossRef]
- Harilal, S.; Farid, N.; Freeman, J.; Diwakar, P.; LaHaye, N.; Hassanein, A. Background gas collisional effects on expanding fs and ns laser ablation plumes. Appl. Phys. A 2014, 117, 319–326. [Google Scholar] [CrossRef]
- Pereira, A.; Delaporte, P.; Sentis, M.; Marine, W.; Thomann, A.L.; Boulmer-Leborgne, C. Optical and morphological investigation of backward-deposited layer induced by laser ablation of steel in ambient air. Int. J. Appl. Phys. 2005, 98, 064902. [Google Scholar] [CrossRef]
- Harilal, S.S.; Brumfield, B.E.; Cannon, B.D.; Phillips, M.C. Shock wave mediated plume chemistry for molecular formation in laser ablation plasmas. Anal. Chem. 2016, 88, 2296–2302. [Google Scholar] [CrossRef]
- Bulgakova, N.M.; Panchenko, A.N.; Zhukov, V.P.; Kudryashov, S.I.; Pereira, A.; Marine, W.; Mocek, T.; Bulgakov, A.V. Impacts of ambient and ablation plasmas on short-and ultrashort-pulse laser processing of surfaces. Micromachines 2014, 5, 1344–1372. [Google Scholar] [CrossRef] [Green Version]
- Taylor, R.; Leopold, K.; Singleton, D.; Paraskevopoulos, G.; Irwin, R. The effect of debris formation on the morphology of excimer laser ablated polymers. Int. J. Appl. Phys. 1988, 64, 2815–2818. [Google Scholar] [CrossRef]
- Miotello, A.; Kelly, R.; Braren, B.; Otis, C.E. Novel geometrical effects observed in debris when polymers are laser sputtered. Appl. Phys. Lett. 1992, 61, 2784–2786. [Google Scholar] [CrossRef]
- Küper, S.; Brannon, J. Ambient gas effects on debris formed during KrF laser ablation of polyimide. Appl. Phys. Lett. 1992, 60, 1633–1635. [Google Scholar] [CrossRef]
- Burghardt, B.; Scheede, S.; Senczuk, R.; Kahlert, H.-J. Ablation plume effects on high precision excimer laser-based micromachining. Appl. Phys. A 1999, 69, S137–S140. [Google Scholar] [CrossRef]
- Garrelie, F.; Champeaux, C.; Catherinot, A. Study by a Monte Carlo simulation of the influence of a background gas on the expansion dynamics of a laser-induced plasma plume. Appl. Phys. A 1999, 69, 45–50. [Google Scholar] [CrossRef]
- Ravi-Kumar, S.; Lies, B.; Zhang, X.; Lyu, H.; Qin, H. Laser ablation of polymers: A review. Polym. Int. 2019, 68, 1391–1401. [Google Scholar] [CrossRef]
- Jacob, J.; Shanmugavelu, P.; Balasubramaniam, R. Investigation of the performance of 248 nm excimer laser assisted photoresist removal process in gaseous media by response surface methodology and artificial neural network. J. Manuf. Process. 2019, 38, 516–529. [Google Scholar] [CrossRef]
- Elaboudi, I.; Lazare, S.; Belin, C.; Talaga, D.; Labrugère, C. Underwater excimer laser ablation of polymers. Appl. Phys. A 2008, 92, 743–748. [Google Scholar] [CrossRef]
- Péreira, A.; Cros, A.; Delaporte, P.; Itina, T.; Sentis, M.; Marine, W.; Thomann, A.; Boulmer-Leborgne, C. Formation of Iron Oxide Nanoparticles by Pulsed Laser Ablation. In Proceedings of Atomic and Molecular Pulsed Lasers VI; SPIE: Bellingham, WA, USA, 2006; p. 62630Q. [Google Scholar]
- Pereira, A.; Delaporte, P.; Sentis, M.; Cros, A.; Marine, W.; Basillais, A.; Thomann, A.; Leborgne, C.; Semmar, N.; Andreazza, P. Laser treatment of a steel surface in ambient air. Thin Solid Films 2004, 453, 16–21. [Google Scholar] [CrossRef]
- Förster, D.J.; Faas, S.; Gröninger, S.; Bauer, F.; Michalowski, A.; Weber, R.; Graf, T. Shielding effects and re-deposition of material during processing of metals with bursts of ultra-short laser pulses. Appl. Surf. Sci. 2018, 440, 926–931. [Google Scholar] [CrossRef]
- Kraft, S.; Schille, J.; Mauersberger, S.; Schneider, L.; Loeschner, U. Time-resolved visualization of high-repetition ultrashort pulse laser ablation. Appl. Opt. 2020, 59, 452–458. [Google Scholar] [CrossRef]
- Kraft, S.; Schille, J.; Mauersberger, S.; Schneider, L.; Loeschner, U. Pump-Probe Imaging for Process Control and Optimization in High-Speed Laser Micro Machining. In Laser-Based Micro-and Nanoprocessing XIV; SPIE: Bellingham, WA, USA, 2020; p. 112681H. [Google Scholar]
- Vreugdenhil, M.; Van Oosten, D.; Hernandez-Rueda, J. Dynamics of femtosecond laser-induced shockwaves at a water/air interface using multiple excitation beams. Opt. Lett. 2018, 43, 4899–4902. [Google Scholar] [CrossRef]
- Baasanjav, D.; Hernandez-Rueda, J.; Mosk, A.P.; Van Oosten, D. Optical method for micrometer-scale tracerless visualization of ultrafast laser induced gas flow at a water/air interface. Appl. Opt. 2020, 59, 5205–5209. [Google Scholar] [CrossRef]
- Gupta, S.L.; Thareja, R.K. ZnO thin film deposition using colliding plasma plumes and single plasma plume: Structural and optical properties. Int. J. Appl. Phys. 2013, 114, 224903. [Google Scholar] [CrossRef]
- Pandey, P.K.; Thareja, R.K.; Singh, R.P.; Costello, J.T. Deposition of nanocomposite Cu–TiO2 using heterogeneous colliding plasmas. Appl. Phys. B 2018, 124, 1–8. [Google Scholar] [CrossRef]
- Donnelly, T.; O’Connell, G.; Lunney, J.G. Metal nanoparticle film deposition by femtosecond laser ablation at atmospheric pressure. Nanomaterials 2020, 10, 2118. [Google Scholar] [CrossRef]
- Serra, P.; Piqué, A. Laser-induced forward transfer: Fundamentals and applications. Adv. Mater. Technol. 2019, 4, 1800099. [Google Scholar] [CrossRef] [Green Version]
- Liu, J. Simple technique for measurements of pulsed Gaussian-beam spot sizes. Opt. Lett. 1982, 7, 196–198. [Google Scholar] [CrossRef]
- Zhao, W.; Wang, W.; Jiang, G.; Li, B.Q.; Mei, X. Ablation and morphological evolution of micro-holes in stainless steel with picosecond laser pulses. Int. J. Adv. Manuf. Technol. 2015, 80, 1713–1720. [Google Scholar] [CrossRef]
- Taylor, G.I. The formation of a blast wave by a very intense explosion I. Theoretical discussion. Proc. R. Soc. Lond. 1950, 201, 159–174. [Google Scholar]
- Singh, S.; Argument, M.; Tsui, Y.; Fedosejevs, R. Effect of ambient air pressure on debris redeposition during laser ablation of glass. Int. J. Appl. Phys. 2005, 98, 113520. [Google Scholar] [CrossRef]
- Liu, H.; Mao, X.; Yoo, J.; Russo, R. Early phase laser induced plasma diagnostics and mass removal during single-pulse laser ablation of silicon. Spectrochim. Acta B 1999, 54, 1607–1624. [Google Scholar] [CrossRef]
- Kramida, A.; Ralchenko, Y.; Reader, J. NIST Atomic Spectra Database (ver. 5.3); NIST: Gaithersburg, MA, USA, 2015. [Google Scholar]
- Harilal, S.; Bindhu, C.; Tillack, M.; Najmabadi, F.; Gaeris, A. Internal structure and expansion dynamics of laser ablation plumes into ambient gases. Int. J. Appl. Phys. 2003, 93, 2380–2388. [Google Scholar] [CrossRef] [Green Version]
- Cargill, P.; Goodrich, C.; Papadopoulos, K. Interaction of two collisionless shocks. Phys. Rev. Lett. 1986, 56, 1988. [Google Scholar] [CrossRef] [PubMed]
- Fallon, C.; Hayden, P.; Walsh, N.; Kennedy, E.; Costello, J. The effect of wedge angle on the evolution of a stagnation layer in a colliding plasma experiment. J. Phys. Conf. Ser. 2014, 548, 12036. [Google Scholar] [CrossRef] [Green Version]
- Fallon, C.; Hayden, P.; Walsh, N.; Kennedy, E.; Costello, J. Target geometrical effects on the stagnation layer formed by colliding a pair of laser produced copper plasmas. Phys. Plasmas 2015, 22, 93506. [Google Scholar] [CrossRef]
- Gibbon, P. Introduction to plasma physics. arXiv 2020, arXiv:2007.04783. [Google Scholar]
- Yang, Z.; Wei, W.; Han, J.; Wu, J.; Li, X.; Jia, S. Experimental study of the behavior of two laser produced plasmas in air. Phys. Plasmas 2015, 22, 073511. [Google Scholar] [CrossRef]
- Rambo, P.; Denavit, J. Interpenetration and ion separation in colliding plasmas. Phys. Plasmas 1994, 1, 4050–4060. [Google Scholar] [CrossRef]
- Guthikonda, N.; Manikanta, E.; Chelikani, L.; Sai Shiva, S.; Sree Harsha, S.; Ikkurthi, V.; Prem Kiran, P. Interaction of two counterpropagating laser induced plasmas and shock waves in air. Phys. Plasmas 2020, 27, 23107. [Google Scholar] [CrossRef]
- Jin, Y.; Perrie, W.; Harris, P.; Allegre, O.; Abrams, K.; Dearden, G. Patterning of Aluminium thin film on polyethylene terephthalate by multi-beam picosecond laser. Opt. Lasers Eng. Opt. Laser Eng. 2015, 74, 67–74. [Google Scholar] [CrossRef]
- Tang, Y.; Perrie, W.; Rico Sierra, D.; Li, Q.; Liu, D.; Edwardson, S.P.; Dearden, G. Laser–material interactions of high-quality ultrashort pulsed vector vortex beams. Micromachines 2021, 12, 376. [Google Scholar] [CrossRef]
- Taylor, J. Relaxation and magnetic reconnection in plasmas. Rev. Mod. Phys. 1986, 58, 741. [Google Scholar] [CrossRef]
- Totorica, S.; Abel, T.; Fiuza, F. Particle acceleration in laser-driven magnetic reconnection. Phys. Plasmas 2017, 24, 41408. [Google Scholar] [CrossRef] [Green Version]
- Nilson, P.; Willingale, L.; Kaluza, M.; Kamperidis, C.; Minardi, S.; Wei, M.; Fernandes, P.; Notley, M.; Bandyopadhyay, S.; Sherlock, M. Bidirectional jet formation during driven magnetic reconnection in two-beam laser–plasma interactions. Phys. Plasmas 2008, 15, 92701. [Google Scholar] [CrossRef]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, T.; Kraft, S.; Perrie, W.; Schille, J.; Löschner, U.; Edwardson, S.; Dearden, G. Backward Flux Re-Deposition Patterns during Multi-Spot Laser Ablation of Stainless Steel with Picosecond and Femtosecond Pulses in Air. Materials 2021, 14, 2243. https://doi.org/10.3390/ma14092243
Zhou T, Kraft S, Perrie W, Schille J, Löschner U, Edwardson S, Dearden G. Backward Flux Re-Deposition Patterns during Multi-Spot Laser Ablation of Stainless Steel with Picosecond and Femtosecond Pulses in Air. Materials. 2021; 14(9):2243. https://doi.org/10.3390/ma14092243
Chicago/Turabian StyleZhou, Tong, Sebastian Kraft, Walter Perrie, Jörg Schille, Udo Löschner, Stuart Edwardson, and Geoff Dearden. 2021. "Backward Flux Re-Deposition Patterns during Multi-Spot Laser Ablation of Stainless Steel with Picosecond and Femtosecond Pulses in Air" Materials 14, no. 9: 2243. https://doi.org/10.3390/ma14092243
APA StyleZhou, T., Kraft, S., Perrie, W., Schille, J., Löschner, U., Edwardson, S., & Dearden, G. (2021). Backward Flux Re-Deposition Patterns during Multi-Spot Laser Ablation of Stainless Steel with Picosecond and Femtosecond Pulses in Air. Materials, 14(9), 2243. https://doi.org/10.3390/ma14092243







