Photocatalytic Degradation of 4,4′-Isopropylidenebis(2,6-dibromophenol) on Sulfur-Doped Nano TiO2
Abstract
:1. Introduction
2. Materials and Methods
2.1. Chemicals
2.2. Photocatalysts Characterization
2.3. Photocatalytic Degradation of Aromatic Compounds
2.4. Computational Methods
3. Results and Discussion
3.1. Photocatalysts Characterization
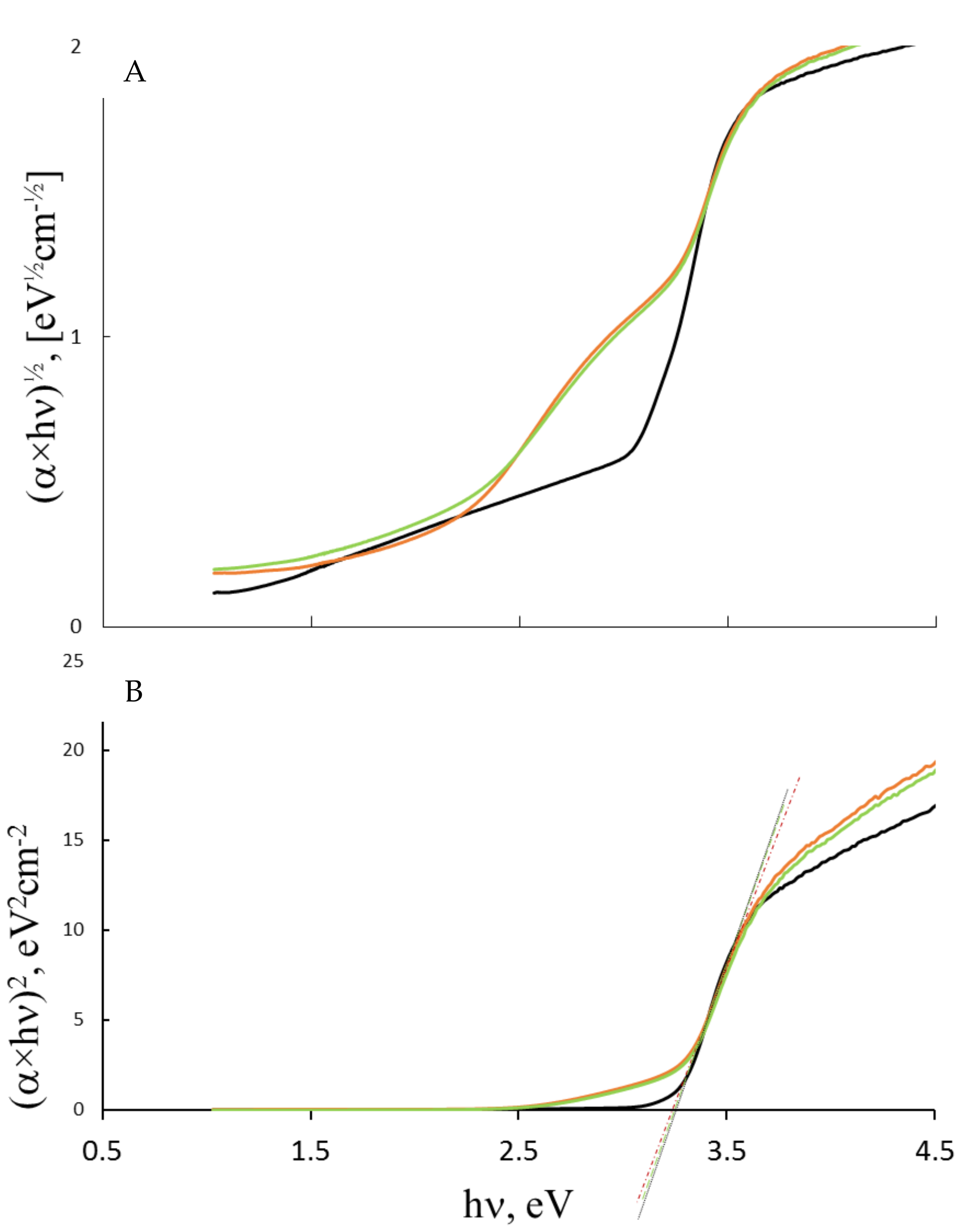
3.2. Optical Energy Evaluations
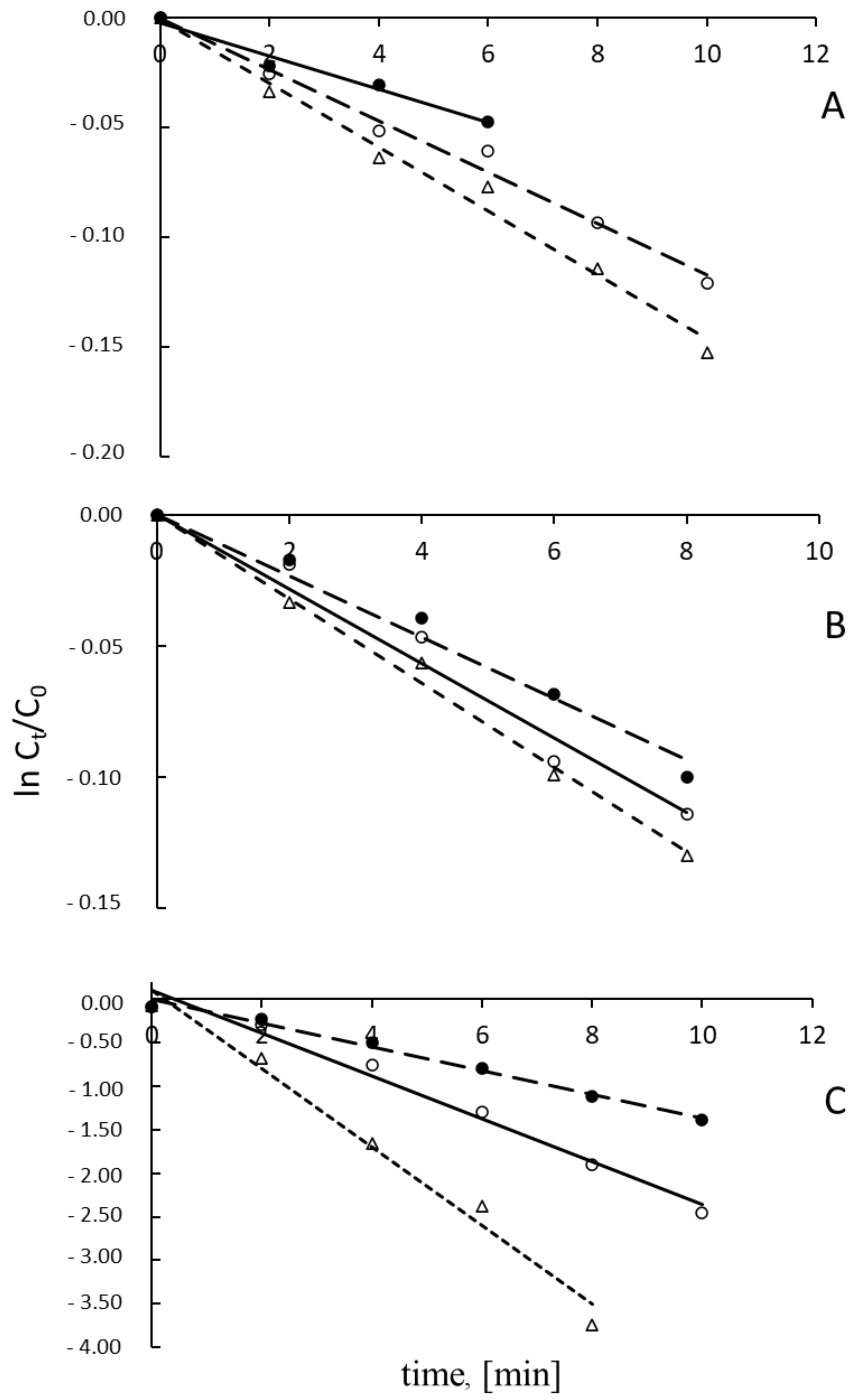
3.3. Photocatalytic Activity
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Etacheri, V.; Di Valentin, C.; Schneider, J.; Bahnemann, D.B.D.; Pillai, S.C. Visible-light activation of TiO2 photocatalysts: Advances in theory and experiments. J. Photochem. Photobiol. C Photochem. Rev. 2015, 25, 1–29. [Google Scholar] [CrossRef] [Green Version]
- Asahi, R.; Morikawa, T.; Ohwaki, T.; Aoki, K.; Taga, Y. Visible-Light Photocatalysis in Nitrogen-Doped Titanium Oxides. Science 2001, 293, 269–271. [Google Scholar] [CrossRef]
- Pelaez, M.; de la Cruz, A.A.; Stathatos, E.; Falaras, P.; Dionysiou, D.D. Visible light-activated N-F-codoped TiO2 nanoparticles for the photocatalytic degradation of microcystin-LR in water. Catal. Today 2009, 144, 19–25. [Google Scholar] [CrossRef]
- Pelaez, M.; Falaras, P.; Likodimos, V.; Kontos, A.G.; De La Cruz, A.A.; O’Shea, K.; Dionysiou, D.D. Synthesis, structural characterization and evaluation of sol–gel-based NF-TiO2 films with visible light-photoactivation for the removal of microcystin-LR. Appl. Catal. B Environ. 2010, 99, 378–387. [Google Scholar] [CrossRef]
- Kisch, H. Semiconductor Photocatalysis-Mechanistic and Synthetic Aspects. Angew. Chem. Int. Ed. 2012, 52, 812–847. [Google Scholar] [CrossRef]
- Khan, S.U.M.; Al-Shahry, M.; Ingler, W.B. Efficient Photochemical Water Splitting by a Chemically Modified n-TiO2. Science 2002, 297, 2243–2245. [Google Scholar] [CrossRef]
- Sakthivel, S.; Kisch, H. Daylight Photocatalysis by Carbon-Modified Titanium Dioxide. Angew. Chem. Int. Ed. 2003, 42, 4908–4911. [Google Scholar] [CrossRef]
- El-Sheikh, S.M.; Zhang, G.; El-Hosainy, H.M.; Ismail, A.A.; O’Shea, K.E.; Falaras, P.; Kontos, A.G.; Dionysiou, D.D. High performance sulfur, nitrogen and carbon doped mesoporous anatase–brookite TiO2 photocatalyst for the removal of microcystin-LR under visible light irradiation. J. Hazard. Mater. 2014, 280, 723–733. [Google Scholar] [CrossRef]
- Ohno, T.; Mitsui, T.; Matsumura, M. Photocatalytic Activity of S-doped TiO2Photocatalyst under Visible Light. Chem. Lett. 2003, 32, 364–365. [Google Scholar] [CrossRef] [Green Version]
- Ma, D.; Xin, Y.; Gao, M.; Wu, J. Fabrication and photocatalytic properties of cationic and anionic S-doped TiO2 nanofibers by electrospinning. Appl. Catal. B Environ. 2014, 147, 49–57. [Google Scholar] [CrossRef]
- Umebayashi, T.; Yamaki, T.; Itoh, H.; Asai, K. Band gap narrowing of titanium dioxide by sulfur doping. Appl. Phys. Lett. 2002, 81, 454–456. [Google Scholar] [CrossRef]
- Sun, H.; Bai, Y.; Cheng, Y.; Jin, W.; Xu, N. Preparation and Characterization of Visible-Light-Driven Carbon−Sulfur-Codoped TiO2 Photocatalysts. Ind. Eng. Chem. Res. 2006, 45, 4971–4976. [Google Scholar] [CrossRef]
- Ohno, T.; Akiyoshi, M.; Umebayashi, T.; Asai, K.; Mitsui, T.; Matsumura, M. Preparation of S-doped TiO2 photocatalysts and their photocatalytic activities under visible light. Appl. Catal. A Gen. 2004, 265, 115–121. [Google Scholar] [CrossRef]
- Rockafellow, E.M.; Stewart, L.K.; Jenks, W.S. Is sulfur-doped TiO2 an effective visible light photocatalyst for remediation? Appl. Catal. B Environ. 2009, 91, 554–562. [Google Scholar] [CrossRef]
- Han, C.; Pelaez, M.; Likodimos, V.; Kontos, A.G.; Falaras, P.; O’Shea, K.; Dionysiou, D.D. Innovative visible light-activated sulfur doped TiO2 films for water treatment. Appl. Catal. B Environ. 2011, 107, 77–87. [Google Scholar] [CrossRef]
- Livraghi, S.; Votta, A.; Paganini, M.C.; Giamello, E. The nature of paramagnetic species in nitrogen doped TiO2 active in visible light photocatalysis. Chem. Commun. 2004, 498–500. [Google Scholar] [CrossRef]
- Sano, T.; Mera, N.; Kanai, Y.; Nishimoto, C.; Tsutsui, S.; Hirakawa, T.; Negishi, N. Origin of visible-light activity of N-doped TiO2 photocatalyst: Behaviors of N and S atoms in a wet N-doping process. Appl. Catal. B Environ. 2012, 128, 77–83. [Google Scholar] [CrossRef]
- Yan, G.; Zhang, M.; Hou, J.; Yang, J. Photoelectrochemical and photocatalytic properties of N+S co-doped TiO2 nanotube array films under visible light irradiation. Mater. Chem. Phys. 2011, 129, 553–557. [Google Scholar] [CrossRef] [Green Version]
- Rengifo-Herrera, J.; Kiwi, J.; Pulgarin, C. N, S co-doped and N-doped Degussa P-25 powders with visible light response prepared by mechanical mixing of thiourea and urea. Reactivity towards E. coli inactivation and phenol oxidation. J. Photochem. Photobiol. A Chem. 2009, 205, 109–115. [Google Scholar] [CrossRef]
- Chen, X.; Burda, C. The Electronic Origin of the Visible-Light Absorption Properties of C-, N- and S-Doped TiO2 Nanomaterials. J. Am. Chem. Soc. 2008, 130, 5018–5019. [Google Scholar] [CrossRef]
- Ilisz, I.; Dombi, A. Investigation of the photodecomposition of phenol in near-UV-irradiated aqueous TiO2 suspensions. II. Effect of charge-trapping species on product distribution. Appl. Catal. A Gen. 1999, 180, 35–45. [Google Scholar] [CrossRef]
- Ivanov, S.; Barylyak, A.; Besaha, K.; Bund, A.; Bobitski, Y.; Wojnarowska-Nowak, R.; Yaremchuk, I.; Kus-Liśkiewicz, M. Synthesis, Characterization, and Photocatalytic Properties of Sulfur- and Carbon-Codoped TiO2 Nanoparticles. Nanoscale Res. Lett. 2016, 11, 1–12. [Google Scholar] [CrossRef] [Green Version]
- Booth, F. Theory of Electrokinetic Effects. Nature 1948, 161, 83–86. [Google Scholar] [CrossRef]
- Saad, J.G.; Sherman, M.N. Using Zeta Potential to Determine Equivalency of Generic and Non-Generic Oral Suspensions. 2018. Available online: https://www.particulatesystems.com/wp-content/uploads/2017/08/application-note-ps-029_v2.pdf (accessed on 14 November 2019).
- Scherrer, P. Bestimmung der inneren Struktur und der Größe von Kolloidteilchen mittels Röntgenstrahlen. In Kolloidchemie Ein Lehrbuch; Springer: Berlin/Heidelberg, Germany, 1912; pp. 387–409. [Google Scholar] [CrossRef]
- Langford, J.I.; Wilson, A.J.C. Scherrer after sixty years: A survey and some new results in the determination of crystallite size. J. Appl. Crystallogr. 1978, 11, 102–113. [Google Scholar] [CrossRef]
- Valiev, M.; Bylaska, E.; Govind, N.; Kowalski, K.; Straatsma, T.; van Dam, H.J.J.; Wang, D.; Nieplocha, J.; Aprà, E.; Windus, T.; et al. NWChem: A comprehensive and scalable open-source solution for large scale molecular simulations. Comput. Phys. Commun. 2010, 181, 1477–1489. [Google Scholar] [CrossRef] [Green Version]
- Feller, D. The Role of Databases in Support of Computational Chemistry Calculations. J. Comput. Chem. 1996, 17, 1571–1586. [Google Scholar] [CrossRef]
- Stumm, W. Chemistry of the Solid–Water Interface: Processes at the Mineral–Water and Particle–Water Interface in Natural Systems; John Wiley & Son Inc.: Hoboken, NJ, USA, 1992; ISBN 978-0-471-57672-3. [Google Scholar]
- Niu, Y.; Xing, M.; Tian, B.; Zhang, J. Improving the visible light photocatalytic activity of nano-sized titanium dioxide via the synergistic effects between sulfur doping and sulfation. Appl. Catal. B Environ. 2012, 115–116, 253–260. [Google Scholar] [CrossRef]
- Siva, S.; Sudharsan, S.; Kannan, R.S. Synthesis, characterization and ion-exchange properties of novel hybrid polymer nanocomposites for selective and effective mercury(ii) removal. RSC Adv. 2015, 5, 79665–79678. [Google Scholar] [CrossRef]
- Sraw, A.; Kaur, T.; Pandey, Y.; Verma, A.; Sobti, A.; Wanchoo, R.K.; Toor, A.P. Photocatalytic degradation of monocrotophos and quinalphos using solar-activated S-doped TiO2. Int. J. Environ. Sci. Technol. 2020, 17, 4895–4908. [Google Scholar] [CrossRef]
- Piątkowska, A.; Janus, M.; Szymański, K.; Mozia, S. C-,N- and S-Doped TiO2 Photocatalysts: A Review. Catalysts 2021, 11, 144. [Google Scholar] [CrossRef]
- Makuła, P.; Pacia, M.; Macyk, W. How to Correctly Determine the Band Gap Energy of Modified Semiconductor Photocatalysts Based on UV–Vis Spectra. J. Phys. Chem. Lett. 2018, 9, 6814–6817. [Google Scholar] [CrossRef] [Green Version]
- Urbach, F. The Long-Wavelength Edge of Photographic Sensitivity and of the Electronic Absorption of Solids. Phys. Rev. 1953, 92, 1324. [Google Scholar] [CrossRef]
- Khashan, M.; El-Naggar, A. A new method of finding the optical constants of a solid from the reflectance and transmittance spectrograms of its slab. Opt. Commun. 2000, 174, 445–453. [Google Scholar] [CrossRef]
- Linsebigler, A.L.; Lu, G.; Yates, J.T., Jr. Photocatalysis on TiO2 Surfaces: Principles, Mechanisms, and Selected Results. Chem. Rev. 1995, 95, 735–758. [Google Scholar] [CrossRef]
- Wardman, P. Reduction Potentials of One-Electron Couples Involving Free Radicals in Aqueous Solution. J. Phys. Chem. Ref. Data 1989, 18, 1637–1755. [Google Scholar] [CrossRef] [Green Version]
- Tanaka, S.; Nakata, Y.; Kimura, T.; Kawasaki, M.; Kuramitz, H. Electrochemical decomposition of bisphenol A using Pt/Ti and SnO2/Ti anodes. J. Appl. Electrochem. 2002, 32, 197–201. [Google Scholar] [CrossRef]
- Lide, D.R. CRC Handbook of Chemistry and Physics, Internet Version; CRC Press LLC: Boca Raton, FL, USA, 2005; Available online: http://www.hbcpnetbase.com (accessed on 8 October 2019).
- Kosky, P.G.; Silva, J.M.; Guggenheim, E.A. The aqueous phase in the interfacial synthesis of polycarbonates. Part 1. Ionic equilibria and experimental solubilities in the BPA-sodium hydroxide-water system. Ind. Eng. Chem. Res. 1991, 30, 462–467. [Google Scholar] [CrossRef]
- Risk Assessment Of 2,2′,6,6′-Tetrabromo-4,4′-Isopropylidene Diphenol (Tetrabromobisphenol-A), n.d. Available online: https://echa.europa.eu/documents/10162/17c7379e-f47b-4a76-aa43-060da5830c07 (accessed on 13 November 2019).
- Gutz, I.G.R. pH Calculation and Acid-Base Titration Curves—Freeware for Data Analysis and Simulation. Available online: http://www.iq.usp.br/gutz/Curtipot_.html (accessed on 6 April 2020).
- Bourikas, K.; Vakros, J.; Kordulis, C.; Lycourghiotis, A. Potentiometric Mass Titrations: Experimental and Theoretical Establishment of a New Technique for Determinig the Piont of Zero Charge (PZC) of Metal (Hydr)Oxides. J. Phys. Chem. B 2003, 107, 9441–9451. [Google Scholar] [CrossRef]
- Isse, A.A.; Mussini, P.R.; Gennaro, A. New Insights into Electrocatalysis and Dissociative Electron Transfer Mechanisms: The Case of Aromatic Bromides. J. Phys. Chem. C 2009, 113, 14983–14992. [Google Scholar] [CrossRef]
- Andrieux, C.P.; Blocman, C.; Dumas-Bouchiat, J.M.; Saveant, J.M. Heterogeneous and homogeneous electron transfers to aromatic halides. An electrochemical redox catalysis study in the halobenzene and halopyridine series. J. Am. Chem. Soc. 1979, 101, 3431–3441. [Google Scholar] [CrossRef]
- Horikoshi, S.; Miura, T.; Kajitani, M.; Horikoshi, N.; Serpone, N. Photodegradation of tetrahalobisphenol-A (X = Cl, Br) flame retardants and delineation of factors affecting the process. Appl. Catal. B Environ. 2008, 84, 797–802. [Google Scholar] [CrossRef] [Green Version]
- Sobczyński, A.; Duczmal, Ł.; Zmudziński, W. Phenol destruction by photocatalysis on TiO2: An attempt to solve the reaction mechanism. J. Mol. Catal. A Chem. 2004, 213, 225–230. [Google Scholar] [CrossRef]
- Ethiraj, A.S.; Uttam, P.; Varunkumar, K.; Chong, K.F.; Ali, G.A. Photocatalytic performance of a novel semiconductor nanocatalyst: Copper doped nickel oxide for phenol degradation. Mater. Chem. Phys. 2019, 242, 122520. [Google Scholar] [CrossRef]
- Morjène, L.; Aloulou, F.; Tasbihi, M.; Schwarze, M.; Schomäcker, R.; Seffen, M. New composite material based on Kaolinite, cement, TiO2 for efficient removal of phenol by photocatalysis. Environ. Sci. Pollut. Res. 2021, 28, 35991–36003. [Google Scholar] [CrossRef]
- Pei, S.; Shi, H.; Zhang, J.; Wang, S.; Ren, N.; You, S. Electrochemical removal of tetrabromobisphenol A by fluorine-doped titanium suboxide electrochemically reactive membrane. J. Hazard. Mater. 2021, 419, 126434. [Google Scholar] [CrossRef]
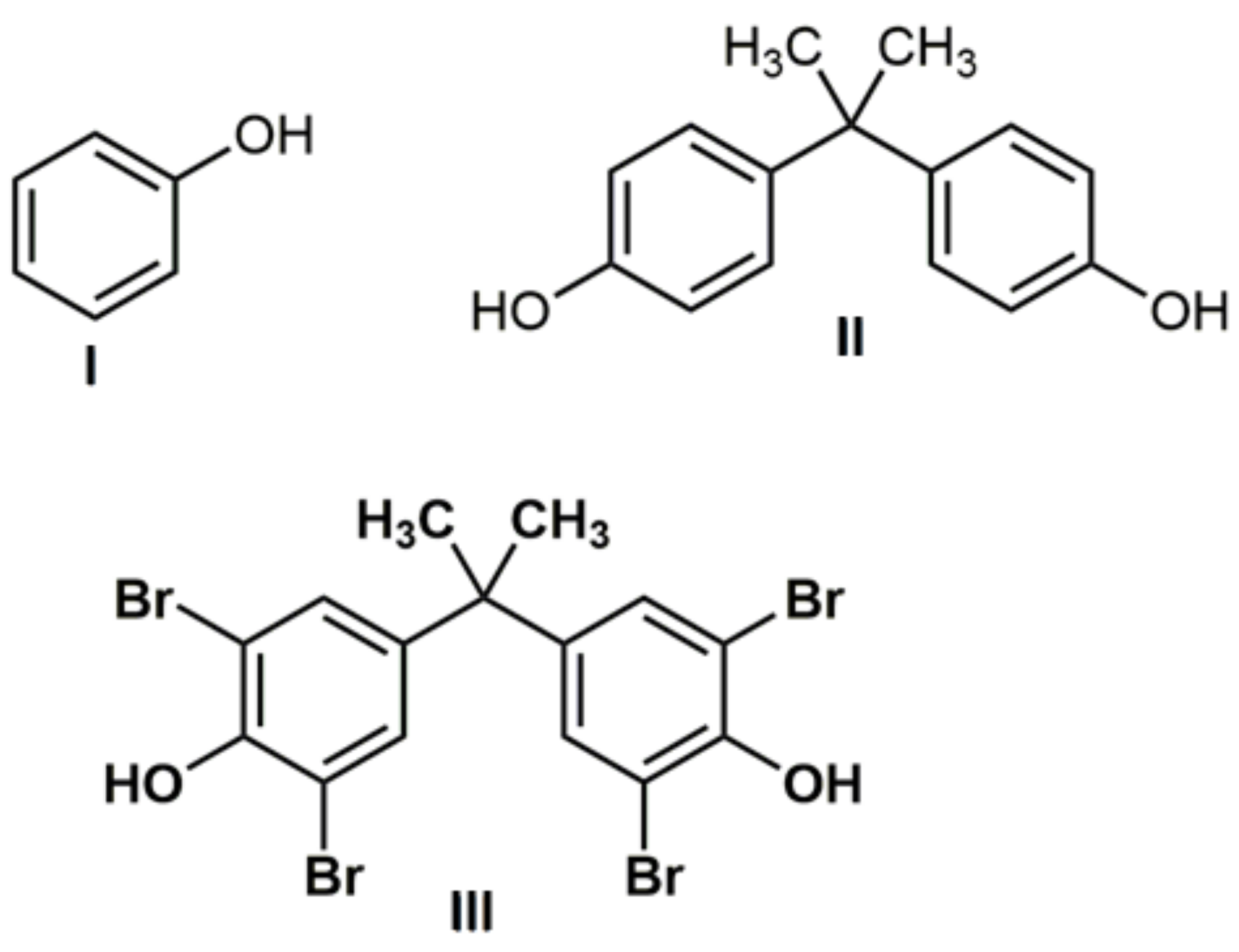

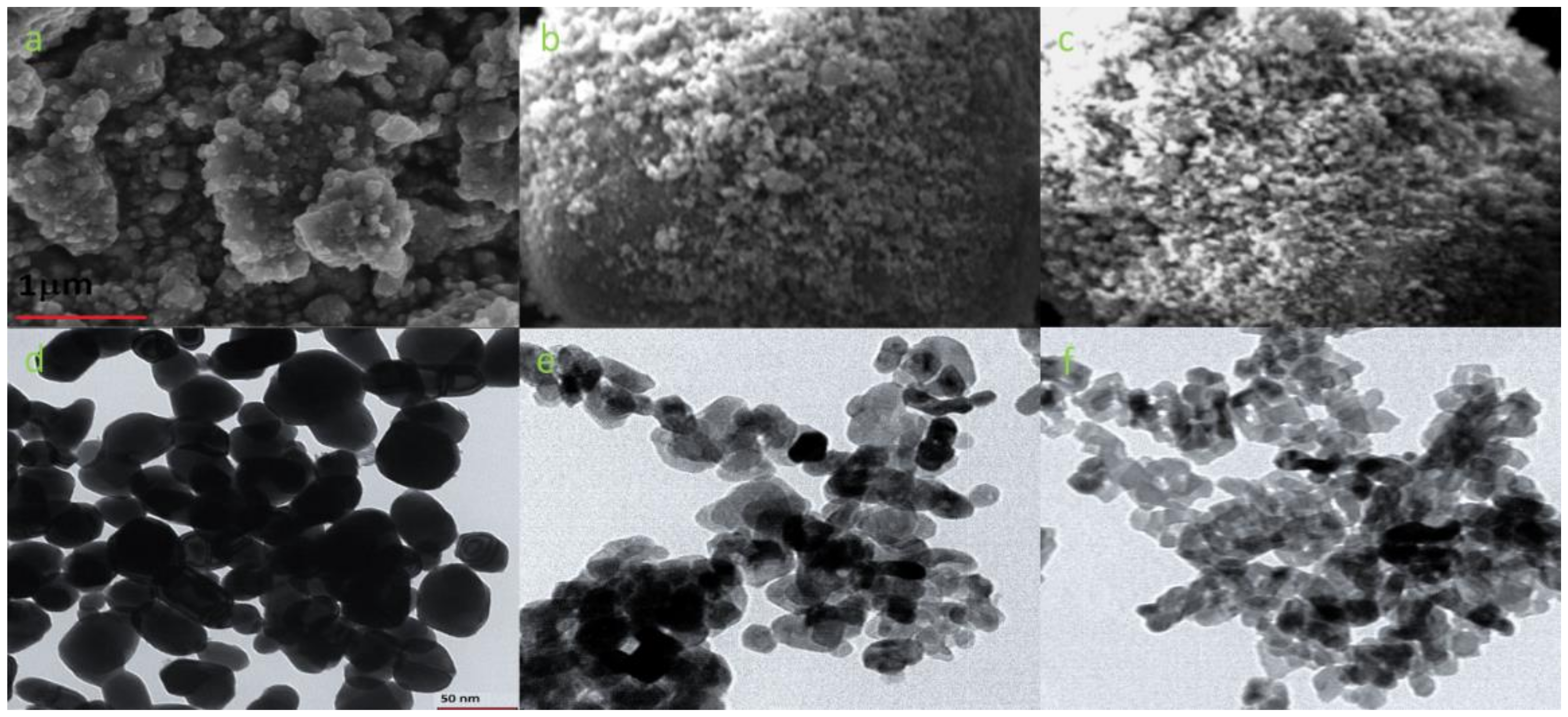


| Sample | ξ-Potential # [mV] | Hydrodynamic Diameter # [nm] | Đ # | Surface Area, m2g−1 | Size [nm] XRD | Sulfur Content [atom%] ## |
|---|---|---|---|---|---|---|
| S0 | −52.17 ± 1.01 | 340.00 | 0.22 | 8.90 | 46.00 | 0 |
| S1 | −36.64 ± 0.55 | 416.00 | 0.24 | 52.30 | 20.00 | 0.15 ± 0.05 |
| S2 | −34.73 ± 0.47 | 504.00 | 0.27 | 55.70 | 18.50 | 0.36 ± 0.04 |
| Sample | pK1 | pK2 | pHpzc | Surface Charge Density [C m−2] pH = 10 | ζ Potential [mV] pH = 10 |
|---|---|---|---|---|---|
| S0 | 2.51 ± 0.2 | 9.63 ± 0.2 | 6.07 ± 0.1 | −321.00 | −52.17 ± 1.01 |
| S1 | 2.39 ± 0.2 | 11.28 ± 0.2 | 6.84 ± 0.1 | −59.50 | −36.64 ± 0.55 |
| S2 | 2.61 ± 0.2 | 11.30 ± 0.2 | 6.96 ± 0.1 | −53.00 | −34.73 ± 0.47 |
| Substrate/Catalyst | C0 [g dm−3]/[mol dm−3] | KL [dm3 g−1] | Qmax [g g−1]/[mol g−1] | RL |
|---|---|---|---|---|
| I | 1.88 × 10−2/2.00 × 10−4 | |||
| S0 | 3.74 | 2.28 × 10−2/2.42 × 10−4 | 0.93 | |
| Ca. | 7.47 | 1.00 × 10−2/1.07 × 10−4 | 0.88 | |
| S2 | 6.59 | 1.33 × 10−2/1.41 × 10−4 | 0.89 | |
| II | 4.57 × 10−2/2.00 × 10−4 | |||
| S0 | 1.01 | 19.91 × 10−2/8.72 × 10−4 | 0.96 | |
| S1 | 7.27 | 6.11 × 10−2/2.67 × 10−4 | 0.75 | |
| S2 | 8.30 | 9.23 × 10−2/4.04 × 10−4 | 0.72 | |
| III | 1.10 × 10−2/2.00 × 10−4 | |||
| S0 | 3.10 | 8.96 × 10−2/1.65 × 10−4 | 0.75 | |
| S1 | 3.72 | 4.07 × 10−2/0.75 × 10−4 | 0.71 | |
| S2 | 0.89 | 12.13 × 10−2/2.23 × 10−4 | 0.91 |
| Sample | XRD Size, nm | Ebgd a, eV | Ebgi b, eV | E intra Gap State [eV] | Eu, eV | EF, eV | ECB, eV | E0CB, V vs. SHE | EVB, eV | E0VB, V vs. SHE |
|---|---|---|---|---|---|---|---|---|---|---|
| S0 | 46.0 | 3.26 | 2.86 | - | 0.27 | 3.46 | 3.69 | −0.23 | 0.43 | +3.03 |
| S1 | 20.0 | 3.24 | 2.68 | 1.81 | 0.32 | 3.59 | 3.77 | −0.16 | 0.53 | +3.06 |
| S2 | 18.5 | 3.22 | 2.64 | 1.89 | 0.33 | 3.60 | 3.78 | −0.18 | 0.56 | +3.04 |
| I | II | III | ||||
|---|---|---|---|---|---|---|
| Photocatalyst | t1/2, min | kapp × 10−3, min−1 | t1/2, min | kapp × 10−3, min−1 | t1/2, min | kapp × 10−3, min−1 |
| S0 | 91.20 | 7.60 | 59.24 | 11.70 | 5.28 | 131.40 |
| S1 | 59.24 | 11.70 | 49.16 | 14.10 | 2.99 | 231.40 |
| S2 | 47.48 | 14.60 | 43.05 | 16.10 | 1.54 | 450.10 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kisała, J.B.; Hörner, G.; Barylyak, A.; Pogocki, D.; Bobitski, Y. Photocatalytic Degradation of 4,4′-Isopropylidenebis(2,6-dibromophenol) on Sulfur-Doped Nano TiO2. Materials 2022, 15, 361. https://doi.org/10.3390/ma15010361
Kisała JB, Hörner G, Barylyak A, Pogocki D, Bobitski Y. Photocatalytic Degradation of 4,4′-Isopropylidenebis(2,6-dibromophenol) on Sulfur-Doped Nano TiO2. Materials. 2022; 15(1):361. https://doi.org/10.3390/ma15010361
Chicago/Turabian StyleKisała, Joanna B., Gerald Hörner, Adriana Barylyak, Dariusz Pogocki, and Yaroslav Bobitski. 2022. "Photocatalytic Degradation of 4,4′-Isopropylidenebis(2,6-dibromophenol) on Sulfur-Doped Nano TiO2" Materials 15, no. 1: 361. https://doi.org/10.3390/ma15010361
APA StyleKisała, J. B., Hörner, G., Barylyak, A., Pogocki, D., & Bobitski, Y. (2022). Photocatalytic Degradation of 4,4′-Isopropylidenebis(2,6-dibromophenol) on Sulfur-Doped Nano TiO2. Materials, 15(1), 361. https://doi.org/10.3390/ma15010361







