The Optimization of the Transition Zone of the Planar Heterogeneous Interface for High-Performance Seawater Desalination
Abstract
:1. Introduction
2. Materials and Methods
2.1. Numerical Calculation
2.2. Models Parameters
| Parameter | Description | Value | Parameters Involved in the Manuscript |
|---|---|---|---|
| D | Interlayer distance | 2 nm | Figure 1 and Figure 2, Figures 4 and 5, |
| Figures S1–S3, Figures S6–S9 | |||
| 2, 4, 6, 8 10 nm | Figure 3 | ||
| 2, 6, 10 nm | Figure S4 | ||
| 2, 10 nm | Figure S5 | ||
| L | Length of nanochannel | 100 mM | Figure 1, Figure 2, Figure 3, Figure 4 and Figure 5, Figures S1–S9 |
| C0 | Electrolyte concentration | 100 mM | Figure 1, Figure 2, Figure 3, Figure 4 and Figure 5, Figures S1–S9 |
| σ | Surface charge density | ±0.06 C/m2 | Figure 1, Figure 2, Figure 3, Figure 4 and Figure 5, Figures S1–S9 |
| eL | Length of one-side charge region | 49 nm | Figure 1, Figure 2 and Figure 3, Figures S1–S5 |
| 49, 30, 20, 10, 2 nm | Figure 4, Figure 5, Figure S7 | ||
| 47.5, 10, 2 nm | Figure 5 | ||
| 30 nm, 2 nm | Figures S8 and S9 | ||
| 49, 40, 30, 20, 10, 2 nm | Figure S6 | ||
| d | Length of the transition zone | 2 nm | Figure 1, Figure 2 and Figure 3, Figures S1–S5 |
| 2, 40, 60, 80, 96 nm | Figure 4 and Figure 5, Figure S7 | ||
| 5, 80, 96 nm | Figure 5 | ||
| 40 nm, 96 nm | Figures S8 and S9 | ||
| 2, 20, 40, 60, 80, 96 nm | Figure S6 | ||
| Dp | Diffusion coefficient of Na+ | 1.344 × 10−5 cm2 s−1 | Figure 1, Figure 2, Figure 3, Figure 4 and Figure 5, Figures S1–S9 |
| Dn | Diffusion coefficient of Cl− | 2.032 × 10−5 cm2 s−1 | Figure 1, Figure 2, Figure 3, Figure 4 and Figure 5, Figures S1–S9 |
| ΔU | Voltage | 0.2 V | Figure 1, Figure 3, Figure 5, Figure S1–S9 |
| 0.1, 0.2, 0.3, 0.4, 0.5 V | Figure 2, | ||
| 0.1, 0.2, 0.5 V | Figure 4, | ||
| ΔP | Pressure | 0.1 MPa | Figure 1, Figure 3, Figure 4 and Figure 5, Figures S1–S9 |
| 0.02, 0.04, 0.06, 0.08, 0.1 MPa | Figure S2 |
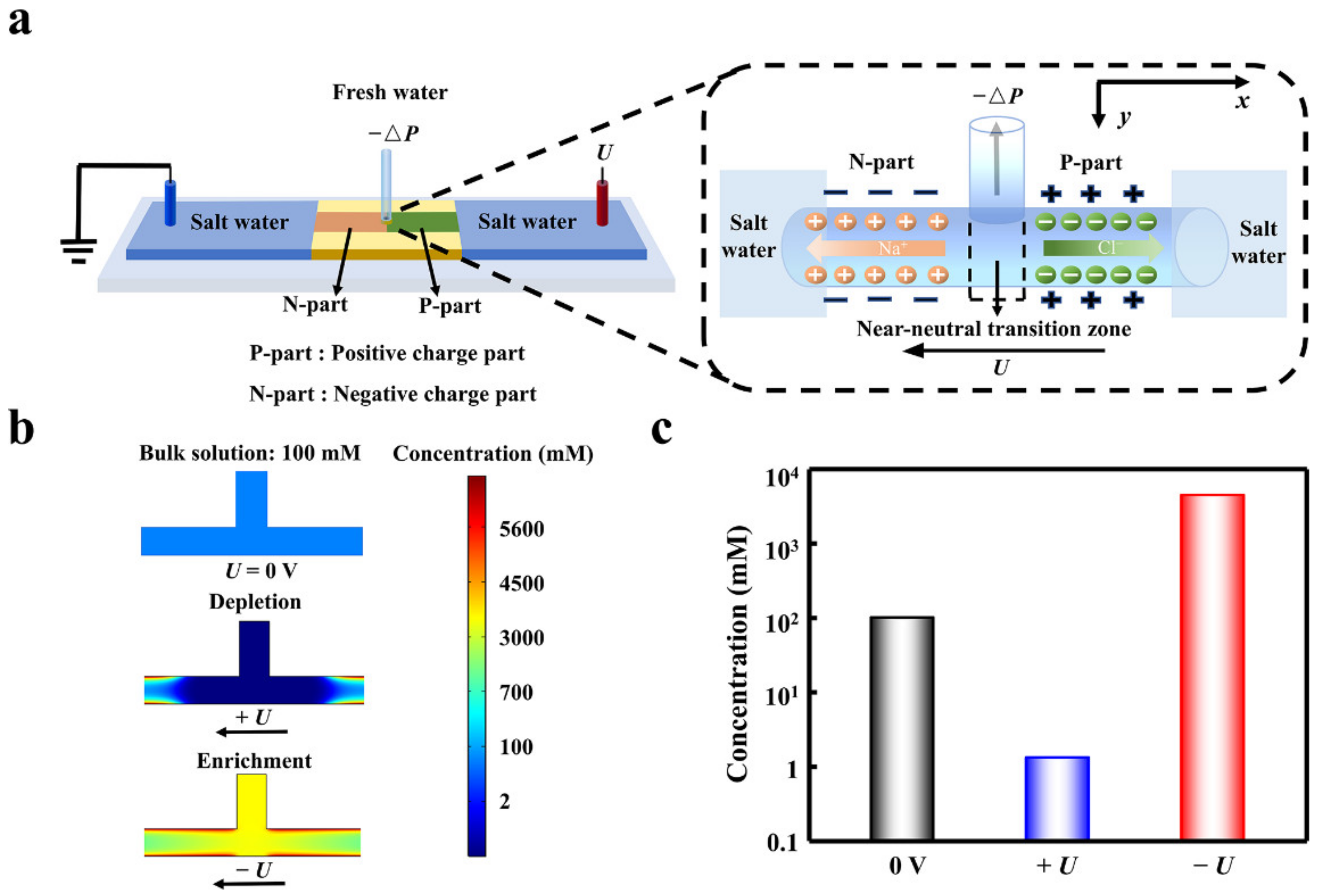
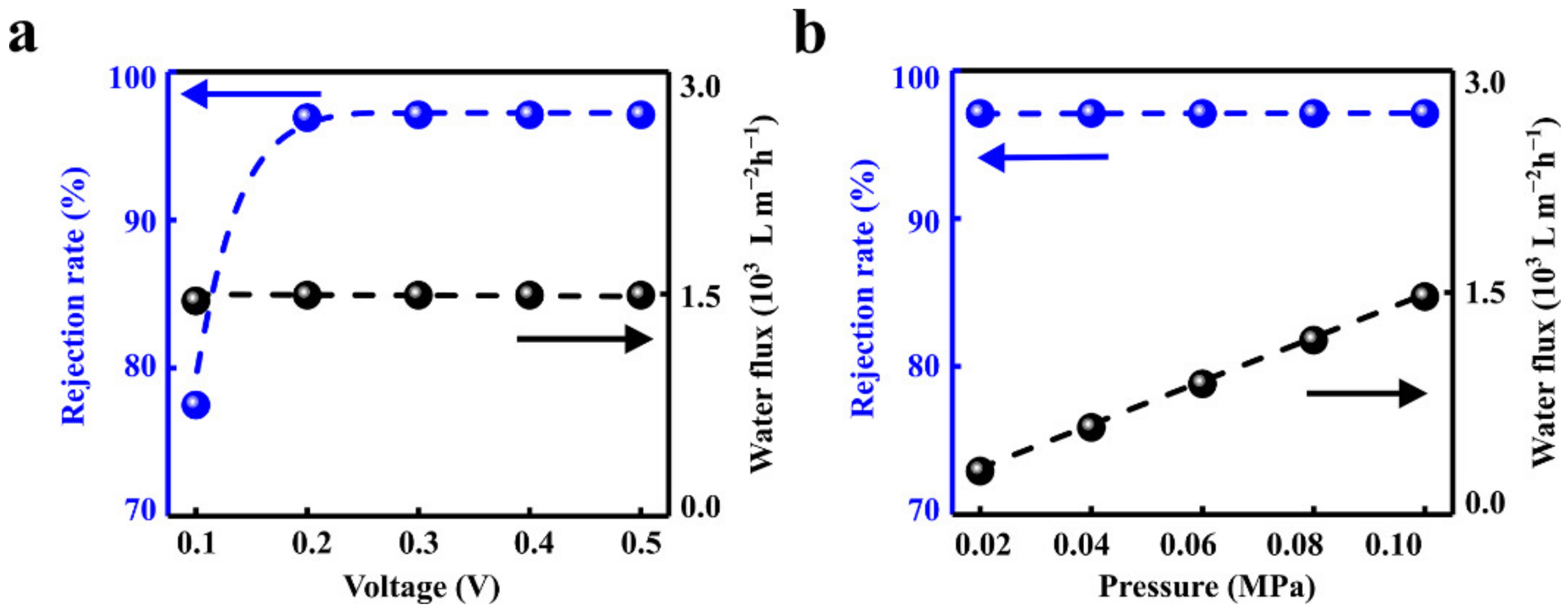

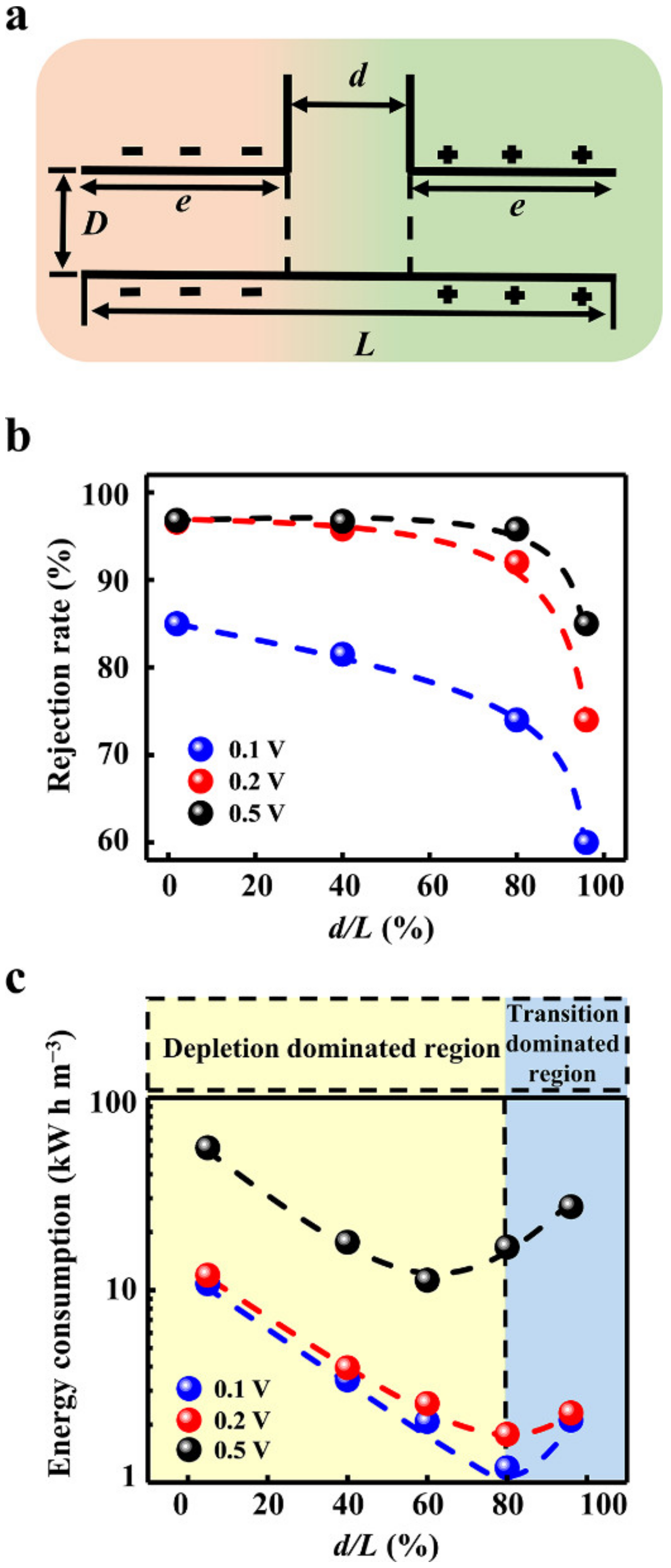
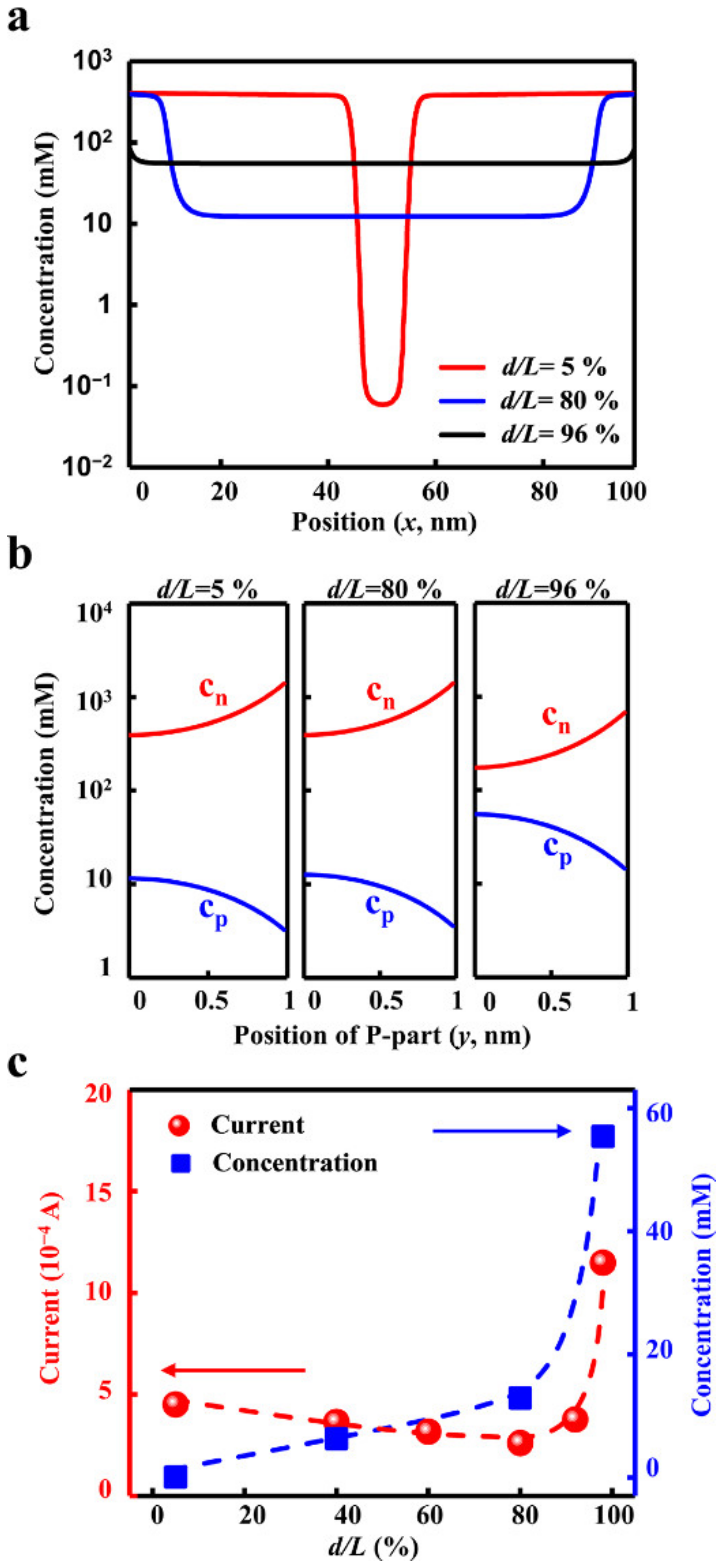
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| 2DMs | two-dimensional membranes |
| EDL | electric double layer |
| NS | Navier–Stokes |
| PNP | Poisson–Nernst–Planck |
| ci | local ion concentration |
| c+ | concentration of cations |
| c- | concentration of anions |
| Di | diffusion coefficient |
| e | dielectric constant of the electrolyte solution |
| Faraday constant | |
| i | ionic species |
| local ionic flux | |
| kB | Boltzmann constant |
| the unit vector in normal direction | |
| p | pressure |
| T | temperature |
| fluid velocity | |
| zi | valence |
| z+ | charge number of cations |
| z- | charge number of anions |
| ε | electron charge |
| ε0 | permittivity of vacuum |
| εr | relative dielectric constant of the electrolyte solution |
| viscosity of solution | |
| density of solution | |
| Φ | local electrical potential |
References
- Grant, S.B.; Saphores, J.-D.; Feldman, D.L.; Hamilton, A.J.; Fletcher, T.D.; Cook, P.L.; Stewardson, M.; Sanders, B.F.; Levin, L.A.; Ambrose, R.F.J.S. Taking the “waste” out of “wastewater” for human water security and ecosystem sustainability. Science 2012, 337, 681–686. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Tang, J.Y.M.; Busetti, F.; Charrois, J.W.A.; Escher, B.I. Which chemicals drive biological effects in wastewater and recycled water? Water Res. 2014, 60, 289–299. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Liu, H.; Huang, Z.; Liu, K.; Hu, X.; Zhou, J. Interfacial Solar-to-Heat Conversion for Desalination. Adv. Energy Mater. 2019, 9, 1900310. [Google Scholar] [CrossRef]
- Werber, J.R.; Osuji, C.O.; Elimelech, M. Materials for next-generation desalination and water purification membranes. Nat. Rev. Mater. 2016, 1, 16018. [Google Scholar] [CrossRef]
- Dudchenko, A.V.; Chen, C.; Cardenas, A.; Rolf, J.; Jassby, D. Frequency-dependent stability of CNT Joule heaters in ionizable media and desalination processes. Nat. Nanotechnol. 2017, 12, 557–563. [Google Scholar] [CrossRef] [PubMed]
- Lin, S. Energy Efficiency of Desalination: Fundamental Insights from Intuitive Interpretation. Environ. Sci. Technol. 2020, 54, 76–84. [Google Scholar] [CrossRef]
- Hepburn, C.; Qi, Y.; Stern, N.; Ward, B.; Xie, C.; Zenghelis, D. Towards carbon neutrality and China’s 14th Five-Year Plan: Clean energy transition, sustainable urban development, and investment priorities. Environ. Sci. Ecotechnol. 2021, 8, 100130. [Google Scholar] [CrossRef]
- Elimelech, M.; Phillip, W.A. The Future of Seawater Desalination: Energy, Technology, and the Environment. Science 2011, 333, 712–717. [Google Scholar] [CrossRef]
- Abraham, J.; Vasu, K.S.; Williams, C.D.; Gopinadhan, K.; Su, Y.; Cherian, C.T.; Dix, J.; Prestat, E.; Haigh, S.J.; Grigorieva, I.V.; et al. Tunable sieving of ions using graphene oxide membranes. Nat. Nanotechnol. 2017, 12, 546–550. [Google Scholar] [CrossRef]
- Amy, G.; Ghaffour, N.; Li, Z.; Francis, L.; Linares, R.V.; Missimer, T.; Lattemann, S. Membrane-based seawater desalination: Present and future prospects. Desalination 2017, 401, 16–21. [Google Scholar] [CrossRef]
- Zhang, Z.; Shen, W.; Lin, L.; Wang, M.; Li, N.; Zheng, Z.; Liu, F.; Cao, L. Vertically Transported Graphene Oxide for High-Performance Osmotic Energy Conversion. Adv. Sci. 2020, 7, 2000286. [Google Scholar] [CrossRef] [PubMed]
- Ghaffour, N.; Missimer, T.M.; Amy, G.L. Technical review and evaluation of the economics of water desalination: Current and future challenges for better water supply sustainability. Desalination 2013, 309, 197–207. [Google Scholar] [CrossRef] [Green Version]
- Alkaisi, A.; Mossad, R.; Sharifian-Barforoush, A. A review of the water desalination systems integrated with renewable energy. Energy Procedia 2017, 110, 268–274. [Google Scholar] [CrossRef]
- Lim, Y.J.; Goh, K.; Kurihara, M.; Wang, R. Seawater desalination by reverse osmosis: Current development and future challenges in membrane fabrication—A review. J. Membr. Sci. 2021, 629, 119292. [Google Scholar] [CrossRef]
- Ihsanullah, I.; Atieh, M.A.; Sajid, M.; Nazal, M.K. Desalination and environment: A critical analysis of impacts, mitigation strategies, and greener desalination technologies. Sci. Total Environ. 2021, 780, 146585. [Google Scholar] [CrossRef] [PubMed]
- Voutchkov, N. Energy use for membrane seawater desalination–current status and trends. Desalination 2018, 431, 2–14. [Google Scholar] [CrossRef]
- Lin, S.; Zhao, H.; Zhu, L.; He, T.; Chen, S.; Gao, C.; Zhang, L. Seawater desalination technology and engineering in China: A review. Desalination 2021, 498, 114728. [Google Scholar] [CrossRef]
- Pendergast, M.M.; Hoek, E.M.J.E. A review of water treatment membrane nanotechnologies. Science 2011, 4, 1946–1971. [Google Scholar] [CrossRef] [Green Version]
- Ma, P.; Zheng, J.; Zhao, D.; Zhang, W.; Lu, G.; Lin, L.; Zhao, Z.; Huang, Z.; Cao, L. The Selective Transport of Ions in Charged Nanopore with Combined Multi-Physics Fields. Materials 2021, 14, 7012. [Google Scholar] [CrossRef]
- Imbault, A.; Wang, Y.; Kruse, P.; Strelcov, E.; Comini, E.; Sberveglieri, G.; Kolmakov, A. Ultrathin gas permeable oxide membranes for chemical sensing: Nanoporous Ta2O5 test study. Materials 2015, 8, 6677–6684. [Google Scholar] [CrossRef] [Green Version]
- Kim, I.I.; Kihm, K.D. Nano sensing and energy conversion using surface plasmon resonance (SPR). Materials 2015, 8, 4332–4343. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Morelos-Gomez, A.; Cruz-Silva, R.; Muramatsu, H.; Ortiz-Medina, J.; Araki, T.; Fukuyo, T.; Tejima, S.; Takeuchi, K.; Hayashi, T.; Terrones, M.; et al. Effective NaCl and dye rejection of hybrid graphene oxide/graphene layered membranes. Nat. Nanotechnol. 2017, 12, 1083–1088. [Google Scholar] [CrossRef] [PubMed]
- Xiao, F.; Ji, D.; Li, H.; Tang, J.; Feng, Y.; Ding, L.; Cao, L.; Li, N.; Jiang, L.; Guo, W. Simulation of osmotic energy conversion in nanoporous materials: A concise single-pore model. Inorg. Chem. Front. 2018, 5, 1677–1682. [Google Scholar] [CrossRef]
- Qu, R.; Zeng, X.; Lin, L.; Zhang, G.; Liu, F.; Wang, C.; Ma, S.; Liu, C.; Miao, H.; Cao, L. Vertically-Oriented Ti3C2Tx MXene Membranes for High Performance of Electrokinetic Energy Conversion. ACS Nano 2020, 14, 16654–16662. [Google Scholar] [CrossRef] [PubMed]
- Cao, L.; Wang, Y. Fabrication and investigation of single track-etched nanopore and its applications. Radiat. Measur. 2009, 44, 1093–1099. [Google Scholar] [CrossRef]
- Qiu, L.; Zhang, X.; Yang, W.; Wang, Y.; Simon, G.P.; Li, D. Controllable corrugation of chemically converted graphene sheets in water and potential application for nanofiltration. Chem. Commun. 2011, 47, 5810–5812. [Google Scholar] [CrossRef] [PubMed]
- Tseng, S.; Li, Y.M.; Lin, C.Y.; Hsu, J.P. Salinity gradient power: Influences of temperature and nanopore size. Nanoscale 2016, 8, 2350–2357. [Google Scholar] [CrossRef]
- Xie, X.; Crespo, G.A.; Mistlberger, G.; Bakker, E. Photocurrent generation based on a light-driven proton pump in an artificial liquid membrane. Nat. Chem. 2014, 6, 202–207. [Google Scholar] [CrossRef]
- Zhang, X.; Wen, Q.; Wang, L.; Ding, L.; Yang, J.; Ji, D.; Zhang, Y.; Jiang, L.; Guo, W. Asymmetric Electrokinetic Proton Transport through 2D Nanofluidic Heterojunctions. ACS Nano 2019, 13, 4238–4245. [Google Scholar] [CrossRef]
- Jia, P.; Wen, Q.; Liu, D.; Zhou, M.; Jin, X.; Ding, L.; Dong, H.; Lu, D.; Jiang, L.; Guo, W. Highly Efficient Ionic Photocurrent Generation through WS2-Based 2D Nanofluidic Channels. Small 2019, 15, 1905355. [Google Scholar] [CrossRef]
- Chen, L.; Shi, G.; Shen, J.; Peng, B.; Zhang, B.; Wang, Y.; Bian, F.; Wang, J.; Li, D.; Qian, Z.; et al. Ion sieving in graphene oxide membranes via cationic control of interlayer spacing. Nature 2017, 550, 380–383. [Google Scholar] [CrossRef] [PubMed]
- Yang, X.; Cheng, C.; Wang, Y.; Qiu, L.; Li, D. Liquid-Mediated Dense Integration of Graphene Materials for Compact Capacitive Energy Storage. Science 2013, 341, 534–537. [Google Scholar] [CrossRef] [PubMed]
- Su, Y.; Kravets, V.G.; Wong, S.L.; Waters, J.; Geim, A.K.; Nair, R.R. Impermeable barrier films and protective coatings based on reduced graphene oxide. Nat. Commun. 2014, 5, 4843. [Google Scholar] [CrossRef] [PubMed]
- Park, H.B.; Kamcev, J.; Robeson, L.M.; Elimelech, M.; Freeman, B.D. Maximizing the right stuff: The trade-off between membrane permeability and selectivity. Science 2017, 356, 1137. [Google Scholar] [CrossRef] [Green Version]
- Kim, S.J.; Ko, S.H.; Kang, K.H.; Han, J. Direct seawater desalination by ion concentration polarization. Nat. Nanotechnol. 2010, 5, 297–301. [Google Scholar] [CrossRef]
- Cao, L.; Xiao, F.; Feng, Y.; Zhu, W.; Geng, W.; Yang, J.; Zhang, X.; Li, N.; Guo, W.; Jiang, L. Anomalous Channel-Length Dependence in Nanofluidic Osmotic Energy Conversion. Adv. Funct. Mater. 2017, 27, 1604302. [Google Scholar] [CrossRef]
- Guo, W.; Tian, Y.; Jiang, L. Asymmetric Ion Transport through Ion-Channel-Mimetic Solid-State Nanopores. Acc. Chem. Res. 2013, 46, 2834–2846. [Google Scholar] [CrossRef]
- Li, H.; Xiao, F.; Hong, G.; Su, J.; Li, N.; Cao, L.; Wen, Q.; Guo, W. On the Role of Heterogeneous Nanopore Junction in Osmotic Power Generation. Chin. J. Chem. 2019, 37, 469–473. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, C.; Lin, L.; Xu, M.; Wu, Y.; Cao, L. Rectified Ion Transport in Ultra-thin Membrane Governed by Outer Membrane Electric Double Layer. Chin. J. Chem. 2020, 38, 1757–1761. [Google Scholar] [CrossRef]
- Han, Y.; Xu, Z.; Gao, C. Ultrathin Graphene Nanofiltration Membrane for Water Purification. Adv. Funct. Mater. 2013, 23, 3693–3700. [Google Scholar] [CrossRef]
- Feng, Y.; Zhu, W.; Guo, W.; Jiang, L. Bioinspired Energy Conversion in Nanofluidics: A Paradigm of Material Evolution. Adv. Mater. 2017, 29, 1702773. [Google Scholar] [CrossRef] [PubMed]
- Ji, D.; Wen, Q.; Cao, L.; Kang, Q.; Lin, S.; Zhang, X.; Jiang, L.; Guo, W. Electrokinetically Controlled Asymmetric Ion Transport through 1D/2D Nanofluidic Heterojunctions. Adv. Mater. Technol. 2019, 4, 1800742. [Google Scholar] [CrossRef]
- Wang, L.; Wen, Q.; Jia, P.; Jia, M.; Lu, D.; Sun, X.; Jiang, L.; Guo, W. Light-Driven Active Proton Transport through Photoacid- and Photobase-Doped Janus Graphene Oxide Membranes. Adv. Mater. 2019, 31, 1903029. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Li, F.; Kong, X.; Xue, T.; Liu, D.; Jia, P.; Wang, L.; Ding, L.; Dong, H.; Lu, D.; et al. Photoinduced Directional Proton Transport through Printed Asymmetric Graphene Oxide Superstructures: A New Driving Mechanism under Full-Area Light Illumination. Adv. Funct. Mater. 2020, 30, 1907549. [Google Scholar] [CrossRef]
- Wen, Q.; Jia, P.; Cao, L.; Li, J.; Quan, D.; Wang, L.; Zhang, Y.; Lu, D.; Jiang, L.; Guo, W. Electric-Field-Induced Ionic Sieving at Planar Graphene Oxide Heterojunctions for Miniaturized Water Desalination. Adv. Mater. 2020, 32, 1903954. [Google Scholar] [CrossRef]
- Al-Karaghouli, A.; Kazmerski, L.L. Energy consumption and water production cost of conventional and renewable-energy-powered desalination processes. Renew. Sustain. Energ. Rev. 2013, 24, 343–356. [Google Scholar] [CrossRef]
- Zarzo, D.; Prats, D. Desalination and energy consumption. What can we expect in the near future? Desalination 2018, 427, 1–9. [Google Scholar] [CrossRef]
- Cao, L.; Wen, Q.; Feng, Y.; Ji, D.; Li, H.; Li, N.; Jiang, L.; Guo, W. On the Origin of Ion Selectivity in Ultrathin Nanopores: Insights for Membrane-Scale Osmotic Energy Conversion. Adv. Funct. Mater. 2018, 28, 1804189. [Google Scholar] [CrossRef]
- Ji, J.; Kang, Q.; Zhou, Y.; Feng, Y.; Chen, X.; Yuan, J.; Guo, W.; Wei, Y.; Jiang, L. Osmotic Power Generation with Positively and Negatively Charged 2D Nanofluidic Membrane Pairs. Adv. Funct. Mater. 2017, 27, 1603623. [Google Scholar] [CrossRef]
- Adham, S.; Hussain, A.; Matar, J.M.; Dores, R.; Janson, A. Application of Membrane Distillation for desalting brines from thermal desalination plants. Desalination 2013, 314, 101–108. [Google Scholar] [CrossRef]
- Millero, F.J.; Feistel, R.; Wright, D.G.; McDougall, T.J. The composition of Standard Seawater and the definition of the Reference-Composition Salinity Scale. Deep Sea Res. 2008, 55, 50–72. [Google Scholar] [CrossRef]
- Ai, Y.; Qian, S. Electrokinetic particle translocation through a nanopore. Phys. Chem. Chem. Phys. 2011, 13, 4060–4071. [Google Scholar] [CrossRef] [PubMed]
- Gao, J.; Guo, W.; Feng, D.; Wang, H.; Zhao, D.; Jiang, L. High-performance ionic diode membrane for salinity gradient power generation. J. Am. Chem. Soc. 2014, 136, 12265–12272. [Google Scholar] [CrossRef] [PubMed]
- Wen, Q.; Yan, D.; Liu, F.; Wang, M.; Ling, Y.; Wang, P.; Kluth, P.; Schauries, D.; Trautmann, C.; Apel, P.J.A.F.M. Highly selective ionic transport through subnanometer pores in polymer films. Adv. Funct. Mater. 2016, 26, 5796–5803. [Google Scholar] [CrossRef]
- Astar, W. Applications of multidimensional distributions in electrostatics. IEEE Trans. Educ. 1998, 41, 104–111. [Google Scholar] [CrossRef]
- Zhen-Peng, G.E.; Yan-Chao, S.H.I.; Xiao-Yi, L.I. Effects of Orthogonal Electric Field on Water Flux through a Carbon Nanotube. Acta Phys.-Chim. Sin. 2013, 29, 1655–1660. [Google Scholar] [CrossRef]
- Shahryari, M.; Nazari-Golshan, A.; Nourazar, S.S. The study of heat flux and external electric field effects on carbon nanotube behavior as an atomic nano-pump. App. Phys. A 2022, 128, 218. [Google Scholar] [CrossRef]
- Thomas, M.; Corry, B. A computational assessment of the permeability and salt rejection of carbon nanotube membranes and their application to water desalination. Philos. Trans. A Math. Phys. Eng. Sci. 2016, 374, 20150020. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, C.; Liu, H.; Ma, P.; Liu, Y.; Cai, R.; Yin, R.; Zhang, B.; Wei, S.; Miao, H.; Cao, L. The Optimization of the Transition Zone of the Planar Heterogeneous Interface for High-Performance Seawater Desalination. Materials 2022, 15, 3561. https://doi.org/10.3390/ma15103561
Liu C, Liu H, Ma P, Liu Y, Cai R, Yin R, Zhang B, Wei S, Miao H, Cao L. The Optimization of the Transition Zone of the Planar Heterogeneous Interface for High-Performance Seawater Desalination. Materials. 2022; 15(10):3561. https://doi.org/10.3390/ma15103561
Chicago/Turabian StyleLiu, Chang, Hui Liu, Pengfei Ma, Yan Liu, Ruochong Cai, Ran Yin, Biao Zhang, Shiqi Wei, Huifang Miao, and Liuxuan Cao. 2022. "The Optimization of the Transition Zone of the Planar Heterogeneous Interface for High-Performance Seawater Desalination" Materials 15, no. 10: 3561. https://doi.org/10.3390/ma15103561
APA StyleLiu, C., Liu, H., Ma, P., Liu, Y., Cai, R., Yin, R., Zhang, B., Wei, S., Miao, H., & Cao, L. (2022). The Optimization of the Transition Zone of the Planar Heterogeneous Interface for High-Performance Seawater Desalination. Materials, 15(10), 3561. https://doi.org/10.3390/ma15103561






