Evaluation and Prediction of the Bending Behavior of Circular Hollow Steel Tube Sections Using Finite Element Analysis
Abstract
1. Introduction
2. Experimental Study
2.1. Materials Properties
2.2. Experimental Specimens
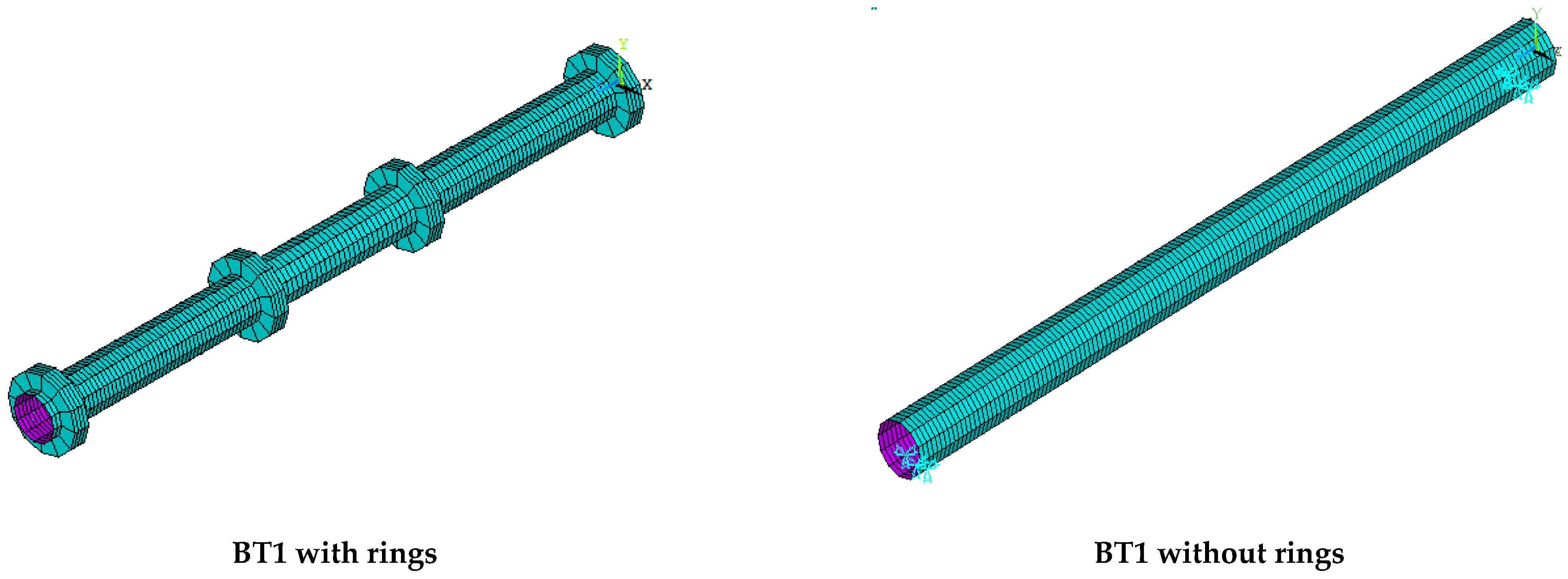
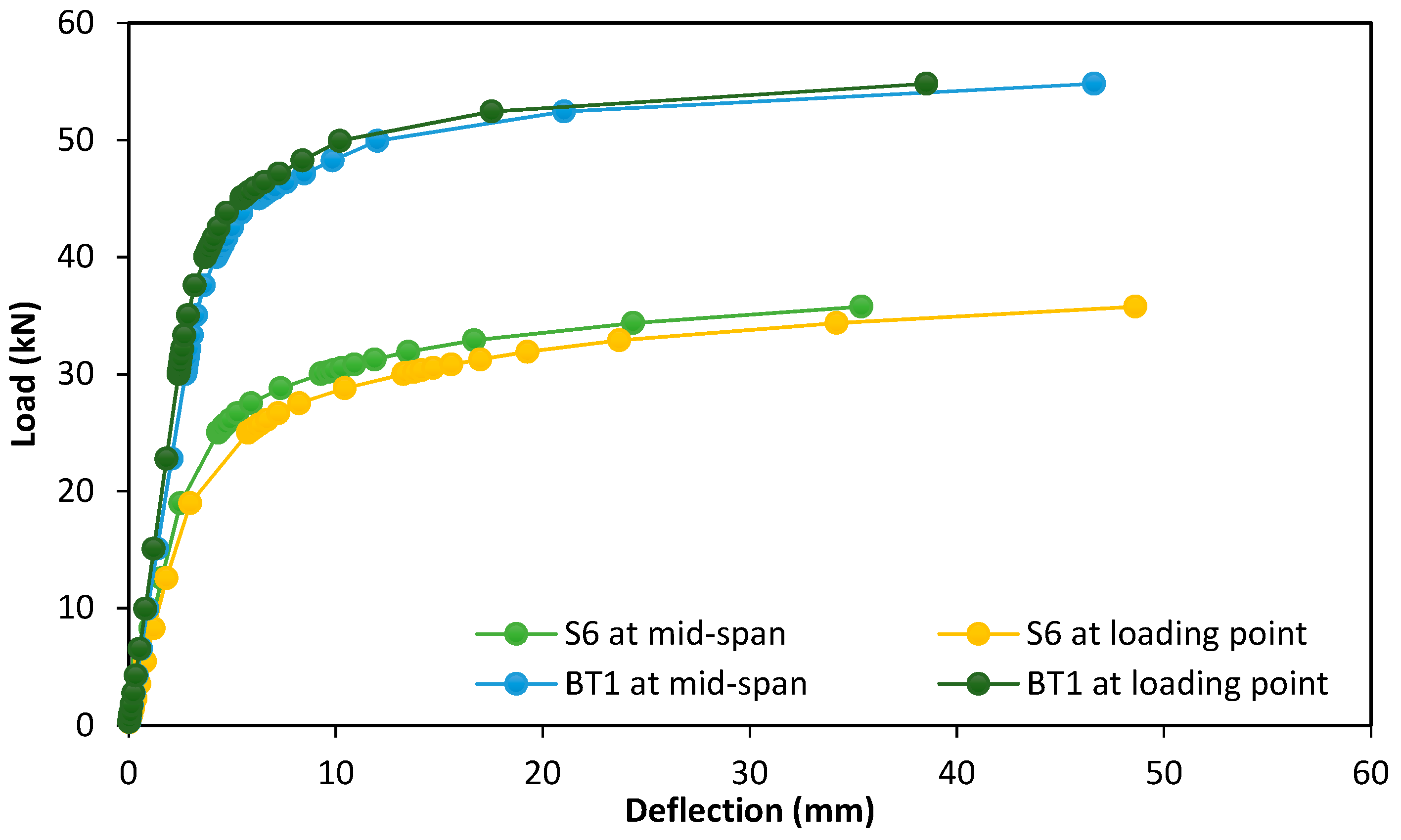
2.2.1. Reference Specimen
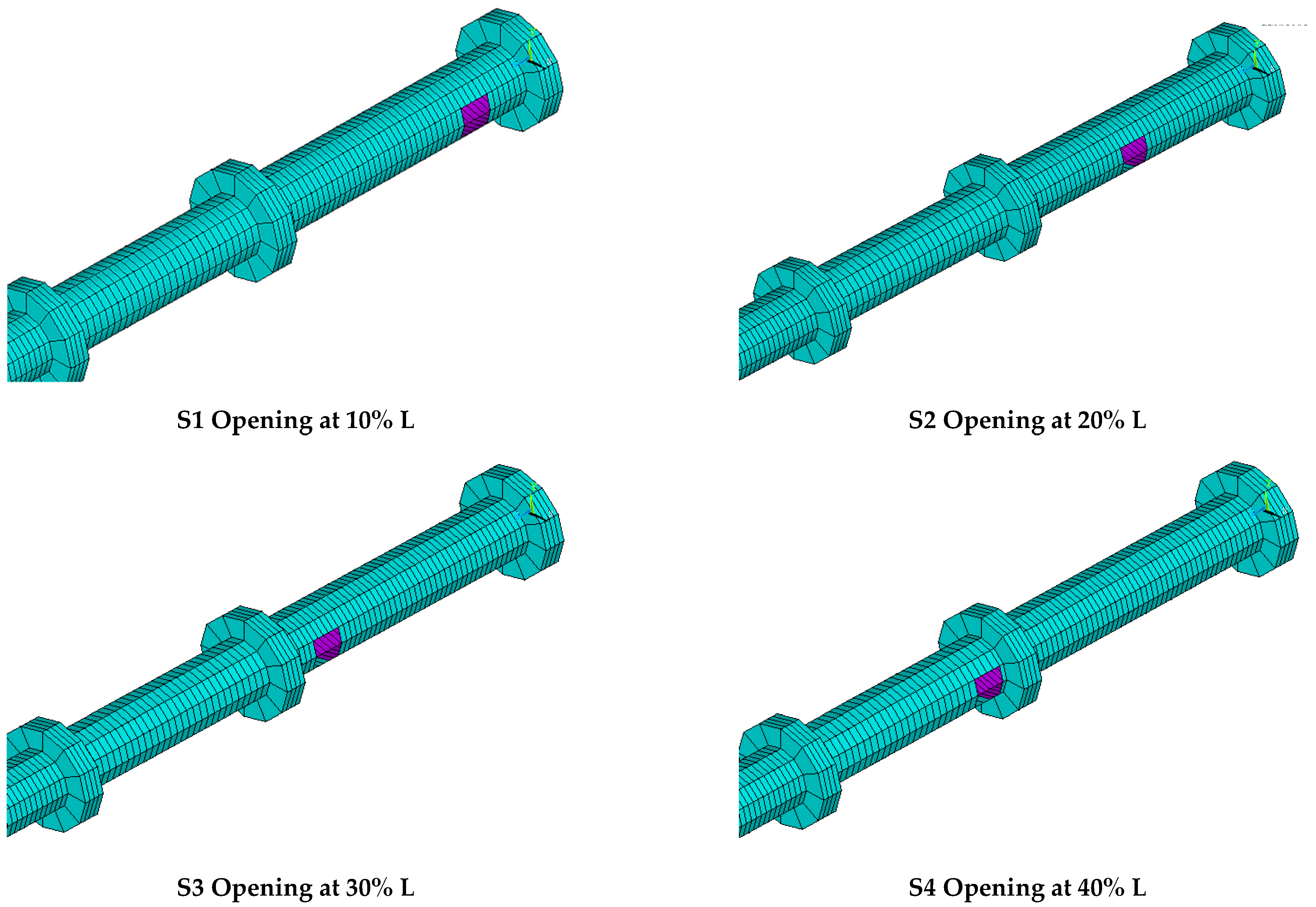
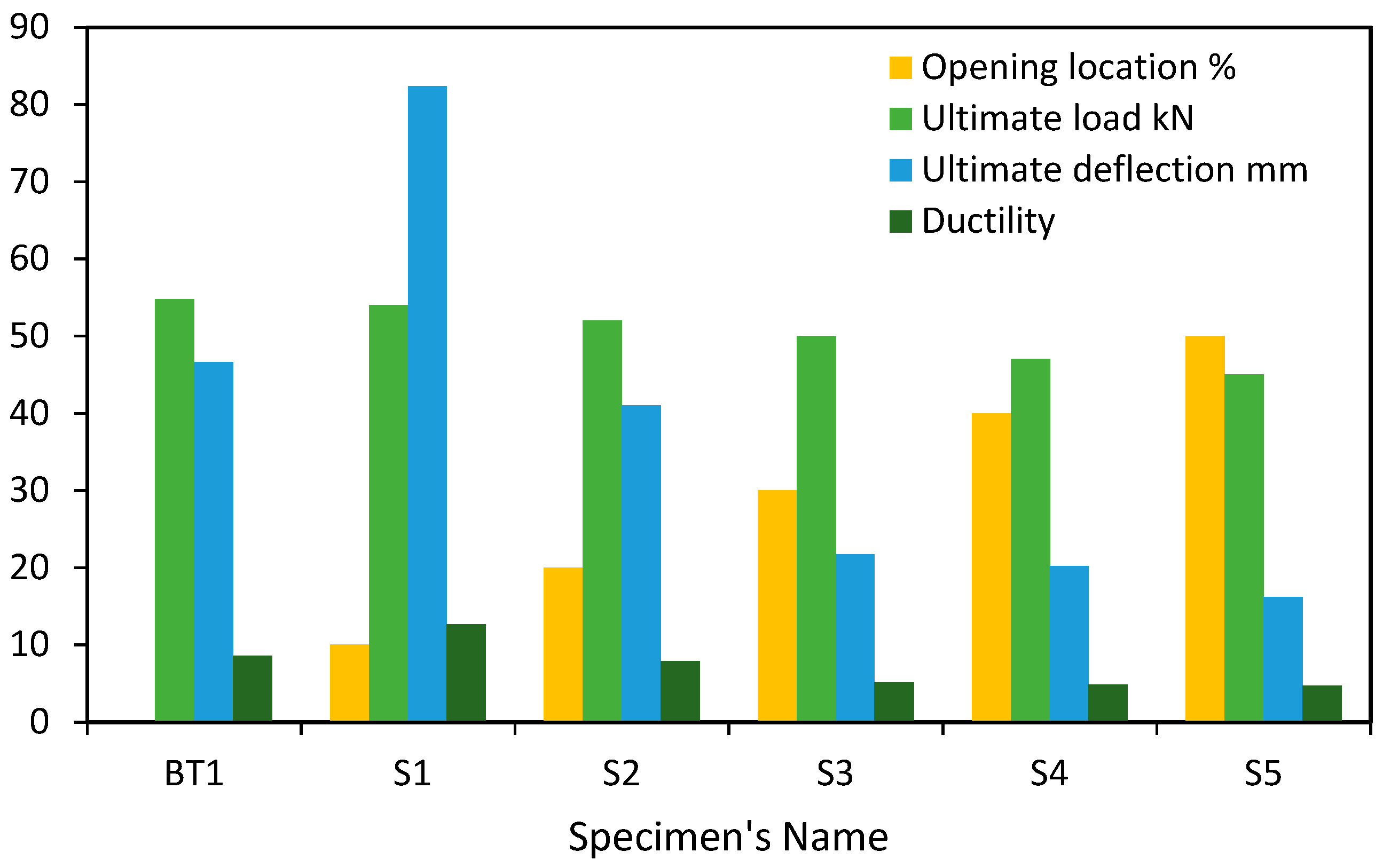
2.2.2. Modified Specimens
- First Group
- 2.
- Second Group:
- 3.
- Third Group
- 4.
- Fourth Group
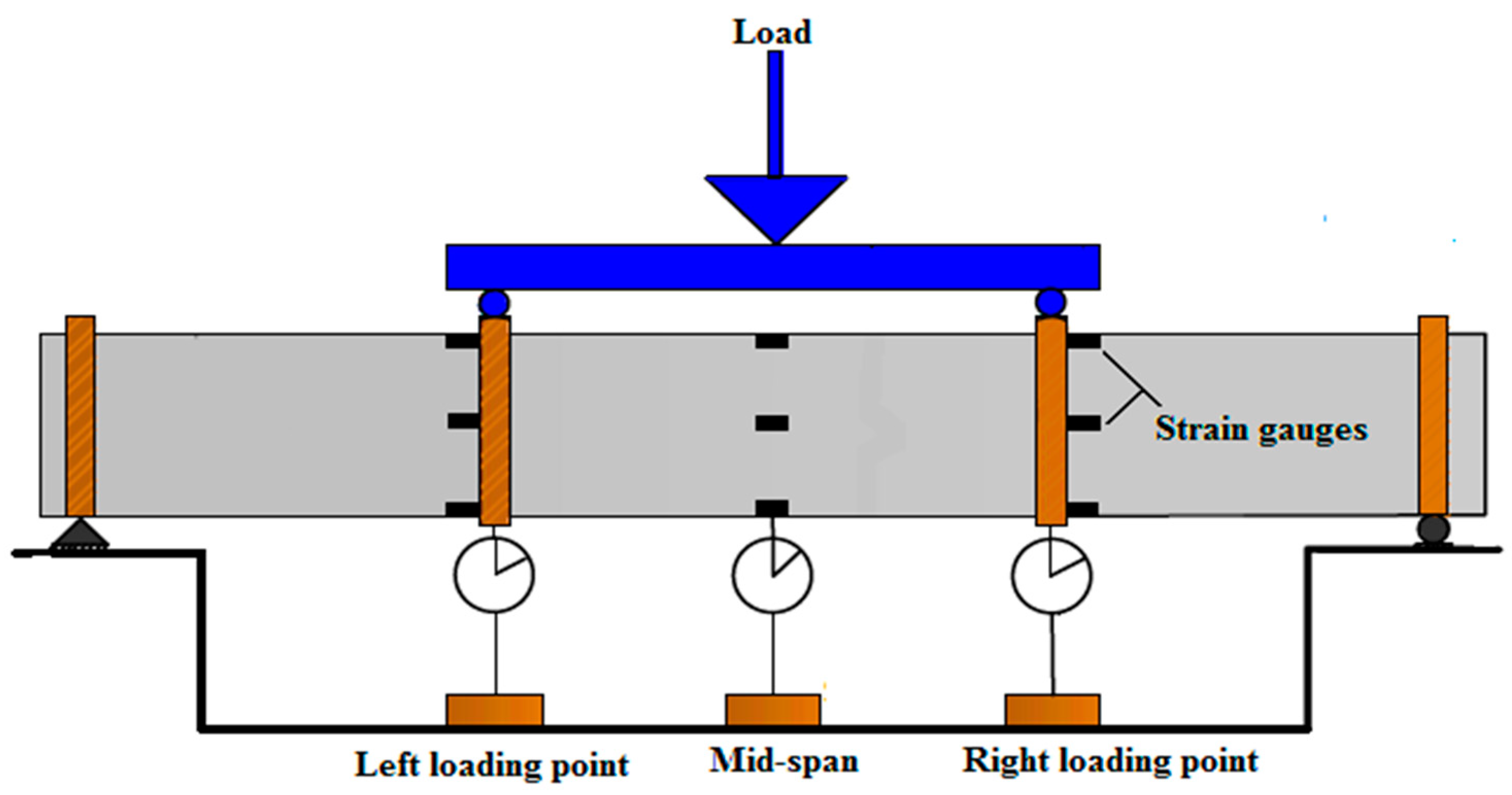
2.3. Test Procedure
- Ensure that the applied load was distributed axially to avoid stress concentration at a single place;
- Support the vertical load;
- Due to the high rigidity and stiffness of these rings, limit radial displacement at loading locations;
- Compress the section of the specimen so it was stiffened and supported adequately, preventing local buckling at the stress locations;
- Prevent the specimens’ sudden failure when they reached the peak load.
2.4. The Measurement Instruments
2.4.1. Dial Gauges
2.4.2. Data Logger
2.4.3. Strain Gauges
3. Numerical Analysis (Finite Element Method (FEM))
3.1. The ANSYS Models
3.1.1. Types of Element and Material Properties
- SHELL181
- SOLID45
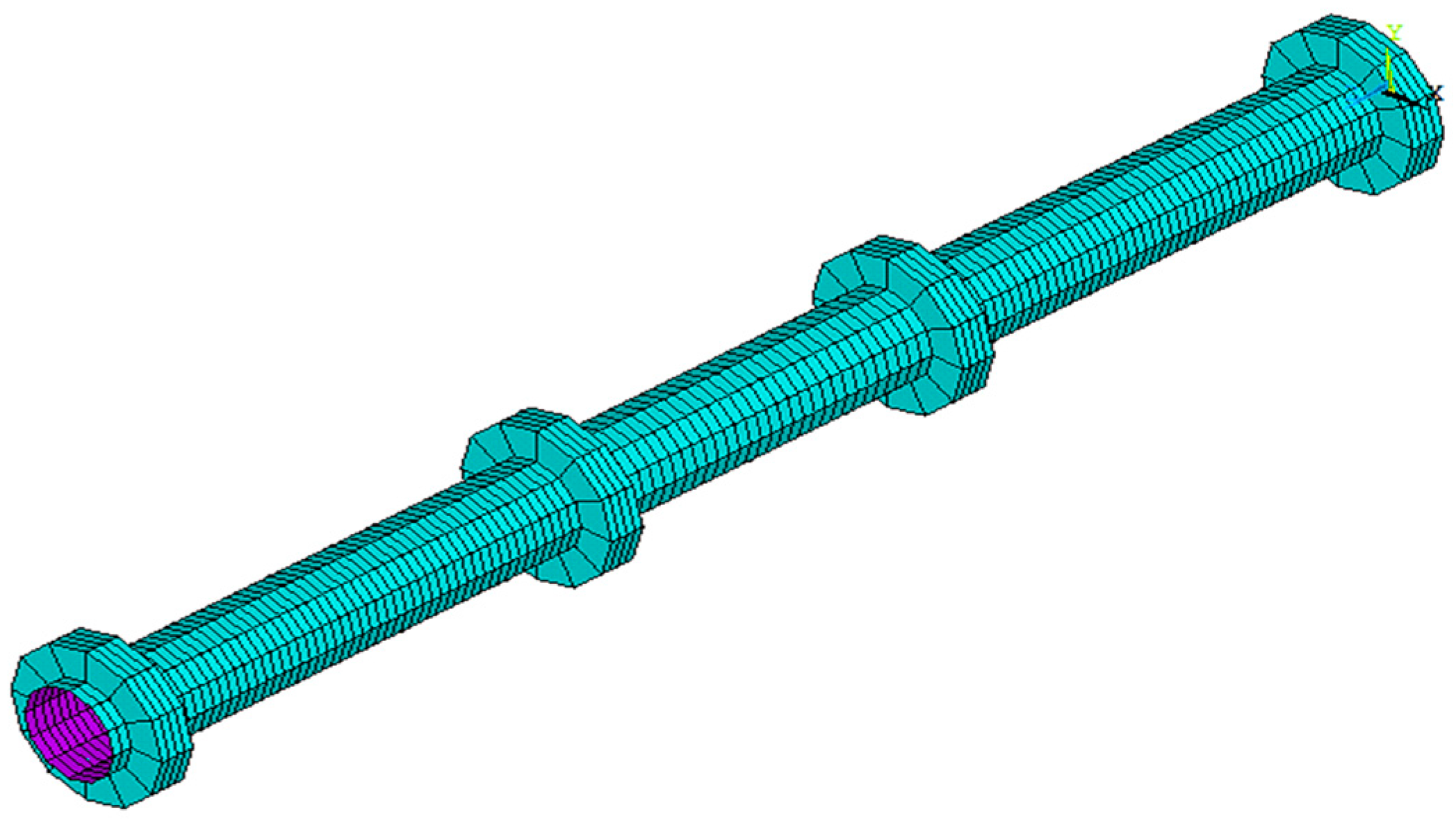
3.1.2. Modeling and Meshing of Circular Hollow Specimens
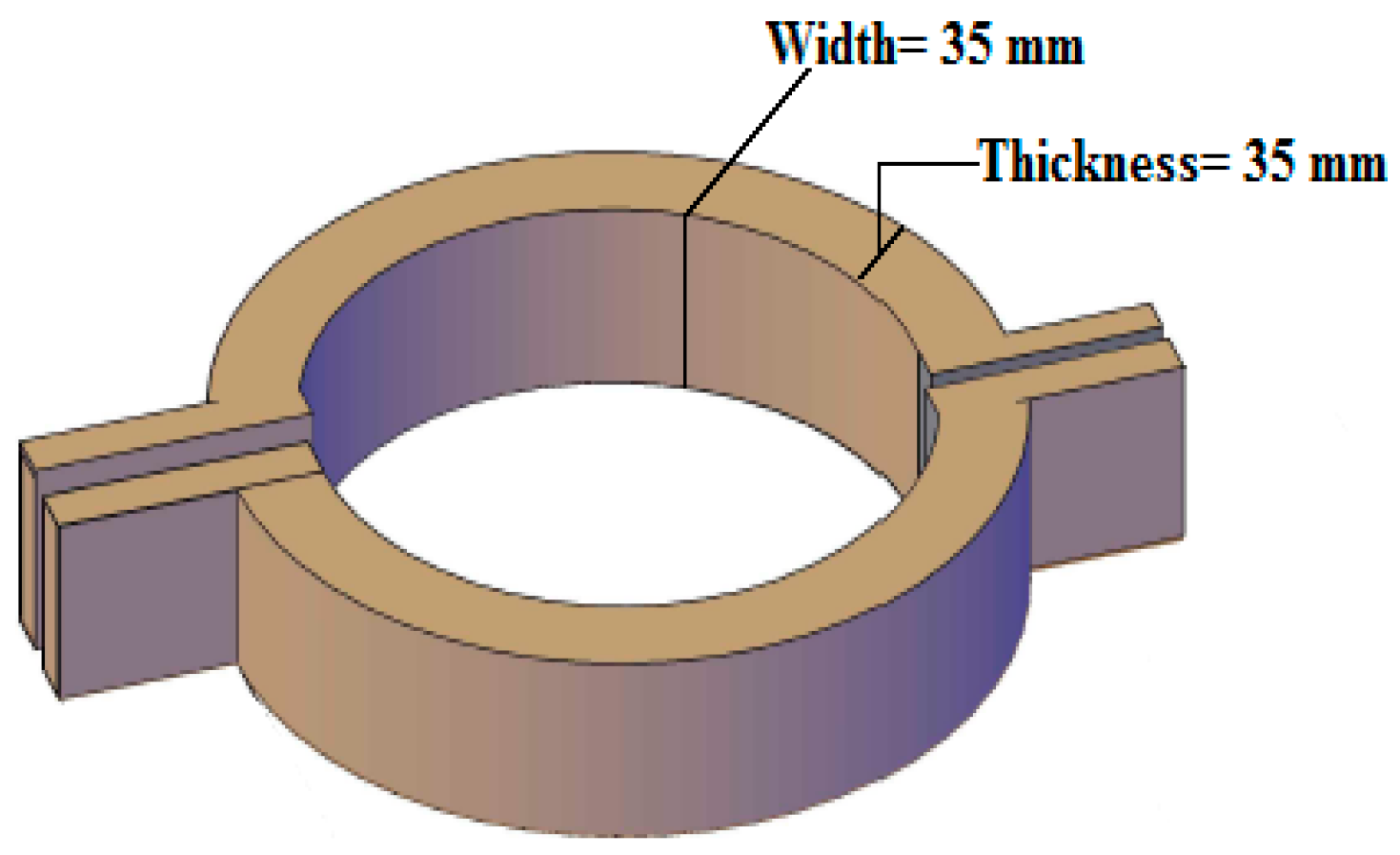
3.1.3. Modeling and Meshing of Circular Rings
3.1.4. Boundary Conditions and Applied Load
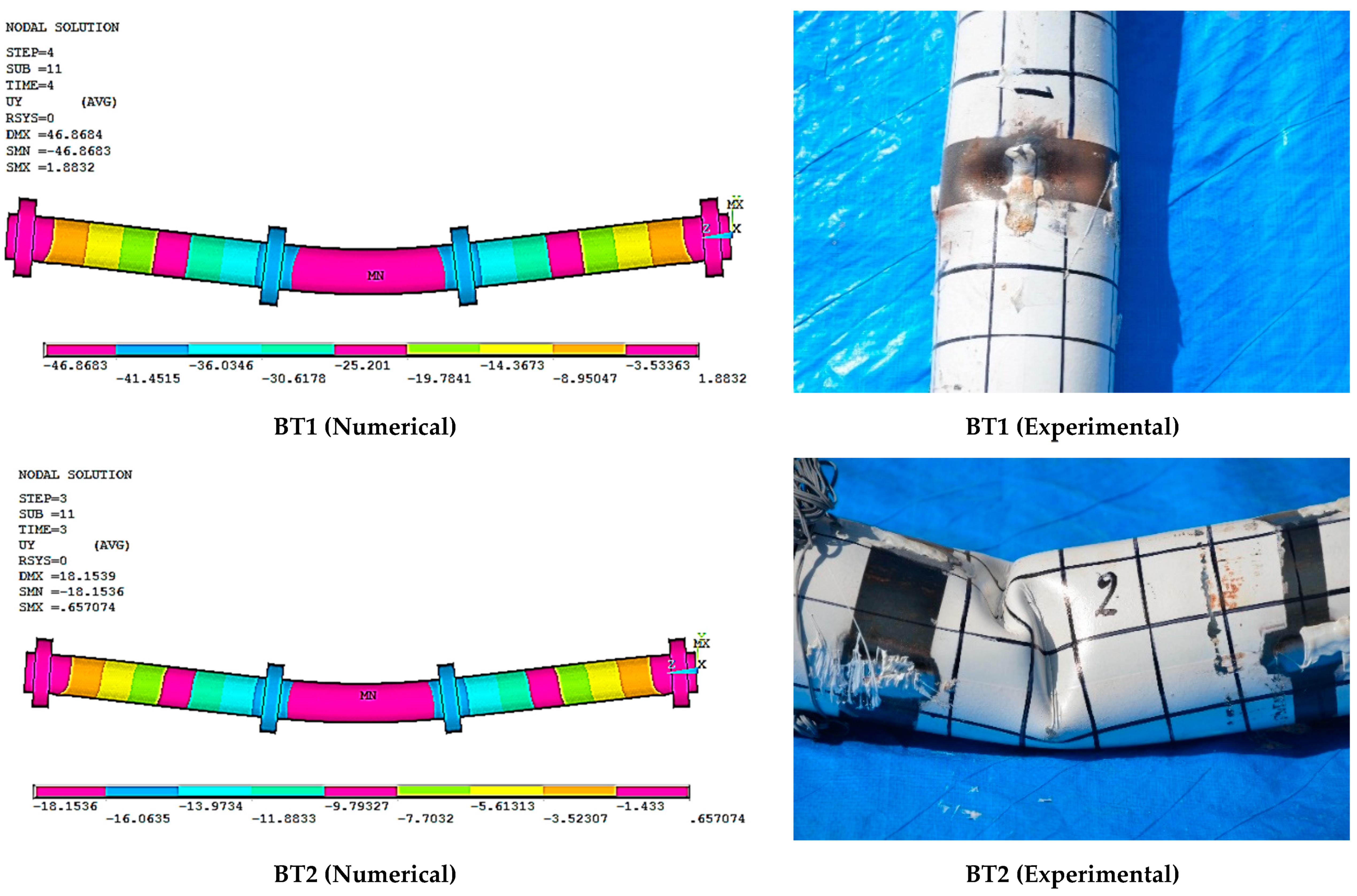
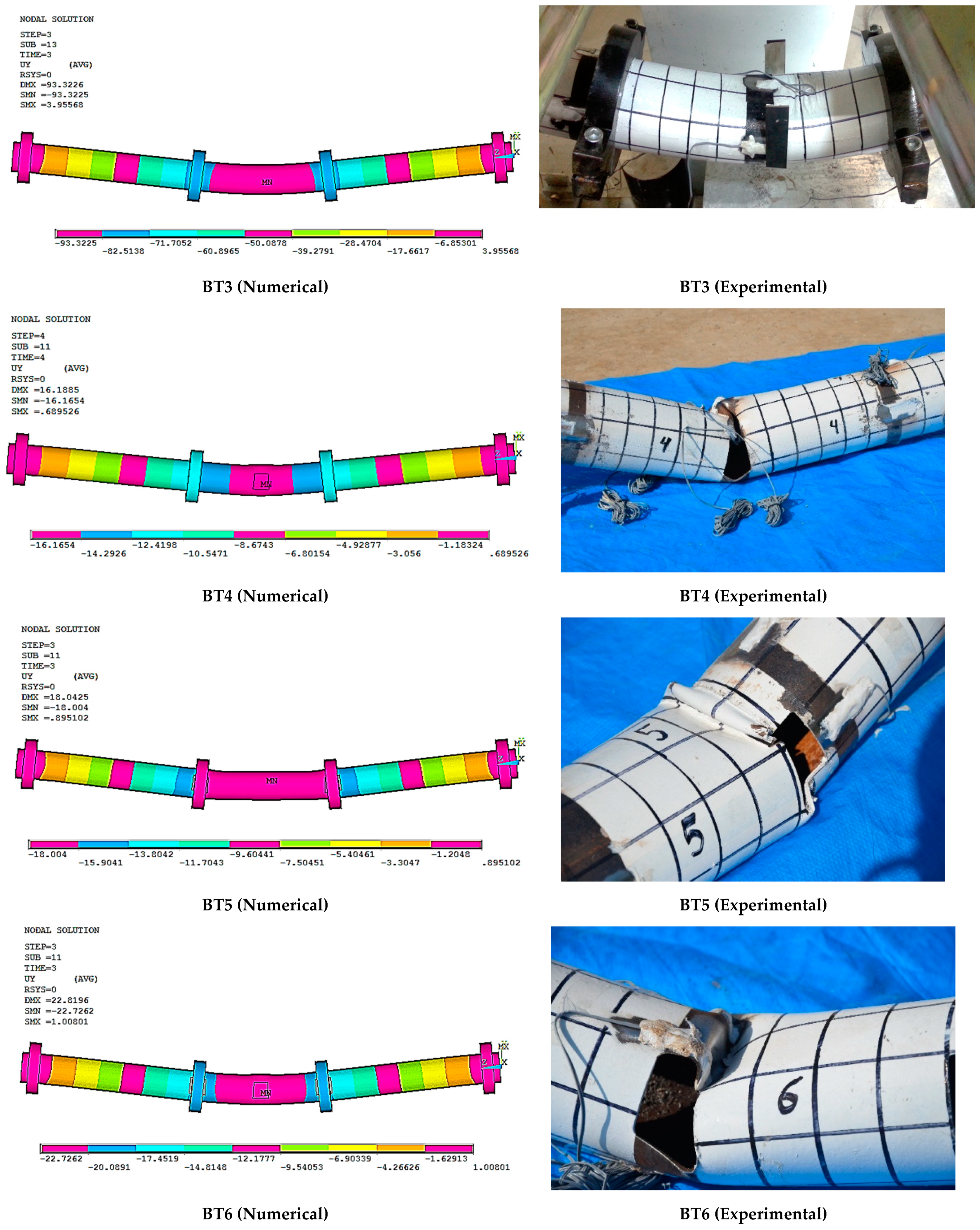
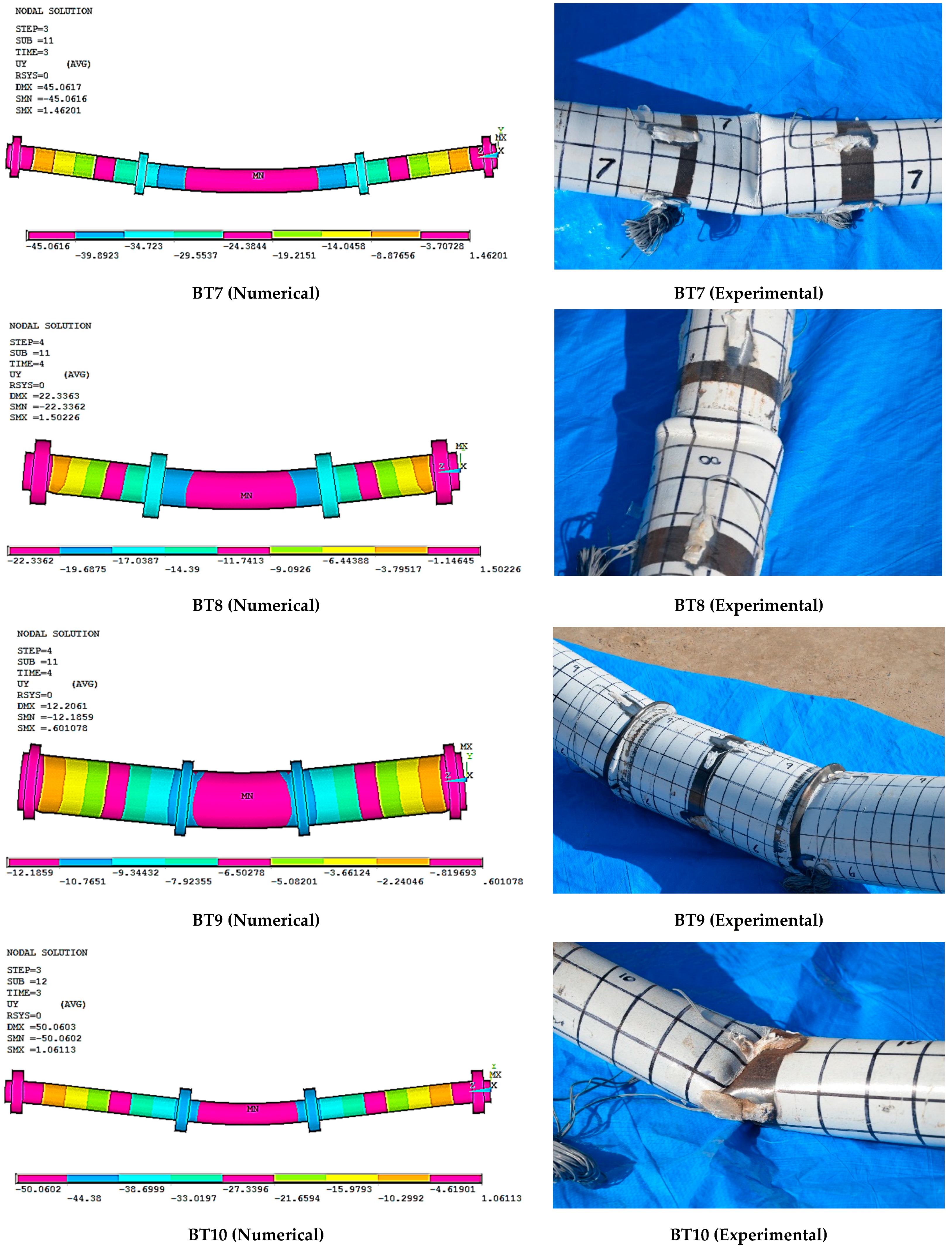
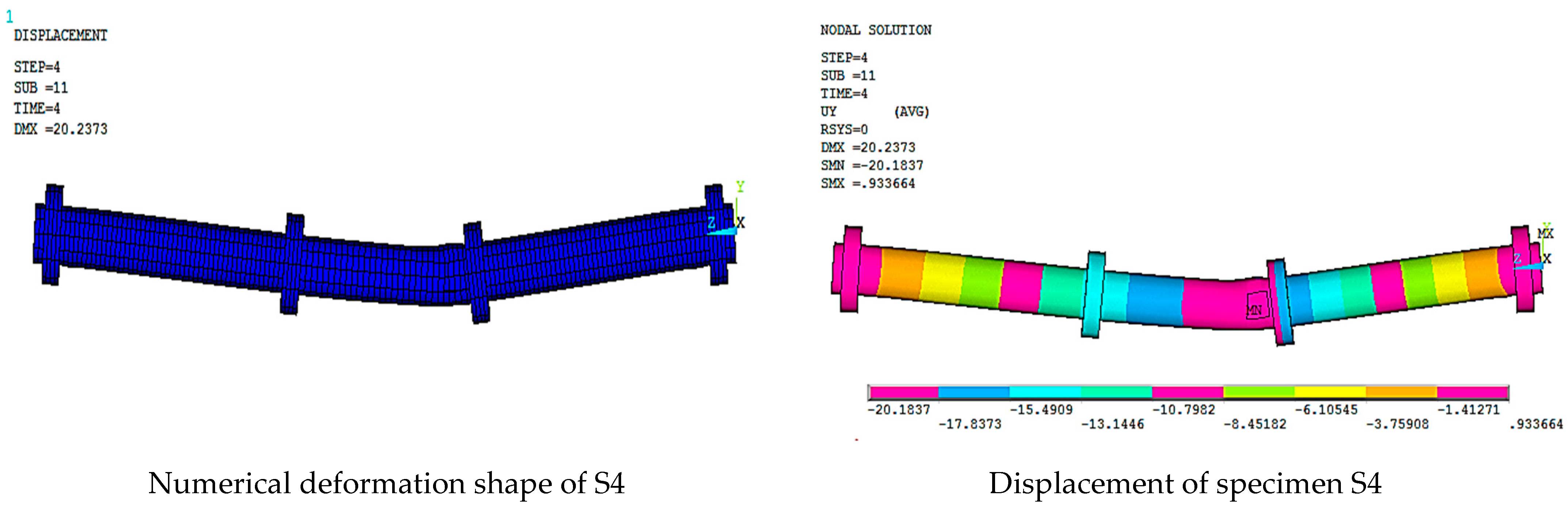
4. Comparison between the Experimental and Numerical Results
5. Parametric Case Studies
- First group
- Second group
6. Results and Discussion
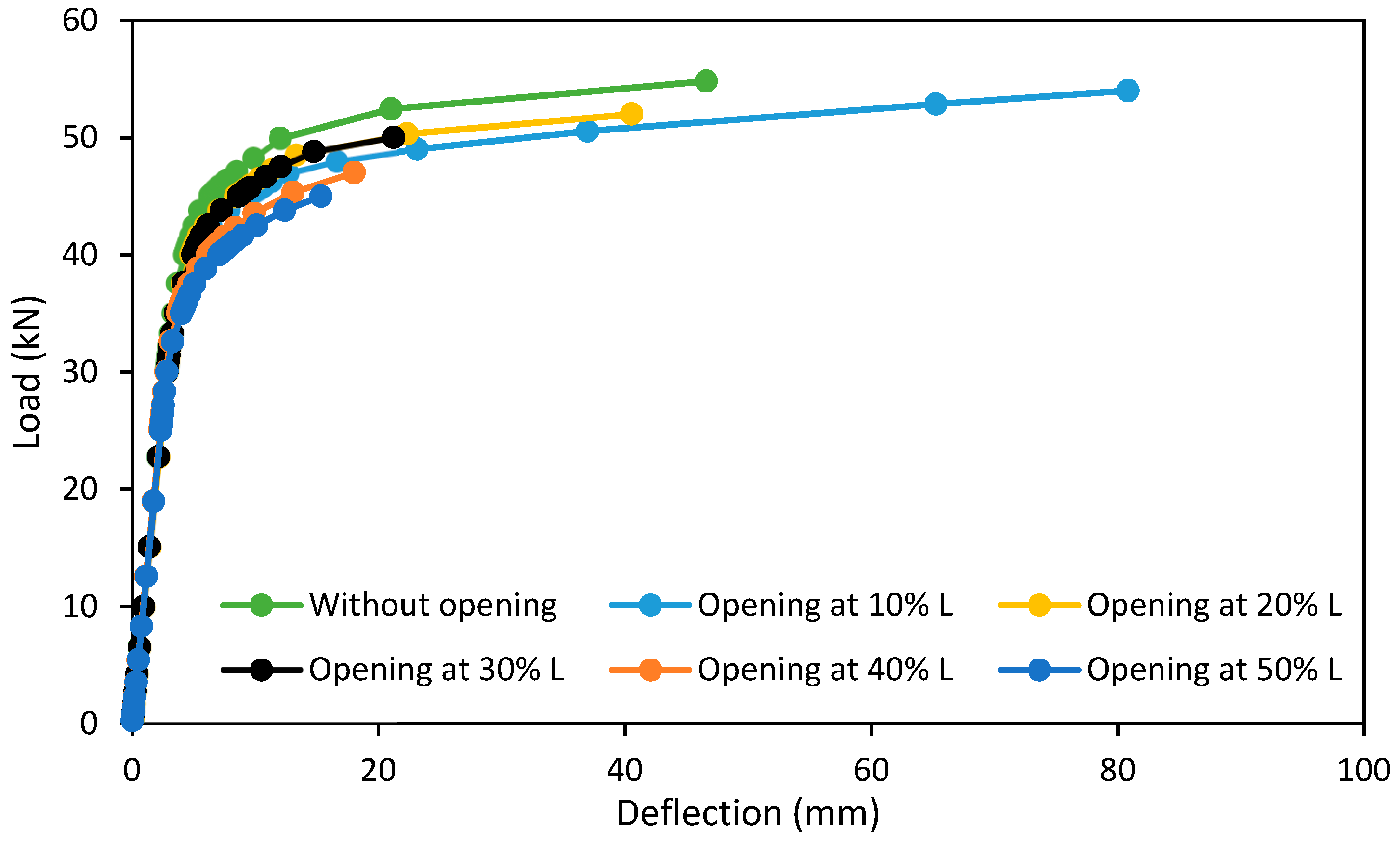
6.1. First Group
- The linear elastic stage: in this stage, the specimen exhibited a linear relationship between the vertical load and the resulting deflection. This stage is represented by an inclined line with a high inclination as a result of a high increase in the applied loads compared with a little increase in the specimen deflection.
- The ovalization stage: this stage is represented by a flat line with a small slope as a result of a high increase in the specimen deflection compared with a little increase in the specimen loads.
- The failure stage: this stage begins at ultimate load and refers to the specimen’s structural collapse.
6.2. Second Group
7. Conclusions
- The load-deflection results of this analysis showed a good agreement with the experimental results.
- The change of opening location in the specimens did not affect the general behavior of these specimens but affected the specimens’ strength capacity and their deflection values, and this led to different locations of failure.
- The presence of an opening in the critical region (at the loading points and pure bending region) reduced the structural strength capacity and the ultimate deflection of the specimens, which led to reduced resistance to collapse.
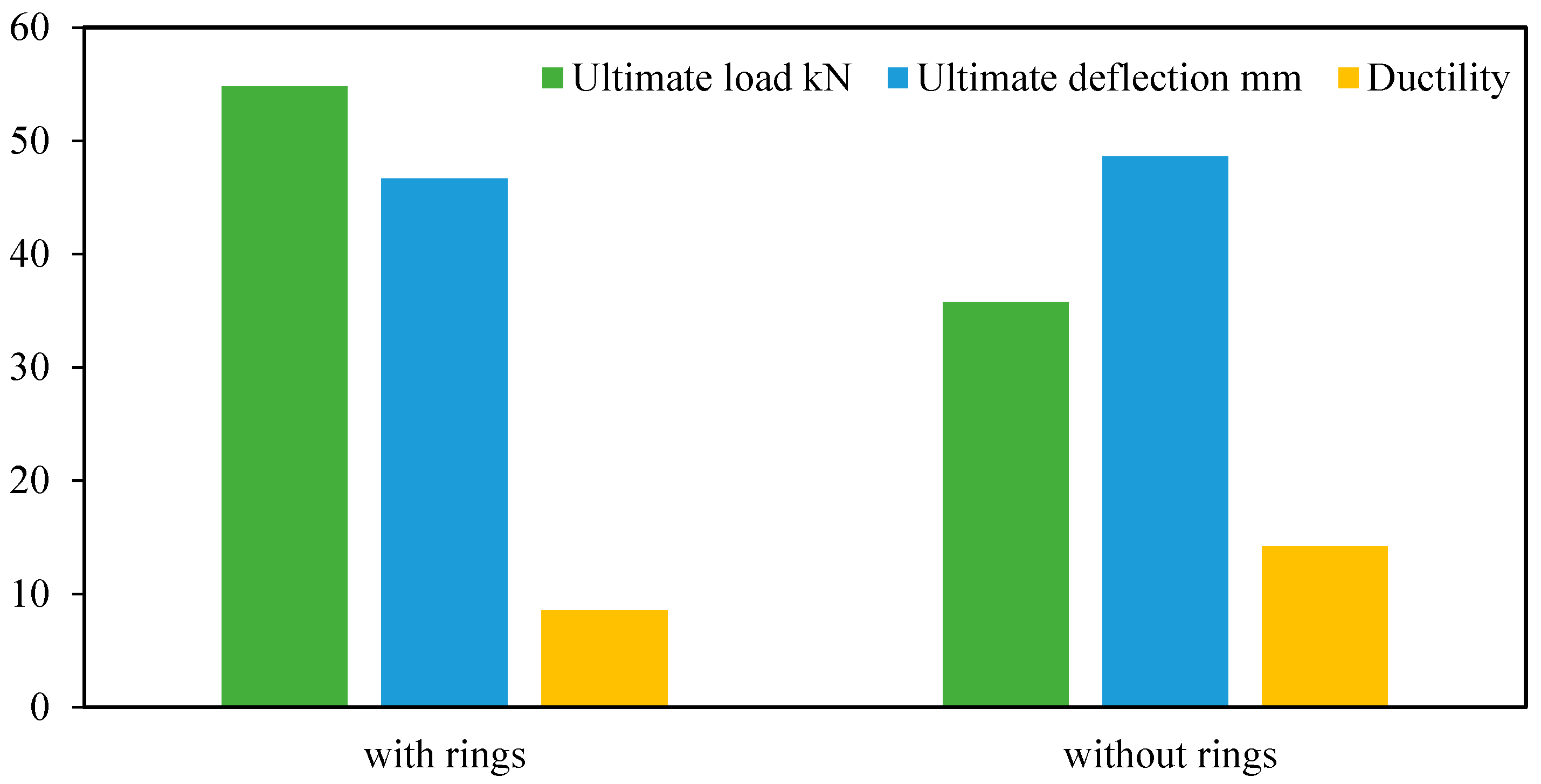
- The presence of rings at loading points enhanced and restricted the loading points and reduced their effect on applied loads, thus preventing the local buckling failure at these points; at the same time, these rings increased the specimens’ load capacity.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Key, P.W.; Hancock, G.J. A Theoretical Investigation of the Column Behaviour of Cold-Formed Square Hollow Sections. Thin-Walled Struct. 1993, 16, 31–64. [Google Scholar] [CrossRef]
- Annan, C.D.; Youssef, M.A.; el Naggar, M.H.; Chris, I.; Construction, M.; Fathieh, A.; Garrison, J.; Tweedie, A.; Hand, D.; Holding, S.; et al. Identification of Structural Issues in Design and Construction of Multi- Story Modular Buildings Identification of Different Structural Type of Multi-Story Modular Building (Msmb). Kullman Build. Corp. Garrison Archit. 2008, 67, 294–303. [Google Scholar]
- Elchalakani, M.; Zhao, X.-L.; Grzebieta, R. Tests of Cold-Formed Circular Tubular Braces under Cyclic Axial Loading. J. Struct. Eng. 2003, 129, 507–514. [Google Scholar] [CrossRef]
- Nuñez, E.; Boainy, N.; González, F.; Torres, R.; Picón, R.; Guerrero, N. Cyclic Behavior of Hollow Section Beam–Column Moment Connection: Experimental and Numerical Study. Metals 2020, 10, 1608. [Google Scholar] [CrossRef]
- ASTM. A500 Standard Specification for Cold-Formed Welded and Seamless Carbon Steel Structural Tubing in Rounds and Shapes 1. ASTM Int. 2021, 1, 6. [Google Scholar]
- Lu, L.H.; Wardenier, J. Static Strength of Uniplanar and Multiplanar Connections between I-Beams and RHS Columns Loaded by Axial Compression. In Proceedings of the International Conference on Offshore Mechanics and Arctic Engineering—OMAE, New York, NY, USA, 31 December 1995; Volume 3. [Google Scholar]
- Lu, L.H.; Wardenier, J. Ultimate Strength of I-Beam to RHS Column Connections. J. Constr. Steel Res. 1998, 46, 250–251. [Google Scholar] [CrossRef]
- Hajjar, J.F. Concrete-Filled Steel Tube Columns under Earthquake Loads. Prog. Struct. Eng. Mater. 2000, 2, 72–81. [Google Scholar] [CrossRef]
- Nakashima, M.; Liu, D. Instability and Complete Failure of Steel Columns Subjected to Cyclic Loading. J. Eng. Mech. 2005, 131, 559–567. [Google Scholar] [CrossRef]
- Fadden, M.; McCormick, J. Finite Element Model of the Cyclic Bending Behavior of Hollow Structural Sections. J. Constr. Steel Res. 2014, 94, 64–75. [Google Scholar] [CrossRef]
- Packer, J. Tubular Construction. Prog. Struct. Eng. Mater. 2000, 2, 41–49. [Google Scholar] [CrossRef]
- Wang, T.; McCormick, J.; Yoshitake, N.; Pan, P.; Murata, Y.; Nakashiman, M. Collapse Simulation of a Four-Story Steel Moment Frame by a Distributed Online Hybrid Test. Earthq. Eng. Struct. Dyn. 2008, 37, 955–974. [Google Scholar] [CrossRef]
- Hancock, G.J.; Zhao, X.L. Research into the Strength of Cold-Formed Tubular Sections. J. Constr. Steel Res. 1992, 23, 55–72. [Google Scholar] [CrossRef]
- Hasan, S.W.; Hancock, G.J. Plastic Bending Tests of Cold-Formed Rectangular Hollow Sections. Steel Constr. 1989, 23, 2–19. [Google Scholar]
- Ibrahim, A.M.; Khalaf, M.S. Elastic-Plastic Behavior of Circular Hollow Section Steel Tubes Subjected to Bending. J. Eng. Sci. Technol. Rev. 2018, 11, 42–49. [Google Scholar] [CrossRef]
- Yao, Y.; Quach, W.M.; Young, B. Finite Element-Based Method for Residual Stresses and Plastic Strains in Cold-Formed Steel Hollow Sections. Eng. Struct. 2019, 188, 24–42. [Google Scholar] [CrossRef]
- Korol, R.M.; Hudoba, J. Plastic Behavior of Hollow Structural Sections. Asce J. Struct. Div. 1972, 98, 1007–1023. [Google Scholar] [CrossRef]
- Nishiyama, I.; Morino, S. US-Japan Cooperative Earthquake Research Program on CFT Structures: Achievements on the Japanese Side. Prog. Struct. Eng. Mater. 2004, 6, 39–55. [Google Scholar] [CrossRef]
- Dwyer, T.J.; Galambos, T.V. Plastic Behavior of Tubular Beams Columns. J. Struct. Div. 1965, 91, 153–168. [Google Scholar] [CrossRef]
- Fadden, M.; McCormick, J. Parametric Finite Element Analysis of the Cyclic Flexural Behavior of Hollow Structural Sections. In Proceedings of the Tubular Structures XIV—Proceedings of the 14th International Symposium on Tubular Structures, ISTS 2012, London, UK, 12–14 September 2012. [Google Scholar]
- Fadden, M.; McCormick, J. Effect of Width-Thickness and Depth-Thickness on the Cyclic Flexural Buckling Behavior of Hollow Structural Sections. In Proceedings of the Structural Stability Research Council Annual Stability Conference 2012, Grapevine, TX, USA, 18–21 April 2012. [Google Scholar]
- Hu, Y.F.; Chung, K.F.; Ban, H.; Nethercot, D.A. Structural Testing and Numerical Modelling of T-Joints between Cold-Formed S690 Circular Hollow Sections under Brace in-Plane Bending. Eng. Struct. 2022, 250, 113317. [Google Scholar] [CrossRef]
- Gallegos, M.; Nuñez, E.; Herrera, R. Numerical Study on Cyclic Response of End-Plate Biaxial Moment Connection in Box Columns. Metals 2020, 10, 523. [Google Scholar] [CrossRef]
- Hassan, A.; Arif, M.; Shariq, M.; Alomayri, T. Experimental Test and Finite Element Modelling Prediction on Geopolymer Concrete Beams Subject to Flexural Loading. Innov. Infrastruct. Solut. 2022, 7, 13. [Google Scholar] [CrossRef]
- Uenaka, K. CFDST Stub Columns Having Outer Circular and Inner Square Sections under Compression. J. Constr. Steel Res. 2016, 120, 1–7. [Google Scholar] [CrossRef]
- Yoshitake, N.; Wang, T.; McCormick, J.; Murata, Y.; Nakashima, M. Collapse Simulation of Steel Moment Frame by Distributed Online Hybrid Test with Multiple Tests. J. Struct. Constr. Eng. 2008, 73, 441–447. [Google Scholar] [CrossRef][Green Version]
- Sohal, I.S.; Chen, W.F. Local Buckling and Inelastic Cyclic Behavior of Tubular Sections. Thin-Walled Struct. 1988, 6, 63–80. [Google Scholar] [CrossRef]
- Key, P.W.; Hasan, S.W.; Hancock, G.J. Column Behavior of Cold-Formed Hollow Sections. J. Struct. Eng. 1988, 114, 390–407. [Google Scholar] [CrossRef]
- Goto, Y.; Wang, Q.; Obata, M. FEM Analysis for Hysteretic Behavior of Thin-Walled Columns. J. Struct. Eng. 1998, 124, 1290–1301. [Google Scholar] [CrossRef]
- Kurata, M.; Nakashima, M.; Suita, K. Effect of Column Base Behaviour on the Seismic Response of Steel Moment Frames. J. Earthq. Eng. 2005, 9, 415–438. [Google Scholar] [CrossRef]
- Denavit, M.D.; Hajjar, J.F.; Perea, T.; Leon, R.T. Cyclic Evolution of Damage and Beam-Column Interaction Strength of Concrete-Filled Steel Tube Beam-Columns. In Proceedings of the 9th US National and 10th Canadian Conference on Earthquake Engineering 2010, Including Papers from the 4th International Tsunami Symposium, Toronto, ON, Canada, 25–29 July 2010; Volume 5. [Google Scholar]
- Goto, Y.; Kumar, G.P.; Kawanishi, N. Nonlinear Finite-Element Analysis for Hysteretic Behavior of Thin-Walled Circular Steel Columns with In-Filled Concrete. J. Struct. Eng. 2010, 136, 1413–1422. [Google Scholar] [CrossRef]
- Kurobane, Y. Connections in Tubular Structures. Prog. Struct. Eng. Mater. 2002, 4, 35–45. [Google Scholar] [CrossRef]
- Earls, C.J. Geometric Factors Influencing Structural Ductility in Compact I-Shaped Beams. J. Struct. Eng. 2000, 126, 780–789. [Google Scholar] [CrossRef]
- Wilkinson, T.; Hancock, G. Predicting the Rotation Capacity of Cold-Formed RHS Beams Using Finite Element Analysis. J. Constr. Steel Res. 2002, 58, 1455–1471. [Google Scholar] [CrossRef]
- Zhou, W.; Shao, Z.; Yu, J.; Lin, J. Advances and Trends in Forming Curved Extrusion Profiles. Materials 2021, 14, 1603. [Google Scholar] [CrossRef] [PubMed]
- Allouzi, R.A.; Almasaeid, H.H.; Salman, D.G.; Abendeh, R.M.; Rabayah, H.S. Prediction of Bond-Slip Behavior of Circular/Squared Concrete-Filled Steel Tubes. Buildings 2022, 12, 456. [Google Scholar] [CrossRef]
- Jiang, J.; Chen, S. Experimental and Numerical Study of Double-through Plate Connections to CFST Column. J. Constr. Steel Res. 2019, 153, 385–394. [Google Scholar] [CrossRef]
- Tong, L.W.; Zheng, H.Z.; Mashiri, F.R.; Zhao, X.L. Stress-Concentration Factors in Circular Hollow Section and Square Hollow Section T-Connections: Experiments, Finite-Element Analysis, and Formulas. J. Struct. Eng. 2013, 139, 1866–1881. [Google Scholar] [CrossRef]
- ASTM. International ASTM A370 Standard Test Methods and Definitions for Mechanical Testing of Steel Products. ASTM Int. 2014, 1, 50. [Google Scholar] [CrossRef]






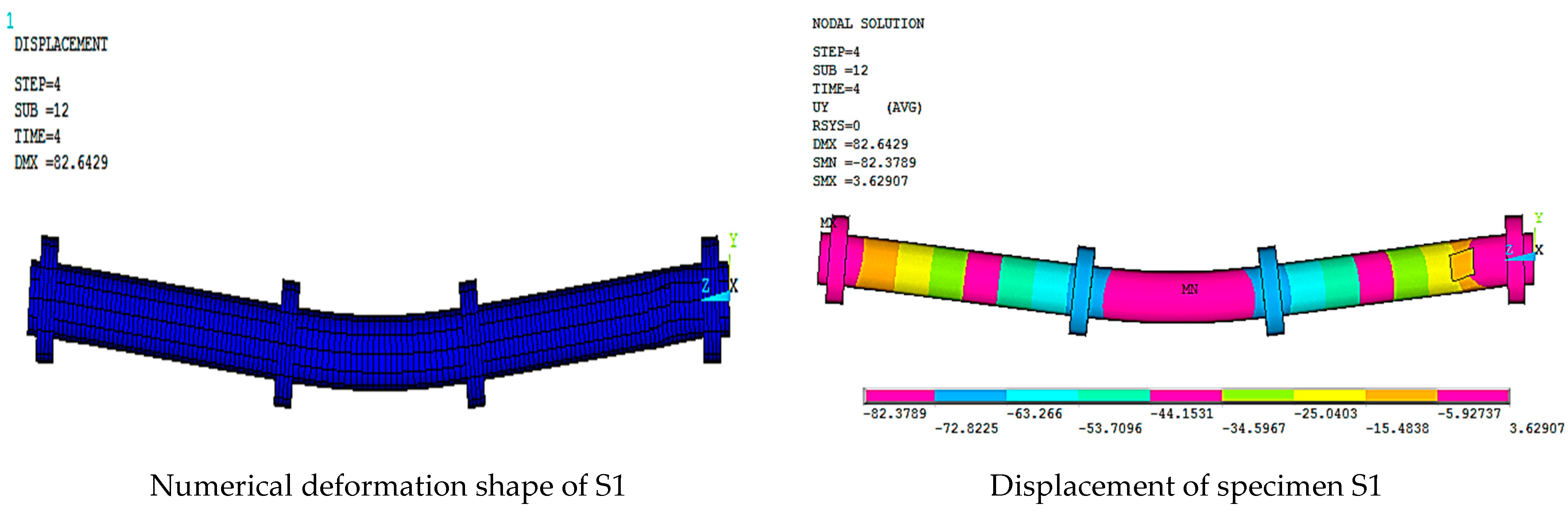
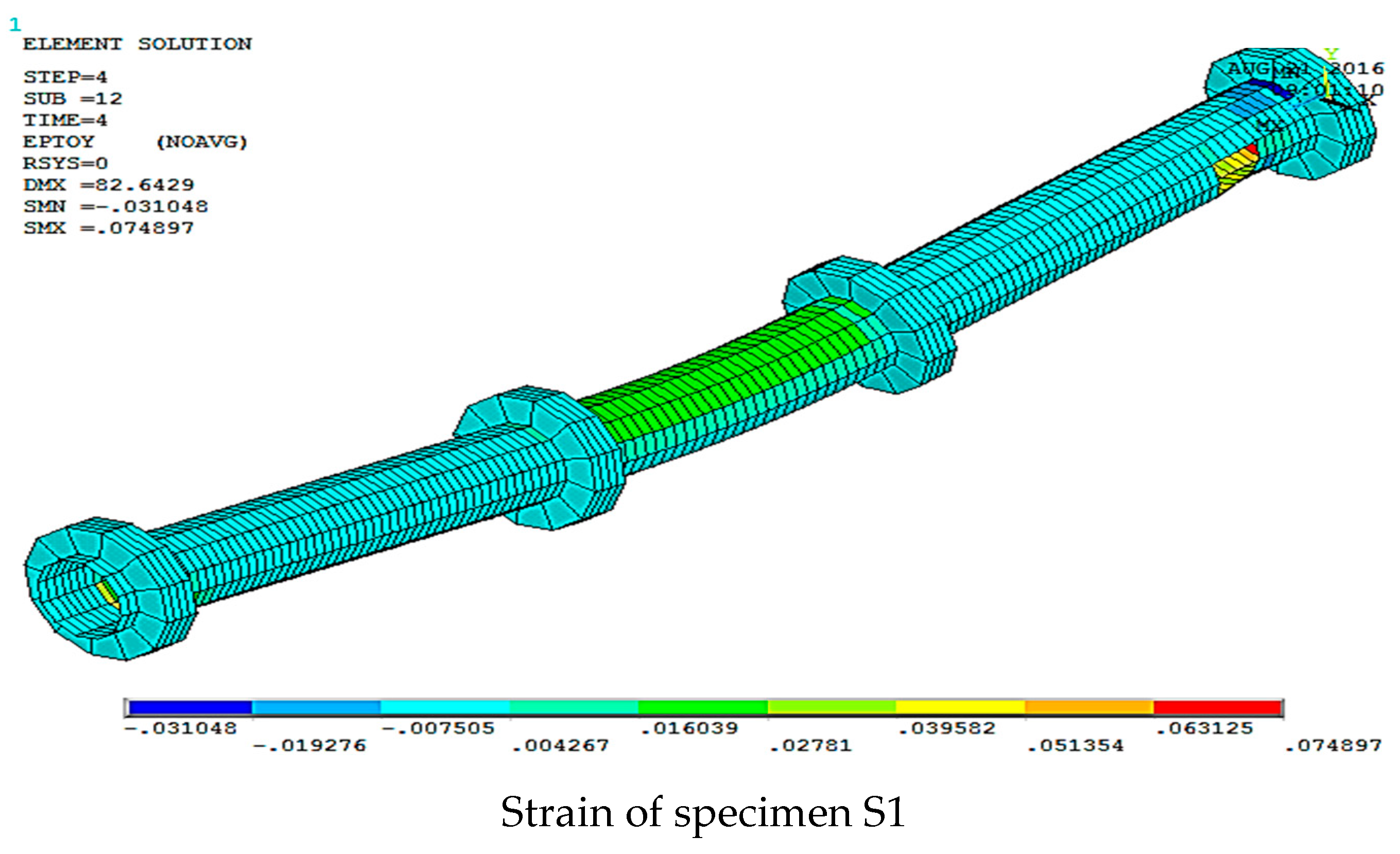
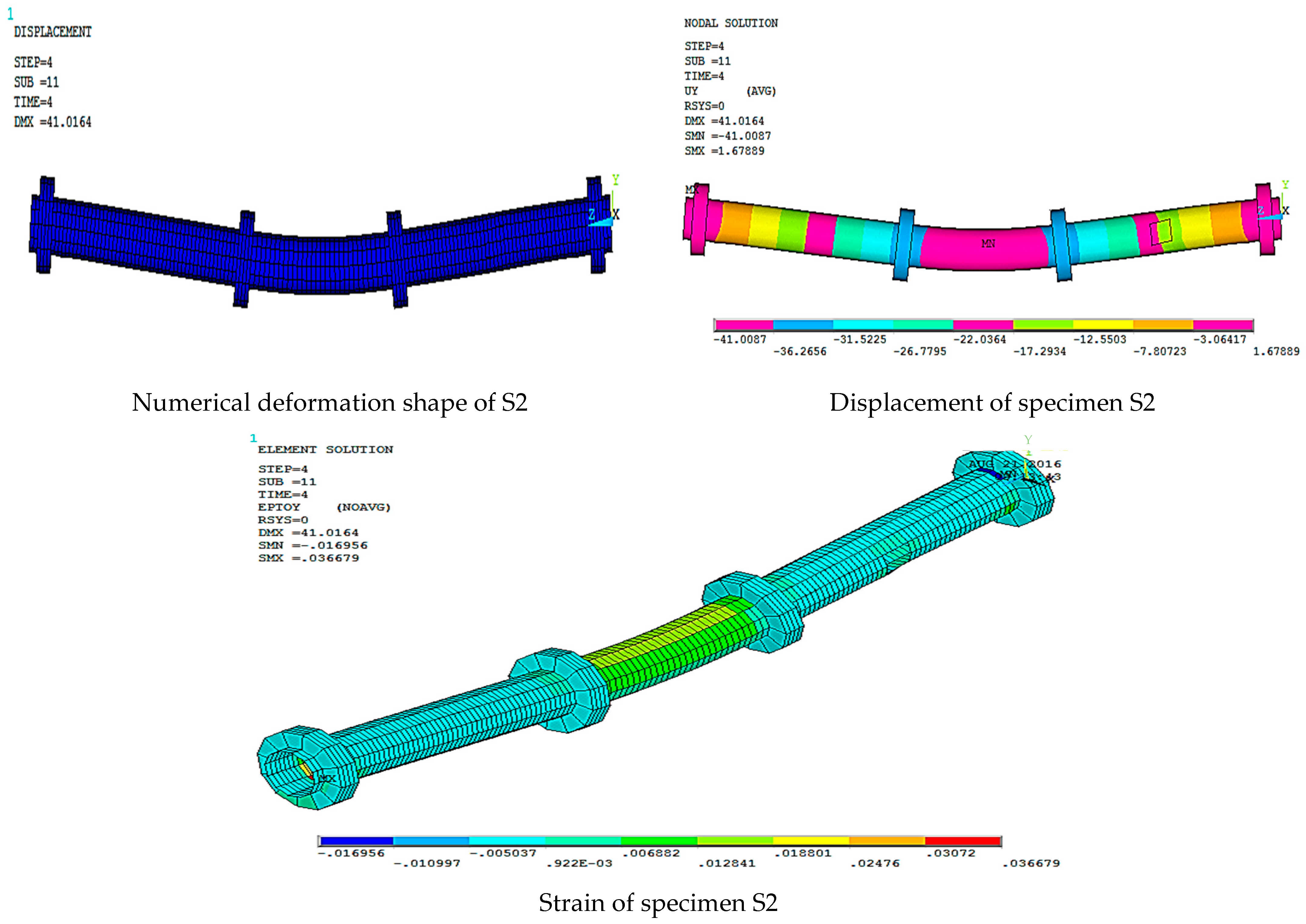
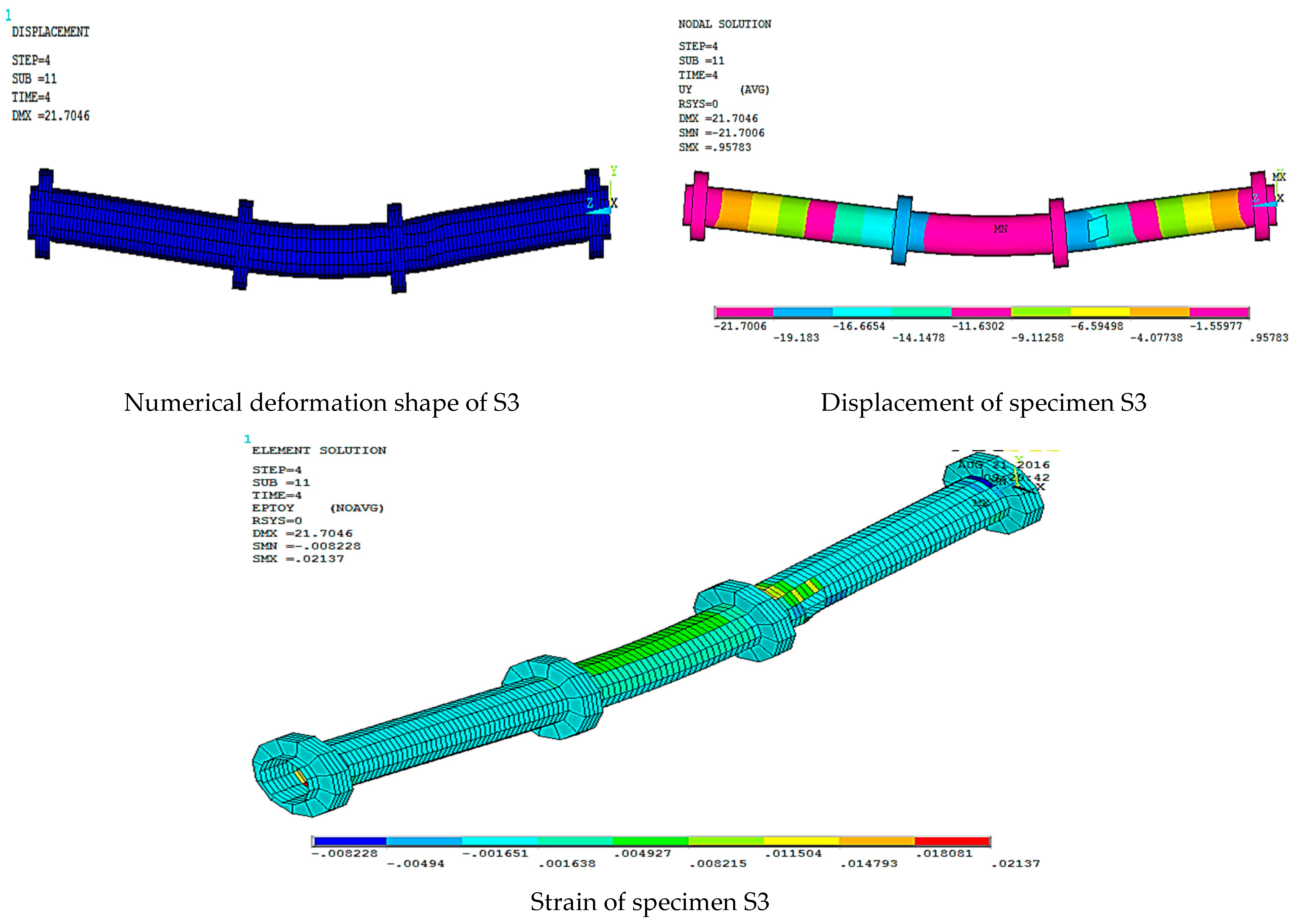



| Label | Thickness t (cm) | Ultimate Stress Fu (MPa) | Yield Stress Fy (MPa) |
|---|---|---|---|
| BT1 | 0.3 | 370 | 290 |
| BT2 | 0.2 | 430 | 360 |
| BT3 | 0.6 | 440 | 285 |
| BT4 | 0.3 | 370 | 290 |
| BT5 | 0.3 | 370 | 290 |
| BT6 | 0.3 | 370 | 290 |
| BT7 | 0.3 | 370 | 290 |
| BT8 | 0.3 | 370 | 290 |
| BT9 | 0.3 | 370 | 290 |
| BT10 | 0.3 | 370 | 290 |
| Label | Diameter D (mm) | Span L (mm) | Thickness t (mm) | D/t |
|---|---|---|---|---|
| BT1 | 101.6 | 1500 | 3 | 33.87 |
| BT2 | 101.6 | 1500 | 2 | 50.80 |
| BT3 | 101.6 | 1500 | 6 | 16.93 |
| BT4 | 101.6 | 1500 | 3 | 33.87 |
| BT5 | 101.6 | 1500 | 3 | 33.87 |
| BT6 | 101.6 | 1500 | 3 | 33.87 |
| BT7 | 101.6 | 2000 | 3 | 33.87 |
| BT8 | 101.6 | 1000 | 3 | 33.87 |
| BT9 | 219.0 | 1500 | 3 | 73.00 |
| BT10 | 76.20 | 1500 | 3 | 25.40 |
| Label | Exp. Deflection at Ultimate Load (mm) | FEM Deflection at Ultimate Load (Pu) (mm) | Deflection FEM/Exp. (%) | Absolute Percentage Error |
|---|---|---|---|---|
| BT1 | 51.88 | 46. 86 | 90.33 | 9.67 |
| BT2 | 22.10 | 18.15 | 82.13 | 17.8733 |
| BT3 | 108.06 | 93.32 | 86.36 | 13.64057 |
| BT4 | 17.57 | 16.16 | 91.97 | 8.025043 |
| BT5 | 20.48 | 18.00 | 87.89 | 12.10938 |
| BT6 | 24.35 | 22.72 | 93.31 | 6.694045 |
| BT7 | 55.31 | 45.06 | 81.47 | 18.53191 |
| BT8 | 25.80 | 22.33 | 86.55 | 13.44961 |
| BT9 | 14.15 | 12.18 | 86.08 | 13.92226 |
| BT10 | 58.25 | 50.06 | 85.94 | 14.06009 |
| Label | t (mm) | D (mm) | L (mm) | Primary Variable |
|---|---|---|---|---|
| BT1 | 3 | 101.6 | 1500 | Without opening |
| S1 | 3 | 101.6 | 1500 | Opening at 10% L |
| S2 | 3 | 101.6 | 1500 | Opening at 20% L |
| S3 | 3 | 101.6 | 1500 | Opening at 30% L |
| S4 | 3 | 101.6 | 1500 | Opening at 40% L |
| S5 | 3 | 101.6 | 1500 | Opening at 50% L |
| Label | t (mm) | D (mm) | L (mm) | Primary Variable |
|---|---|---|---|---|
| BT1 | 3 | 101.6 | 1500 | With rings |
| S6 | 3 | 101.6 | 1500 | Without rings |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khalaf, M.S.; Ibrahim, A.M.; Najm, H.M.; Hassan, A.; Sabri, M.M.S.; Alamir, M.A.; Alarifi, I.M. Evaluation and Prediction of the Bending Behavior of Circular Hollow Steel Tube Sections Using Finite Element Analysis. Materials 2022, 15, 3919. https://doi.org/10.3390/ma15113919
Khalaf MS, Ibrahim AM, Najm HM, Hassan A, Sabri MMS, Alamir MA, Alarifi IM. Evaluation and Prediction of the Bending Behavior of Circular Hollow Steel Tube Sections Using Finite Element Analysis. Materials. 2022; 15(11):3919. https://doi.org/10.3390/ma15113919
Chicago/Turabian StyleKhalaf, Manahel Shahath, Amer M. Ibrahim, Hadee Mohammed Najm, Amer Hassan, Mohanad Muayad Sabri Sabri, Mohammed A. Alamir, and Ibrahim M. Alarifi. 2022. "Evaluation and Prediction of the Bending Behavior of Circular Hollow Steel Tube Sections Using Finite Element Analysis" Materials 15, no. 11: 3919. https://doi.org/10.3390/ma15113919
APA StyleKhalaf, M. S., Ibrahim, A. M., Najm, H. M., Hassan, A., Sabri, M. M. S., Alamir, M. A., & Alarifi, I. M. (2022). Evaluation and Prediction of the Bending Behavior of Circular Hollow Steel Tube Sections Using Finite Element Analysis. Materials, 15(11), 3919. https://doi.org/10.3390/ma15113919









