Atomic Interactions and Order–Disorder Transition in FCC-Type FeCoNiAl1−xTix High-Entropy Alloys
Abstract
:1. Introduction
2. Computational Method
2.1. Atomic Interactions
- (i)
- In the binary system of Ni–X, the X–X solute pair interaction at the pth coordination shell is calculated by [27]:where is the total energy of a supercell with two X atoms at the pth coordination shell, is the total energy of a supercell with one X atom, and is the total energy with no impurity;
- (ii)
- In the ternary system of Ni–X–Y, the X–Y solute pair interaction in the pth coordination shell is calculated by:where is the total energy of a supercell with two Y atoms at the pth coordination shell, and is the total energy of the supercell with one X atom and one Y atom at the pth coordination shell. In this work, a negative interaction energy indicates the attraction between solutes.
2.2. Thermodynamic Monte Carlo Method
2.3. Formation Enthalpy of L12-A3B Precipitate
2.4. First-Principles Method
2.5. CALPHAD Method
3. Results and Discussion
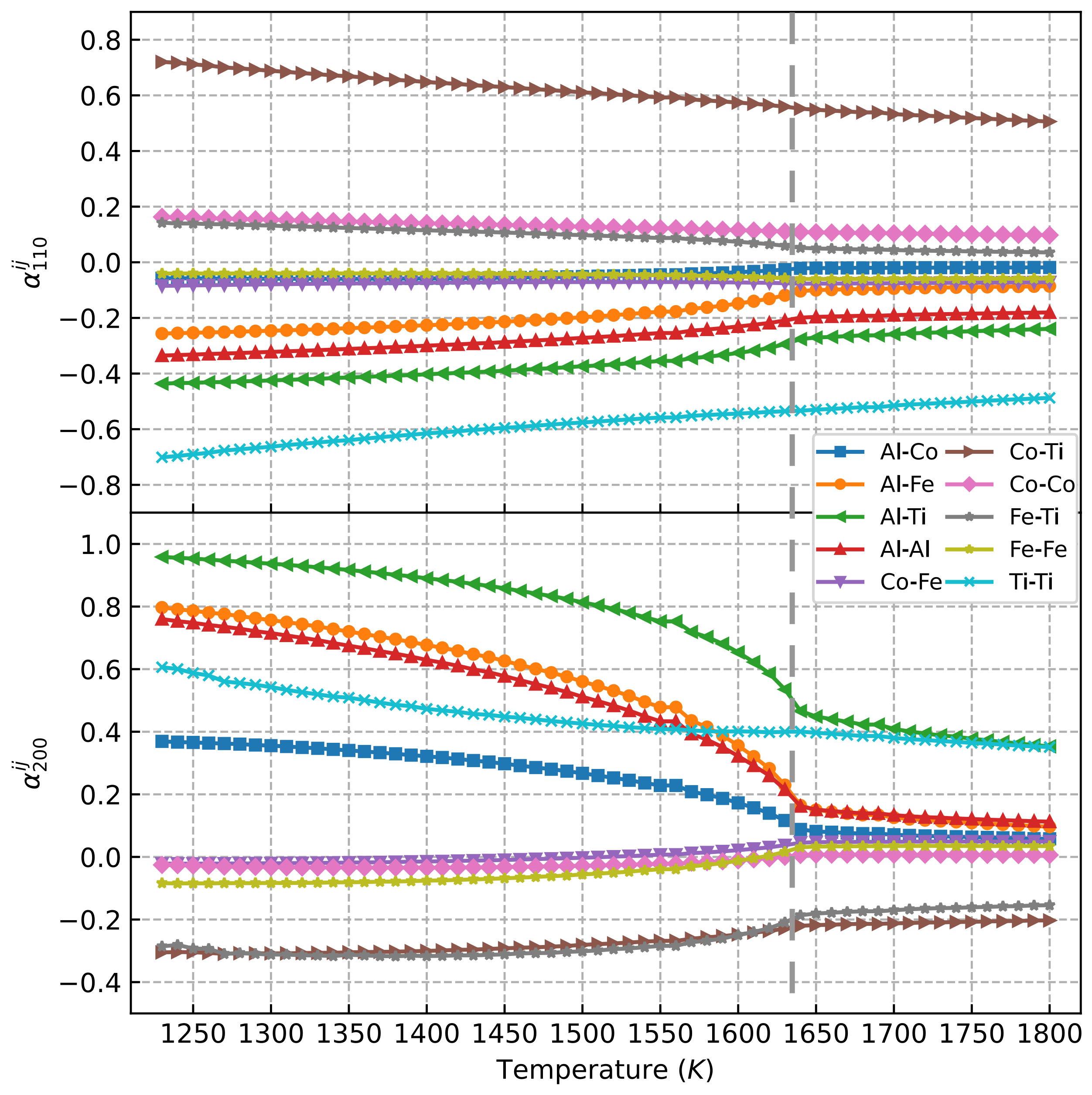
3.1. Atomic Interactions of Solute Pairs in FeCoNiAl1−xTix HEAs
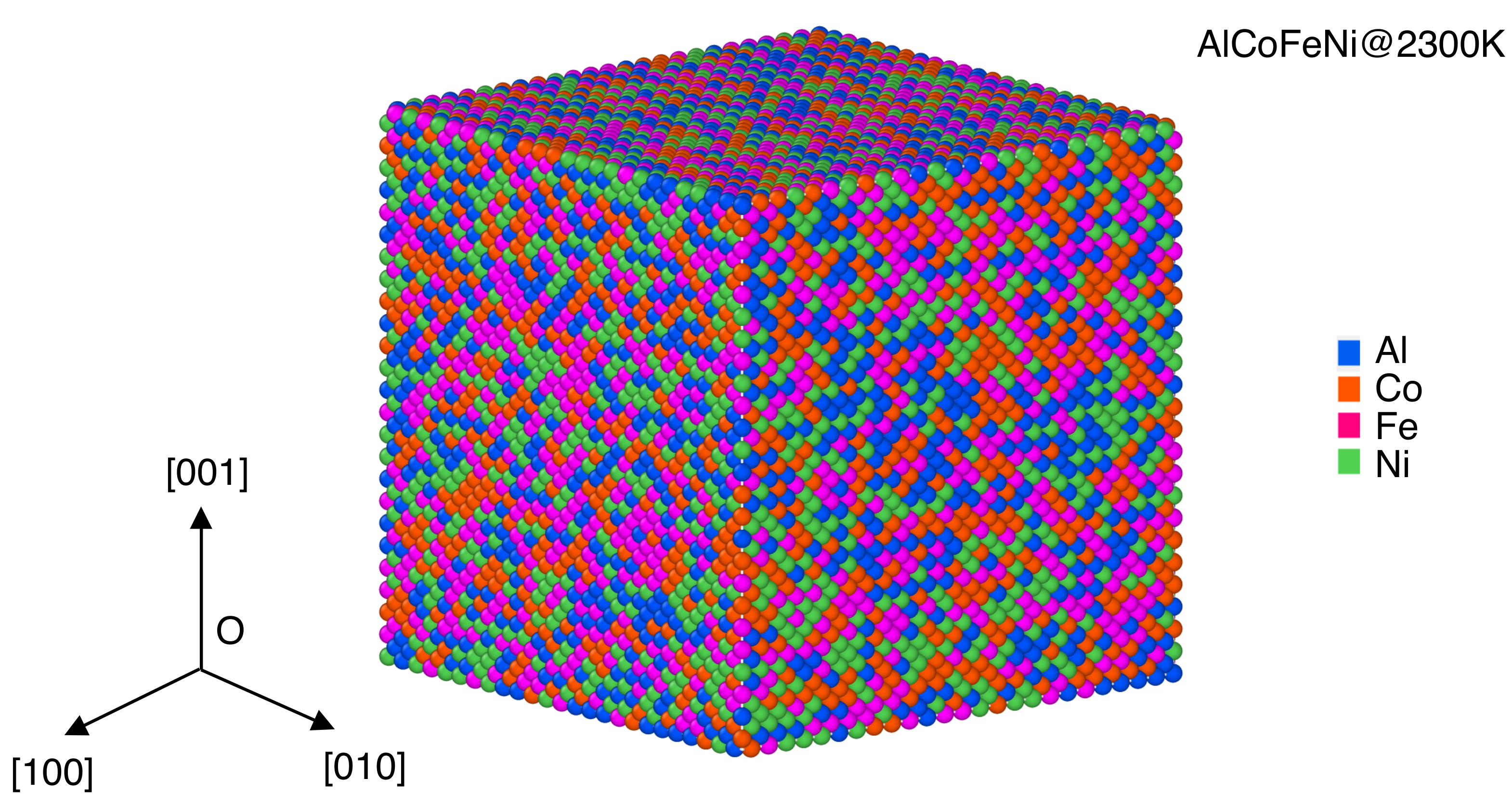
3.2. The Order–Disorder Transition in Equal-Atomic AlCoFeNi HEAs
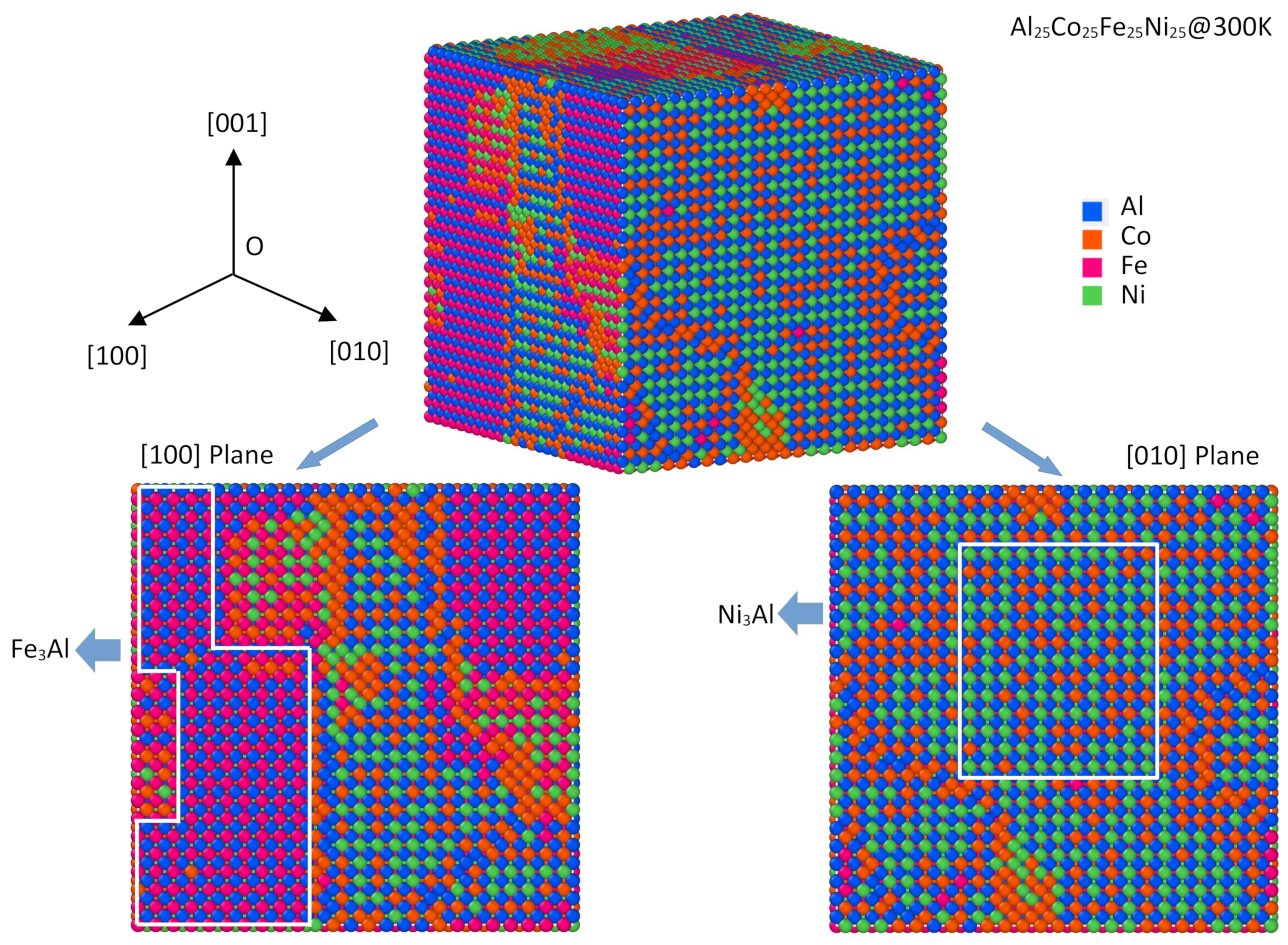
3.3. The Microstructure of AlCoFeNi at Elevated and Ambient Temperatures
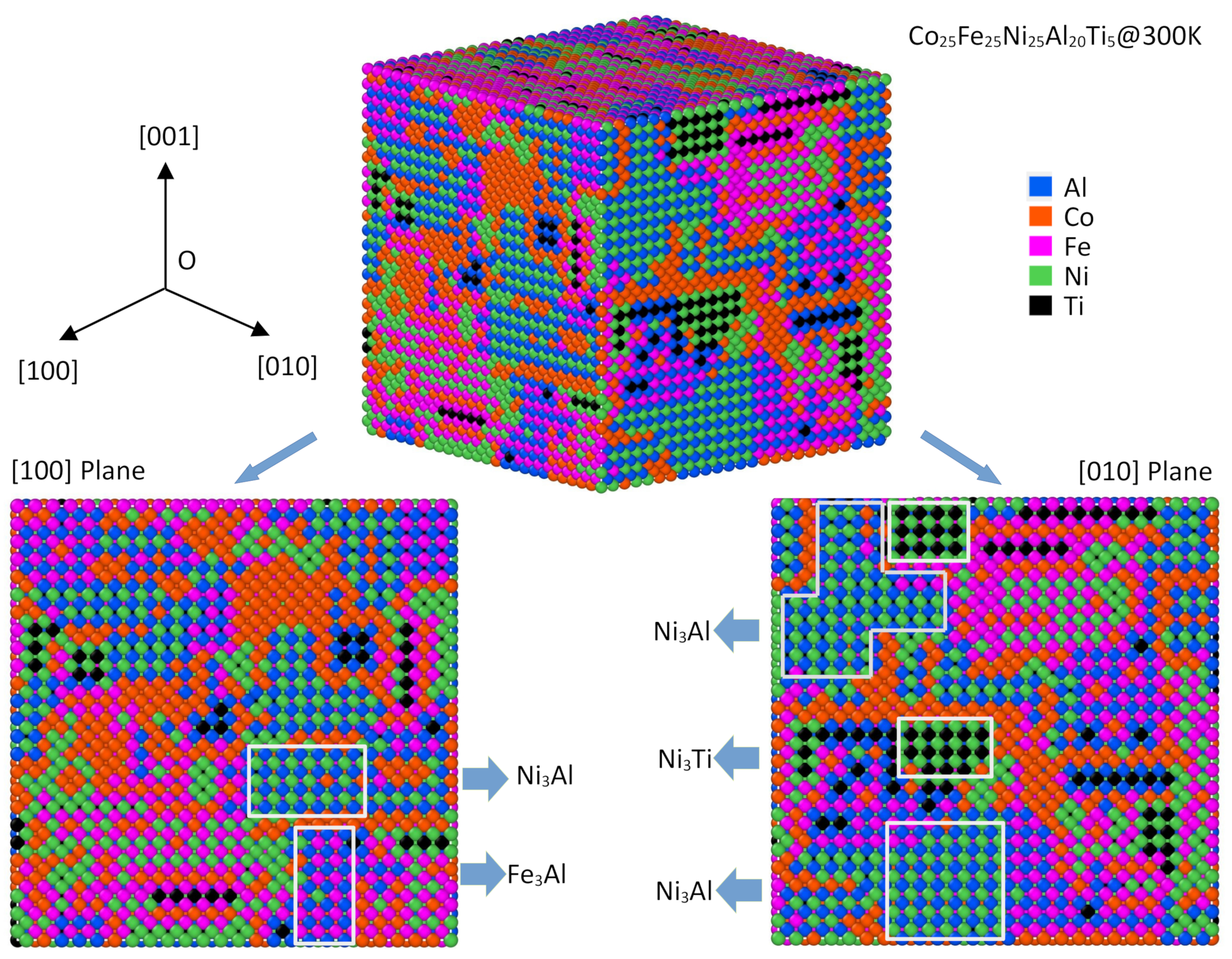
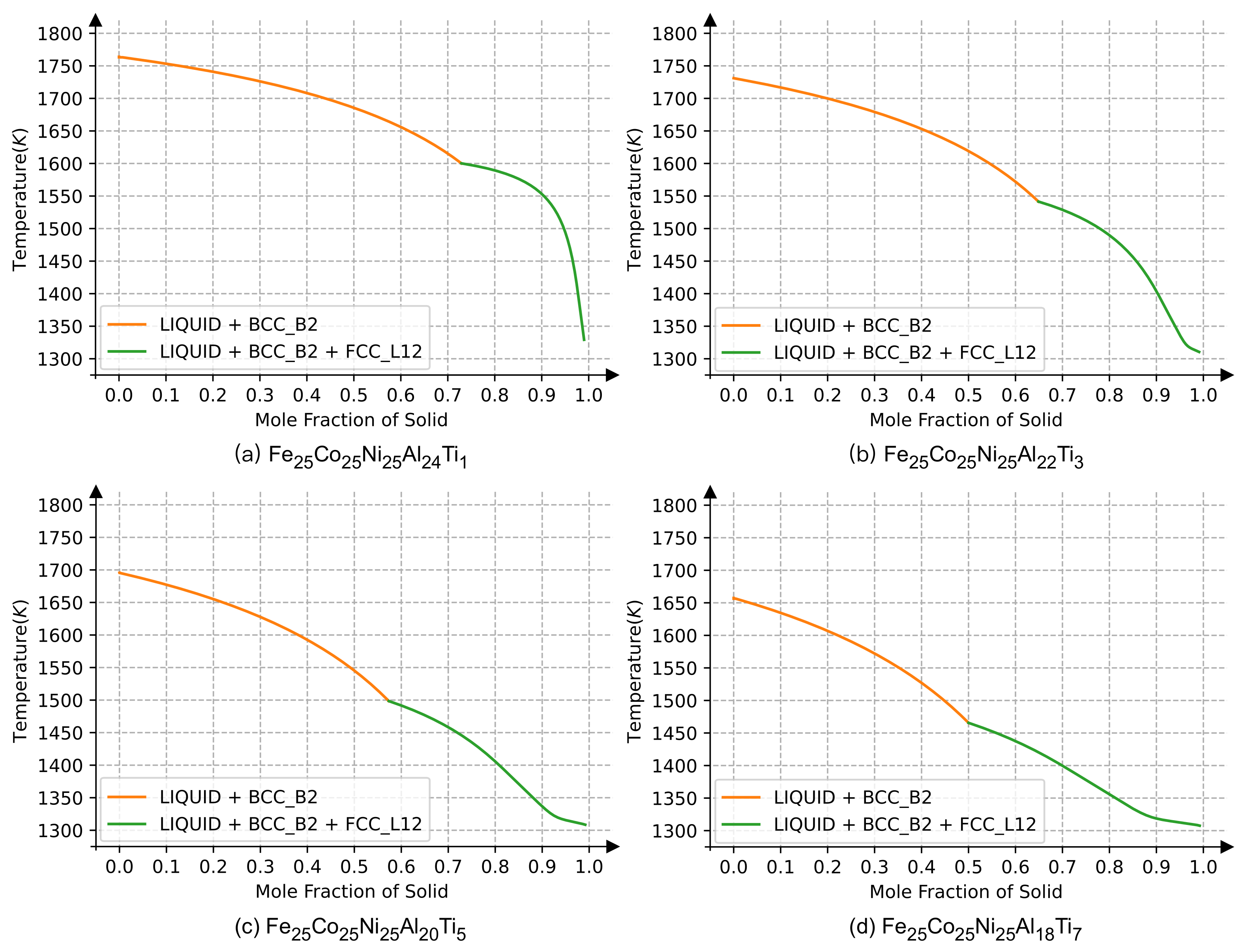
3.4. Alloying Effect of Ti on The Microstructure Evolution of FeCoNiAl1−xTix HEAs
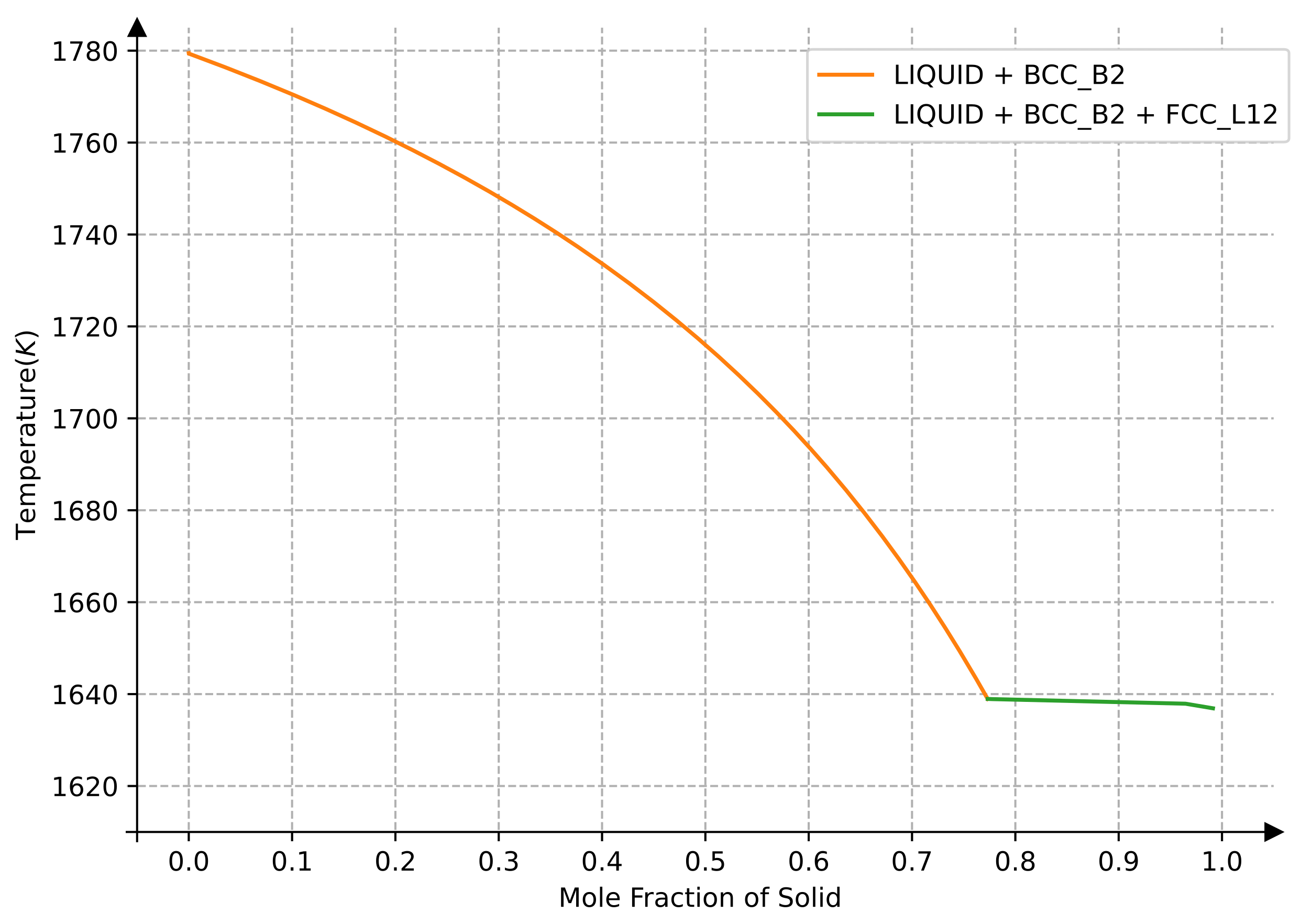
3.5. The Verification from CALPHAD
4. Conclusions
- (1)
- The chemical ordering in fcc-type FeCoNiAl1−xTix HEAs is short-range, while strain-induced interactions play an important role in the atomic ordering. Co atoms were found to accumulate at the interfaces between precipitates and matrix due to their weak interaction in FeCoNiAl1−xTix, the strong interactions of Al–Al and Ti–Ti atom pairs caused Al and Ti to act as the main components for forming the L12 precipitates.
- (2)
- The presence of the L12 phase and the chemical composition of L12 have been investigated comprehensively for the FeCoNiAl1−xTix high-entropy alloys. The site occupation in the L12 phase was found to be complex, and it requires further study. The order–disorder transition temperature from first-principles-based Monte Carlo simulations was 1650 K in FeCoNiAl HEAs, which is also consistent with the CALPHAD results. Moreover, the transition temperature decreased with increasing Ti contents in FeCoNiAl1−xTix HEAs.
- (3)
- The order–disorder transition results are in line with existing experimental observations, which means that a selected computer-aided design of the chemical composition can be used for further optimization of the microstructure characteristics in the FeCoNiAl1−xTix HEAs.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| HEAs | High-entropy alloys |
| CALPHAD | Calculation of phase diagrams |
| VASP | Vienna ab initio simulation package |
| PAW | Projector augmented-wave |
| GGA | Gerneralized gradient approximation |
| PBE | Perdew–Burke–Ernzerhof |
| DFT | Density functional theory |
| MC | Monte Carlo |
| SRO | Short-range order |
| BCC | Body-centered cubic |
| FCC | Face-centered cubic |
| HCP | Hexagonal close-packed |
References
- Yeh, J.W.; Chen, S.K.; Lin, S.J.; Gan, J.Y.; Chin, T.S.; Shun, T.T.; Tsau, C.H.; Chang, S.Y. Nanostructured High-Entropy Alloys with Multiple Principal Elements: Novel Alloy Design Concepts and Outcomes. Adv. Eng. Mater. 2004, 6, 299–303. [Google Scholar] [CrossRef]
- Cantor, B.; Chang, I.; Knight, P.; Vincent, A. Microstructural development in equiatomic multicomponent alloys. Mater. Sci. Eng. A 2004, 375–377, 213–218. [Google Scholar] [CrossRef]
- Otto, F.; Dlouhý, A.; Somsen, C.; Bei, H.; Eggeler, G.; George, E. The influences of temperature and microstructure on the tensile properties of a CoCrFeMnNi high-entropy alloy. Acta Mater. 2013, 61, 5743–5755. [Google Scholar] [CrossRef] [Green Version]
- Srivatsan, T.; Gupta, M. High Entropy Alloys:Innovations, Advances, and Applications, 1st ed.; CRC Press: Boca Raton, FL, USA, 2020. [Google Scholar] [CrossRef]
- Yang, T.; Zhao, Y.; Liu, W.; Kai, J.; Liu, C. L12-strengthened high-entropy alloys for advanced structural applications. J. Mater. Res. 2018, 33, 2983–2997. [Google Scholar] [CrossRef] [Green Version]
- Wu, Z.; Bei, H.; Pharr, G.; George, E. Temperature dependence of the mechanical properties of equiatomic solid solution alloys with face-centered cubic crystal structures. Acta Mater. 2014, 81, 428–441. [Google Scholar] [CrossRef]
- Zhang, Y.; Zuo, T.T.; Tang, Z.; Gao, M.C.; Dahmen, K.A.; Liaw, P.K.; Lu, Z.P. Microstructures and properties of high-entropy alloys. Prog. Mater. Sci. 2014, 61, 1–93. [Google Scholar] [CrossRef]
- Liu, W.; Lu, Z.; He, J.; Luan, J.; Wang, Z.; Liu, B.; Liu, Y.; Chen, M.; Liu, C. Ductile CoCrFeNiMox high entropy alloys strengthened by hard intermetallic phases. Acta Mater. 2016, 116, 332–342. [Google Scholar] [CrossRef]
- Liu, W.; Yang, T.; Liu, C. Precipitation hardening in CoCrFeNi-based high entropy alloys. Mater. Chem. Phys. 2018, 210, 2–11. [Google Scholar] [CrossRef]
- He, J.; Wang, H.; Huang, H.; Xu, X.; Chen, M.; Wu, Y.; Liu, X.; Nieh, T.; An, K.; Lu, Z. A precipitation-hardened high-entropy alloy with outstanding tensile properties. Acta Mater. 2016, 102, 187–196. [Google Scholar] [CrossRef] [Green Version]
- Zhao, Y.; Yang, T.; Tong, Y.; Wang, J.; Luan, J.; Jiao, Z.; Chen, D.; Yang, Y.; Hu, A.; Liu, C.; et al. Heterogeneous precipitation behavior and stacking-fault-mediated deformation in a CoCrNi-based medium-entropy alloy. Acta Mater. 2017, 138, 72–82. [Google Scholar] [CrossRef]
- Wang, Z.; Zhou, W.; Fu, L.; Wang, J.; Luo, R.; Han, X.; Chen, B.; Wang, X. Effect of coherent L12 nanoprecipitates on the tensile behavior of a fcc-based high-entropy alloy. Mater. Sci. Eng. A 2017, 696, 503–510. [Google Scholar] [CrossRef]
- Gwalani, B.; Choudhuri, D.; Soni, V.; Ren, Y.; Styles, M.; Hwang, J.; Nam, S.; Ryu, H.; Hong, S.; Banerjee, R. Cu assisted stabilization and nucleation of L12 precipitates in Al0.3CuFeCrNi2 fcc-based high entropy alloy. Acta Mater. 2017, 129, 170–182. [Google Scholar] [CrossRef] [Green Version]
- Chen, D.; He, F.; Han, B.; Wu, Q.; Tong, Y.; Zhao, Y.; Wang, Z.; Wang, J.; Kai, J. Synergistic effect of Ti and Al on L12-phase design in CoCrFeNi-based high entropy alloys. Intermetallics 2019, 110, 106476. [Google Scholar] [CrossRef]
- Chang, Y.J.; Yeh, A.C. The formation of cellular precipitate and its effect on the tensile properties of a precipitation strengthened high entropy alloy. Mater. Chem. Phys. 2018, 210, 111–119. [Google Scholar] [CrossRef]
- Fu, Z.; Chen, W.; Chen, Z.; Wen, H.; Lavernia, E.J. Influence of Ti addition and sintering method on microstructure and mechanical behavior of a medium-entropy Al0.6CoNiFe alloy. Mater. Sci. Eng. A 2014, 619, 137–145. [Google Scholar] [CrossRef]
- Fu, Z.; Chen, W.; Wen, H.; Morgan, S.; Chen, F.; Zheng, B.; Zhou, Y.; Zhang, L.; Lavernia, E.J. Microstructure and mechanical behavior of a novel Co20Ni20Fe20Al20Ti20 alloy fabricated by mechanical alloying and spark plasma sintering. Mater. Sci. Eng. A 2015, 644, 10–16. [Google Scholar] [CrossRef]
- Joseph, J.; Annasamy, M.; Kada, S.; Hodgson, P.; Barnett, M.; Fabijanic, D. Optimising the Al and Ti compositional window for the design of γ′ L12-strengthened Al-Co-Cr-Fe-Ni-Ti high entropy alloys. Mater. Sci. Eng. A 2022, 835, 142620. [Google Scholar] [CrossRef]
- Ferrari, A.; Dutta, B.; Gubaev, K.; Ikeda, Y.; Srinivasan, P.; Grabowski, B.; Körmann, F. Frontiers in atomistic simulations of high entropy alloys. J. Appl. Phys. 2020, 128, 150901. [Google Scholar] [CrossRef]
- Feng, R.; Liaw, P.K.; Gao, M.C.; Widom, M. First-Principles prediction of high-entropy-alloy stability. Npj Comput. Mater. 2017, 3, 50. [Google Scholar] [CrossRef] [Green Version]
- Chen, S.M.; Ma, Z.J.; Qiu, S.; Zhang, L.J.; Zhang, S.Z.; Yang, R.; Hu, Q.M. Phase decomposition and strengthening in HfNbTaTiZr high entropy alloy from first-principles calculations. Acta Mater. 2022, 225, 117582. [Google Scholar] [CrossRef]
- Nonh, Z.-S.; Zhu, J.-C.; Yu, H.-L.; Lai, Z.-H. First principles calculation of intermetallic compounds in FeTiCoNiVCrMnCuAl system high entropy alloy. Trans. Nonfer. Met. Soc. China 2012, 22, 1437–1444. [Google Scholar] [CrossRef]
- Huang, S. The chemical ordering and elasticity in FeCoNiAl1−xTix high-entropy alloys. Scr. Mater. 2019, 168, 5–9. [Google Scholar] [CrossRef]
- Körmann, F.; Ruban, A.V.; Sluiter, M.H. Long-ranged interactions in bcc NbMoTaW high-entropy alloys. Mater. Res. Lett. 2017, 5, 35–40. [Google Scholar] [CrossRef] [Green Version]
- Peng, H.; Hu, L.; Li, L.; Gao, J.; Zhang, Q. On the correlation between L12 nanoparticles and mechanical properties of (NiCo)52+2x(AlTi)4+2xFe29−4xCr15 (x = 0–4) high-entropy alloys. J. Alloys Compd. 2020, 817, 152750. [Google Scholar] [CrossRef]
- Guo, L.; Gu, J.; Gong, X.; Ni, S.; Song, M. CALPHAD aided design of high entropy alloy to achieve high strength via precipitate strengthening. Sci. China Mater. 2020, 63, 288–299. [Google Scholar] [CrossRef] [Green Version]
- He, S.; Peng, P.; Gorbatov, O.I.; Ruban, A.V. Effective interactions and atomic ordering in Ni-rich Ni-Re alloys. Phys. Rev. B 2016, 94, 024111. [Google Scholar] [CrossRef] [Green Version]
- Drexler, A.; He, S.; Razumovskiy, V.; Romaner, L.; Ecker, W.; Pippan, R. Verification of the generalised chemical potential for stress-driven hydrogen diffusion in nickel. Philos. Mag. Lett. 2020, 100, 513–523. [Google Scholar] [CrossRef]
- Drexler, A.; He, S.; Pippan, R.; Romaner, L.; Razumovskiy, V.; Ecker, W. Hydrogen segregation near a crack tip in nickel. Scr. Mater. 2021, 194, 113697. [Google Scholar] [CrossRef]
- He, S.; Popov, M.N.; Ecker, W.; Pippan, R.; Razumovskiy, V. A theoretical insight into hydrogen clustering at defects in Ni. Philos. Mag. Lett. 2021, 101, 68–78. [Google Scholar] [CrossRef]
- He, S.; Peng, P.; Peng, L.; Chen, Y.; Wei, H.; Hu, Z. An interplay of sulfur and phosphorus at the γ-Ni/γ′-Ni3Al interface. J. Alloys Compd. 2014, 597, 243–248. [Google Scholar] [CrossRef]
- Kulo, N.; He, S.; Ecker, W.; Pippan, R.; Antretter, T.; Razumovskiy, V.I. Thermodynamic and mechanical stability of Ni3X-type intermetallic compounds. Intermetallics 2019, 114, 106604. [Google Scholar] [CrossRef]
- Blöchl, P.E. Projector augmented-wave method. Phys. Rev. B 1994, 50, 17953–17979. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kresse, G.; Hafner, J. Ab Initio Molecular Dynamics for Open-Shell Transition Metals. Phys. Rev. B 1993, 48, 13115–13118. [Google Scholar] [CrossRef] [PubMed]
- Kresse, G.; Furthmüller, J. Efficiency of ab initio total energy calculations for metals and semiconductors using a plane-wave basis set. Comput. Mater. Sci. 1996, 6, 15–50. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [Green Version]
- Kresse, G.; Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 1999, 59, 1758. [Google Scholar] [CrossRef]
- Monkhorst, H.J.; Pack, J.D. Special points for Brillouin-zone integrations. Phys. Rev. B 1976, 13, 5188–5192. [Google Scholar] [CrossRef]
- He, S.; Scheiber, D.; Jechtl, T.; Moitzi, F.; Peil, O.; Romaner, L.; Zamberger, S.; Povoden-Karadeniz, E.; Razumovskiy, V.; Ruban, A.V. Solubility and segregation of B in paramagnetic fcc Fe. Phys. Rev. Mater. 2022, 6, 023604. [Google Scholar] [CrossRef]
- Bandyopadhyay, J.; Gupta, K. Low temperature lattice parameter of nickel and some nickel-cobalt alloys and Grüneisen parameter of nickel. Cryogenics 1977, 17, 345–347. [Google Scholar] [CrossRef]
- He, S.; Ecker, W.; Pippan, R.; Razumovskiy, V.I. Hydrogen-enhanced decohesion mechanism of the special Σ5(012)[100] grain boundary in Ni with Mo and C solutes. Comput. Mater. Sci. 2019, 167, 100–110. [Google Scholar] [CrossRef]
- He, S.; Ecker, W.; Peil, O.E.; Pippan, R.; Razumovskiy, V.I. The effect of solute atoms on the bulk and grain boundary cohesion in Ni: Implications for hydrogen embrittlement. Materialia 2022, 21, 101293. [Google Scholar] [CrossRef]
- Andersson, J.O.; Helander, T.; Hoglund, L.; Shi, P.; Sundman, B. Thermo-Calc and DICTRA, computational tools for materials science. Calphad 2002, 26, 273–312. [Google Scholar] [CrossRef]
- Mao, H.H.; Chen, H.L.; Chen, Q. TCHEA1 A Thermodynamic Database Not Limited for High Entropy Alloys. J. Phase Equilib. Diffus. 2017, 38, 353–368. [Google Scholar] [CrossRef] [Green Version]
- Schönfeld, B.; Sax, C.R.; Zemp, J.; Engelke, M.; Boesecke, P.; Kresse, T.; Boll, T.; Al-Kassab, T.; Peil, O.E.; Ruban, A.V. Local order in Cr-Fe-Co-Ni: Experiment and electronic structure calculations. Phys. Rev. B 2019, 99, 014206. [Google Scholar] [CrossRef] [Green Version]
- Chen, Y.; He, S.; Yi, Z.; Peng, P. Impact of correlative defects induced by double Re-addition on the ideal shear strength of γ′-Ni3Al phases. Comput. Mater. Sci. 2018, 152, 408–416. [Google Scholar] [CrossRef]
- Chen, Y.; He, S.; Yi, Z.; Peng, P. A synergistic reinforcement of Re and W for ideal shear strengths of γ′-Ni3Al phases. J. Phys. Chem. Solids 2019, 131, 34–43. [Google Scholar] [CrossRef]
- Slater, J.C. Atomic Radii in Crystals. J. Chem. Phys. 1964, 41, 3199–3204. [Google Scholar] [CrossRef]
- Chang, Y.J.; Yeh, A.C. The evolution of microstructures and high temperature properties of AlxCo1.5CrFeNi1.5Tiy high entropy alloys. J. Alloys Compd. 2015, 653, 379–385. [Google Scholar] [CrossRef]
- Kostiuchenko, T.; Ruban, A.V.; Neugebauer, J.; Shapeev, A.; Körmann, F. Short-range order in face-centered cubic VCoNi alloys. Phys. Rev. Mater. 2020, 4, 113802. [Google Scholar] [CrossRef]
- Zheng, W.; He, S.; Selleby, M.; He, Y.; Li, L.; Lu, X.-G.; Ågren, J. Thermodynamic assessment of the Al-C-Fe system. Calphad 2017, 58, 34–49. [Google Scholar] [CrossRef]
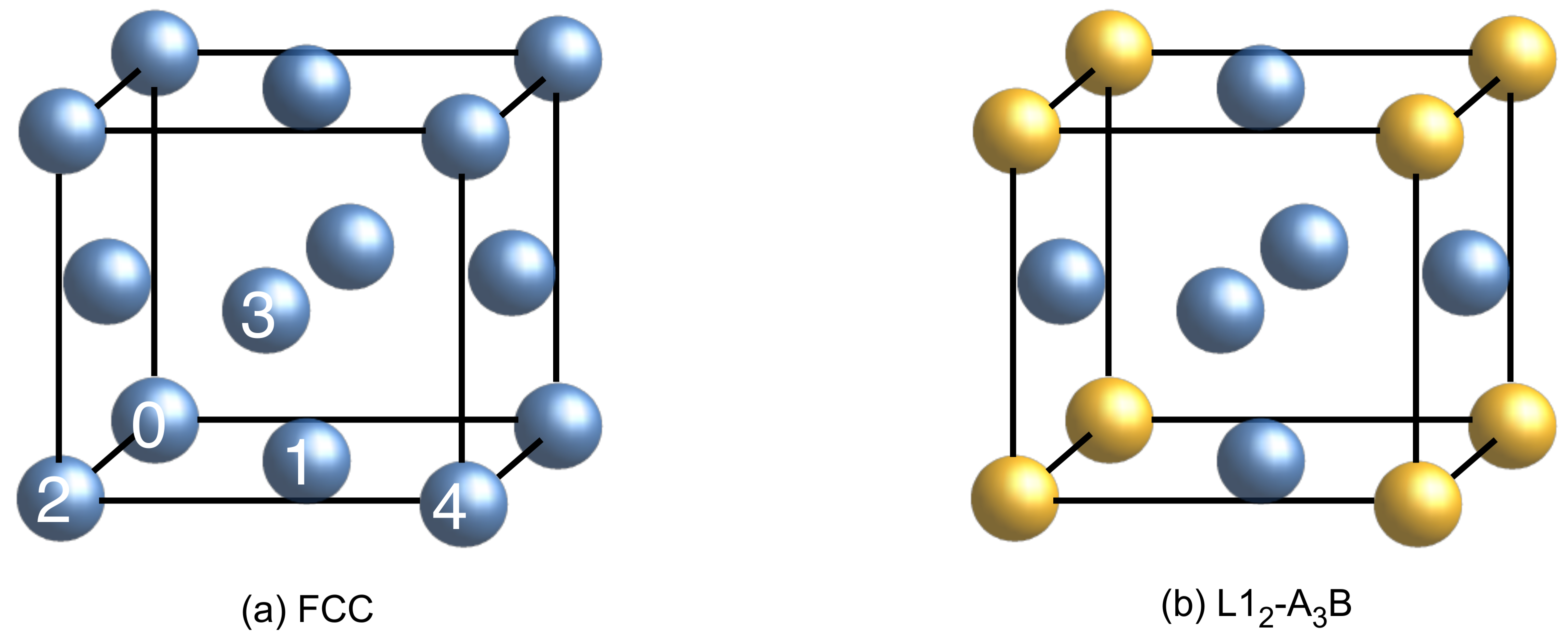




| 110 | 200 | 211 | 220 | 110 | 200 | 211 | 220 | |
|---|---|---|---|---|---|---|---|---|
| Al–Al | 0.243 | −0.009 | 0.003 | −0.013 | −0.099 | −0.002 | −0.003 | 0.044 |
| Al–Co | 0.063 | −0.010 | −0.016 | −0.012 | −0.083 | −0.003 | 0.000 | −0.012 |
| Al–Fe | 0.077 | −0.021 | −0.013 | 0.006 | −0.173 | −0.003 | 0.002 | 0.044 |
| Al–Ti | 0.127 | −0.024 | −0.012 | 0.007 | −0.144 | 0.003 | −0.195 | −0.001 |
| Co–Co | 0.003 | −0.002 | 0.001 | 0.010 | 0.000 | −0.001 | 0.001 | −0.001 |
| Co–Fe | 0.029 | 0.001 | 0.004 | −0.002 | −0.018 | 0.001 | −0.001 | 0.001 |
| Co–Ti | 0.203 | −0.082 | −0.022 | −0.020 | −0.011 | −0.005 | −0.007 | −0.012 |
| Fe–Fe | 0.115 | −0.015 | 0.015 | 0.010 | −0.014 | 0.000 | 0.001 | 0.000 |
| Fe–Ti | 0.129 | −0.073 | −0.018 | −0.013 | −0.025 | −0.004 | −0.003 | −0.016 |
| Ti–Ti | 0.367 | −0.129 | −0.052 | −0.054 | 0.087 | −0.053 | −0.059 | 0.087 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, Y.; Li, Z.; Feng, H.; He, S. Atomic Interactions and Order–Disorder Transition in FCC-Type FeCoNiAl1−xTix High-Entropy Alloys. Materials 2022, 15, 3992. https://doi.org/10.3390/ma15113992
Wu Y, Li Z, Feng H, He S. Atomic Interactions and Order–Disorder Transition in FCC-Type FeCoNiAl1−xTix High-Entropy Alloys. Materials. 2022; 15(11):3992. https://doi.org/10.3390/ma15113992
Chicago/Turabian StyleWu, Ying, Zhou Li, Hui Feng, and Shuang He. 2022. "Atomic Interactions and Order–Disorder Transition in FCC-Type FeCoNiAl1−xTix High-Entropy Alloys" Materials 15, no. 11: 3992. https://doi.org/10.3390/ma15113992
APA StyleWu, Y., Li, Z., Feng, H., & He, S. (2022). Atomic Interactions and Order–Disorder Transition in FCC-Type FeCoNiAl1−xTix High-Entropy Alloys. Materials, 15(11), 3992. https://doi.org/10.3390/ma15113992







