Quantitative Distribution Characterization and Correlation Study of Composition, Structure and Hardness of Rim Region in Railway Wheel
Abstract
1. Introduction
2. Materials and Methods
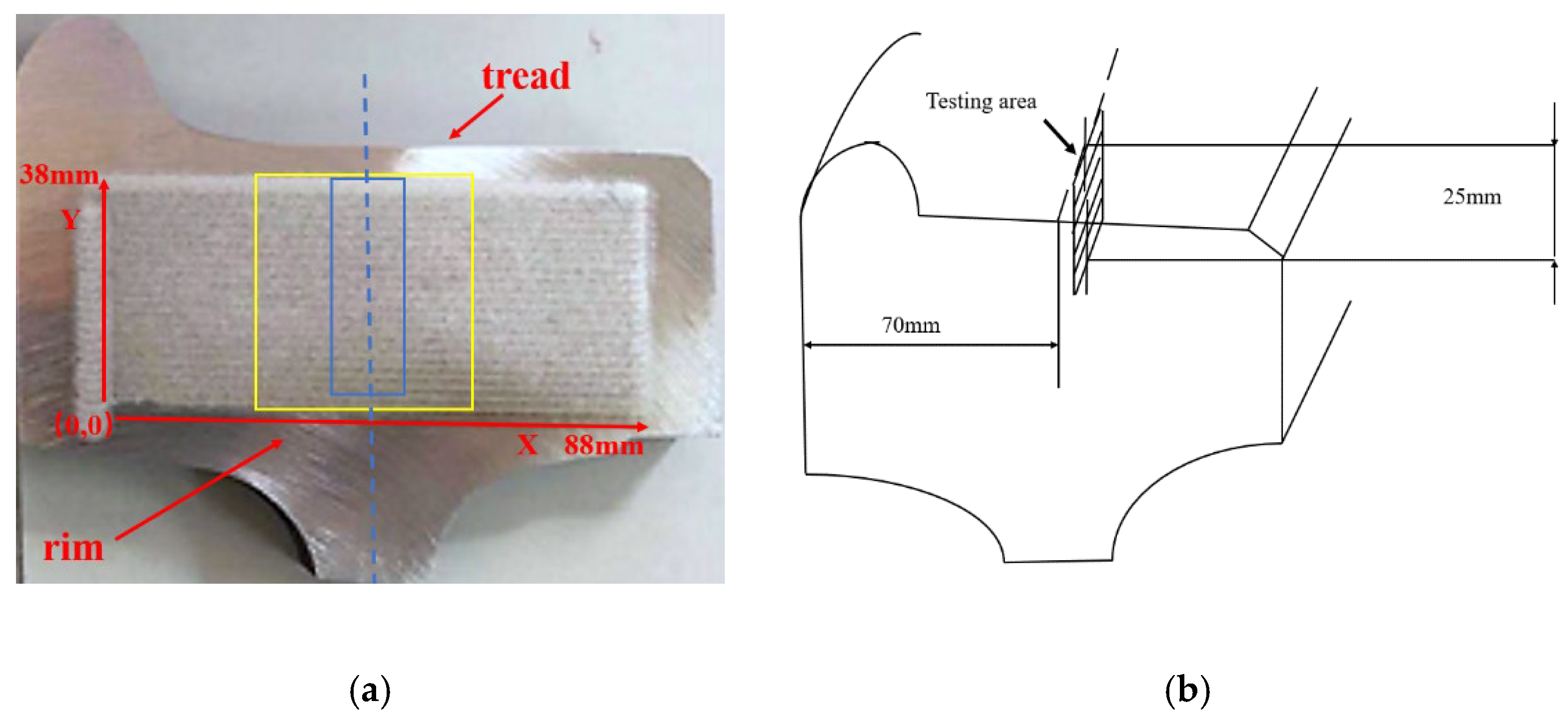
2.1. Composition and Process of Experimental Samples
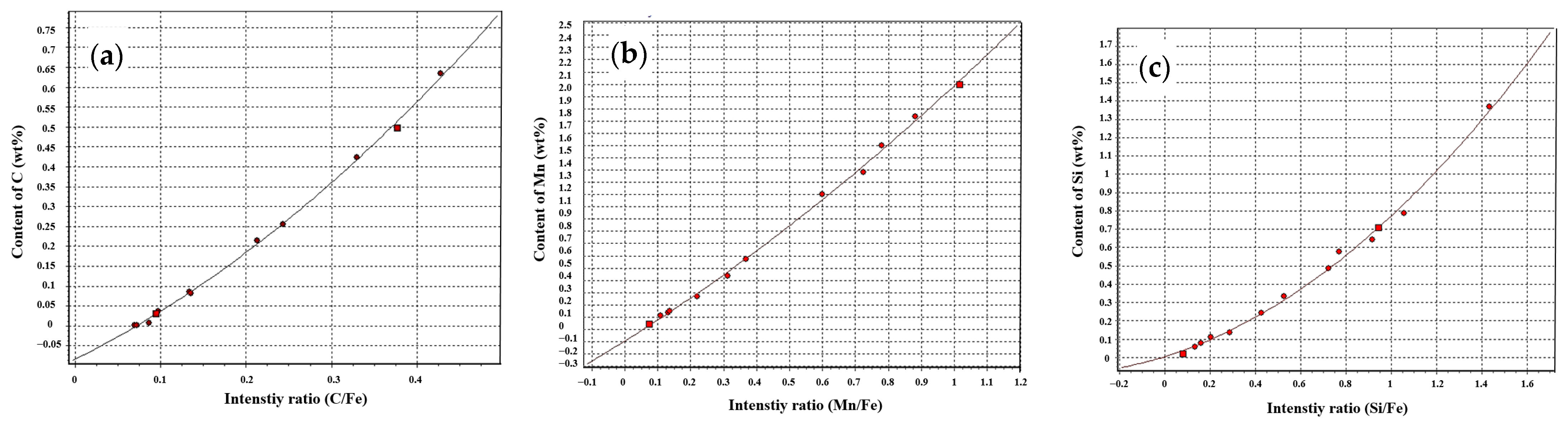
2.2. Quantitative Distribution Characterization of Compositions and Inclusions in Rim Region
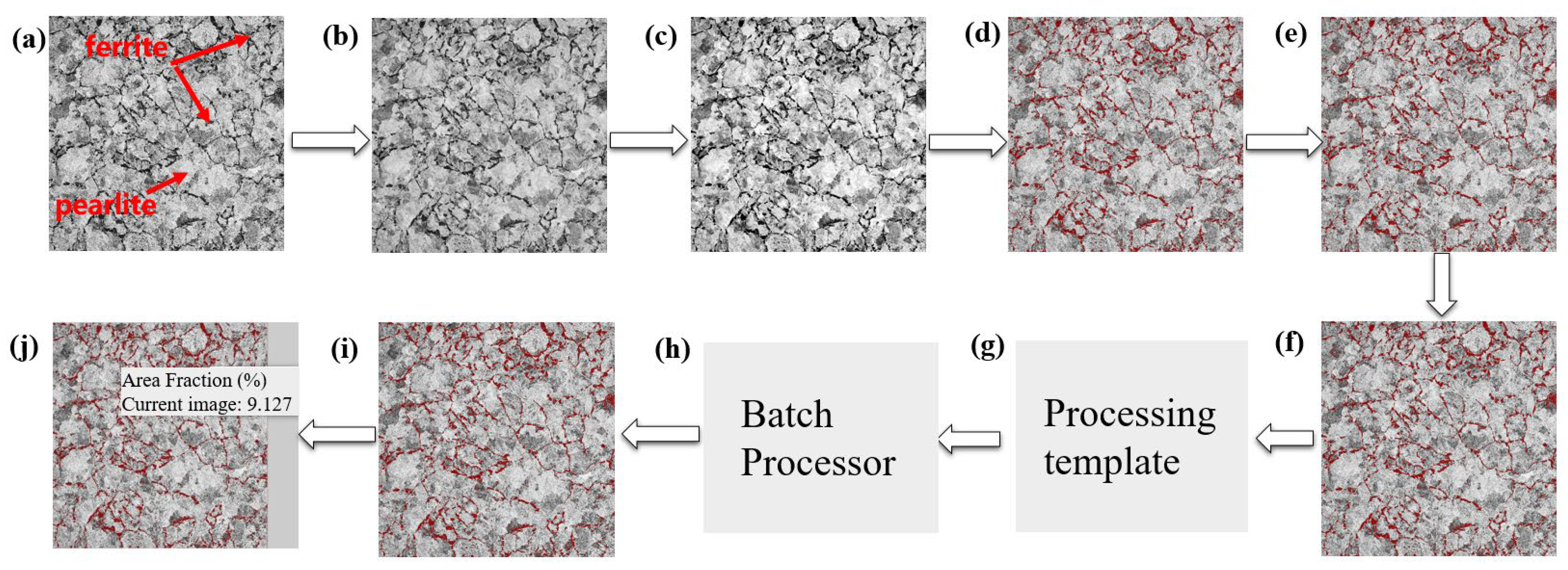
2.3. Quantitative Distribution Characterization of Microstructure and Hardness on the Wheel Rim
3. Results
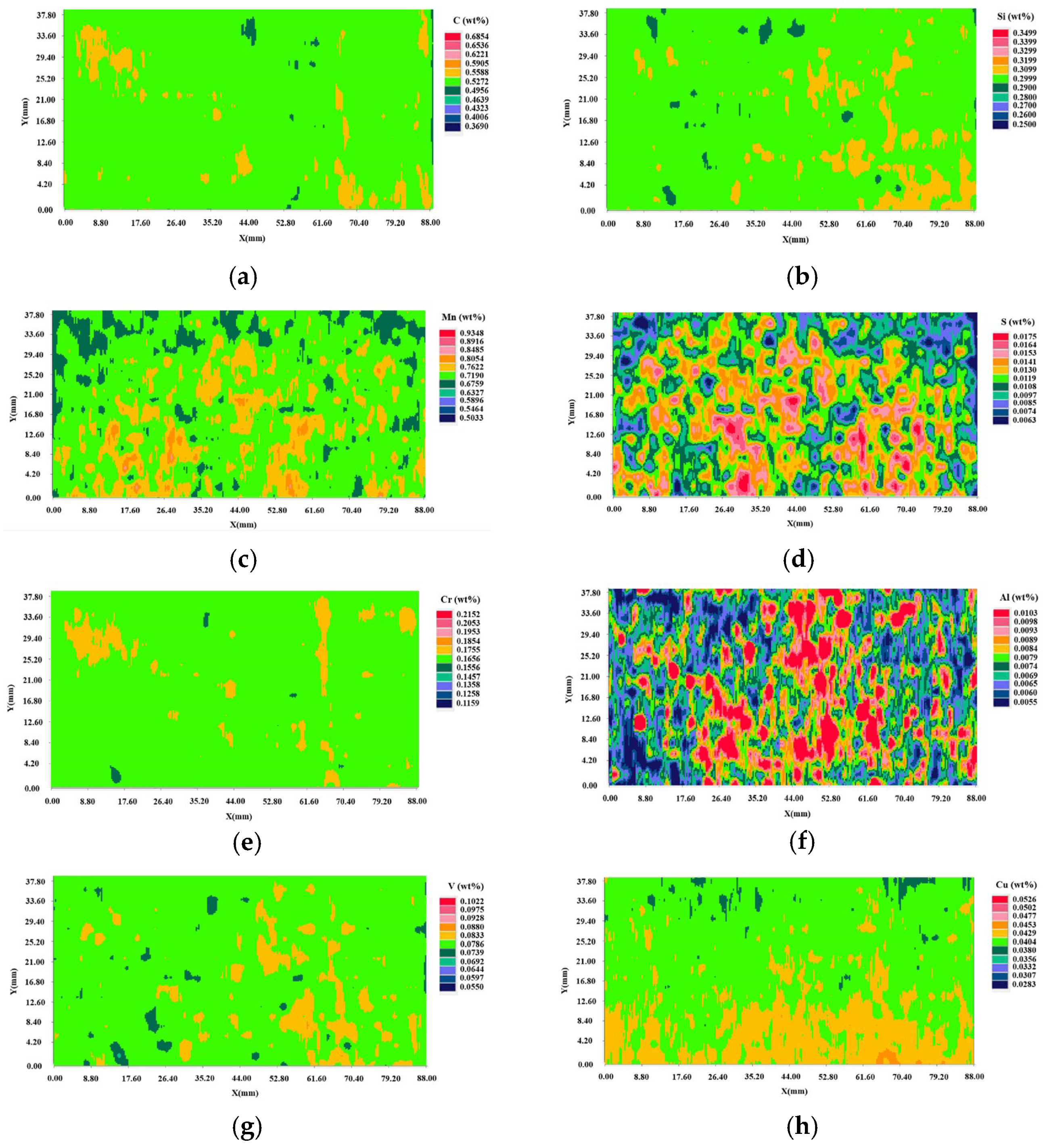
3.1. Elemental Distribution in Rim Area of Railway Wheel
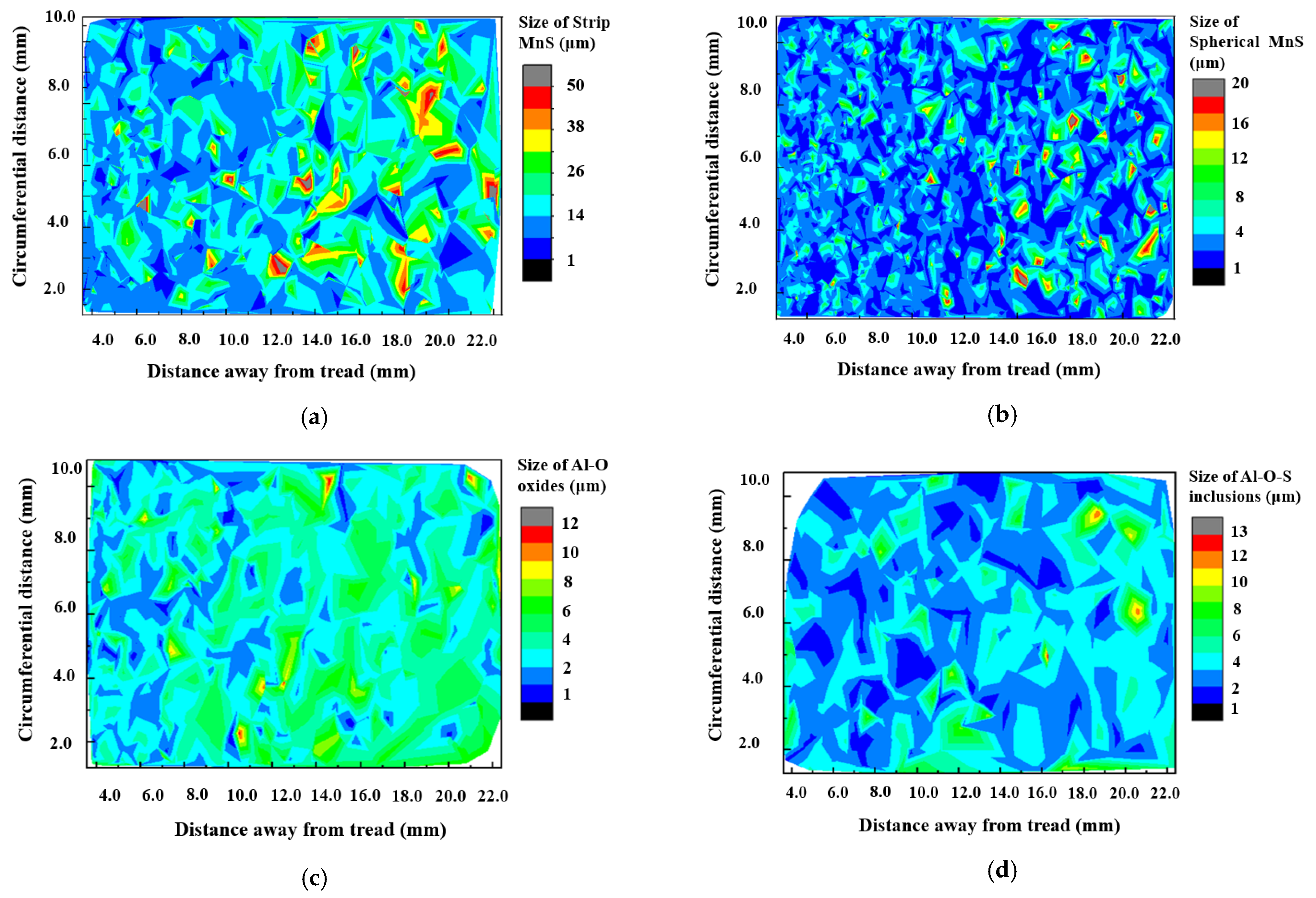
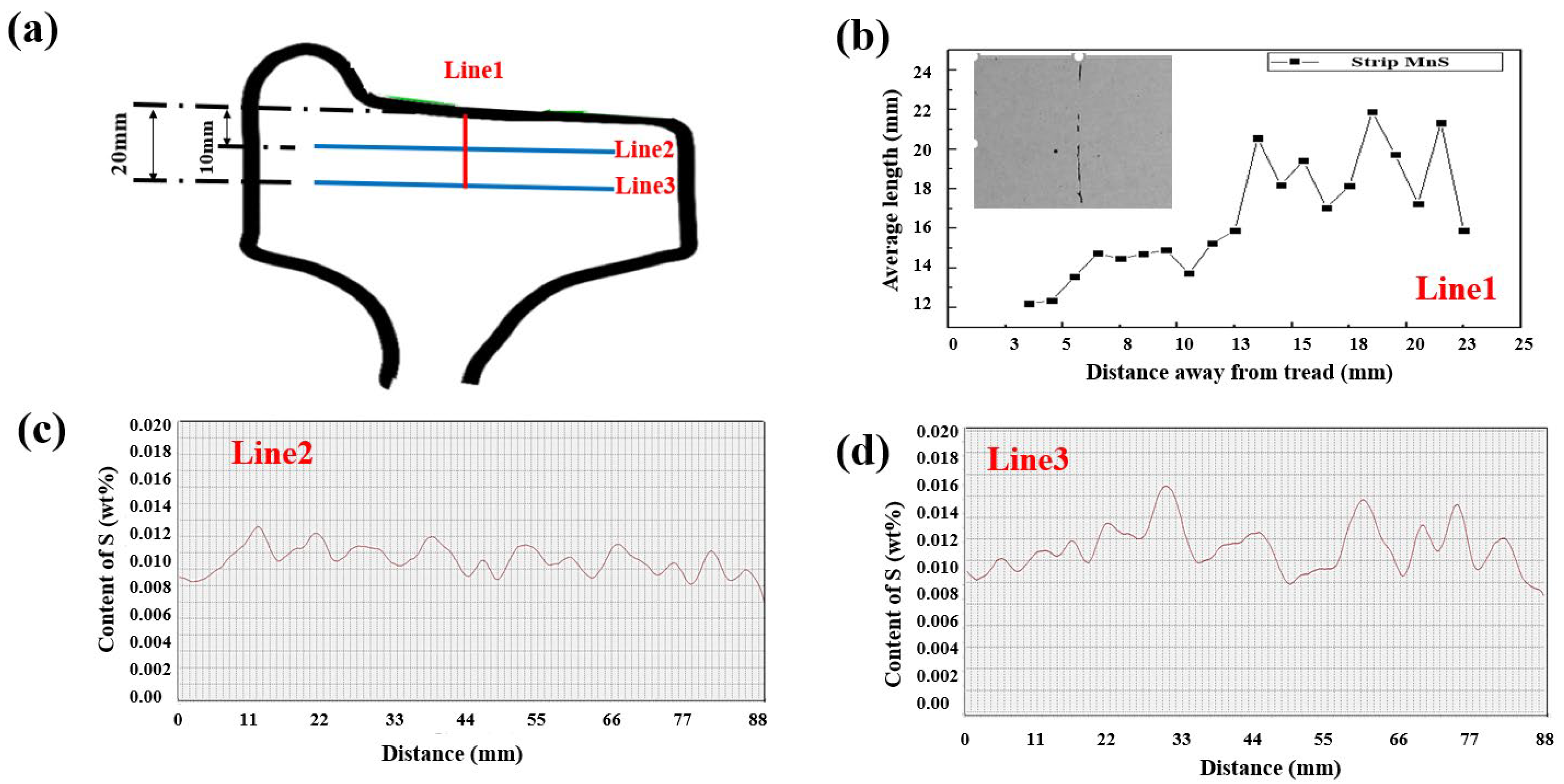
3.2. Distribution of Inclusions near Tread Area in Rim Center
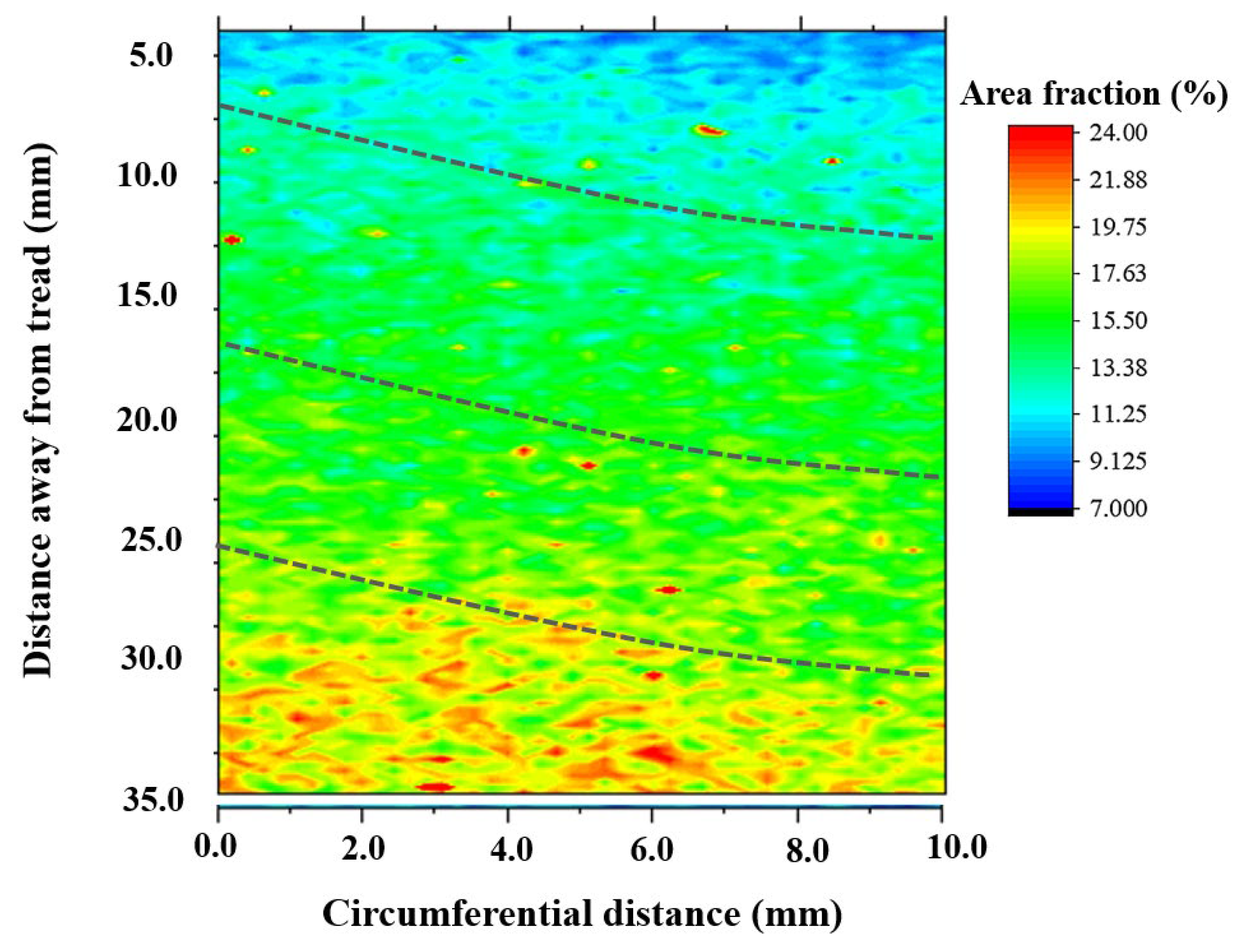
3.3. Ferrite Structure and Micro Hardness Distribution in the Central Area of Rim
4. Discussion
4.1. Influence of Inclusions Distribution on the Element Segregation
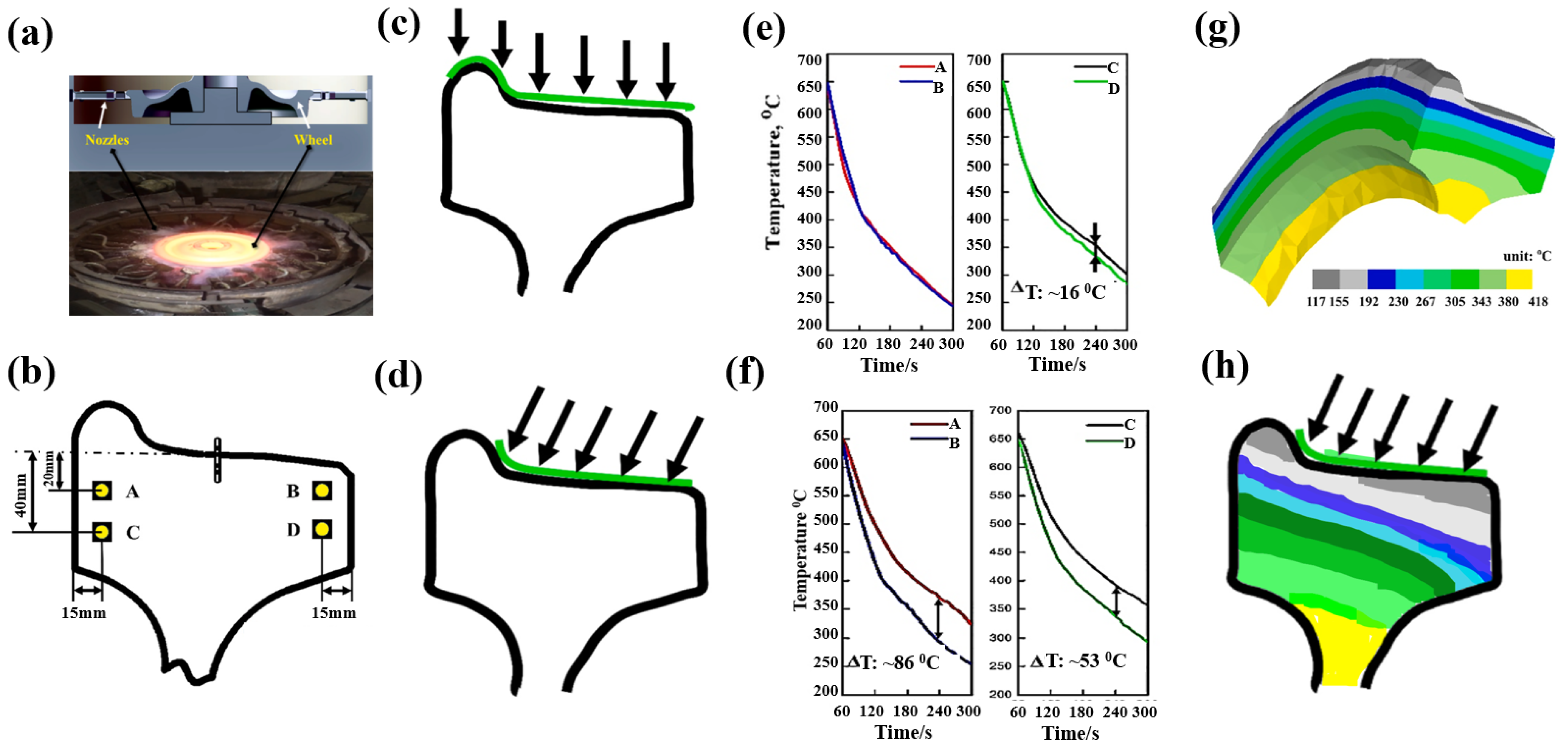
4.2. The Relationship between Quenching Process, Microstructure and Hardness Distribution of Wheel
5. Conclusions
- (1)
- There was serious segregation of S and Al elements on the region of wheel rim with the statistical segregation degree of S and Al exceeded 0.1. The segregation trend of S and Mn presented on the two-dimensional distribution map was also very similar. The distribution of C, Si, Cr, and V elements was relatively homogeneous and the values of the statistical segregation degree for these elements were all less than 0.05.
- (2)
- The size distribution of long strip sulfides has a great impact on the segregation degree of sulfur elements. With the length increase of elongated MnS sulfides, the segregation degree of S element increased. Inclusion modification techniques through sulfides (MnS) enveloping reduced effectively the stress concentration generated by the oxides and the size of these oxides enveloped by sulfides ranged mainly from 1 to 5 μm and inclusions larger than 10 μm were rarely observed.
- (3)
- Asymmetrical graded distribution of ferrite area fraction and micro hardness appeared from the tread surface to the radical depth of 35−50 mm below the surface as a result of the unsynchronized cooling on both sides of the rim. Due to the influence of tread water spray cooling process, the phase behavior of ferrite was altered. In the radial depth of 5 mm below the tread surface, the ferrite area fraction was about 10%, but in the radial depth of 35 mm away from the tread, the ferrite area fraction in-creased to more than 20%. The distribution of ferrite structure at the rim has a great impact on the hardness distribution. The micro hardness reduced linearly with radical depth below the tread surface the increase of ferrite area fraction. The Vickers hardness value varied in range from 260 HV to 290 HV, with the trend of increasing hardness toward the tread sur-face and toward the right-hand (field) side of the wheel rim.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Paul, M.B.; Claire, D.; Adam, B. The Influence of Wheel/Rail Contact Conditions on the Microstructure and Hardness of Railway Wheels. Sci. World J. 2014, 4, 209752. [Google Scholar]
- Liu, C.P.; Zhao, X.J.; Liu, P.T.; Pan, J.Z.; Ren, R.M. Influence of Contact Stress on Surface Microstructure and Wear Property of D2/U71Mn Wheel-Rail Material. Materials 2019, 12, 3268. [Google Scholar] [CrossRef] [PubMed]
- Liu, C.-P.; Pan, J.-Z.; Liu, P.-T.; Ren, R.-M.; Zhao, X.-J. Influence of original microstructure on rolling contact fatigue property of D2 wheel steel. Wear 2020, 456–457, 203380. [Google Scholar] [CrossRef]
- Chen, Y.D.; Ren, R.M.; Zhao, X.J.; Chen, C.H.; Pan, R. Study on the surface microstructure evolution and wear property of bainitic rail steel under dry sliding wear. Wear 2020, 448–449, 203217. [Google Scholar] [CrossRef]
- Zhou, S.T.; Li, Z.D.; Jiang, L.; Wang, X.; Xu, P.; Ma, Y.X.; Yan, Y.P.; Yang, C.F.; Yong, Q.L. An investigation into the role of non-metallic inclusions in cleavage fracture of medium carbon pearlitic steels for high-speed railway wheel. Eng. Fail. Anal. 2022, 131, 105860. [Google Scholar] [CrossRef]
- Gong, T.; Liu, X.L.; Wu, S.; Zhang, G.Z.; Chen, E.Q.; Qian, G.A.; Berto, F. Study on damage tolerance and remain fatigue life of shattered rim of railway wheels. Eng. Fail. Anal. 2021, 123, 105322. [Google Scholar]
- Li, Z.D.; Zhou, S.T.; Yang, C.F.; Yong, Q.L. High/very high cycle fatigue behaviors of medium carbon pearlitic wheel steels and the effects of microstructure and non-metallic inclusions. Mater. Sci. Eng. A 2019, 764, 138208. [Google Scholar] [CrossRef]
- Zhang, G.Z.; Ren, R.M. Study on typical failure forms and causes of high-speed railway wheels. Eng. Fail. Anal. 2019, 105, 1287–1295. [Google Scholar] [CrossRef]
- Diao, G.; Yan, Q.; Shi, X.; Zhang, X.; Wen, Z.; Jin, X. Improvement of wear resistance in ferrite-pearlite railway wheel steel via ferrite strengthening and cementite spheroidization. Mater. Res. Express 2019, 6, 106513. [Google Scholar] [CrossRef]
- Rezende, A.B.; Fonseca, S.T.; Miranda, R.S.; Fernanes, F.M.; Grijalba, F.A.F.; Farina, P.F.S.; Mei, P.R. Effect of niobium and molybdenum addition on the wear resistance and the rolling contact fatigue of railway wheels. Wear 2021, 466–467, 203571. [Google Scholar] [CrossRef]
- Zuo, Y.; Zhou, S.T.; Li, Z.D.; Pan, T.; Xiang, J.Z.; Yong, Q.L. Effect of V and Si on Microstructure and Mechanical Properties of Medium-carbon Pearlitic Steels for Wheel. Chin. J. Mater. Res. 2016, 30, 401–408. [Google Scholar]
- Shi, J.P.; Liu, Z.X.; Xiao, Q. The effect of chemical composition, microstructure on the mechanical properties of wheel steels. China Railw. Sci. 2012, 33, 44–50. [Google Scholar]
- Zeng, D.F.; Lu, L.T.; Gong, Y.H.; Zhang, N.; Gong, Y.B. Optimization of strength and toughness of railway wheel steel by alloy design. Mater. Des. 2016, 92, 998–1006. [Google Scholar] [CrossRef]
- Ma, Y.; Pan, T.; Jiang, B.; Cui, Y.H.; Su, H. Study of the effect of sulfur contents on fracture toughness of railway wheel steels for high speed train. Acta Metall. Sin. 2011, 47, 978–983. [Google Scholar]
- Sourmail, T.; Garcia, C.M.; Caballero, F.G.; Cazottes, S.; Epicier, T.; Danoix, F.; Milbourn, D. The Influence of Vanadium on Ferrite and Bainite Formation in a Medium Carbon Steel. Metall. Mater. Trans. A 2017, 48, 3985–3996. [Google Scholar] [CrossRef]
- Gao, B.; Tan, Z.L.; Liu, Z.N.; Gao, G.H.; Zhang, M.; Zhang, G.Z. Influence of non-uniform microstructure on rolling contact fatigue behavior of high-speed wheel steels. Eng. Fail. Anal. 2019, 100, 485–491. [Google Scholar] [CrossRef]
- Zhang, R.J.; Zheng, C.L.; Lv, B.; Zhang, P.J.; Gao, G.H.; Yang, Y.Q.; Zhang, F.C. Effect of non-uniform microstructure on rolling contact fatigue performance of bainitic rail steel. Int. J. Fatigue 2022, 159, 106795. [Google Scholar]
- Li, G.; Hong, Z.Y.; Yan, Q.Z. The influence of microstructure on the rolling contact fatigue of steel for high-speed-train wheel. Wear 2015, 342–343, 349–355. [Google Scholar] [CrossRef]
- Liu, X.D.; Liu, P.T.; Zhao, X.J.; Ren, R.M. Influence of Original Microstructure on Rolling Contact Fatigue Properties of ER9 Wheel Steel. Thibology 2021, 41, 902–912. [Google Scholar]
- Zhang, X.F.; Jia, Y.H.; Sheng, L.; Yuan, L.J.; Li, J.Q. Characterization of segregation degree for large size metal component and application on high-speed train wheel. Anal. Chim. Acta 2022, 1203, 337719. [Google Scholar] [CrossRef]
- Suetrong, C.; Uthaisangsuk, V. Investigation of fatigue crack propagation in ER8 railway wheel steel with varying microstructures. Mater. Sci. Eng. A 2022, 840, 142980. [Google Scholar] [CrossRef]
- Tian, Y.; Tan, Z.L.; Wang, J.; Wang, R.; Liu, Y.R.; Zhang, M. Experiment and finite element analysis of asymmetrical hardness induced by quenching in railway wheel. Eng. Fail. Anal. 2022, 133, 105959. [Google Scholar] [CrossRef]
- Jia, Y.H.; Cheng, H.M.; Yang, J.W.; Sun, J.J. Key points and application of revised spark discharge atomic emission spectrometry standard including GB/T 14203-2016 and GB/T 4336-2016. Metall. Anal. 2017, 37, 1–10. [Google Scholar]
- Li, D.L.; Wang, H.Z. Original position statistic distribution analysis for the sulfides in gear steels. ISIJ Int. 2014, 54, 160–164. [Google Scholar] [CrossRef][Green Version]
- Hui, W.; Chen, S.; Zhang, Y.; Shao, C.; Dong, H. Effect of vanadium on the high-cycle fatigue fracture properties of medium-carbon microalloyed steel for fracture splitting connecting rod. Mater. Des. 2015, 66, 227–234. [Google Scholar] [CrossRef]
- Murakami, Y.; Kodama, S.; Konuma, S. Quantitative evaluation of effects of non-metallic inclusions on fatigue strength of high strength steels. I: Basic fatigue mechanism and evaluation of correlation between the fatigue fracture stress and the size and location of non-metallic inclusions. Int. J. Fatigue 1989, 11, 291–298. [Google Scholar] [CrossRef]
- Stiénon, A.; Fazekas, A.; Buffière, J.-Y.; Vincent, A.; Daguier, P.; Merchi, F. A new methodology based on X-ray micro-tomography to estimate stress concentrations around inclusions in high strength steels. Mater. Sci. Eng. A 2009, 513–514, 376–383. [Google Scholar] [CrossRef]
- Pan, T.; Yang, Z.G.; Bai, B.Z.; Fang, H.S. Study of thermal stress and strain energy in γ-Fe matrix around inclusion caused by thermal coefficient difference. Acta Metall. Sin. 2003, 39, 1037–1042. [Google Scholar]
- Cong, T.; Qian, G.A.; Zhang, G.Z.; Wu, S.; Pan, X.N.; Du, L.M.; Liu, X.L. Effects of inclusion size and stress ratio on the very-high-cycle fatigue behavior of pearlitic steel. Int. J. Fatigue 2021, 142, 105958. [Google Scholar] [CrossRef]
- Furuuhara, T.; Kikumoto, K.; Saito, H.; Sekine, T.; Ogawa, T.; Morito, S.; Maki, T. Phase transformation from fine grained austenite. ISIJ Int. 2008, 48, 1038–1045. [Google Scholar] [CrossRef]





| Sample | C | Si | Mn | P | S | Cr | Cu | Mo | V | Al | Fe |
|---|---|---|---|---|---|---|---|---|---|---|---|
| D2 | 0.520 | 0.304 | 0.720 | 0.006 | 0.011 | 0.167 | 0.039 | 0.008 | 0.087 | 0.009 | 98.129 |
| SD | 0.0041 | 0.0022 | 0.0044 | 0.0005 | 0.0010 | 0.0019 | 0.0035 | 0.0009 | 0.0015 | 0.0008 | 0.0208 |
| Element | Average Content% | Maximum Segregation Degree | Position (X, Y) * | Statistical Segregation Degree |
|---|---|---|---|---|
| C | 0.527 | 1.072 | (22.59, 4.05) | 0.0283 |
| Si | 0.305 | 1.032 | (36.37, 5.95) | 0.0166 |
| Mn | 0.728 | 1.133 | (28.16, 4.05) | 0.0556 |
| S | 0.010 | 1.527 | (59.84, 10.01) | 0.2926 |
| Cr | 0.166 | 1.067 | (80.67, 25.97) | 0.0247 |
| Cu | 0.040 | 1.080 | (45.76, 5.95) | 0.0409 |
| V | 0.084 | 1.083 | (28.16, 4.05) | 0.0316 |
| Al | 0.008 | 4.189 | (54.56, 25.97) | 0.3900 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, D.; Wang, H.; Shen, X.; Lin, S.; Feng, H.; Peng, Y.; Jiang, F.; Zhou, X. Quantitative Distribution Characterization and Correlation Study of Composition, Structure and Hardness of Rim Region in Railway Wheel. Materials 2022, 15, 4762. https://doi.org/10.3390/ma15144762
Li D, Wang H, Shen X, Lin S, Feng H, Peng Y, Jiang F, Zhou X. Quantitative Distribution Characterization and Correlation Study of Composition, Structure and Hardness of Rim Region in Railway Wheel. Materials. 2022; 15(14):4762. https://doi.org/10.3390/ma15144762
Chicago/Turabian StyleLi, Dongling, Haizhou Wang, Xuejing Shen, Shuangping Lin, Haozhou Feng, Ya Peng, Fan Jiang, and Xuefan Zhou. 2022. "Quantitative Distribution Characterization and Correlation Study of Composition, Structure and Hardness of Rim Region in Railway Wheel" Materials 15, no. 14: 4762. https://doi.org/10.3390/ma15144762
APA StyleLi, D., Wang, H., Shen, X., Lin, S., Feng, H., Peng, Y., Jiang, F., & Zhou, X. (2022). Quantitative Distribution Characterization and Correlation Study of Composition, Structure and Hardness of Rim Region in Railway Wheel. Materials, 15(14), 4762. https://doi.org/10.3390/ma15144762






