Research on Mechanical Behavior of the Steel–Concrete–Steel Composite Structures Subjected to High Temperature of Fire
Abstract
:1. Introduction
2. Structural Form of Immersed Tube Tunnel Sections
3. Experimental Scheme of Mechanical Behavior of the Steel–Concrete–Steel Composite Structure under a High Temperature of Fire
3.1. Processing of Components
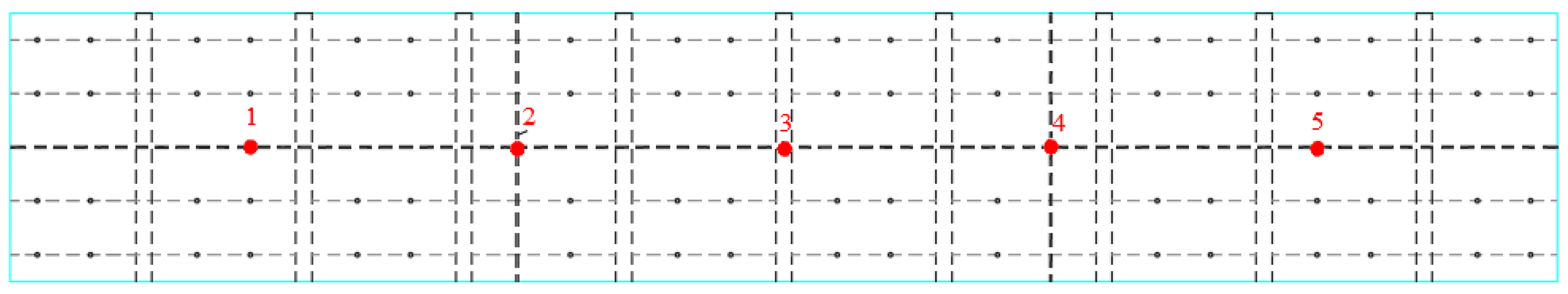
3.2. Arrangement of Measuring Points
3.2.1. Arrangement of Internal Temperature Measuring Points
3.2.2. Arrangement of the Measuring Points of the Roof Displacement
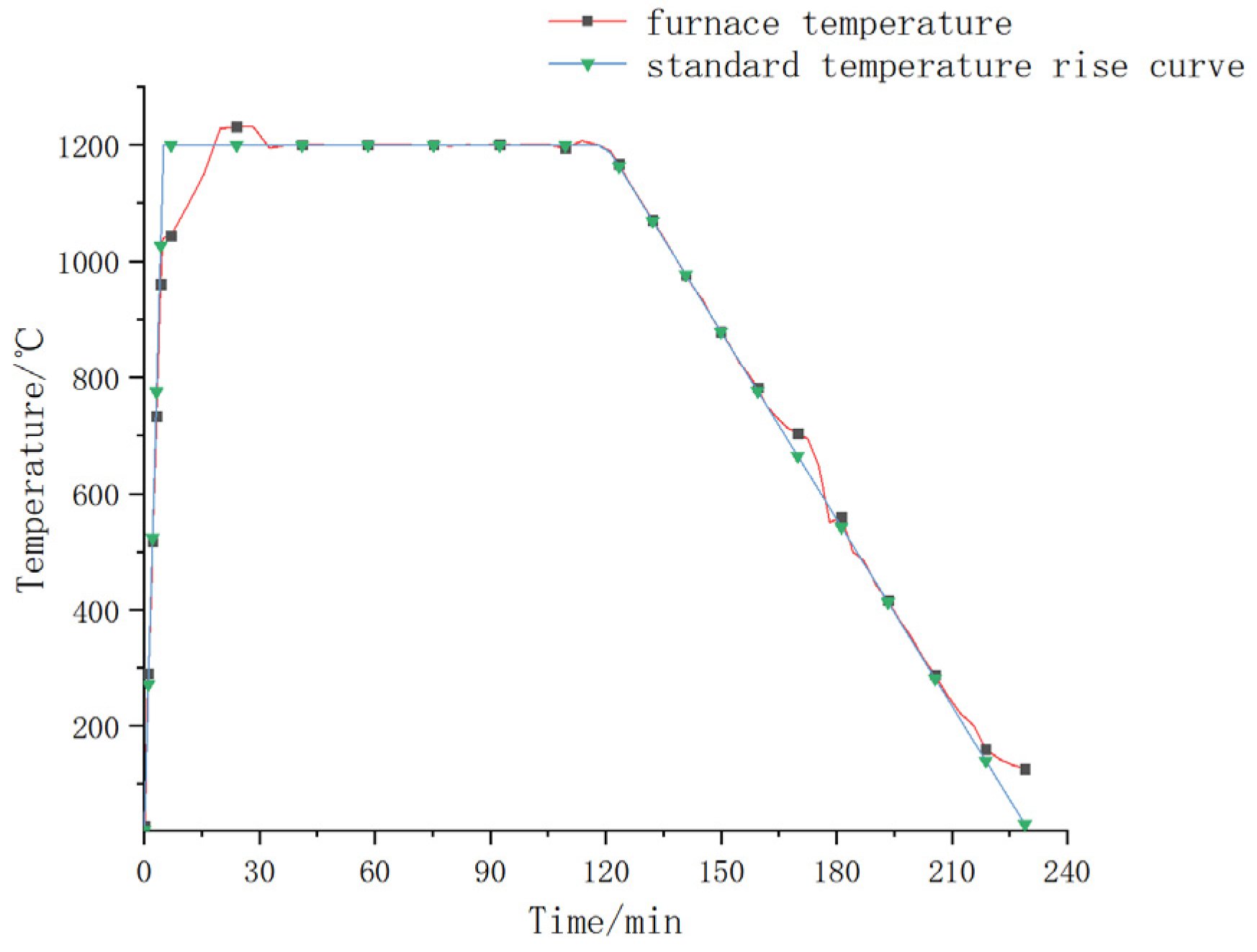
3.3. Test Implementation
4. Analysis of Test Results
4.1. The Overall Test Phenomena
4.2. Analysis of Temperature Transfer and Deformation Law of Composite Structures under a High Temperature of Fire
4.2.1. Temperature Distribution Law in Component
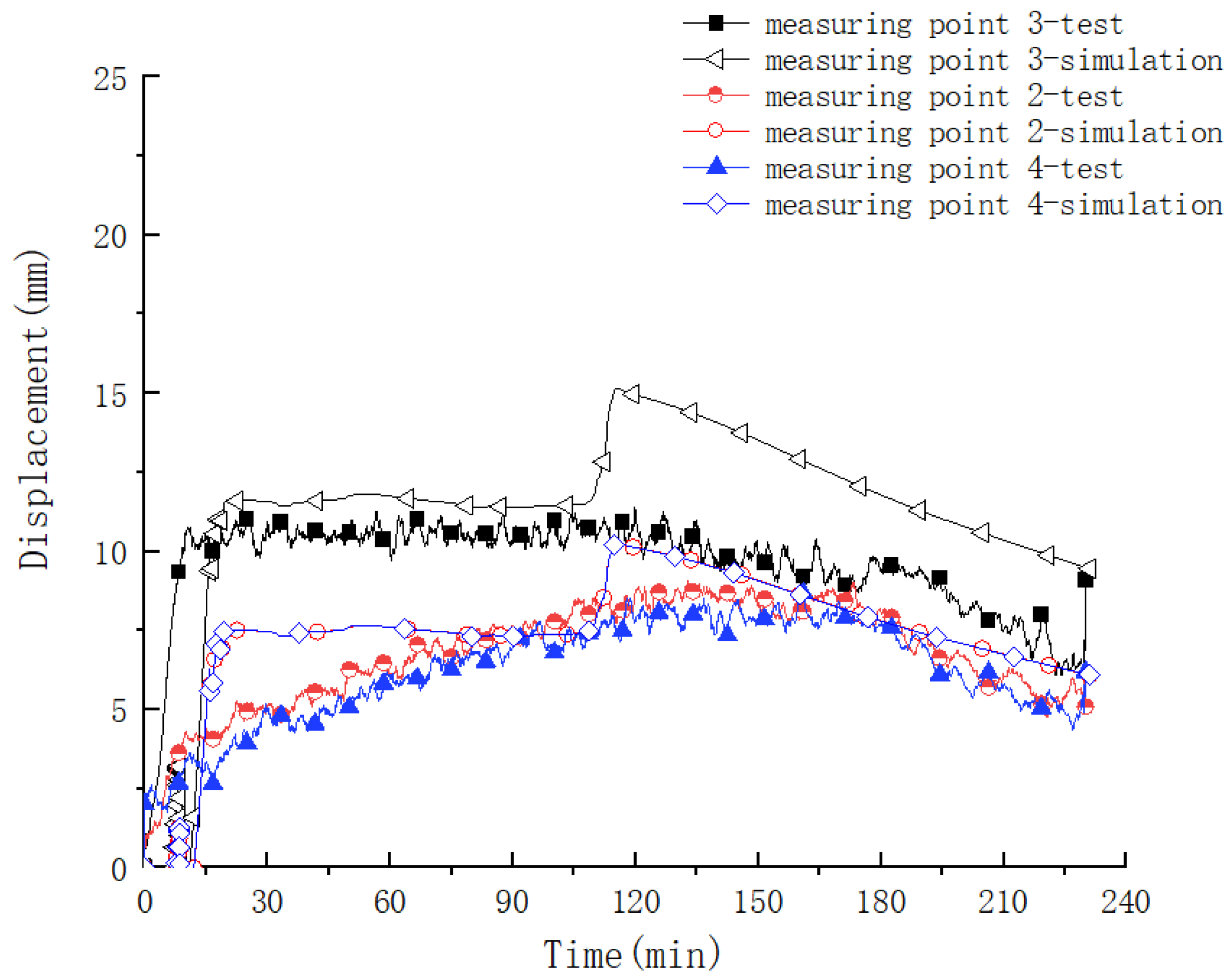
4.2.2. Variation Rule of Roof Displacement
5. Numerical Simulation Research on Mechanical Behavior of Steel–Concrete–Steel Composite Structure
5.1. Material Parameters
5.1.1. Thermal Parameters
5.1.2. Heat Transfer Parameters
5.1.3. Material Constitutive Relations at High Temperature
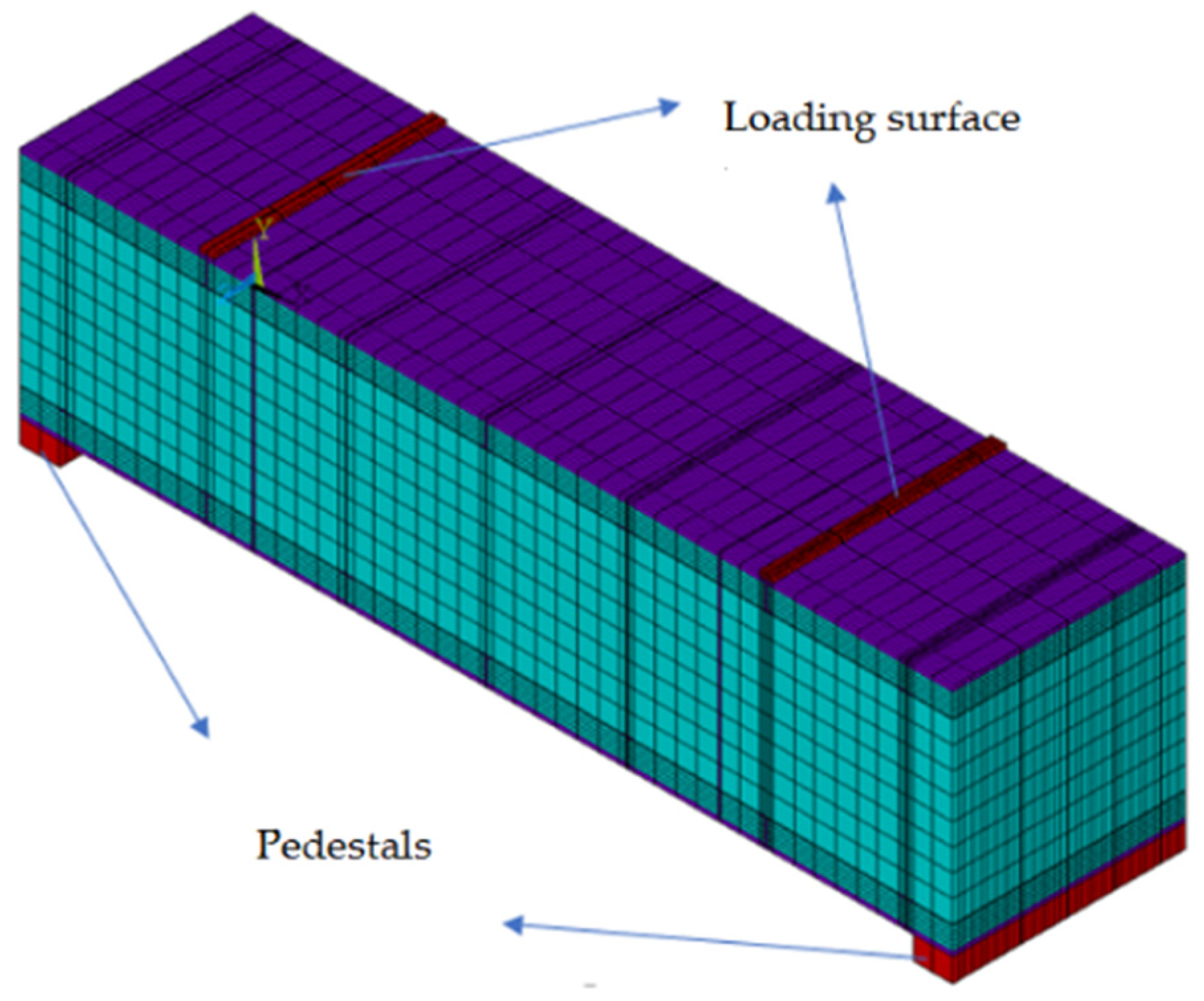
5.2. Finite Element Model
- Steel and concrete are homogeneous and isotropic structural materials, and their thermal parameters such as thermal conductivity and specific heat capacity are the same in each direction at the same temperature;
- A complete heat transfer between steel and concrete, ignoring the influence of contact thermal resistance;
- The influence of structural high-temperature mechanical response on structure temperature field distribution is not considered;
5.3. Comparative Analysis of Physical Model Test and Numerical Simulation Results
- (1)
- Comparative analysis of temperature transfer laws
- (2)
- Contrastive analysis of roof deformation laws
- (3)
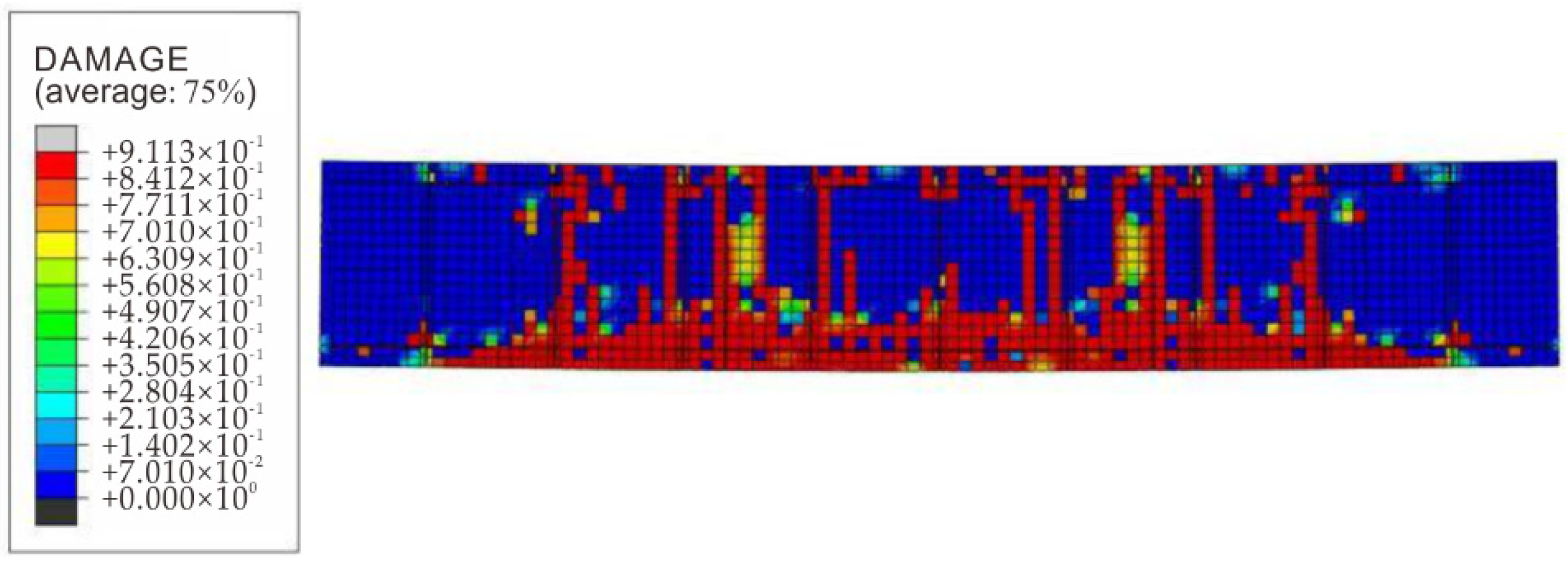
- Distribution analysis of structural plastic zone under a high temperature of fire
- (4)
- Analysis of structural crack distribution under a high temperature of fire
6. Conclusions
- (1)
- Under the RABT standard temperature rise curve, the area directly affected by the high temperature under fire in the steel–concrete–steel composite structure was within a range of about 10 cm from the fire surface, and within 3 cm away from the fire surface, the concrete showed a powdered state; the crack distribution modes in the composite structures under thermal–mechanical coupling were given.
- (2)
- The temperature transfer law of the measuring points at different depths of the combined structure were given: the temperature distribution of the measuring points closer to the fire surface presented a stepped distribution, and there was an obvious heating and cooling stage; the temperature of the far measuring points showed a continuous growth law due to the hysteresis effect of temperature transfer; the results of the numerical simulation and physical model test were compared to verify the temperature transfer law in the composite structure under a high temperature of fire.
- (3)
- The evolution law of the roof displacement of the composite structure was given: the overall deformation trend of the structural component under the action of thermal–mechanical coupling was that the middle sank and the two ends upturned. Affected by the high temperature of the fire, the roof deformation presented three distinct stages with time: a rapid growth stage, a deformation stabilization stage, and a deformation recovery stage. The results of the numerical simulation and physical model test were compared to verify the mechanical response mechanism of composite structure under the high temperature of fire.
- (4)
- The high temperature of fire had limited impact on the plastic development of the whole structure. The plastic zone was concentrated in the lower flange near the fire surface, and the plastic zone of the other parts was less distributed.
- (5)
- The composite structure presented a shear failure mode as a whole: the crack distribution of the whole component had good symmetry, a 45° oblique crack appeared on the line connecting the beam end support and the loading point, and the midspan cracks developed vertically.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hong, K.R.; Feng, H.H. Development and thinking of tunnels and underground engineering in China in recent 2 years (from 2019 to 2020). Tunn. Constr. 2021, 41, 1259–1280. [Google Scholar]
- Hong, K.R.; Feng, H.H. Development trends and views of highway tunnels in China over the past decade. China J. Highw. Transp. 2020, 33, 62–76. [Google Scholar]
- Chen, X.S.; Xu, Z.H.; Bao, X.H.; Wang, X.T.; Fu, Y.B. Challenges and technological breakthroughs in tunnel construction in China. China J. Highw. Transp. 2020, 33, 1–14. [Google Scholar]
- Lin, W.; Liang, J.Z.; Liu, L.F.; Zou, W.; Lin, M. Development and prospect of immersed tunnel and artificial technology. Tunn. Constr. 2020, 41, 2029–2036. [Google Scholar]
- Liu, L.F.; Lin, W.; Yin, H.Q.; Liu, Y.P.; Zou, W.; Xu, S.Y.; Ji, Y.N. Construction of immersed tunnel engineering in the world and development status of immersed tunnel technology in China. Cina Harb. Eng. 2021, 41, 71–79. [Google Scholar]
- Chen, J.Q.; Feng, X.Y.; Wei, H.; Feng, H.H. Statistics of underwater tunnels in China. Tunn. Constr. 2021, 41, 483–516. [Google Scholar]
- Chen, Y. Application and developing trends of immersed tunnel. Tunn. Constr. 2017, 37, 387–393. [Google Scholar]
- Zhang, E.Q. Technical Study on Fire Protection for Joints and Segments of Undersea Immersed Tube Tunnel; Chongqing Jiaotong University: Chongqing, China, 2013. [Google Scholar]
- Zhang, M.X.; Huang, J.; He, X.Q. Coupling analysis of thermal field and stress field for immersed tunnels under fire load. China Civ. Eng. J. 2007, 40, 83–87. [Google Scholar]
- Zhang, Z.Y. Research of Mechanics Behavior for Immersed Tunnel under Fire Load; Chongqing Jiaotong University: Chongqing, China, 2013. [Google Scholar]
- Lennon, T.; Moore, D. The natural fire safety concept—full-scale tests at Cardington. Fire Saf. J. 2003, 38, 623–643. [Google Scholar] [CrossRef]
- Wang, W.Y.; Engelhardt, M.D.; Li, G.Q.; Huang, G.S. Behavior of Steel-Concrete Partially Composite Beams Subjected to Fire-Part 1: Experimental Study. Fire Technol. 2017, 53, 1039–1058. [Google Scholar] [CrossRef]
- Wang, W.Y.; Wang, K.; Engelhardt, M.D.; Li, G. Behavior of Steel-Concrete artially Composite Beams Subjected to Fire-Part 2: Analytical Study. Fire Technol. 2017, 53, 1147–1170. [Google Scholar] [CrossRef]
- Zhou, H.Y.; Li, G.Q.; Wang, Y.Z. Parametric studies on fire-resistance of simaply-supported steel-concrete composite beams. Steel Struct. 2005, 06, 97–101. [Google Scholar]
- Wang, W.Y.; Li, G.Q.; Chen, L.Z.; Jiang, S.; Huang, G. Experimental study on fire resistance of steel bar truss slab and steel composite beams. China Civ. Eng. J. 2015, 48, 67–75. [Google Scholar]
- Ma, Q.J. Investigation on the Fire Resistance of Composite Cellular Beams; Shandong University: Jinan, China, 2014. [Google Scholar]
- Han, Y.L. Experimental Research on Mechanical Property of Stainless Steel-Concrete Composite Beams in Fire; Southeast University: Nanjing, China, 2020. [Google Scholar]
- Li, G.Q.; Wang, W.Y.; Zhou, H.Y. Simplified approach for fire-resistance design of simple-supported composite beams. Build. Struct. 2010, 40, 35–38. [Google Scholar]
- Song, T.Y.; Han, L.H. Some new developments of the fire performance research on composite structures. Eng. Mech. 2008, 25, 230–253. [Google Scholar]
- Song, S.Y.; Nie, J.G.; Xu, G.P.; Fan, J.S.; Tang, L.; Guo, Y.T. Development and application of steel-concrete-steel composite structures in immersed tunnels. China Civ. Eng. J. 2019, 52, 109–120. [Google Scholar]
- ANSI/AISC 360-10; Specification for Structural Steel Buildings. American Institute of Steel Construction: Chicago, IL, USA, 2010.
- Lie, T.T. A Procedure to calculate fire resistance of structural members. Fire. Mater. 1984, 8, 40–48. [Google Scholar] [CrossRef]
- BS EN 1992-1-2:2004; Eurocode 2: Design of Concrete Structures, Part 1-2: General Rules—Structural Fire Design. British Standards Institution: London, UK, 2004.
- National Standarad of the People’s Republic of China GB 51249-2017; Code for Fire Safety of Steel Structures in Buildings. Ministry of Housing and Urban-Rural Development of the Peoples’s Republic of China: Beijing, China, 2017.










| Crack Number | Crack Type | Development Direction | Crack Length (cm) |
|---|---|---|---|
| 1 | Vertical crack | Vertical | 34 |
| 2 | Oblique crack | Slanted 45° inward | 57 |
| 3 | Oblique crack | Slanted 45° inward | 57 |
| 4 | Vertical crack | Vertical | 34 |
| 5 | Vertical crack | Vertical | 34 |
| 6 | Oblique crack | Obliquely outward | 17 |
| 7 | Oblique crack | Obliquely outward | 9 |
| 8 | Oblique crack | Obliquely outward | 21 |
| 9 | Oblique crack | Obliquely outward | 11 |
| 10 | Horizontal crack | Longitudinal along the component | 210 |
| Material | Density kg/m3 | Heat Transfer Coefficient w/m/°C | Specific Heat Capacity J/m/°C |
|---|---|---|---|
| Steel | 7850 | 45.0 | 600 |
| Concrete | 2300 | With the change of temperature, Equation (1) | With the change of temperature, Equation (2) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, P.; Hu, X.; Liu, E.; Chen, J.; Jiang, S.; Ding, H. Research on Mechanical Behavior of the Steel–Concrete–Steel Composite Structures Subjected to High Temperature of Fire. Materials 2022, 15, 4872. https://doi.org/10.3390/ma15144872
Cao P, Hu X, Liu E, Chen J, Jiang S, Ding H. Research on Mechanical Behavior of the Steel–Concrete–Steel Composite Structures Subjected to High Temperature of Fire. Materials. 2022; 15(14):4872. https://doi.org/10.3390/ma15144872
Chicago/Turabian StyleCao, Peng, Xuebing Hu, Enlong Liu, Jianzhong Chen, Shouchao Jiang, and Hao Ding. 2022. "Research on Mechanical Behavior of the Steel–Concrete–Steel Composite Structures Subjected to High Temperature of Fire" Materials 15, no. 14: 4872. https://doi.org/10.3390/ma15144872
APA StyleCao, P., Hu, X., Liu, E., Chen, J., Jiang, S., & Ding, H. (2022). Research on Mechanical Behavior of the Steel–Concrete–Steel Composite Structures Subjected to High Temperature of Fire. Materials, 15(14), 4872. https://doi.org/10.3390/ma15144872






