PTFE Crystal Growth in Composites: A Phase-Field Model Simulation Study
Abstract
:1. Introduction
2. Methods
3. Results and Discussion
3.1. Effects of Polymer Intrinsic Properties
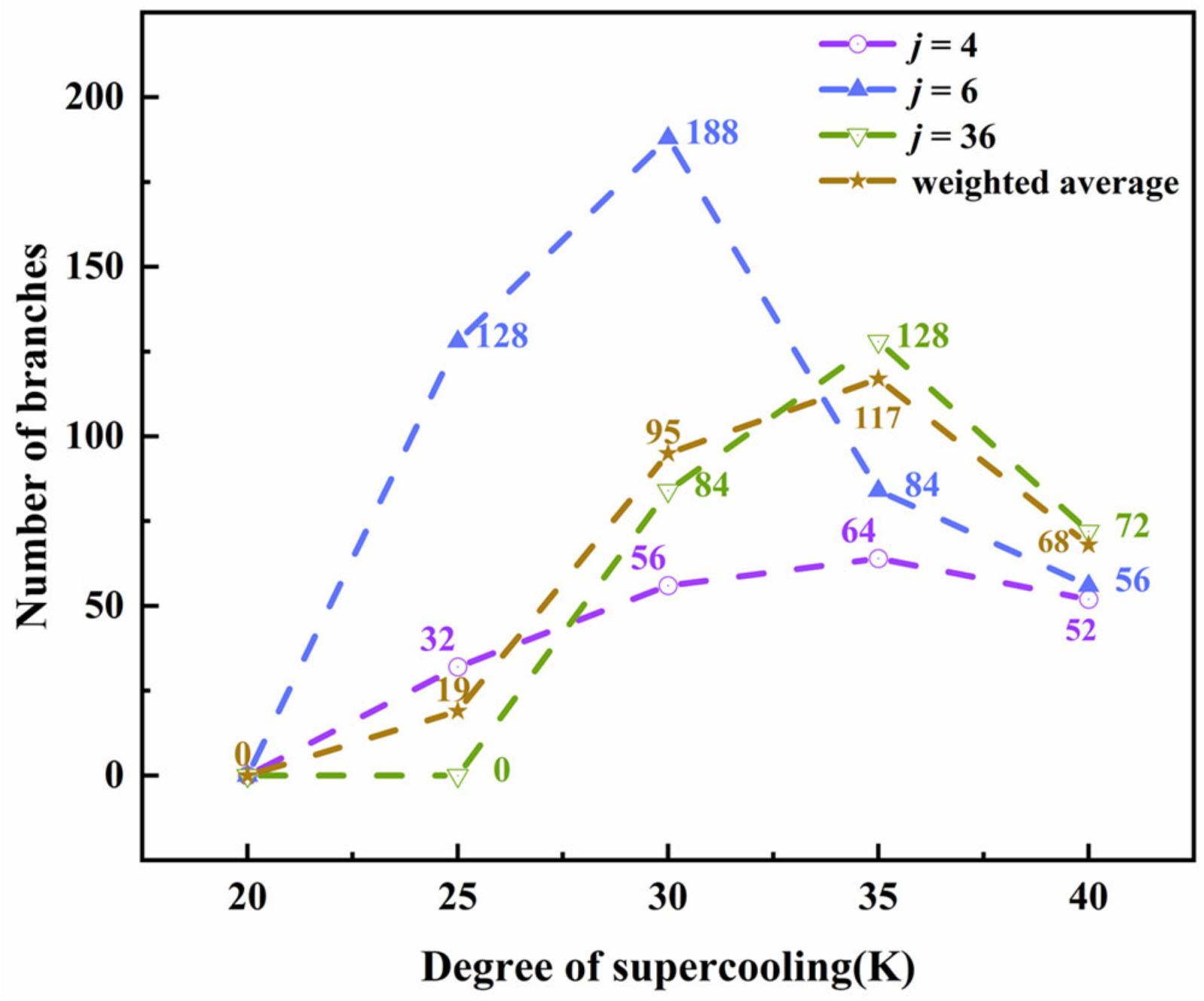
3.2. Effects of Crystallization Process Factors
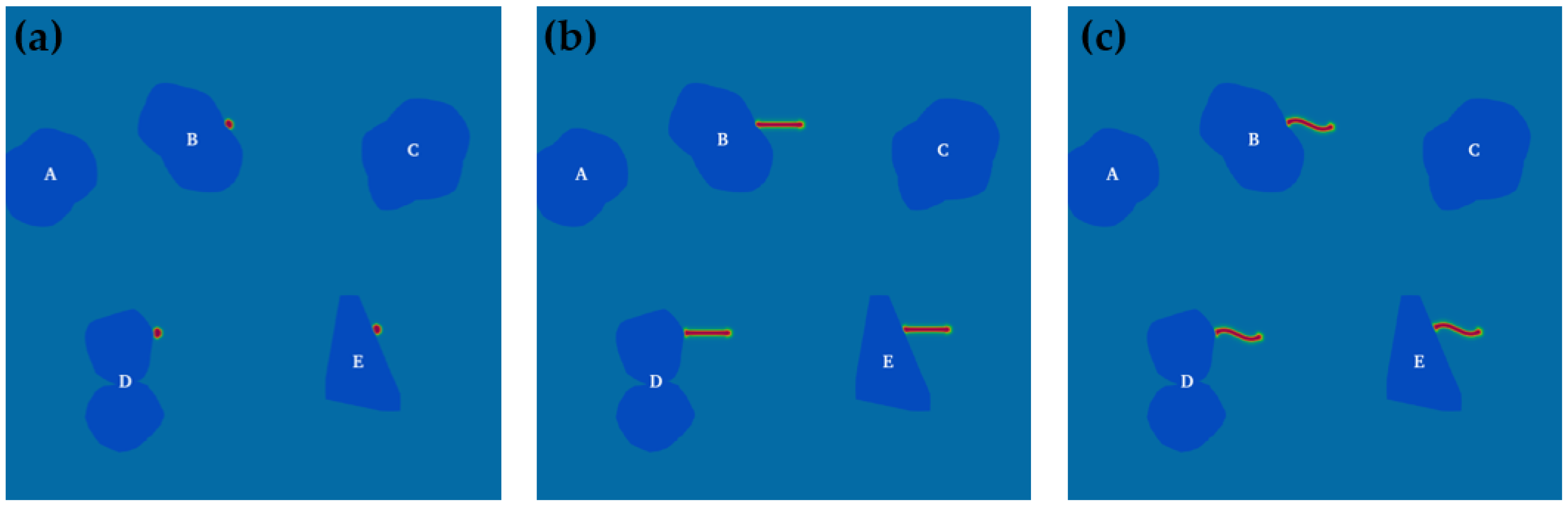
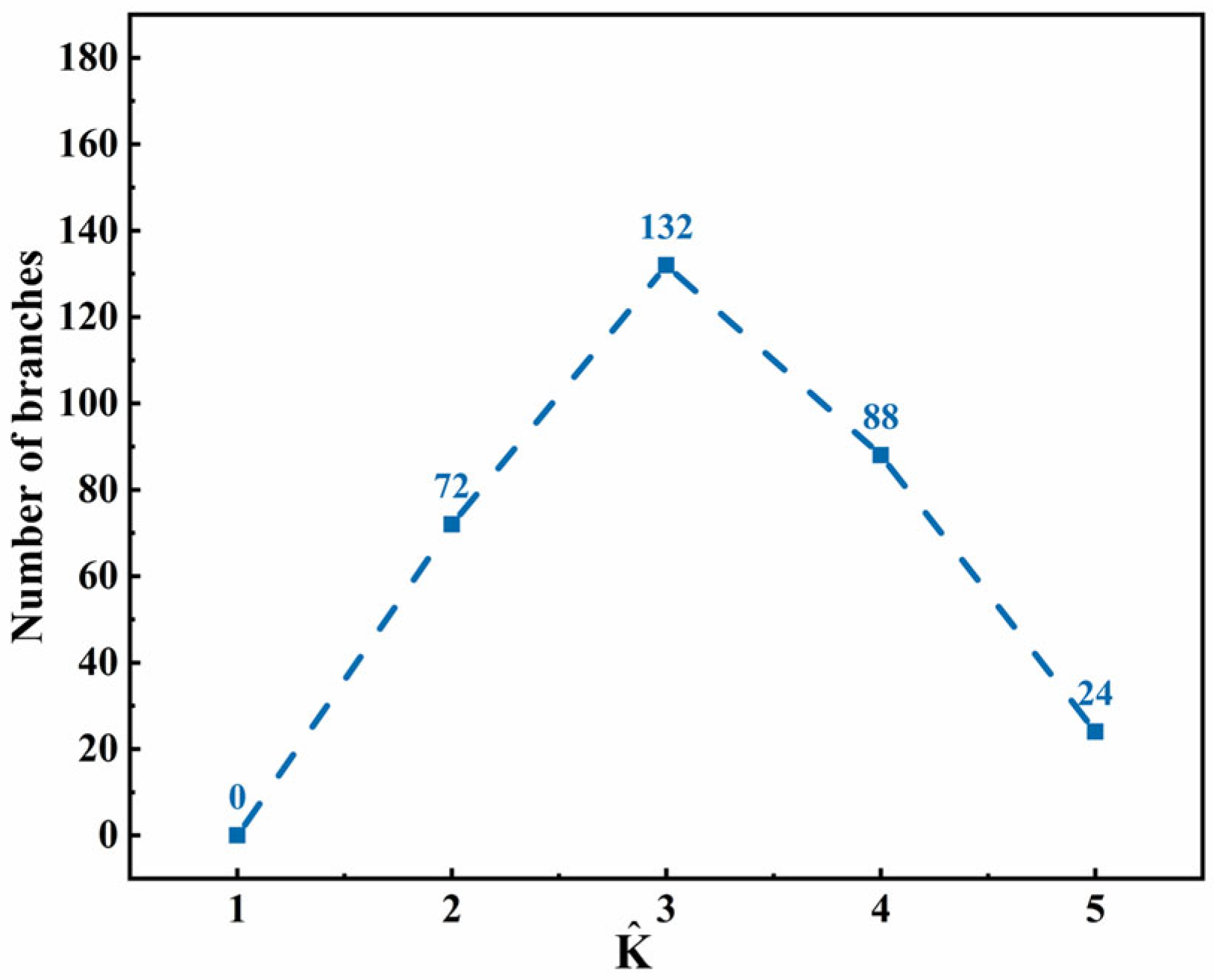
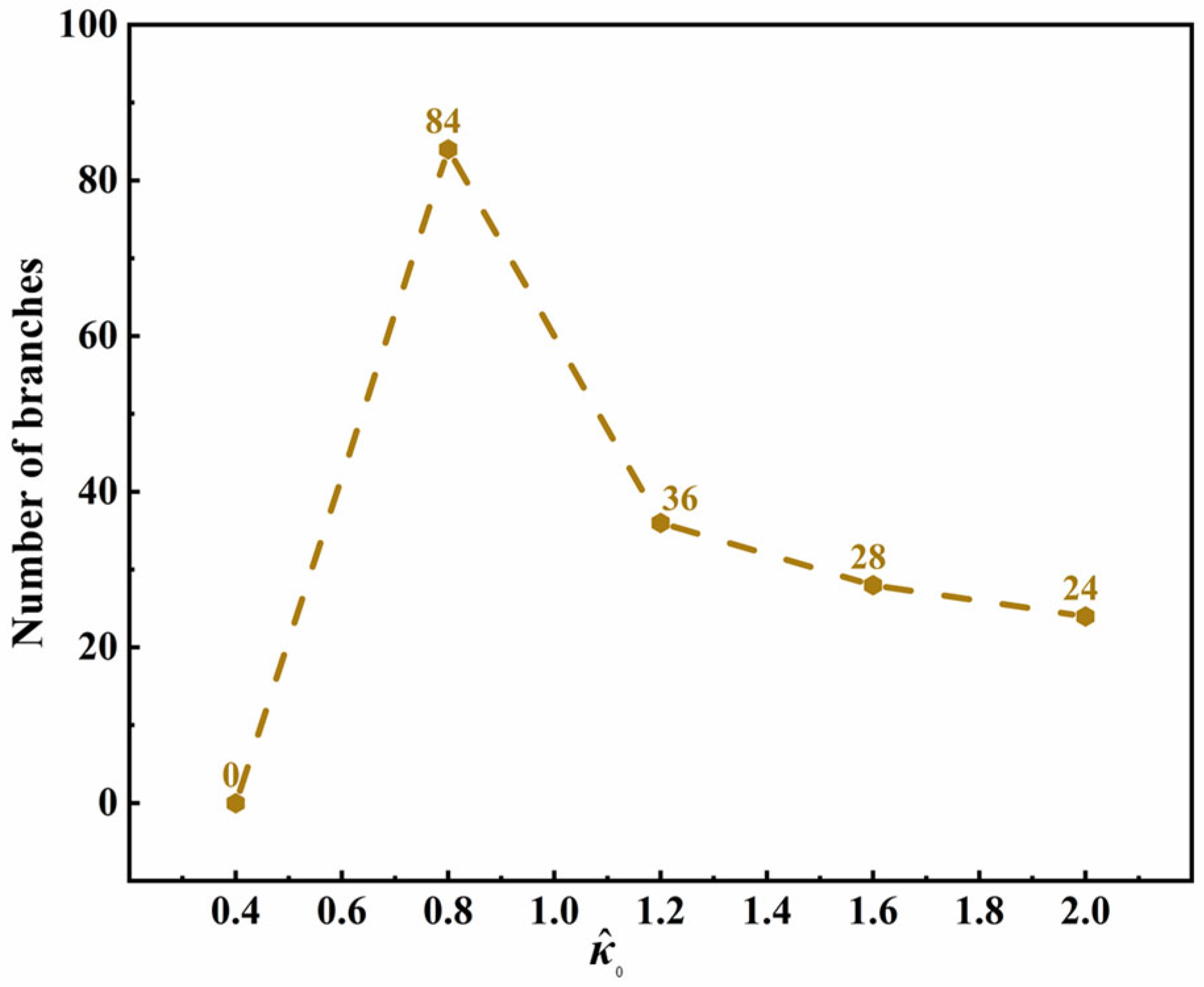
3.3. Effects of Biphasic Interface and Crystal Nucleus Shape
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xia, Z.F.; Wedel, A.; Danz, R. Charge storage and its dynamics in porous polytetrafluoroethylene (PTFE) film electrets. IEEE Trans. Dielectr. Electr. Insul. 2003, 10, 102–108. [Google Scholar]
- Li, Q.; Liu, P.; Mahmood, K. Improved properties of PTFE composites filled with glass fiber modified by sol-gel method. J. Mater. Sci. Mater. Electron. 2021, 32, 23090–23102. [Google Scholar]
- Apeldorn, T.; Keilholz, C.; Wolff-Fabris, F. Dielectric properties of highly filled thermoplastics for printed circuit boards. J. Appl. Polym. Sci. 2013, 128, 3758–3770. [Google Scholar] [CrossRef]
- Luan, B.C.D.; Huang, J.; Zhang, J. Enhanced thermal conductivity and wear resistance of polytetrafluoroethylene composites through boron nitride and zinc oxide hybrid fillers. J. Appl. Polym. Sci. 2015, 132, 30. [Google Scholar] [CrossRef]
- Peng, G.R.; Guo, Z.L.; Zeng, P.Z. Study on glass fabric reinforced polytetrafluoroethylene composites infused with melted cyanate ester resin. J. Reinf. Plast. Compos. 2009, 28, 2221–2230. [Google Scholar] [CrossRef]
- Atta, A. Enhanced dielectric properties of flexible Cu/polymer nanocomposite films. Surf. Innov. 2021, 9, 17–24. [Google Scholar] [CrossRef]
- Zhao, W.; Su, Y.; Müller, A.J. Direct relationship between interfacial microstructure and confined crystallization in poly (ethylene oxide)/silica composites: The study of polymer molecular weight effects. J. Polym. Sci. Pt. B-Polym. Phys. 2017, 55, 1608–1616. [Google Scholar] [CrossRef]
- Poerschke, D.L.; Braithwaite, A.; Park, D. Crystallization behavior of polymer-derived Si-OC for ceramic matrix composite processing. Acta Mater. 2018, 147, 329–341. [Google Scholar] [CrossRef]
- Li, Y.; Han, C.; Yu, Y. Isothermal and nonisothermal cold crystallization kinetics of poly (l-lactide)/functionalized eggshell powder composites. J. Therm. Anal. Calorim. 2018, 131, 2213–2223. [Google Scholar] [CrossRef]
- Liu, Y.; Qiu, Z. Enhanced melt crystallization of biobased Poly (ethylene 2, 5-furandicarboxylate) by low loading of octavinyl-polyhedral oligomeric silsesquioxanes. Compos. Commun. 2020, 19, 20–24. [Google Scholar] [CrossRef]
- Chang, B.; Schneider, K.; Lu, B. Accelerating shear-induced crystallization and enhancing crystal orientation of isotactic-polypropylene via nucleating agent self-assembly. Polymer 2018, 158, 213–222. [Google Scholar] [CrossRef]
- Gohn, A.M.; Seo, J.; Ferris, T. Quiescent and flow-induced crystallization in polyamide 12/cellulose nanocrystal composites. Thermochim. Acta 2019, 677, 99–108. [Google Scholar] [CrossRef]
- Wang, J.; Kim, H.K.; Shi, F.G. Thickness dependence of morphology and mechanical properties of on-wafer low-k PTFE dielectric films. Thin Solid Films 2000, 377, 413–417. [Google Scholar] [CrossRef]
- Bosq, N.; Guigo, N.; Zhuravlev, E. Nonisothermal crystallization of polytetrafluoroethylene in a wide range of cooling rates. J. Phys. Chem. B 2013, 117, 3407–3415. [Google Scholar] [CrossRef] [PubMed]
- Radusch, H.J. Analysis of reversible melting in polytetrafluoroethylene. J. Therm. Anal. Calorim. 2005, 79, 615–621. [Google Scholar] [CrossRef]
- Symons, N.K.J. Crystals of polytetrafluoroethylene grown from solution. J. Polym. Sci. 1961, 51, 21–25. [Google Scholar] [CrossRef]
- Melillo, L.; Wunderlich, B. Extended-chain crystals. Colloid Polym. Sci. 1972, 250, 417–425. [Google Scholar] [CrossRef]
- Khatipov, S.A.; Serov, S.A.; Sadovskaya, N.V. Morphology of polytetrafluoroethylene before and after irradiation. Radiat. Phys. Chem. 2012, 81, 256–263. [Google Scholar] [CrossRef]
- Laird, E.D.; Bose, R.K.; Wang, W. Carbon nanotube-directed polytetrafluoroethylene crystal growth via initiated chemical vapor deposition. Macromol. Rapid Commun. 2013, 34, 251–256. [Google Scholar] [CrossRef]
- Choy, C.L. Thermal conductivity of polymers. Polymer 1977, 18, 984–1004. [Google Scholar] [CrossRef]
- Choy, C.L.; Wong, Y.W.; Yang, G.W. Elastic modulus and thermal conductivity of ultradrawn polyethylene. J. Polym. Sci. Pt. B-Polym. Phys. 1999, 37, 3359–3367. [Google Scholar] [CrossRef]
- Dong, S.Y.; Zhu, P.; Liu, J.G. Thermal Treatment Effects on the Microstructure and Tensile Properties of Transparent Polyamides. Acta Polym. Sin. 2019, 50, 189–198. [Google Scholar]
- Ding, Y.C.; Hou, H.Q.; Zhao, Y. Electrospun polyimide nanofibers and their applications. Prog. Polym. Sci. 2016, 61, 67–103. [Google Scholar] [CrossRef]
- Singh, V.; Bougher, T.L.; Weathers, A. High thermal conductivity of chain-oriented amorphous polythiophene. Nat. Nanotechnol. 2014, 9, 384–390. [Google Scholar] [CrossRef]
- Sung, D.H.; Kim, M.; Park, Y.B. Prediction of thermal conductivities of carbon-containing fiber-reinforced and multiscale hybrid composites. Compos. Pt. B-Eng. 2018, 133, 232–239. [Google Scholar] [CrossRef]
- Bian, P.L.; Verestek, W.; Yan, S. A multiscale modeling on fracture and strength of graphene platelets reinforced epoxy. Eng. Fract. Mech. 2020, 235, 107197. [Google Scholar] [CrossRef]
- Fang, W.J.; Yang, X.J.; Li, Q. Improved mode I interlaminar fracture toughness of random polypropylene composite laminate via multiscale reinforcing formed by introducing functional nanofibrillated cellulose. Compos. Pt. B-Eng. 2020, 203, 108481. [Google Scholar] [CrossRef]
- Moelans, N.; Blanpain, B.; Wollants, P. An introduction to phase-field modeling of microstructure evolution. Calphad-Comput. Coupling Ph. Diagrams Thermochem. 2008, 32, 268–294. [Google Scholar] [CrossRef]
- Kyu, T.; Chiu, H.W.; Guenthner, A.J. Rhythmic growth of target and spiral spherulites of crystalline polymer blends. Phys. Rev. Lett. 1999, 83, 2749. [Google Scholar] [CrossRef]
- Xu, H.; Matkar, R.; Kyu, T. Phase-field modeling on morphological landscape of isotactic polystyrene single crystals. Phys. Rev. E 2005, 72, 011804. [Google Scholar] [CrossRef]
- Schultz, J.M. Self-generated fields and polymer crystallization. Macromolecules 2012, 45, 6299–6323. [Google Scholar] [CrossRef]
- Wang, X.D.; Ou, Y.J.; Su, J. A phase-field model for simulating various spherulite morphologies of semi-crystalline polymers. Chin. Phys. B 2013, 22, 106103. [Google Scholar] [CrossRef]
- Wang, X.D.; Ou, Y.J.; Su, J. Phase field modeling of the ring-banded spherulites of crystalline polymers: The role of thermal diffusion. Chin. Phys. B 2014, 23, 126103. [Google Scholar] [CrossRef]
- Wang, X.D.; Ou, Y.J.; Su, J. Investigating the role of oriented nucleus in polymer shish-kebab crystal growth via phase-field method. J. Chem. Phys. 2014, 140, 114102. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.D.; Ou, Y.J.; Zhou, W. A phase field technique for modeling and predicting flow induced crystallization morphology of semi-crystalline polymers. Polymers 2016, 8, 230. [Google Scholar] [CrossRef]
- Wang, X.D.; Ou, Y.J.; Liu, Y. Prediction of Flow Effect on Crystal Growth of Semi-Crystalline Polymers Using a Multi-Scale Phase-Field Approach. Polymers 2017, 9, 634. [Google Scholar] [CrossRef]
- Liu, F.; Shen, J. Stabilized semi-implicit spectral deferred correction methods for Allen-Cahn and Cahn-Hilliard equations. Math. Meth. Appl. Sci. 2015, 38, 4564–4575. [Google Scholar] [CrossRef]
- Hohenberg, P.C.; Krekhov, A.P. An introduction to the Ginzburg-Landau theory of phase transitions and nonequilibrium patterns. Phys. Rep. Rev. Sec. Phys. Lett. 2015, 572, 1–42. [Google Scholar] [CrossRef]
- Harrowell, P.R.; Oxtoby, D.W. On the interaction between order and a moving interface: Dynamical disordering and anisotropic growth rates. J. Chem. Phys. 1987, 86, 2932–2942. [Google Scholar] [CrossRef]
- Costa, A.R.D.M.; Santos, R.M.; Ito, E.N. Melt and cold crystallization in a poly (3-hydroxybutyrate)poly(butylene adipate-co-terephthalate) blend. J. Therm. Anal. Calorim. 2019, 137, 1341–1346. [Google Scholar] [CrossRef]
- Wang, X.D.; Zhang, H.X.; Zhou, W. A 3D phase-field model for simulating the crystal growth of semi-crystalline polymers. Int. J. Heat Mass Transf. 2017, 115, 194–205. [Google Scholar] [CrossRef]
- Wu, S. Polymer Interface and Adhesion, 1st ed.; Routledge: New York, NY, USA, 2017; p. 66. [Google Scholar]
- Mark, J.E. Polymer Data Handbook, 1st ed.; Oxford University Press: New York, NY, USA, 1999; p. 842. [Google Scholar]
- Xu, H.J.; Chiu, H.W.; Okabe, Y. Breakup of spiral and concentric ringed spherulites in polymer crystallization. Phys. Rev. E 2006, 74, 011801. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.P.; Ye, Z.L.; Hu, X.M. Shape modification and deformation of parametric surfaces. Comput. Aided Drafting, Des. Manuf. 2002, 12, 1–7. [Google Scholar]
- Karma, A.; Rappel, W.J. Quantitative phase-field modeling of dendritic growth in two and three dimensions. Phys. Rev. E 1998, 57, 4323. [Google Scholar] [CrossRef] [Green Version]
- Sun, X.; Xue, B.; Yang, S.D. Structural conversion of PLLA/ZnO composites facilitated by interfacial crystallization to potential application in oil-water separation. Appl. Surf. Sci. 2020, 517, 146135. [Google Scholar] [CrossRef]
- Hohenberg, P.C.; Halperin, B.I. Theory of dynamic critical phenomena. Rev. Mod. Phys. 1977, 49, 435. [Google Scholar] [CrossRef]
- Landau, L.D. The theory of phase transitions. Nature 1936, 138, 840–841. [Google Scholar] [CrossRef]
- Hoffman, J.D.; Weeks, J.J. Melting process and the equilibrium melting temperature of polychlorotrifluoroethylene. J. Res. Natl. Bur. Stand. Sect. A 1962, 66, 13–28. [Google Scholar] [CrossRef]
- Housmans, J.W.; Peters, G.; Meijer, H. Flow-induced crystallization of propylene/ethylene random copolymers. J. Res. Natl. Inst. Stand. Technol. 2009, 98, 693–705. [Google Scholar] [CrossRef] [Green Version]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fan, M.; He, W.; Li, Q.; Zhou, J.; Shen, J.; Chen, W.; Yu, Y. PTFE Crystal Growth in Composites: A Phase-Field Model Simulation Study. Materials 2022, 15, 6286. https://doi.org/10.3390/ma15186286
Fan M, He W, Li Q, Zhou J, Shen J, Chen W, Yu Y. PTFE Crystal Growth in Composites: A Phase-Field Model Simulation Study. Materials. 2022; 15(18):6286. https://doi.org/10.3390/ma15186286
Chicago/Turabian StyleFan, Ming, Wenhao He, Qiangzhi Li, Jing Zhou, Jie Shen, Wen Chen, and Yuanying Yu. 2022. "PTFE Crystal Growth in Composites: A Phase-Field Model Simulation Study" Materials 15, no. 18: 6286. https://doi.org/10.3390/ma15186286







