Influence of Strain Gradient on Fatigue Life of Carbon Steel for Pressure Vessels in Low-Cycle and High-Cycle Fatigue Regimes
Abstract
:1. Introduction
2. Materials and Methods
2.1. Specimen Material
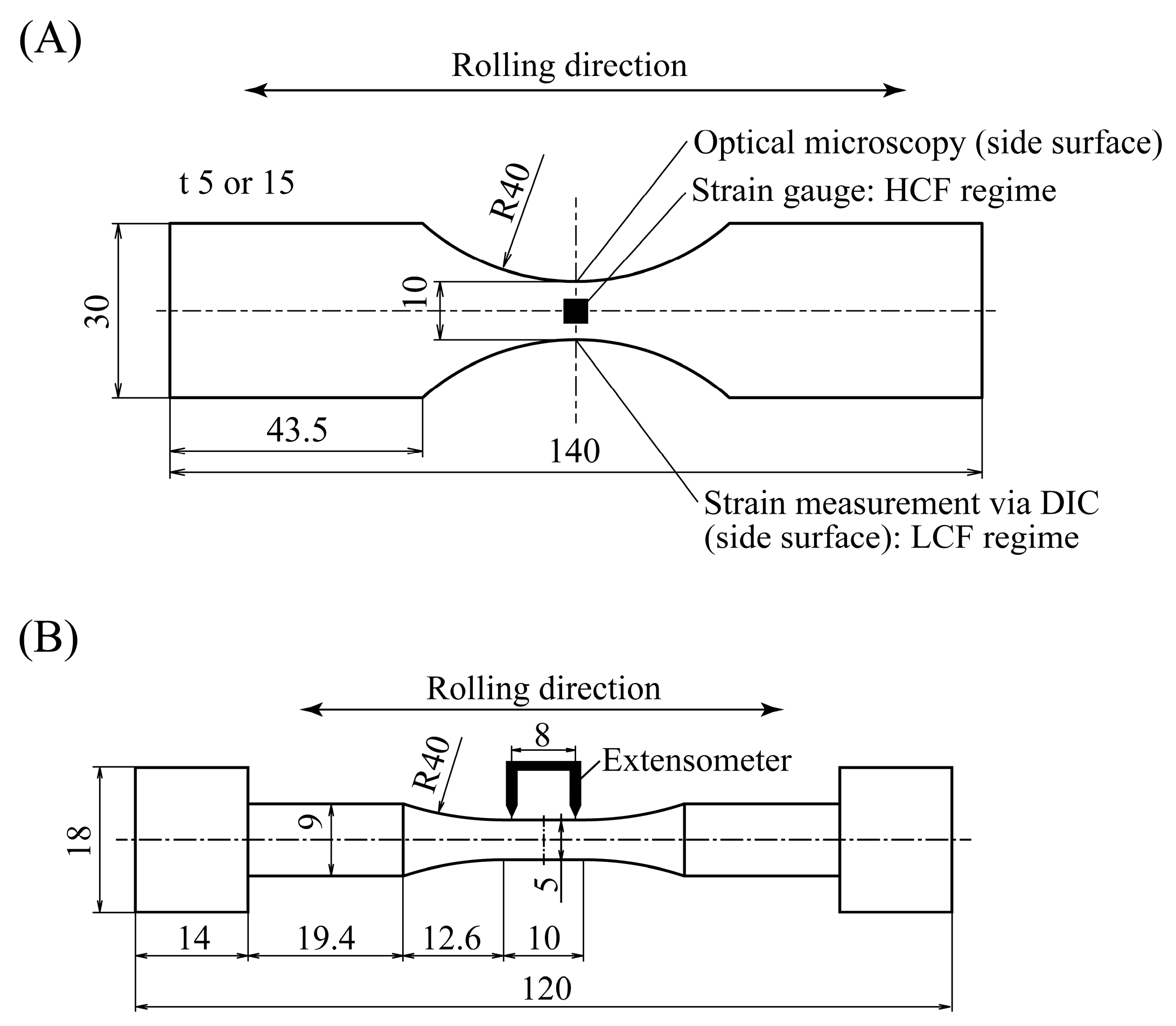
2.2. Alternating Bending Testing
2.3. Tension–Compression Testing
2.4. Fatigue Life Evaluation Based on Strain-Based Approach
3. Experimental Results and Discussion
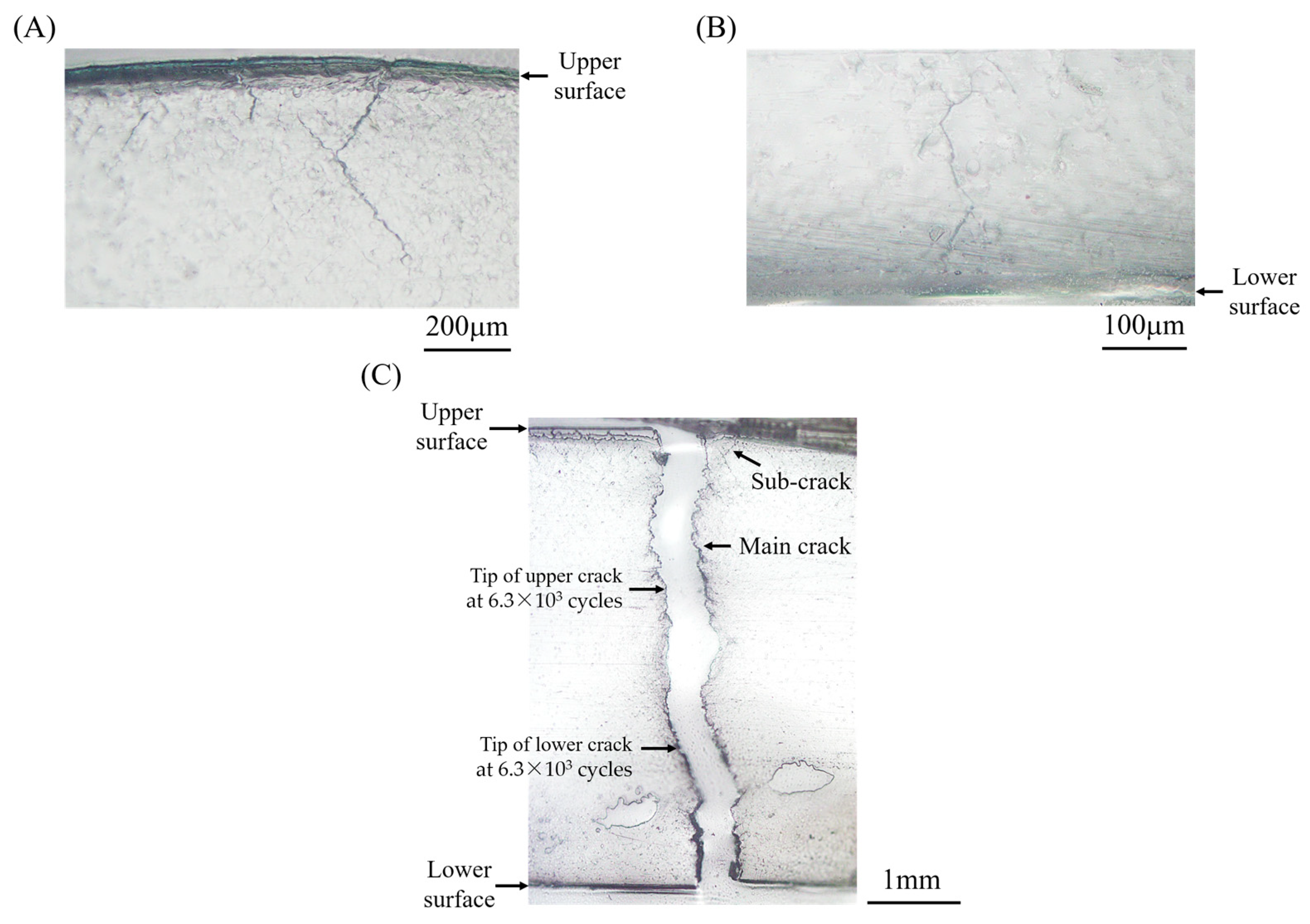
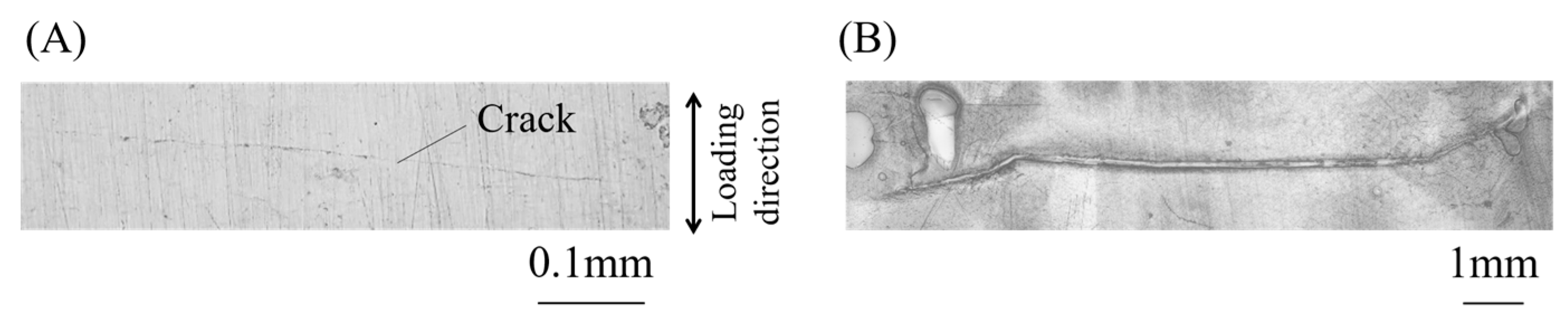
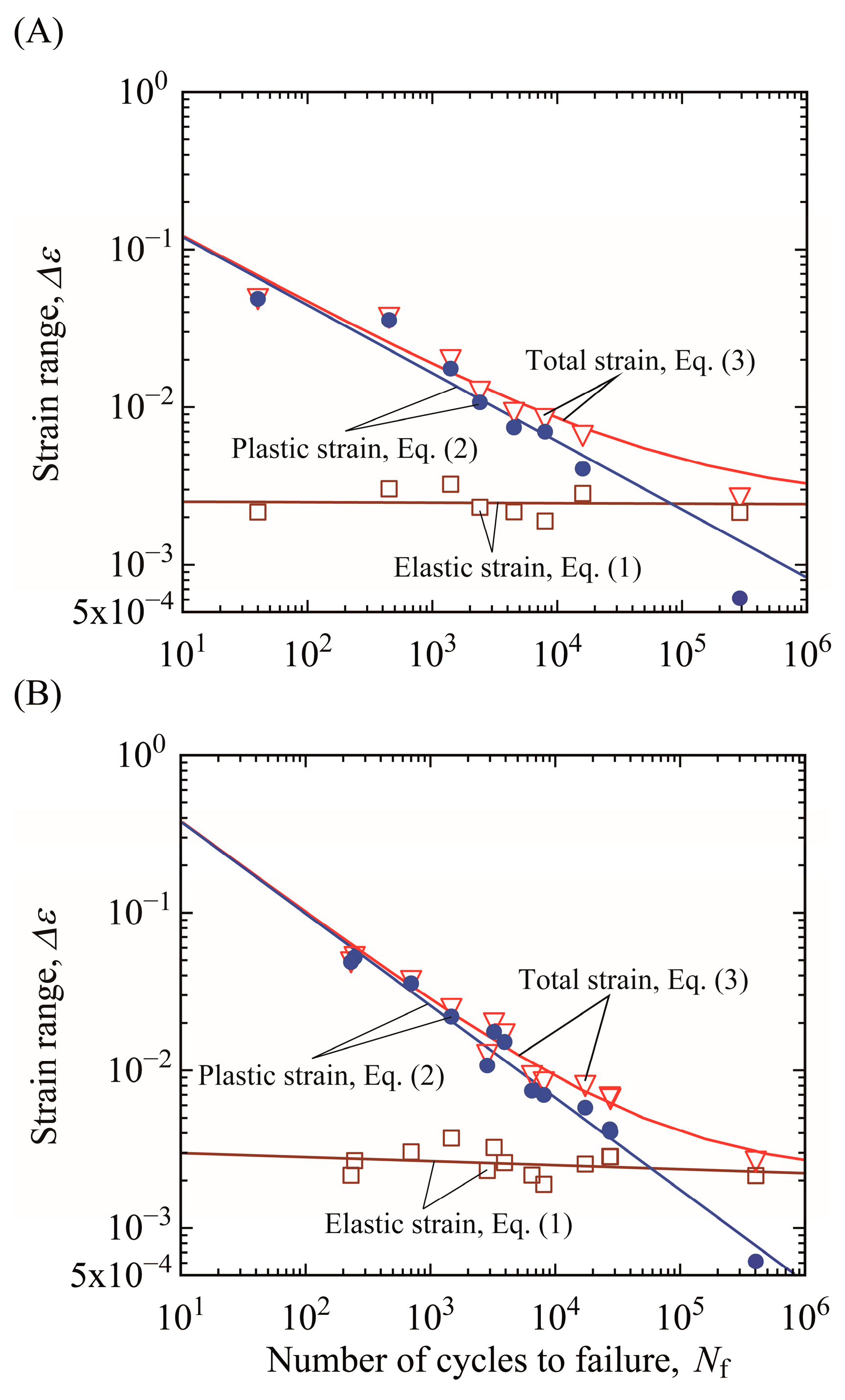
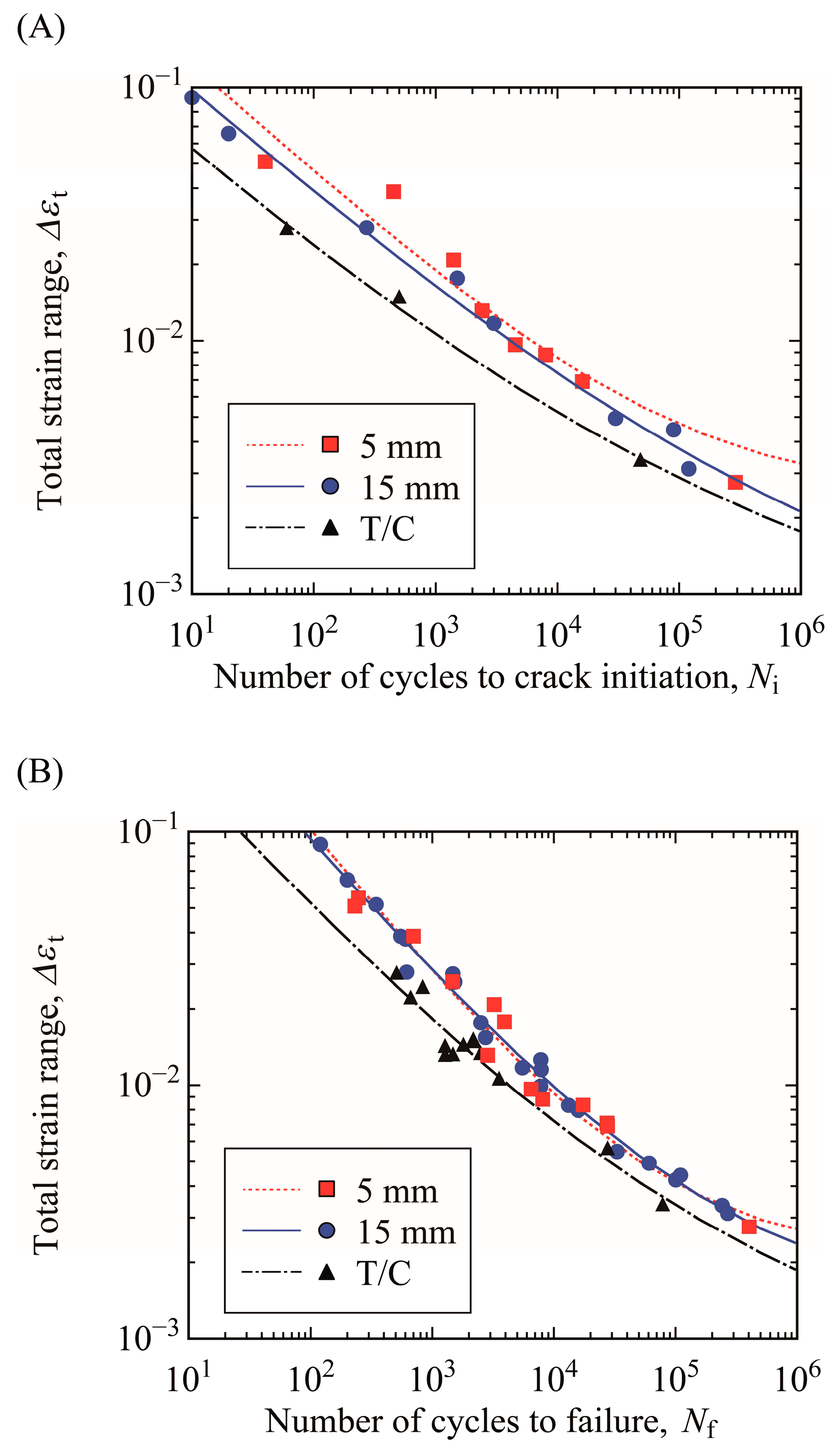
3.1. Evaluation of Crack Initiation and Total Failure Lives
3.2. Influence of Strain Gradient on Fatigue Life
4. Conclusions
- The strain-based approach based on the forms of the Basquin and Coffin–Manson equations was effective for evaluating fatigue life, irrespective of loading condition.
- The crack initiation life was affected by not only strain but also the strain gradient at the crack initiation site, and the crack initiation life increased with increasing strain gradient.
- The total failure life of the specimen subjected to cyclic T/C was the shortest, while the total failure life of the specimens with thicknesses of 5 and 15 mm subjected to alternating bending was almost the same. This is because the crack initiation life and crack propagation life become shorter and longer, respectively, with increasing thickness of the bending specimen.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A

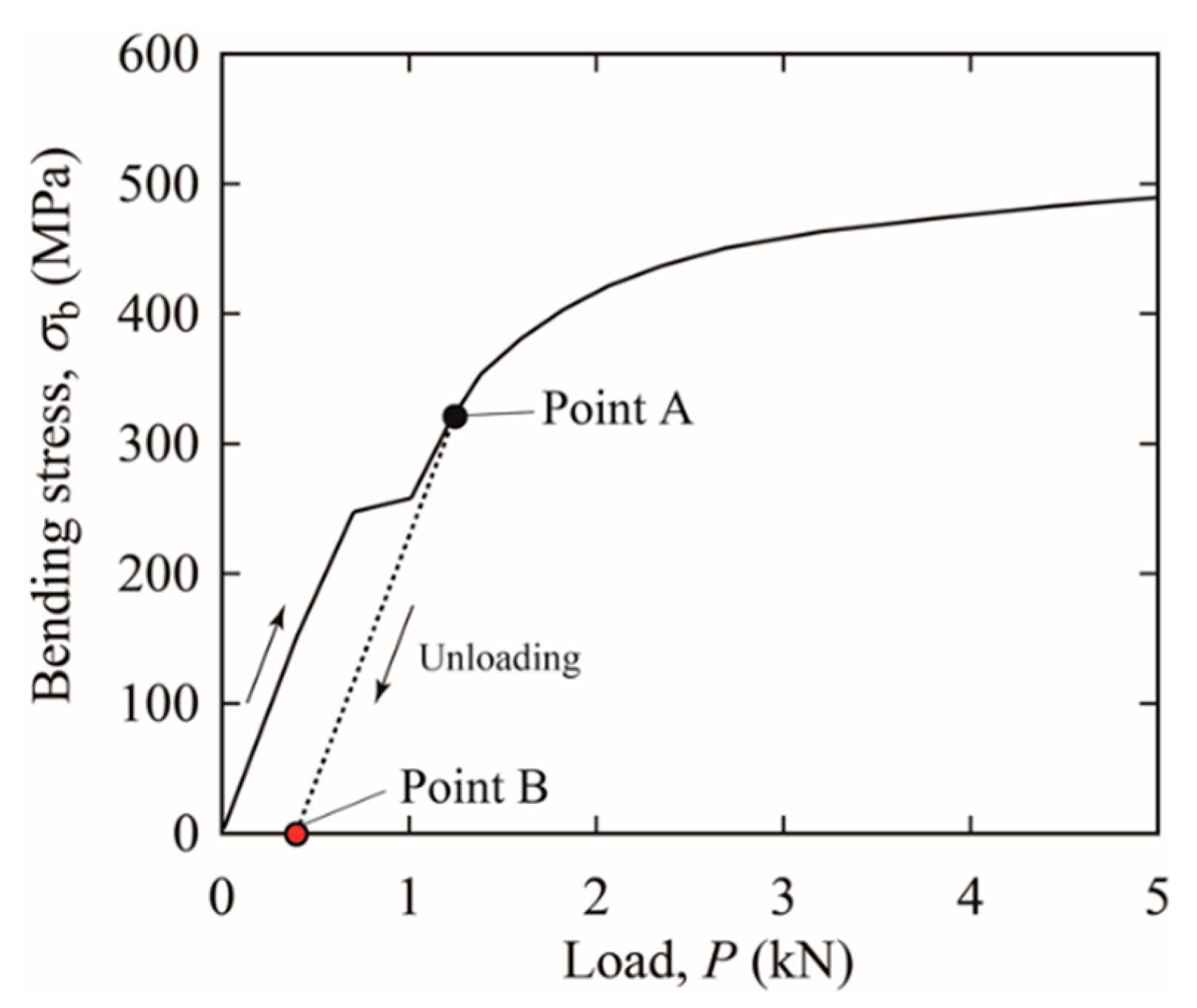

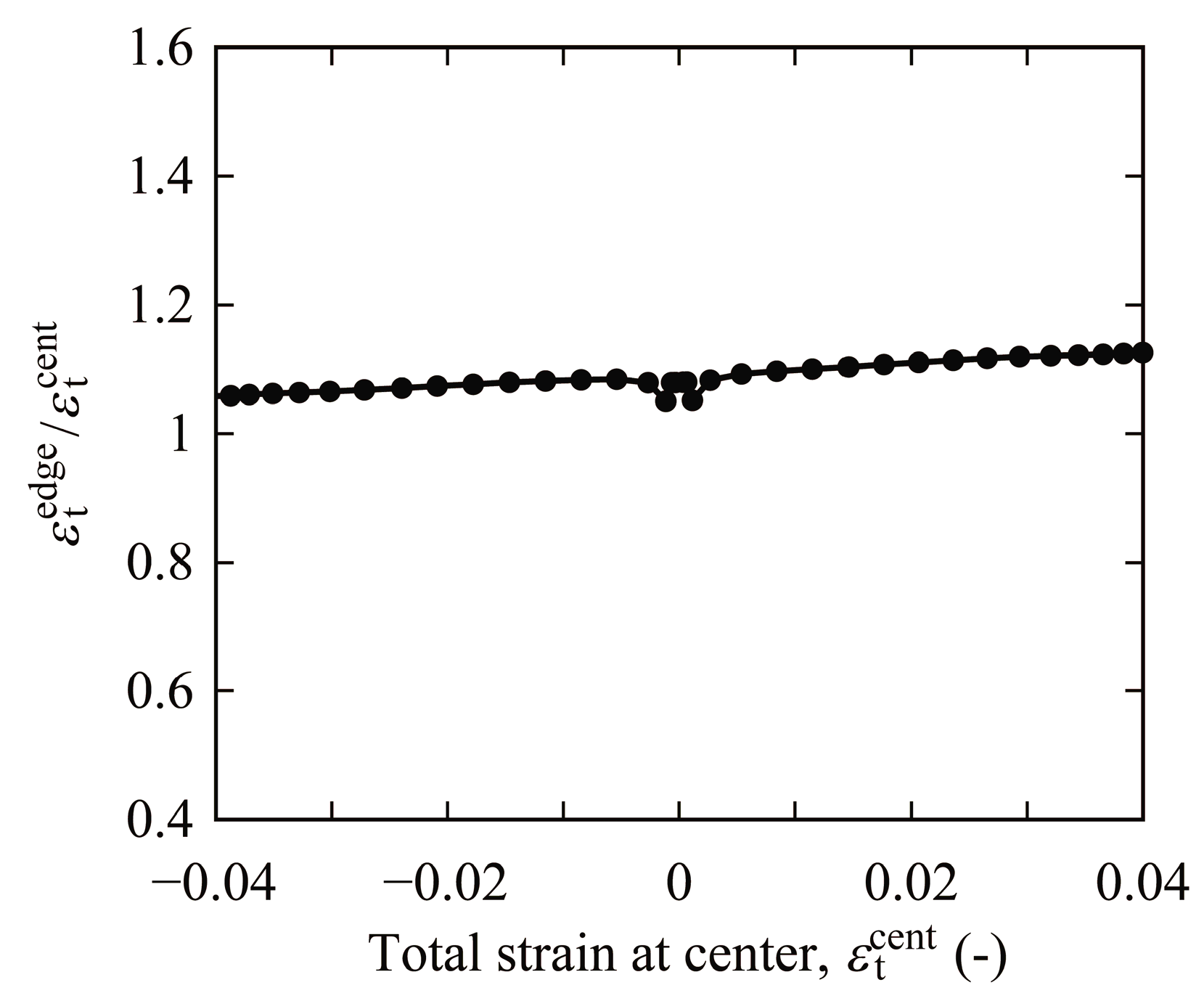
References
- Dowling, N.E. Local Strain Approach to Fatigue, Comprehensive Structural Integrity; Ritchie, R.O., Murakami, Y., Eds.; Elsevier Science: Amsterdam, The Netherlands, 2003; pp. 77–94. [Google Scholar]
- Kawashita, M.; Shimodaira, T.; Aoyama, H. Development of a method for fatigue life evaluation using strain gradient parameters. J. Soc. Mater. Sci. 2018, 67, 269–275. (In Japanese) [Google Scholar] [CrossRef] [Green Version]
- Qin, S.; Xiong, Z.; Ma, Y.; Zhang, K. Low cycle fatigue life evaluation of notched specimens considering strain gradient. Materials 2020, 13, 1001. [Google Scholar] [CrossRef] [Green Version]
- Peterson, R.E. Stress Concentration Factors; John Wiley & Sons: New York, NY, USA, 1974. [Google Scholar]
- Neuber, H. Theory of Notch Stresses: Principles for Exact Calculation of Strength with Reference to Structural Form and Material, 2nd ed.; Springer: Berlin, Germany, 1958; p. 292. [Google Scholar]
- Ishibashi, T. Fatigue of Metals and Prevention of Fracture; Yokendo: Tokyo, Japan, 1969. (In Japanese) [Google Scholar]
- Siebel, E.; Stieler, M. Significance of dissimilar stress distributions for cyclic loading. Zeitschr. VDI 1955, 97, 146–148. (In German) [Google Scholar]
- Ye, W.L.; Zhu, S.P.; Niu, X.P.; He, J.C.; Wang, Q. Fatigue life prediction of notched components under size effect using stress gradient-based approach. Int. J. Fract. 2021. [Google Scholar] [CrossRef]
- Kulesa, A.; Kurek, A.; Łagoda, T.; Achtelik, H.; Kluger, K. Low cycle fatigue of steel in strain controlled cyclic bending. Acta Mech. Autom. 2016, 10, 62–65. [Google Scholar]
- Kurek, A.; Koziarska, J.; Łagoda, T. The influence of the strain and stress gradient in determining strain fatigue characteristics for oscillatory bending. Materials 2020, 13, 173. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kurek, A. Using fatigue characteristics to analyses test results for 16Mo3 steel under tension-compression and oscillatory bending conditions. Materials 2020, 13, 1197. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yao, J.T.P.; Munse, W.H. Low-Cycle Fatigue of Metals—Literature Review, Serial no. SSC-137, First Progress Report of Project SR-149 to the Ship Structure Committee on Low-Cycle Fatigue of Metals—Literature Review; Defense Technical Information Center: Fort Belvoir, VA, USA, 1961.
- Basquin, O.H. The exponential law of endurance tests. Proc. ASTM 1910, 10, 625–630. [Google Scholar]
- Coffin, L.F., Jr. A study of the effects of cyclic thermal stress on a ductile metal. Trans. Am. Soc. Mech. Eng. 1954, 76, 931–950. [Google Scholar]
- Manson, S.S. Fatigue: A complex subject—Some simple approximation. Exper. Mech. 1965, 5, 193–226. [Google Scholar] [CrossRef]
- Karolczuk, A. Non-local area approach to fatigue life evaluation under combined reversed bending and torsion. Int. J. Fatigue 2008, 30, 1985–1996. [Google Scholar] [CrossRef]
- Karolczuk, A.; Blacha, L. Fatigue life estimation under variable amplitude bending using the non-local damage parameter and multisurface plasticity model. Int. J. Fatigue 2011, 33, 1376–1383. [Google Scholar] [CrossRef]
- Fujii, T.; Tohgo, K.; Fujioka, M.; Muhamad Azmi, M.S.; Kikushima, K.; Shimamura, Y. Proposal of an alternating bending technique for evaluating low-to-high cycle fatigue of structural steels. Fatigue Fract. Eng. Mater. Struct. 2020, 43, 1917–1927. [Google Scholar] [CrossRef]
- Muralidharan, U.; Manson, S.S. A Modified Universal Slopes Equation for Estimation of Fatigue Characteristic of Metals. Trans. ASME J. Eng. Mater Tech. 1986, 110, 55–58. [Google Scholar] [CrossRef]
- JIS G 3115. Steel Plates for Pressure Vessels for Intermediate Temperature Service; Japanese Standards Association: Tokyo, Japan, 2016. [Google Scholar]
- Pan, B.; Qian, K.; Xie, H.; Asundi, A. Two-dimensional digital image correlation for in-plane displacement and strain measurement. Meas. Sci. Technol. 2009, 20, 062001. [Google Scholar] [CrossRef]
- Blaber, J.; Adair, B.; Antoniou, A. Ncorr: Open-source 2D digital image correlation matlab software. Exper. Mech. 2015, 55, 1105–1122. [Google Scholar] [CrossRef]
- Socie, D.F.; Morrow, J.D. Review of contemporary approaches to fatigue damage analysis. In Risk and Failure Analysis for Improved Performance and Reliability; Burke, J.J., Weiss, V., Eds.; Plenum Publication Corp: New York, NY, USA, 1980; pp. 141–194. [Google Scholar]
- Smith, R.N.; Watson, P.; Topper, T.H. A Stress-Strain Parameter for the Fatigue of Metals. J. Mater. 1970, 5, 767–778. [Google Scholar]
- Manson, S.S.; Halford, G.R. Practical implementation of the double linear damage rule and damage curve approach for treating cumulative fatigue damage. Int. J. Fracture 1981, 17, 169–172. [Google Scholar] [CrossRef] [Green Version]
- Kitano, T.; Tagawa, T.; Aihara, S.; Miyata, T. Influence of Microstructures on Fatigue Crack Initiation and Propagation in Low-carbon Steel. Tetsu-to-Hagane 1997, 83, 395–400. (In Japanese) [Google Scholar] [CrossRef]
- Murakami, Y. (Ed.) Stress Intensity Factors Handbook; Pergamon Press: Oxford, UK, 1987; Volume 1, pp. 11–12. [Google Scholar]
- Muhamad Azmi, M.S.; Fujii, T.; Tohgo, K.; Shimamura, Y. On the ΔJ-integral to characterize elastic -plastic fatigue crack growth. Eng. Fract. Mech. 2017, 176, 300. [Google Scholar] [CrossRef]
- The Society of Materials Science, Japan. Data Book on Fatigue Crack Growth Rates of Metallic Materials; Elsevier Science Ltd.: Amsterdam, The Netherlands, 1996. [Google Scholar]
- ISO 12107:2012. Metallic materials—Fatigue testing—Statistical planning and analysis of data; ISO: Genève, Switzerland, 2012. [Google Scholar]
- ASTM E739-10. Statistical Analysis for Fatigue Data (ASTM E739-10); ASTM International: West Conshohocken, PA, USA, 2015. [Google Scholar]






| C | Si | Mn | P | S | Cu | Ni | Cr |
|---|---|---|---|---|---|---|---|
| 0.12 | 0.22 | 1.04 | 0.015 | 0.004 | 0.01 | 0.02 | 0.03 |
| Young’s Modulus, GPa | Poisson’s Ratio | Yield Stress, MPa | Tensile Strength, MPa |
|---|---|---|---|
| 215 | 0.32 | 247 | 430 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fujii, T.; Muhamad Azmi, M.S.B.; Tohgo, K.; Shimamura, Y. Influence of Strain Gradient on Fatigue Life of Carbon Steel for Pressure Vessels in Low-Cycle and High-Cycle Fatigue Regimes. Materials 2022, 15, 445. https://doi.org/10.3390/ma15020445
Fujii T, Muhamad Azmi MSB, Tohgo K, Shimamura Y. Influence of Strain Gradient on Fatigue Life of Carbon Steel for Pressure Vessels in Low-Cycle and High-Cycle Fatigue Regimes. Materials. 2022; 15(2):445. https://doi.org/10.3390/ma15020445
Chicago/Turabian StyleFujii, Tomoyuki, Muhamad Safwan Bin Muhamad Azmi, Keiichiro Tohgo, and Yoshinobu Shimamura. 2022. "Influence of Strain Gradient on Fatigue Life of Carbon Steel for Pressure Vessels in Low-Cycle and High-Cycle Fatigue Regimes" Materials 15, no. 2: 445. https://doi.org/10.3390/ma15020445
APA StyleFujii, T., Muhamad Azmi, M. S. B., Tohgo, K., & Shimamura, Y. (2022). Influence of Strain Gradient on Fatigue Life of Carbon Steel for Pressure Vessels in Low-Cycle and High-Cycle Fatigue Regimes. Materials, 15(2), 445. https://doi.org/10.3390/ma15020445






