Some Theoretical and Experimental Extensions Based on the Properties of the Intrinsic Transfer Matrix
Abstract
1. Introduction
2. The Idea of an Intrinsic Transfer Matrix
3. Practical Consequences of Applying the Intrinsic Transfer Matrix Method
3.1. A New Form of Resonance Condition
3.2. Intrinsic Transfer Matrix in Case of Attenuation
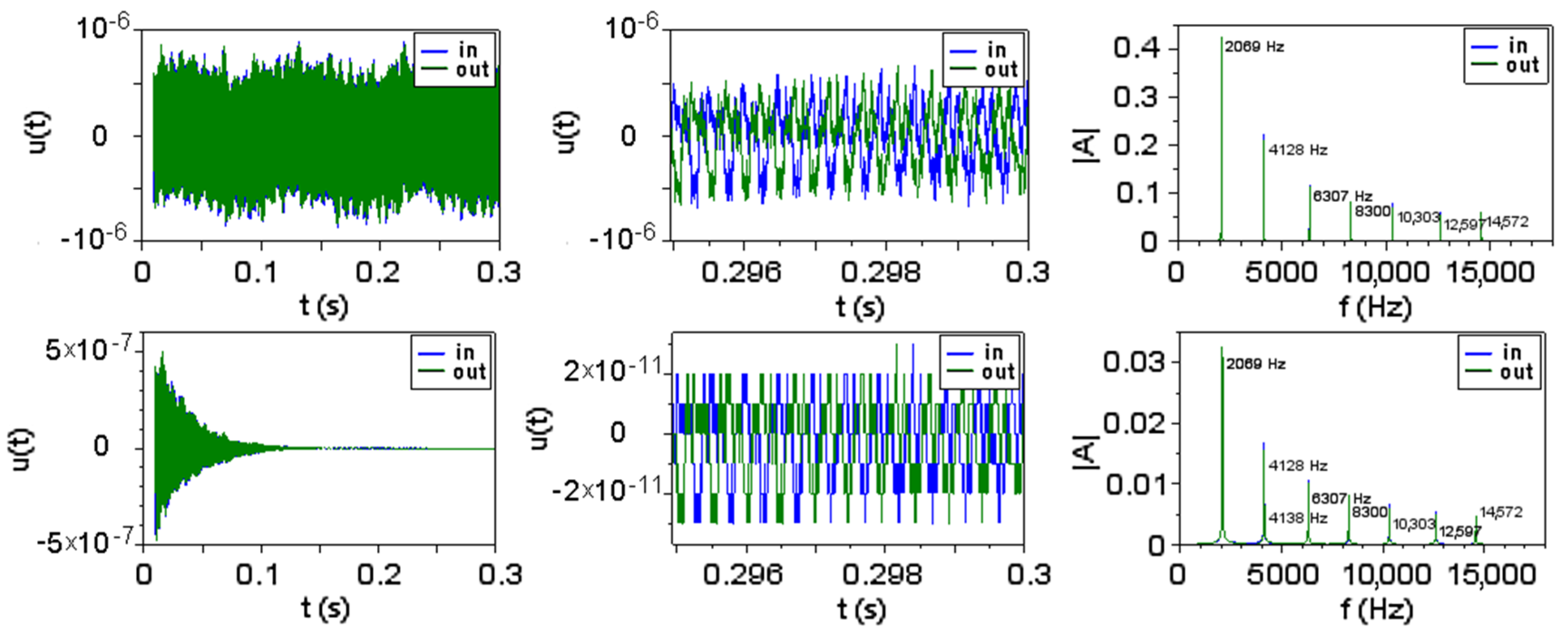
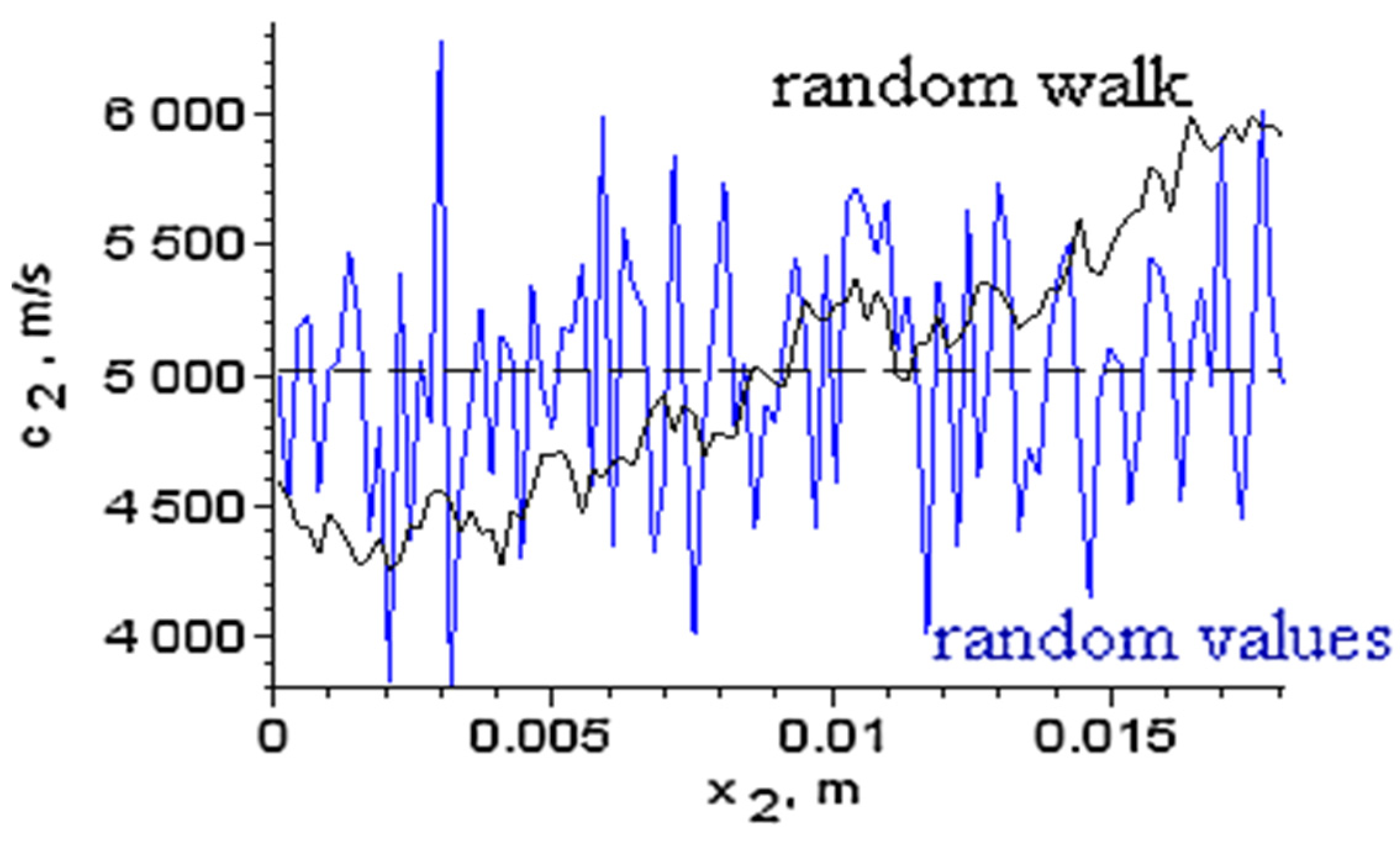
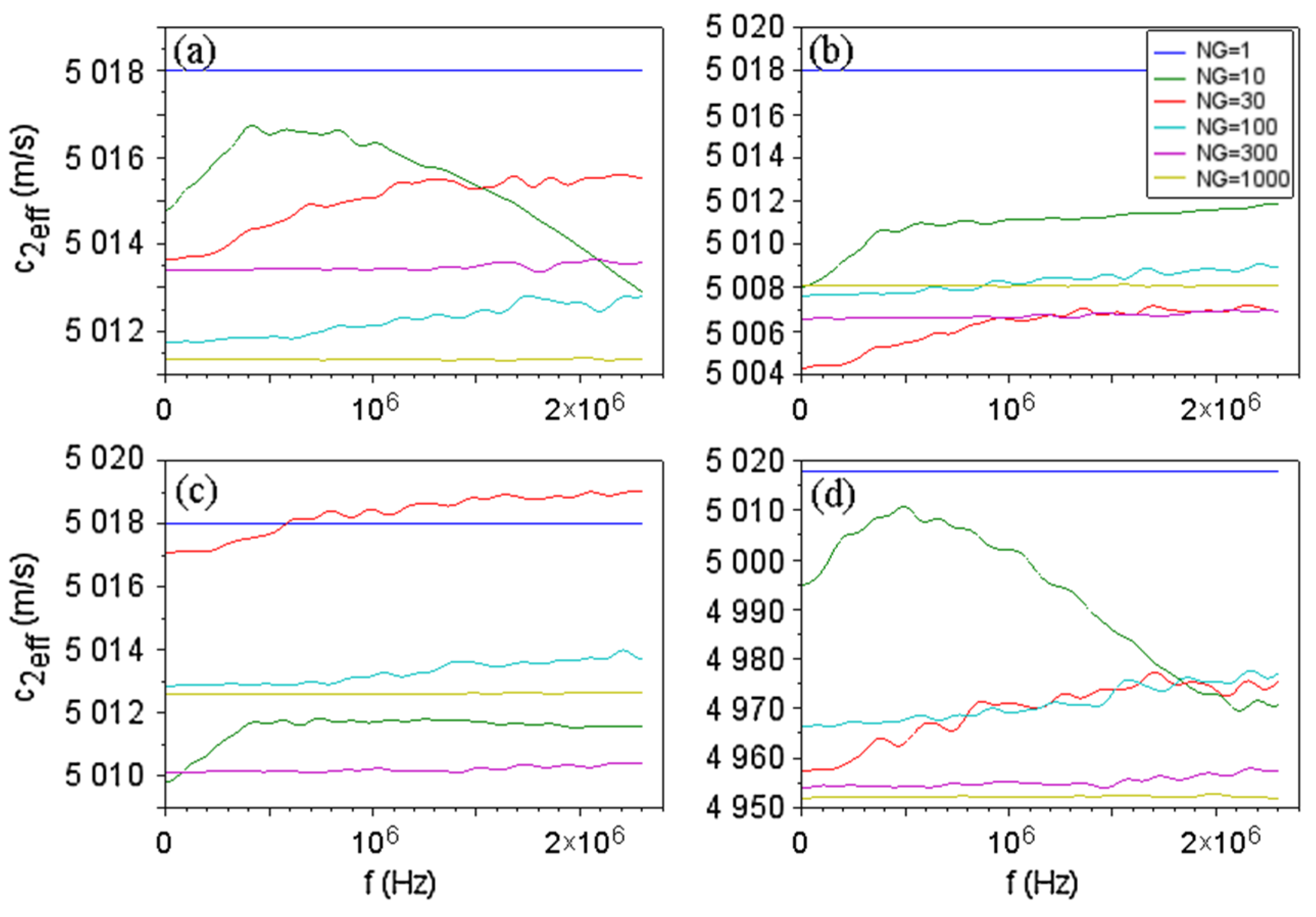
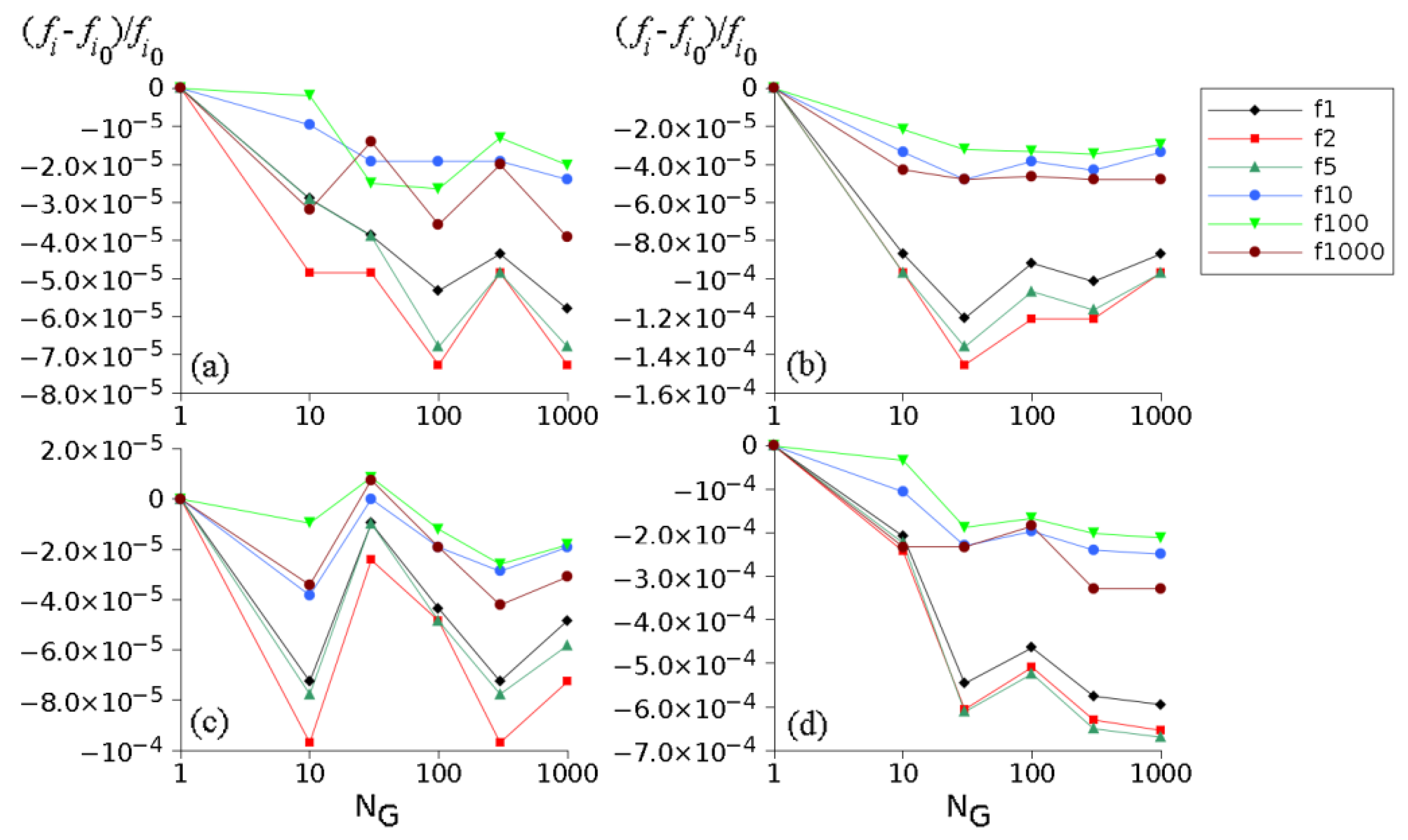
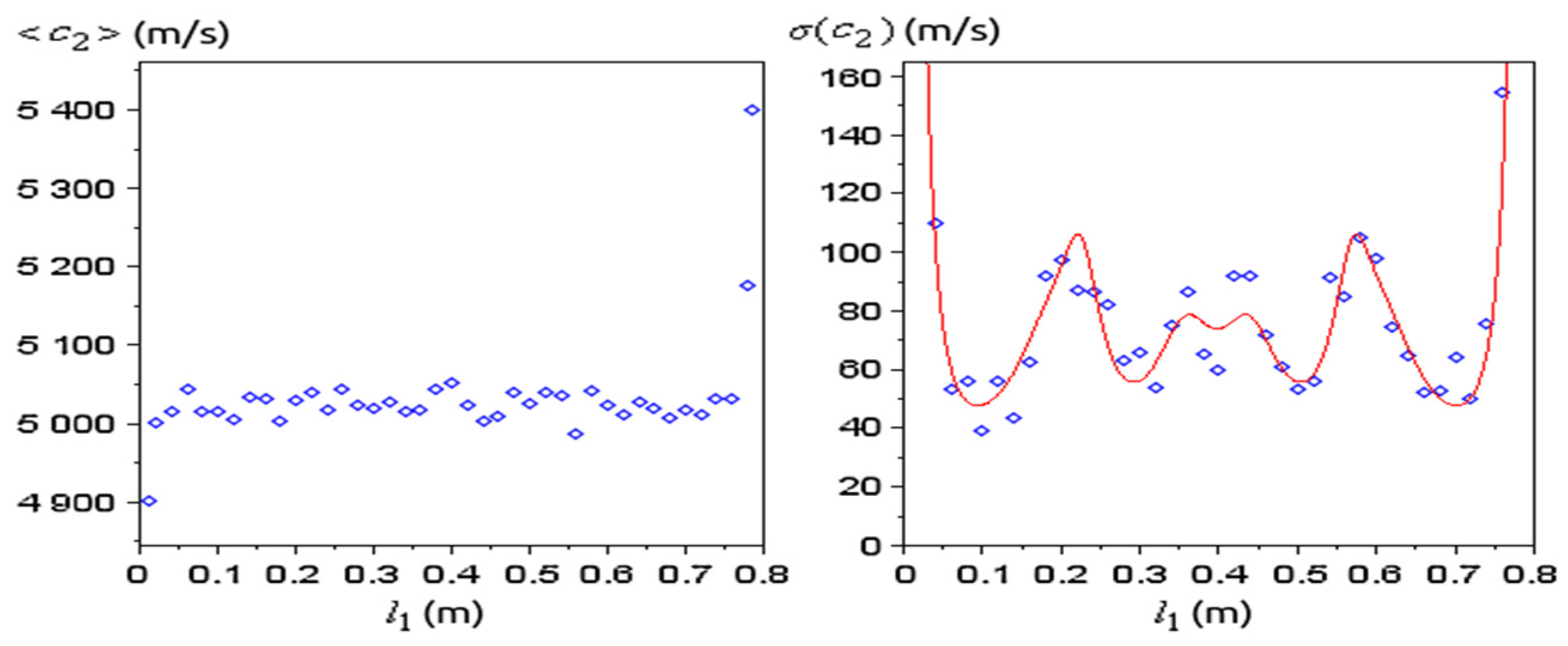
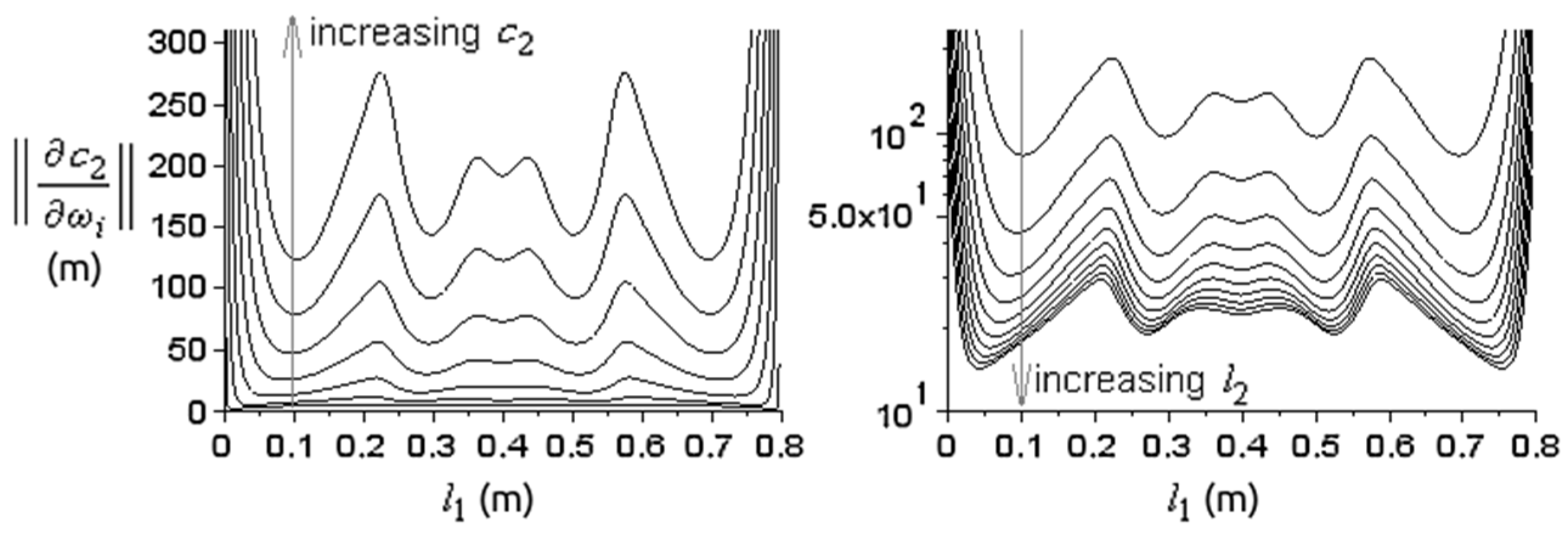
3.3. Influence of the Inhomogeneity Studied by Intrinsic Transfer Matrix
- (a)
- In the case of random values of , taking into account 1877 profiles, we obtained a fitting function: ;
- (b)
- In the case of random walk values of taking into consideration 646 profiles, the fitting function is .
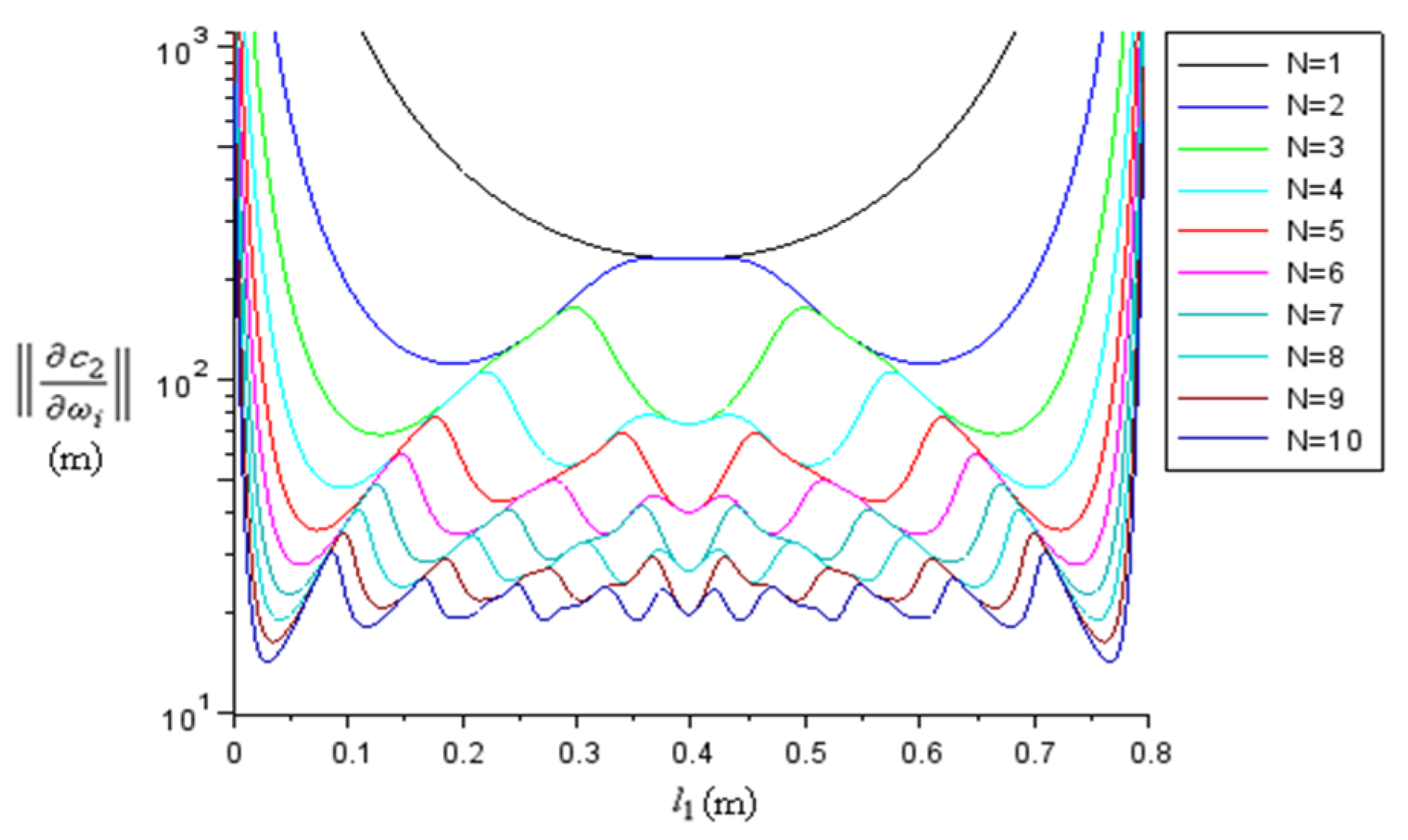
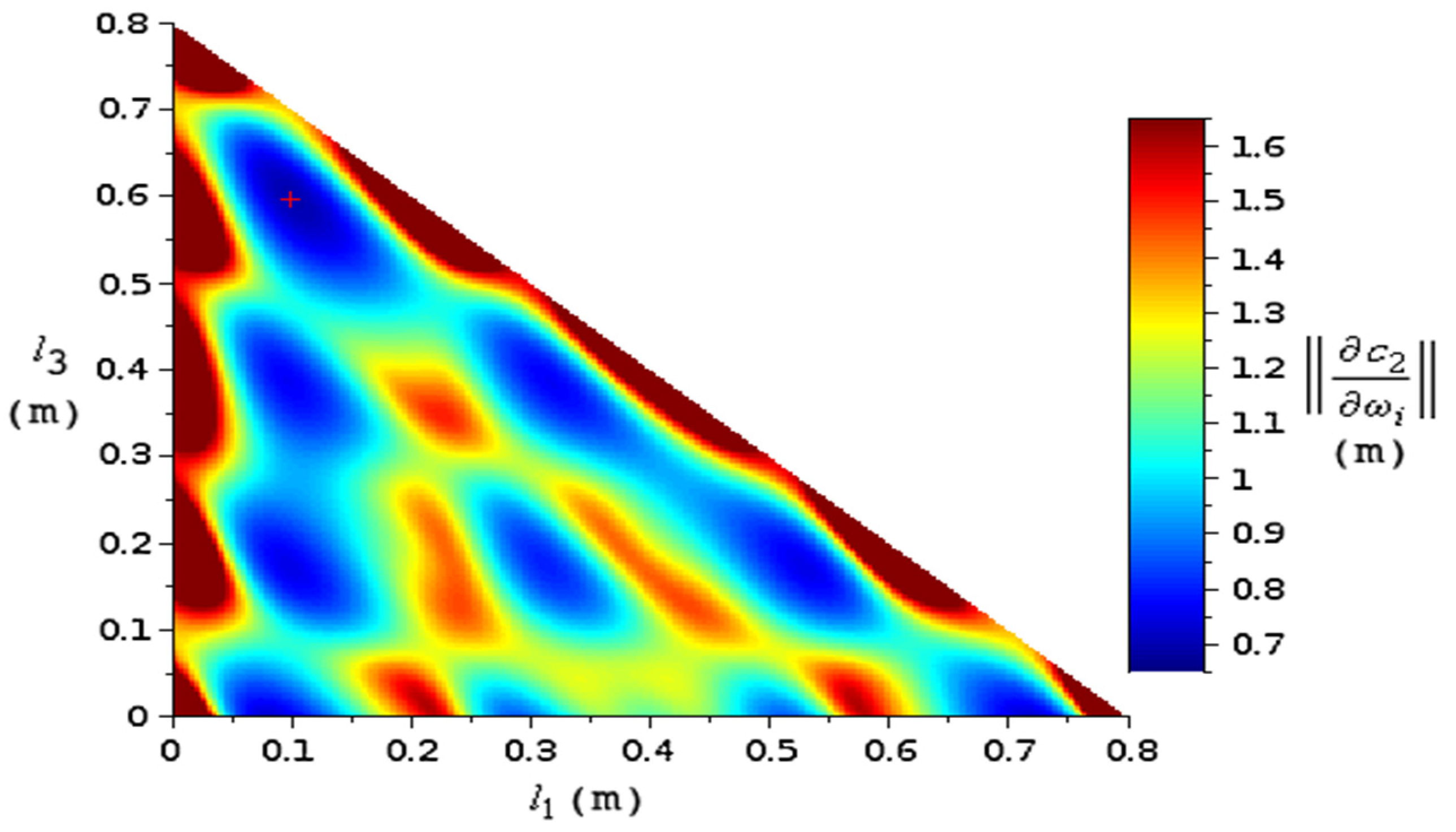
3.4. A Proposed Optimization Alghorithm Based on the Intrinsic Transfer Matrix
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Fold, D.L.; Loggins, C.D. Transmission and reflection of ultrasonic waves in layered media. J. Acoust. Soc. Am. 1977, 67, 1102–1109. [Google Scholar] [CrossRef]
- Song, B.H.; Bolton, J.S. A transfer-matrix approach for estimating the characteristic impedance and wave numbers of limp and rigid porous materials. J. Acoust. Soc. Am. 2000, 107, 1131–1152. [Google Scholar] [CrossRef]
- Khurana, P.; Lauriks, W. On imperfect interface in multilayered poroelastic structures (conference paper). In Proceedings of the Poromechanics IV-4th Biot Conference on Poromechanics, New York, NY, USA, 8–10 June 2009; pp. 830–835, ISBN 978-160595006. [Google Scholar]
- Wesolowski, Z. Wave speed in periodic elastic layers. Arch. Mech. 1991, 43, 271–282. [Google Scholar]
- Aylo, R.; Nehmetallah, G.; Li, H.; Banerjee, P.P. Multilayer Periodic and Random Metamaterial Structures: Analysis and Applications. IEEE Access 2014, 2, 437–450. [Google Scholar] [CrossRef]
- Bolton, J.S.; Yoo, T.; Olivieri, O. Measurement of normal incidence Transmission Loss and Other Acoustical Properties of Materials Placed in a Standing Wave Tube. Bruel Kjaer Tech. Rev. 2007, 1, 1–40. [Google Scholar]
- Lee, C.-M.; Xu, Y. A modified transfer matrix method for prediction of transmission loss of multilayer acoustic materials. J. Sound Vib. 2009, 326, 290–301. [Google Scholar] [CrossRef]
- Delsanto, P.; Schechter, R.; Mignogna, R. Connection machine simulation of ultrasonic wave propagation in materials III: The three-dimensional case. Wave Motion 1997, 26, 329–339. [Google Scholar] [CrossRef]
- Cretu, N.; Nita, G. Pulse propagation in finite elastic inhomogeneous media. Comput. Mater. Sci. 2004, 31, 329–336. [Google Scholar] [CrossRef]
- Nicolae, C.; Gelu, N. A simplified modal analysis based on the properties of the transfer matrix. Mech. Mater. 2013, 60, 121–128. [Google Scholar] [CrossRef]
- Verdière, K.; Panneton, R.; Elkoun, S.; Dupont, T.; LeClaire, P. Transfer matrix method applied to the parallel assembly of sound absorbing materials. J. Acoust. Soc. Am. 2013, 134, 4648–4658. [Google Scholar] [CrossRef]
- Carbajo, J.; Ramis, J.; Godinho, L.; Amado-Mendes, P. Modeling of grooved acoustic panels. Appl. Acoust. 2017, 120, 9–14. [Google Scholar] [CrossRef]
- Guo, R.; Tang, W.-B. Transfer matrix methods for sound attenuation in resonators with perforated intruding inlets. Appl. Acoust. 2017, 116, 14–23. [Google Scholar] [CrossRef]
- Mimani, A.; Munjal, M. 3-D acoustic analysis of elliptical chamber mufflers having an end-inlet and a side-outlet: An impedance matrix approach. Wave Motion 2012, 49, 271–295. [Google Scholar] [CrossRef]
- Vijayasree, N.; Munjal, M. On an Integrated Transfer Matrix method for multiply connected mufflers. J. Sound Vib. 2012, 331, 1926–1938. [Google Scholar] [CrossRef]
- Lardeau, A.; Groby, J.-P.; Romero-García, V. Broadband Transmission Loss Using the Overlap of Resonances in 3D Sonic Crystals. Crystals 2016, 6, 51. [Google Scholar] [CrossRef]
- Lagarrigue, C.; Groby, J.P.; Tournat, V. Sustainable sonic crystal made of resonating bamboo rods. J. Acoust. Soc. Am. 2013, 133, 247–254. [Google Scholar] [CrossRef]
- Crețu, N.; Pop, M.I.; Boer, A. Quaternion Formalism for the Intrinsic Transfer Matrix. Phys. Procedia 2015, 70, 262–265. [Google Scholar] [CrossRef][Green Version]
- Laly, Z.; Panneton, R.; Atalla, N. Characterization and development of periodic acoustic metamaterials using a transfer matrix approach. Appl. Acoust. 2022, 185, 108381. [Google Scholar] [CrossRef]
- Létourneau, P.-D.; Wu, Y.; Papanicolaou, G.; Garnier, J.; Darve, E. A numerical study of super-resolution through fast 3D wideband algorithm for scattering in highly-heterogeneous media. Wave Motion 2017, 70, 113–134. [Google Scholar] [CrossRef]
- Belyayev, Y.N. Method for calculating multiwave scattering by layered anisotropic media. Wave Motion 2020, 99, 102664. [Google Scholar] [CrossRef]
- Chevillotte, F.; Panneton, R. Coupling transfer matrix method to finite element method for analyzing the acoustics of complex hollow body networks. Appl. Acoust. 2011, 72, 962–968. [Google Scholar] [CrossRef]
- Fink, K.S.; Johnson, G.; Carroll, T.; Mar, D.; Pecora, L. Three coupled oscillators as a universal probe of synchronization stability in coupled oscillator arrays. Phys. Rev. E 2000, 61, 5080–5090. [Google Scholar] [CrossRef]
- Dryburgh, P.; Li, W.; Pieris, D.; Fuentes-Domínguez, R.; Patel, R.; Smith, R.J.; Clark, M. Measurement of the single crystal elasticity matrix of polycrystalline materials. Acta Mater. 2021, 225, 117551. [Google Scholar] [CrossRef]
- Pellegrini, Y.-P. Causal Stroh formalism for uniformly-moving dislocations in anisotropic media: Somigliana dislocations and Mach cones. Wave Motion 2017, 68, 128–148. [Google Scholar] [CrossRef]
- Grigg, H.; Gallacher, B.J.; Craig, N.P. Robust, high-resolution, indexed 3D slowness surfaces for Rayleigh-type waves on Lithium Niobate via parallelised Newtonian flow phase tracking. J. Sound Vib. 2021, 116533. [Google Scholar] [CrossRef]
- Maio, L.; Fromme, P. On ultrasound propagation in composite laminates: Advances in numerical simulation. Prog. Aerosp. Sci. 2022, 129, 100791. [Google Scholar] [CrossRef]
- Drelich, R.; Kaczmarek, M.; Piwakowski, B. Accuracy of parameter identification using the dispersion of surface waves and the role of data quality for inhomogeneous concrete. NDT E Int. 2018, 98, 195–207. [Google Scholar] [CrossRef]
- Huang, M.; Sha, G.; Huthwaite, P.; Rokhlin, S.I.; Lowe, M.J.S. Elastic wave velocity dispersion in polycrystals with elongated grains: Theoretical and numerical analysis. J. Acoust. Soc. Am. 2020, 148, 3645–3662. [Google Scholar] [CrossRef]
- Sha, G.; Huang, M.; Lowe, M.; Rokhlin, S.I. Attenuation and velocity of elastic waves in polycrystals with generally anisotropic grains: Analytic and numerical modeling. J. Acoust. Soc. Am. 2020, 147, 2442–2465. [Google Scholar] [CrossRef] [PubMed]
- Van Pamel, A.; Sha, G.; Rokhlin, S.I.; Lowe, M. Finite-element modelling of elastic wave propagation and scattering within heterogeneous media. Proc. R. Soc. A Math. Phys. Eng. Sci. 2017, 473, 20160738. [Google Scholar] [CrossRef]
- Man, C.-S.; Paroni, R.; Xiang, Y.; Kenik, E.A. On the geometric autocorrelation function of polycrystalline materials. J. Comput. Appl. Math. 2006, 190, 200–210. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cretu, N.; Pop, M.-I.; Andia Prado, H.S. Some Theoretical and Experimental Extensions Based on the Properties of the Intrinsic Transfer Matrix. Materials 2022, 15, 519. https://doi.org/10.3390/ma15020519
Cretu N, Pop M-I, Andia Prado HS. Some Theoretical and Experimental Extensions Based on the Properties of the Intrinsic Transfer Matrix. Materials. 2022; 15(2):519. https://doi.org/10.3390/ma15020519
Chicago/Turabian StyleCretu, Nicolae, Mihail-Ioan Pop, and Hank Steve Andia Prado. 2022. "Some Theoretical and Experimental Extensions Based on the Properties of the Intrinsic Transfer Matrix" Materials 15, no. 2: 519. https://doi.org/10.3390/ma15020519
APA StyleCretu, N., Pop, M.-I., & Andia Prado, H. S. (2022). Some Theoretical and Experimental Extensions Based on the Properties of the Intrinsic Transfer Matrix. Materials, 15(2), 519. https://doi.org/10.3390/ma15020519






