Effect of Paste Fluidity and Paste-to-Aggregate Ratio on the Strength and Permeability of Porous Mortar from Manufactured Sand
Abstract
:1. Introduction
2. The Review of Previous Studies
3. Experimental Work
3.1. Materials
3.2. Mixture Design
3.3. Specimens Preparation
3.4. Measurements
3.5. RSM Modeling
4. Results and Discussion
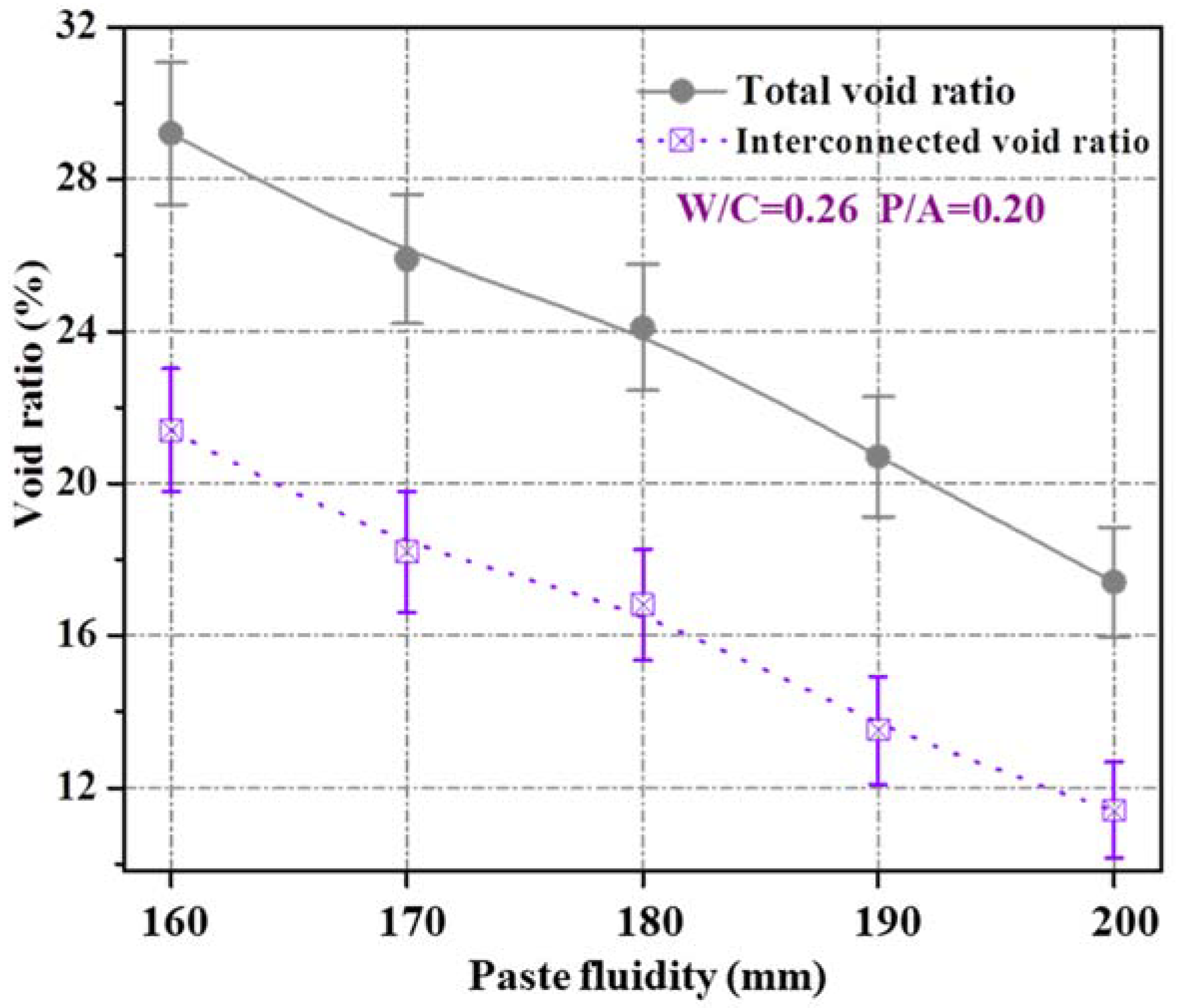
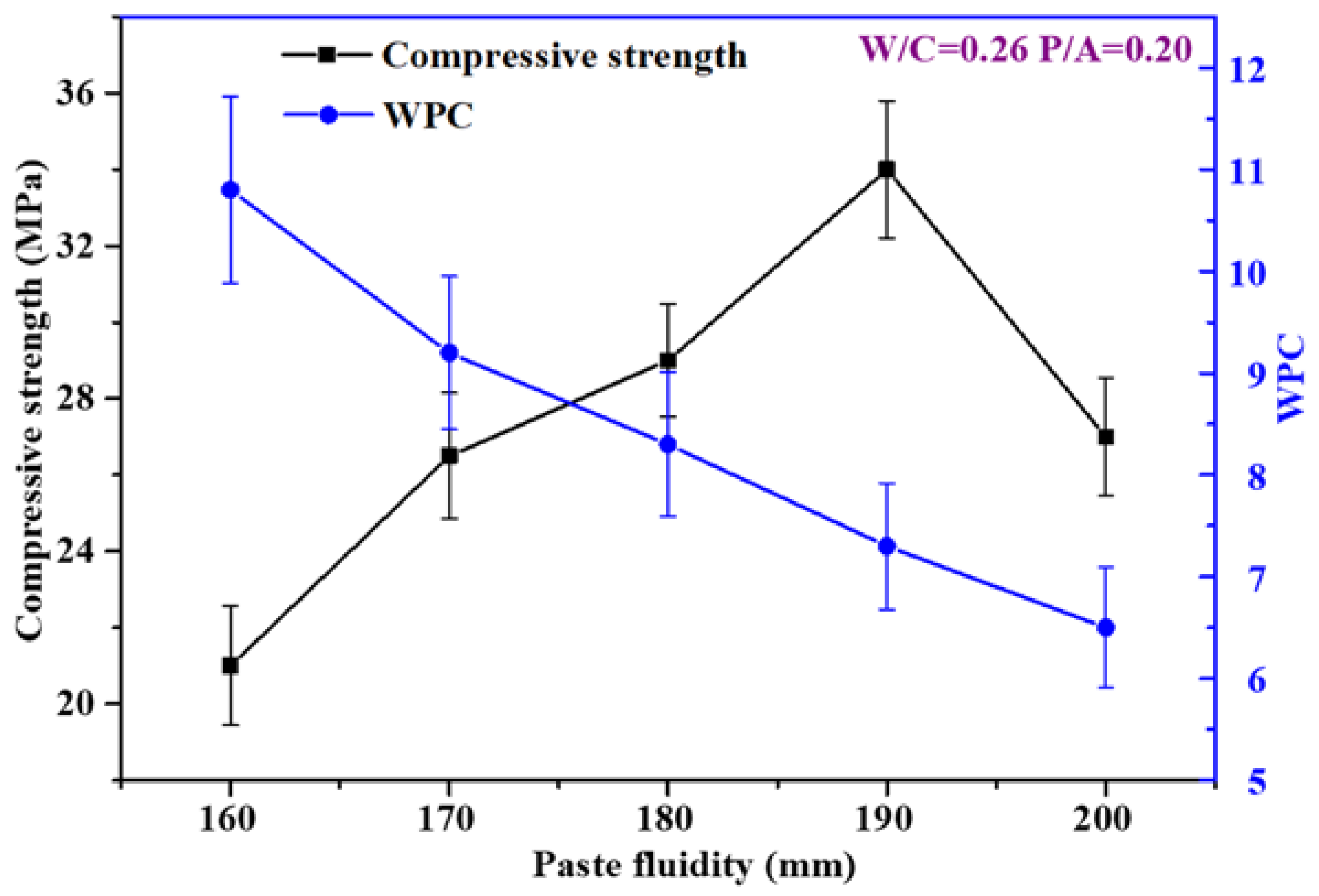
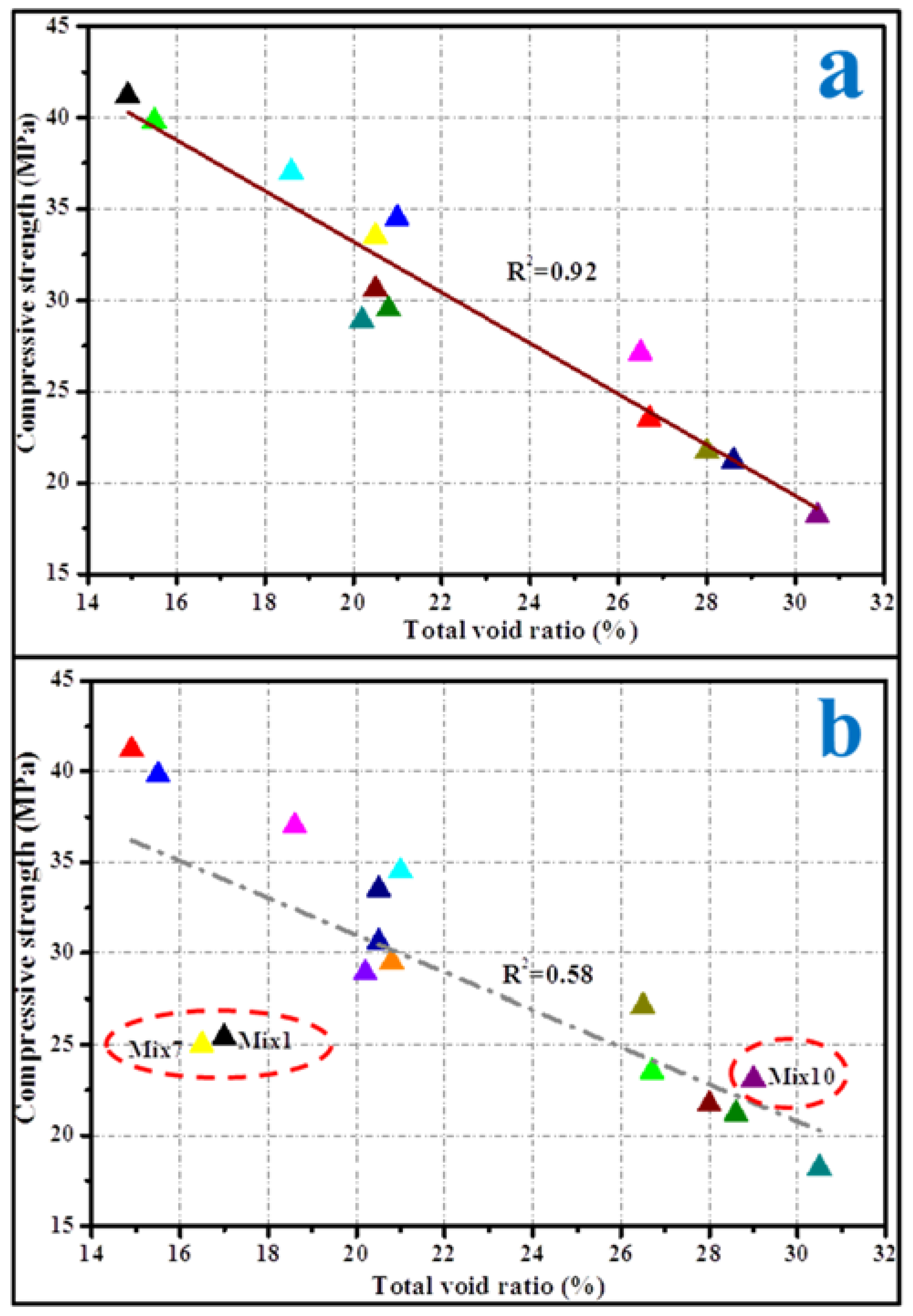
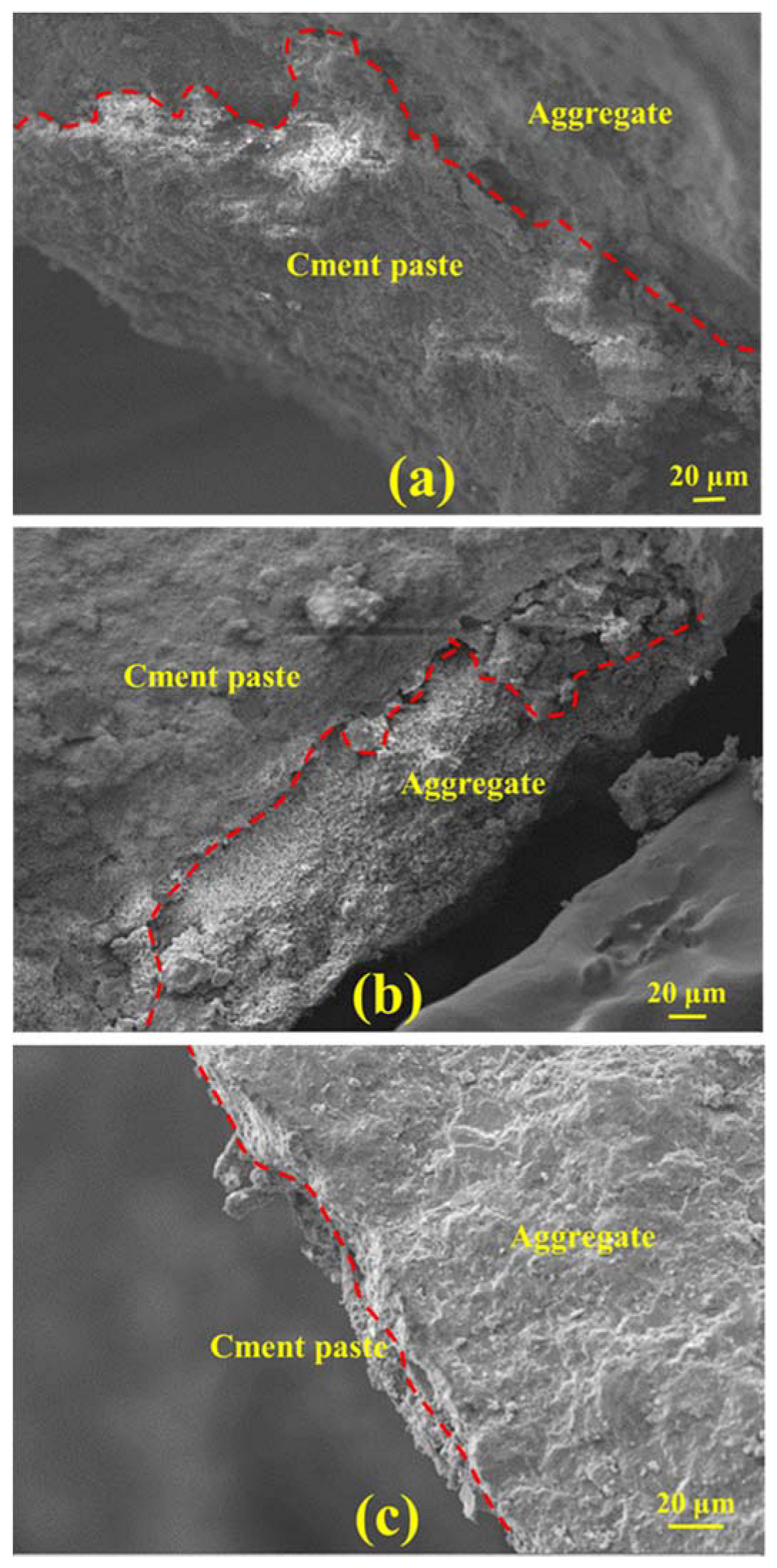
4.1. Effect of Cement Paste Fluidity on the Basic Physical Properties of Porous Mortar
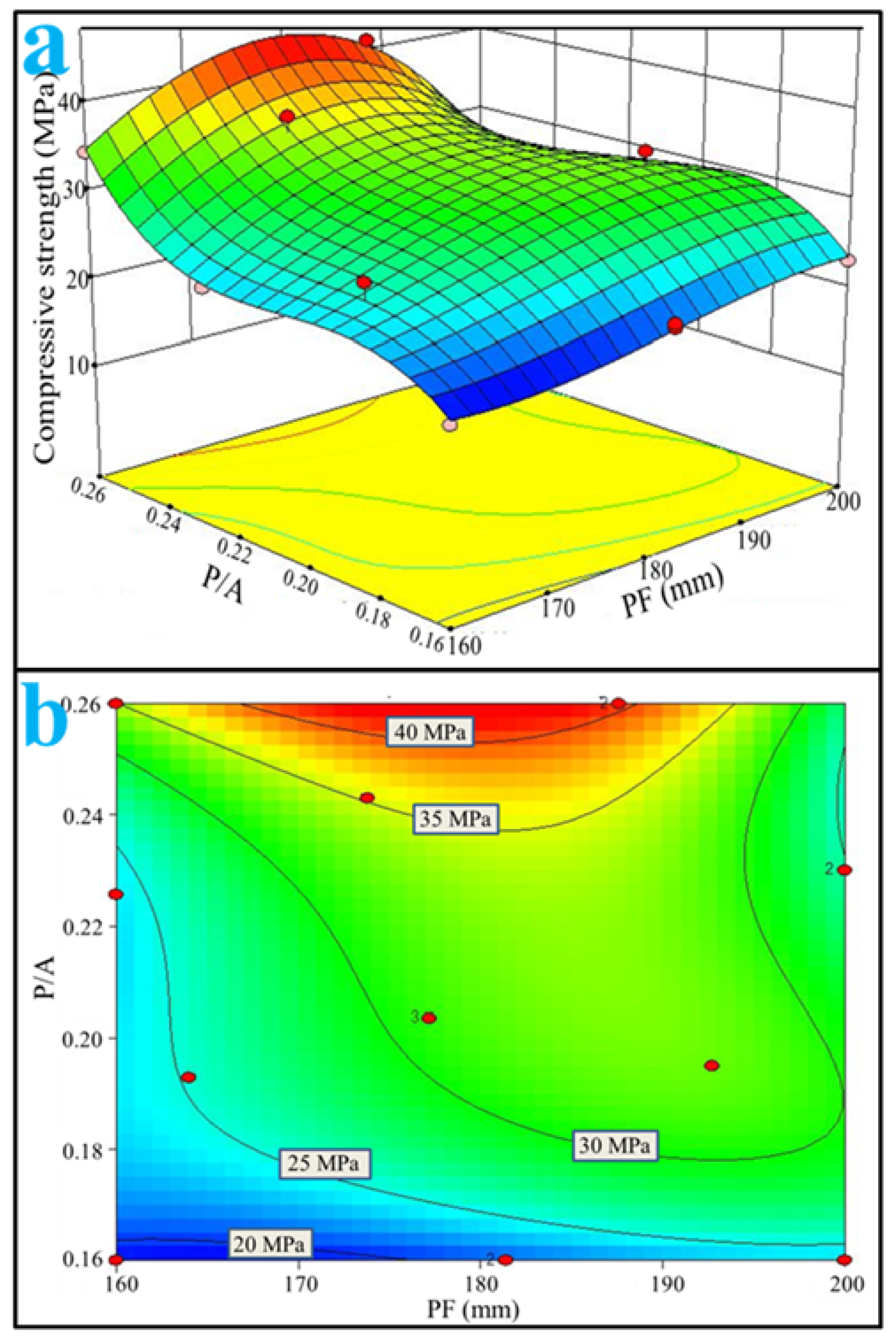
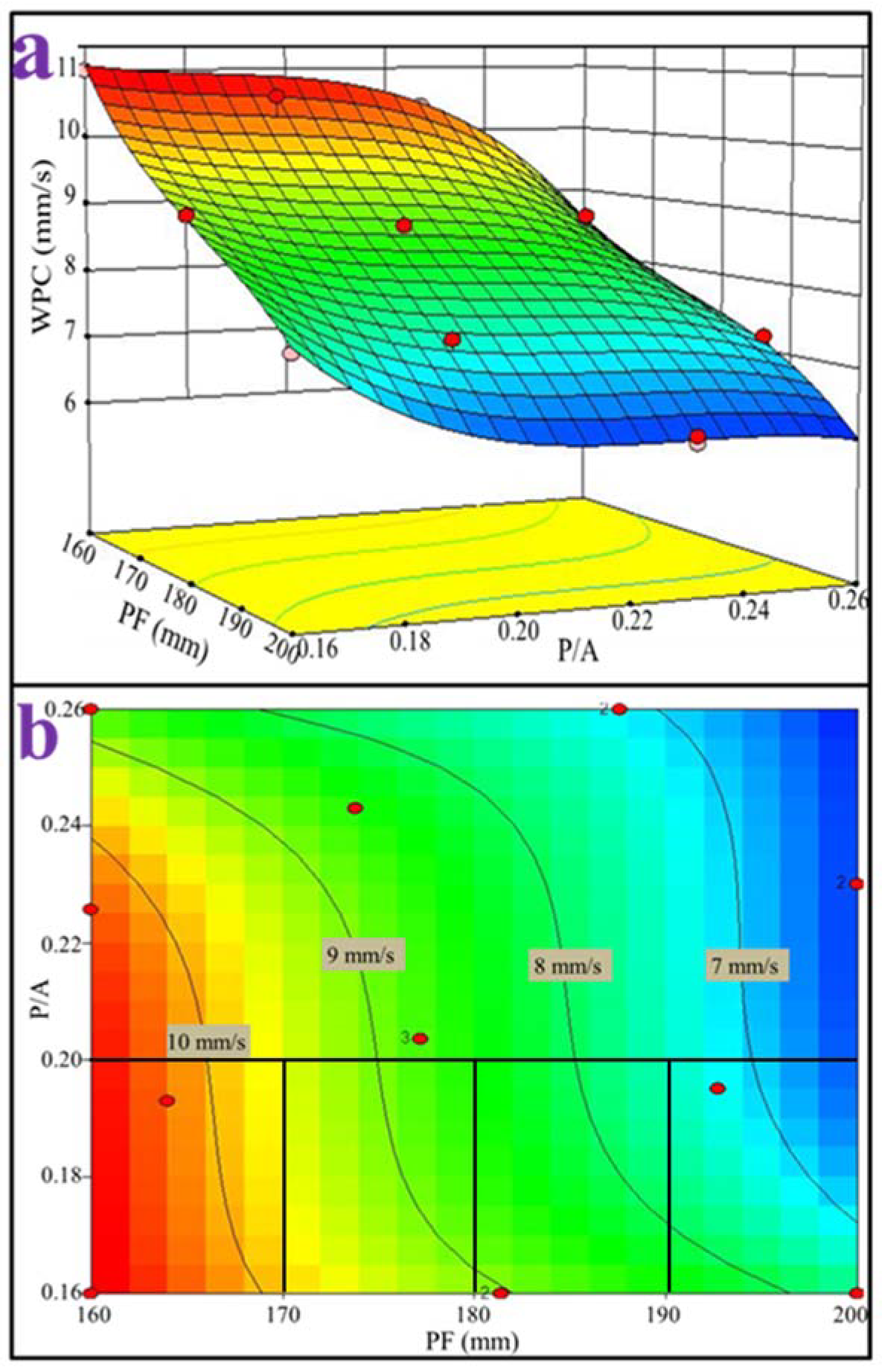
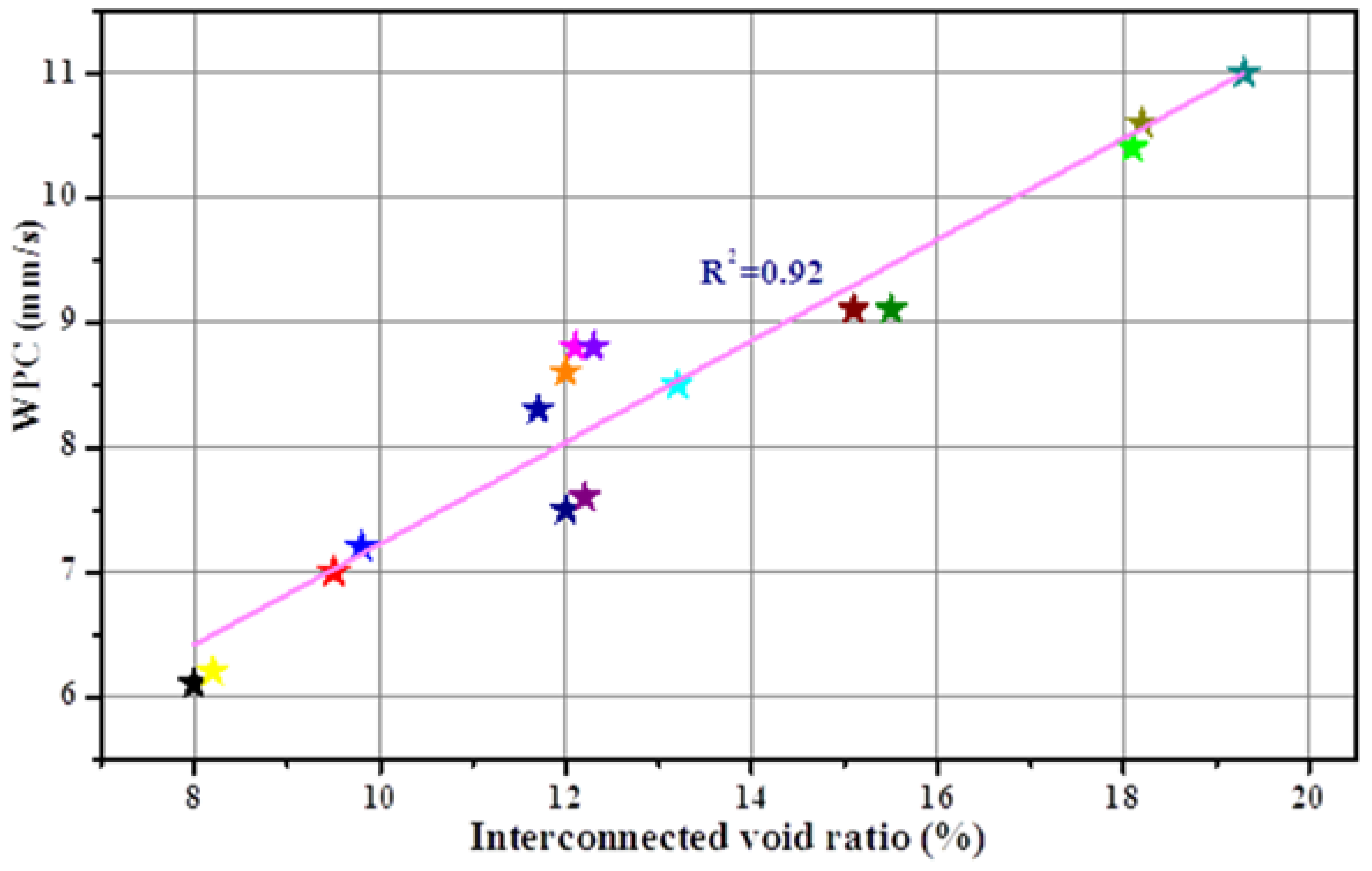
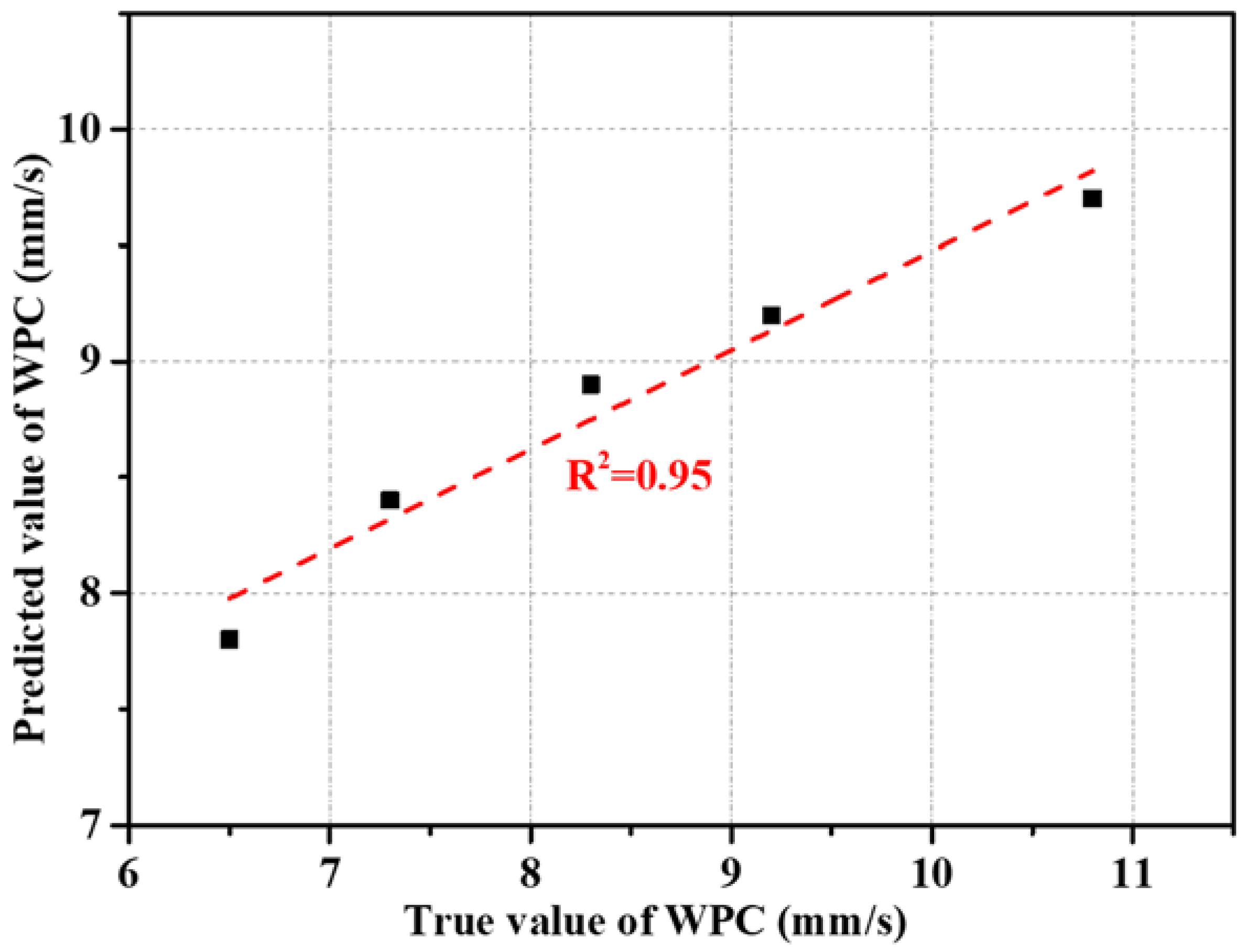
4.2. Effect of Cement Paste Fluidity and Paste-to-Aggregate Ratio on the Properties of Porous Mortar Using RSM
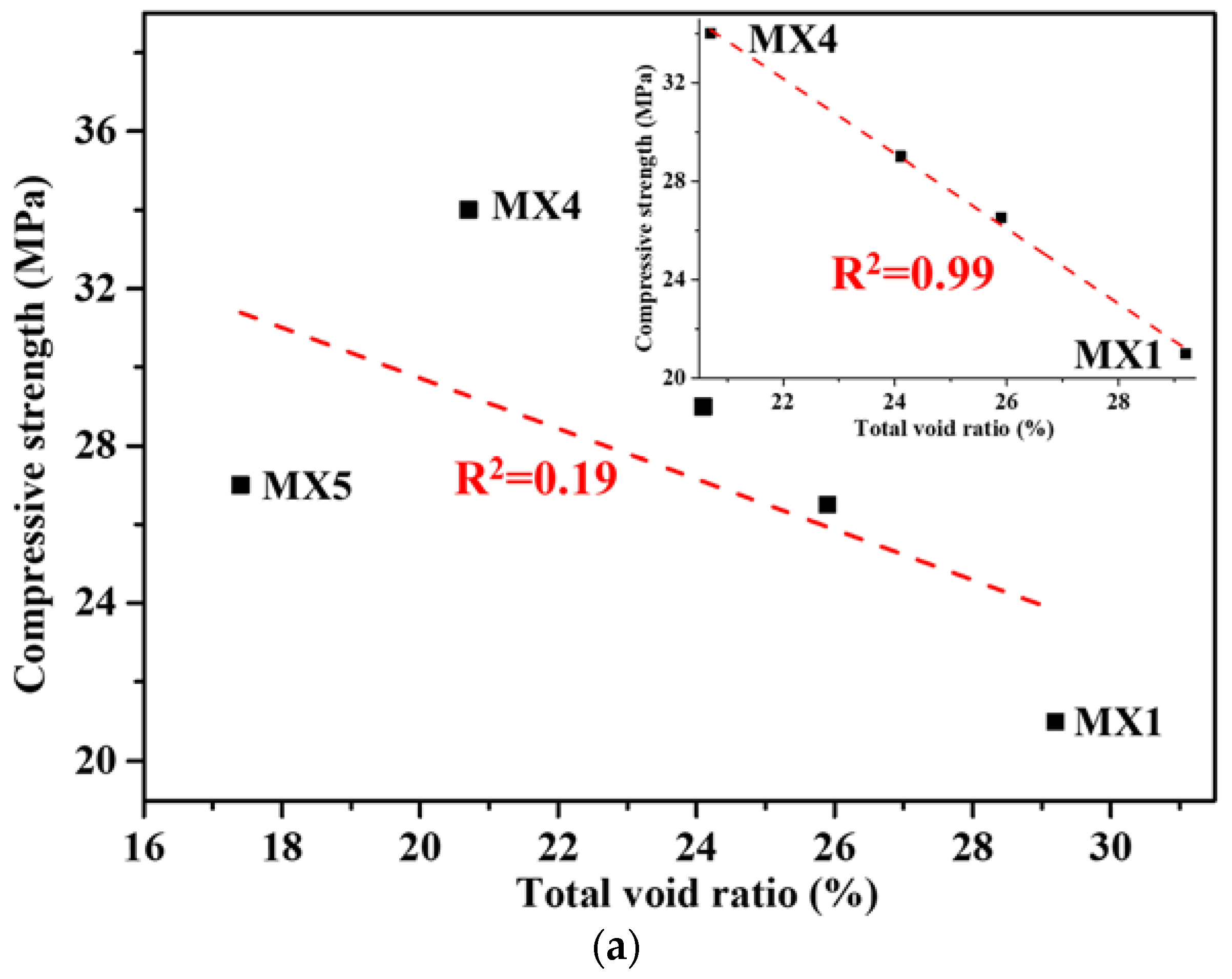
4.3. Statistical Analysis of Responses
5. Conclusions
- (1)
- At a fixed P/A of 0.20, with the increase in PF from 160 mm to 190 mm, the void ratio and WPC of the porous mortar decreased, while the compressive strength of the porous mortar increased. However, in the cases where the PF was more than 190 mm, the compressive strength dropped dramatically due to the detachment of the cement paste and its accumulation at the bottom of the porous mortar.
- (2)
- The relationship models between the responses and the two variables were accurate, suggesting the RSM technique was a useful tool in optimizing the PF and P/A of porous mortar from manufactured sand (M-sand) with the desired properties as responses. The interaction of the two variables with the responses were visually observed by the 3D surface diagram, and the contour diagrams provided the intervals of the different responses.
- (3)
- The WPC and mechanical strength are impacted by PF and P/A in a contradictive way, to some extent. The RSM could be utilized to set the goal parameters and design the experiment for porous mortar from manufactured sand based on the experimental outcome variables in order to reduce the design time, improve the performance of the existing process and product, improve reliability, and achieve product and process robustness.
- (4)
- This paper made a systematic study of the effects of paste fluidity and paste-to-aggregate ratio on the properties of permeable mortar. In the future, the influence of the shape of M-sand on the mechanical properties, permeability, porosity and other basic physical properties, and the durability of permeable mortar will be investigated.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhang, W.-L.; Yuan, Z.-J.; Li, D.-Q.; Zhang, K.; Zhao, L.-Y. Mechanical and vegetation performance of porous concrete with recycled aggregate in riparian buffer area. J. Clean. Prod. 2022, 332, 130015. [Google Scholar] [CrossRef]
- Al-Oraimi, S.; Taha, R.; Hassan, H. The effect of the mineralogy of coarse aggregate on the mechanical properties of high-strength concrete. Constr. Build. Mater. 2006, 20, 499–503. [Google Scholar] [CrossRef]
- Thomas, M.D.A.; Folliard, K.J. Concrete aggregates and the durability of concrete. Durab. Concr. Cem. Compos. 2007, 247–281. [Google Scholar] [CrossRef]
- Park, S.-B.; Tia, M. An experimental study on the water-purification properties of porous concrete. Cem. Concr. Res. 2004, 34, 177–184. [Google Scholar] [CrossRef]
- Chindaprasirt, P.; Hatanaka, S.; Chareerat, T.; Mishima, N.; Yuasa, Y. Cement paste characteristics and porous concrete properties. Constr. Build. Mater. 2008, 22, 894–901. [Google Scholar] [CrossRef]
- Park, S.B.; Seo, D.S.; Lee, J. Studies on the sound absorption characteristics of porous concrete based on the content of recycled aggregate and target void ratio. Cem. Concr. Res. 2005, 35, 1846–1854. [Google Scholar] [CrossRef]
- Tarnai, M.; Mizuguchi, H.; Hatanaka, S. Design, construction and recent applications of porous concrete in Japan. In Proceedings of the 28th Conference on Our World in Concrete & Structures, Singapore, 28–29 August 2013; pp. 22–28. [Google Scholar]
- Kim, H.-K.; Lee, H. Acoustic absorption modeling of porous concrete considering the gradation and shape of aggregates and void ratio. J. Sound Vib. 2010, 329, 866–879. [Google Scholar] [CrossRef]
- Kim, H.; Lee, H. Influence of cement flow and aggregate type on the mechanical and acoustic characteristics of porous concrete. Appl. Acoust. 2010, 71, 607–615. [Google Scholar] [CrossRef]
- Ghafoori, N.; Dutta, S. Building and Nonpavement Applications of No-Fines Concrete. J. Mater. Civ. Eng. 1995, 7, 286–289. [Google Scholar] [CrossRef]
- Volder, A.; Watson, T.; Viswanathan, B. Potential use of pervious concrete for maintaining existing mature trees during and after urban development. Urban For. Urban Green. 2009, 8, 249–256. [Google Scholar] [CrossRef]
- Al-Jabri, K.S.; Hisada, M.; Al-Oraimi, S.K.; Al-Saidy, A.H. Copper slag as sand replacement for high performance concrete. Cem. Concr. Compos. 2009, 31, 483–488. [Google Scholar] [CrossRef]
- Tu, T.-Y.; Chen, Y.-Y.; Hwang, C.-L. Properties of HPC with recycled aggregates. Cem. Concr. Res. 2006, 36, 943–950. [Google Scholar] [CrossRef]
- Martínez-García, R.; de Rojas, M.S.; Jagadesh, P.; López-Gayarre, F.; Morán-Del-Pozo, J.M.; Juan-Valdes, A. Effect of pores on the mechanical and durability properties on high strength recycled fine aggregate mortar. Case Stud. Constr. Mater. 2022, 16, e01050. [Google Scholar] [CrossRef]
- Oliveira, T.V.; Cordeiro, L.D.N.P.; Bessa, S.A.L. Experimental study of self-leveling mortars produced with recycled concrete aggregates. Case Stud. Constr. Mater. 2022, 17, e01294. [Google Scholar] [CrossRef]
- Azevedo, A.R.; Marvila, M.T.; Zanelato, E.B.; Alexandre, J.; Xavier, G.C.; Cecchin, D. Development of mortar for laying and coating with pineapple fibers. Rev. Bras. Eng. Agrícola E Ambient. 2020, 24, 187–193. [Google Scholar] [CrossRef]
- Yan, X.; Gong, C.; Wang, S.; Lu, L. Effect of aggregate coating thickness on pore structure features and properties of porous ecological concrete. Mag. Concr. Res. 2013, 65, 962–969. [Google Scholar] [CrossRef]
- Gong, C.; Zhou, X.; Dai, W.; Liu, Y.; Lu, L.; Cheng, X. Effects of carbamide on fluidity and setting time of sulphoaluminate cement and properties of planting concrete from sulphoaluminate cement. Constr. Build. Mater. 2018, 182, 290–297. [Google Scholar] [CrossRef]
- Montgomery, D.C. Design and Analysis of Experiments, 7th ed.; John Wiley & Sons, Inc.: New York, NY, USA, 2008. [Google Scholar]
- Jang, J.; Ahn, Y.; Souri, H.; Lee, H. A novel eco-friendly porous concrete fabricated with coal ash and geopolymeric binder: Heavy metal leaching characteristics and compressive strength. Constr. Build. Mater. 2015, 79, 173–181. [Google Scholar] [CrossRef]
- Chen, M.; Zheng, Y.; Zhou, X.; Li, L.; Wang, S.; Zhao, P.; Lu, L.; Cheng, X. Recycling of paper sludge powder for achieving sustainable and energy-saving building materials. Constr. Build. Mater. 2019, 229, 116874. [Google Scholar] [CrossRef]
- Chen, M.; Li, L.; Zheng, Y.; Zhao, P.; Lu, L.; Cheng, X. Rheological and mechanical properties of admixtures modified 3D printing sulphoaluminate cementitious materials. Constr. Build. Mater. 2018, 189, 601–611. [Google Scholar] [CrossRef]
- Nanthagopalan, P.; Santhanam, M. Fresh and hardened properties of self-compacting concrete produced with manufactured sand. Cem. Concr. Compos. 2011, 33, 353–358. [Google Scholar] [CrossRef]
- Hooton, R.; Nataraja, M.; Nagaraj, T.; Reddy, A. Proportioning Concrete Mixes with Quarry Wastes. Cem. Concr. Aggreg. 2001, 23, 81–87. [Google Scholar] [CrossRef]
- M. Alexander, S. Mindess, Aggregates in Concrete; Taylor & Francis: Abingdon, UK, 2005. [Google Scholar]
- Gonçalves, J.; Tavares, L.; Filho, R.T.; Fairbairn, E.; Cunha, E. Comparison of natural and manufactured fine aggregates in cement mortars. Cem. Concr. Res. 2007, 37, 924–932. [Google Scholar] [CrossRef]
- Kim, J.-K.; Lee, C.-S.; Park, C.-K.; Eo, S.-H. The fracture characteristics of crushed limestone sand concrete. Cem. Concr. Res. 1997, 27, 1719–1729. [Google Scholar] [CrossRef]
- Donza, H.; Cabrera, O.; Irassar, E. High-strength concrete with different fine aggregate. Cem. Concr. Res. 2002, 32, 1755–1761. [Google Scholar] [CrossRef]
- Branavan, A.; Konthesingha, K.M.C.; Nanayakkara, S.M.A.; Premasiri, H.M.R. Optimizing blending of manufactured sand with offshore sand based on physical and virtue characteristics. J. Mater. Sci. Res. Rev. 2020, 6, 11–31. [Google Scholar]
- Kirthika, S.K.; Singh, S.; Chourasia, A. Alternative fine aggregates in production of sustainable concrete—A review. J. Clean. Prod. 2020, 268, 122089. [Google Scholar] [CrossRef]
- Li, L.; Chen, M.; Zhou, X.; Lu, L.; Wang, Y.; Cheng, X. Evaluation of the preparation and fertilizer release performance of planting concrete made with recycled-concrete aggregates from demolition. J. Clean. Prod. 2018, 200, 54–64. [Google Scholar] [CrossRef]
- GB/T 50081-2002; Standard for Test Method of Mechanical Properties on Ordinary Concrete. Standardization Administration of the People’s Republic of China: Beijing, China, 2003. (In Chinese)
- Allaix, D.; Carbone, V. An improvement of the response surface method. Struct. Saf. 2011, 33, 165–172. [Google Scholar] [CrossRef]
- Li, D.-Q.; Zheng, D.; Cao, Z.-J.; Tang, X.-S.; Phoon, K.-K. Response surface methods for slope reliability analysis: Review and comparison. Eng. Geol. 2016, 203, 3–14. [Google Scholar] [CrossRef]
- Zhao, Y.; Wang, X.; Jiang, J.; Zhou, L. Characterization of interconnectivity, size distribution and uniformity of air voids in porous asphalt concrete using X-ray CT scanning images. Constr. Build. Mater. 2019, 213, 182–193. [Google Scholar] [CrossRef]
- Bhutta, M.A.R.; Hasanah, N.; Farhayu, N.; Hussin, M.W.; Tahir, M.b.M.; Mirza, J. Properties of porous concrete from waste crushed concrete (recycled aggregate). Constr. Build. Mater. 2013, 47, 1243–1248. [Google Scholar] [CrossRef]
- Bhutta, M.A.R.; Tsuruta, K.; Mirza, J. Evaluation of high-performance porous concrete properties. Constr. Build. Mater. 2012, 31, 67–73. [Google Scholar] [CrossRef]
- Hashemi, S.S.G.; Bin Mahmud, H.; Djobo, J.N.Y.; Tan, C.G.; Ang, B.C.; Ranjbar, N. Microstructural characterization and mechanical properties of bottom ash mortar. J. Clean. Prod. 2018, 170, 797–804. [Google Scholar] [CrossRef]
- Kumar, G.S. Influence of fluidity on mechanical and permeation performances of recycled aggregate mortar. Constr. Build. Mater. 2019, 213, 404–412. [Google Scholar] [CrossRef]
- Zhang, S.; He, Y.; Zhang, H.; Chen, J.; Liu, L. Effect of fine sand powder on the rheological properties of one-part alkali-activated slag semi-flexible pavement grouting materials. Constr. Build. Mater. 2022, 333, 127328. [Google Scholar] [CrossRef]
- Ma, Z.; Wang, H.; Hui, B.; Jelagin, D.; You, Z.; Feng, P. Optimal design of fresh sand fog seal mortar using response surface methodology (RSM): Towards to its workability and rheological properties. Constr. Build. Mater. 2022, 340, 127638. [Google Scholar] [CrossRef]


| Composition | CaO | SiO2 | Al2O3 | SO3 | MgO | Fe2O3 | K2O | TiO2 | Los |
|---|---|---|---|---|---|---|---|---|---|
| OPC | 60.19 | 20.57 | 5.58 | 3.8 | 2.59 | 2.59 | 1.3 | 0.3 | 1.7 |
| Properties | Gradation (mm) | Apparent Density (kg/m3) | Water Absorption (%) | Bulk Density (kg/m3) |
|---|---|---|---|---|
| Results | 2.5–5 | 2425 | 2.49 | 1625 |
| Mixture No. | W/C a | P/A b | PF c (mm) |
|---|---|---|---|
| MX1 | 0.26 | 0.20 | 160 |
| MX2 | 170 | ||
| MX3 | 180 | ||
| MX4 | 190 | ||
| MX5 | 200 |
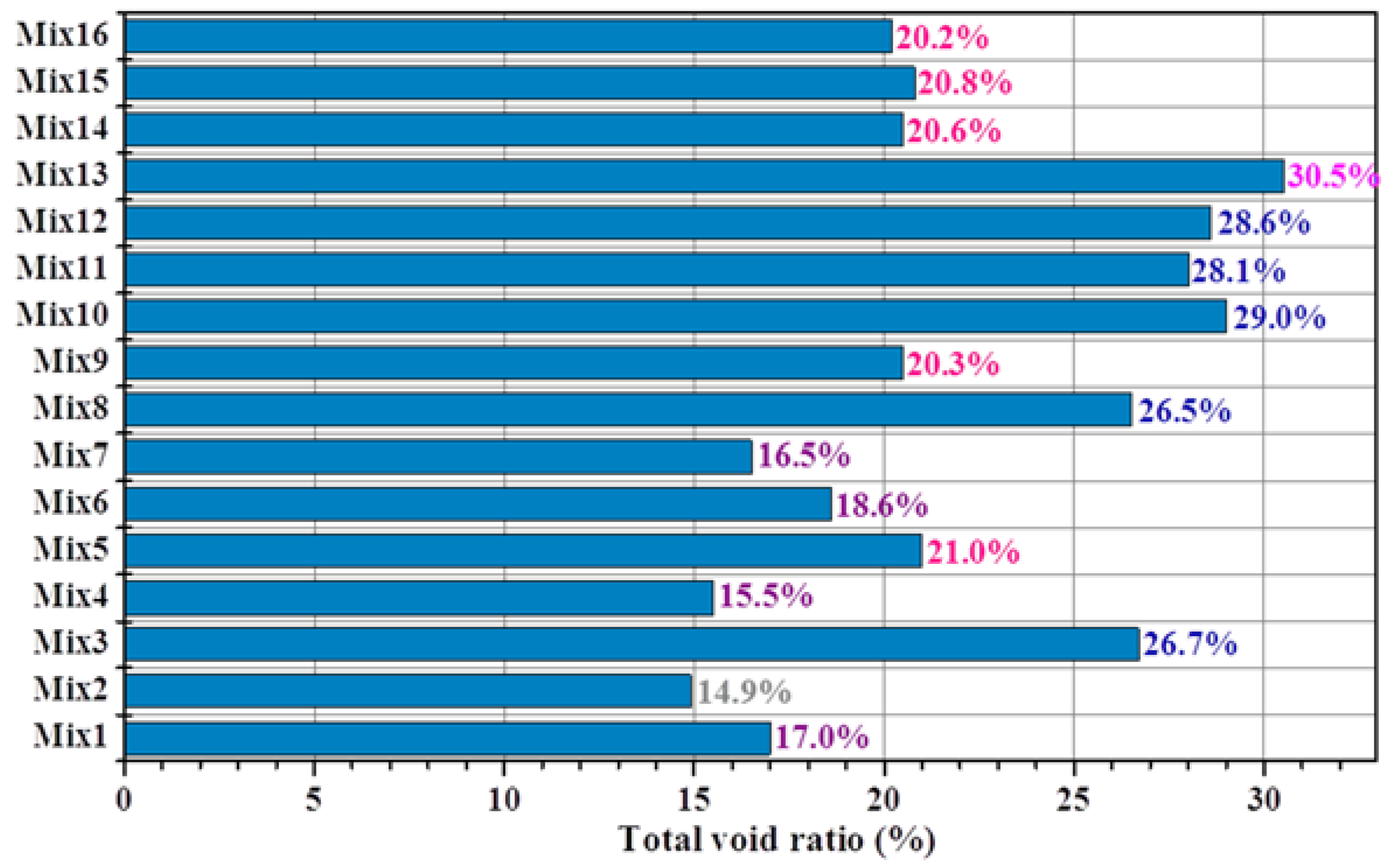
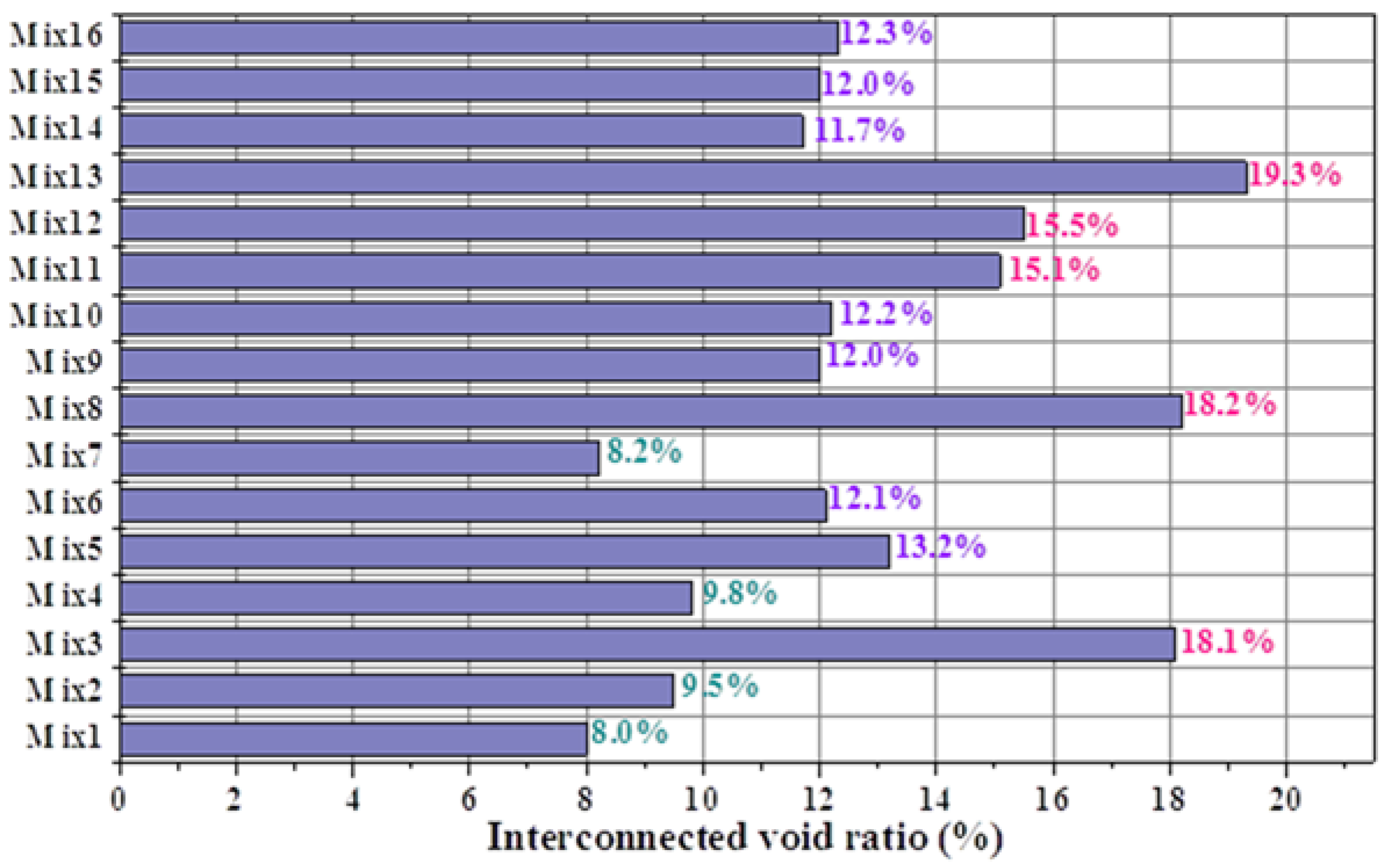
| Mixture No. | Mixture Design | Responses | |||
|---|---|---|---|---|---|
| W/C | PF (mm) | P/A | 28-Day Compressive Strength (MPa) | WPC (mm/s) | |
| Mix1 | 0.26 | 200.0 | 0.23 | 25.4 | 6.1 |
| Mix2 | 187.6 | 0.26 | 41.2 | 7 | |
| Mix3 | 160.0 | 0.23 | 23.5 | 10.4 | |
| Mix4 | 187.6 | 0.26 | 39.8 | 7.2 | |
| Mix5 | 160.0 | 0.26 | 34.5 | 8.5 | |
| Mix6 | 173.8 | 0.24 | 37.0 | 8.8 | |
| Mix7 | 200.0 | 0.23 | 25.0 | 6.2 | |
| Mix8 | 164.0 | 0.19 | 27.1 | 10.6 | |
| Mix9 | 192.7 | 0.20 | 33.5 | 7.5 | |
| Mix10 | 200.0 | 0.16 | 23.1 | 7.6 | |
| Mix11 | 181.4 | 0.16 | 21.7 | 9.1 | |
| Mix12 | 181.4 | 0.16 | 21.2 | 9.1 | |
| Mix13 | 160.0 | 0.16 | 18.2 | 11 | |
| Mix14 | 177.2 | 0.20 | 30.6 | 8.3 | |
| Mix15 | 177.2 | 0.20 | 29.5 | 8.6 | |
| Mix16 | 177.2 | 0.20 | 28.9 | 8.8 | |
| 28-Day Compressive Strength | WPC | |||||||
|---|---|---|---|---|---|---|---|---|
| Source of Data | Sum of Squares | df | F-Value | p-Value > F-Value | Sum of Squares | df | F-Value | p-Value > F-Value |
| Model | 692.50 | 9 | 25.94 | 0.0004 | 31.42 | 9 | 41.06 | 0.0001 |
| A-PF | 5.55 | 1 | 1.87 | 0.2202 | 1.11 | 1 | 13.10 | 0.0111 |
| B-P/A ratio | 2.55 | 1 | 0.86 | 0.3899 | 0.014 | 1 | 0.17 | 0.6943 |
| AB | 26.67 | 1 | 8.99 | 0.0240 | 0.11 | 1 | 1.33 | 0.2919 |
| A2 | 78.89 | 1 | 26.60 | 0.0021 | 3.588 × 10−4 | 1 | 0.00422 | 0.9503 |
| B2 | 0.025 | 1 | 0.00833 | 0.9302 | 0.037 | 1 | 0.43 | 0.5349 |
| A2 B | 44.85 | 1 | 15.12 | 0.0081 | 0.056 | 1 | 0.66 | 0.4485 |
| AB2 | 8.86 | 1 | 2.99 | 0.1346 | 0.56 | 1 | 6.62 | 0.0422 |
| A3 | 0.96 | 1 | 0.32 | 0.5901 | 0.042 | 1 | 0.49 | 0.5082 |
| B3 | 25.51 | 1 | 8.60 | 0.0262 | 0.13 | 1 | 1.47 | 0.2704 |
| Residual | 17.80 | 6 | 0.51 | 6 | ||||
| Lack of fit | 15.13 | 1 | 28.31 | 0.8531 | 0.36 | 1 | 11.82 | 0.1185 |
| Pure error | 2.67 | 5 | 0.15 | 5 | ||||
| R2 | 0.9749 | 0.9840 | ||||||
| Adj. R2 | 0.9374 | 0.9601 | ||||||
| AP | 10.07 | 9.97 | ||||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, W.; Li, L.; Li, Y.; Li, Y.; Lu, L.; Cheng, X. Effect of Paste Fluidity and Paste-to-Aggregate Ratio on the Strength and Permeability of Porous Mortar from Manufactured Sand. Materials 2022, 15, 9065. https://doi.org/10.3390/ma15249065
Li W, Li L, Li Y, Li Y, Lu L, Cheng X. Effect of Paste Fluidity and Paste-to-Aggregate Ratio on the Strength and Permeability of Porous Mortar from Manufactured Sand. Materials. 2022; 15(24):9065. https://doi.org/10.3390/ma15249065
Chicago/Turabian StyleLi, Weichao, Laibo Li, Yali Li, Yanlin Li, Lingchao Lu, and Xin Cheng. 2022. "Effect of Paste Fluidity and Paste-to-Aggregate Ratio on the Strength and Permeability of Porous Mortar from Manufactured Sand" Materials 15, no. 24: 9065. https://doi.org/10.3390/ma15249065
APA StyleLi, W., Li, L., Li, Y., Li, Y., Lu, L., & Cheng, X. (2022). Effect of Paste Fluidity and Paste-to-Aggregate Ratio on the Strength and Permeability of Porous Mortar from Manufactured Sand. Materials, 15(24), 9065. https://doi.org/10.3390/ma15249065





