Influence of Doping on the Topological Surface States of Crystalline Bi2Se3 Topological Insulators
Abstract
:1. Introduction
2. Experimental
3. Results
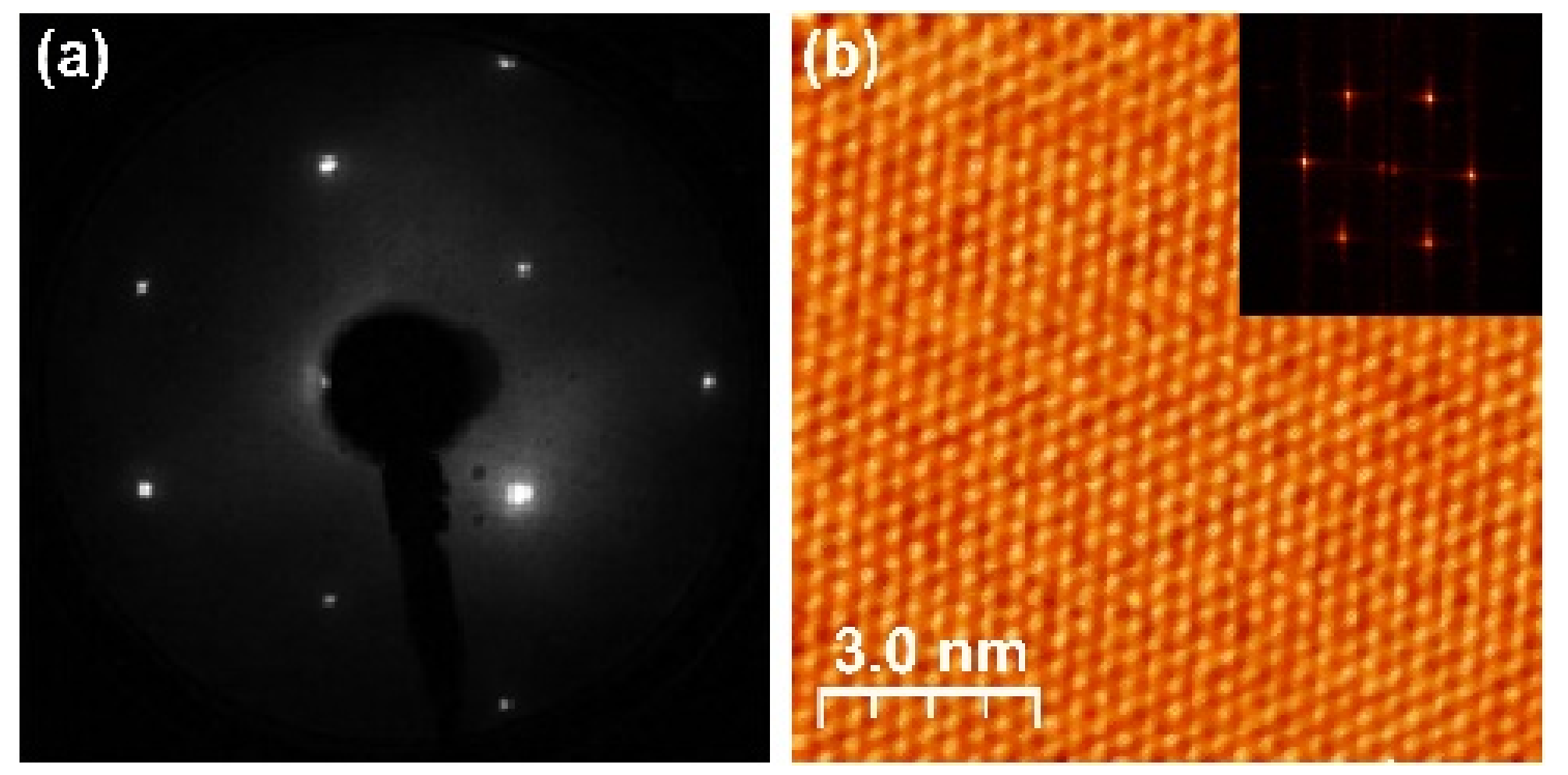
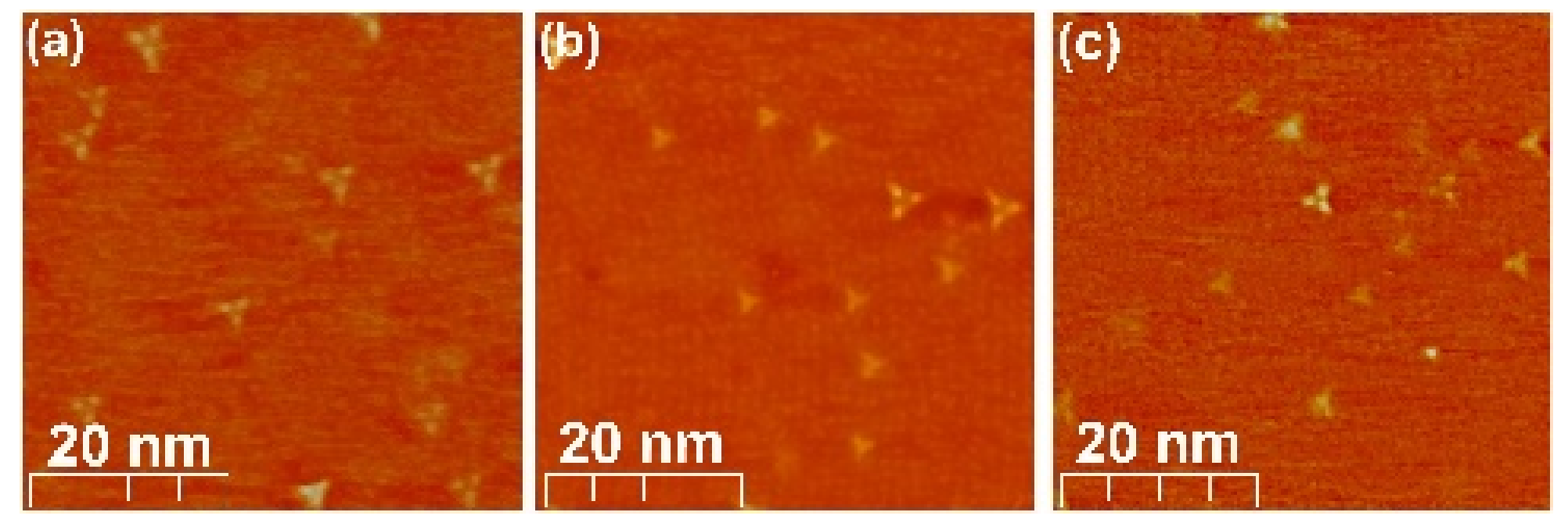
3.1. Scanning Tunneling Microscopy
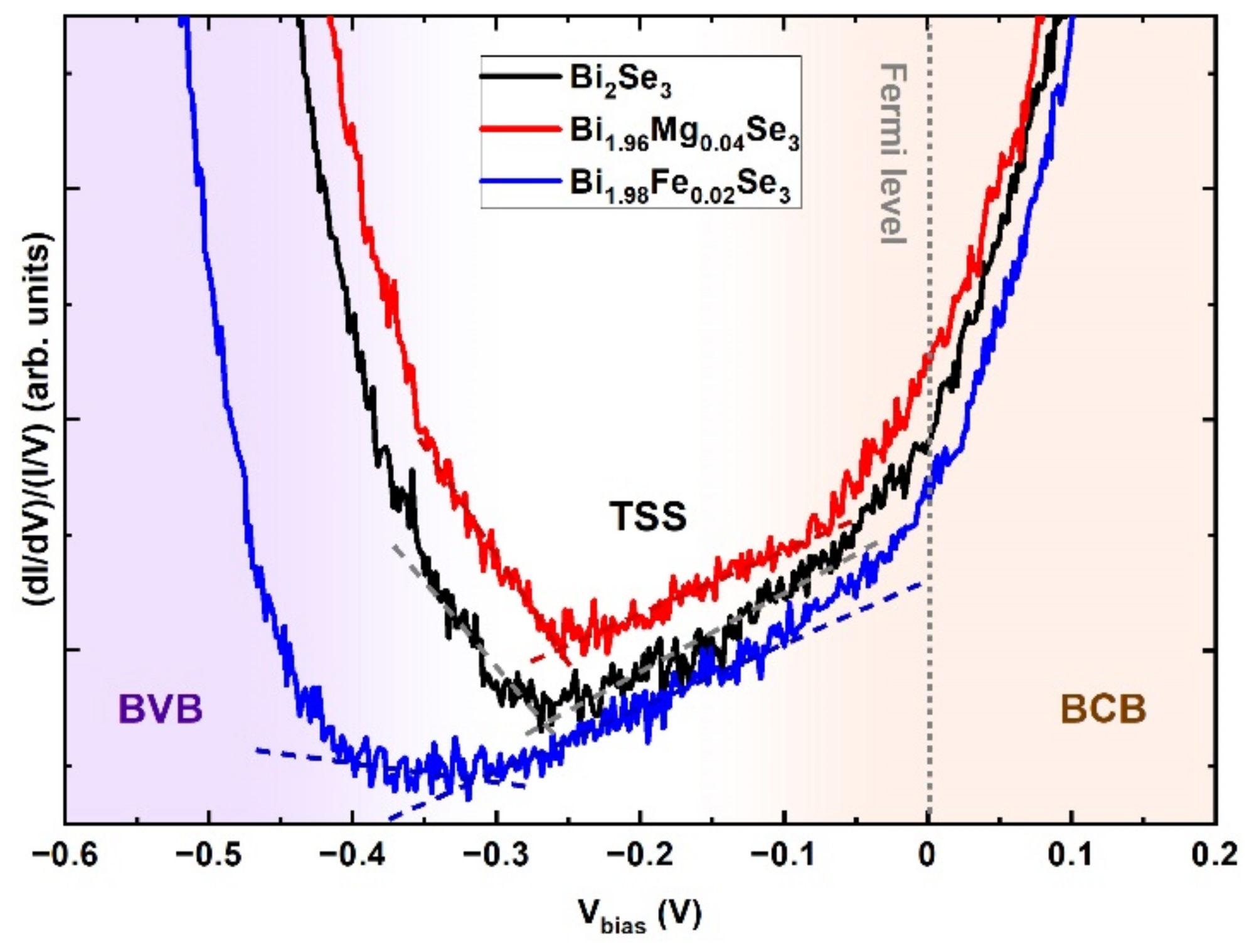
3.2. Scanning Tunneling Spectroscopy
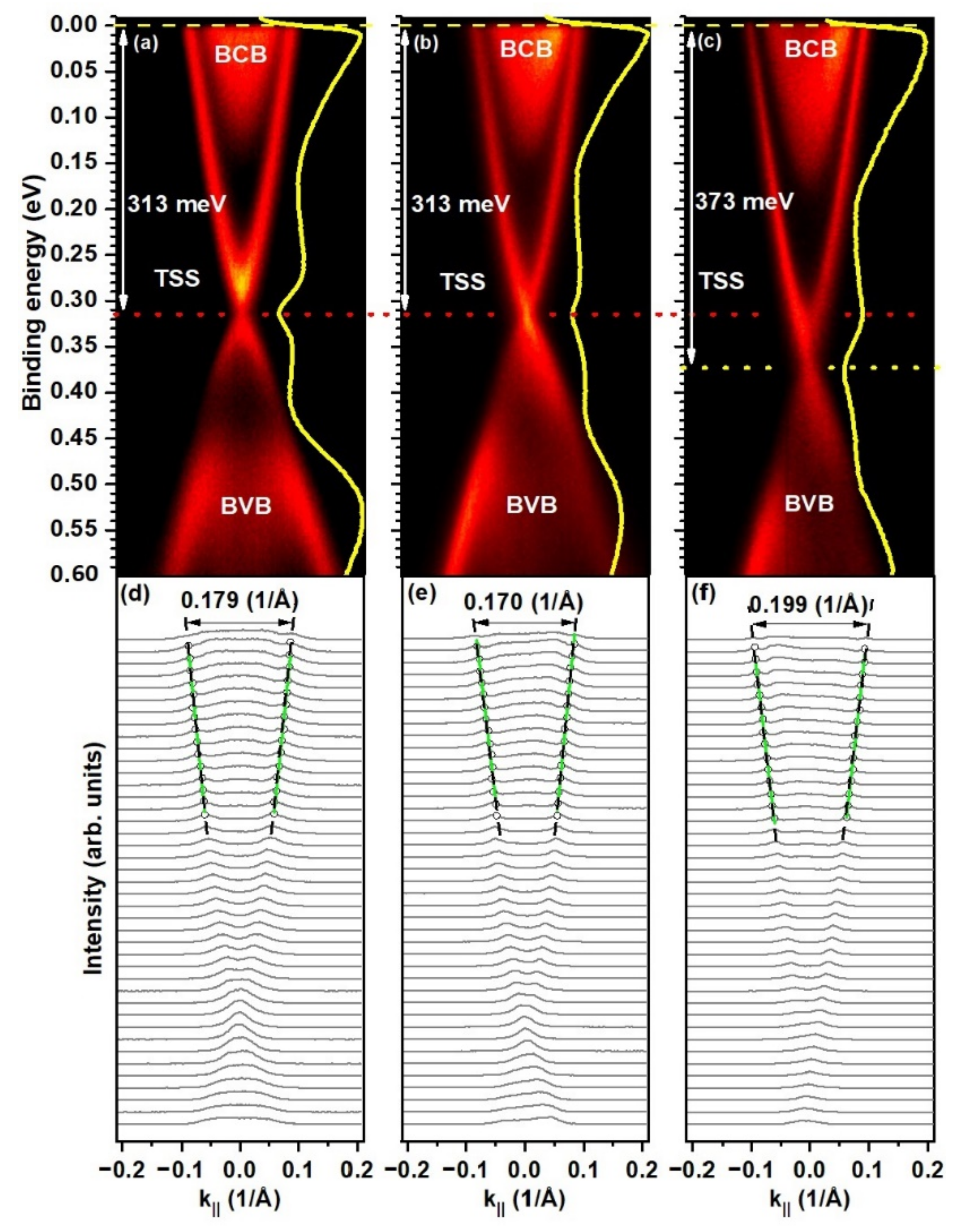
3.3. Angle–Resolved Photoemission Spectroscopy
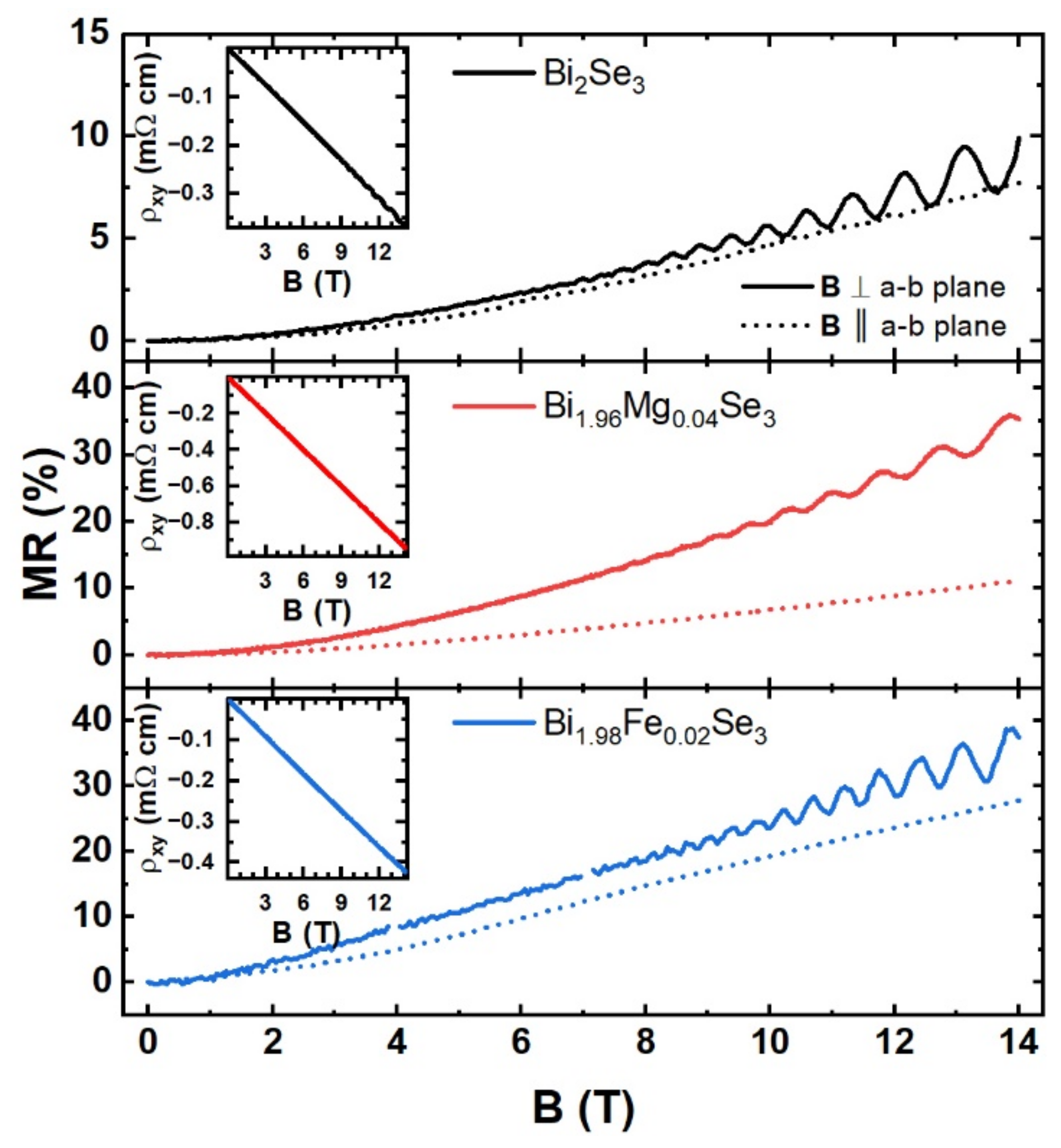
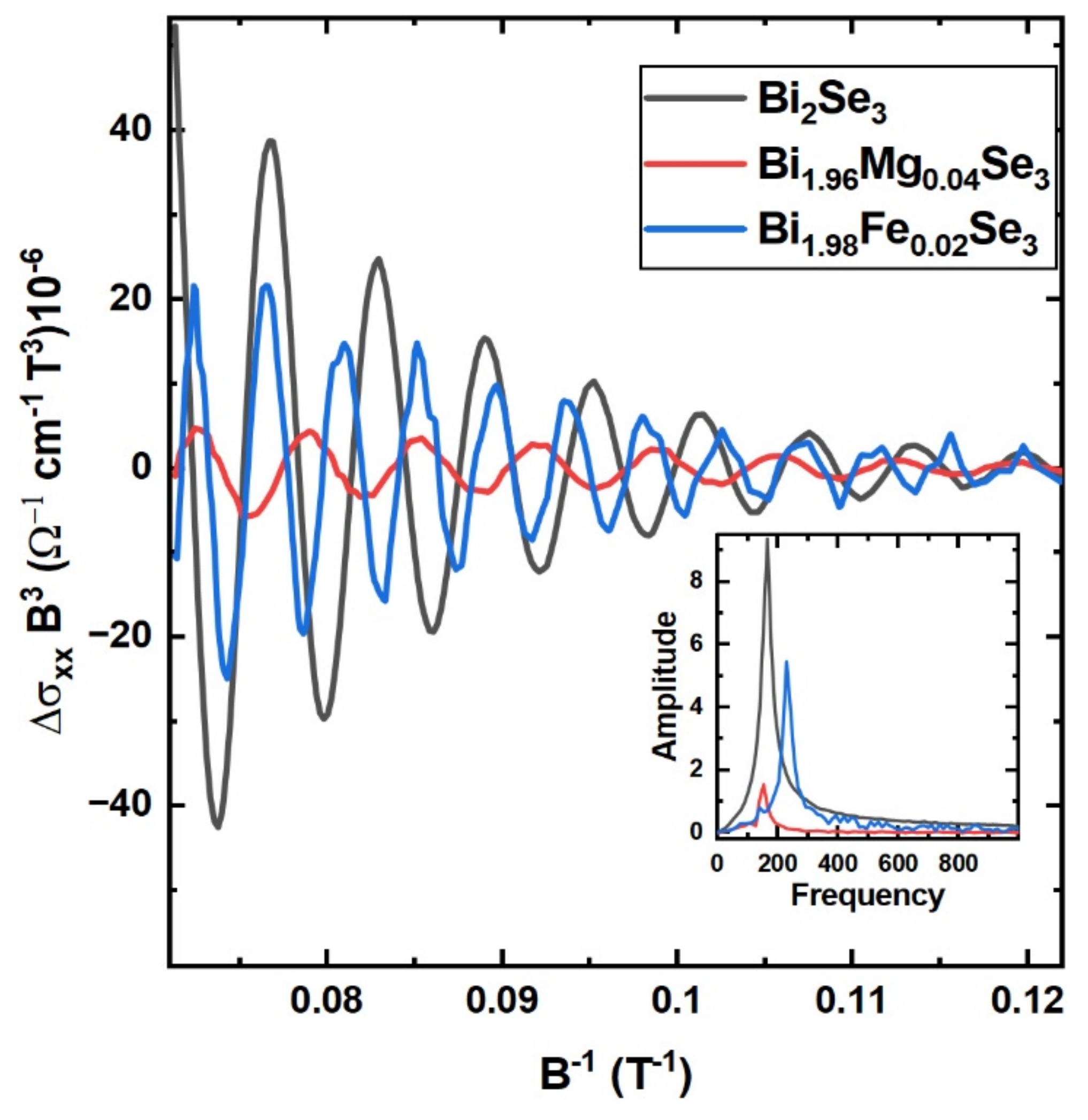
3.4. Magneto-Transport
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Roushan, P.; Seo, J.; Parker, C.V.; Hor, Y.S.; Hsieh, D.; Qian, D.; Richardella, A.; Hasan, M.Z.; Cava, R.J.; Yazdani, A. Topological surface states protected from backscattering by chiral spin texture. Nature 2009, 460, 1106–1109. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hsieh, D.; Qian, D.; Wray, L.; Xia, Y.; Hor, Y.S.; Cava, R.J.; Hasan, M.Z. A topological Dirac insulator in a quantum spin Hall phase. Nature 2008, 452, 970–974. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhang, H.; Liu, C.X.; Qi, X.L.; Dai, X.; Fang, Z.; Zhang, S.C. Topological insulators in Bi2Se3, Bi2Te3 and Sb2Te3 with a single Dirac cone on the surface. Nat. Phys. 2009, 5, 438–442. [Google Scholar] [CrossRef]
- Chen, Y.L.; Analytis, J.G.; Chu, J.H.; Liu, Z.K.; Mo, S.K.; Qi, X.L.; Zhang, H.J.; Lu, D.H.; Dai, X.; Fang, Z.; et al. Experimental Realization of a Three-Dimensional Topological Insulator, Bi2Te3. Science 2009, 325, 178–181. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Xia, Y.; Qian, D.; Hsieh, D.; Wray, L.; Pal, A.; Lin, H.; Bansil, A.; Grauer, D.; Hor, Y.S.; Cava, R.J.; et al. Observation of a large-gap topological-insulator class with a single Dirac cone on the surface. Nat. Phys. 2009, 5, 398–402. [Google Scholar] [CrossRef] [Green Version]
- Xu, N.; Xu, Y.; Zhu, J. Topological insulators for thermoelectrics. NPJ Quant Mater 2017, 2, 51. [Google Scholar] [CrossRef]
- Politano, A.; Viti, L.; Vitiello, M.S. Optoelectronic devices, plasmonics, and photonics with topological insulators. APL Mater. 2017, 5, 035504. [Google Scholar] [CrossRef] [Green Version]
- Wu, H.; Chen, A.; Zhang, P.; He, H.; Nance, J.; Gou, C.; Sasaki, J.; Shirokura, T.; Hai, P.N.; Fang, B.; et al. Magnetic memory driven by topological insulators. Nat. Commun. 2021, 12, 6251. [Google Scholar] [CrossRef]
- Navrátil, J.; Horák, J.; Plecháček, T.; Kamba, S.; Lošt’ák, P.; Dyck, J.S.; Chen, W.; Uher, C. Conduction band splitting and transport properties of Bi2Se3. J. Solid State Chem. 2004, 177, 1704–1712. [Google Scholar] [CrossRef]
- Gao, Y.B.; He, B.; Parker, D.; Androulakis, I.; Heremans, J.P. Experimental study of the valence band of Bi2Se3. Phys. Rev. B 2014, 90, 125204. [Google Scholar] [CrossRef] [Green Version]
- Song, C.L.; Jiang, Y.P.; Wang, Y.L.; Li, Z.; Wang, L.; He, K.; Chen, X.; Ma, X.C.; Xue, Q.K. Gating the charge state of single Fe dopants in the topological insulator Bi2Se3 with a scanning tunneling microscope. Phys. Rev. B 2012, 86, 045441. [Google Scholar] [CrossRef] [Green Version]
- Schlenk, T.; Bianchi, M.; Koleini, M.; Eich, A.; Pietzsch, O.; Wehling, T.O.; Frauenheim, T.; Balatsky, A.; Mi, J.-L.; Iversen, B.B.; et al. Controllable Magnetic Doping of the Surface State of a Topological Insulator. Phys. Rev. Lett. 2013, 110, 126804. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hor, Y.S.; Richardella, A.; Roushan, P.; Xia, Y.; Checkelsky, J.G.; Yazdani, A.; Hasan, M.Z.; Ong, N.P.; Cava, R.J. p-type Bi2Se3 for topological insulator and low-temperature thermoelectric applications. Phys. Rev. B 2009, 79, 195208. [Google Scholar] [CrossRef] [Green Version]
- Hsieh, D.; Xia, Y.; Qian, D.; Wray, L.; Dil, J.H.; Meier, F.; Osterwalder, J.; Patthey, L.; Checkelsky, J.G.; Ong, N.P.; et al. A tunable topological insulator in the spin helical Dirac transport regime. Nature 2009, 460, 1101–1105. [Google Scholar] [CrossRef] [Green Version]
- Kuroda, K.; Arita, M.; Miyamoto, K.; Ye, M.; Jiang, J.; Kimura, A.; Krasovskii, E.E.; Chulkov, E.V.; Iwasawa, H.; Okuda, T.; et al. Hexagonally Deformed Fermi Surface of the 3D Topological Insulator Bi2Se3. Phys. Rev. Lett. 2010, 105, 076802. [Google Scholar] [CrossRef] [Green Version]
- Liu, M.; Zhang, J.; Chang, C.Z.; Zhang, Z.; Feng, X.; Li, K.; He, K.; Wang, L.; Chen, X.; Dai, X.; et al. Crossover between Weak Antilocalization and Weak Localization in a Magnetically Doped Topological Insulator. Phys. Rev. Lett. 2012, 108, 036805. [Google Scholar] [CrossRef] [Green Version]
- Liu, W.; He, L.; Xu, Y.; Murata, K.; Onbasli, M.C.; Lang, M.; Maltby, N.J.; Li, S.; Wang, X.; Ross, C.A.; et al. Enhancing Magnetic Ordering in Cr-Doped Bi2Se3 Using High-TC Ferrimagnetic Insulator. Nano Lett. 2015, 15, 764–769. [Google Scholar] [CrossRef] [Green Version]
- Zhang, W.; West, D.; Lee, S.H.; Qiu, Y.; Chang, C.Z.; Moodera, J.S.; Hor, Y.S.; Zhang, S.; Wu, W. Electronic fingerprints of Cr and V dopants in the topological insulator Sb2Te3. Phys. Rev. B 2018, 98, 115165. [Google Scholar] [CrossRef] [Green Version]
- Chrobak, M.; Maćkosz, K.; Jurczyszyn, M.; Dobrzański, M.; Nowak, K.; Ślęzak, T.; Zając, M.; Sikora, M.; Rams, M.; Eelbo, T.; et al. Fe dopants and surface adatoms versus nontrivial topology of single-crystalline Bi2Se3. New J. Phys. 2020, 22, 063020. [Google Scholar] [CrossRef]
- Wray, L.A.; Xu, S.Y.; Xia, Y.; Hsieh, D.; Fedorov, A.V.; Hor, Y.S.; Cava, R.J.; Bansil, A.; Lin, H.; Hasan, M.Z. A topological insulator surface under strong Coulomb, magnetic and disorder perturbations. Nat. Phys. 2011, 7, 32–37. [Google Scholar] [CrossRef] [Green Version]
- Ye, M.; Eremeev, S.V.; Kuroda, K.; Krasovskii, E.E.; Chulkov, E.V.; Takeda, Y.; Saitoh, Y.; Okamoto, K.; Zhu, S.Y.; Miyamoto, K.; et al. Quasiparticle interference on the surface of Bi2Se3 induced by cobalt adatom in the absence of ferromagnetic ordering. Phys. Rev. B 2012, 85, 205317. [Google Scholar] [CrossRef] [Green Version]
- Walsh, L.A.; Green, A.J.; Addou, R.; Nolting, W.; Cormier, C.R.; Barton, A.T.; Mowll, T.R.; Yue, R.; Lu, N.; Kim, J.; et al. Fermi Level Manipulation through Native Doping in the Topological Insulator Bi2Se3. ACS Nano 2018, 12, 6310–6318. [Google Scholar] [CrossRef] [PubMed]
- Dai, J.; West, D.; Wang, X.; Wang, Y.; Kwok, D.; Cheong, S.W.; Zhang, S.B.; Wu, W. Toward the Intrinsic Limit of the Topological Insulator Bi2Se3. Phys. Rev. Lett. 2016, 117, 106401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Romanowich, M.; Lee, M.S.; Chung, D.Y.; Mahanti, S.D.; Kanatzidis, M.G.; Tessmer, S.H. Interplay of topological surface and bulk electronic states in Bi2Se3. Phys. Rev. B 2013, 87, 085310. [Google Scholar] [CrossRef] [Green Version]
- Tokura, Y.; Yasuda, K.; Tsukazaki, A. Magnetic topological insulators. Nat. Rev. Phys. 2019, 1, 126–143. [Google Scholar] [CrossRef]
- Ptok, A.; Kapcia, K.J.; Ciechan, A. Electronic properties of Bi2Se3 dopped by 3d transition metal (Mn, Fe, Co, or Ni) ions. J. Phys. Condens. Matter. 2021, 33, 065501. [Google Scholar] [CrossRef]
- Kumar, R.; Bhattacharyya, D. Ab-initio electronic structure simulations of transition metal doped Bi2Se3 topological insulator. Superlattices Microstruct. 2021, 159, 107033. [Google Scholar] [CrossRef]
- Cao, H.; Xu, S.; Miotkowski, I.; Tian, J.; Pandey, D.; Hasan, M.Z.; Chen, Y.P. Structural and electronic properties of highly doped topological insulator Bi2Se3 crystals. Phys. Status Solidi (RRL)-Rapid Res. Lett. 2013, 7, 133–135. [Google Scholar] [CrossRef] [Green Version]
- Horcas, I.; Fernández, R.; Gomez-Rodriguez, J.M.; Colchero, J.W.S.X.; Gómez-Herrero, J.W.S.X.M.; Baro, A.M. WSXM: A software for scanning probe microscopy and a tool for nanotechnology. Rev. Sci. Instrum. 2007, 78, 013705. [Google Scholar] [CrossRef]
- Jurczyszyn, M.; Sikora, M.; Chrobak, M.; Jurczyszyn, L. Studies of surface states in Bi2Se3 induced by the BiSe substitution in the crystal subsurface structure. Appl. Surf. Sci. 2020, 528, 146978. [Google Scholar] [CrossRef]
- Bardeen, J. Tunnelling from a Many-Particle Point of View. Phys. Rev. Lett. 1961, 6, 57. [Google Scholar] [CrossRef]
- Feenstra, R.M.; Stroscio, J.A.; Fein, A.P. Tunneling spectroscopy of the Si(111) 2×1 surface. Surf. Sci. 1987, 181, 295–306. [Google Scholar] [CrossRef]
- Feenstra, R.M. Tunneling spectroscopy of the (110) surface of direct-gap III-V semiconductors. Phys. Rev. B 1994, 50, 4561. [Google Scholar] [CrossRef] [PubMed]
- Urazhdin, S.; Bilc, D.; Tessmer, S.H.; Mahanti, S.D.; Kyratsi, T.; Kanatzidis, M.G. Scanning tunneling microscopy of defect states in the semiconductor Bi2Se3. Phys. Rev. B 2002, 66, 161306. [Google Scholar] [CrossRef] [Green Version]
- Ando, Y. Topological Insulator Materials. J. Phys. Soc. Jpn. 2013, 82, 102001. [Google Scholar] [CrossRef] [Green Version]
- Urazhdin, S.; Bilc, D.; Mahanti, S.D.; Tessmer, S.H.; Kyratsi, T.; Kanatzidis, M.G. Surface effects in layered semiconductors Bi2Se3 and Bi2Te3. Phys. Rev. B 2004, 69, 085313. [Google Scholar] [CrossRef]
- Xue, L.; Zhou, P.; Zhang, C.X.; He, C.Y.; Hao, G.L.; Sun, L.Z.; Zhong, J.X. First-principles study of native point defects in Bi2Se3. AIP Adv. 2013, 3, 052105. [Google Scholar] [CrossRef] [Green Version]
- Shoenberg, D. Magnetic Oscillations in Metals; Cambridge University Press: Cambridge, UK, 1984. [Google Scholar]
- Ren, Z.; Taskin, A.A.; Sasaki, S.; Segawa, K.; Ando, Y. Large bulk resistivity and surface quantum oscillations in the topological insulator Bi2Te2Se. Phys. Rev. B 2010, 82, 241306. [Google Scholar] [CrossRef] [Green Version]
- Chiatti, O.; Riha, C.; Lawrenz, D.; Busch, M.; Dusari, S.; Sánchez-Barriga, J.; Mogilatenko, A.; Yashina, L.V.; Valencia, S.; Ünal, A.A.; et al. 2D layered transport properties from topological insulator Bi2Se3 single crystals and micro flakes. Sci. Rep. 2016, 6, 27483. [Google Scholar] [CrossRef] [Green Version]
- Petrushevsky, M.; Lahoud, E.; Ron, A.; Maniv, E.; Diamant, I.; Neder, I.; Wiedmann, S.; Guduru, V.K.; Chiappini, F.; Zeitler, U.; et al. Probing the surface states in Bi2Se3 using the Shubnikov–de Haas effect. Phys. Rev. B 2012, 86, 045131. [Google Scholar] [CrossRef] [Green Version]
- Taskin, A.A.; Sasaki, S.; Segawa, K.; Ando, Y. Manifestation of Topological Protection in Transport Properties of Epitaxial Bi2Se3 Thin Films. Phys. Rev. Lett. 2012, 109, 066803. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wright, A.R.; McKenzie, R.H. Quantum oscillations and Berry’s phase in topological insulator surface states with broken particle-hole symmetry. Phys. Rev. B 2013, 87, 085411. [Google Scholar] [CrossRef] [Green Version]
- Eto, K.; Ren, Z.; Taskin, A.A.; Segawa, K.; Ando, Y. Angular-dependent oscillations of the magnetoresistance in Bi2Se3 due to the three-dimensional bulk Fermi surface. Phys. Rev. B 2010, 81, 195309. [Google Scholar] [CrossRef] [Green Version]

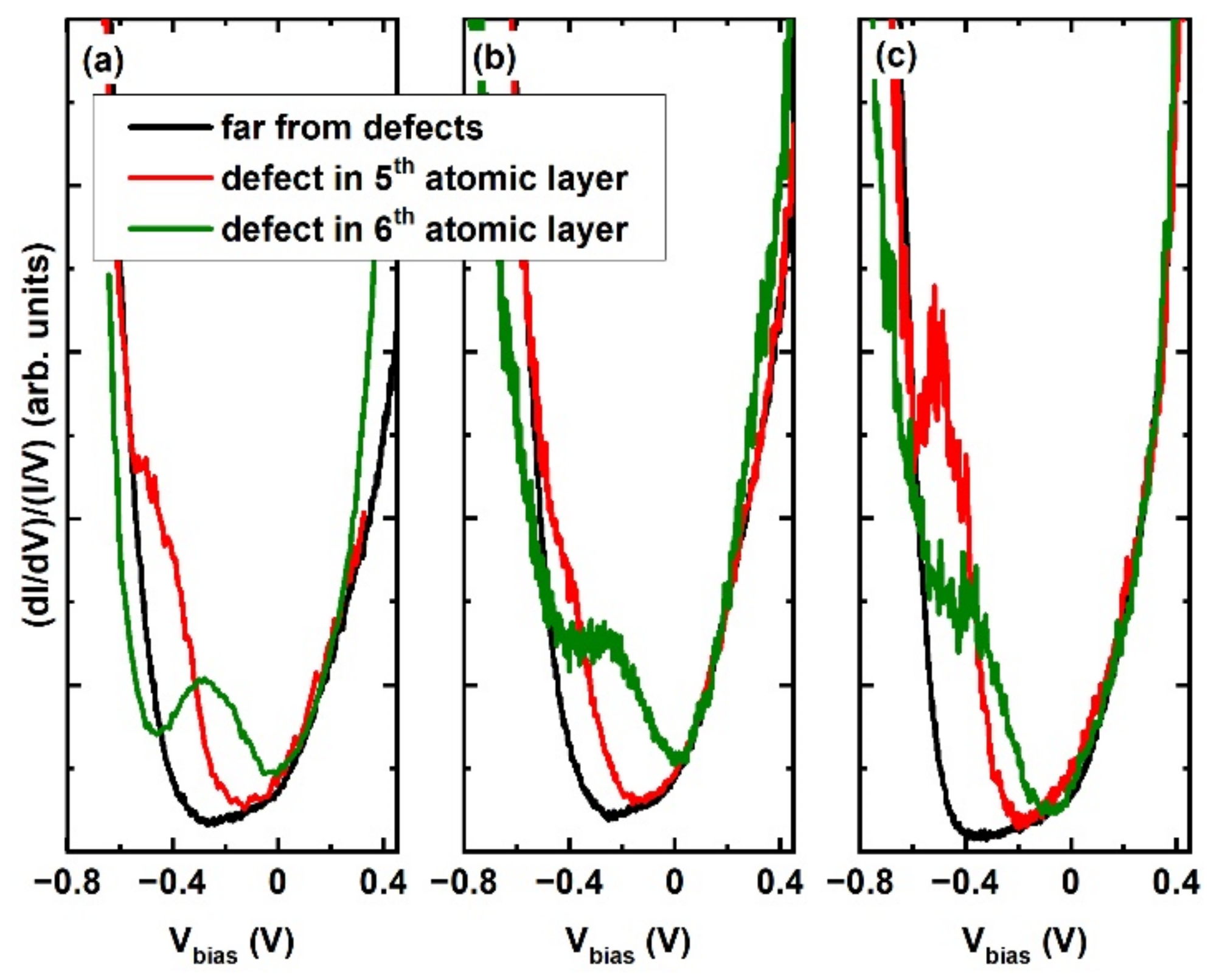
| Bi2Se3 (V) | Bi1.96Mg0.04Se3 (V) | Bi1.98Fe0.02Se3 (V) | |
|---|---|---|---|
| Defect in the 5th atomic layer | −0.41 | −039 | −0.45 |
| Defect in the 6th atomic layer | −0.26 | −0.26 | −0.32 |
| DP far from defects (from Figure 4) | −0.27 | −0.26 | −0.32 |
| Sample | 2∙kF (Å−1) | vF∙105 (m/s) | m* (me) | DP Position (eV) |
|---|---|---|---|---|
| Bi2Se3 | 0.180 | 6.788 | 0.154 | −0.313 |
| Bi1.96Mg0.04Se3 | 0.174 | 6.968 | 0.144 | −0.313 |
| Bi1.98Fe0.02Se3 | 0.201 | 5.940 | 0.197 | −0.373 |
| Sample | ρxx (B = 0) (mΩ∙cm) | nHall (1017 cm−3) | μ (cm2/Vs) | SdH Freq (T) | kF (Å−1) | n2D (1012 cm−2) |
|---|---|---|---|---|---|---|
| Bi2Se3 | 0.290 | 2.49 | 8720 | 165 | 0.071 | 3.98 |
| Bi1.96Mg0.04Se3 | 0.203 | 0.95 | 3245 | 152 | 0.068 | 3.67 |
| Bi1.98Fe0.02Se3 | 0.059 | 2.05 | 5128 | 230 | 0.084 | 5.55 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nowak, K.; Jurczyszyn, M.; Chrobak, M.; Maćkosz, K.; Naumov, A.; Olszowska, N.; Rosmus, M.; Miotkowski, I.; Kozłowski, A.; Sikora, M.; et al. Influence of Doping on the Topological Surface States of Crystalline Bi2Se3 Topological Insulators. Materials 2022, 15, 2083. https://doi.org/10.3390/ma15062083
Nowak K, Jurczyszyn M, Chrobak M, Maćkosz K, Naumov A, Olszowska N, Rosmus M, Miotkowski I, Kozłowski A, Sikora M, et al. Influence of Doping on the Topological Surface States of Crystalline Bi2Se3 Topological Insulators. Materials. 2022; 15(6):2083. https://doi.org/10.3390/ma15062083
Chicago/Turabian StyleNowak, Kamil, Michał Jurczyszyn, Maciej Chrobak, Krzysztof Maćkosz, Andrii Naumov, Natalia Olszowska, Marcin Rosmus, Ireneusz Miotkowski, Andrzej Kozłowski, Marcin Sikora, and et al. 2022. "Influence of Doping on the Topological Surface States of Crystalline Bi2Se3 Topological Insulators" Materials 15, no. 6: 2083. https://doi.org/10.3390/ma15062083
APA StyleNowak, K., Jurczyszyn, M., Chrobak, M., Maćkosz, K., Naumov, A., Olszowska, N., Rosmus, M., Miotkowski, I., Kozłowski, A., Sikora, M., & Przybylski, M. (2022). Influence of Doping on the Topological Surface States of Crystalline Bi2Se3 Topological Insulators. Materials, 15(6), 2083. https://doi.org/10.3390/ma15062083






