Effective Viscoplastic-Softening Model Suitable for Brain Impact Modelling
Abstract
1. Introduction
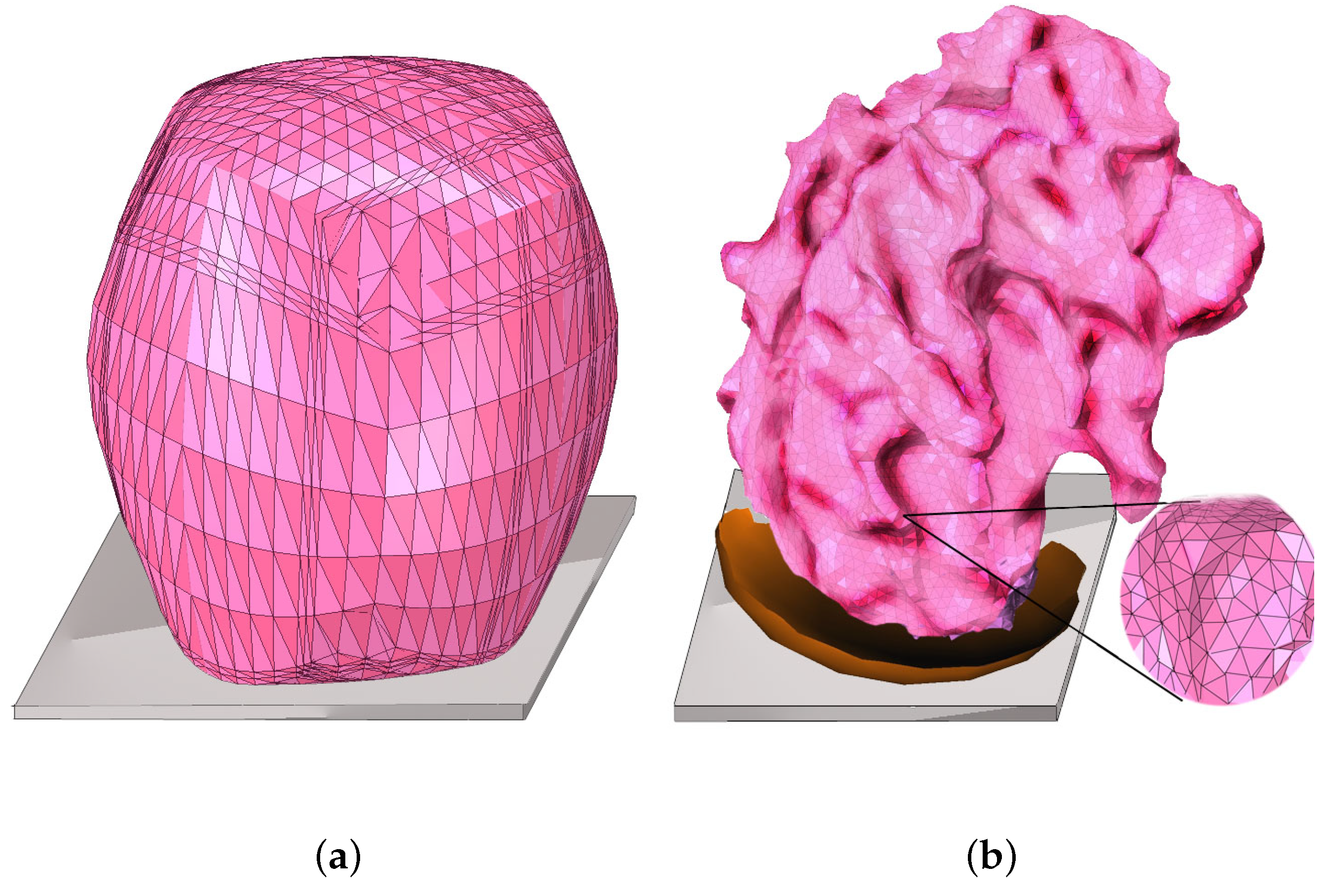
2. Materials and Methods
2.1. Beyond the Viscoelastic Model
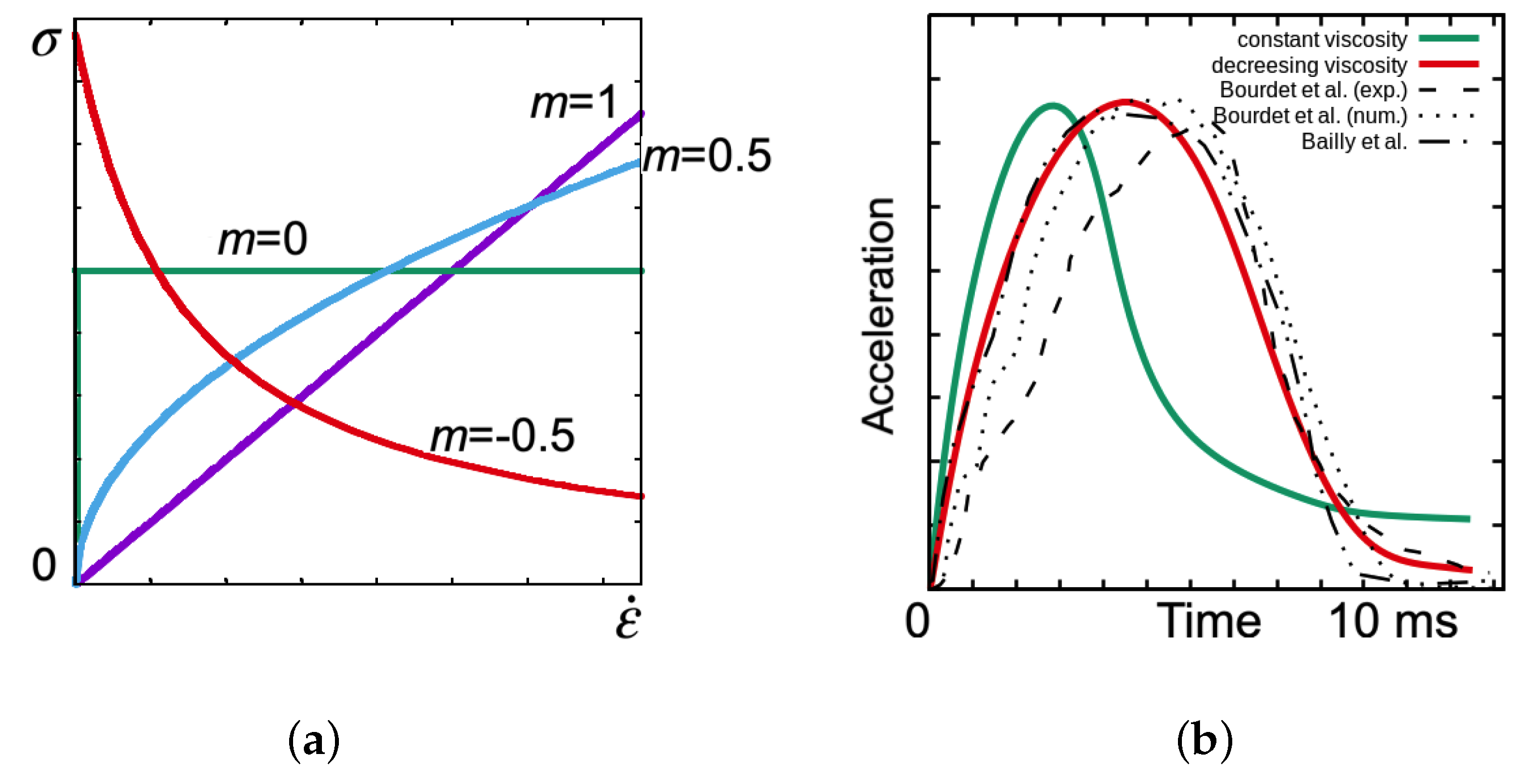
2.2. Space–Time Viscoplastic Model
3. Results
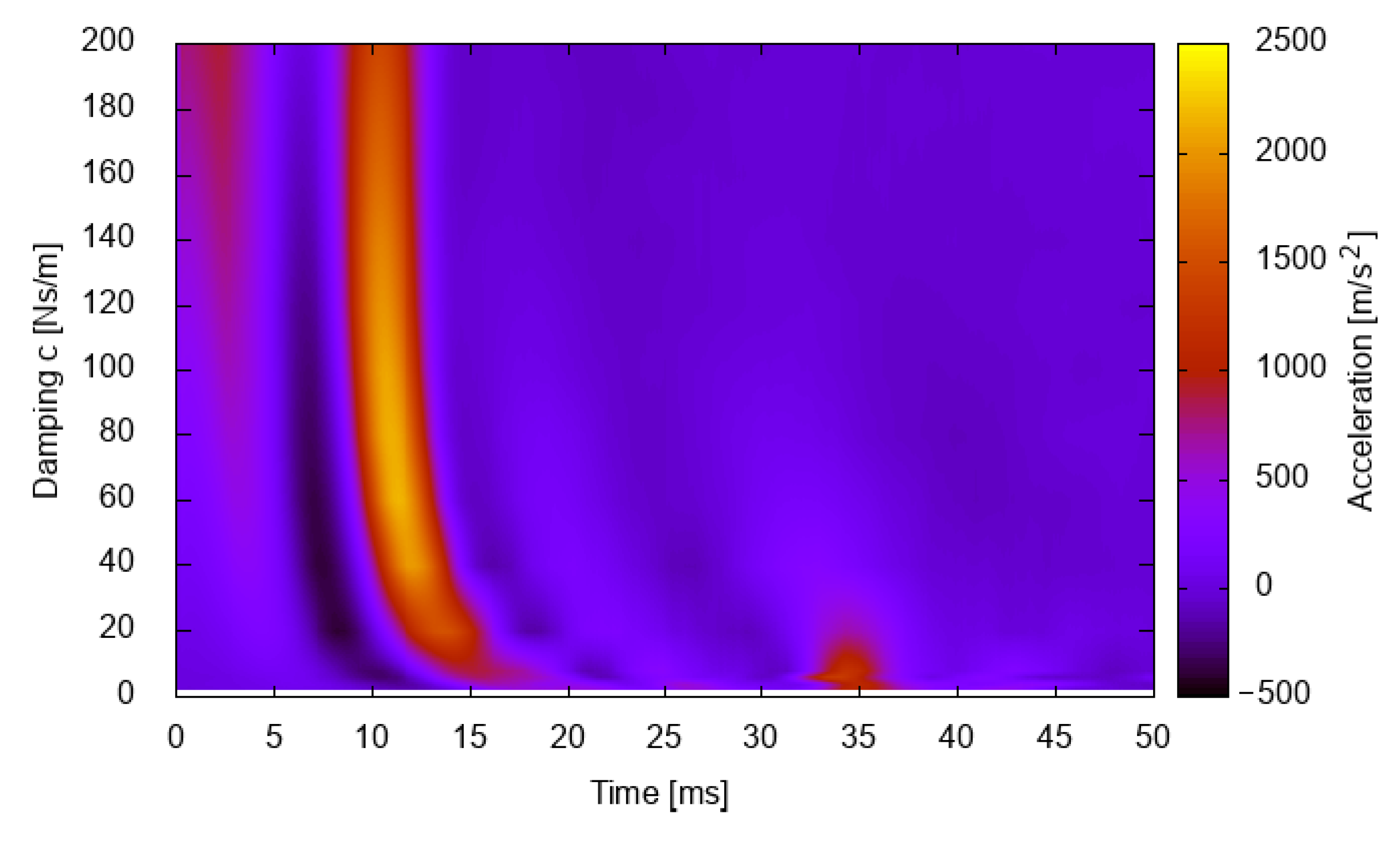
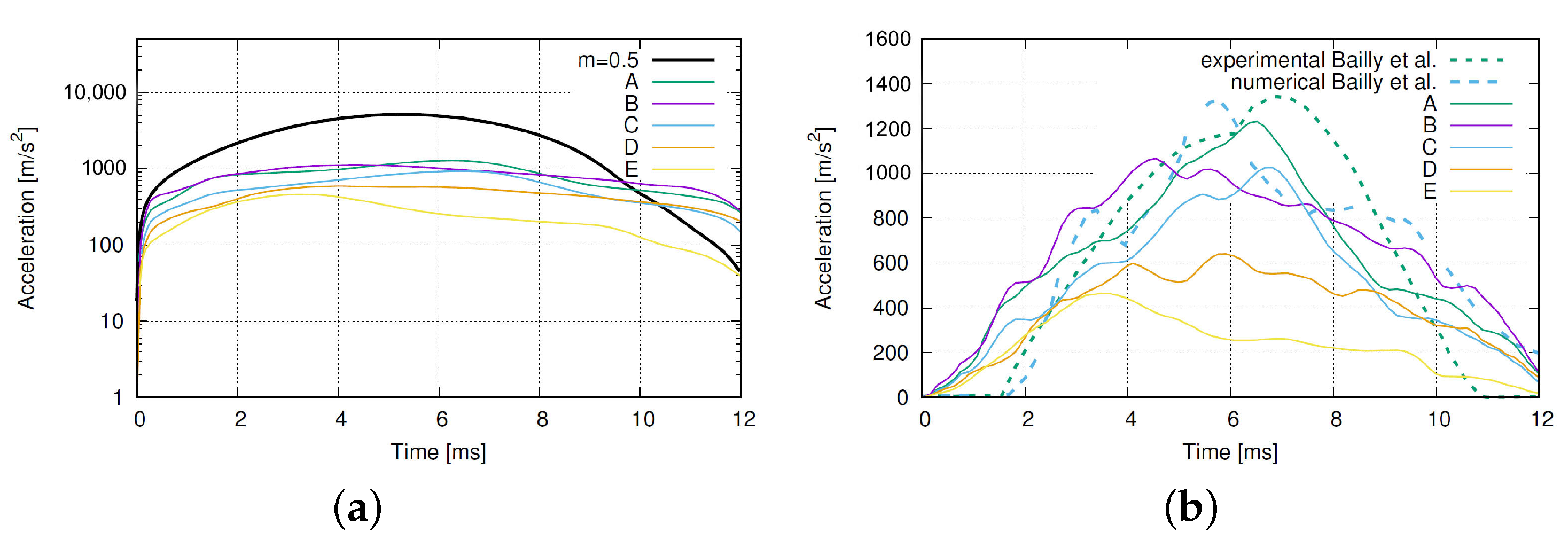
3.1. Parameter Identification
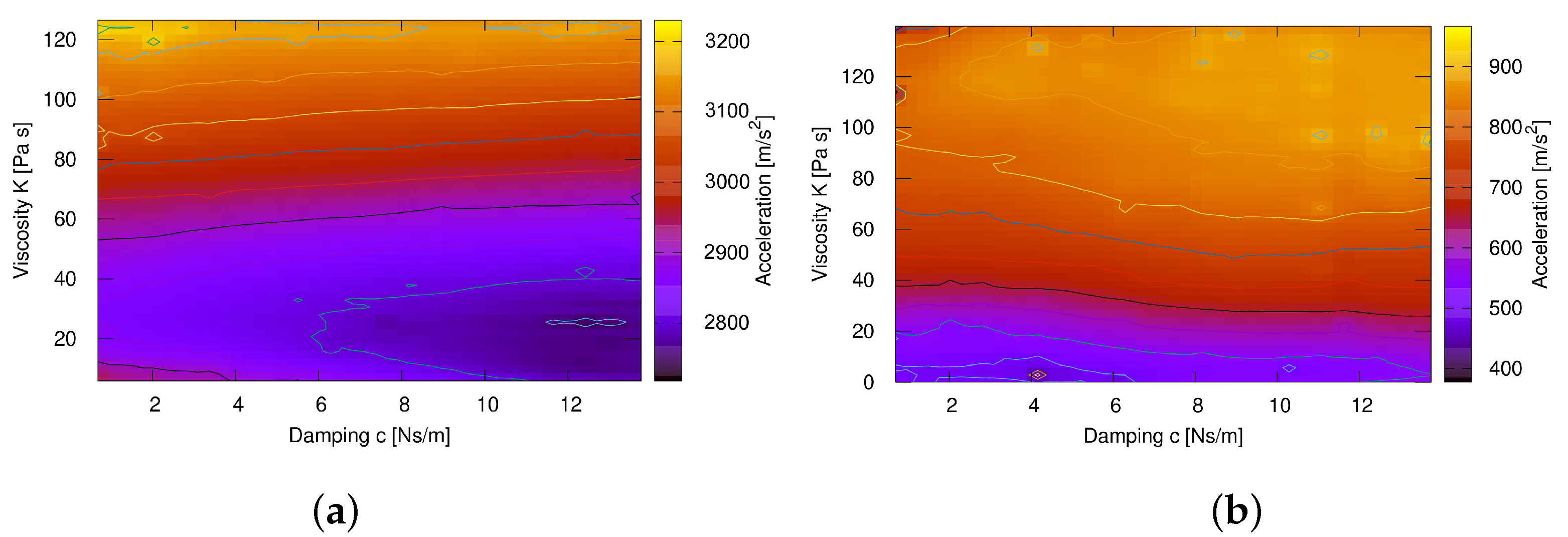
4. Simulation and Acceleration Mapping
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Miga, M.I.; Paulsen, K.D.; Kennedy, F.E.; Hoopes, P.J.; Hartov, A.; Roberts, D.W. Modeling surgical loads to account for subsurface tissue deformation during stereotactic neurosurgery. Photon. West Biomed. Opt. 1998, 3254, 501–511. [Google Scholar] [CrossRef]
- Raul, J.S.; Deck, C.; Willinger, R.; Ludes, B. Finite-element models of the human head and their applications in forensic practice. Int. J. Leg. Med. 2008, 122, 359–366. [Google Scholar] [CrossRef] [PubMed]
- Zuckerman, S.L.; Reynolds, B.B.; Yengo-Kahn, A.M.; Kuhn, A.W.; Chadwell, J.; Goodale, S.E.; Lafferty, C.E.; Langford, K.T.; McKeithan, L.J.; Kirby, P.; et al. A football helmet prototype that reduces linear and rotational acceleration with the addition of an outer shell. J. Neurosurg. 2018, 130, 1634–1641. [Google Scholar] [CrossRef]
- Zhao, W.; Choate, B.; Ji, S. Material properties of the brain in injury-relevant conditions – Experiments and computational modeling. J. Mech. Behav. Biomed. Mater. 2018, 80, 222–234. [Google Scholar] [CrossRef] [PubMed]
- Johnson, E.A.C.; Young, P.G. On the use of a patient-specific rapid-prototyped model to simulate the response of the human head to impact and comparison with analytical and finite element models. J. Biomech. 2005, 38, 39–45. [Google Scholar] [CrossRef]
- Kimpara, H.; Nakahira, Y.; Iwamoto, M.; Miki, K.; Ichihara, K.; Kawano, S.; Taguchi, T. Investigation of Anteroposterior Head-Neck Responses during Severe Frontal Impacts Using a Brain-Spinal Cord Complex FE Model. Stapp Car Crash J. 2006, 50, 509–544. [Google Scholar] [CrossRef]
- Rashid, B.; Destrade, M.; Gilchrist, M.D. Mechanical characterization of brain tissue in compression at dynamic strain rates. J. Mech. Behav. Biomed. Mater. 2012, 10, 23–38. [Google Scholar] [CrossRef]
- Tse, K.M.; Lim, S.P.; Tan, V.B.C.; Lee, H.P. A Review of Head Injury and Finite Element Head Models. J. Eng. Technol. 2015, 1, 28. [Google Scholar]
- Ratajczak, M.; Sąsiadek, M.; Będziński, R. An analysis of the effect of impact loading on the destruction of vascular structures in the brain. Acta Bioeng. Biomech. 2016, 18, 21–31. [Google Scholar] [CrossRef]
- De Rooij, R.; Kuhl, E. Constitutive Modeling of Brain Tissue: Current Perspectives. Appl. Mech. Rev. 2016, 68, 010801. [Google Scholar] [CrossRef]
- Eslaminejad, A.; Farid, M.H.; Ziejewski, M.; Karami, G. Brain tissue constitutive material models and the finite element analysis of blast-induced traumatic brain injury. Sci. Iran. 2018, 25, 3141–3150. [Google Scholar] [CrossRef]
- Brands, D.W.; Peters, G.W.; Bovendeerd, P.H. Design and numerical implementation of a 3-D non-linear viscoelastic constitutive model for brain tissue during impact. J. Biomech. 2004, 37, 127–134. [Google Scholar] [CrossRef]
- Prevost, T.P.; Balakrishnan, A.; Suresh, S.; Socrate, S. Biomechanics of brain tissue. Acta Biomater. 2011, 7, 83–95. [Google Scholar] [CrossRef] [PubMed]
- Calhoun, M.A.; Bentil, S.A.; Elliott, E.; Otero, J.J.; Winter, J.O.; Dupaix, R.B. Beyond Linear Elastic Modulus: Viscoelastic Models for Brain and Brain Mimetic Hydrogels. ACS Biomater. Sci. Eng. 2019, 5, 3964–3973. [Google Scholar] [CrossRef]
- Bailly, N.; Llari, M.; Donnadieu, T.; Masson, C.; Arnoux, P. Head impact in a snowboarding accident. Scand. J. Med. Sci. Sport 2017, 27, 964–974. [Google Scholar] [CrossRef]
- Gilchrist, M.D. Modelling and Accident Reconstruction of Head Impact Injuries. In Key Engineering Materials; Damage Assessment of Structures V. Trans Tech Publications Ltd.: Uetikon-Zurich, Switzerland, 2003; Volume 245, Available online: www.scientific.net/KEM.245-246.417 (accessed on 3 January 2022).
- Moran, R.; Smith, J.; Garcia, J. Fitted hyperelastic parameters for Human brain tissue from reported tension, compression, and shear tests. J. Biomech. 2014, 47, 3762–3766. [Google Scholar] [CrossRef]
- Bourdet, N.; Deck, C.; Tinard, V.; Willinger, R. Behaviour of helmets during head impact in real accident cases of motorcyclists. Int. J. Crashworth. 2012, 17, 51–61. [Google Scholar] [CrossRef]
- Wittek, A.; Bourantas, G.; Zwick, B.; Joldes, G.; Esteban, L.; Miller, K. Mathematical modeling and computer simulation of needle insertion into soft tissue. PLoS ONE 2020, 15, e0242704. [Google Scholar] [CrossRef]
- Yakovenko, A.A.; Lai, Y.X.; Goryacheva, I.G.; Ju, M.S.; Dosaev, M.Z.; Selyutskiy, Y.D. Modeling and experimental study of the needle indentation into a brain phantom. Int. J. Non-Linear Mech. 2021, 137, 103832. [Google Scholar] [CrossRef]
- Perepelyuk, M.; Chin, L.; Cao, X.; Oosten, A.; Shenoy, V.; Janmey, P.; Wells, R. Normal and Fibrotic Rat Livers Demonstrate Shear Strain Softening and Compression Stiffening: A Model for Soft Tissue Mechanics. PLoS ONE 2016, 11, e0146588. [Google Scholar] [CrossRef]
- Miller, K.; Chinzei, K. Constitutive modelling of brain tissue: Experiment and theory. J. Biomech. 1997, 30, 1115–1121. [Google Scholar] [CrossRef]
- Miller, K.; Chinzei, K. Mechanical properties of brain tissue in tension. J. Biomech. 2002, 35, 483–490. [Google Scholar] [CrossRef]
- Horgan, T.J.; Gilchrist, M.D. Influence of FE model variability in predicting brain motion and intracranial pressure changes in head impact simulations. Int. J. Crashworth. 2004, 9, 401–418. [Google Scholar] [CrossRef]
- Prabhu, R.; Begonia, M.; Whittington, W.; Mao, M.M.Y.; Liao, J.; Williams, L.; Horstemeyer, M.; Sheng, J. Compressive Mechanical Properties of Porcine Brain: Experimentation and Modeling of the Tissue Hydration Effects. Bioengineering 2019, 6, 40. [Google Scholar] [CrossRef] [PubMed]
- Ganpule, S.; Daphalapurkar, N.; Ramesh, K.; Knutsen, A.K.; Pham, D.L.; Bayly, P.; Prince, J.L. A Three-Dimensional Computational Human Head Model That Captures Live Human Brain Dynamics. J. Neurotrauma 2017, 34, 2154–2166. [Google Scholar] [CrossRef]
- Mihai, L.A.; Budday, S.; Holzapfel, G.; Kuhl, E.; Goriely, A. A family of hyperelastic models for human brain tissue. J. Mech. Phys. Solids 2017, 106, 60–79. [Google Scholar] [CrossRef]
- Hosseini-Farid, M.; Ramzanpour, M.; Ziejewski, M.; Karami, G. A compressible hyper-viscoelastic material constitutive model for human brain tissue and the identification of its parameters. Int. J. Non-Linear Mech. 2019, 116, 147–154. [Google Scholar] [CrossRef]
- Wu, S.W.; Jiang, C.; Jiang, C.; Liu, G.R. A selective smoothed finite element method with visco-hyperelastic constitutive model for analysis of biomechanical responses of brain tissues. Int. J. Numer. Methods Eng. 2020, 121, 5123–5149. [Google Scholar] [CrossRef]
- Ratajczak, M.; Ptak, M.; Chybowski, L.; Gawdzińska, K.; Będziński, R. Material and Structural Modeling Aspects of Brain Tissue Deformation under Dynamic Loads. Materials 2019, 12, 271. [Google Scholar] [CrossRef]
- Gasser, T.C.; Holzapfel, G.A. A rate-independent elastoplastic constitutive model for biological fiber-reinforced composites at finite strains: Continuum basis, algorithmic formulation and finite element implementation. Comput. Mech. 2002, 29, 340–360. [Google Scholar] [CrossRef]
- Franceschini, G.; Bigoni, D.; Regitnig, P.; Holzapfel, G. Brain tissue deforms similarly to filled elastomers and follows consolidation theory. J. Mech. Phys. Solids 2006, 54, 2592–2620. [Google Scholar] [CrossRef]
- El Sayed, T.; Mota, A.; Fraternali, F.; Ortiz, M. A variational constitutive model for soft biological tissues. J. Biomech. 2008, 41, 1458–1466. [Google Scholar] [CrossRef] [PubMed]
- Bergström, J.S.; Boyce, M.C. Constitutive modeling of the time-dependent and cyclic loading of elastomers and application to soft biological tissues. Mech. Mater. 2001, 33, 523–530. [Google Scholar] [CrossRef]
- Ruan, J.S.; Khalil, T.; King, A.I. Dynamic response of the human head to impact by three-dimensional finite element analysis. J. Biomech. Eng. 1994, 116 1, 44–50. [Google Scholar] [CrossRef]
- Brands, D.W.; Bovendeerd, P.H.; Wismans, J. On the potential importance of non-linear viscoelastic material modelling for numerical prediction of brain tissue response: Test and application. Stapp Car Crash J 2002, 46, 103–121. [Google Scholar] [CrossRef][Green Version]
- Ashrafi, H.; Shariyat, M. A Visco-hyperelastic model for prediction of the brain tissue response and the traumatic brain injuries. Arch. Trauma Res. 2017, 6, 41–48. [Google Scholar] [CrossRef]
- El Sayed, T.; Mota, A.; Fraternali, F.; Ortiz, M. Biomechanics of traumatic brain injury. Comput. Methods Appl. Mech. Eng. 2008, 197, 4692–4701. [Google Scholar] [CrossRef]
- Miller, K.; Joldes, G.; Bourantas, G.; Warfield, S.; Hyde, D.; Kikinis, R.; Wittek, A. Biomechanical modeling and computer simulation of the brain during neurosurgery. Int. J. Numer. Method Biomed. Eng. 2019, 35, e3250. [Google Scholar] [CrossRef] [PubMed]
- Wang, F.; Han, Y.; Wang, B.; Peng, Q.; Huang, X.; Miller, K.; Wittek, A. Prediction of brain deformations and risk of traumatic brain injury due to closed-head impact: Quantitative analysis of the effects of boundary conditions and brain tissue constitutive model. Biomech. Model. Mechanobiol. 2018, 17, 1165–1185. [Google Scholar] [CrossRef]
- Budday, S.; Ovaert, T.; Holzapfel, G.; Steinmann, P.; Kuhl, E. Fifty Shades of Brain: A Review on the Mechanical Testing and Modeling of Brain Tissue. Arch. Comput. Methods Eng. 2020, 27, 1187–1230. [Google Scholar] [CrossRef]
- Hoff, H.J. Approximate analysis of structures in presence of moderately large creep deformations. Q. Appl. Math. 1954, 12, 49–55. [Google Scholar] [CrossRef]
- Bajer, C.I.; Bohatier, C. The soft way method and the velocity formulation. Comput. Struct. 1995, 55, 1015–1025. [Google Scholar] [CrossRef]
- Maxey, J. Viscosity and Gel Structure: The Unseen Results of Their Manipulation. In Proceedings of the 2011 AADE National Technical Conference and Exhibition, Houston, TX, USA, 12–14 April 2011; pp. 1–10. [Google Scholar]
- Hang, S. TetGen, a Delaunay-Based Quality Tetrahedral Mesh Generator. ACM Trans. Math. Softw. 2015, 41, 36. [Google Scholar] [CrossRef]




| Case | Coefficient s | Acceleration [m/s] |
|---|---|---|
| case with m = 0.5 | — | 2750 |
| m = −0.5, case A | 2.00 | 1260 |
| m = −0.5, case B | 1.00 | 1070 |
| m = −0.5, case C | 0.50 | 1030 |
| m = −0.5, case D | 0.20 | 650 |
| m = −0.5, case E | 0.05 | 470 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dyniewicz, B.; Bajkowski, J.M.; Bajer, C.I. Effective Viscoplastic-Softening Model Suitable for Brain Impact Modelling. Materials 2022, 15, 2270. https://doi.org/10.3390/ma15062270
Dyniewicz B, Bajkowski JM, Bajer CI. Effective Viscoplastic-Softening Model Suitable for Brain Impact Modelling. Materials. 2022; 15(6):2270. https://doi.org/10.3390/ma15062270
Chicago/Turabian StyleDyniewicz, Bartłomiej, Jacek M. Bajkowski, and Czesław I. Bajer. 2022. "Effective Viscoplastic-Softening Model Suitable for Brain Impact Modelling" Materials 15, no. 6: 2270. https://doi.org/10.3390/ma15062270
APA StyleDyniewicz, B., Bajkowski, J. M., & Bajer, C. I. (2022). Effective Viscoplastic-Softening Model Suitable for Brain Impact Modelling. Materials, 15(6), 2270. https://doi.org/10.3390/ma15062270








