Static Electro-Mechanical Response of Axisymmetric One-Dimensional Piezoelectric Quasicrystal Circular Actuator
Abstract
:1. Introduction
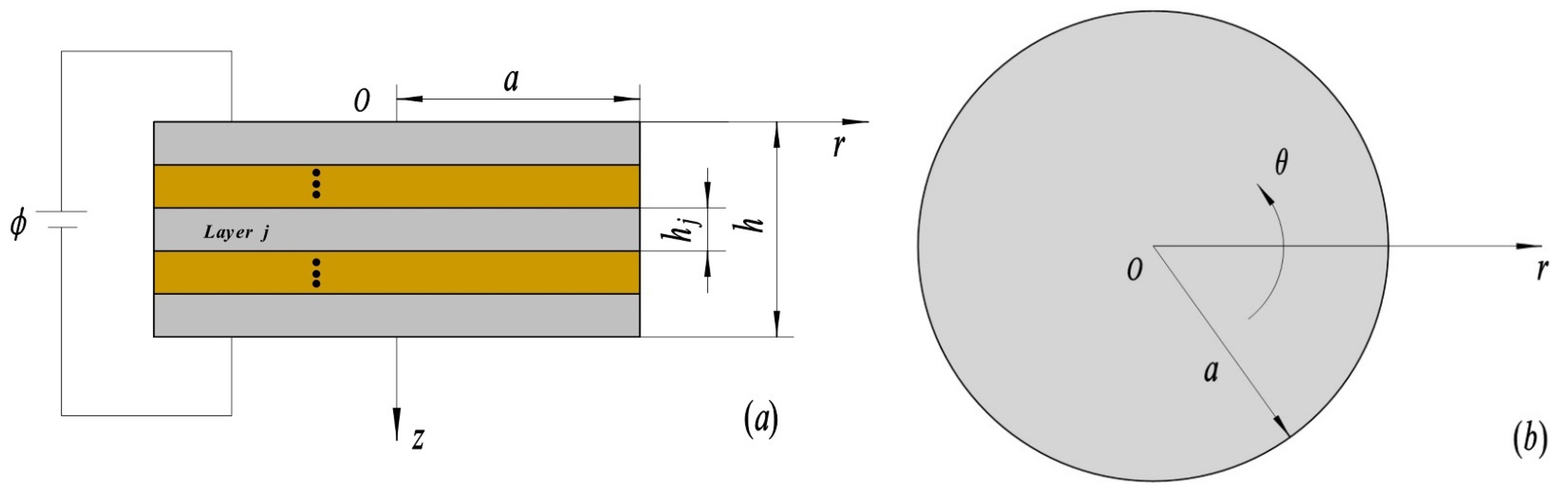
2. Description of Actuator and Governing Equations
3. State Formulation and Hankel Transform
4. Boundary Condition and Its Solutions
5. Numerical Examples
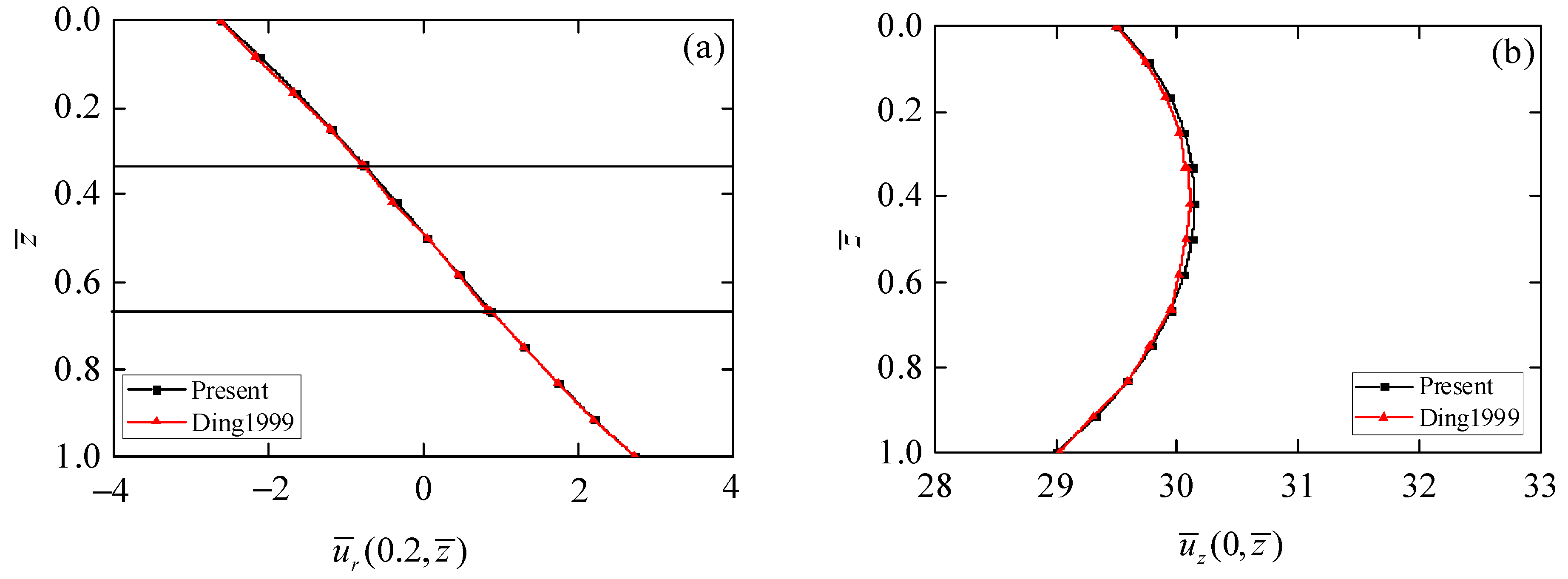
5.1. Verification of the Present Method
5.2. Effect of the Thickness to Span Ratio on the Circular Actuator
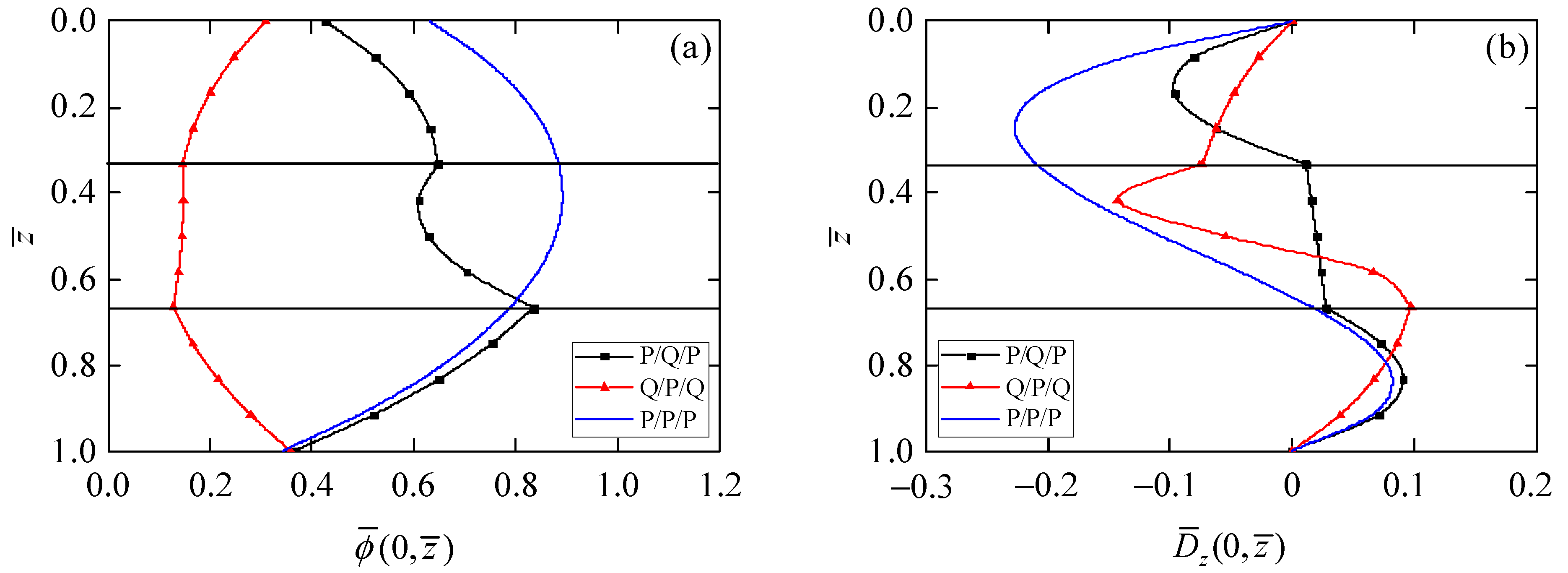
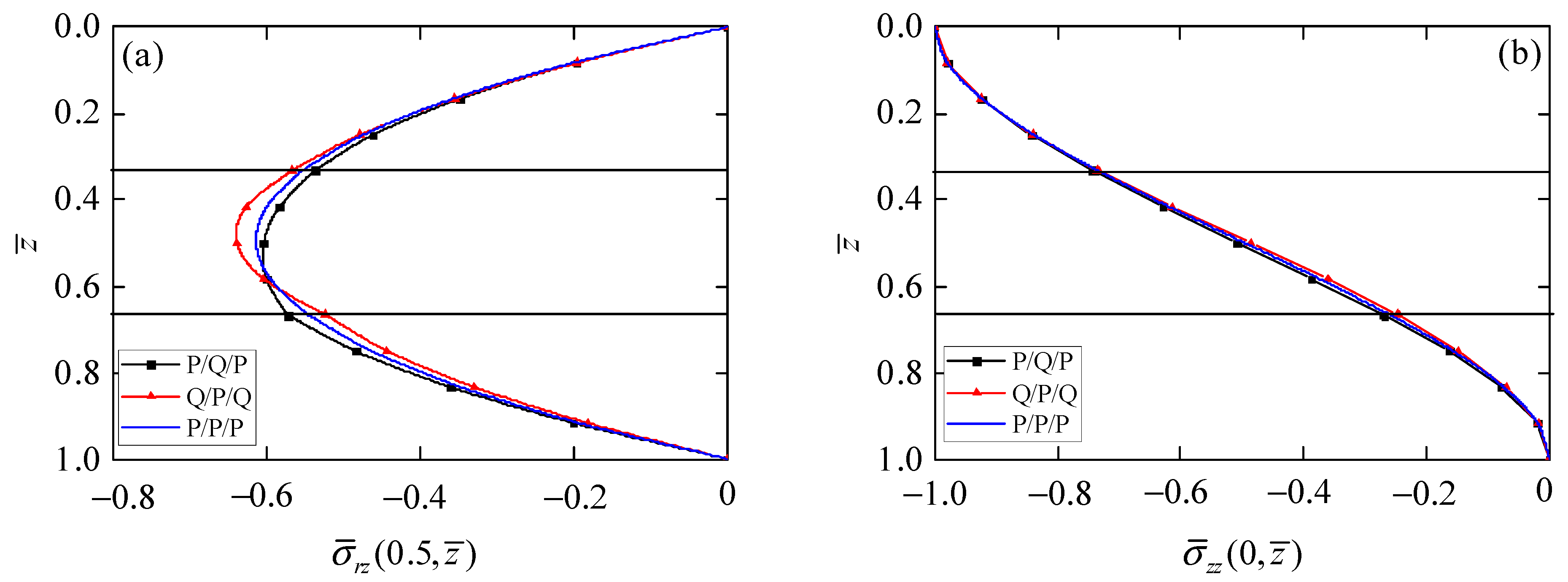
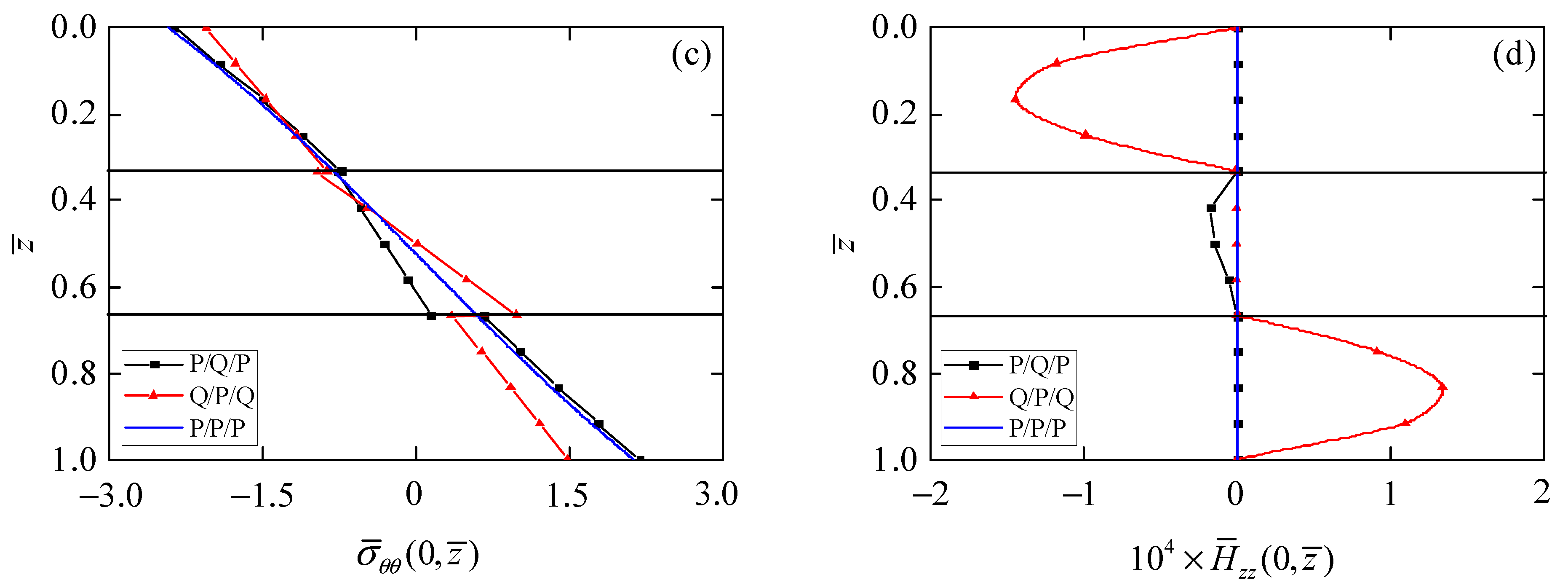
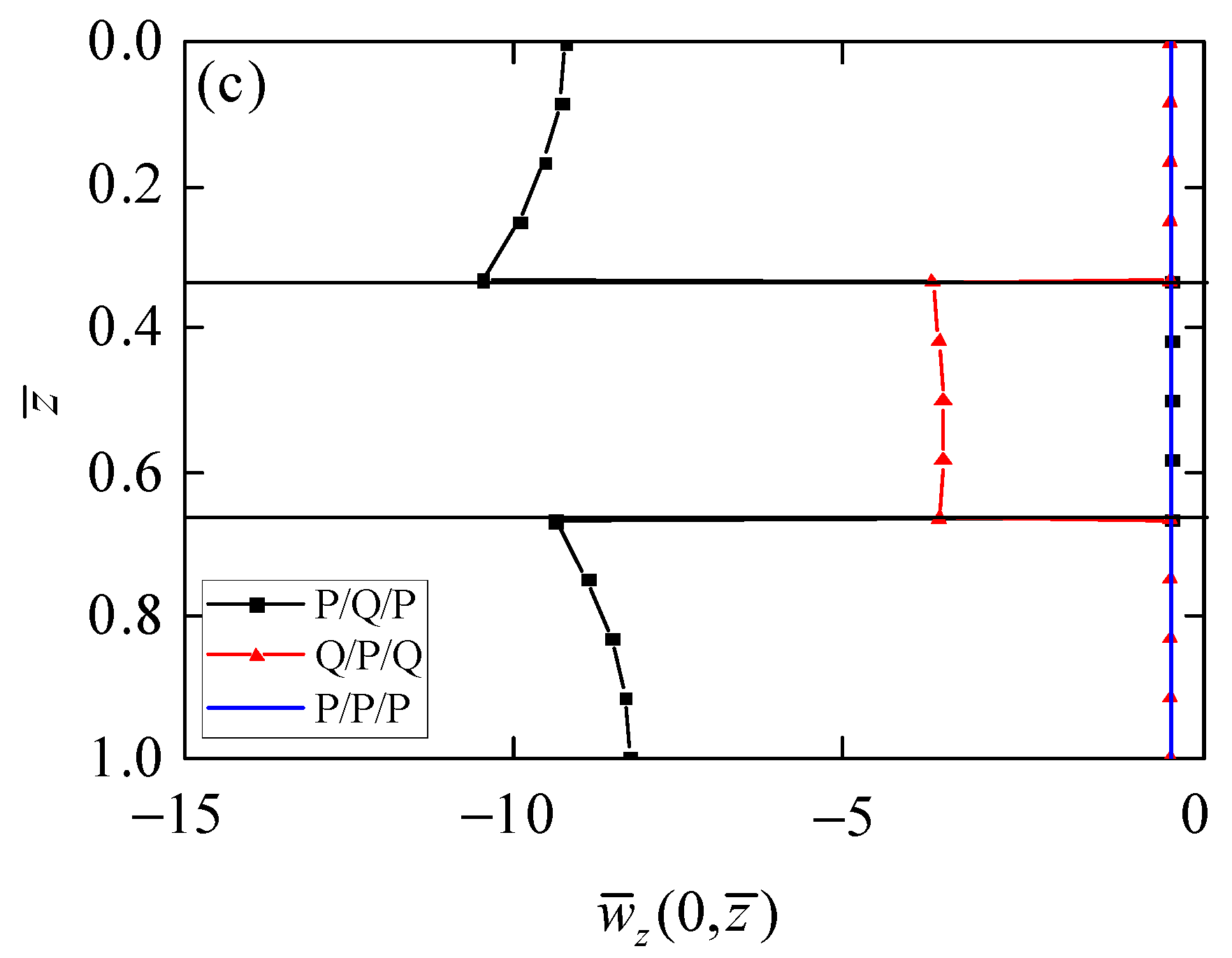
5.3. Effect of the Stacking Sequence on the Circular Actuator
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Ozdemir, O.; Kaya, M.O. Energy derivation and extension-flapwise bending vibration analysis of a rotating piezolaminated composite timoshenko beam. Mech. Adv. Mater. Struct. 2014, 21, 477–489. [Google Scholar] [CrossRef]
- Wei, C.P.; Xue, C.X. Bending waves of a rectangular piezoelectric laminated beam. Acta Mech. Sin. 2020, 36, 1099–1108. [Google Scholar] [CrossRef]
- Zhu, C.; Fang, X.; Liu, J.; Nie, G.; Zhang, C. An analytical solution for nonlinear vibration control of sandwich shallow doubly-curved nanoshells with functionally graded piezoelectric nanocomposite sensors and actuators. Mech. Based Des. Struct. Mach. 2020, 1–27. [Google Scholar] [CrossRef]
- Dong, Y.; Li, Y.; Li, X.; Yang, J. Active control of dynamic behaviors of graded graphene reinforced cylindrical shells with piezoelectric actuator/sensor layers. Appl. Math. Model. 2020, 82, 252–270. [Google Scholar] [CrossRef]
- Dehsaraji, M.L.; Loghman, A.; Arefi, M. Three-dimensional thermo-electro-mechanical buckling analysis of functionally graded piezoelectric micro/nano-shells based on modified couple stress theory considering thickness stretching effect. Mech. Adv. Mater. Struct. 2021, 28, 2030–2045. [Google Scholar] [CrossRef]
- Min, H.; Zhang, J.; Fan, M. Size effect of a piezoelectric patch on a rectangular plate with the neural network model. Materials 2021, 14, 3240. [Google Scholar] [CrossRef] [PubMed]
- Shechtman, D.; Blech, I.; Gratias, D.; Cahn, J.W. Metallic phase with long-range orientational order and no translational symmetry. Phys. Rev. Lett. 1984, 53, 1951–1953. [Google Scholar] [CrossRef] [Green Version]
- Bak, P. Phenomenological theory of icosahedral incommensurate (“quasiperiodic”) order in Mn-Al alloys. Phys. Rev. Lett. 1985, 54, 1517–1519. [Google Scholar] [CrossRef] [PubMed]
- Hu, C.Z.; Wang, R.H.; Ding, D.H.; Yang, W.G. Piezoelectric effects in quasicrystals. Phys. Rev. B 1997, 56, 2463–2468. [Google Scholar] [CrossRef]
- Rao, K.R.M.; Rao, P.H.; Chaitanya, B.S.K. Piezoelectricity in quasicrystals: A group-theoretical study. J. Phys. 2007, 68, 481–487. [Google Scholar]
- Fujiwara, T.; Laissardière, G.T.d.; Yamamoto, S. Electronic structure and electron transport in quasicrystals. Mater. Sci. Forum 1994, 150, 387–394. [Google Scholar] [CrossRef]
- Li, X.Y.; Li, P.D.; Wu, T.H.; Shi, M.X.; Zhu, Z.W. Three-dimensional fundamental solutions for one-dimensional hexagonal quasicrystal with piezoelectric effect. Phys. Lett. A 2014, 378, 826–834. [Google Scholar] [CrossRef]
- Zhou, Y.B.; Li, X.F. Two collinear mode-III cracks in one-dimensional hexagonal piezoelectric quasicrystal strip. Eng. Fract. Mech. 2018, 189, 133–147. [Google Scholar] [CrossRef]
- Zhang, B.; Yu, J.G.; Zhang, X.M.; Elmaimouni, L. Guided wave propagating in a 1-D hexagonal piezoelectric quasi-crystal plate. Acta Mech. 2021, 232, 135–151. [Google Scholar] [CrossRef]
- Li, L.; Cui, X.; Guo, J. Interaction between a screw dislocation and an elliptical hole with two asymmetrical cracks in a one-dimensional hexagonal quasicrystal with piezoelectric effect. Appl. Math. Mech. 2020, 41, 899–908. [Google Scholar] [CrossRef]
- Li, Y.; Yang, L.Z.; Gao, Y. An exact solution for a functionally graded multilayered one-dimensional orthorhombic quasicrystal plate. Acta Mech. 2019, 230, 1257–1273. [Google Scholar] [CrossRef]
- Hu, K.; Gao, C.; Zhong, Z.; Chen, Z. Interaction of collinear interface cracks between dissimilar one-dimensional hexagonal piezoelectric quasicrystals. Z. Angew. Math. Mech. 2021, 101, e202000360. [Google Scholar] [CrossRef]
- Hu, K.; Meguid, S.A.; Wang, L.; Jin, H. Electro-elastic field of a piezoelectric quasicrystal medium containing two cylindrical inclusions. Acta Mech. 2021, 232, 2513–2533. [Google Scholar] [CrossRef]
- Ding, H.J.; Xu, R.Q.; Chi, Y.W.; Chen, W.Q. Free axisymmetric vibration of transversely isotropic piezoelectric circular plates. Int. J. Solids Struct. 1999, 36, 4629–4652. [Google Scholar]
- Li, Y.; Li, Y.; Qin, Q.; Yang, L.; Gao, Y. Axisymmetric bending analysis of functionally graded one-dimensional hexagonal piezoelectric quasi-crystal circular plate. Proc. R. Soc. A 2020, 476, 20200301. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Xu, R.Q.; Ding, H.J. Three-dimensional solution of axisymmetric bending of functionally graded circular plates. Compos. Struct. 2010, 92, 1683–1693. [Google Scholar]
- Yang, Z.X.; He, X.T.; Xue, L.; Lian, Y.S.; Sun, J.Y. An electroelastic solution for functionally graded piezoelectric circular plates under the action of combined mechanical loads. Materials 2018, 11, 1168. [Google Scholar] [CrossRef] [Green Version]
- Zhao, X.; Li, X.Y.; Li, Y.H. Axisymmetric analytical solutions for a heterogeneous multi-ferroic circular plate subjected to electric loading. Mech. Adv. Mater. Struct. 2018, 25, 795–804. [Google Scholar] [CrossRef]
- Lv, X.; Ke, L.L.; Su, J.; Tian, J.Y. Axisymmetric contact vibration analysis of a rigid spherical punch on a piezoelectric half-space. Int. J. Solids Struct. 2021, 210–211, 224–236. [Google Scholar] [CrossRef]
- Wu, Y.F.; Chen, W.Q.; Li, X.Y. Indentation on one-dimensional hexagonal quasicrystals: General theory and complete exact solutions. Philos. Mag. 2013, 93, 858–882. [Google Scholar] [CrossRef]
- Sneddon, I.N. Fourier Transforms; McGraw-Hil: New York, NY, USA, 1951. [Google Scholar]
- Ding, H.J.; Xu, R.Q.; Guo, F.L. Exact axisymmetric solution of laminated transversely isotropic piezoelectric circular plates. Sci. China 1999, 42, 470–478. [Google Scholar] [CrossRef]

| 1D Hexagonal Piezoelectric QCs | Piezoelectric Materials (PZT4) | |
|---|---|---|
| Phonon elastic (Gpa) | ||
| Phason elastic (Gpa) | ||
| Coupling (Gpa) | ||
| Piezoelectric (C/m2) | ||
| Dielectric (C2·N−1 m−2) | ||
| s | P/Q/P | Q/P/Q | P/P/P |
|---|---|---|---|
| 0.1 | 4249.292 | 6688.337 | 4194.802 |
| 0.2 | 275.8201 | 426.0097 | 272.1027 |
| 0.3 | 57.82975 | 86.74357 | 56.99289 |
| 0.4 | 19.7639 | 28.57065 | 19.45356 |
| 0.5 | 8.855762 | 12.27743 | 8.704454 |
| 0.6 | 4.710162 | 6.246838 | 4.622687 |
| 0.7 | 2.815862 | 3.569921 | 2.759141 |
| 0.8 | 1.829975 | 2.218651 | 1.790067 |
| 0.9 | 1.264742 | 1.467806 | 1.234922 |
| 1.0 | 0.915595 | 1.018318 | 0.892282 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, L.; Zhang, H.; Li, Y.; Wang, J.; Lu, C. Static Electro-Mechanical Response of Axisymmetric One-Dimensional Piezoelectric Quasicrystal Circular Actuator. Materials 2022, 15, 3157. https://doi.org/10.3390/ma15093157
Zhang L, Zhang H, Li Y, Wang J, Lu C. Static Electro-Mechanical Response of Axisymmetric One-Dimensional Piezoelectric Quasicrystal Circular Actuator. Materials. 2022; 15(9):3157. https://doi.org/10.3390/ma15093157
Chicago/Turabian StyleZhang, Linyan, Hongliang Zhang, Yang Li, Jingbo Wang, and Changguo Lu. 2022. "Static Electro-Mechanical Response of Axisymmetric One-Dimensional Piezoelectric Quasicrystal Circular Actuator" Materials 15, no. 9: 3157. https://doi.org/10.3390/ma15093157





