Quantification of the Hardened Cement Paste Content in Fine Recycled Concrete Aggregates by Means of Salicylic Acid Dissolution
Abstract
:1. Introduction
2. Materials and Methods
2.1. Laboratory-Produced RCA
2.2. Industrial RCA
2.3. Experimental Methods
2.3.1. Water Absorption
2.3.2. Density
2.3.3. Hardened Cement Paste Content
3. Results and Discussion
3.1. Measurement of Hardened Cement Paste Content in RCA
3.1.1. Experimental Approach
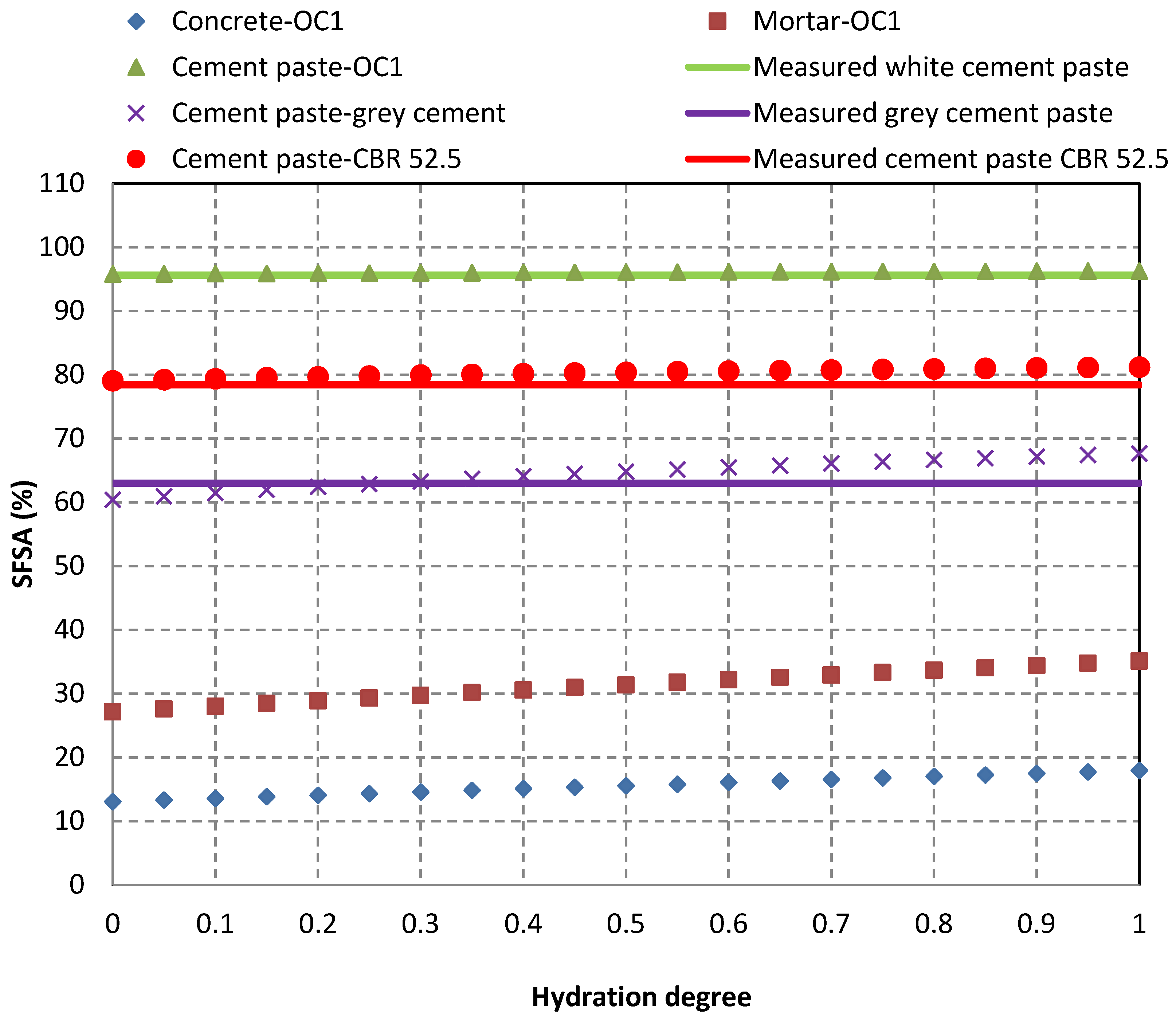
3.1.2. Theoretical Approach to the Estimation of SFSA
3.1.3. Application to Pure Cement Pastes
3.1.4. Hardened Cement Paste Content in RCA
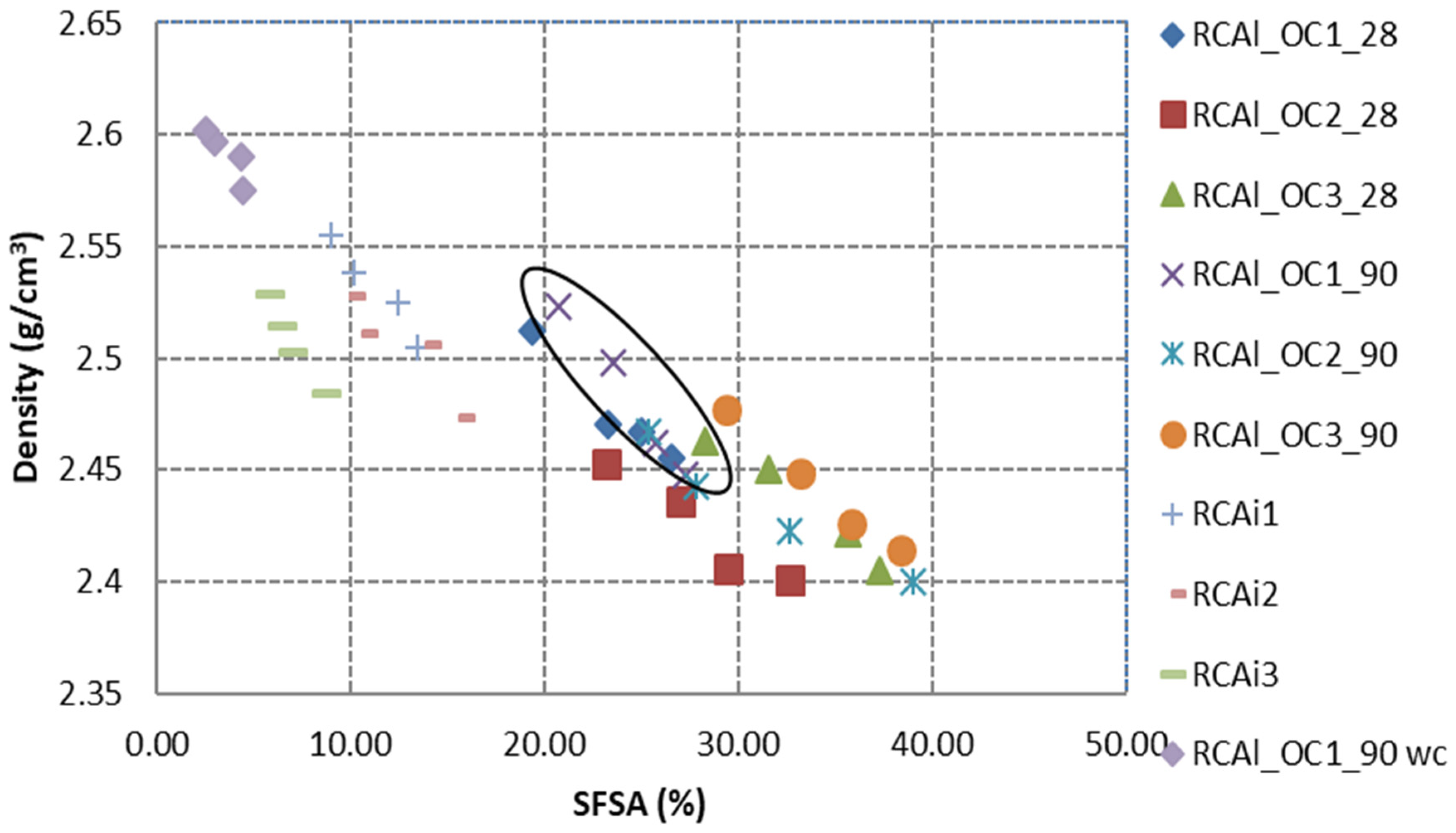
3.2. Relationships between HCPC and the Other Properties
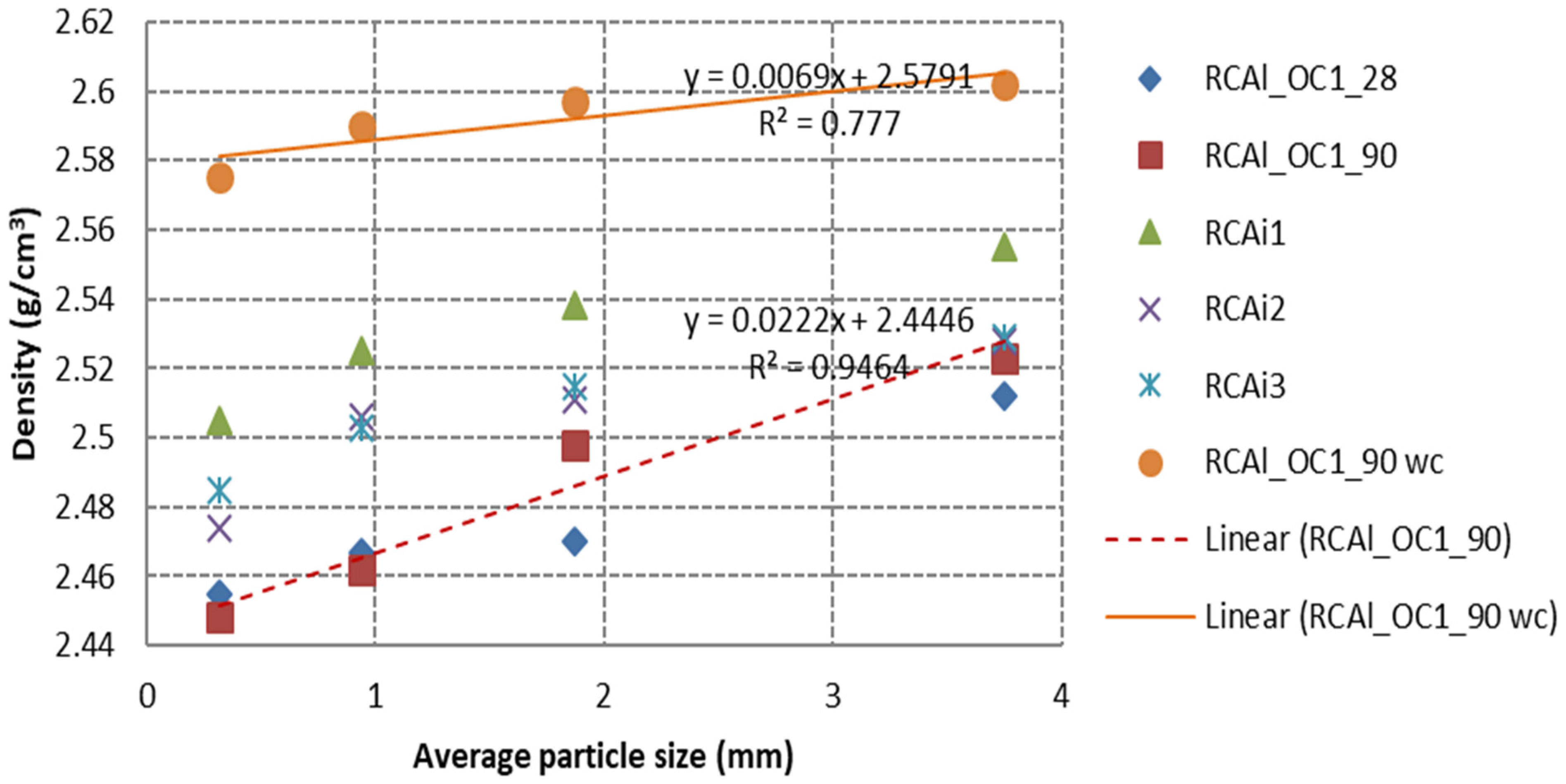
3.2.1. Relationship between HCPC and Density
3.2.2. Relationship between HCPC and Water Absorption
4. Conclusions
- (1)
- The XRD results confirmed that the salicylic acid allowed us to dissolve most of the phases contained in OPC cement paste (Ca(OH)2, C-S-H, ettringite, C2S, C3S) but not the main phases contained in natural aggregates and especially limestone;
- (2)
- The experimental results showed that 95.57% of white cement paste and 62.99% of grey cement paste were dissolved while only 0.83% of siliceous sand and 3.21% of calcareous aggregate were dissolved. For white cement paste, the soluble fraction in salicylic acid (SFSA) was almost identical to the HCPC. For the grey cement paste (higher content of C3A, C4AF and calcite), SFSA corresponded to only 62.99% of HCPC. For cement paste made with CBR CEM 52.5 N after a long time cured in water, SFSA corresponded to 78.42% of HCPC. Most phases of cement paste made with blend cement (CBR CEM III/A 42.5 N: Portland cement combined with slag) can dissolve in salicylic acid, which corresponded to 80.14% of HCPC;
- (3)
- The difference between SFSA and HCPC could be estimated theoretically by modelling the hydration of cement paste with the chemical equations and the corresponding stoichiometric ratios. The experimentally obtained SFSA values were very close to the values calculated for the three pure cement pastes. The model results were consistent with the experimental results;
- (4)
- SFSA in RCA obtained from industrial center increased as the particle size decreased, which showed a similar tendency as laboratory-manufactured RCA. In addition, all experimental values were within the range of the calculated values based on mortar and concrete composition. This indicated that the RCA contained both crushed mortar and part of coarse NA from parent concrete. The SFSA obtained for industrial RCA was significantly lower than those measured for laboratory-manufactured RCA. These results might be attributed to the nature of cement used in parent concrete (e.g., CEM II contains a higher insoluble fraction in salicylic acid such as limestone) and any carbonation of cement paste also reducing the soluble fraction. Therefore, SFSA did not give the exact value of HCPC for industrial RCA, but it was demonstrated that the SFSA was sufficient to correlate HCPC with the other properties of RCA.
- (5)
- The properties of RCA including specific density, water absorption and porosity were strongly correlated to HCPC. The higher the HCPC or SFSA, the higher the water absorption and porosity, and the lower the specific density. The linear relationship can be obtained between the water absorption (porosity, specific density) and the HCPC or SFSA. The water absorption could be estimated with good accuracy for very fine RCA (laboratory-manufactured RCA or industrial RCA) by extrapolating the relationship obtained between water absorption and HCPC or SFSA with coarser granular class, which was important for the formulation of concrete made with RCA, but the value was quite difficult to accurately measure.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Eurostat Generation of Waste by Economic Activity. Statistical Classification of Economic Activities in the European Community (NACE Rev.2): Construction. Available online: https://ec.europa.eu/eurostat/databrowser/view/ten00106/default/bar?lang=en (accessed on 28 January 2022).
- UEPG. European Aggregates Association—Annual Review 2019–2020; UEPG: Brussels, Belgium, 2020. [Google Scholar]
- Xiao, J.; Li, W.; Fan, Y.; Huang, X. An overview of study on recycled aggregate concrete in China (1996–2011). Constr. Build. Mater. 2012, 31, 364–383. [Google Scholar] [CrossRef]
- Zhao, Z.; Courard, L.; Groslambert, S.; Jehin, T.; Léonard, A.; Xiao, J. Use of recycled concrete aggregates from precast block for the production of new building blocks: An industrial scale study. Resour. Conserv. Recycl. 2020, 157, 104786. [Google Scholar] [CrossRef]
- Xiao, J.; Xie, H.; Zhang, C. Investigation on building waste and reclaim in Wenchuan earthquake disaster area. Resour. Conserv. Recycl. 2012, 61, 109–117. [Google Scholar] [CrossRef]
- Batayneh, M.; Marie, I.; Asi, I. Use of selected waste materials in concrete mixes. Waste Manag. 2007, 27, 1870–1876. [Google Scholar] [CrossRef]
- Zaharieva, R.; Buyle-Bodin, F.; Wirquin, E. Frost resistance of recycled aggregate concrete. Cem. Concr. Res. 2004, 34, 1927–1932. [Google Scholar] [CrossRef]
- Etxeberria, M.; Vázquez, E.; Marí, A.; Barra, M. Influence of amount of recycled coarse aggregates and production process on properties of recycled aggregate concrete. Cem. Concr. Res. 2007, 37, 735–742. [Google Scholar] [CrossRef]
- Katz, A. Properties of concrete made with recycled aggregate from partially hydrated old concrete. Cem. Concr. Res. 2003, 33, 703–711. [Google Scholar] [CrossRef]
- Pedro, D.; de Brito, J.; Evangelista, L. Structural concrete with simultaneous incorporation of fine and coarse recycled concrete aggregates: Mechanical, durability and long-term properties. Constr. Build. Mater. 2017, 154, 294–309. [Google Scholar] [CrossRef]
- Manzi, S.; Mazzotti, C.; Bignozzi, M.C. Short and long-term behavior of structural concrete with recycled concrete aggregate. Cem. Concr. Compos. 2013, 37, 312–318. [Google Scholar] [CrossRef]
- Zhao, Z.; Remond, S.; Damidot, D.; Courard, L.; Michel, F. Improving the properties of recycled concrete aggregates by accelerated carbonation. Proc. Inst. Civ. Eng. Constr. Mater. 2018, 171, 126–132. [Google Scholar] [CrossRef] [Green Version]
- Zhao, Z.; Courard, L.; Michel, F.; Remond, S.; Damidot, D. Influence of granular fraction and origin of recycled concrete aggregates on their properties. Eur. J. Environ. Civ. Eng. 2018, 22, 1457–1467. [Google Scholar] [CrossRef] [Green Version]
- Kou, S.C.; Poon, C.S. Properties of concrete prepared with crushed fine stone, furnace bottom ash and fine recycled aggregate as fine aggregates. Constr. Build. Mater. 2009, 23, 2877–2886. [Google Scholar] [CrossRef]
- Padmini, A.K.; Ramamurthy, K.; Mathews, M.S. Influence of parent concrete on the properties of recycled aggregate concrete. Constr. Build. Mater. 2009, 23, 829–836. [Google Scholar] [CrossRef]
- Hansen, T.C. Recycled aggregates and recycled aggregate concrete second state-of-the-art report developments 1945–1985. Mater. Struct. 1986, 19, 201–246. [Google Scholar] [CrossRef]
- Kou, S.C.; Poon, C.S. Enhancing the durability properties of concrete prepared with coarse recycled aggregate. Constr. Build. Mater. 2012, 35, 69–76. [Google Scholar] [CrossRef]
- Thomas, C.; Setién, J.; Polanco, J.A.; Alaejos, P.; De Juan, M.S. Durability of recycled aggregate concrete. Constr. Build. Mater. 2013, 40, 1054–1065. [Google Scholar] [CrossRef]
- Sagoe-Crentsil, K.K.; Brown, T.; Taylor, A.H. Performance of concrete made with commercially produced coarse recycled concrete aggregate. Cem. Concr. Res. 2001, 31, 707–712. [Google Scholar] [CrossRef]
- Kwan, W.H.; Ramli, M.; Kam, K.J.; Sulieman, M.Z. Influence of the amount of recycled coarse aggregate in concrete design and durability properties. Constr. Build. Mater. 2012, 26, 565–573. [Google Scholar] [CrossRef]
- Olorunsogo, F.T.; Padayachee, N. Performance of recycled aggregate concrete monitored by durability indexes. Cem. Concr. Res. 2002, 32, 179–185. [Google Scholar] [CrossRef]
- Wagih, A.M.; El-Karmoty, H.Z.; Ebid, M.; Okba, S.H. Recycled construction and demolition concrete waste as aggregate for structural concrete. HBRC J. 2013, 9, 193–200. [Google Scholar] [CrossRef] [Green Version]
- Khatib, J.M. Properties of concrete incorporating fine recycled aggregate. Cem. Concr. Res. 2005, 35, 763–769. [Google Scholar] [CrossRef]
- Evangelista, L.; de Brito, J. Durability performance of concrete made with fine recycled concrete aggregates. Cem. Concr. Compos. 2010, 32, 9–14. [Google Scholar] [CrossRef]
- de Juan, M.S.; Gutiérrez, P.A. Study on the influence of attached mortar content on the properties of recycled concrete aggregate. Constr. Build. Mater. 2009, 23, 872–877. [Google Scholar] [CrossRef]
- Abbas, A.; Fathifazl, G.; Fournier, B.; Isgor, O.B.; Zavadil, R.; Razaqpur, A.G.; Foo, S. Quantification of the residual mortar content in recycled concrete aggregates by image analysis. Mater. Charact. 2009, 60, 716–728. [Google Scholar] [CrossRef]
- Topçu, I.B.; Şengel, S. Properties of concretes produced with waste concrete aggregate. Cem. Concr. Res. 2004, 34, 1307–1312. [Google Scholar] [CrossRef]
- Hansen, T.C.; Narud, H. Strength of recycled concrete made from crushed concrete coarse aggregate. Concr. Int. 1983, 5, 79–83. [Google Scholar]
- Ulsen, C.; Contessotto, R.; dos Santos Macedo, R.; Kahn, H. Quantification of the cement paste and phase’s association in fine recycled aggregates by SEM-based image analysis. Constr. Build. Mater. 2022, 320, 126206. [Google Scholar] [CrossRef]
- dos Santos Macedo, R.; Ulsen, C.; Mueller, A. Quantification of residual cement paste on recycled concrete aggregates containing limestone by selective dissolution. Constr. Build. Mater. 2019, 229, 116875. [Google Scholar] [CrossRef]
- Yagishita, F.; Sano, M.; Yamada, M. Behavior of Reinforced Concrete Beams Containing Recycled Coarse Aggregate. Demolition and Reuse of Concrete and Masonry; CRC Press: London, UK, 1994. [Google Scholar]
- Nagataki, S.; Gokce, A.; Saeki, T.; Hisada, M. Assessment of recycling process induced damage sensitivity of recycled concrete aggregates. Cem. Concr. Res. 2004, 34, 965–971. [Google Scholar] [CrossRef]
- Ulsen, C.; Tseng, E.; Angulo, S.C.; Landmann, M.; Contessotto, R.; Balbo, J.T.; Kahn, H. Concrete aggregates properties crushed by jaw and impact secondary crushing. J. Mater. Res. Technol. 2019, 8, 494–502. [Google Scholar] [CrossRef]
- Lu, B.; Shi, C.; Zhang, J.; Wang, J. Effects of carbonated hardened cement paste powder on hydration and microstructure of Portland cement. Constr. Build. Mater. 2018, 186, 699–708. [Google Scholar] [CrossRef]
- van de Wouw, P.M.F.; Florea, M.V.A.; Brouwers, H.J.H.J. Methods for determining and tracking the residual cement paste content of recycled concrete. In Proceedings of the 2nd International Conference of Sustainable Building Materials (ICSBM 2019), Eindhoven, The Netherlands, 12–15 August 2019; pp. 226–233. [Google Scholar]
- Poon, C.S.; Shui, Z.H.; Lam, L.; Fok, H.; Kou, S.C. Influence of moisture states of natural and recycled aggregates on the slump and compressive strength of concrete. Cem. Concr. Res. 2004, 34, 31–36. [Google Scholar] [CrossRef]
- Théréné, F.; Keita, E.; Nael-Redolfi, J.; Boustingorry, P.; Bonafous, L.; Roussel, N. Water absorption of recycled aggregates: Measurements, influence of temperature and practical consequences. Cem. Concr. Res. 2020, 137, 106196. [Google Scholar] [CrossRef]
- Bouarroudj, M.E.; Rémond, S.; Bulteel, D.; Potier, G.; Michel, F.; Zhao, Z.; Courard, L. Use of grinded hardened cement pastes as mineral addition for mortars. J. Build. Eng. 2021, 34, 101863. [Google Scholar] [CrossRef]
- Taboada, G.L.; Seruca, I.; Sousa, C.; Pereira, Á. Exploratory data analysis and data envelopment analysis of construction and demolitionwaste management in the European economic area. Sustainability 2020, 12, 4995. [Google Scholar] [CrossRef]
- Zhang, C.; Hu, M.; Di Maio, F.; Sprecher, B.; Yang, X.; Tukker, A. An overview of the waste hierarchy framework for analyzing the circularity in construction and demolition waste management in Europe. Sci. Total Environ. 2022, 803, 149892. [Google Scholar] [CrossRef]
- Fang, X.; Xuan, D.; Zhan, B.; Li, W.; Poon, C.S. A novel upcycling technique of recycled cement paste powder by a two-step carbonation process. J. Clean. Prod. 2021, 290, 125192. [Google Scholar] [CrossRef]
- Zhao, Z.; Remond, S.; Damidot, D.; Xu, W. Influence of fine recycled concrete aggregates on the properties of mortars. Constr. Build. Mater. 2015, 81, 179–186. [Google Scholar] [CrossRef]
- CEN EN 1097; Tests for Mechanical and Physical Properties of Aggregates-Part 6: Determination of Particle Density and Water Absorption. British Standard Institution: London, UK, 2014.
- ASTM C 128; Standard Test Method for Density, Relative Density (Specific Gravity), and Absorption of Fine Aggregate. ASTM International: West Conshohocken, PA, USA, 2004.
- IFSTTAR Test N° 78; Tests on Granulates in Concrete: Measurement of Total Water Absorption of Crushed Sand. Institut Français des Sciences et Technologies des Transports, de L’améNagement et des Réseaux: Paris, France, 2011.
- Djerbi Tegguer, A. Determining the water absorption of recycled aggregates utilizing hydrostatic weighing approach. Constr. Build. Mater. 2012, 27, 112–116. [Google Scholar] [CrossRef]
- Tam, V.W.Y.; Gao, X.F.; Tam, C.M.; Chan, C.H. New approach in measuring water absorption of recycled aggregates. Constr. Build. Mater. 2008, 22, 364–369. [Google Scholar] [CrossRef] [Green Version]
- CEN EN 197-1; Cement–Part 1: Composition, Specifications and Conformity Criteria for Common Cements. CEN: Brussels, Belgium, 2011.
- Zhao, Z.; Remond, S.; Damidot, D.; Xu, W. Influence of hardened cement paste content on the water absorption of fine recycled concrete aggregates. J. Sustain. Cem. Mater. 2013, 2, 186–203. [Google Scholar] [CrossRef]
- de Larrard, F.; Colina, H. The French National Project Recybéton: To Bring the Concrete World into Circular Economy. Available online: https://www.pnrecybeton.fr/en/ (accessed on 9 April 2019).
- Luke, K.; Glasser, F. Selective dissolution of hydrated blast furnace slag cements. Cem. Concr. Res. 1987, 17, 273–282. [Google Scholar] [CrossRef]
- Gutteridge, W.A. On the dissolution of the interstitial phases in Portland cement. Cem. Concr. Res. 1979, 9, 319–324. [Google Scholar] [CrossRef]
- Ohsawa, S.; Asaga, K.; Goto, S.; Daimon, M. Quantitative determination of fly ash in the hydrated fly ash—CaSO4·2H2O-Ca(OH)2 system. Cem. Concr. Res. 1985, 15, 357–366. [Google Scholar] [CrossRef]
- Zhao, Z. Re-Use of Fine Recycled Concrete Aggregates for the Manufacture of Mortars. Doctoral Dissertation, University of Lille, Lille, France, 2014. [Google Scholar]
- CEN EN 196; Methods of testing cement–Part 1: Determination of strength. European Committee for Standardization. CEN: Brussels, Belgium, 2005.
- Zhao, Z.; Remond, S.; Damidot, D.; Courard, L. Toward the quantification of the cement paste content of fine recycled concrete aggregates by salicylic acid dissolution corrected by a theoretical approach. In Proceedings of the 14th International Congress on the Chemistry of Cement, Beijing, China, 13 October 2015. [Google Scholar]
- Thiery, M.; Villain, G.; Dangla, P.; Platret, G. Investigation of the carbonation front shape on cementitious materials: Effects of the chemical kinetics. Cem. Concr. Res. 2007, 37, 1047–1058. [Google Scholar] [CrossRef]
- Shi, Z.; Lothenbach, B.; Geiker, M.R.; Kaufmann, J.; Leemann, A.; Ferreiro, S.; Skibsted, J. Experimental studies and thermodynamic modeling of the carbonation of Portland cement, metakaolin and limestone mortars. Cem. Concr. Res. 2016, 88, 60–72. [Google Scholar] [CrossRef]
- Grabiec, A.M.; Klama, J.; Zawal, D.; Krupa, D. Modification of recycled concrete aggregate by calcium carbonate biodeposition. Constr. Build. Mater. 2012, 34, 145–150. [Google Scholar] [CrossRef]
- Ngala, V.T.; Page, C.L. Effects of carbonation on pore structure and diffusional properties of hydrated cement pastes. Cem. Concr. Res. 1997, 27, 995–1007. [Google Scholar] [CrossRef]
- Lawrence, R.M.; Mays, T.J.; Rigby, S.P.; Walker, P.; D’Ayala, D. Effects of carbonation on the pore structure of non-hydraulic lime mortars. Cem. Concr. Res. 2007, 37, 1059–1069. [Google Scholar] [CrossRef]




| Author (Reference No.) | Test Methods | Fractions of RCA (mm) | Adherent Mortar or Hardened Cement Paste Content (%) | Advantages/Disadvantages |
|---|---|---|---|---|
| Etxeberria et al. [8] | Not mentioned | CRCA 4/10, 10/25 | 40% for fraction 4/10; 20% for fraction 10/25 | - |
| De Juan et al. [25] | Thermal method | 15 samples of CRCA 4/8, 8/16 | 33–55% for fraction 4/8; 23–44% for fraction 8/16 | This method is only suitable for CRCA because the removal of mortar necessitates “brushing” the RCA, which is difficult with small particles. |
| Nagataki et al. [32] | Hydrochloric acid solution method | CRCA 5/20 | 52.3–55% for level 1; 30.2–32.4% for level 3 | This method cannot be used for RCA containing limestone aggregates and filler, which are also dissolved by hydrochloric acid. |
| Yagishita et al. [31] | Hydrochloric acid solution method | CRCA 5/10, 10/20 | 40.2% for low-grade fraction 10/20, 35.2 for low-grade fraction 5/10; 26% for medium-grade fraction 10/20, 16.7 for low-grade fraction 5/10 | |
| Abbas et al. [26] | Image analysis method and sodium sulphate solution method | Two CRCA 4.75/9.5, 9.5/12.7,12.7/19 | Image analysis: 30%, 21%, 21% for 4.75/9.5, 9.5/12.7, 12.7/19, respectively; Sodium sulphate solution method: 26%,22%,21% for 4.75/9.5, 9.5/12.7, 12.7/19, respectively | The image analysis method is suitable for the quantification of residual mortar in CRCA. Moreover, this method is long to perform as a statistical approach is needed. The sodium sulphate solution method is only suitable for CRCA. |
| Hansen and Narud [28] | Linear traverse method | CRCA 4/8, 8/16, 16/32 | 58–64% for fraction 4/8; 38–39% for fraction 8/16; 25–35% for fraction 16/32 | This linear traverse method is only suitable for adherent mortar content of CRCA. |
| Topçu et al. [27] | Linear traverse method | CRCA 4/8, 16/32 | 60% for fraction 4/8; 30% for fraction 16/32 | |
| Ulsen et al. [29] | X-ray SEM-based image analysis | FRCA 0.15/3.0 | HCPC: 10% for the fraction 0.15/3.0; 15% for the fraction 0.15/0.3 | The correlations between the sum of CaO and LOI and cement paste + carbonates, and the comparison to the cement plus carbonate content by HCl leaching stated the reliability SEM-based image analysis. |
| Macedo et al. [30] | The dissolution method in deionized water (10 days) | Simulated 1%, 5%, 10% and 20% hardened cement paste | The deionized method presents good efficiency in the removal of the calcium ions from the hydrated cement phases, C-S-H and CH in the simulated samples | There is the carbonation contamination and dissolution in deionized water takes ten days, which is too long. In addition, the ICP analysis is expensive. |
| C3S | C2S | C3A | C4AF | Anhydrite | Calcite | Periclase | Gypsum | Quartz | Slag | |
|---|---|---|---|---|---|---|---|---|---|---|
| White cement CEM I 52.5 N | 73.90 | 21.87 | 1.46 | - | 0.52 | 1.53 | 0.72 | - | - | - |
| Grey cement CEM II/A-L 52.5 N | 52.37 | 8.01 | 8.86 | 8.89 | 0.74 | 17.93 | 0.46 | 2.05 | 0.7 | - |
| CBR CEM III/A 42.5 N | 35.1 | 7.91 | 3.29 | 5.22 | 0.16 | 0.03 | - | 0.86 | - | 44.01 |
| CBR CEM I 52.5 N | 66.97 | 12.08 | 7.19 | 9.47 | 0.02 | 1.03 | - | 1.76 | - | - |
| Sample | Insoluble Phases |
|---|---|
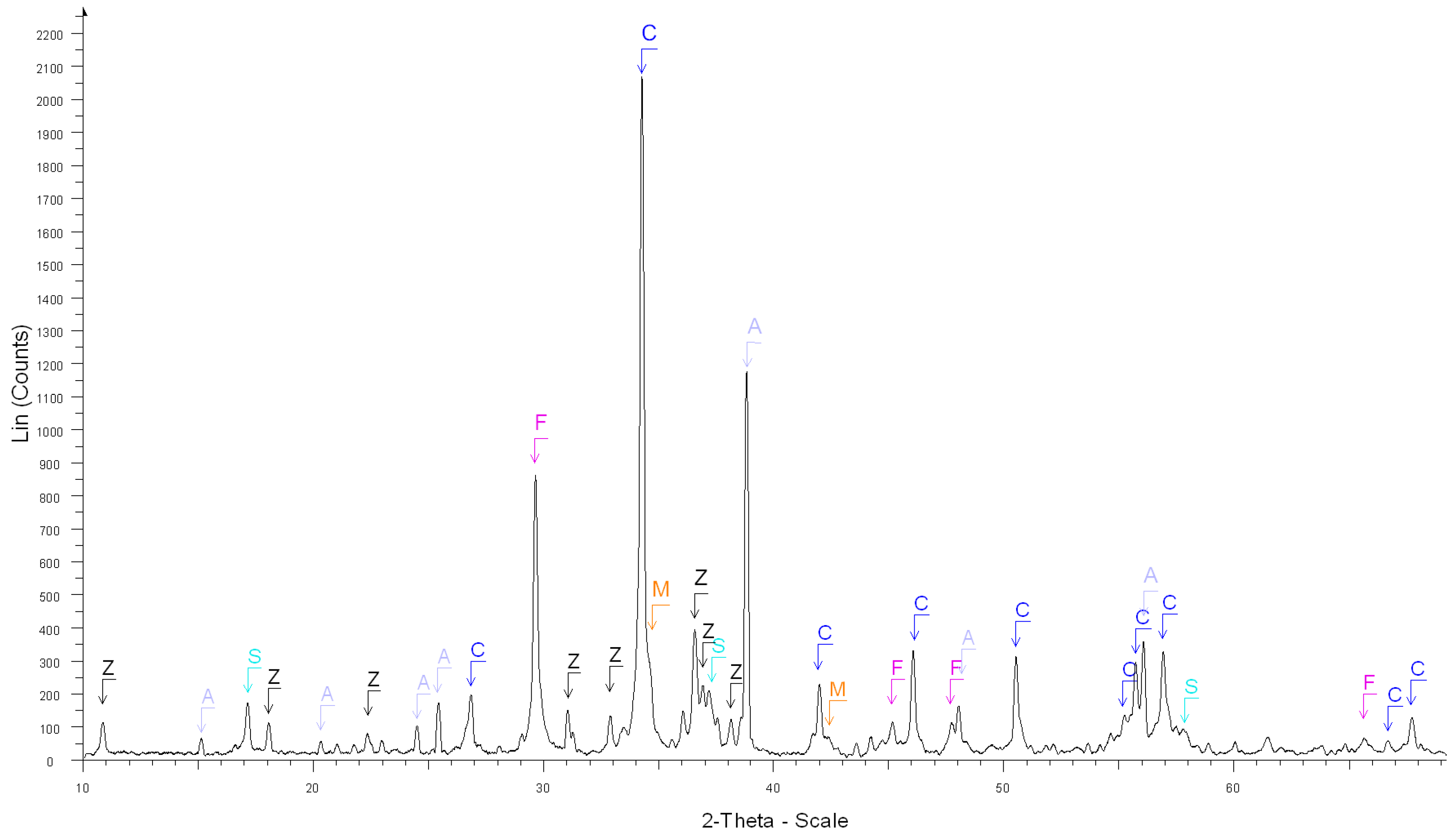
| 1. After the dissolution of white cement (Figure 2) | Calcite Ca(CO3) ****, |
| Calcium Sulfate Ca(SO4) **, | |
| Syngenite K2Ca(SO4)2.H2O *, | |
| Calcium Sulfate Hydrate Ca(SO4)(H2O)0.5 *, | |
| Calcium Aluminum Oxide Ca3Al2O6 ***, | |
| Calcite, magnesian Ca,Mg(CO3) ** | |
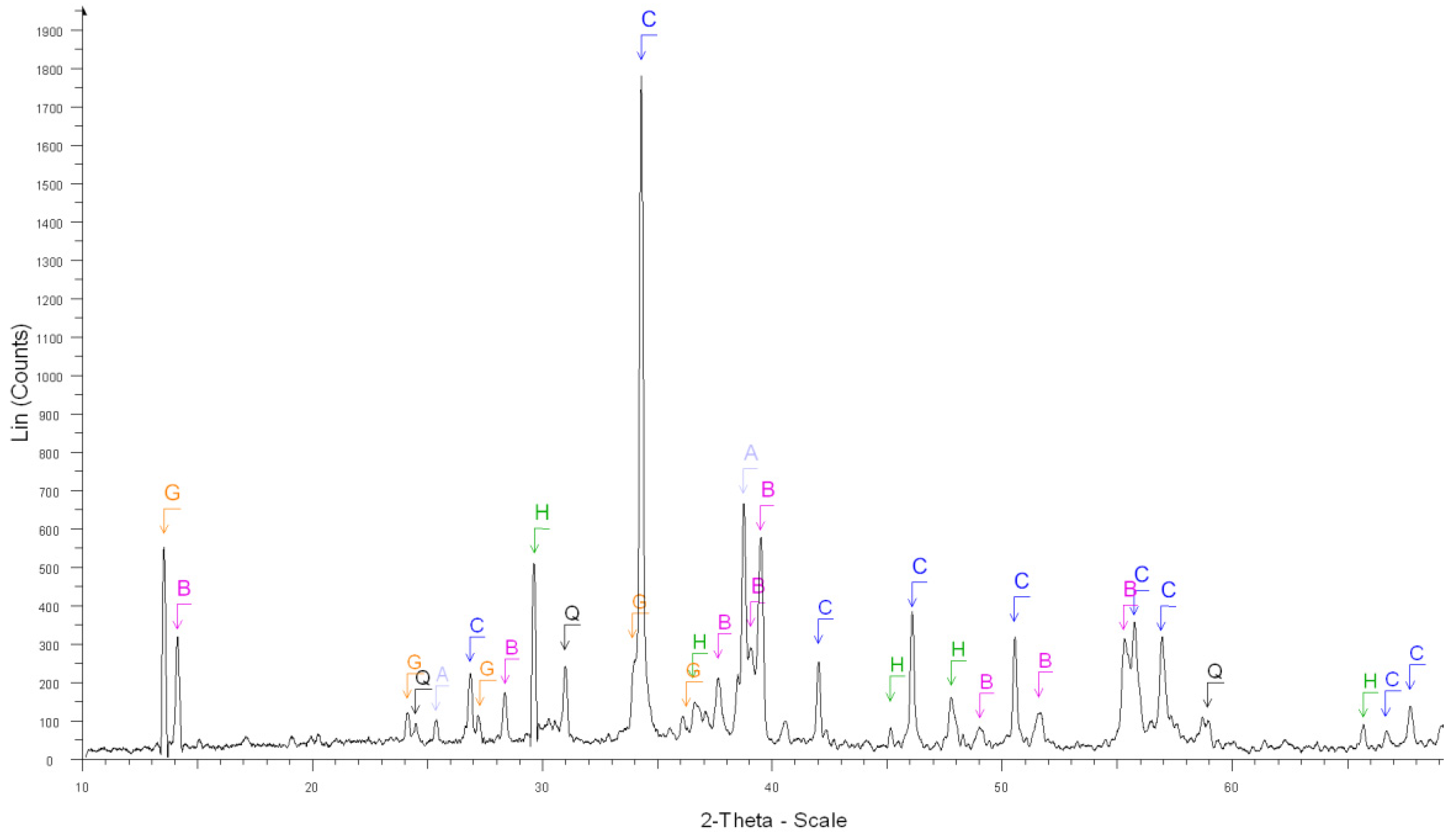
| 2. After the dissolution of grey cement (Figure 3) | Calcite Ca(CO3) ****, |
| Brownmillerite Ca2(Al, Fe + 3)2O5 **, | |
| Anhydrite Ca(SO4) **, | |
| Gypsum Ca(SO4).2H2O *, Quartz SiO2 *, | |
| Calcium Aluminum Oxide Ca3Al2O6 *** | |
| Calcite, magnesian Ca,Mg(CO3) * | |
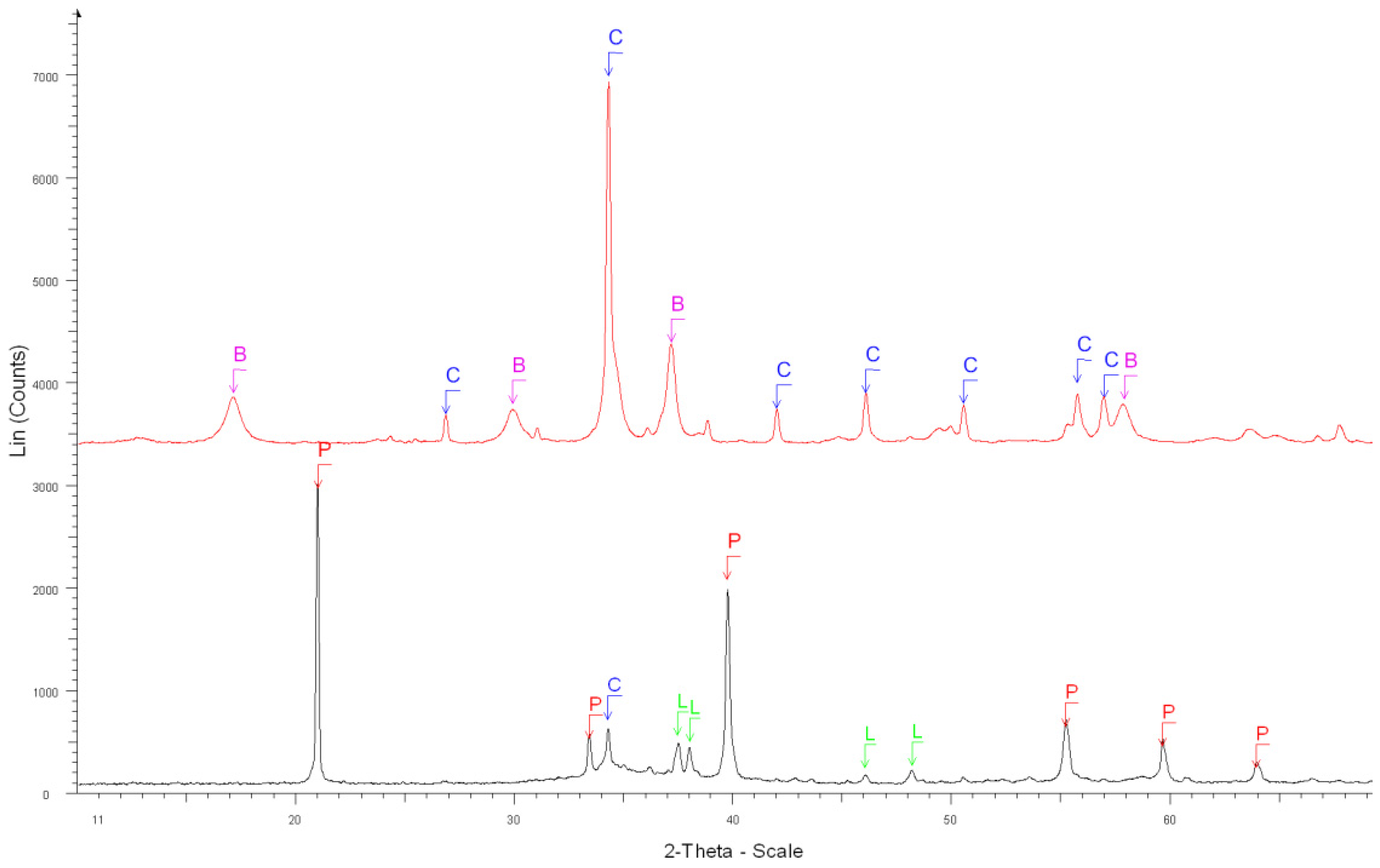
| 3. Before the dissolution of white cement paste (Figure 4) | Portlandite Ca(OH)2 ****, β-dicalcium Silicate Ca2SiO4 **, |
| Calcite Ca(CO3) *, Tricalcium Silicate Ca3SiO5 *, | |
| Calcium Silicate Hydrate Ca1.5SiO3.5 ×H2O * | |
| 4. After the dissolution of white cement paste (Figure 4) | Calcite Ca(CO3) ****, |
| Calcium Sulfate Hydrate Ca(SO4).0.5H2O ***, | |
| Bassanite Ca(SO4).0.5H2O *** | |
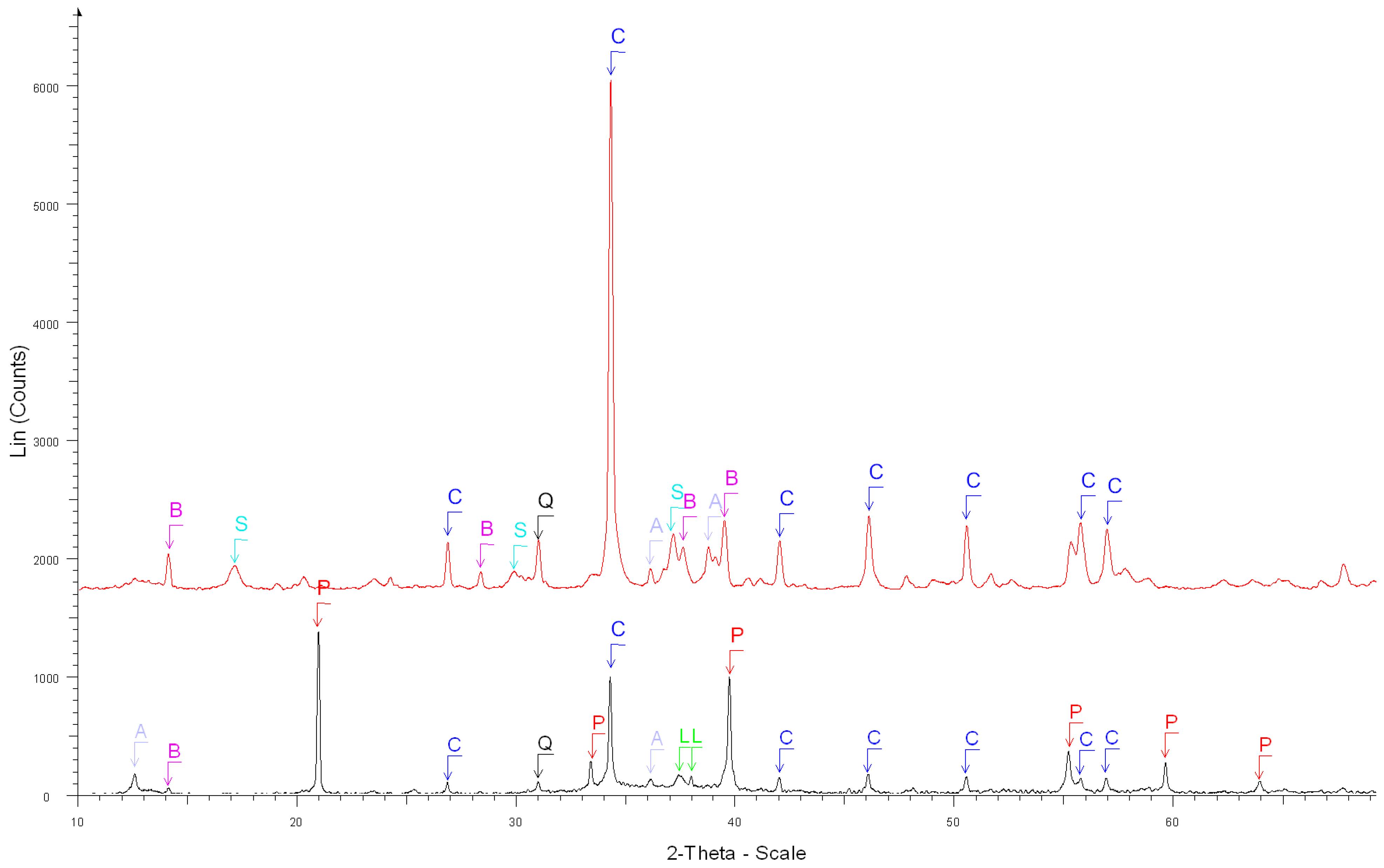
| 5. Before the dissolution of grey cement paste (Figure 5) | Portlandite Ca(OH)2 ****, Calcite Ca(CO3) ***, Quartz SiO2 *, |
| β-dicalcium Silicate Ca2SiO4 **, | |
| Calcium Aluminum Oxide Carbonate Hydroxide Hydrate (AFm hemi carbonate) Ca4Al2O6(CO3)0.5(OH).11.5H2O **, | |
| Brownmillerite Ca2(Al, Fe +3)2O5 * | |
| 6. After the dissolution of grey cement paste(Figure 5) | Calcite Ca(CO3) ****, Quartz SiO2 * |
| Calcium Aluminum Oxide Ca3Al2O6 *, | |
| Brownmillerite Ca2(Al, Fe + 3)2O5 **, | |
| Calcium Sulfate Hydrate Ca(SO4).0.5H2O *, | |
| Calcium Aluminum Iron Oxide Ca3(Al, Fe)2O6 * | |
| 7. Before the dissolution of RCAl_OC1_90 0.63/1.25 mm (Figure 6) | Calcite Ca(CO3) ****, Quartz SiO2 **, |
| Portlandite Ca(OH)2 ** | |
| 8. After the dissolution of RCAl_OC1_90 0.63/1.25 mm (Figure 6) | Calcite Ca(CO3) ****, Quartz SiO2 **, |
| Dolomite CaMg(CO3)2 * |
| Test 1 | Test 2 | Test 3 | Mean Value | Standard Deviation Value | |
|---|---|---|---|---|---|
| White cement paste (CEM I 52.5 N) | 95.46 | 96.35 | 94.89 | 95.57 | 0.74 |
| Grey cement paste (CEM II/A-L 52.5 N) | 62.56 | 63.08 | 63.33 | 62.99 | 0.39 |
| Cement paste (CBR CEM III/A 42.5 N) | 79.87 | 80.09 | 80.48 | 80.14 | 0.31 |
| Cement paste (CBR CEM I 52.5 N) | 78.29 | 78.26 | 78.70 | 78.42 | 0.24 |
| Siliceous sand | 0.76 | 0.86 | 0.88 | 0.83 | 0.06 |
| Calcareous aggregate | 3.42 | 3.03 | 3.18 | 3.21 | 0.20 |
| Chemical Equations | Stoichiometric Ratios |
|---|---|
| x | y | Csol | (E/C)sol | (E/C)moy | |
|---|---|---|---|---|---|
| White cement (CEM I 52.5 N) | 0.9775 | 0.9797 | 0.9614 | 0.4370 | 0.4527 |
| Grey cement (CEM II/A-L 52.5 N) | 0.8092 | 0.7462 | 0.6482 | 0.3940 | 0.5408 |
| CBR CEM I 52.5 N | 0.9749 | 0.8109 | 0.8322 | 0.4645 | 0.5968 |
| Fractions (mm) | RCAl_OC1_28 | RCAl_OC1_90 | RCAl_OC2_28 | RCAl_OC2_90 | RCAl_OC3_28 | RCAl_OC3_90 |
|---|---|---|---|---|---|---|
| 0/0.63 | 26.54 | 27.28 | 32.66 | 39.01 | 37.31 | 38.36 |
| 0.63/1.25 | 24.98 | 25.72 | 29.51 | 32.63 | 35.68 | 35.86 |
| 1.25/2.5 | 23.25 | 23.60 | 27.06 | 27.79 | 31.53 | 33.22 |
| 2.5/5 | 19.35 | 20.76 | 23.16 | 25.35 | 28.29 | 29.34 |
| 0/5 | 22.58 | 23.35 | 26.55 | 29.27 | 31.59 | 32.63 |
| Calculated value on concrete | 16.53 | 17.48 | 21.36 | 22.50 | 25.90 | 27.20 |
| Calculated value on mortar | 32.90 | 34.38 | 40.10 | 41.70 | 46.16 | 47.80 |
| a | b | R2 | Density of NA (x = 0) | Density of Hardened Cement Paste (x = 100%) | |
|---|---|---|---|---|---|
| RCAi1 | −0.010 | 2.64 | 0.95 | 2.64 | 1.64 |
| RCAi2 | −0.008 | 2.60 | 0.82 | 2.60 | 1.84 |
| RCAi3 | −0.014 | 2.61 | 0.96 | 2.61 | 1.17 |
| RCAl_OC1_90 | −0.011 | 2.63 | 0.78 | 2.63 | 1.55 |
| RCAl_OC1_90 wc | −0.012 | 2.77 | 0.98 | 2.77 | 1.57 |
| WAEN | WAIF | |||||||
|---|---|---|---|---|---|---|---|---|
| 0/0.63 | 0.63/1.25 | 1.25/2.5 | 2.5/5 | 0/0.63 | 0.63/1.25 | 1.25/2.5 | 2.5/5 | |
| RCAl_OC1_28 | 7.61 | 10.46 | 8.22 | 7.76 | 21.90 | 11.15 | 9.36 | 7.83 |
| RCAl_OC2_28 | 8.05 | 12.78 | 10.90 | 9.33 | 23.18 | 13.59 | 11.52 | 9.45 |
| RCAl_OC3_28 | 9.74 | 10.07 | 8.10 | 7.68 | 21.44 | 10.89 | 8.84 | 7.71 |
| RCAl_OC1_90 | 9.42 | 9.37 | 7.79 | 7.12 | 17.66 | 10.36 | 8.76 | 8.39 |
| RCAl_OC2_90 | 9.77 | 11.02 | 8.67 | 7.99 | 22.84 | 11.94 | 9.50 | 8.66 |
| RCAl_OC3_90 | 6.52 | 8.75 | 7.31 | 6.67 | 16.79 | 10.08 | 8.76 | 7.58 |
| RCAl_OC1_90 wc | 6.30 | 6.27 | 5.57 | 5.25 | 13.10 | 7.14 | 6.05 | 5.64 |
| RCAi1 | 6.14 | 8.01 | 7.03 | 6.43 | 16.76 | 8.59 | 7.23 | 6.61 |
| RCAi2 | 8.62 | 9.17 | 7.65 | 6.70 | 21.33 | 10.10 | 7.92 | 7.05 |
| RCAi3 | 6.32 | 8.09 | 6.99 | 6.66 | 15.85 | 8.91 | 7.24 | 6.92 |
| Tested Value of IFSTTAR (%) | Tested Value of EN 1097-6 (%) | Extrapolated Value of IFSTTAR (%) | Extrapolated Value of EN 1097-6 (%) | Difference between Two Extrapolated Values (%) | |
|---|---|---|---|---|---|
| RCAi1 | 16.76 | 6.14 | 9.16 | 8.48 | 0.68 |
| RCAi2 | 21.33 | 8.62 | 11.44 | 10.23 | 1.21 |
| RCAi3 | 15.85 | 6.32 | 11.44 | 9.94 | 1.05 |
| RCAl_OC1_90 | 17.66 | 9.42 | 10.67 | 9.82 | 0.85 |
| RCAl_OC1_90 wc | 13.1 | - | 7.26 | 6.35 | 0.91 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, Z.; Xiao, J.; Damidot, D.; Rémond, S.; Bulteel, D.; Courard, L. Quantification of the Hardened Cement Paste Content in Fine Recycled Concrete Aggregates by Means of Salicylic Acid Dissolution. Materials 2022, 15, 3384. https://doi.org/10.3390/ma15093384
Zhao Z, Xiao J, Damidot D, Rémond S, Bulteel D, Courard L. Quantification of the Hardened Cement Paste Content in Fine Recycled Concrete Aggregates by Means of Salicylic Acid Dissolution. Materials. 2022; 15(9):3384. https://doi.org/10.3390/ma15093384
Chicago/Turabian StyleZhao, Zengfeng, Jianzhuang Xiao, Denis Damidot, Sébastien Rémond, David Bulteel, and Luc Courard. 2022. "Quantification of the Hardened Cement Paste Content in Fine Recycled Concrete Aggregates by Means of Salicylic Acid Dissolution" Materials 15, no. 9: 3384. https://doi.org/10.3390/ma15093384
APA StyleZhao, Z., Xiao, J., Damidot, D., Rémond, S., Bulteel, D., & Courard, L. (2022). Quantification of the Hardened Cement Paste Content in Fine Recycled Concrete Aggregates by Means of Salicylic Acid Dissolution. Materials, 15(9), 3384. https://doi.org/10.3390/ma15093384








